Complex Permittivity Measurement of Low-Loss Anisotropic Dielectric Materials at Hundreds of Megahertz
Abstract
:1. Introduction
2. Theory
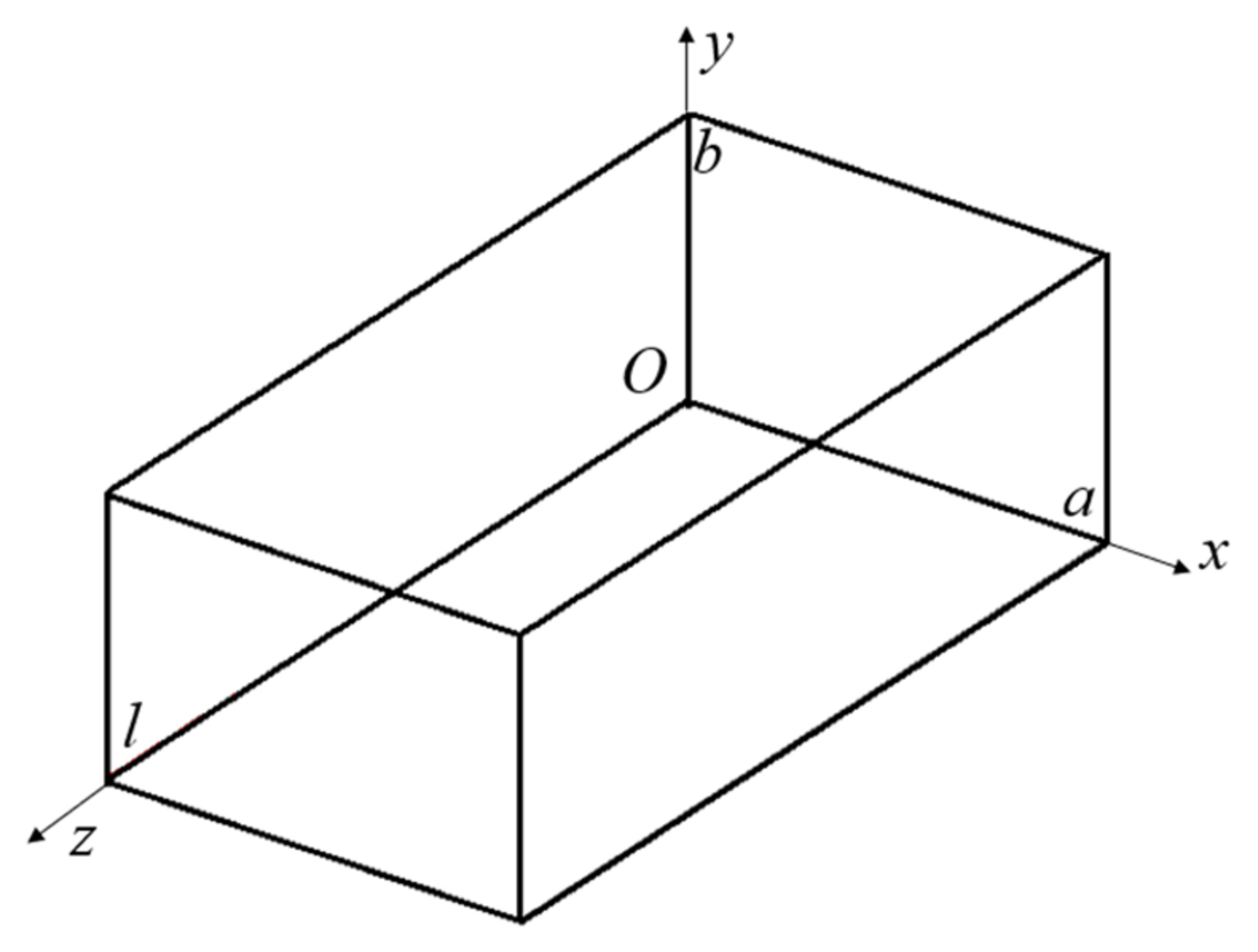
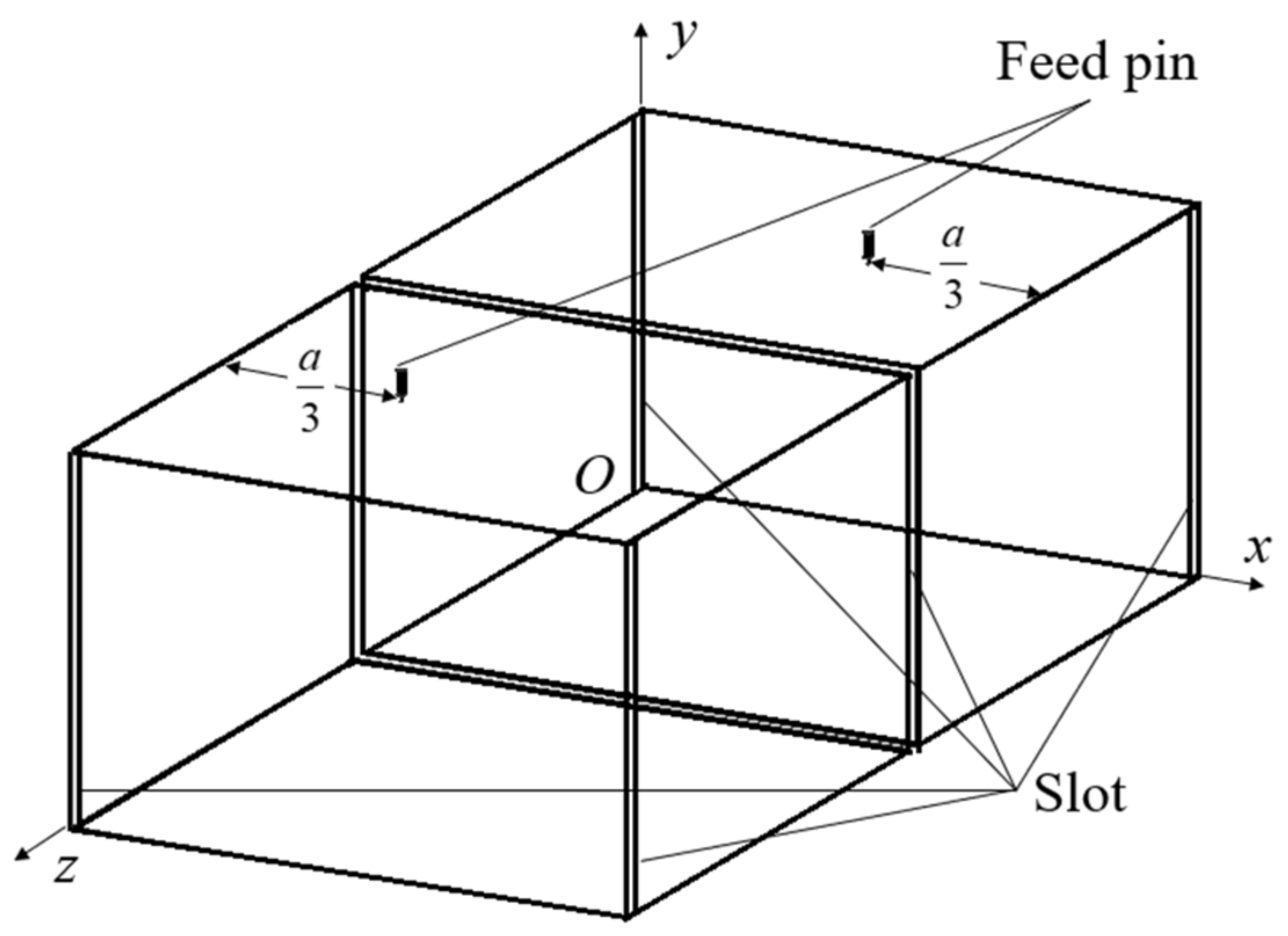
2.1. Rectangular Cavity Design
2.2. Complex Permittivity Determination by Perturbation Method
2.3. Rigorous Iterative Complex Permittivity Determination
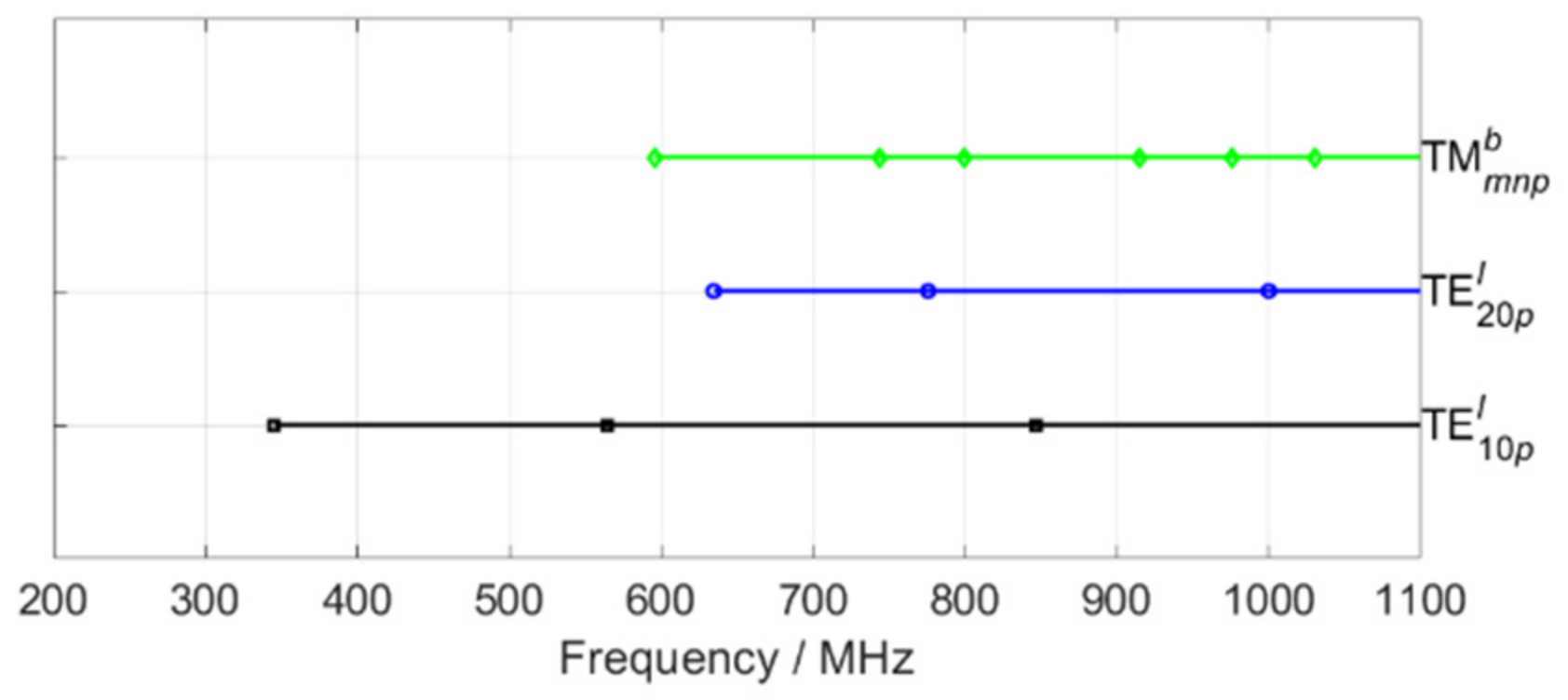
3. Simulation
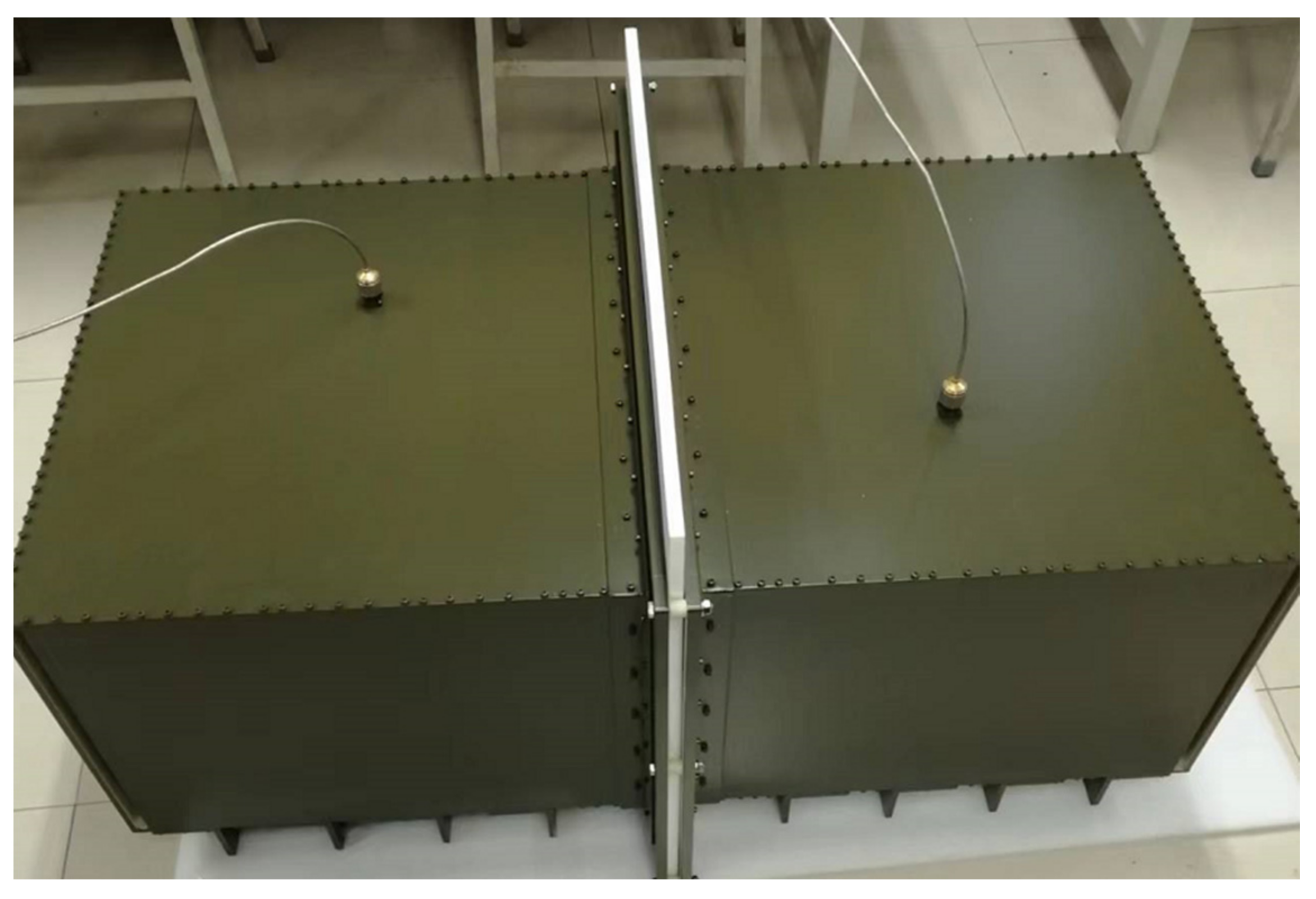
4. Measurement
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Hasar, U.C.; Bute, M. Electromagnetic characterization of thin dielectric materials from amplitude-only measurements. IEEE Sens. J. 2017, 17, 5093–5103. [Google Scholar] [CrossRef]
- Costa, F.; Borgese, M.; Degiorgi, M.; Monorchio, A. Electromagnetic characterization of materials by using transmission/reflection (T/R) devices. Electronics 2017, 6, 95. [Google Scholar] [CrossRef] [Green Version]
- Krupka, J. Frequency domain complex permittivity measurements at microwave frequencies. Meas. Sci. Technol. 2006, 17, 55–70. [Google Scholar] [CrossRef]
- Nicolson, A.; Ross, G. Measurement of the intrinsic properties of materials by time-dome techniques. IEEE Trans. Instrum. Meas. 1970, 19, 791–795. [Google Scholar] [CrossRef] [Green Version]
- Gorriti, A.; Slob, E. A new tool for accurate S-parameters measurements and permittivity reconstruction. IEEE Trans. Geosci. Remote Sens. 2005, 43, 1722–1735. [Google Scholar] [CrossRef]
- Weir, W. Automatic measurement of complex dielectric constant and permeability at microwave frequency. Proc. IEEE 1974, 62, 33–36. [Google Scholar] [CrossRef]
- Akhtar, M.; Feher, L.; Thumm, M. A waveguide-based two-step approach for measuring complex permittivity tensor of uniaxial composite materials. IEEE Trans. Microw. Theory Tech. 2006, 54, 2011–2022. [Google Scholar] [CrossRef]
- Wu, C.; Liu, Y.; Lu, S.; Gruszczynski, S.; Yashchyshyn, Y. Convenient waveguide technique for determining permittivity and permeability of materials. IEEE Trans. Microw. Theory Tech. 2020, 68, 4905–4912. [Google Scholar] [CrossRef]
- Shahpari, M. Determination of complex conductivity of thin strips with a transmission method. Electronics 2019, 8, 21. [Google Scholar] [CrossRef] [Green Version]
- Catala-Civera, J.; Canos, A.; Penarand-Foix, F.; Davos, E. Accurate determination of the complex permittivity of materials with transmission reflection measurements in partially filler rectangular waveguides. IEEE Trans. Microw. Theory Tech. 2003, 51, 16–24. [Google Scholar] [CrossRef]
- Hasar, U. Accurate complex permittivity inversion from measurements of a sample partially filling a waveguide aperture. IEEE Trans. Microw. Theory Tech. 2010, 58, 451–457. [Google Scholar] [CrossRef]
- Bao, X.; Wang, L.; Wang, Z.; Zhang, J.; Zhang, M.; Crupi, G.; Zhang, A. Simple, fast, and accurate broadband complex permittivity characterization algorithm: Methodology and experimental validation from 140 GHz up to 220 GHz. Electronics 2022, 11, 366. [Google Scholar] [CrossRef]
- Ghodgaonkar, D.K.; Varadan, V.V.; Varadan, V.K. Free-space measurement of complex permittivity and complex permeability of magnetic materials at microwave frequencies. IEEE Trans. Instrum. Meas. 1990, 39, 387–394. [Google Scholar] [CrossRef]
- Hasar, U.C. Non-destructive testing of hardened cement specimens at microwave frequencies using a simple free-space method. NDTE Int. 2009, 42, 550–557. [Google Scholar] [CrossRef]
- Hegler, S.; Seiler, P.; Dinkelaker, M.; Schladitz, F.; Plettemeier, D. Electrical material properties of carbon reinforced concrete. Electronics 2020, 9, 857. [Google Scholar] [CrossRef]
- Yang, X.; Liu, X.; Yu, S.; Gan, L.; Zhou, J.; Zeng, Y. Permittivity of undoped silicon in the millimeter wave range. Electronics 2019, 8, 886. [Google Scholar] [CrossRef] [Green Version]
- Gonçalves, F.J.F.; Pinto, A.G.M.; Mesquita, R.C.; Silva, E.J.; Brancaccio, A. Free-space materials characterization by reflection and transmission measurement using frequency-by-frequency and multi-frequency algorithms. Electronics 2018, 7, 260. [Google Scholar] [CrossRef] [Green Version]
- Eyraud, C.; Geffrin, J.M.; Litman, A.; Tortel, H. Complex permittivity determination from far-field scattering patterns. IEEE Antennas Wirel. Propag. Lett. 2015, 14, 309–312. [Google Scholar] [CrossRef]
- Abbato, R. Dielectric constant measurements using RCS data. Proc. IEEE 1965, 53, 1095–1097. [Google Scholar] [CrossRef]
- Horner, F.; Taylor, T.A.; Dunsmuir, R.; Lamb, J.; Jackson, W. Resonance methods of dielectric measurement at centimetre wavelength. J. Inst. Electr. Eng.-Part III Radio Commun. Eng. 1946, 93, 53–68. [Google Scholar]
- Krupka, J.; Milewski, A. Accurate method of the measurement of complex permittivity of semiconductors in H01n cylindrical cavity. Electron. Technol. 1978, 11, 11–31. [Google Scholar]
- Li, S.; Akyel, C.; Bosisio, R.G. Precise calculations and measurement on the complex dielectric constant of lossy materials using TM010 perturbation techniques. IEEE Trans. Microw. Theory Tech. 1981, 29, 1041–1048. [Google Scholar]
- Risman, P.O.; Ohlsson, T. Theory for and experiments with a TM020 applicator. J. Microw. Power 1975, 10, 271–280. [Google Scholar] [CrossRef]
- Ma, J.; Wu, Z.; Xia, Q.; Wang, S.; Tang, J.; Wang, K.; Guo, L.; Jiang, H.; Zeng, B.; Gong, Y. Complex permittivity measurement of high-loss biological material with improved cavity perturbation method in the range of 26.5–40 GHz. Electronics 2020, 9, 1200. [Google Scholar] [CrossRef]
- Jha, A.K.; Akhtar, M.J. A generalized rectangular cavity approach for determination of complex permittivity of materials. IEEE Trans. Instrum. Meas. 2014, 63, 2632–2641. [Google Scholar] [CrossRef]
- Jones, R.G. Precise dielectric measurements at 35 GHz using a microwave open-resonator. Proc. Inst. Electr. Eng. 1976, 123, 285–290. [Google Scholar] [CrossRef]
- Lynch, A.C.; Clarke, R.N. Open resonators: Improvement of confidence in measurement of loss. IEEE Proc. 1992, 139, 221–225. [Google Scholar] [CrossRef]
- Hakki, B.W.; Coleman, P.D. A dielectric resonator method of measuring inductive capacities in the millimeter range. IEEE Trans. Microw. Theory Tech. 1960, 8, 402–410. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Katoh, M. Microwave measurement of dielectric properties of low-loss materials by the dielectric rod resonator method. IEEE Trans. Microw. Theory Tech. 1985, 33, 586–592. [Google Scholar] [CrossRef]
- Krupka, J.; Derzakowski, K.; Riddle, B.; Baker-Jarvis, J. A dielectric resonator for measurements of complex permittivity of low loss dielectric materials as a function of temperature. Meas. Sci. Technol. 1998, 9, 1751–1756. [Google Scholar] [CrossRef]
- Krupka, J.; Huang, W.T.; Tung, M.J. Complex permittivity measurements of low loss microwave ceramics employing higher order quasi TE0np modes excited in a cylindrical dielectric sample. Meas. Sci. Technol. 2005, 16, 1014–1020. [Google Scholar] [CrossRef]
- Krupka, J.; Derzakowski, K.; Abramowicz, A.; Tobar, M.E.; Geyer, R.G. Whispering gallery modes for complex permittivity measurements of ultra-low loss dielectric materials. IEEE Trans. Microw. Theory Tech. 1999, 47, 752–759. [Google Scholar] [CrossRef]
- Krupka, J.; Derzakowski, K.; Tobar, M.E.; Hartnett, J.; Geyer, R.G. Complex permittivity of some ultralow loss dielectric crystals at cryogenic temperatures. Meas. Sci. Technol. 1999, 10, 387–392. [Google Scholar] [CrossRef]
- Roelvink, J.; Trabelsi, S.; Nelson, S.O. Measuring the complex permittivity tensor of uniaxial biological materials with coplanar waveguide transmission line. IEEE Microw. Wirel. Compon. Lett. 2014, 24, 811–813. [Google Scholar] [CrossRef]
- Hashimoto, O.; Shimizu, Y. Reflecting characteristics of anisotropic rubber sheets and measurement of complex permittivity tensor. IEEE Trans. Microw. Theory Tech. 1986, 34, 1202–1207. [Google Scholar] [CrossRef]
- Damaskos, N.J.; Mack, R.B.; Maffett, A.L.; Parmon, W.; Uslenghi, P.L.E. The inverse problem for biaxial materials. IEEE Trans. Microw. Theory Tech. 1984, 32, 400–405. [Google Scholar] [CrossRef]
- Belhadj-Tahar, N.E.; Fourrier-Lamer, A. Broad-band simultaneous measurement of the complex permittivity tensor for uniaxial materials using a coaxial discontinuity. IEEE Trans. Microw. Theory Tech. 1991, 39, 1718–1724. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Tanaka, S. Resonant modes of a dielectric rod resonator short-circuited at both ends by parallel conducting plates. IEEE Trans. Microw. Theory Tech. 1980, 28, 1077–1085. [Google Scholar] [CrossRef]
- Geyer, R.G.; Krupka, J. Microwave dielectric properties of anisotropic materials at cryogenic temperatures. IEEE Trans. Instrum. Meas. 1995, 44, 329–331. [Google Scholar] [CrossRef]
- Krupka, J.; Geyer, R.G.; Kuhn, M.; Hinken, J. Dielectric properties of single crystal Al2O3, LaAlO3, NdGaO3, SrTiO3, and MgO at cryogenic temperatures and microwave frequencies. IEEE Trans. Microw, Theory Tech. 1994, 42, 1886–1890. [Google Scholar] [CrossRef]
- Chen, L.; Ong, C.K.; Tan, B.T.G. Cavity perturbation technique for the measurement of permittivity tensor of uniaxially anisotropic dielectrics. IEEE Trans. Instrum. Meas. 1999, 48, 1023–1030. [Google Scholar] [CrossRef]
- Mumcu, G.; Sertel, K.; Volakis, J.L. A measurement process to characterize natural and engineered low-loss uniaxial dielectric materials at microwave frequencies. IEEE Trans. Microw. Theory Tech. 2008, 56, 217–223. [Google Scholar] [CrossRef]
- Kopyt, P.; Salski, B.; Krupka, J. Measurements of complex anisotropic permittivity of laminates with TM0n0 cavity. IEEE Trans. Microw. Theory Tech. 2022, 70, 432–443. [Google Scholar] [CrossRef]
- Baker-Jarvis, J.; Riddle, B.; Janezic, M.D. Dielectric and Magnetic Properties of Printed Wiring Boards and Other Substrate Materials; NIST: Gaithersburg, MD, USA, 1999; p. 1512. [Google Scholar]




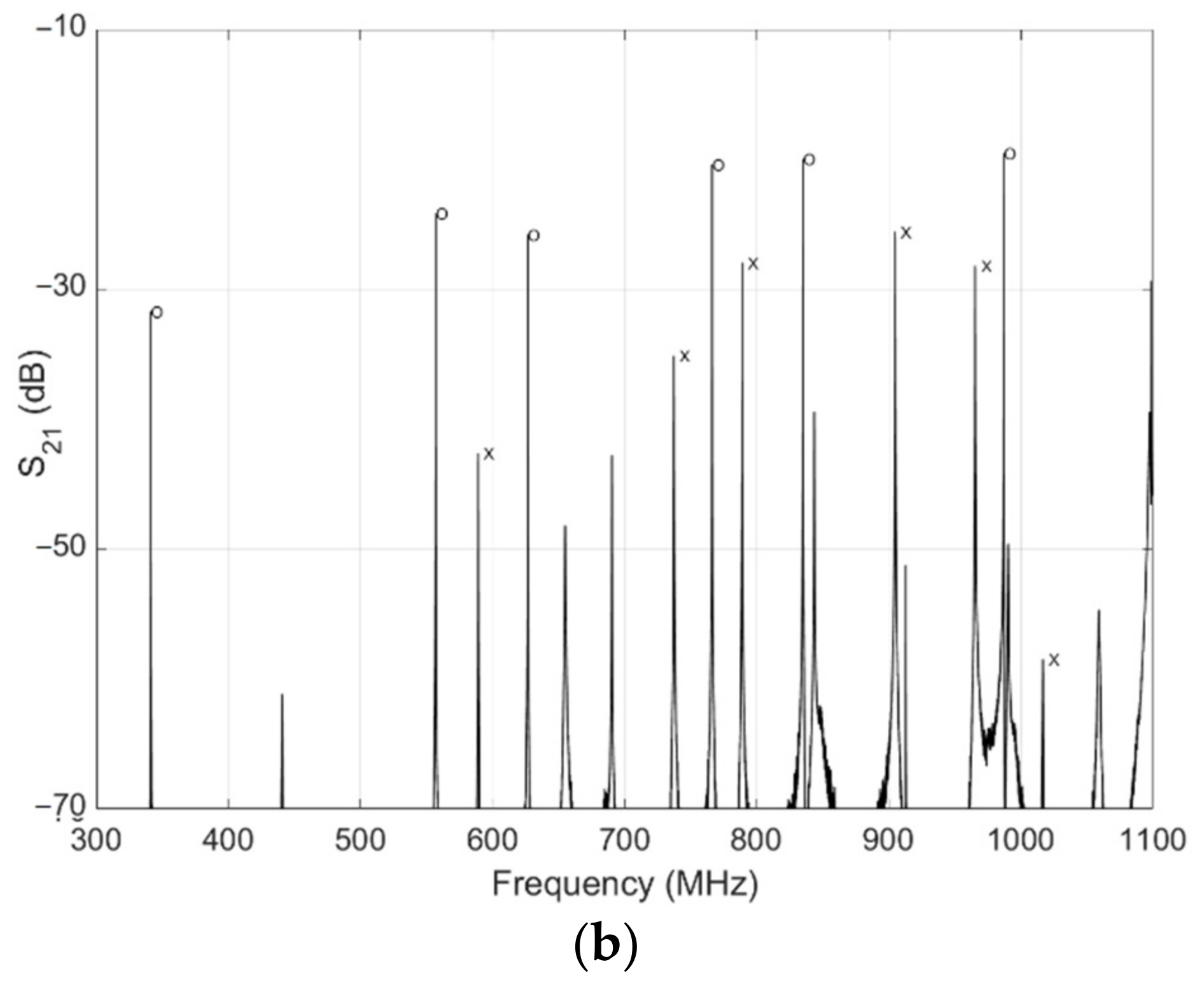
| TEl101 | TEl103 | TEl201 | TEl203 | TEl105 | TEl205 | ||
|---|---|---|---|---|---|---|---|
| Empty freq (MHz) | 345 | 564 | 634 | 775 | 846 | 999 | |
| Empty Q-factor | 32,579 | 48,835 | 42,299 | 51,322 | 61,549 | 62,201 | |
| ∥ | Loaded freq (MHz) | 334 | 547 | 611 | 753 | 821 | 971 |
| Loaded Q-factor | 19,768 | 27,040 | 20,492 | 28,806 | 31,549 | 31,836 | |
| εr | 3.98 | 3.96 | 3.99 | 3.98 | 3.96 | 3.96 | |
| tanδ | 0.00020 | 0.00020 | 0.00020 | 0.00020 | 0.00019 | 0.00021 | |
| ⊥ | Loaded freq (MHz) | 341 | 558 | 627 | 767 | 837 | 988 |
| Loaded Q-factor | 24,184 | 33,744 | 29,417 | 35,045 | 40,218 | 42,320 | |
| εr | 2.09 | 2.09 | 2.10 | 2.09 | 2.09 | 2.09 | |
| tanδ | 0.00020 | 0.00020 | 0.00020 | 0.00020 | 0.00018 | 0.00019 | |
| TEl101 | TEl103 | TEl201 | TEl203 | TEl105 | TEl205 | |
|---|---|---|---|---|---|---|
| Empty freq (MHz) | 341 | 557 | 627 | 766 | 835 | 987 |
| Empty Q-factor | 34,628 | 53,473 | 45,149 | 54,996 | 62,859 | 63,700 |
| Loaded freq (MHz) | 341 | 557 | 627 | 766 | 835 | 987 |
| Loaded Q-factor | 26,863 | 37,586 | 31,113 | 38,219 | 41,536 | 42,483 |
| εr | 2.04 | 2.04 | 2.06 | 2.05 | 2.04 | 2.05 |
| tanδ | 0.00019 | 0.00019 | 0.00021 | 0.00019 | 0.00020 | 0.00019 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Wu, C.; Shen, L. Complex Permittivity Measurement of Low-Loss Anisotropic Dielectric Materials at Hundreds of Megahertz. Electronics 2022, 11, 1769. https://doi.org/10.3390/electronics11111769
Li C, Wu C, Shen L. Complex Permittivity Measurement of Low-Loss Anisotropic Dielectric Materials at Hundreds of Megahertz. Electronics. 2022; 11(11):1769. https://doi.org/10.3390/electronics11111769
Chicago/Turabian StyleLi, Chuanlan, Changying Wu, and Lifei Shen. 2022. "Complex Permittivity Measurement of Low-Loss Anisotropic Dielectric Materials at Hundreds of Megahertz" Electronics 11, no. 11: 1769. https://doi.org/10.3390/electronics11111769
APA StyleLi, C., Wu, C., & Shen, L. (2022). Complex Permittivity Measurement of Low-Loss Anisotropic Dielectric Materials at Hundreds of Megahertz. Electronics, 11(11), 1769. https://doi.org/10.3390/electronics11111769





