Dual-Band Single-Layer Fractal Frequency Selective Surface for 5G Applications
Abstract
:1. Introduction
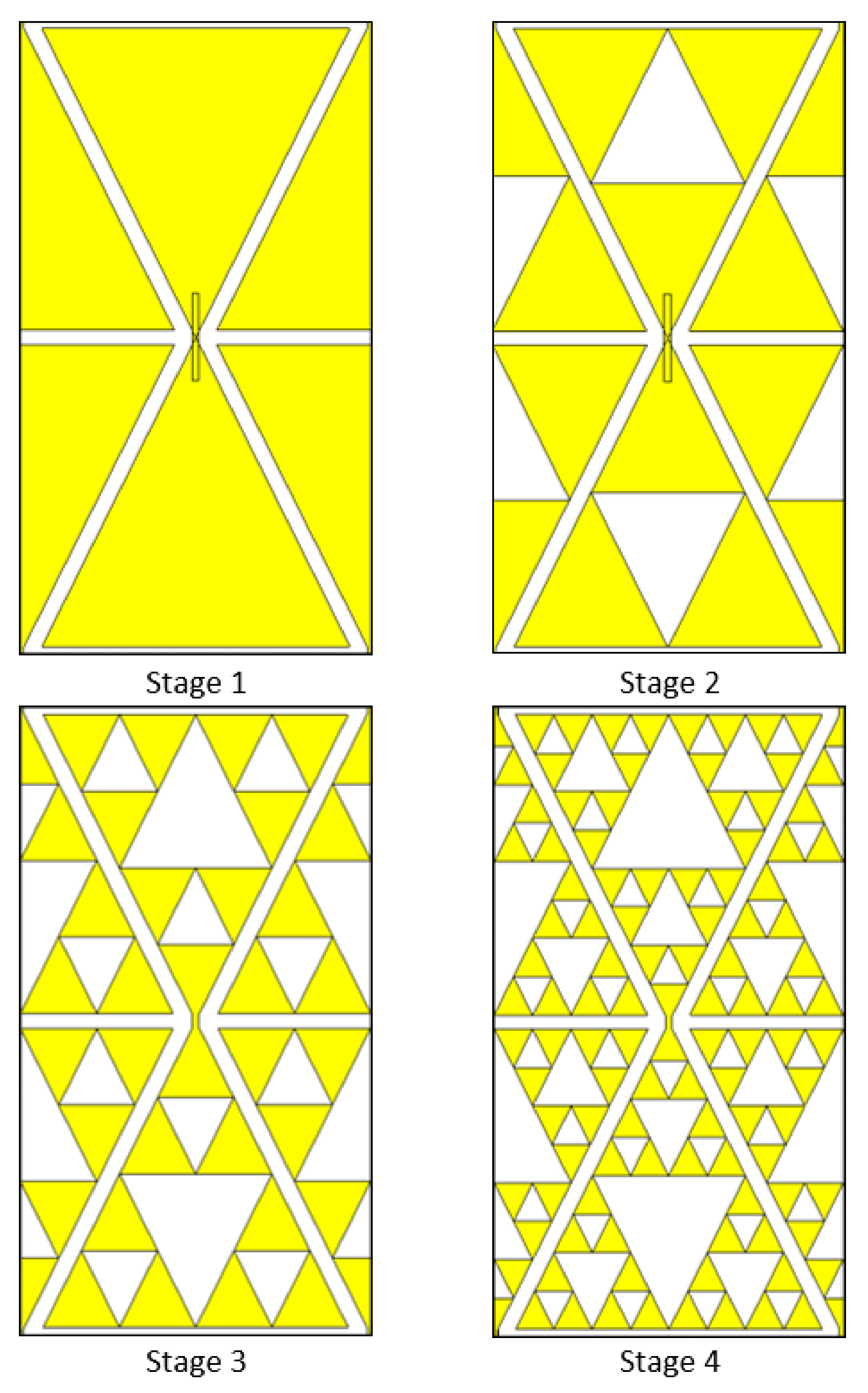
2. Self-Similar Structures and Fractal Antennas
3. The Unit Cell
- is the distance from the center of the metal strip and the corners of Sierpiński triangles to the side;
- is the distance in between the bottom and the top of two neighboring Sierpiński triangles.
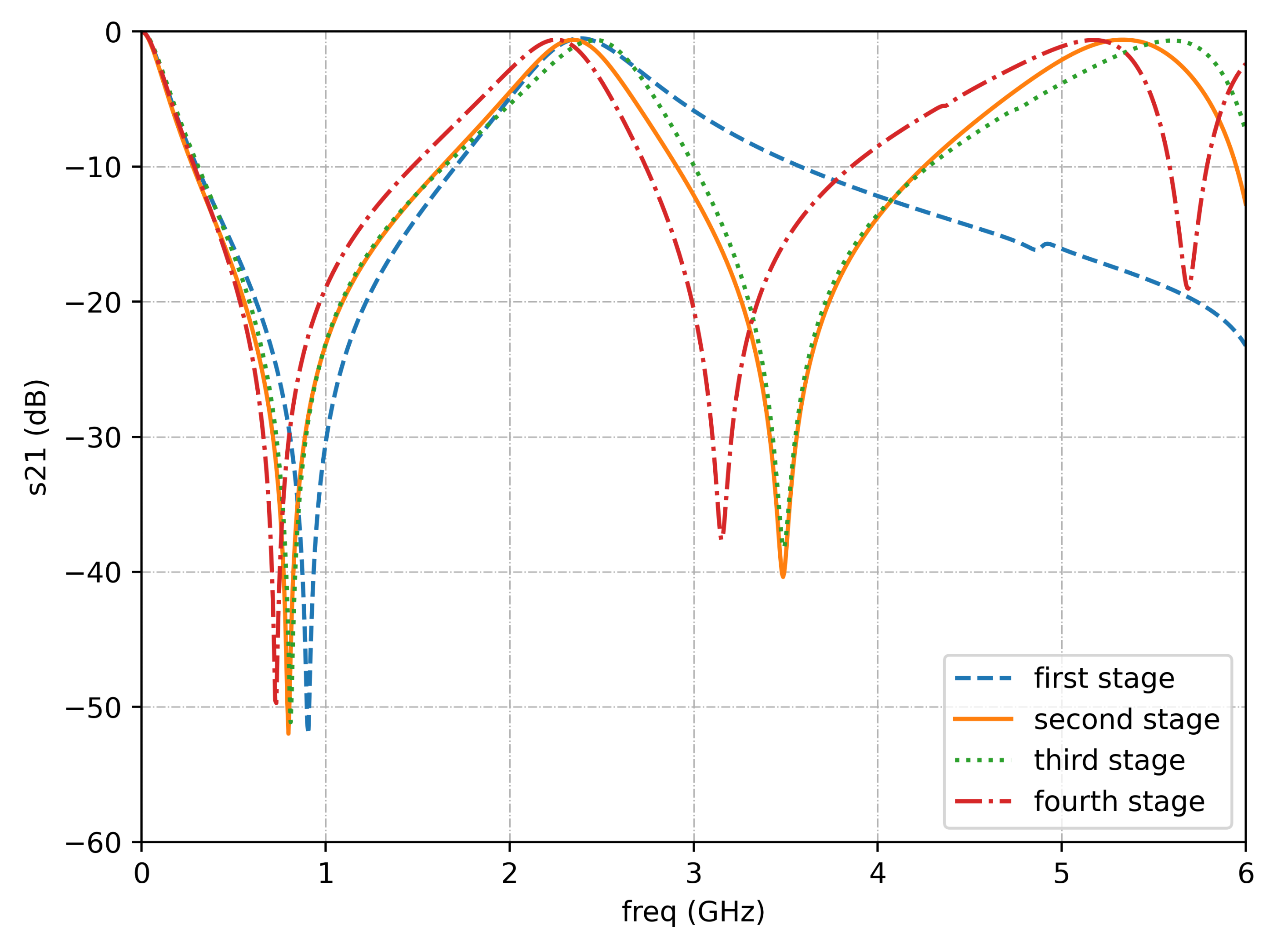
4. Design Parameters and Guidelines
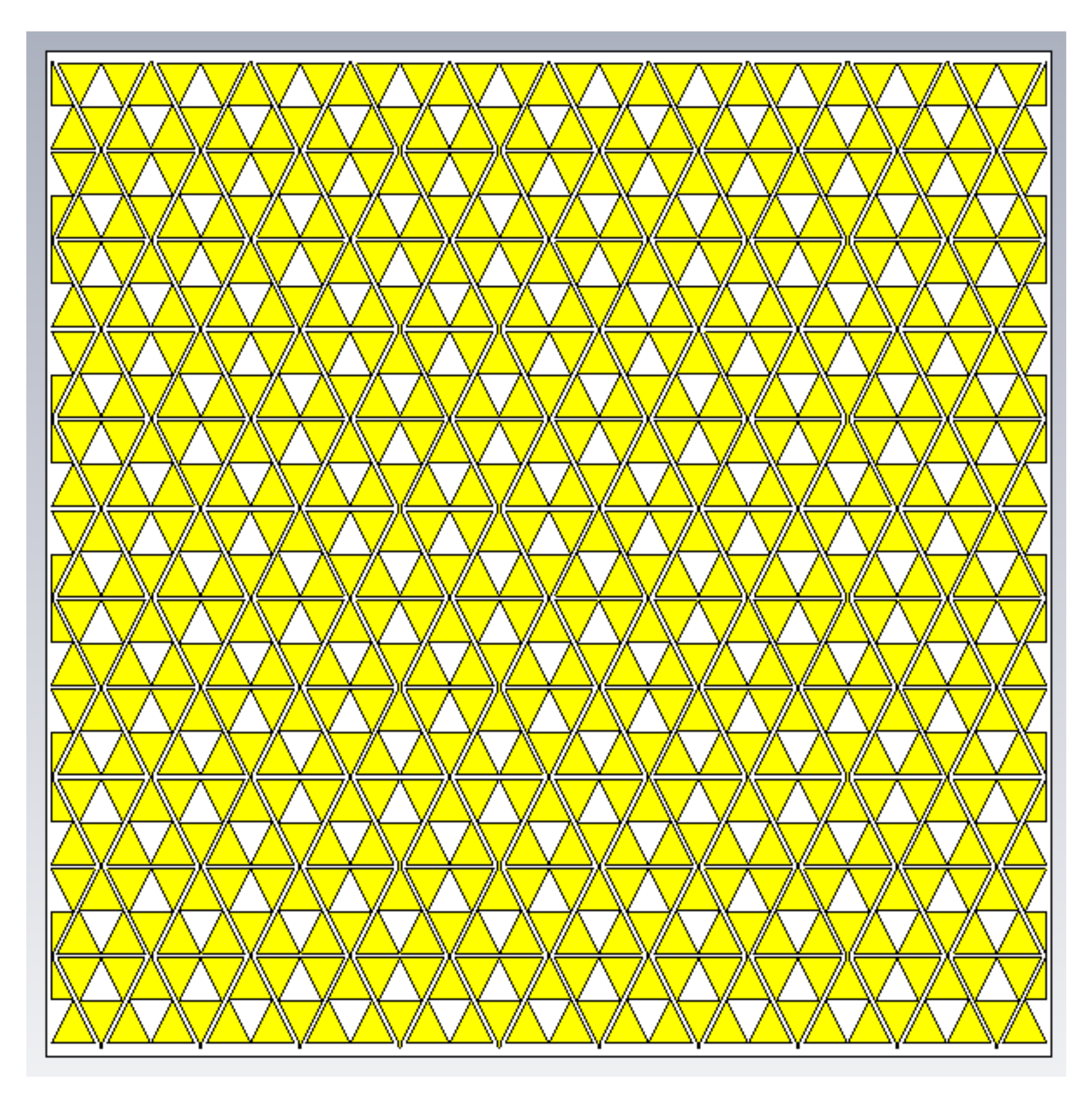
4.1. Simulating in CST Microwave Studio
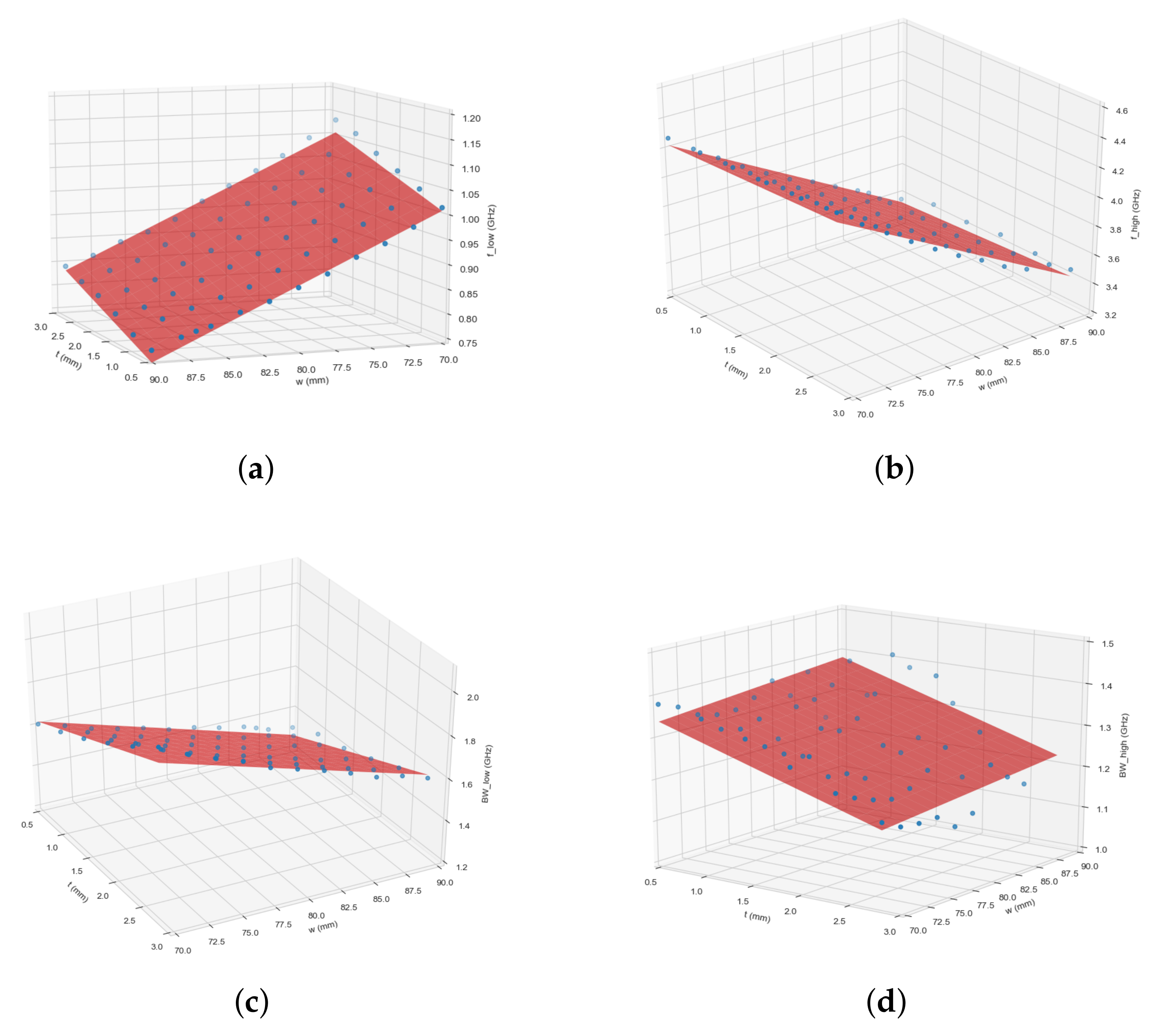
4.2. Unit Cell Size w and Central Element Width t
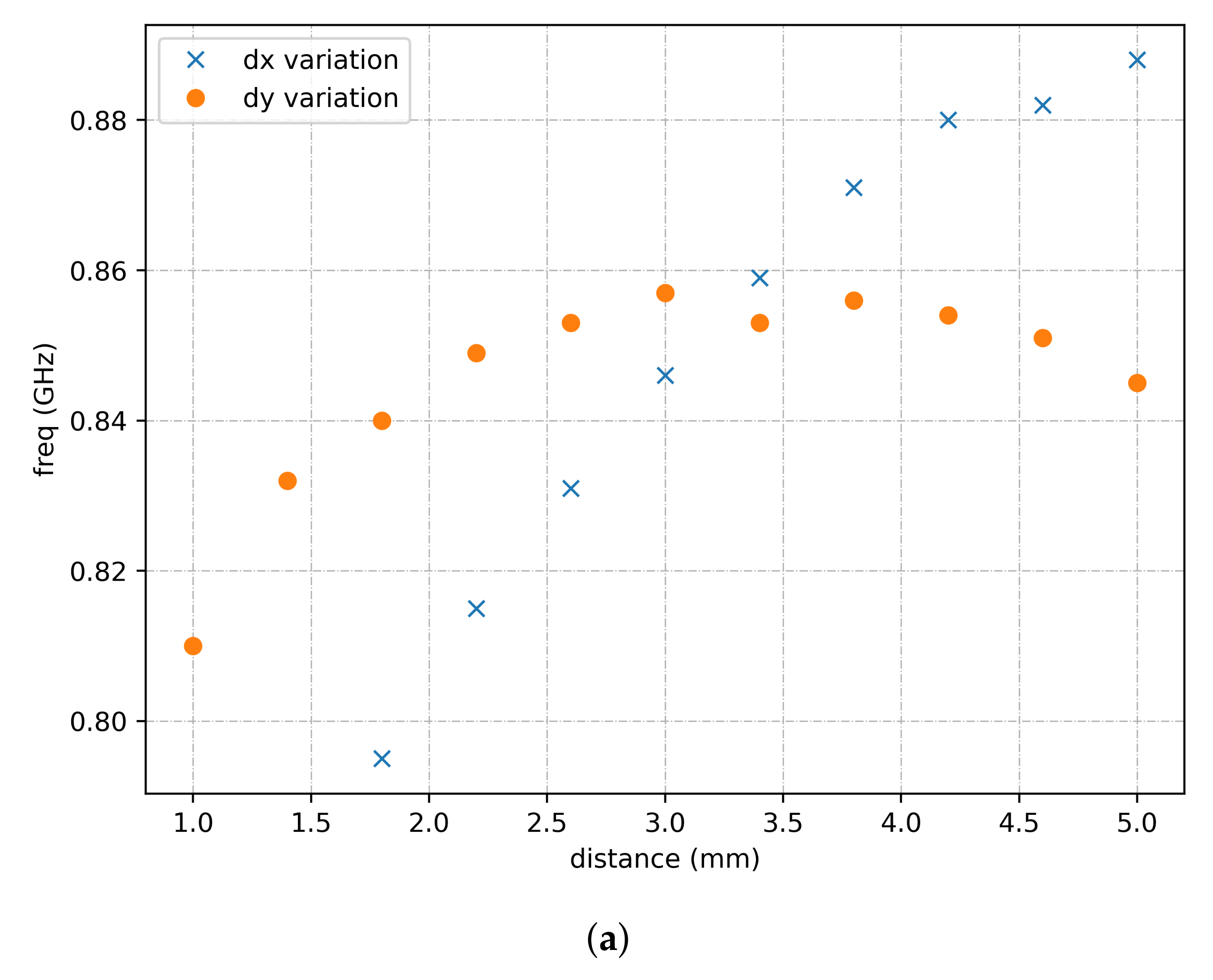
4.3. Distance between Bow Ties dx and dy
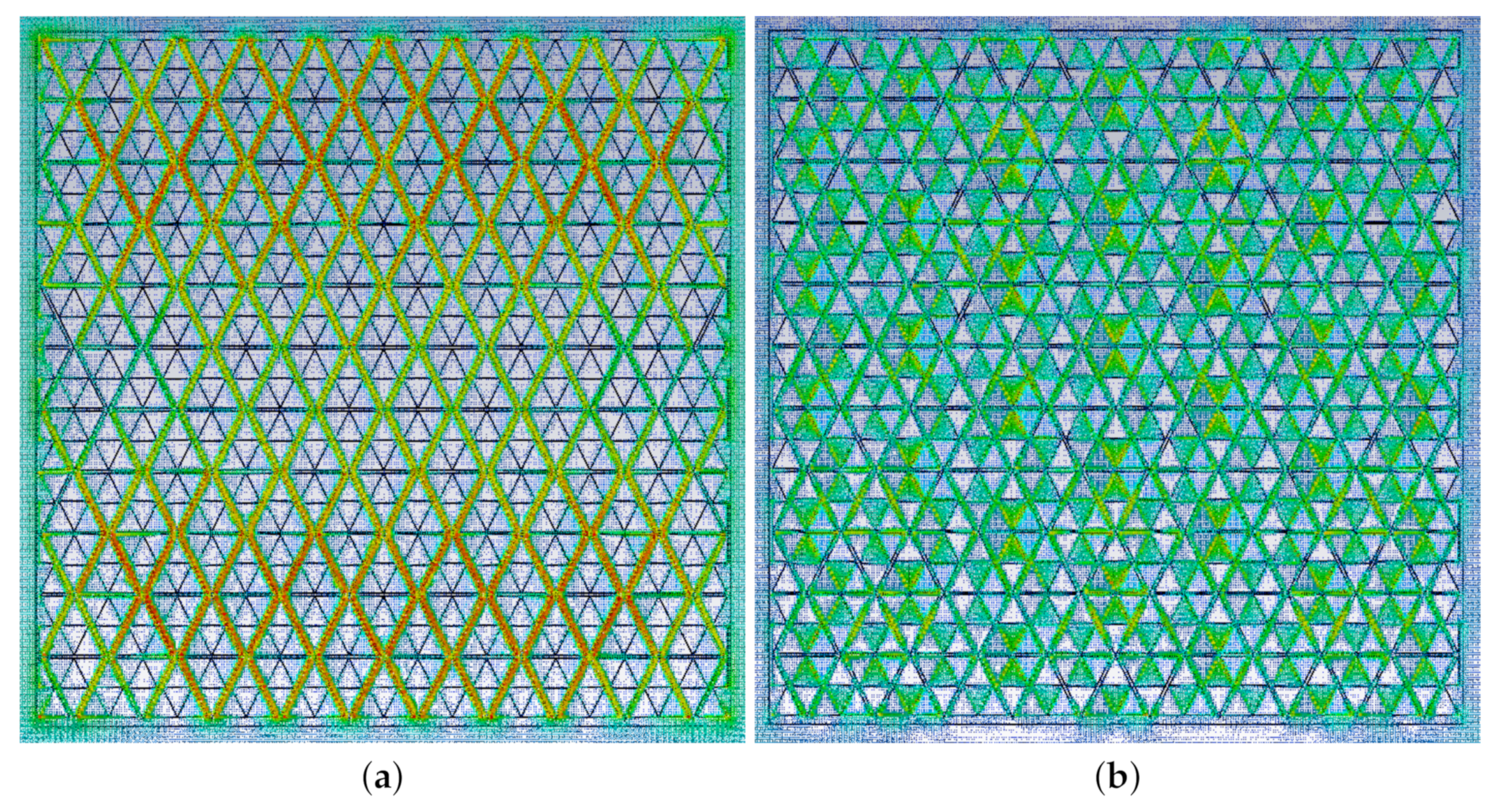
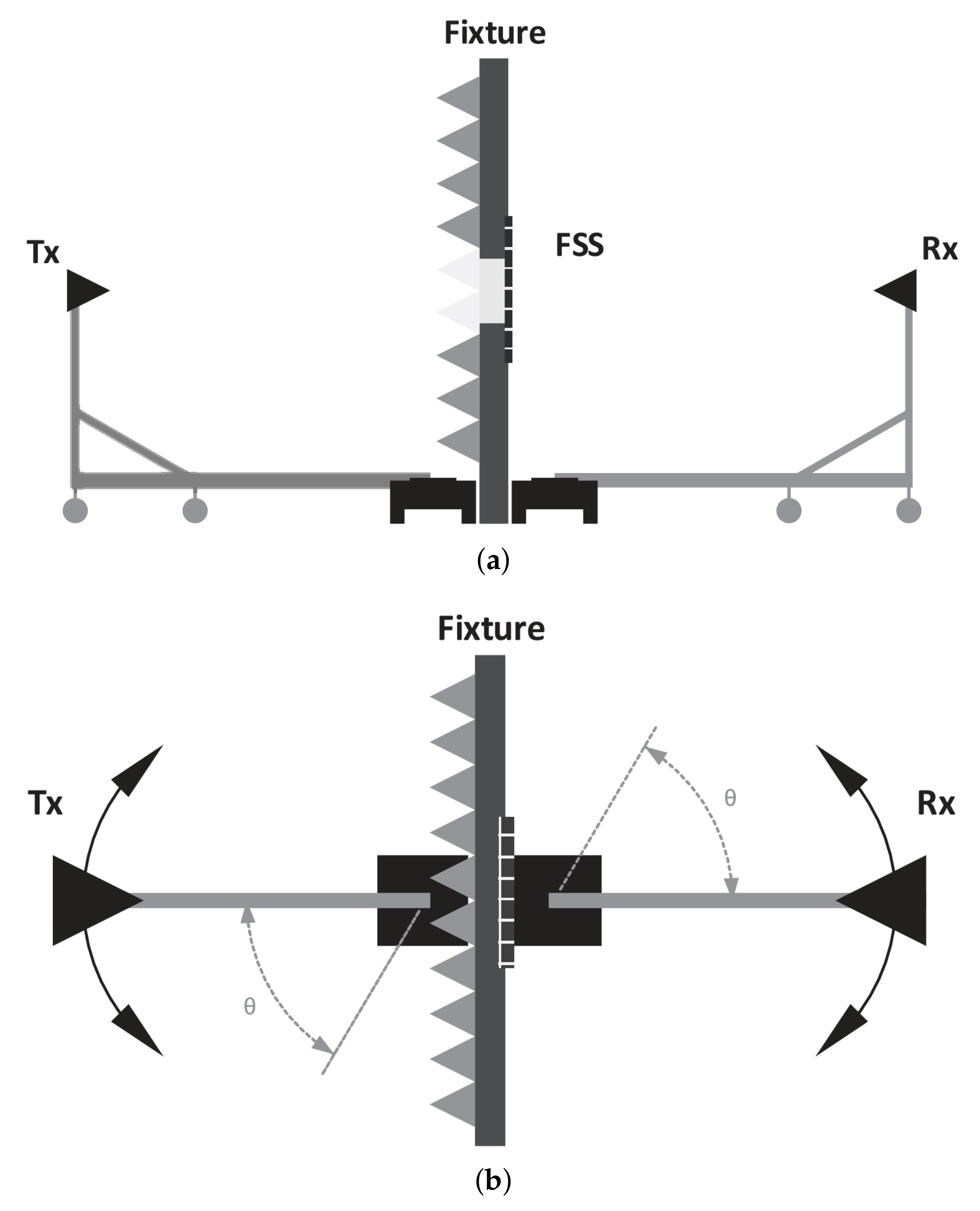
5. Angle of Incidence
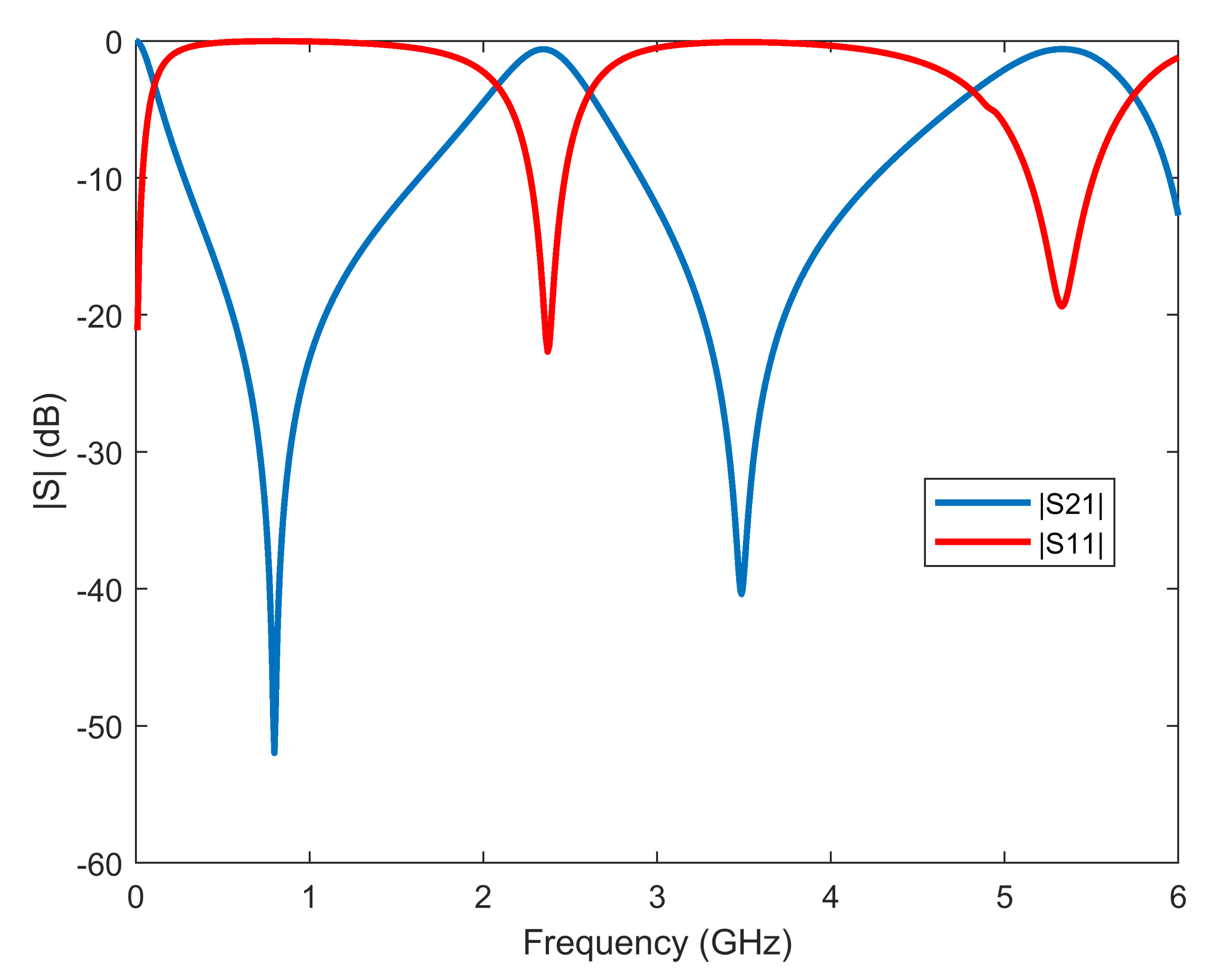
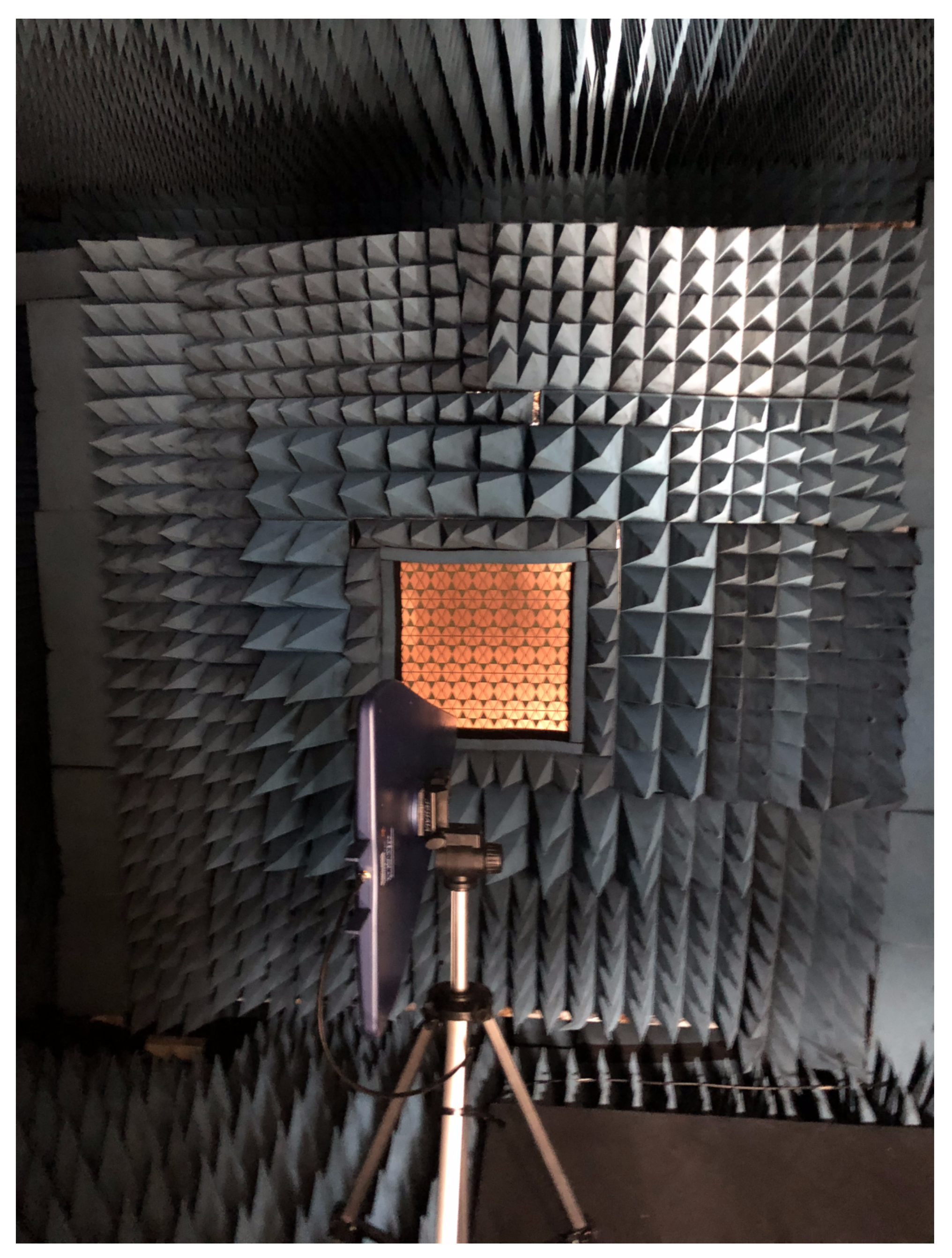
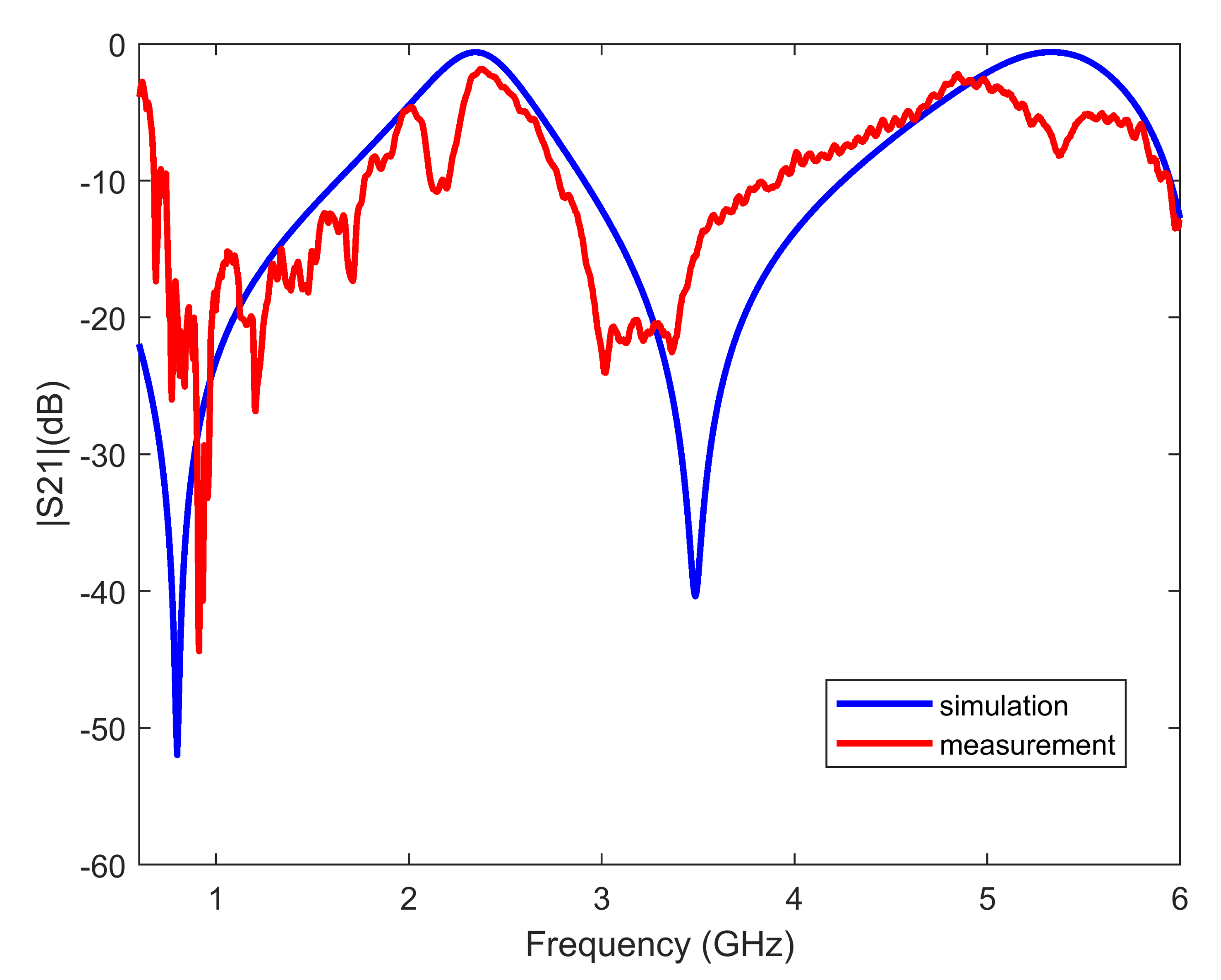
6. Measurements
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Shafi, M.; Molisch, A.F.; Smith, P.J.; Haustein, T.; Zhu, P.; De Silva, P.; Tufvesson, F.; Benjebbour, A.; Wunder, G. 5G: A Tutorial Overview of Standards, Trials, Challenges, Deployment, and Practice. IEEE J. Sel. Areas Commun. 2017, 35, 1201–1221. [Google Scholar] [CrossRef]
- Ghosh, A.; Maeder, A.; Baker, M.; Chandramouli, D. 5G Evolution: A View on 5G Cellular Technology Beyond 3GPP Release 15. IEEE Access 2019, 7, 127639–127651. [Google Scholar] [CrossRef]
- Henry, S.; Alsohaily, A.; Sousa, E.S. 5G is Real: Evaluating the Compliance of the 3GPP 5G New Radio System With the ITU IMT-2020 Requirements. IEEE Access 2020, 8, 42828–42840. [Google Scholar] [CrossRef]
- Tullberg, H.; Popovski, P.; Li, Z.; Uusitalo, M.A.; Hoglund, A.; Bulakci, O.; Fallgren, M.; Monserrat, J.F. The METIS 5G System Concept: Meeting the 5G Requirements. IEEE Commun. Mag. 2016, 54, 132–139. [Google Scholar] [CrossRef]
- Imran, A.; Zoha, A.; Abu-Dayya, A. Challenges in 5G: How to Empower SON with Big Data for Enabling 5G. IEEE Netw. 2014, 28, 27–33. [Google Scholar] [CrossRef]
- South Korea Wraps 5G Auction for 3.5, 28 GHz. Available online: https://www.fiercewireless.com/wireless/south-korea-wraps-5g-auction-for-3-5-28-ghz (accessed on 17 November 2021).
- A Guide to 5G Spectrum Auctions in Western Europe: Nordic Edition. Available online: https://blog.telegeography.com/a-guide-to-5g-spectrum-auctions-in-western-europe-nordic-edition (accessed on 17 November 2021).
- 5G Spectrum. GSMA Public Policy Position; GSMA Head Office: London, UK, March 2020. [Google Scholar]
- Munk, B.A. Frequency Selective Surfaces: Theory and Design; Wiley: New York, NY, USA, 2000. [Google Scholar]
- Ferreira, D.; Caldeirinha, R.F.S.; Cuinas, I.; Fernandes, T.R. A Review of Manufacturing Materials and Production Methods for Frequency-Selective Structures [Wireless Corner]. IEEE Antennas Propag. Mag. 2018, 60, 110–119. [Google Scholar] [CrossRef]
- Romeu, J.; Rahmat-Samii, Y. Fractal FSS: A Novel Dual-Band Frequency Selective Surface. IEEE Trans. Antennas Propag. 2000, 48, 1097–1105. [Google Scholar] [CrossRef] [Green Version]
- CST Microwave Studio. Dassault Système, Vélizy-Villacoublay Cedex. Available online: https://www.3ds.com/products-services/simulia/products/cst-studio-suite/ (accessed on 17 November 2021).
- Numan, A.B.; Sharawi, M.S. Extraction of Material Parameters for Metamaterials Using a Full-Wave Simulator [Education Column]. IEEE Antennas Propag. Mag. 2013, 55, 202–211. [Google Scholar] [CrossRef]
- Dassault Systèmes. CST Studio Suite Help; Dassault Systèmes: Waltham, MA, USA, 2019. [Google Scholar]
- Mandelbrot, B.B. The Fractal Geometry of Nature; W.H.Freeman & Co Ltd.: San Francisco, CA, USA, 1982. [Google Scholar]
- Kim, Y.; Jaggard, D.L. The Fractal Random Array. Proc. IEEE 1986, 74, 1278–1280. [Google Scholar] [CrossRef]
- Felber, P. Fractal Antennas; Technical Report; Illinois Institute of Technology: Chicago, IL, USA, 2000. [Google Scholar]
- Werner, D.H.; Ganguly, S. An Overview of Fractal Antenna Engineering Research. IEEE Antennas Propag. Mag. 2003, 45, 38–57. [Google Scholar] [CrossRef] [Green Version]
- Ghosh, B.; Sinha, S.N.; Kartikeyan, M.V. Fractal Apertures in Waveguides, Conducting Screens and Cavities; Springer Series in Optical Sciences; Springer International Publishing: Cham, Switzerland, 2014; Volume 187. [Google Scholar] [CrossRef]
- Munk, B.A. Finite Antenna Arrays and FSS; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2003. [Google Scholar] [CrossRef]

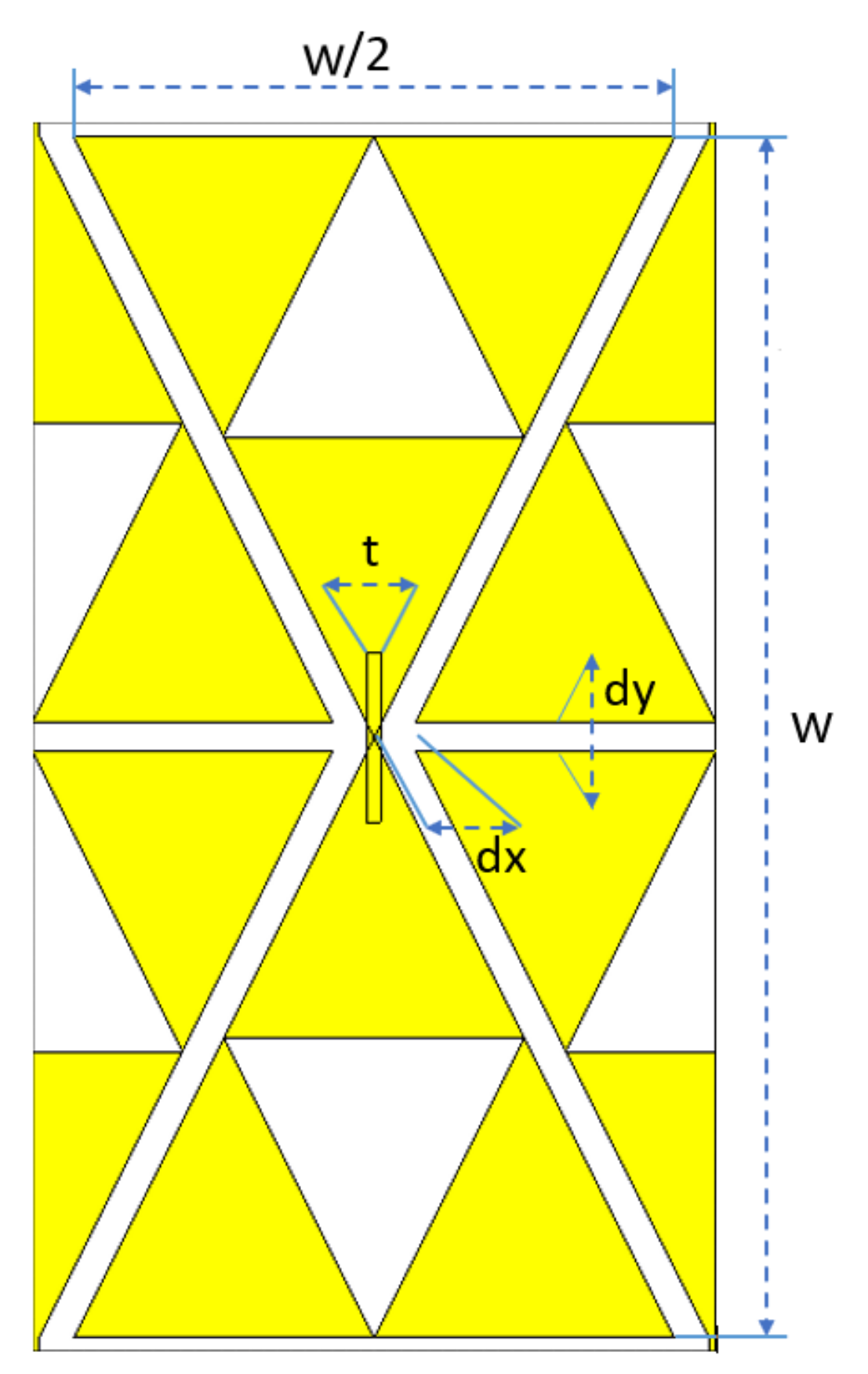



| Parameter | Explanation | Value (mm) |
|---|---|---|
| w | height of the bow tie | 87 |
| t | width of the central element | |
| horizontal distance between elements | 3 | |
| vertical distance between elements | 2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Decoster, B.; Maes, S.; Cuiñas, I.; García Sánchez, M.; Caldeirinha, R.; Verhaevert, J. Dual-Band Single-Layer Fractal Frequency Selective Surface for 5G Applications. Electronics 2021, 10, 2880. https://doi.org/10.3390/electronics10222880
Decoster B, Maes S, Cuiñas I, García Sánchez M, Caldeirinha R, Verhaevert J. Dual-Band Single-Layer Fractal Frequency Selective Surface for 5G Applications. Electronics. 2021; 10(22):2880. https://doi.org/10.3390/electronics10222880
Chicago/Turabian StyleDecoster, Bram, Stephanie Maes, Iñigo Cuiñas, Manuel García Sánchez, Rafael Caldeirinha, and Jo Verhaevert. 2021. "Dual-Band Single-Layer Fractal Frequency Selective Surface for 5G Applications" Electronics 10, no. 22: 2880. https://doi.org/10.3390/electronics10222880
APA StyleDecoster, B., Maes, S., Cuiñas, I., García Sánchez, M., Caldeirinha, R., & Verhaevert, J. (2021). Dual-Band Single-Layer Fractal Frequency Selective Surface for 5G Applications. Electronics, 10(22), 2880. https://doi.org/10.3390/electronics10222880









