2-D Beam Focusing Control Based on Passive Frequency Selective Surface (FSS)
Abstract
:1. Introduction
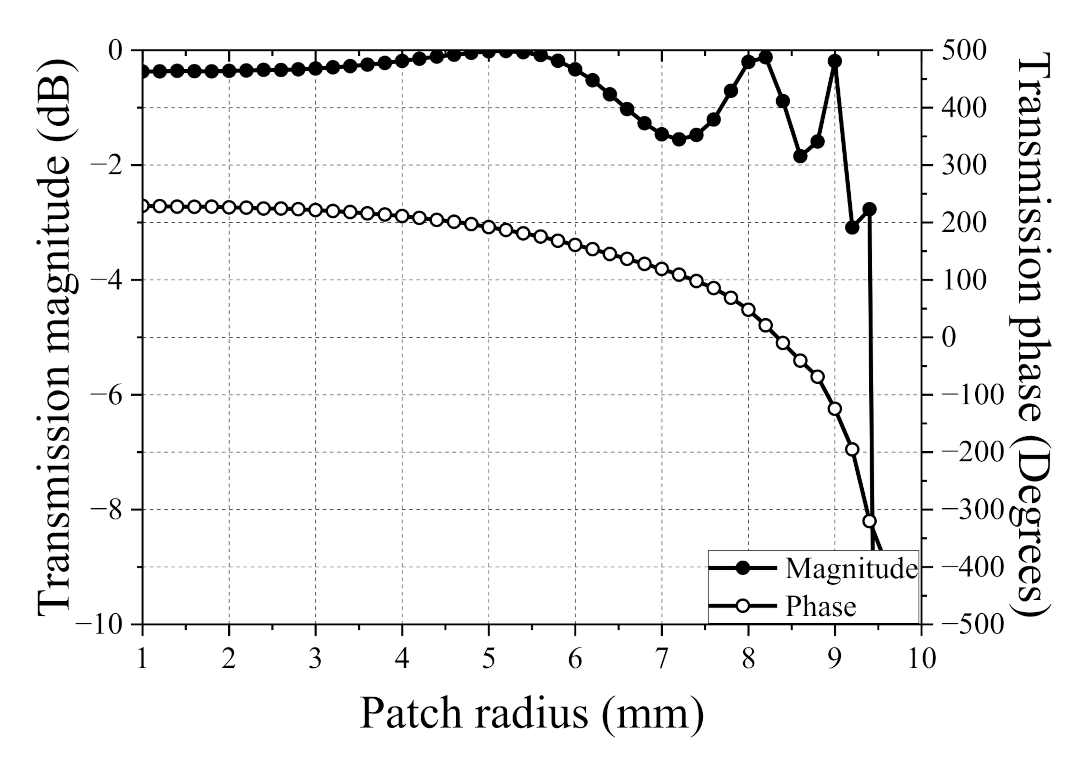
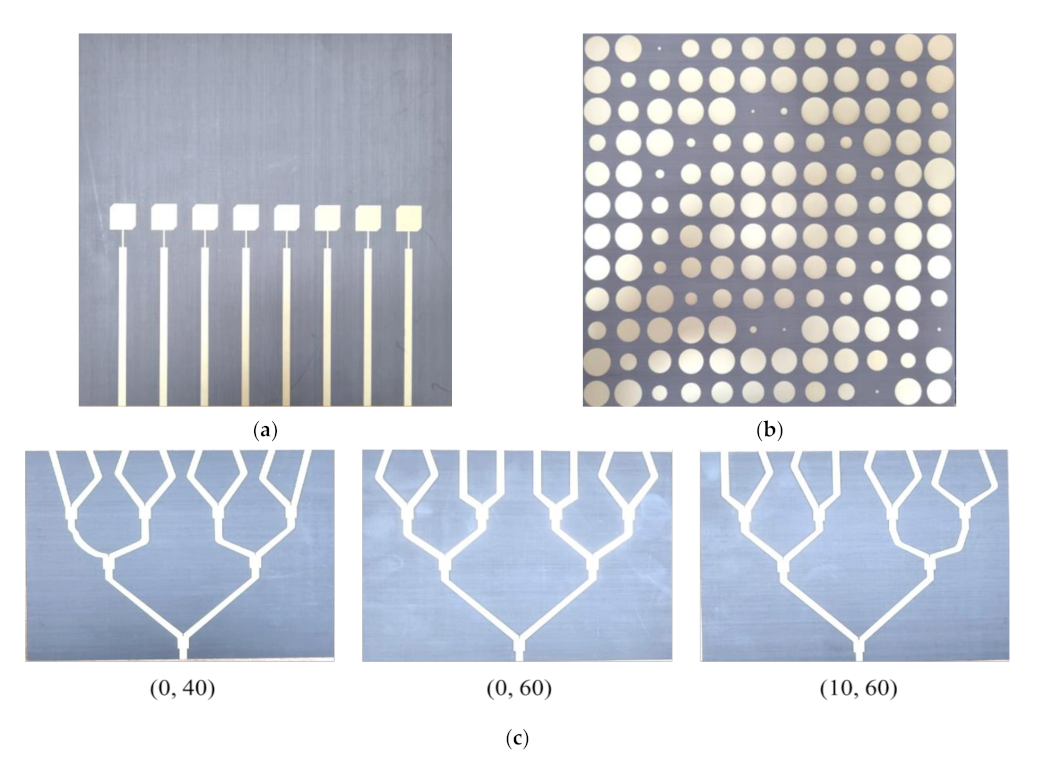
2. Design of Array Source and FSS
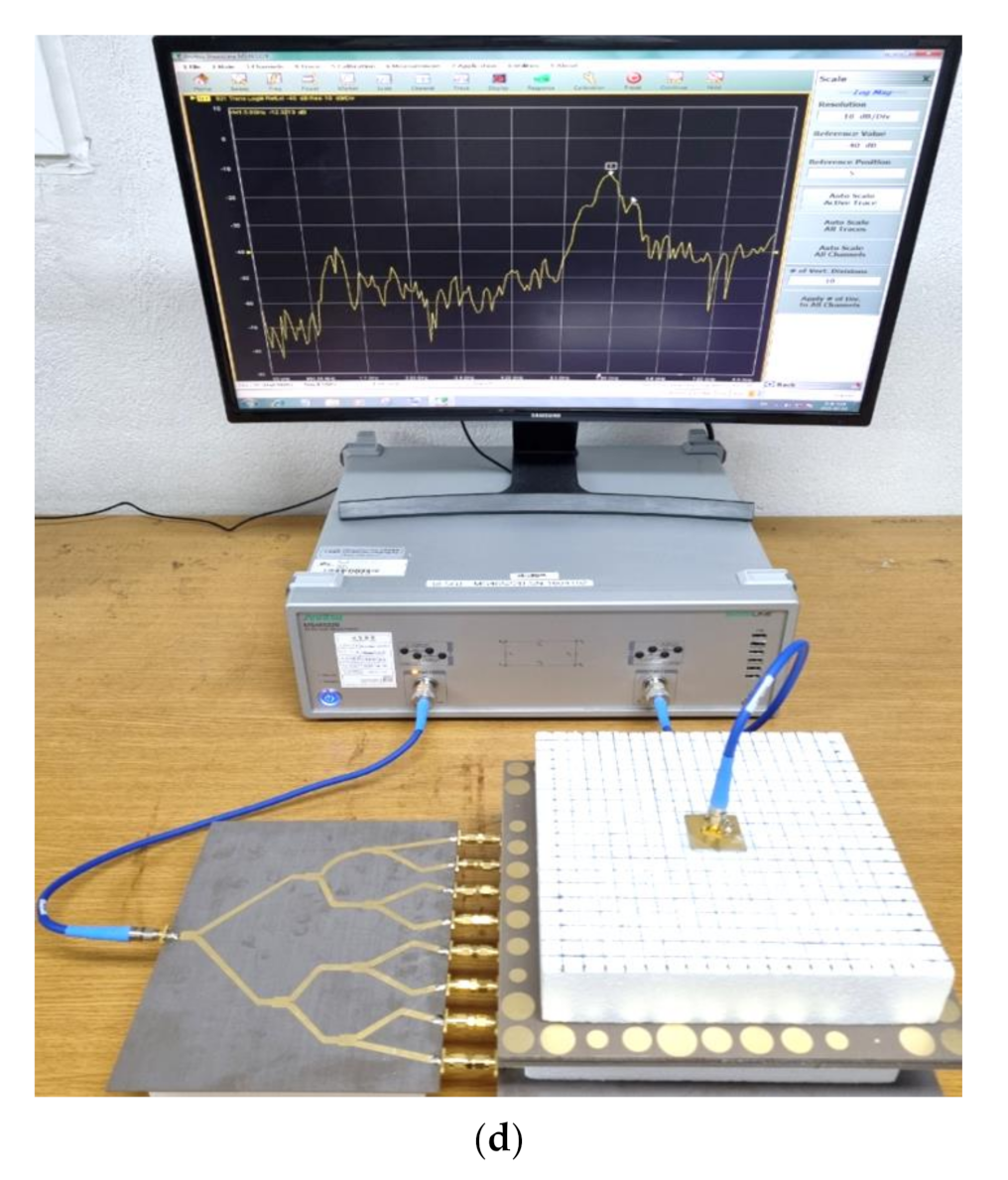
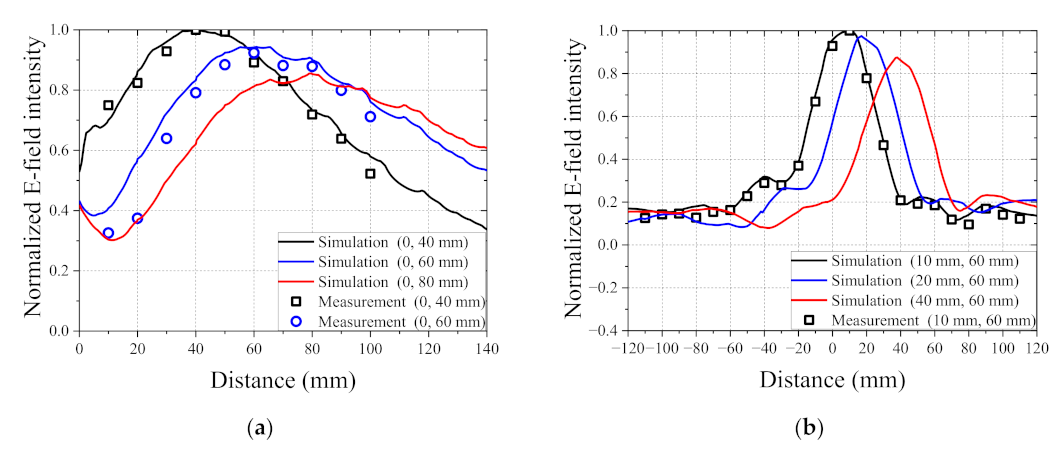
3. 2-D Beam Focusing Control
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lima, E.B.; Matos, S.A.; Costa, J.R.; Fernandes, C.A.; Fonseca, N.J.G. Circular polarization wide-angle beam steering at Ka-band by in-plane translation of a plate lens antenna. IEEE Trans. Antennas Propag. 2015, 63, 5443–5455. [Google Scholar] [CrossRef]
- Huang, C.; Pan, W.; Ma, X.; Zhao, B.; Cui, J.; Luo, X. Using reconfigurable transmitarray to achieve beam-steering and polarization manipulation applications. IEEE Trans. Antennas Propag. 2015, 63, 4801–4810. [Google Scholar] [CrossRef]
- Nicholls, J.G.; Hum, S.V. Full-space electronic beam-steering transmitarray with integrated leaky-wave feed. IEEE Trans. Antennas Propag. 2016, 64, 3410–3422. [Google Scholar] [CrossRef]
- Lee, J.G.; Kwon, T.S.; Lee, J.H. Beam pattern reconfigurable circularly polarized transmitarray antenna by rearrangement of sources. Microw. Opt. Technol. Lett. 2019, 61, 999–1003. [Google Scholar] [CrossRef]
- Palma, L.D.; Clemente, A.; Dussopt, L.; Sauleau, R.; Potier, P.; Pouliguen, P. Circularly-polarized reconfigurable transmitarray in Ka-band with beam scanning and polarization switching capabilities. IEEE Trans. Antennas Propag. 2017, 65, 529–540. [Google Scholar] [CrossRef]
- Pham, K.; Nguyen, N.T.; Clemente, A.; Palma, L.D.; Coq, L.L.; Dussopt, L.; Sauleau, R. Design of wideband dual linearly polarized transmitarray antennas. IEEE Trans. Antennas Propag. 2017, 65, 2022–2026. [Google Scholar] [CrossRef]
- Li, H.; Wang, G.; Liang, J.; Gao, X.; Hou, H.; Jia, X. Single-layer focusing gradient metasurface for ultrathin planar lens antenna application. IEEE Trans. Antennas Propag. 2017, 65, 1452–1457. [Google Scholar] [CrossRef]
- Wang, W.; Guo, Z.; Zhou, K.; Sun, Y.; Shen, F.; Li, Y.; Qu, S.; Liu, S. Polarization-independent longitudinal multi-focusing metalens. Opt. Express 2015, 23, 29855–29866. [Google Scholar] [CrossRef] [PubMed]
- An, W.; Xu, S.; Yang, F.; Li, M. A double-layer transmitarray antenna using malta crosses with vias. IEEE Trans. Antennas Propag. 2016, 64, 1120–1125. [Google Scholar] [CrossRef]
- Rahmati, B.; Hassani, H.R. High-efficient wideband slot transmitarray antenna. IEEE Trans. Antennas Propag. 2015, 63, 5149–5155. [Google Scholar] [CrossRef]
- Liu, G.; Wang, H.J.; Jiang, J.S.; Xue, F.; Yi, M. A high-efficiency transmitarray antenna using double split ring slot elements. IEEE Antennas Wirel. Propag. Lett. 2015, 14, 1415–1418. [Google Scholar] [CrossRef]
- Abdelrahman, A.H.; Elsherbeni, A.Z.; Yang, F. High-gain and broadband transmitarray antenna using triple-layer spiral dipole elements. IEEE Antennas Wirel. Propag. Lett. 2014, 13, 1288–1291. [Google Scholar] [CrossRef]
- Tuloti, S.H.R.; Rezaei, P.; Hamedani, F.T. High-efficient wideband transmitarray antenna. IEEE Antennas Wirel. Propag. Lett. 2018, 17, 817–820. [Google Scholar] [CrossRef]
- Song, C.; Zhu, L.; Pan, L. Design of transmitarray feed by filtering dielectric antenna for 5G. Int. J. RF Microw. CAE 2020, 30. [Google Scholar] [CrossRef]
- Diaby, F.; Clemente, R.; Sauleau, R.; Pham, K.T.; Dussopt, L. 2-bit reconfigurable unit-cell and electronically steerable transmitarray at Ka-band. IEEE Trans. Antennas Propag. 2020, 68, 5003–5008. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, S.; Yang, F.; Li, M. A novel 1 bit wide-angle beam scanning reconfigurable transmitarray antenna using an equivalent magnetic dipole element. IEEE Trans. Antennas Propag. 2020, 68, 5691–5695. [Google Scholar] [CrossRef]
- Wang, M.; Xu, S.; Yang, F.; Hu, N.; Xie, W.; Chen, Z. A novel 1-bit reconfigurable transmitarray antenna using a C-shaped probe-fed patch element with broadened bandwidth and enhanced efficiency. IEEE Access 2020, 8, 120124–120133. [Google Scholar] [CrossRef]
- Li, M.; Behdad, N. Wideband true-time-delay microwave lenses based on metallo-dielectric and all-dielectric lowpass frequency selective surfaces. IEEE Trans. Antennas Propag. 2013, 61, 4109–4119. [Google Scholar] [CrossRef]



| Focal Point (mm) | 1st Antenna Phase | 2nd Antenna Phase | 3rd Antenna Phase | 4th Antenna Phase | 5th Antenna Phase | 6th Antenna Phase | 7th Antenna Phase | 8th Antenna Phase |
|---|---|---|---|---|---|---|---|---|
| (0, 40) | 93.90° | 44.97° | 28.96° | 20.73° | 20.37° | 26.84° | 46.17° | 106.10° |
| (0, 60) | −26.10° | −16.25° | 6.92° | 18.28° | 17.93° | 4.80° | −15.05° | −13.90° |
| (0, 80) | −86.10° | −46.86° | −4.10° | 17.05° | 16.70° | −6.22° | −45.66° | −73.90° |
| (10, 60) | 29.54° | 24.32° | 32.42° | 28.71° | 13.29° | −14.91° | −49.83° | −63.75° |
| (20, 60) | 123.16° | 95.33° | 80.83° | 54.51° | 16.48° | −34.32° | −91.85° | −128.38° |
| (40, 60) | 292.70° | 227.20° | 175.02° | 112.02° | 35.32° | −53.16° | −148.37° | −222.57° |
| Focal Point (0, 60 mm) | 1st Antenna Phase | 2nd Antenna Phase | 3rd Antenna Phase | 4th Antenna Phase | 5th Antenna Phase | 6th Antenna Phase | 7th Antenna Phase | 8th Antenna Phase |
|---|---|---|---|---|---|---|---|---|
| w/o FSS | 240° | 122.45° | 44.08° | 4.90° | 4.90° | 44.08° | 122.45° | 240° |
| w/ FSS | −26.10° | −16.25° | 6.92° | 18.28° | 17.93° | 4.80° | −15.05° | −13.90° |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.-H.; Lee, J.-G. 2-D Beam Focusing Control Based on Passive Frequency Selective Surface (FSS). Electronics 2021, 10, 1938. https://doi.org/10.3390/electronics10161938
Park J-H, Lee J-G. 2-D Beam Focusing Control Based on Passive Frequency Selective Surface (FSS). Electronics. 2021; 10(16):1938. https://doi.org/10.3390/electronics10161938
Chicago/Turabian StylePark, Jeong-Hyun, and Jae-Gon Lee. 2021. "2-D Beam Focusing Control Based on Passive Frequency Selective Surface (FSS)" Electronics 10, no. 16: 1938. https://doi.org/10.3390/electronics10161938
APA StylePark, J.-H., & Lee, J.-G. (2021). 2-D Beam Focusing Control Based on Passive Frequency Selective Surface (FSS). Electronics, 10(16), 1938. https://doi.org/10.3390/electronics10161938






