A Case Study of the Retention Efficiency of a Traditional and Innovative Drainage System
Abstract
:1. Introduction
2. Materials and Methods
2.1. Case Study
2.2. Precipitation Model
2.3. Hydrodynamic Simulation—Storm Water Management Model
2.4. Innovative Drainage Systems (Retention Sewage Canal)
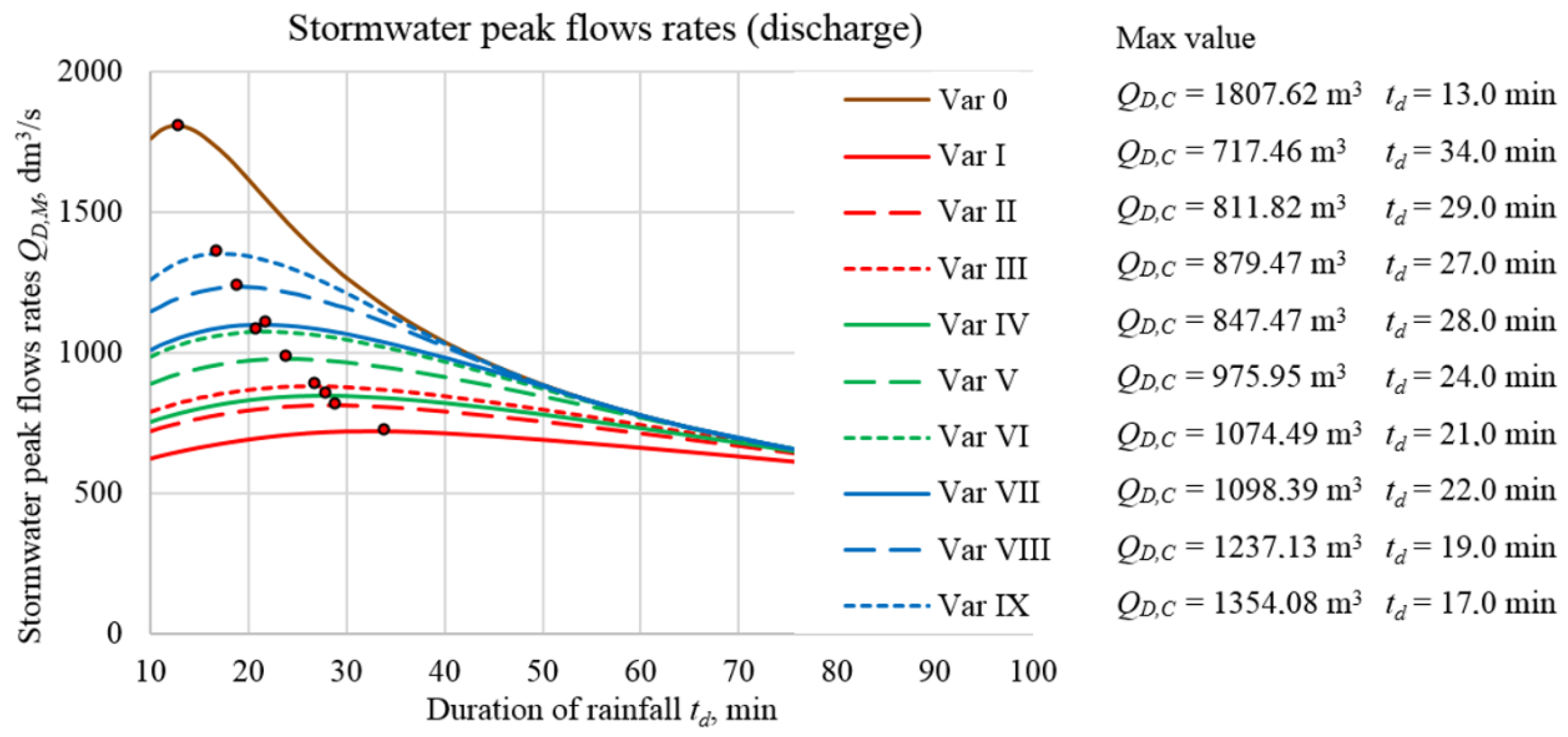
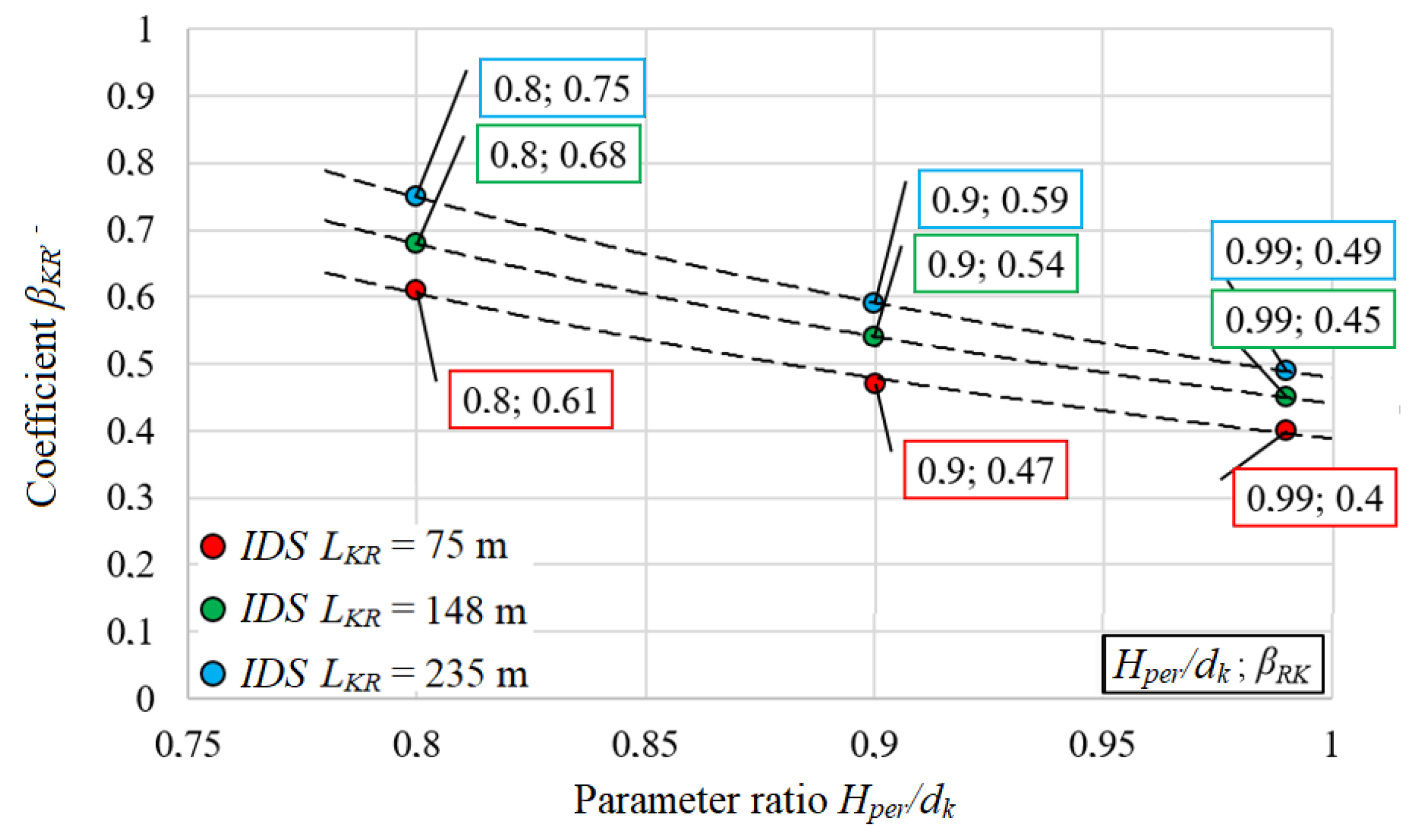
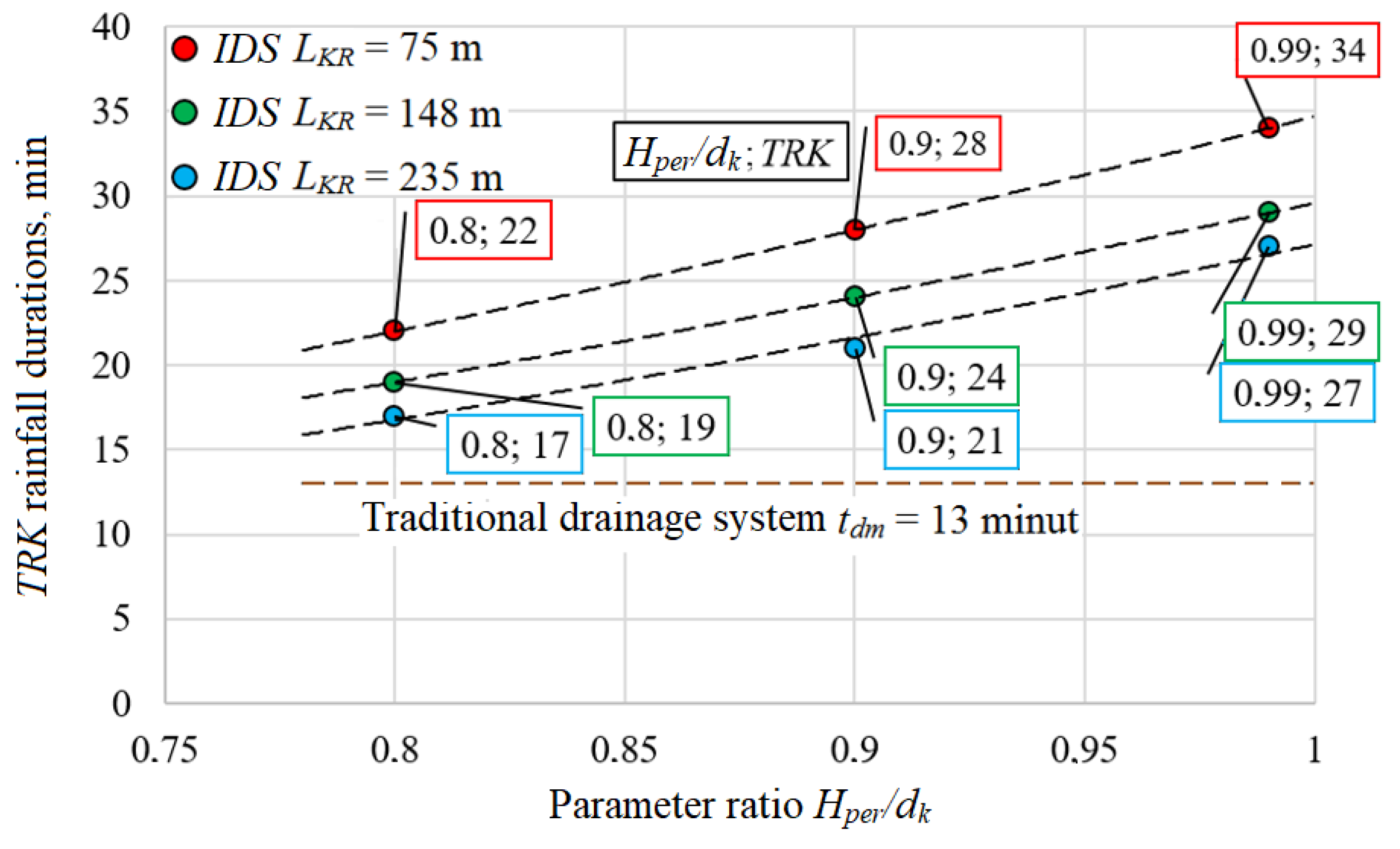
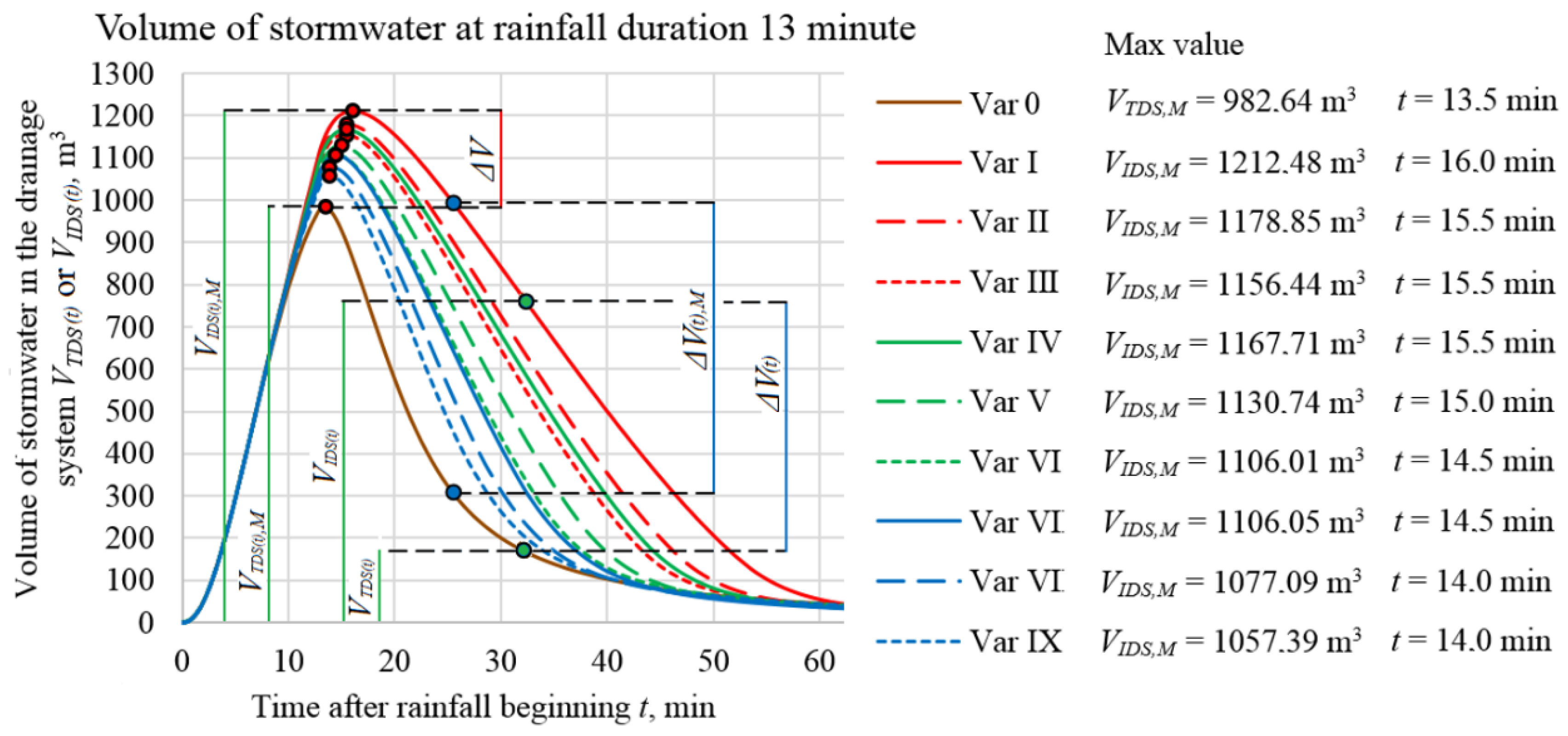
3. Results
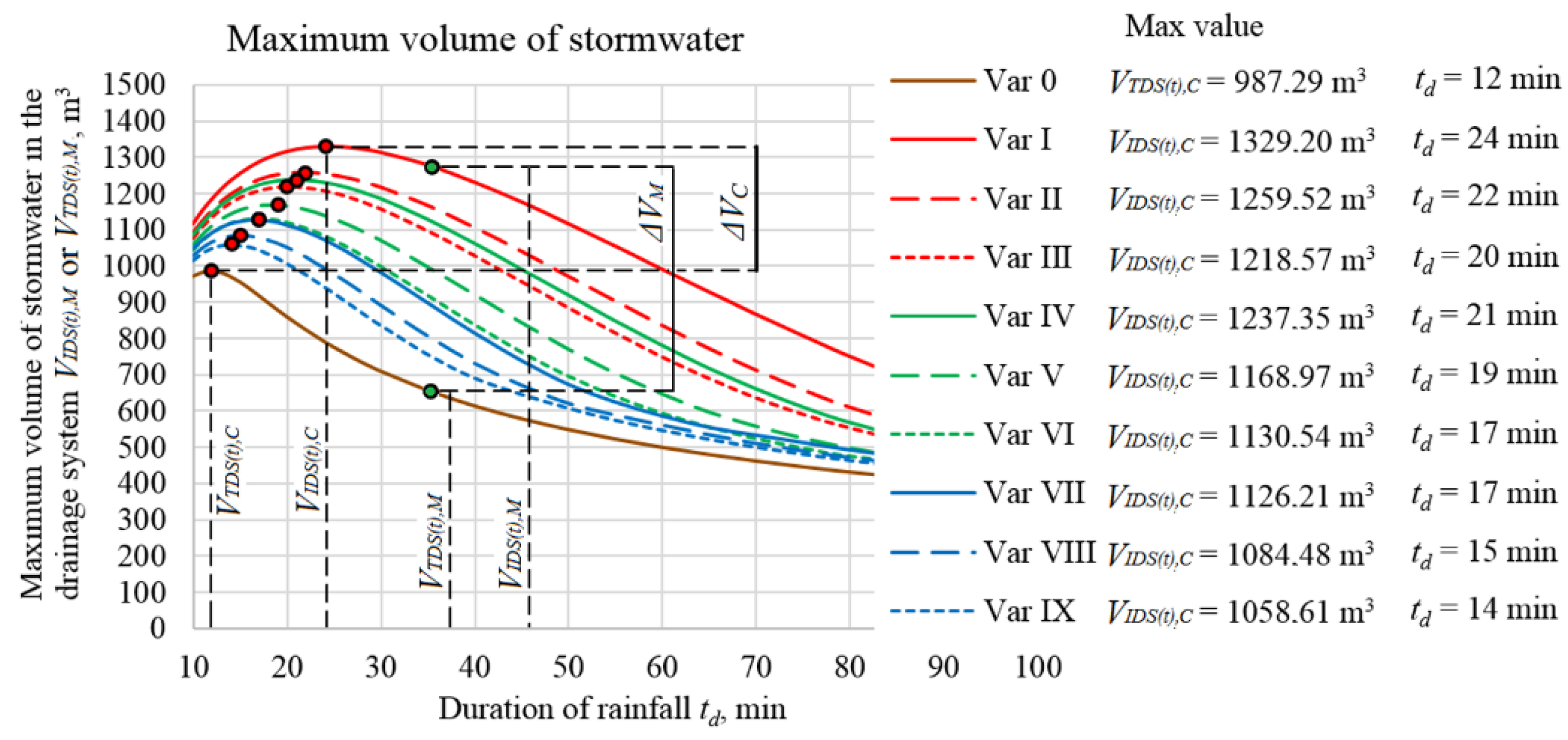
- the maximum value of the instantaneous variable volume of accumulated stormwater VTDS(t),M in the drainage system:VTDS(t),M = max(VTDS(t)) − a system that works traditionallyVIDS(t),M = max(VIDS(t)) − an innovative system
- the value of the instantaneous difference in the variable volume of accumulated stormwater ΔV(t) in a drainage system that works traditionally and innovatively:ΔV(t) = VIDS(t) − VTDS(t)
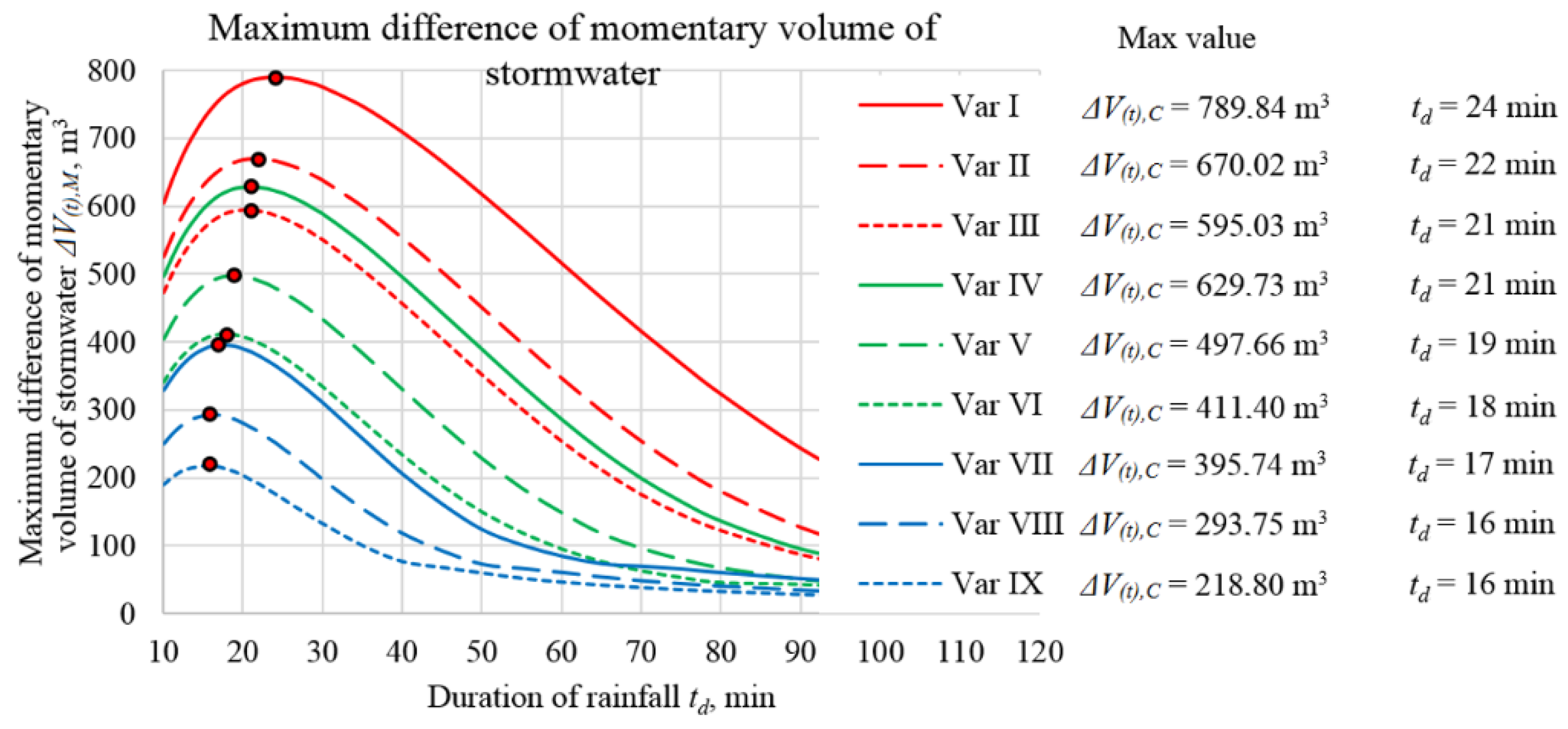
- the value of the maximum instantaneous difference in the variable volume of accumulated stormwater ΔV(t),M in the traditional and innovative drainage system:ΔV(t),M = max(ΔV(t))
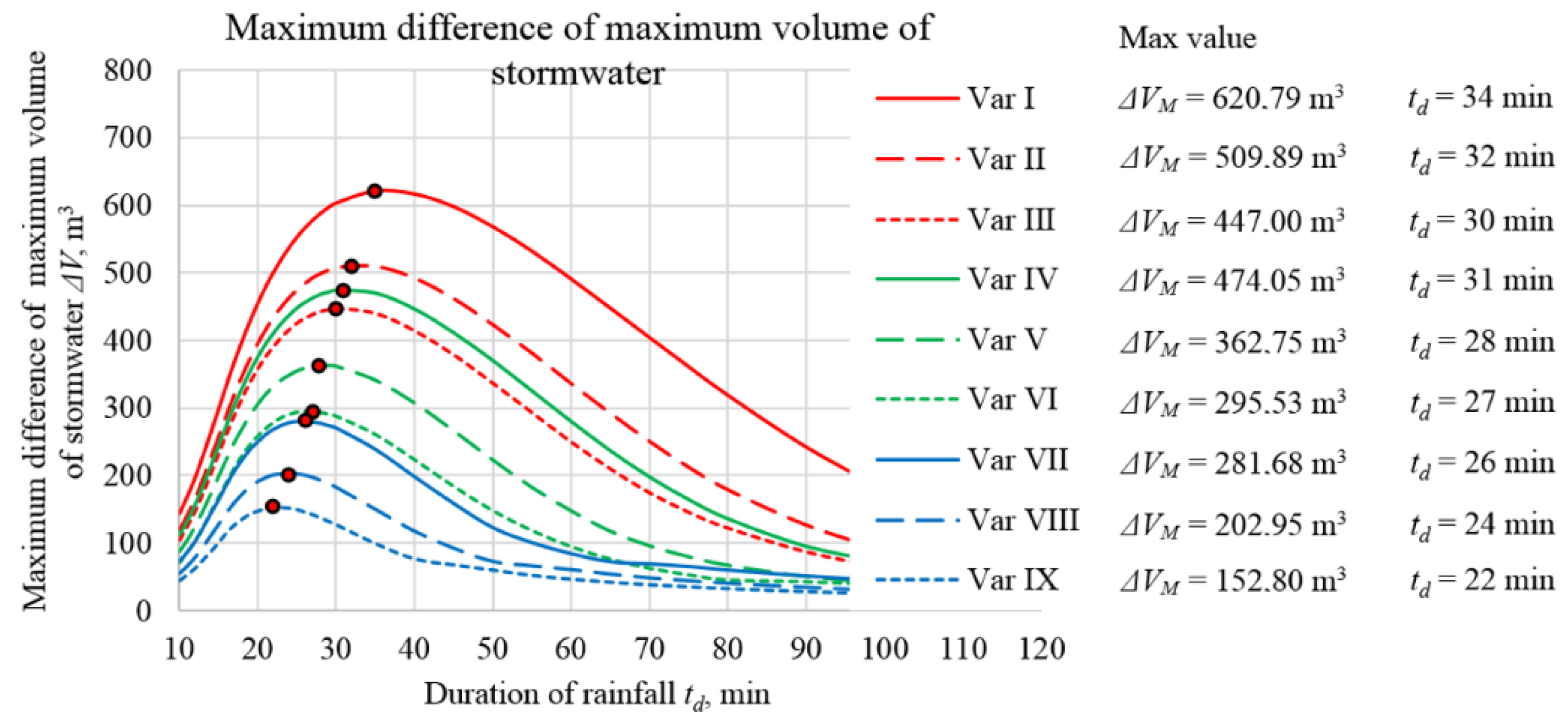
- the difference in the maximum stormwater volume ΔV accumulated in the drainage system functioning in a traditional and innovative way:ΔV = VIDS(t),M − VTDS(t),M
- Critical value of the instantaneous variable volume of accumulated stormwater in the drainage system:VTDS(t),C = max(VTDS(t),M) − traditionally functioning systemVIDS(t),C = max(VIDS(t),M) − an innovative system
- Maximum difference in the maximum volume of stormwater accumulated in a drainage system that operates in a traditional and innovative way:ΔVM = max(ΔV) = VIDS(t),M − VTDS(t),M
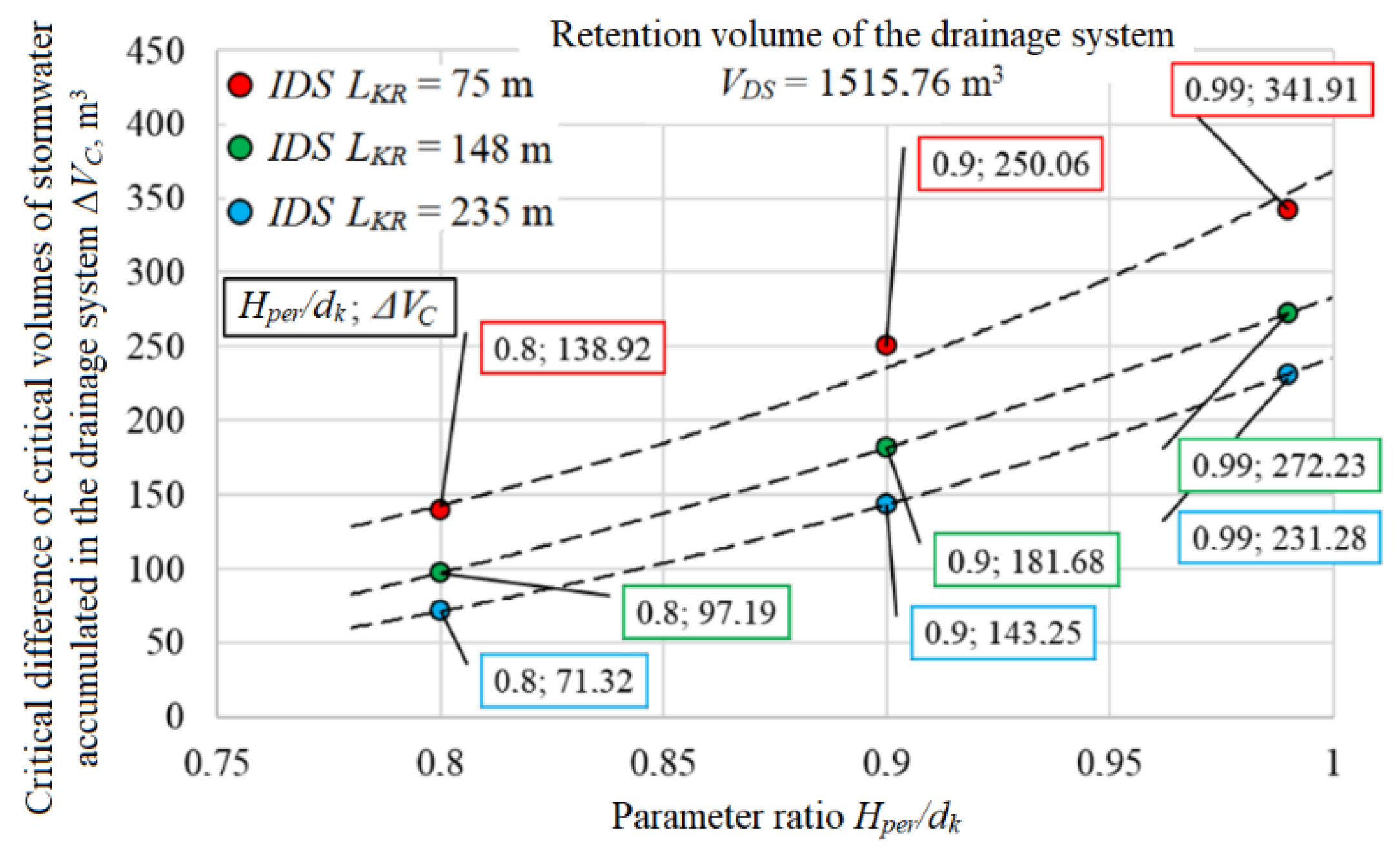
- Critical difference of critical volumes of stormwater accumulated in the drainage system functioning traditionally and innovatively:ΔVC = VIDS(t),C − VTDS(t),C
- Critical value of the maximum instantaneous difference in the variable volume of accumulated stormwater in a drainage system that operates in a traditional and innovative way:ΔV(t),C = max(ΔV(t),M)
4. Conclusions
- (1)
- Reducing the distance between damming partitions LKR (implementation of a greater number of damming partitions) reduces the peak runoff of stormwater from the drained catchment.
- (2)
- The level of stormwater accumulation Hper in canals affects the hydraulic functioning of the drainage system. Increasing the level of stormwater accumulation Hper reduces the peak flows in the drainage system.
- (3)
- A modern approach to the design of innovative drainage systems is a competitive alternative in terms of usability and economy in relation to fairly commonly used retention tanks or other cubature facilities.
- (4)
- The transformation of the traditional drainage system into an innovative drainage system requires only the implementation of damming partitions in the existing manholes.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Trenberth, K.E.; Fasullo, J.T.; Shepherd, T.G. Attribution of climate extreme events. Nat. Clim. Chang. 2015, 5, 725–730. [Google Scholar] [CrossRef]
- Żywiec, J.; Piegdoń, I.; Tchórzewska-Cieślak, B. Failure Analysis of the Water Supply Network in the Aspect of Climate Changes on the Example of the Central and Eastern Europe Region. Sustainability 2019, 11, 6886. [Google Scholar] [CrossRef] [Green Version]
- Shaw, T.A.; Baldwin, M.; Barnes, E.A.; Caballero, R.; Garfinkel, C.I.; Hwang, Y.-T.; Li, C.; O’Gorman, P.A.; Rivière, G.; Simpson, I.R.; et al. Attribution of climate extreme events. Nat. Geosci. 2016, 9, 656–664. [Google Scholar] [CrossRef]
- Yang, T.; Li, Q.; Chen, X.; De Maeyer, P.; Yan, X.; Liu, Y.; Zhao, T.; Li, L. Spatiotemporal variability of the precipitation concentration and diversity in Central Asia. Atmos. Res. 2020, 241, 104954. [Google Scholar] [CrossRef]
- Pei, F.; Wu, C.; Liu, X.; Hu, Z.; Xia, Y.; Liu, L.A.; Wang, K.; Zhou, Y.; Xu, L. Detection and attribution of extreme precipitation changes from 1961 to 2012 in the Yangtze River Delta in China. Catena 2018, 169, 183–194. [Google Scholar] [CrossRef]
- Wang, M.; Zhang, D.Q.; Adhityan, A.; Ng, W.J.; Dong, J.W.; Tan, S.K. Conventional and holistic urban stormwater management in coastal cities: A case study of the practice in Hong Kong and Singapore. Int. J. Water Resour. Dev. 2018, 34, 192–212. [Google Scholar] [CrossRef]
- Heinzlef, C.; Robert, B.; Hemond, Y.; Serre, D. Operating urban resilience strategies to face climate change and associated risks: Some advances from theory to application in Canada and France. Cities 2020, 104, 102762. [Google Scholar] [CrossRef]
- Moore, T.L.; Gulliver, J.S.; Stack, L.; Simpson, M.H. Stormwater management and climate change: Vulnerability and capacity for adaptation in urban and suburban contexts. Clim. Chang. 2016, 138, 491–504. [Google Scholar] [CrossRef]
- Wang, Z.; Zhou, S.; Wang, M.; Zhang, D. Cost-benefit analysis of low-impact development at hectare scale for urban stormwater source control in response to anticipated climatic change. J. Environ. Manag. 2020, 264, 110483. [Google Scholar] [CrossRef]
- Zeleňáková, M.; Hluštík, P.; Abd-Elhamid, H.F.; Vranayová, Z.; Markovič, G.; Hudáková, G.; Tometz, L. Comprehensive study of the percolation of water from surface runoff with an emphasis on the retention capacity and intensity of precipitation. Water Sci. Technol. 2019, 79, 2407–2416. [Google Scholar] [CrossRef]
- Zahmatkesh, Z.; Karamouz, M.; Goharian, E.; Burian, S.J. Analysis of the effects of climate change on urban storm water runoff using statistically downscaled precipitation data and a change factor approach. J. Hydrol. Eng. 2014, 20, 05014022. [Google Scholar] [CrossRef]
- Gordji, L.; Bonta, J.V.; Altinakar, M.S. Climate-Related Trends of Within-Storm Intensities Using Dimensionless Temporal-Storm Distributions. J. Hydrol. Eng. 2020, 25, 04020016. [Google Scholar] [CrossRef]
- Ishida, K.; Kavvas, M.L.; Chen, Z.R.; Dib, A.; Diaz, A.J.; Anderson, M.L.; Trinh, T. Physically based maximum precipitation estimation under future climate change conditions. Hydrol. Process. 2018, 32, 3188–3201. [Google Scholar] [CrossRef]
- Burszta-Adamiak, E.; Licznar, P.; Zaleski, J. Criteria for identifying maximum rainfall determined by the peaks-over-threshold (POT) method under the Polish Atlas of Rainfall Intensities (PANDa) project. Meteorol. Hydrol. Water Manag. Res. Oper. Appl. 2019, 7, 3–13. [Google Scholar] [CrossRef]
- Wartalska, K.; Kaźmierczak, B.; Nowakowska, M.; Kotowski, A. Analysis of Hyetographs for Drainage System Modeling. Water 2020, 12, 149. [Google Scholar] [CrossRef] [Green Version]
- Koszelnik, P.; Gruca-Rokosz, R.; Bartoszek, L. An isotopic model for the origin of autochthonous organic matter contained in the bottom sediments of a reservoir. Int. J. Sediment. Res. 2018, 33, 285–293. [Google Scholar] [CrossRef]
- Grillakis, M.G.; Koutroulis, A.G.; Tsanis, I.K. Improving Seasonal Forecasts for Basin Scale Hydrological Applications. Water 2018, 10, 1593. [Google Scholar] [CrossRef] [Green Version]
- Kim, I.H.; Han, K.Y. Inundation map prediction with rainfall return period and machine learning. Water 2020, 12, 1552. [Google Scholar] [CrossRef]
- Song, Y.; Park, M. A study on setting disaster-prevention rainfall by rainfall duration in urban areas considering natural disaster damage: Focusing on South Korea. Water 2020, 12, 642. [Google Scholar] [CrossRef] [Green Version]
- Cui, Y.; Liang, Q.; Wang, G.; Zhao, J.; Hu, J.; Wang, Y.; Xia, X. Simulation of hydraulic structures in 2d high-resolution urban flood modeling. Water 2019, 11, 2139. [Google Scholar] [CrossRef] [Green Version]
- Starzec, M.; Dziopak, J.; Słyś, D. Designing a retention sewage canal with consideration of the dynamic movement of precipitation over the selected urban catchment. In Underground Infrastructure of Urban Areas 4, 4th ed.; Madryas, C., Kolonko, A., Nienartowicz, B., Szot, A., Eds.; CRC Press: London, UK, 2017; Volume 1, pp. 193–200. ISBN 9781138559530. [Google Scholar]
- Dziopak, J. A wastewater retention canal as a sewage network and accumulation reservoir. E3S Web Conf. 2018, 45, 00016. [Google Scholar] [CrossRef] [Green Version]
- Słyś, D. An innovative retention canal—A case study. E3S Web Conf. 2018, 45, 00084. [Google Scholar] [CrossRef] [Green Version]
- Chan, F.K.S.; Griffiths, J.A.; Higgitt, D.; Xu, S.Y.; Zhu, F.F.; Tang, Y.T.; Xu, Y.Y.; Thorne, C.R. “Sponge City” in China-A breakthrough of planning and flood risk management in the urban context. Land Use Policy 2018, 76, 772–778. [Google Scholar] [CrossRef]
- Burns, M.J.; Fletcher, T.D.; Walsh, C.J.; Ladson, A.R.; Hatt, B.E. Hydrologic shortcomings of conventional urban stormwater management and opportunities for reform. Landsc. Urban Plan. 2012, 105, 230–240. [Google Scholar] [CrossRef]
- Dong, X.; Guo, H.; Zeng, S. Enhancing future resilience in urban drainage system: Green versus grey infrastructure. Water Res. 2017, 124, 280–289. [Google Scholar] [CrossRef]
- Kordana, S.; Słyś, D. An analysis of important issues impacting the development of stormwater management systems in Poland. Sci. Total Environ. 2020, 727, 138711. [Google Scholar] [CrossRef]
- Todeschini, S.; Papiri, S.; Ciaponi, C. Placement Strategies and Cumulative Effects of Wet-weather Control Practices for Intermunicipal Sewerage Systems. Water Resour. Manag. 2018, 32, 2885–2900. [Google Scholar] [CrossRef]
- Abd-Elhamid, H.F.; Zeleňáková, M.; Vranayová, Z.; Fathy, I. Evaluating the impact of urban growth on the design of storm water drainage systems. Water 2020, 12, 1572. [Google Scholar] [CrossRef]
- Piacentini, S.M.; Rossetto, R. Attitude and Actual Behaviour towards Water-Related Green Infrastructures and Sustainable Drainage Systems in Four North-Western Mediterranean Regions of Italy and France. Water 2020, 12, 1474. [Google Scholar] [CrossRef]
- Pochwat, K. The use of artificial neural networks for analyzing the sensitivity of a retention tank. E3S Web Conf. 2018, 45, 00066. [Google Scholar] [CrossRef] [Green Version]
- Stec, A.; Słyś, D. Effect of development of the town of Przemyśl on operation of its sewerage system. Ecol. Chem. Eng. S 2013, 20, 381–396. [Google Scholar] [CrossRef] [Green Version]
- Ngamalieu-Nengoue, U.A.; Martínez-Solano, F.J.; Iglesias-Rey, P.L.; Mora-Meliá, D. Multi-objective optimization for urban drainage or sewer networks rehabilitation through pipes substitution and storage tanks installation. Water 2019, 11, 935. [Google Scholar] [CrossRef] [Green Version]
- Pochwat, K.; Iličić, K. A simplified dimensioning method for high-efficiency retention tanks. E3S Web Conf. 2018, 45, 00065. [Google Scholar] [CrossRef]
- Adem Esmail, B.; Suleiman, L. Analyzing Evidence of Sustainable Urban Water Management Systems: A Review through the Lenses of Sociotechnical Transitions. Sustainability 2020, 12, 4481. [Google Scholar] [CrossRef]
- Li, J.; Alinaghian, S.; Joksimovic, D.; Chen, L. An Integrated Hydraulic and Hydrologic Modeling Approach for Roadside Bio-Retention Facilities. Water 2020, 12, 1248. [Google Scholar] [CrossRef]
- Liang, C.; Zhang, X.; Xia, J.; Xu, J.; She, D. The Effect of Sponge City Construction for Reducing Directly Connected Impervious Areas on Hydrological Responses at the Urban Catchment Scale. Water 2020, 12, 1163. [Google Scholar] [CrossRef] [Green Version]
- Słyś, D.; Stec, A. Centralized or Decentralized Stormwater Harvesting Systems: A Case Study. Resources 2020, 9, 5. [Google Scholar] [CrossRef] [Green Version]
- Wu, J.; Chen, Y.; Yang, R.; Zhao, Y. Exploring the Optimal Cost-Benefit Solution for a Low Impact Development Layout by Zoning, as Well as Considering the Inundation Duration and Inundation Depth. Sustainability 2020, 12, 4990. [Google Scholar] [CrossRef]
- Zdeb, M.; Zamorska, J.; Papciak, D.; Słyś, D. The Quality of Stormwater Collected from Roofs and the Possibility of Its Economic Use. Resources 2020, 9, 12. [Google Scholar] [CrossRef] [Green Version]
- Drosou, N.; Soetanto, R.; Hermawan, F.; Chmutina, K.; Bosher, L.; Hatmoko, J.U.D. Key Factors Influencing Wider Adoption of Blue–Green Infrastructure in Developing Cities. Water 2019, 11, 1234. [Google Scholar] [CrossRef] [Green Version]
- Fenner, R.; O’Donnell, E.; Ahilan, S.; Dawson, D.; Kapetas, L.; Krivtsov, V.; Ncube, S.; Vercruysse, K. Achieving Urban Flood Resilience in an Uncertain Future. Water 2019, 11, 1082. [Google Scholar] [CrossRef] [Green Version]
- Muthanna, T.M.; Sivertsen, E.; Kliewer, D.; Jotta, L. Coupling Field Observations and Geographical Information System (GIS)-Based Analysis for Improved Sustainable Urban Drainage Systems (SUDS) Performance. Sustainability 2018, 10, 4683. [Google Scholar] [CrossRef] [Green Version]
- Šijanec Zavrl, M.; Tanac Zeren, M. Sustainability of Urban Infrastructures. Sustainability 2010, 2, 2950–2964. [Google Scholar] [CrossRef] [Green Version]
- Thiagarajan, M.; Newman, G.; Zandt, S.V. The Projected Impact of a Neighborhood-Scaled Green-Infrastructure Retrofit. Sustainability 2018, 10, 3665. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zevenbergen, C.; Fu, D.; Pathirana, A. Transitioning to Sponge Cities: Challenges and Opportunities to Address Urban Water Problems in China. Water 2018, 10, 1230. [Google Scholar] [CrossRef] [Green Version]
- Kaykhosravi, S.; Khan, U.T.; Jadidi, A. A Comprehensive Review of Low Impact Development Models for Research, Conceptual, Preliminary and Detailed Design Applications. Water 2018, 10, 1541. [Google Scholar] [CrossRef] [Green Version]
- Wang, M.; Zhang, D.; Lou, S.; Hou, Q.; Liu, Y.; Cheng, Y.; Qi, J.; Tan, S.K. Assessing Hydrological Effects of Bioretention Cells for Urban Stormwater Runoff in Response to Climatic Changes. Water 2019, 11, 997. [Google Scholar] [CrossRef] [Green Version]
- LeFevre, G.H.; Paus, K.H.; Natarajan, P.; Gulliver, J.S.; Novak, P.J.; Hozalski, R.M. Review of dissolved pollutants in urban storm water and their removal and fate in bioretention cells. J. Environ. Eng. 2014, 141, 04014050. [Google Scholar] [CrossRef]
- Wan, Z.X.; Li, T.; Liu, Y.T. Effective nitrogen removal during different periods of a field-scale bioretention system. Environ. Sci. Pollut. Res. 2018, 25, 17855–17861. [Google Scholar] [CrossRef]
- Wang, M.; Zhang, D.; Dong, J.; Tan, S.K. Application of constructed wetlands for treating agricultural runoff and agro-industrial wastewater: A review. Hydrobiologia 2018, 805, 1–31. [Google Scholar] [CrossRef]
- Huang, C.L.; Hsu, N.S.; Liu, H.J.; Huang, Y.H. Optimization of low impact development layout designs for megacity flood mitigation. J. Hydrol. 2018, 564, 542–558. [Google Scholar] [CrossRef]
- Liu, Y.; Ahiablame, L.M.; Bralts, V.F.; Engel, B.A. Enhancing a rainfall-runoff model to assess the impacts of BMPs and LID practices on storm runoff. J. Environ. Manag. 2015, 147, 12–23. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Tan, M.; Wan, J.H.; Weise, T.; Wu, Z.Z. Effectiveness evaluation of the coupled LIDs from the watershed scale based on remote sensing image processing and SWMM simulation. Eur. J. Remote Sens. 2020. [Google Scholar] [CrossRef]
- Fletcher, T.D.; Shuster, W.; Hunt, W.F.; Ashley, R.; Butler, D.; Arthur, S.; Trowsdale, S.; Barraud, S.; Semadeni-Davies, A.; Bertrand-Krajewski, J.-L.; et al. SUDS, LID, BMPs, WSUD and more—The evolution and application of terminology surrounding urban drainage. Urban Water J. 2015, 12, 525–542. [Google Scholar] [CrossRef]
- Lafortezza, R.; Chen, J.; van den Bosch, C.K.; Randrup, T.B. Nature-based solutions for resilient landscapes and cities. Environ. Res. 2018, 165, 431–441. [Google Scholar] [CrossRef] [PubMed]
- Jalali, P.; Rabotyagov, S. Quantifying cumulative effectiveness of green stormwater infrastructure in improving water quality. Sci. Total Environ. 2020, 731, 138953. [Google Scholar] [CrossRef]
- Knapp, S.; Schmauck, S.; Zehnsdorf, A. Biodiversity Impact of Green Roofs and Constructed Wetlands as Progressive Eco-Technologies in Urban Areas. Sustainability 2019, 11, 5846. [Google Scholar] [CrossRef] [Green Version]
- Brown, C.; Lundholm, J. Microclimate and substrate depth influence green roof plant community dynamics. Landsc. Urban Plan. 2015, 143, 134–142. [Google Scholar] [CrossRef]
- Panno, A.; Carrus, G.; Lafortezza, R.; Mariani, L.; Sanesi, G. Nature-based solutions to promote human resilience and wellbeing in cities during increasingly hot summers. Environ. Res. 2017, 159, 249–256. [Google Scholar] [CrossRef]
- Frantzeskaki, N. Seven lessons for planning nature-based solutions in cities. Environ. Sci. Pol. 2019, 93, 101–111. [Google Scholar] [CrossRef]
- Kordana, S. The identification of key factors determining the sustainability of stormwater systems. E3S Web Conf. 2018, 45, 00033. [Google Scholar] [CrossRef] [Green Version]
- Pochwat, K.; Kida, M.; Ziembowicz, S.; Koszelnik, P. Odours in Sewerage-A Description of Emissions and of Technical Abatement Measures. Environments 2019, 6, 89. [Google Scholar] [CrossRef] [Green Version]
- Ziembowicz, S.; Kida, M.; Koszelnik, P. The impact of selected parameters on the formation of hydrogen peroxide by sonochemical process. Sep. Purif. Technol. 2018, 204, 149–153. [Google Scholar] [CrossRef]
- Donat, M.G.; Alexander, L.V.; Yang, H.; Durre, I.; Vose, R.; Dunn, R.J.H.; Willett, K.M.; Aguilar, E.; Brunet, M.; Caesar, J.; et al. Updated analyses of temperature and precipitation extreme indices since the beginning of the twentieth century: The HadEX2 dataset. J. Geophys. Res.-Atmos. 2013, 118, 2098–2118. [Google Scholar] [CrossRef]
- Passeport, E.; Vidon, P.; Forshay, K.J.; Harris, L.; Kaushal, S.S.; Kellogg, D.Q.; Lazar, J.; Mayer, P.; Stander, E.K. Ecological engineering practices for the eeduction of excess nitrogen in human-influenced landscapes: A guide for watershed managers. Environ. Manag. 2013, 51, 392–413. [Google Scholar] [CrossRef] [PubMed]
- Kordana, S.; Słyś, D. Decision Criteria for the Development of Stormwater Management Systems in Poland. Resources 2020, 9, 20. [Google Scholar] [CrossRef] [Green Version]
- Ivanovsky, A.; Belles, A.; Criquet, J.; Dumoulin, D.; Noble, P.; Alary, C.; Billon, G. Assessment of the treatment efficiency of an urban stormwater pond and its impact on the natural downstream watercourse. J. Environ. Manag. 2018, 226, 120–130. [Google Scholar] [CrossRef]
- Tchórzewska-Cieślak, B.; Papciak, D.; Koszelnik, P.; Kaleta, J.; Puszkarewicz, A.; Kida, M. Safety analysis of water supply to water treatment plant. In Environmental Engineering V, 1st ed.; Pawlowska, M., Pawlowski, M., Eds.; CRC Press: London, UK, 2017; Volume 1, pp. 1–6. ISBN 978-1-138-03163-0. [Google Scholar]
- Słyś, D.; Dziopak, J. Retention Sewage Canal; Patent Office of the Republic of Poland: Warsaw, Poland, 2014; p. 217405.
- Starzec, M.; Dziopak, J.; Słyś, D. An Analysis of Stormwater Management Variants in Urban Catchments. Resources 2020, 9, 19. [Google Scholar] [CrossRef] [Green Version]
- Rossman, L.A. Storm Water Management Model User’s Manual Version 5.1, United States Environmental Protection Agency. Available online: https://www.epa.gov/water-research/storm-water-management-model-swmm (accessed on 13 June 2020).
- Bogdanowicz, E.; Stachy, J. Maximum Rainfall in Poland. Design Characteristics, 1st ed.; The Publishing House of the Institute of Meteorology and Water Management: Warsaw, Poland, 1998; p. 83. [Google Scholar]
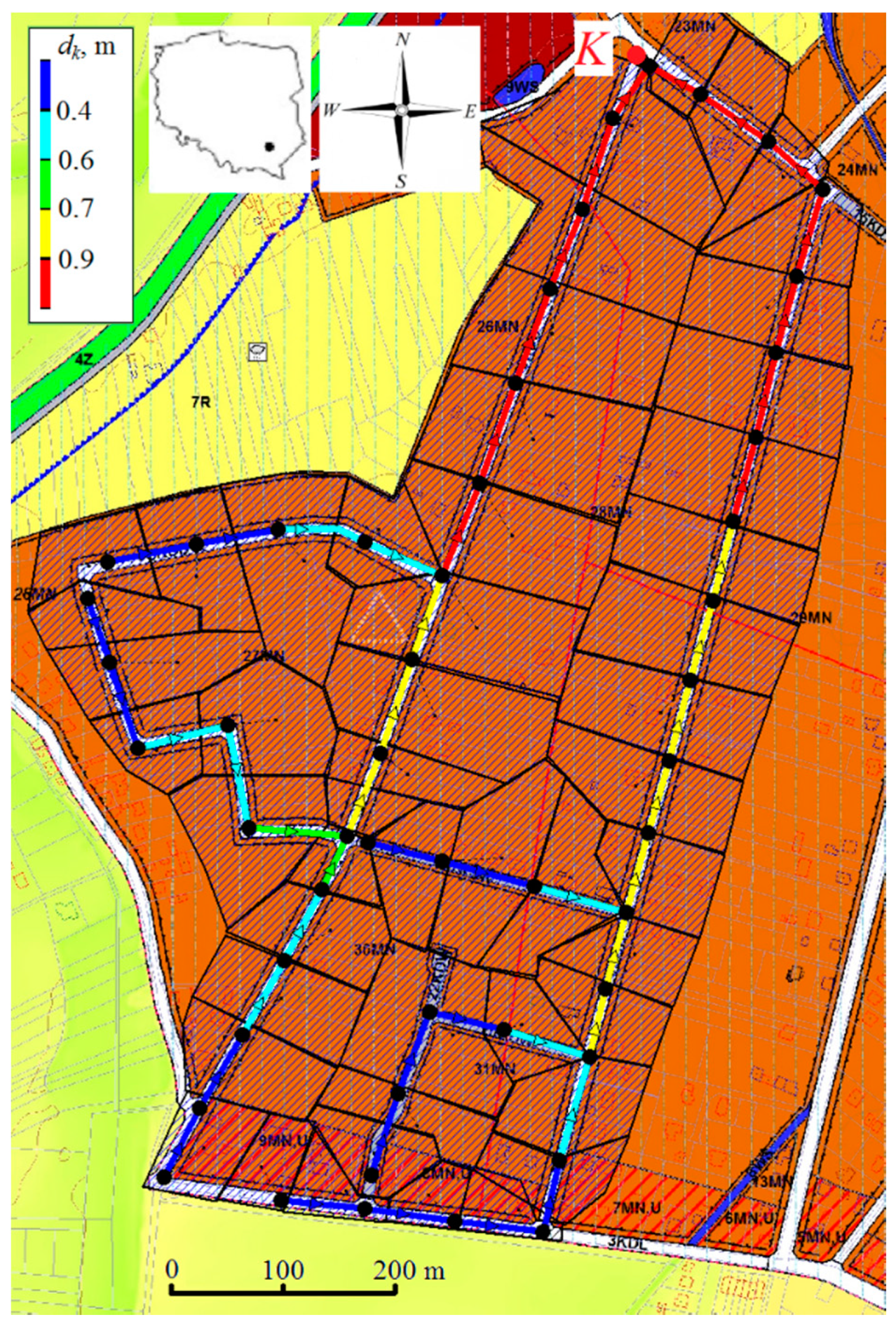
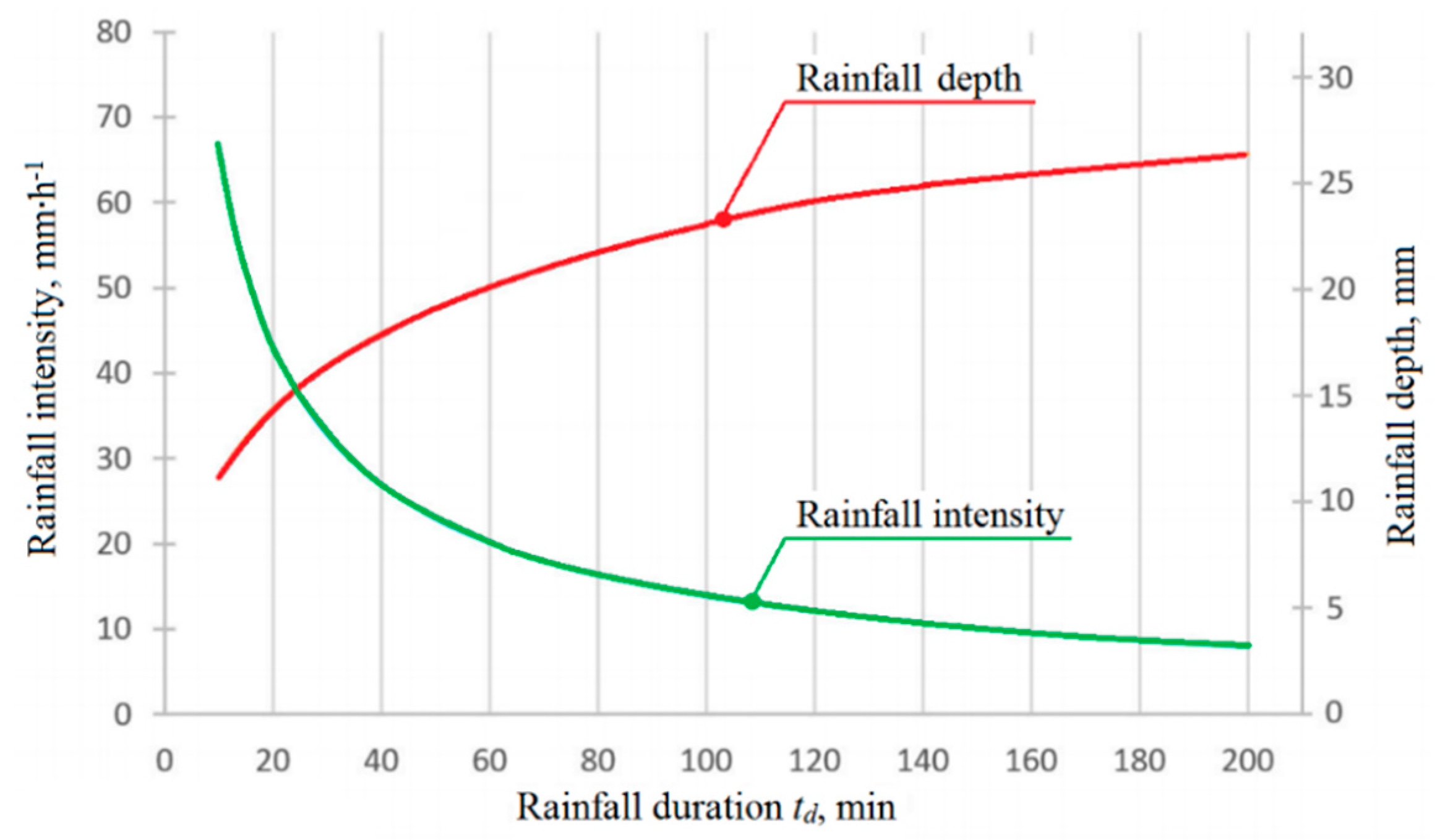

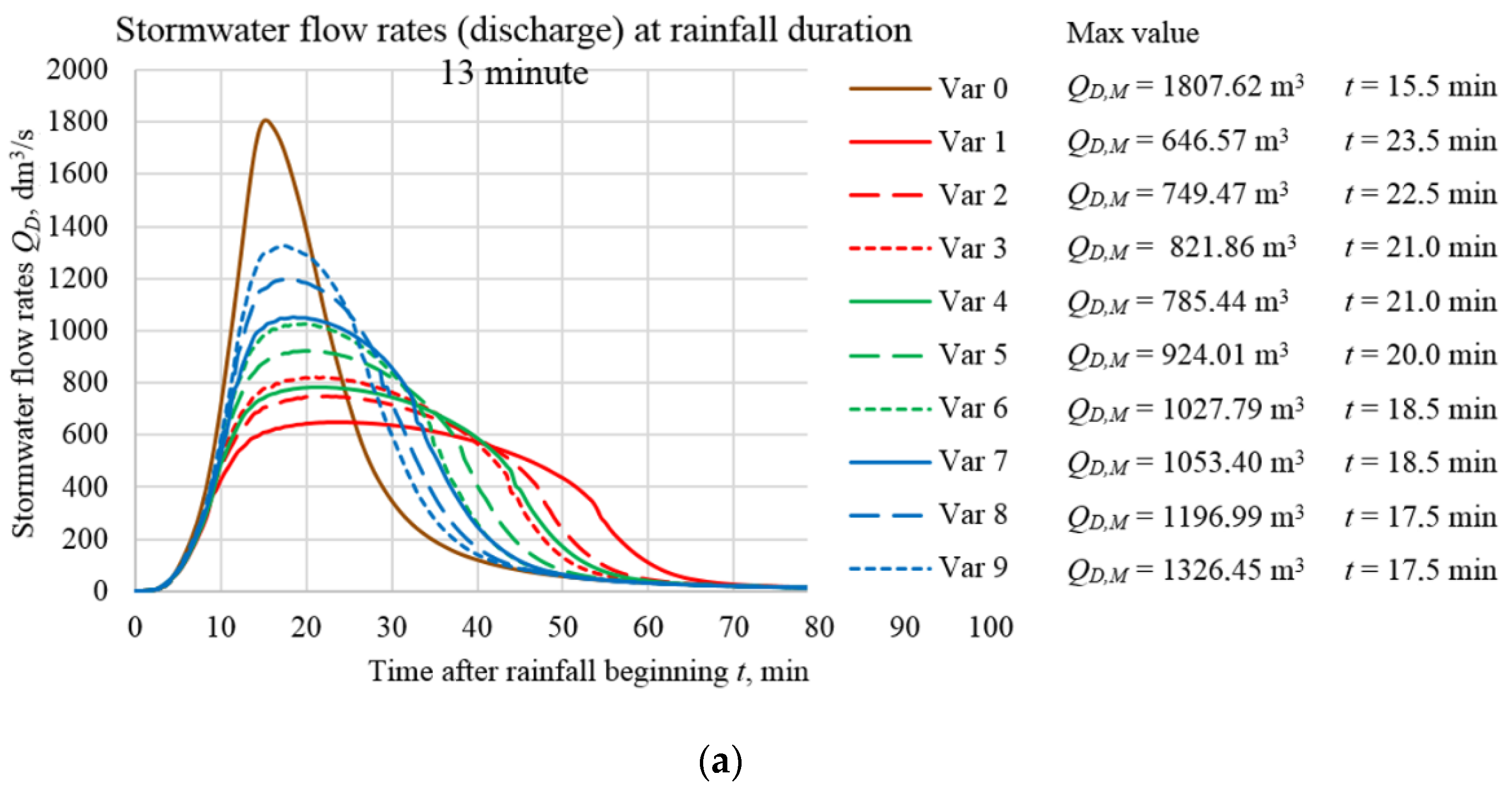
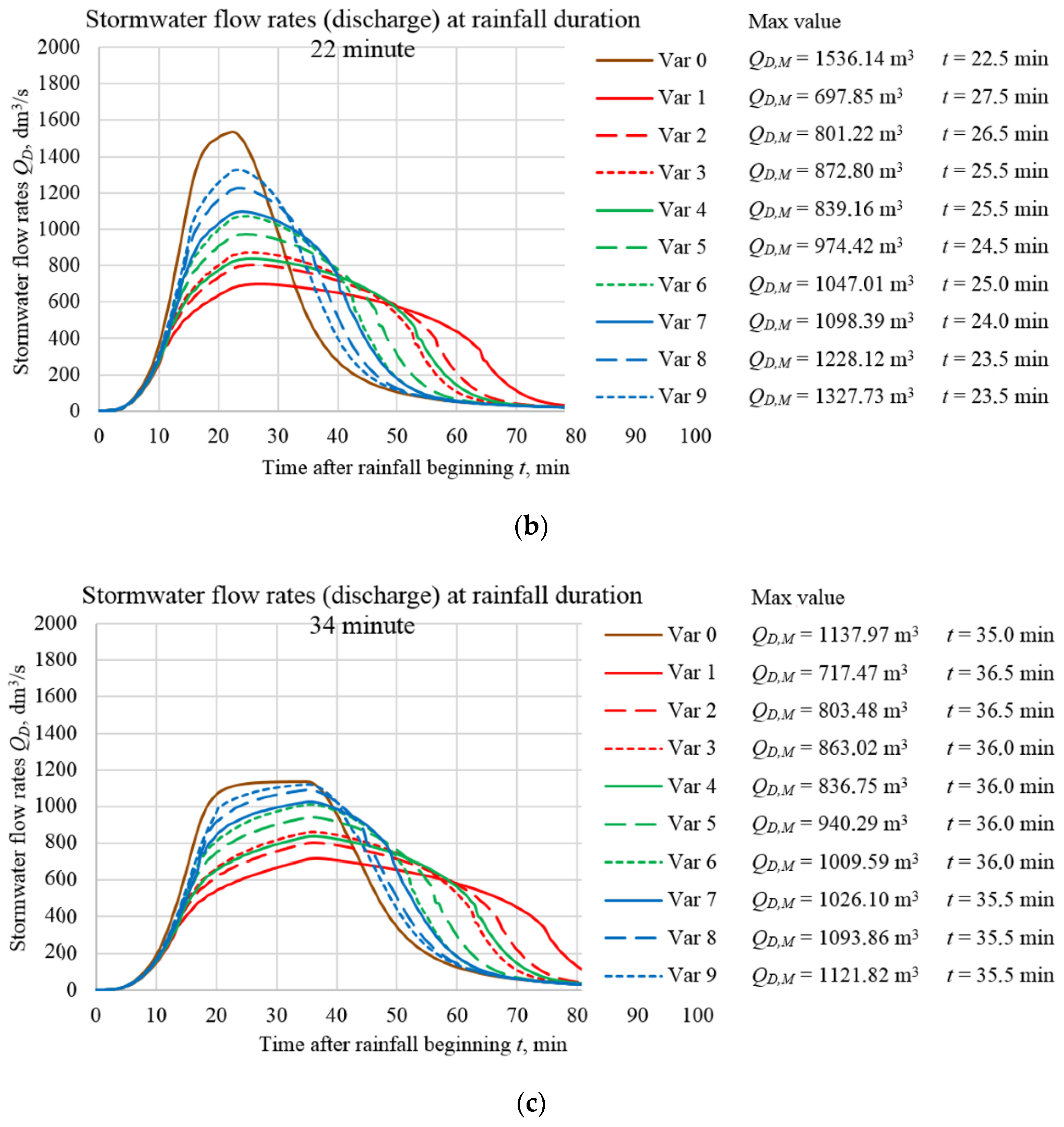

| Land Use | Area | Mannings n | Depth of Depression Storage on Area | |
|---|---|---|---|---|
| (ha) | (%) | (s/m1/3) | (mm) | |
| Rooftop | 4.78 | 10.30 | 0.011–0.012 | 0.3–0.5 |
| Road, pavement and other impervious | 9.60 | 20.70 | 0.011–0.013 | 0.8–1.4 |
| Green area | 32.00 | 69.00 | 0.15 | 3.4 |
| Total areas | 46.38 | 100.00 | - | - |
| Parameter | Value | Units |
|---|---|---|
| Maximum infiltration rate | 122.0 | (mm/h) |
| Minimum infiltration rate | 17.5 | (mm/h) |
| Infiltration rate decay constant | 3.5 | (1/h) |
| Drying Time | 6 | (days) |
| Parameter | Value | |
|---|---|---|
| Minimum | Maximum | |
| Length of links | 19.36 m | 97.40 m |
| Total length of links | 3769.70 m | |
| Slope of links Diameter of links | 1.1‰ 0.3 m | 3.1‰ 1.0 m |
| Drainage system capacity | 1515.76 m3 | |
| Variant | Average Distance between the Damming Partitions, LKR | Parameter Ratio Hper/dk |
|---|---|---|
| Variant 0 | - | - |
| Variant I | 75 m | 0.99 |
| Variant II | 148 m | 0.99 |
| Variant III | 235 m | 0.99 |
| Variant IV | 75 m | 0.90 |
| Variant V | 148 m | 0.90 |
| Variant VI | 235 m | 0.90 |
| Variant VII | 75 m | 0.80 |
| Variant VIII | 148 m | 0.80 |
| Variant IX | 235 m | 0.80 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Starzec, M.; Dziopak, J. A Case Study of the Retention Efficiency of a Traditional and Innovative Drainage System. Resources 2020, 9, 108. https://doi.org/10.3390/resources9090108
Starzec M, Dziopak J. A Case Study of the Retention Efficiency of a Traditional and Innovative Drainage System. Resources. 2020; 9(9):108. https://doi.org/10.3390/resources9090108
Chicago/Turabian StyleStarzec, Mariusz, and Józef Dziopak. 2020. "A Case Study of the Retention Efficiency of a Traditional and Innovative Drainage System" Resources 9, no. 9: 108. https://doi.org/10.3390/resources9090108
APA StyleStarzec, M., & Dziopak, J. (2020). A Case Study of the Retention Efficiency of a Traditional and Innovative Drainage System. Resources, 9(9), 108. https://doi.org/10.3390/resources9090108






