Performance Analysis of Slinky Horizontal Ground Heat Exchangers for a Ground Source Heat Pump System
Abstract
:1. Introduction
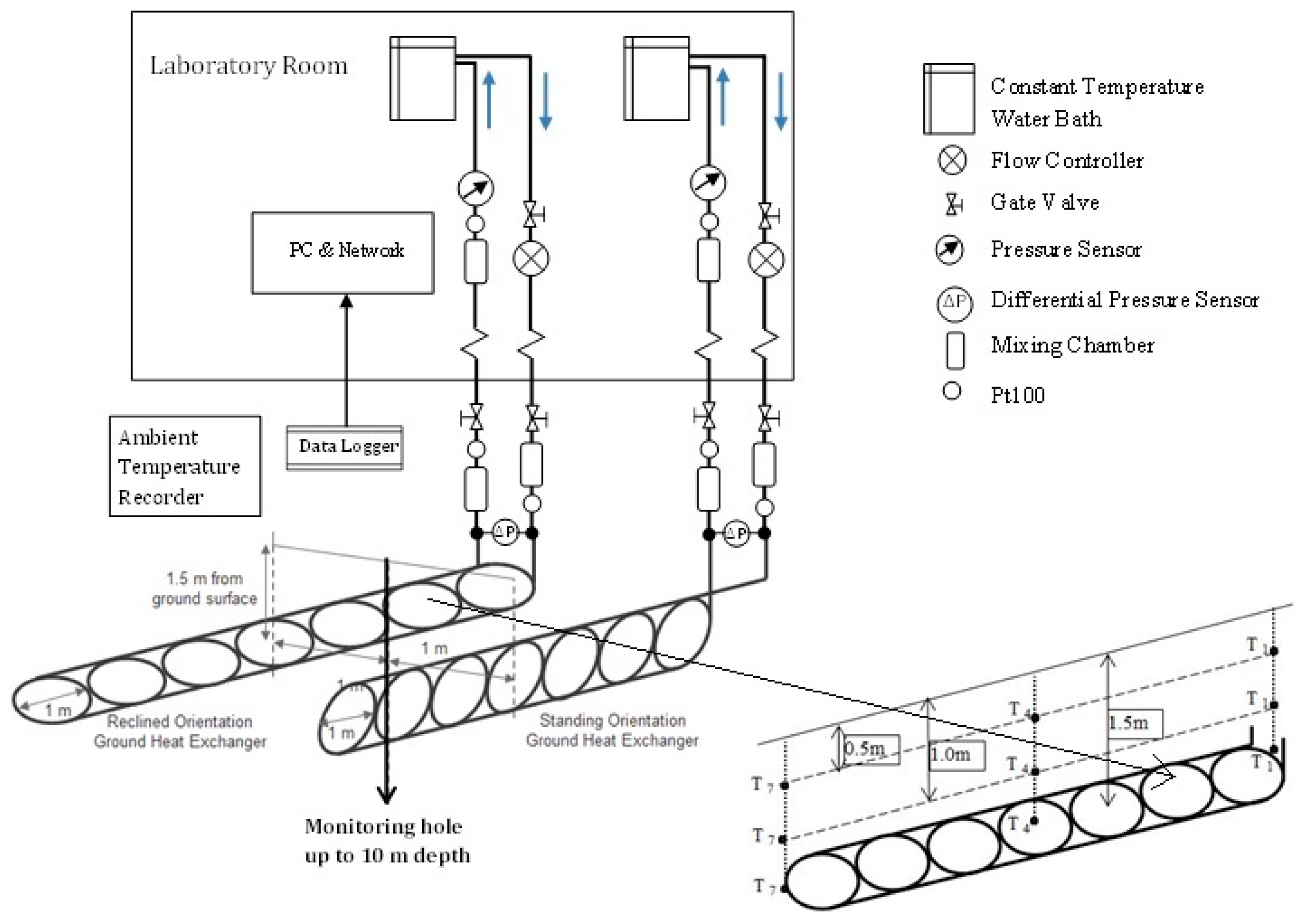
2. Description of the Experimental Set-Up
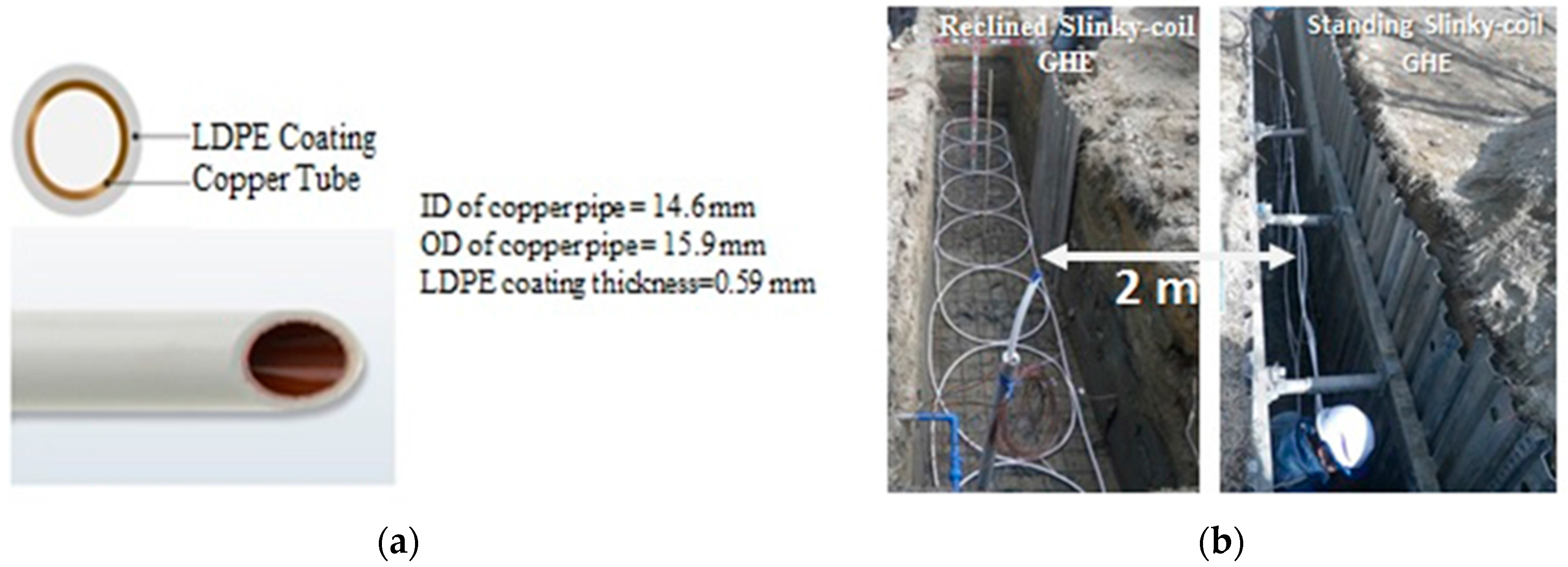
2.1. Material Selection and Ground Soil Characteristics
2.2. Details of Experimental Set-Up
2.3. Experimental Data Analysis
2.4. Uncertainty Analysis
3. Results and Discussion
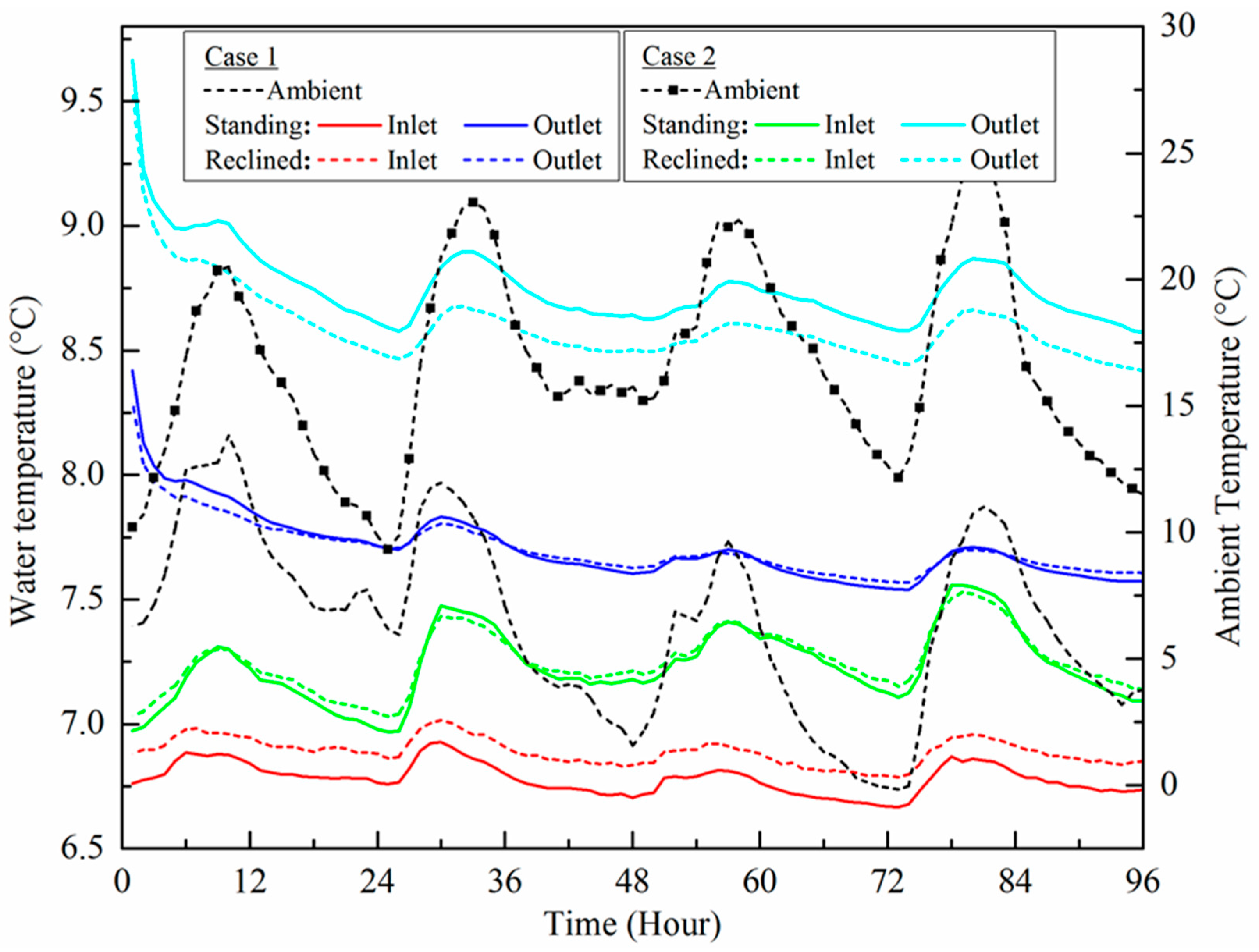
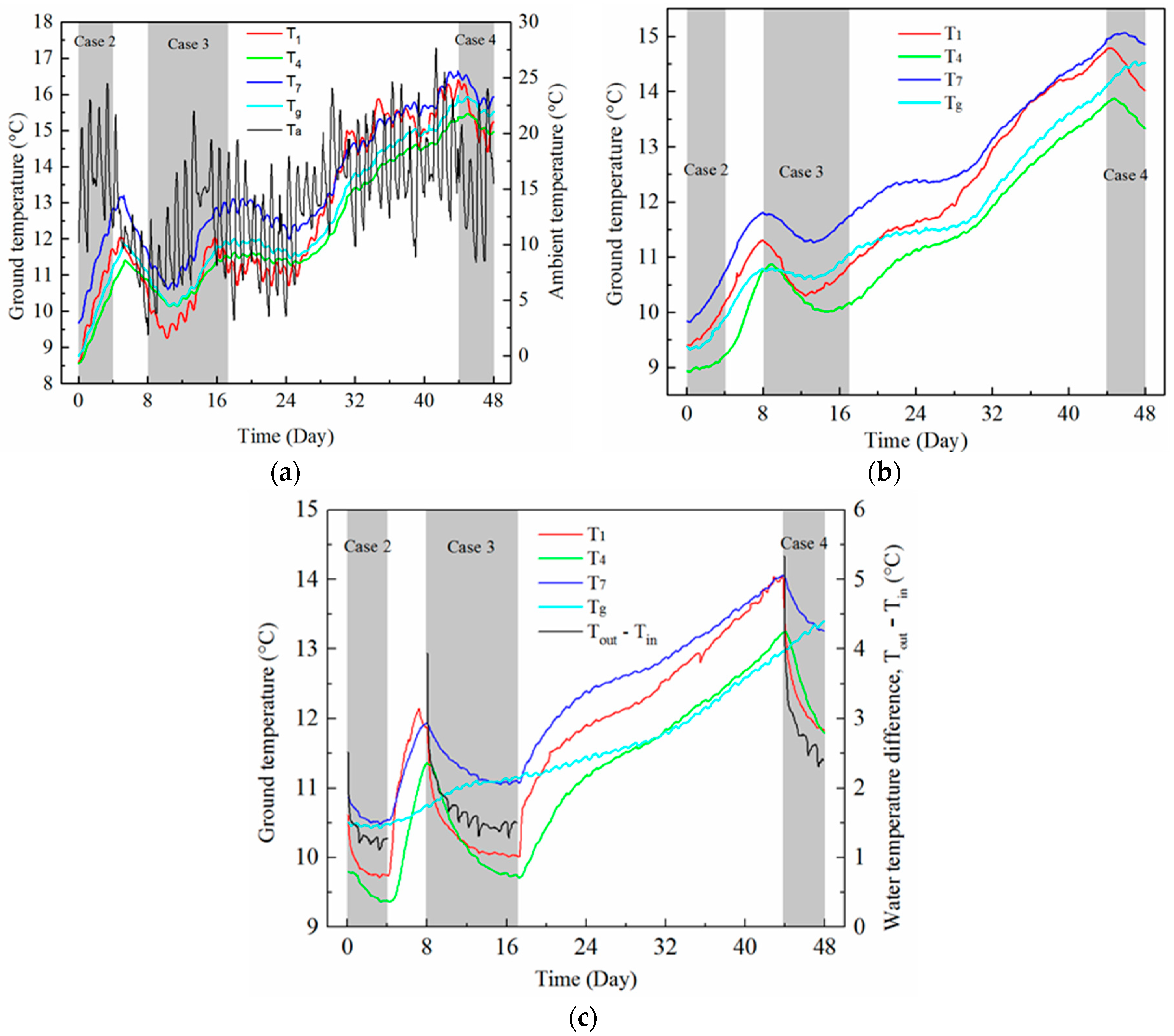
3.1. Daily Average Ground Thermal Behavior (Undisturbed Ground Temperature)
3.2. Comparison of Performance of Standing and Reclined Slinky Horizontal Heat Exchangers
3.2.1. Inlet and Outlet Temperatures of Circulating Water
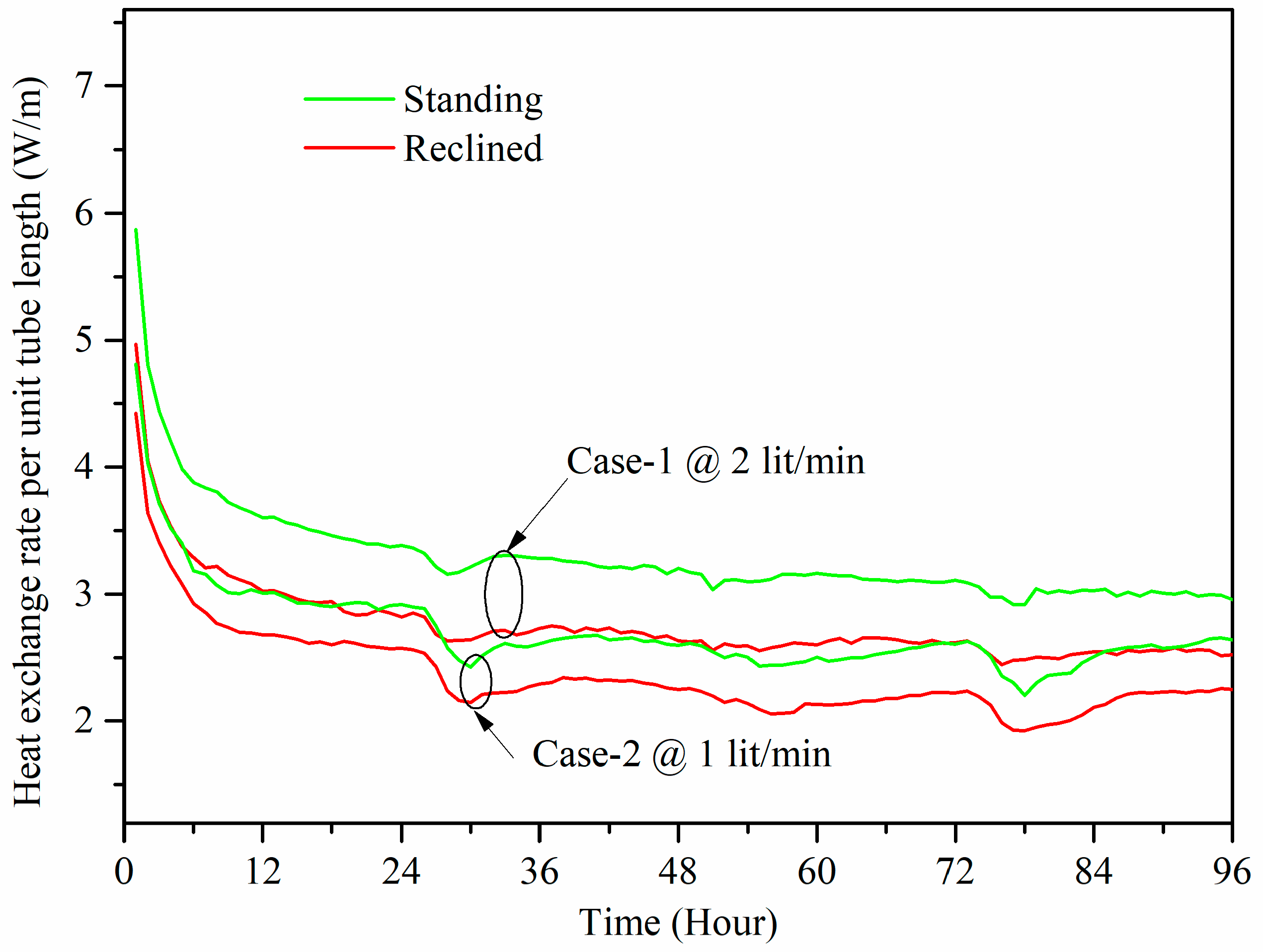
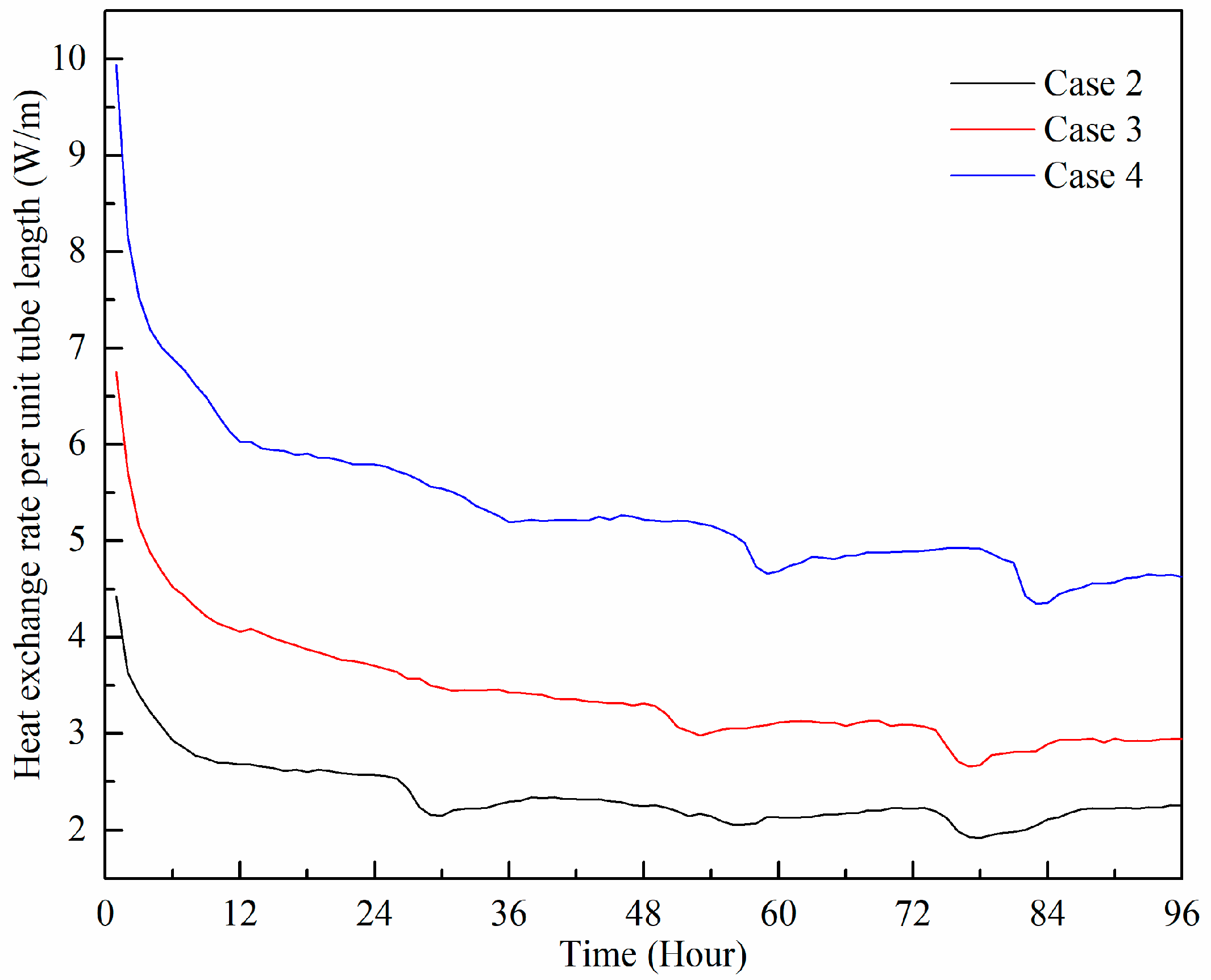
3.2.2. Heat Exchange Rate
3.3. Effect of Heat Extraction on Ground Temperature around GHE with the Reclined Slinky HGHE
3.4. Effect of Variation of Ground Temperature on Thermal Performance of the Reclined Slinky HGHE
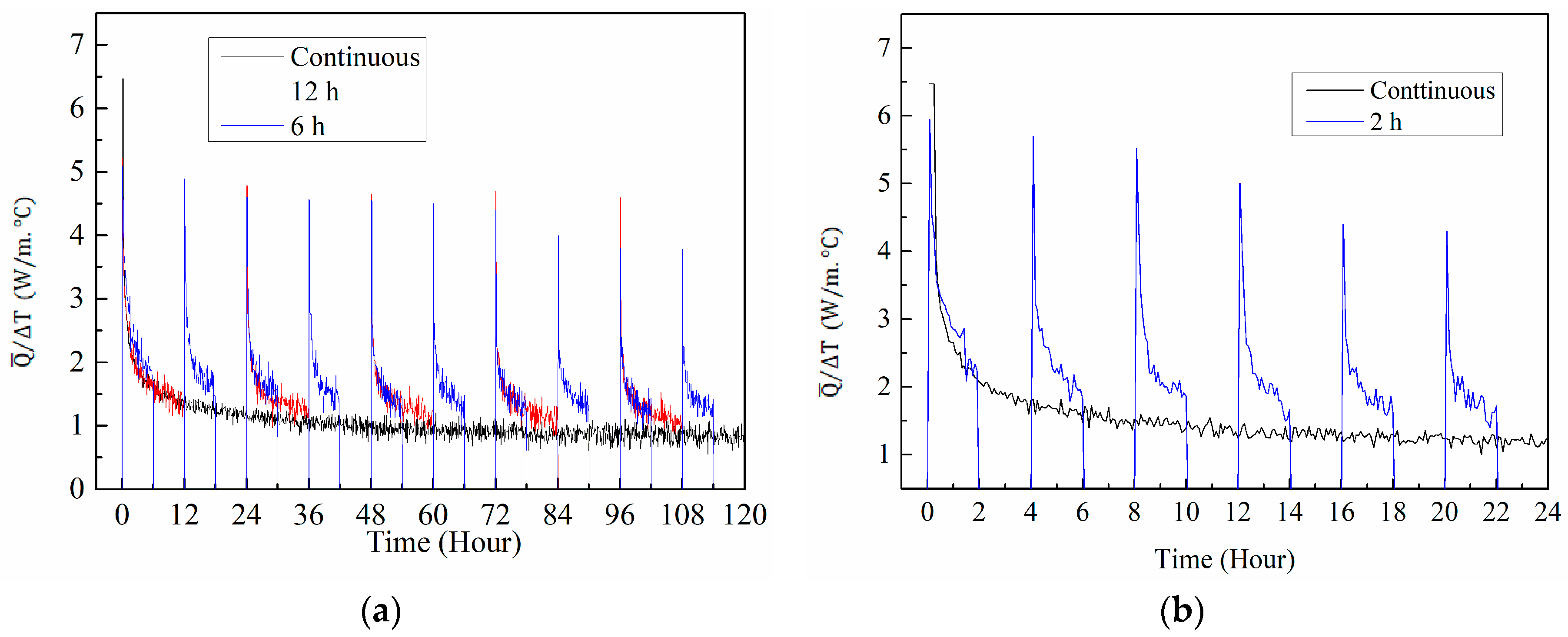
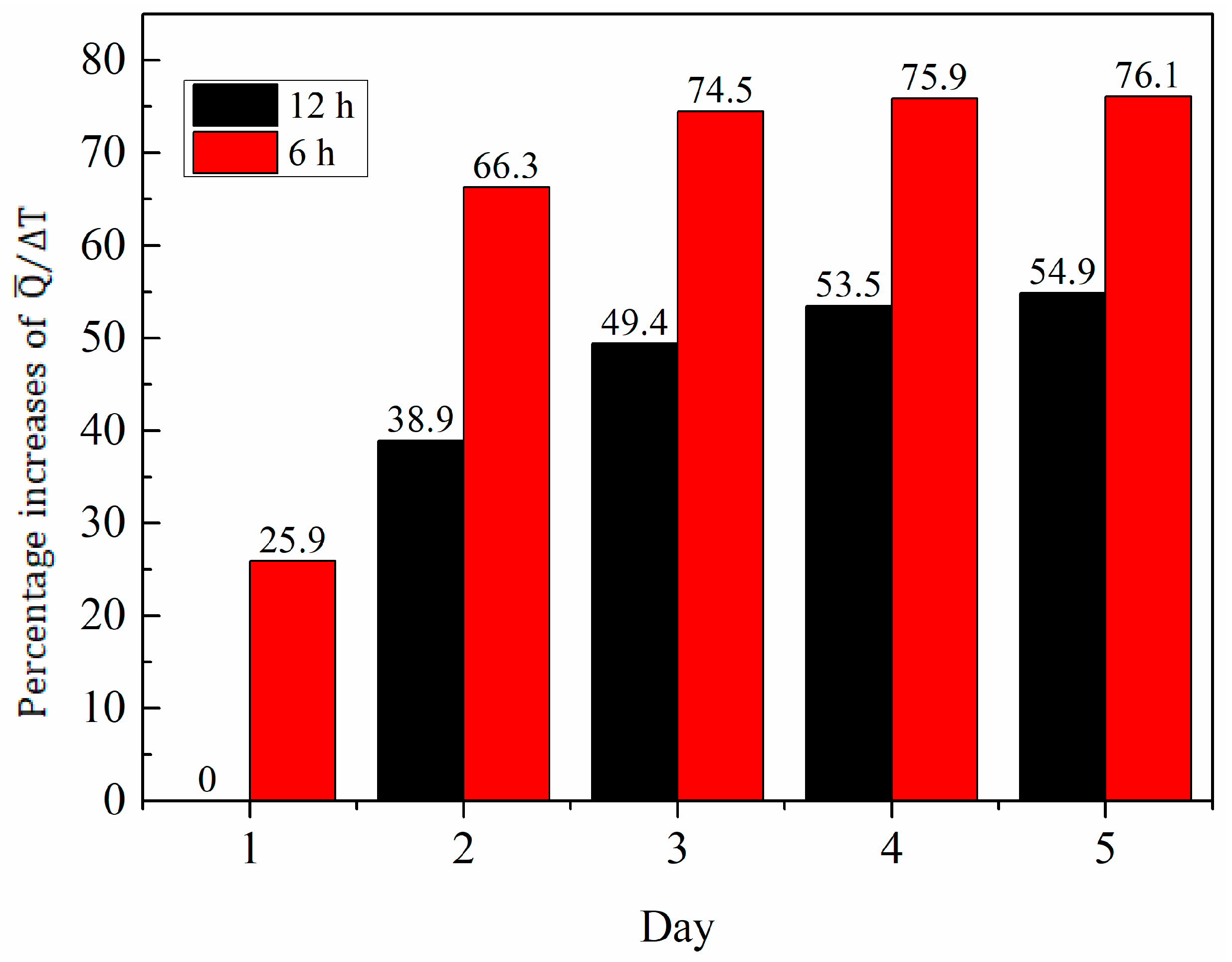
3.5. Intermittent Operation of Reclined HGHE
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Sarbu, I.; Sebarchievici, C. General review of ground-source heat pump systems for heating and cooling of buildings. Energy Build. 2014, 70, 441–454. [Google Scholar] [CrossRef]
- Kavanaugh, S.P.; Rafferty, K. Ground-Source Heat Pumps, Design of Geothermal Systems for Commercial and Institutional Buildings; ASHRAE: Atlanta, GA, USA, 1997; ISBN 1-883413-52-4. [Google Scholar]
- Florides, G.; Kalogirou, S. Ground heat exchangers-A review of systems, models and applications. Renew. Energy 2007, 32, 2461–2478. [Google Scholar] [CrossRef]
- Chiasson, A.D. Modeling horizontal ground heat exchangers in geothermal heat pump systems. In Proceedings of the COMSOL Conference, Boston, MA, USA, 7–9 October 2010. [Google Scholar]
- Adamovsky, D.; Neuberger, P.; Adamovsky, R. Changes in energy and temperature in the ground mass with horizontal heat exchangers-The energy source for heat pumps. Energy Build. 2015, 92, 107–115. [Google Scholar] [CrossRef]
- Selamat, S.; Miyara, A.; Kariya, K. Numerical study of horizontal ground heat exchangers for design optimization. Renew. Energy 2016, 95, 561–573. [Google Scholar] [CrossRef]
- Leong, W.H.; Tarnawski, V.R.; Aittomaki, A. Effect of soil type and moisture content on ground heat pump performance. Int. J. Refriger. 1998, 21, 595–606. [Google Scholar] [CrossRef]
- Naili, N.; Hazami, M.; Attar, I.; Farhat, A. In-field performance analysis of ground source cooling system with horizontal ground heat exchanger in Tunisia. Energy 2013, 61, 319–331. [Google Scholar] [CrossRef]
- Florides, G.; Theofanous, E.; Iosif-Stylianou, I.; Tassou, S.; Christodoulides, P.; Zomeni, Z. Modeling and assessment of the efficiency of horizontal and vertical ground heat exchangers. Energy 2013, 58, 655–663. [Google Scholar] [CrossRef]
- Song, Y.; Yao, Y.; Na, W. Impacts of soil pipe thermal Conductivity on performance of horizontal pipe in a ground-source heat pump. In Proceedings of the Sixth International Conference for Enhanced Building Operations, Shenzhen, China, 6–9 November 2006. [Google Scholar]
- Tarnawski, V.R.; Leong, W.H.; Momose, T.; Hamada, Y. Analysis of ground source heat pumps with horizontal ground heat exchangers for northern Japan. Renew. Energy 2009, 34, 127–134. [Google Scholar] [CrossRef]
- Congedo, P.M.; Colangelo, G.; Starace, G. CFD simulations of horizontal ground heat exchangers: A comparison among different configurations. Appl. Therm. Eng. 2012, 33–34, 24–32. [Google Scholar] [CrossRef]
- Wu, Y.; Gan, G.; Verhoef, A.; Vidale, P.L.; Gonzalez, R.G. Experimental measurement and numerical simulation of horizontal-coupled slinky ground source heat exchangers. Appl. Therm. Eng. 2010, 30, 2574–2583. [Google Scholar] [CrossRef]
- Xiong, Z.; Fisher, D.E.; Spitler, J.D. Development and validation of a slinky ground heat exchanger model. Appl. Energy 2015, 141, 57–69. [Google Scholar] [CrossRef]
- Wu, Y.; Gan, G.; Gonzalez, R.G.; Verhoef, A.; Vidale, P.L. Prediction of the thermal performance of horizontal-coupled ground source heat exchangers. Int. J. Low Carbon Technol. 2011, 6, 261–269. [Google Scholar] [CrossRef]
- Fujii, H.; Nishia, K.; Komaniwaa, Y.; Choub, N. Numerical modeling of slinky-coil horizontal ground heat exchangers. Geothermics 2012, 41, 55–62. [Google Scholar] [CrossRef]
- Demir, H.; Koyun, A.; Temir, G. Heat transfer of horizontal parallel pipe ground heat exchanger and experimental verification. Appl. Therm. Eng. 2009, 29, 224–233. [Google Scholar] [CrossRef]
- Selamat, S.; Miyara, A.; Kariya, K. Considerations for horizontal ground heat exchanger loops operation. Trans. JSRAE 2015, 32, 345–351. [Google Scholar]
- Chong, C.S.A.; Gan, G.; Verhoef, A.; Garcia, R.G.; Vidale, P.L. Simulation of thermal performance of horizontal slinky-loop heat exchangers for ground source heat pumps. Appl. Energy 2013, 104, 603–610. [Google Scholar] [CrossRef]
- Fujii, H.; Okubo, H.; Cho, N.; Ohyama, K. Field tests of horizontal ground heat exchangers. In Proceedings of the World Geothermal Congress, Bali, Indonesia, 25–29 April 2010. [Google Scholar]
- Esen, H.; Inalli, M.; Esen, M. Numerical and experimental analysis of a horizontal ground coupled heat pump system. Build. Environ. 2007, 42, 1126–1134. [Google Scholar] [CrossRef]
- Benazza, A.; Blanco, E.; Aichouba, M.; Río, J.L.; Laouedj, S. Numerical investigation of horizontal ground coupled heat exchanger. Energy Procedia 2011, 6, 29–35. [Google Scholar] [CrossRef]
- Jalaluddin; Miyara, A. Thermal performance investigation of several types of vertical ground heat exchangers with different operation mode. Appl. Therm. Eng. 2012, 33–34, 167–174. [Google Scholar] [CrossRef]
- Selamat, S.; Miyara, A.; Kariya, K. Analysis of short time period of operation of horizontal ground heat exchangers. Resources 2015, 4, 507–523. [Google Scholar] [CrossRef]
- Selamat, S.; Miyara, A.; Kariya, K. Comparison of heat exchange rates between straight and slinky horizontal ground heat exchanger. In Proceedings of the 24th IIR International Congress of Refrigeration, Yokohama, Japan, 16–22 August 2015. [Google Scholar]
- Hino, T.; Koslanant, S.; Onitsuka, K.; Negami, T. Changes in Properties of Holocene Series during Storage in Thin Wall Tube Samplers; Report of the Faculty of Science and Engineering; Saga University: Saga, Japan, 2007; Volume 36. [Google Scholar]
- Jsme Data Book: Heat Transfer, 5th ed.; The Japan Society of Mechanical Engineers: Saporo, Japan, 2009. (In Japanese)
- Holman, J.P. Experimental Methods for Engineers; McGraw-Hill Education: Singapore, 2012. [Google Scholar]
- Hamdhan, I.N.; Clarke, B.G. Determination of thermal conductivity of coarse and fine sand soils. In Proceedings of the World Geothermal Congress, Bali, Indonesia, 25–29 April 2010. [Google Scholar]
- Hillel, D. Environmental Soil Physics; Academic Press, Imprint of Elsevier: San Diego, CA, USA, 1998; ISBN 978-0-12-348525-0. [Google Scholar]


| Material | Inner Diameter (mm) | Outer Diameter (mm) | Density (kg/m3) | Specific Heat (J/(kg·K)) | Thermal Conductivity (W/(m·K)) |
|---|---|---|---|---|---|
| Copper (inner) | 14.6 | 15.9 | 8978 | 381 | 387.6 |
| LDPE (outer) | 15.9 | 17.08 | 920 | 3400 | 0.34 |
| Ground: clay [27] | - | - | 1700 | 1800 | 1.2 |
| Item | Name and Technical Specifications of the Measured Equipment | Uncertainty |
|---|---|---|
| The water temperatures at the inlet and outlet of standing and reclined GHEs | Pt100 Temperature range: −200 to 600 °C Sensor type: Class A, 4 wire | ±0.15 °C |
| Soil temperature in the ground | T-type thermocouple Temperature range: −200 to 200 °C | ±0.5 °C |
| Ambient air temperature | Lutron SD Card Data Logger Model: HT-3007SD Range: 0–50 °C Resolution: 0.1 °C | ±0.8 °C |
| Mass flow rate of water in standing and reclined GHE | TOFCO flow meter Model: FLC-605 Range: 0.5–5 L/min | ±5% |
| Heat exchange rate (W/m) | - | ±5.8% |
| Overall heat transfer coefficient, UA-value (W/°C) | - | ±5.5% |
| Case | Operation Period | Inlet Water Temperature (°C) | Flow Rate (L/min) | Average Initial Ground Temperature around GHE at 1.5 m Depth (°C) | Reynolds Number |
|---|---|---|---|---|---|
| 1 | 23–26 February 2016 | 7 | 2 | 10.4 | 3268 |
| 2 | 4–7 March 2016 | 7 | 1 | 10.4 | 1634 |
| 3 | 12–21 March 2016 | 7 | 1 | 11.3 | 1634 |
| 4 | 17–20 April 2016 | 7 | 1 | 13.7 | 1634 |
| Operation | Flow Rate (L/min) | Average Heat Exchange Rate (W/m) | Average UA-Value (W/°C) | ||
|---|---|---|---|---|---|
| Standing | Reclined | Standing | Reclined | ||
| Case 1 | 2 | 3.17 | 2.66 | 42.66 | 35.58 |
| Case 2 | 1 | 2.61 | 2.25 | 36.12 | 30.75 |
| Cycle Operation Time | Operation | Period of Integral | S (W·h/(m·°C)) |
|---|---|---|---|
| 120 h | Continuous | Over the whole period | |
| Over every 6 h interval | |||
| Over every 12 h interval | |||
| Intermittent | 6 h interval | ||
| 12 h interval | |||
| 24 h | Continuous | Over the whole period | |
| Over every 2 h interval | |||
| Intermittent | 2 h interval |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, M.H.; Kariya, K.; Miyara, A. Performance Analysis of Slinky Horizontal Ground Heat Exchangers for a Ground Source Heat Pump System. Resources 2017, 6, 56. https://doi.org/10.3390/resources6040056
Ali MH, Kariya K, Miyara A. Performance Analysis of Slinky Horizontal Ground Heat Exchangers for a Ground Source Heat Pump System. Resources. 2017; 6(4):56. https://doi.org/10.3390/resources6040056
Chicago/Turabian StyleAli, Md. Hasan, Keishi Kariya, and Akio Miyara. 2017. "Performance Analysis of Slinky Horizontal Ground Heat Exchangers for a Ground Source Heat Pump System" Resources 6, no. 4: 56. https://doi.org/10.3390/resources6040056
APA StyleAli, M. H., Kariya, K., & Miyara, A. (2017). Performance Analysis of Slinky Horizontal Ground Heat Exchangers for a Ground Source Heat Pump System. Resources, 6(4), 56. https://doi.org/10.3390/resources6040056





