Comparison of Empirical Models to Predict Viscosity of Secondary Vacuum Gas Oils
Abstract
:1. Introduction
2. Materials and Methods
- Density, g/cm3 ASTM D4052;
- High temperature simulation distillation (HTSD) Ŵ ASTM D7169;
- Engler specific viscosity ASTM D1665;
- Hydrocarbon composition ASTM D2549.
3. Results
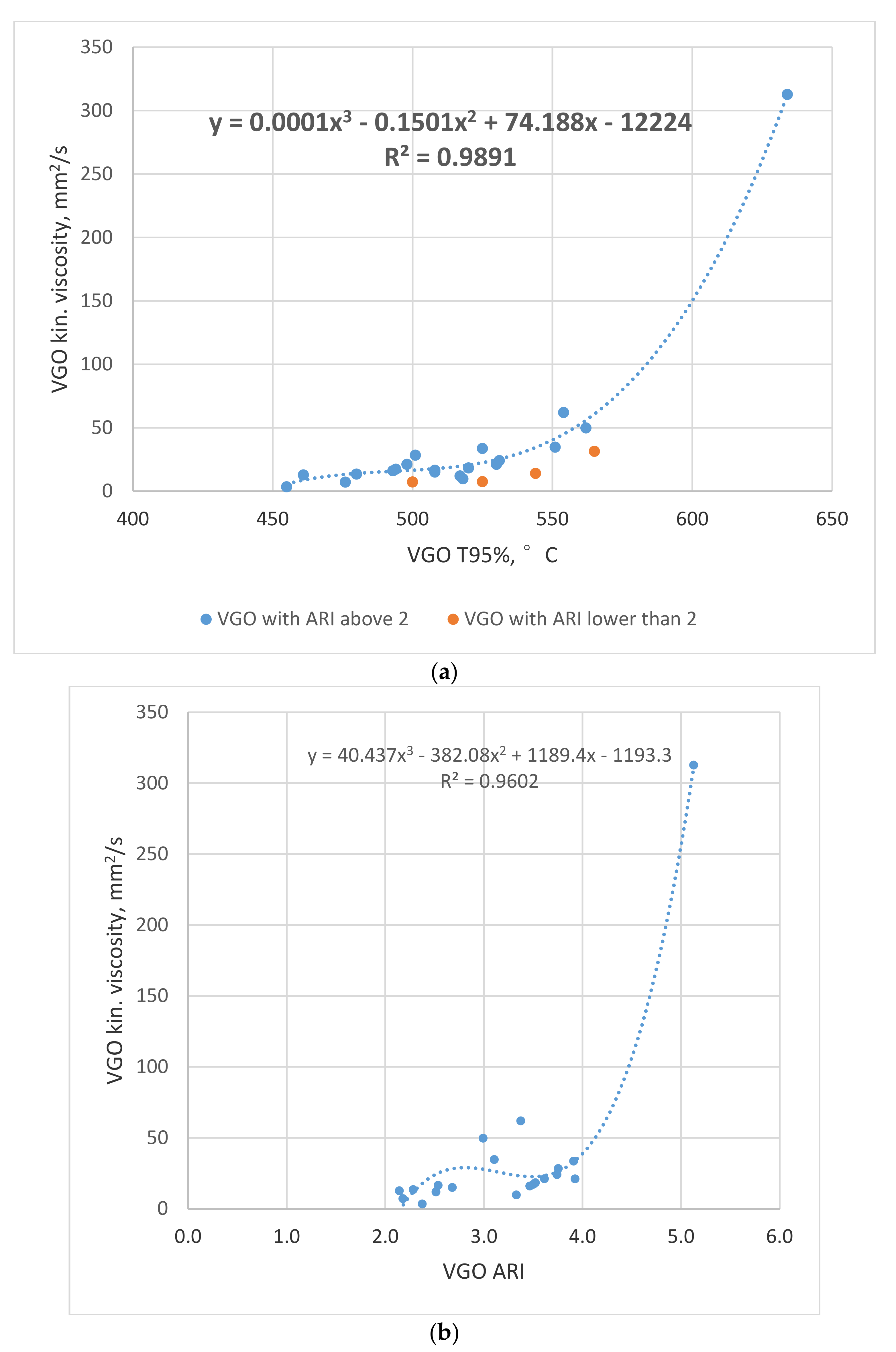
3.1. Relations of the Secondary VGO Properties to Viscosity
- (α, β)–positive consonance, if µCk,Cl > α and νCk,Cl < β;
- (α, β)–negative consonance, if µCk,Cl < β and νCk,Cl > α;
- (α, β)–dissonance, otherwise.
3.2. Evaluation of the Secondary VGO Viscosity Prediction Ability of Studied Empirical Models
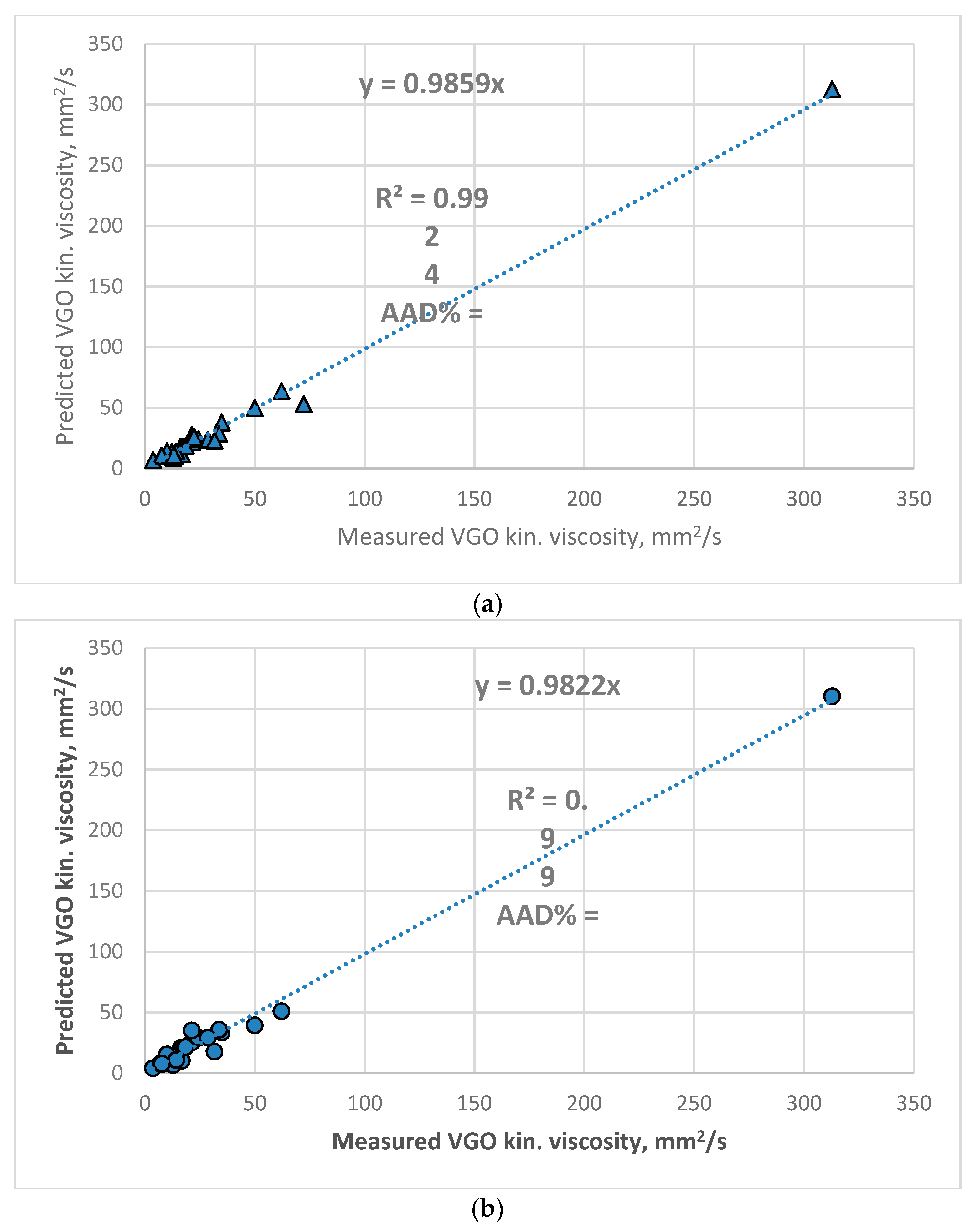
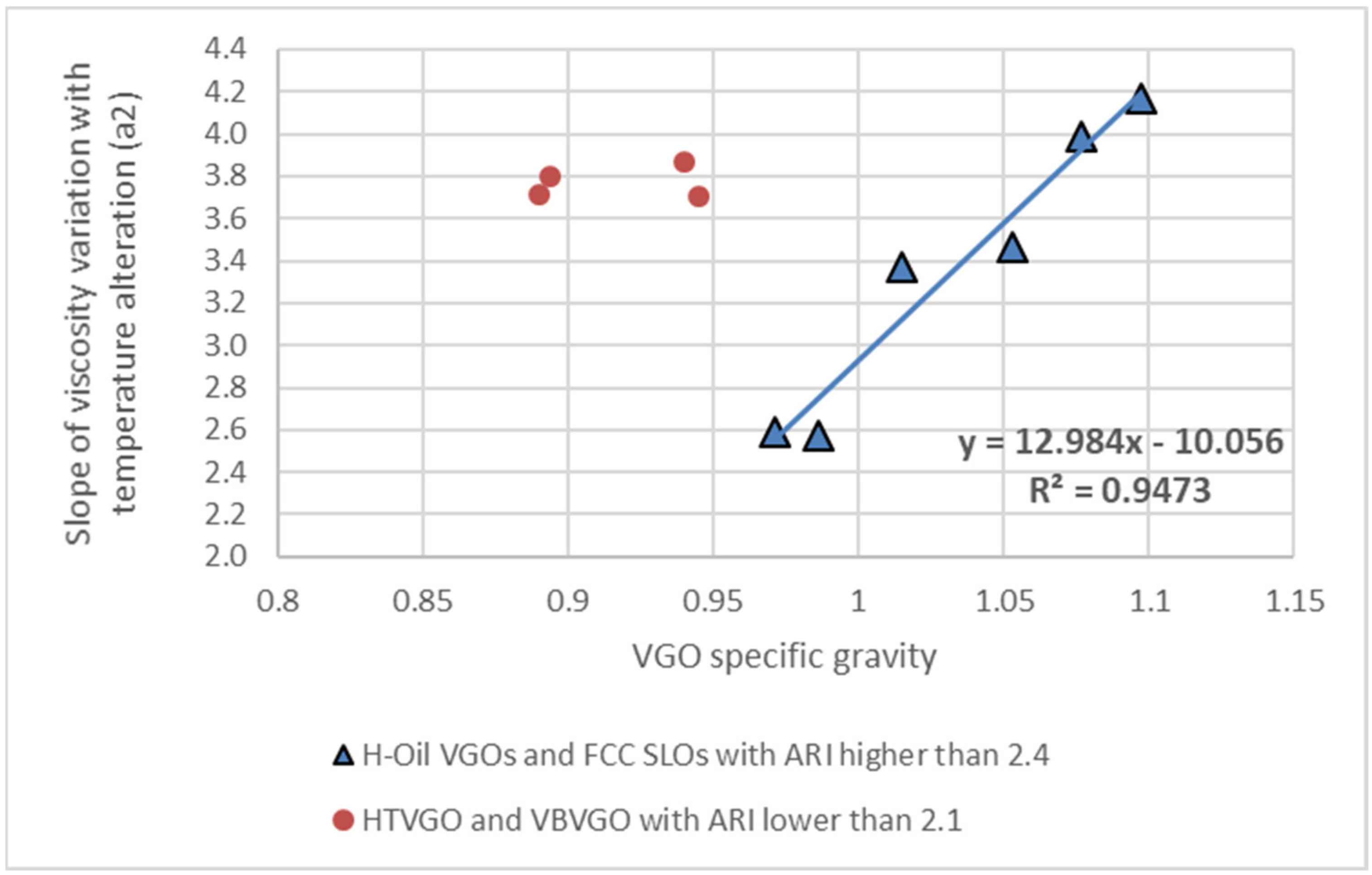
3.3. Development of New Empirical Model
- a = 0.8611313197;
- b = 0.3967069960;
- c = 0.2858346574;
- d = 10.5837141796;
- f = 3.669559682208.
3.4. Validation of the New Empirical Model
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| ABP | Average boiling point | K | nD20 = refractive index at 20 °C |
| SG | Specific gravity | E | Error |
| VIS | Kinematic viscosity, cSt | μ | Dynamic viscosity, cP |
| API | API gravity | SE | Standard error |
| ARI | Aromatic ring index | RSE | Relative standard error |
| d15 | Density at 15 °C, g/cm3 | SSE | Sum of squared errors |
| MW | Molecular weight, | R | Residual |
| VGO | Vacuum gas oil | Kw | Watson K factor |
| %AAD | Average absolute deviation, % | MIN E | Minimum error |
| MAX E | Maximum error | LNR | Lowest number residual |
| HNR | Highest number residual | #R− | Number of negative residuals |
| #R+ | Number of positive residuals | ICrA | Inter-criteria analysis |
| σ | Standard deviation of viscosity measurement |
References
- Touloukian, Y.S.; Saxena, S.C.; Hestermaus, P. Thermophysical Properties of Matter-TPRC Data Series, II. Viscosity; Purdue Research Foundation: West Lafayette, IN, USA, 1975. [Google Scholar]
- Reid, R.C.; Prausnitz, J.M.; Sherwood, T.K. The Properties of Gases and Liquids, 3rd ed; McGraw-Hill: New York, NY, USA, 1977; Available online: https://www.worldcat.org/title/properties-of-gases-and-liquids/oclc/560350942?referer=di&ht=edition (accessed on 28 June 2021).
- Stephan, K.; Lucas, K.D. Viscosity of Dense Fluids; Plenum Press: New York, NY, USA, 1979. [Google Scholar]
- Abbott, M.M.; Kaufmann, T.G.; Domash, L.A. Correlation for predicting liquid viscosities of petroleum fractions. Can. J. Chem. Eng. 1971, 49, 379–384. [Google Scholar] [CrossRef]
- Twu, C.H. Internally consistent correlation for predicting liquid viscosities of petroleum fractions. Ind. Eng. Chem. Proc. Des. Dev. 1985, 24, 1293–1297. [Google Scholar] [CrossRef]
- Reid, R.C.; Prausnitz, J.M.; Poling, B.E. The Properties of Gases and Liquids, 4th ed; McGraw-Hill: New York, NY, USA, 1987; Available online: https://www.worldcat.org/title/properties-of-gases-and-liquids/oclc/14520133 (accessed on 28 June 2021).
- Viswanath, D.S.; Natarajan, G. Data Book on the Viscosity of Liquids; Hemisphere Publishing: New York, NY, USA, 1989. [Google Scholar]
- Miadonye, A.; Puttagunta, V.R.; Singh, B. Prediction of the viscosity of crude oil fractions from a single measurement. Chem. Eng. Commun. 1993, 122, 195–199. [Google Scholar] [CrossRef]
- Monnery, W.D.; Svrcek, W.Y.; Mehrotra, A.K. Viscosity: A critical review of practical predictive and correlative methods. Can. J. Chem. Eng. 1995, 73, 3–40. [Google Scholar] [CrossRef]
- Mehrotra, A.K. A simple equation for predicting the viscosity of crude-oil fractions. Chem. Eng. Res. Des. 1995, 73, 87–90. [Google Scholar]
- Moharam, H.M.; Al-Mehaideb, R.A.; Fahim, M.A. New correlation for predicting the viscosity of heavy petroleum fractions. Fuel 1995, 74, 1776–1779. [Google Scholar] [CrossRef]
- Mehrotra, A.K.; Monnery, W.D.; Svrcek, W.Y. A review of practical calculation methods for the viscosity of liquid hydrocarbons and their mixtures. Fluid Phase Equilibria 1996, 117, 344–355. [Google Scholar] [CrossRef]
- Wakabayashi, T. Viscosity correlation with specific gravity and molecular weight of crude oil fractions. Fuel 1997, 76, 1049–1056. [Google Scholar] [CrossRef]
- Fang, W.; Lei, Q. Generalized correlation for predicting the kinematic viscosity of liquid petroleum fractions. Fluid Phase Equilibria 1999, 166, 125–139. [Google Scholar] [CrossRef]
- Aboul-Seoud, A.L.; Moharam, H.M. A generalized viscosity correlation for undefined petroleum fractions. Chem. Eng. J. 1999, 72, 253–256. [Google Scholar] [CrossRef]
- Al-Maamari, R.S.; Houache, O.; Abdul-Wahab, S.A. New correlating parameter for the viscosity of heavy crude oils. Energy Fuels 2006, 20, 2586–2592. [Google Scholar]
- Sánchez-Minero, F.; Sánchez-Reyna, G.; Ancheyta, J.; Marroquin, G. Comparison of correlations based on API gravity for predicting viscosity of crude oils. Fuel 2014, 138, 193–199. [Google Scholar]
- Bahadori, A. Prediction of heavy-oil viscosities with a simple correlation approach. Oil Gas Facil. 2015, 2, 66–72. [Google Scholar]
- Marinov, I.; Stratiev, D.; Sharafutdinov, I.; Rudnev, N.; Petkov, P. Evaluation of available empirical correlations for viscosity prediction of petroleum fractions originating from different crudes. Oil Gas Eur. Mag. 2016, 42, 145–150. [Google Scholar]
- AlMulla, H.A.; Albahri, T.A. Estimating the kinematic viscosity of petroleum fractions. Int. J. Thermophys. 2017, 38, 58. [Google Scholar]
- Kotzakoulakis, K.; George, S.C. A simple and flexible correlation for predicting the viscosity of crude oils. J. Pet. Sci. Eng. 2017, 158, 416–423. [Google Scholar]
- Kamel, A.; Alomair, O.; Elsharkawy, A. Measurements and predictions of Middle Eastern heavy crude oil viscosity using compositional data. J. Pet. Sci. Eng. 2019, 173, 990–1004. [Google Scholar]
- Kumar, R.; Maheshwari, S.; Voolapalli, R.K.; Upadhyayul, S. Investigation of physical parameters of crude oils and their impact on kinematic viscosity of vacuum residue and heavy product blends for crude oil selection. J. Taiwan Inst. Chem. Eng. 2021, 120, 33–42. [Google Scholar]
- Gabitto, J. Experimental and Theoretical Determination of Heavy Oil Viscosity under Reservoir Conditions. 2003. Available online: https://digital.library.unt.edu/ark:/67531/metadc736112/ (accessed on 28 June 2021).
- Khemka, Y.; Abutaqiya, M.I.L.; Sisco, C.J.; Chapman, W.G.; Vargas, F.M. Accurate prediction of the viscosity of light crude oils using one-parameter friction theory: Effect of crude oil characterization methods and property correlations. Fuel 2021, 283, 118926. [Google Scholar]
- Loria, H.; Motahhari, H.; Satyro, M.A.; Yarranton, H.W. Process simulation using the expanded fluid model for viscosity calculations. Chem. Eng. Res. Des. 2014, 92, 3083–3095. [Google Scholar]
- Hadavimoghaddam, F.; Ostadhassan, M.; Heidaryan, E.; Sadri, M.A.; Chapanova, I.; Popov, E.; Cheremisin, A.; Rafieepour, S. Prediction of dead oil viscosity: Machine learning vs. classical correlations. Energies 2021, 14, 930. [Google Scholar] [CrossRef]
- Torabi, F.; Abedini, A.; Abedini, R. The development of an artificial neural network model for prediction of crude oil viscosities. Pet. Sci. Technol. 2011, 29, 804–816. [Google Scholar] [CrossRef]
- Hemmati-Sarapardeh, A.; Aminshahidy, B.; Pajouhandeh, A.; Yousefi, S.H.; Hosseini-Kaldozakh, S.A. A soft computing approach for the determination of crude oil viscosity: Light and intermediate crude oil systems. J. Taiwan Inst. Chem. Eng. 2016, 59, 1–10. [Google Scholar] [CrossRef]
- Ghorbani, B.; Hamedi, M.; Shirmohammadi, R. A novel multi-hybrid model for estimating optimal viscosity correlations of Iranian crude oil. J. Pet. Sci. Eng. 2016, 142, 68–76. [Google Scholar] [CrossRef]
- Oloso, M.A.; Hassan, M.G.; Bader-El-Den, M.B.; Buick, J.M. Ensemble SVM for characterisation of crude oil viscosity. J. Pet. Explor. Prod. Technol. 2017, 8, 531–546. [Google Scholar] [CrossRef] [Green Version]
- Oloso, M.A.; Khoukhi, A.; Abdulraheem, A.; Elshafei, M. Prediction of crude oil viscosity and gas/oil ratio curves using recent advances to neural networks. In Proceedings of the SPE/EAGE Reservoir Characterization & Simulation Conference, Abu Dhabi, United Arab Emirates, 19–21 October 2009. [Google Scholar]
- Wang, G.; Liu, Y.; Wang, X.; Xu, C.; Gao, J. Studies on the catalytic cracking performance of coker gas oil. Energy Fuels 2009, 23, 1942–1949. [Google Scholar] [CrossRef]
- Stratiev, D.; Shishkova, I.; Yankov, V.; Yordanov, D.; Tankov, I. Fluid catalytic cracking of H-Oil derived heavy oils. Oxid Commun. 2020, 43, 289–301. [Google Scholar]
- Sheng, Q.; Wang, G.; Liu, Y.; Husein, M.M.; Gao, C.; Shi, Q.; Gao, J. Combined hydrotreating and fluid catalytic cracking processing for the conversion of inferior coker gas oil: Effect on nitrogen compounds and condensed aromatics. Energy Fuels 2018, 32, 4979–4987. [Google Scholar] [CrossRef]
- Stratiev, D.; Shishkova, I.; Ivanov, M.; Dinkov, R.; Georgiev, B.; Argirov, G.; Atanassova, V.; Vassilev, P.; Atanassov, K.; Yordanov, D.; et al. Catalytic cracking of diverse vacuum residue hydrocracking gas oils. Chem. Eng. Technol. 2021, 44, 997–1008. [Google Scholar] [CrossRef]
- Mondal, S.; Yadav, A.; Kumar, R.; Bansal, V.; Das, S.K.; Christopher, J.; Kapur, G.S. Molecular-level structural insight into clarified oil by nuclear magnetic resonance (NMR) spectroscopy: Estimation of hydrocarbon types and average structural parameters. Energy Fuels 2017, 31, 7682–7692. [Google Scholar] [CrossRef]
- Redelius, P.; Soenen, H. Relation between bitumen chemistry and performance. Fuel 2015, 140, 34–43. [Google Scholar] [CrossRef]
- Diarov, I.N.; Batueva, I.U.; Sadikov, A.N.; Colodova, N.L. Chemistry of Crude Oil; Chimia Publishers: St. Peterburg, Russia, 1990. (In Russian) [Google Scholar]
- Liñan, L.Z.; Lima, N.M.N.; Maciel, M.R.W.; Filho, M.; Medina, L.C.; Embiruçu, M. Correlation for predicting the molecular weight of Brazilian petroleum residues and cuts: An application for the simulation of a molecular distillation process. J. Pet. Sci. Eng. 2011, 78, 78–85. [Google Scholar] [CrossRef]
- Stratiev, D.S.; Marinov, I.M.; Shishkova, I.K.; Dinkov, R.K.; Stratiev, D.D. Investigation on feasibility to predict the content of saturate plus mono-nuclear aromatic hydrocarbons in vacuum gas oils from bulk properties and empirical correlations. Fuel 2014, 129, 156–162. [Google Scholar] [CrossRef]
- Abutaqiya, M. Advances in Thermodynamic Modeling of Nonpolar Hydrocarbons and Asphaltene Precipitation in Crude Oils. Ph.D. Thesis, Rice University, Houston, TX, USA, 2019. [Google Scholar]
- Abutaqiya, M.I.L.; AlHammadi, A.A.; Sisco, C.J.; Vargas, F.M. Aromatic ring index (ARI): A Characterization factor for nonpolar hydrocarbons from molecular weight and refractive index. Energy Fuels 2021, 35, 1113–1119. [Google Scholar] [CrossRef]
- Atanassov, K. Intuitionistic fuzzy sets. In VII ITKR’s Session; Central Institute for Scientific and Technical Information: Sofia, Bulgaria, 1983. (In Bulgarian) [Google Scholar]
- Atanassov, K. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Atanassov, K. Intuitionistic Fuzzy Sets: Theory and Applications; Springer Physica: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Atanassov, K. On Intuitionistic Fuzzy Sets Theory; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Atanassov, K. Generalized index matrices. Comptes Rendus l’Acad. Bulg. Sci. 1987, 11, 15–18. [Google Scholar]
- Atanassov, K. Index Matrices: Towards an Augmented Matrix Calculus; Springer: Cham, Switzerland, 2014. [Google Scholar]
- InterCriteria Research Portal. 2015. Available online: http://www.intercriteria.net/publications (accessed on 28 June 2021).
- Stratiev, D.S.; Shishkova, I.K.; Dinkov, R.K.; Petrov, I.P.; Kolev, I.V.; Yordanov, D.; Sotirov, S.; Sotirova, E.; Atanassova, V.; Ribagin, S.; et al. Empirical models to characterize the structural and physio-chemical properties of vacuum gas oils with different saturate contents. Resources 2021, 10, 71. [Google Scholar] [CrossRef]
- Hernández, E.A.; Sánchez-Reyna, G.; Ancheyta, J. Comparison of mixing rules based on binary interaction parameters for calculating viscosity of crude oil blends. Fuel 2019, 249, 198–205. [Google Scholar] [CrossRef]
| Nr | Sample | Density at 15 °C, g/cm3 | SG | API | T10% | T50% | T90% | T95% | ABP, °C | Kin. vis. at 80 °C, mm2/s | Kin. vis at 98.9 °C, mm2/s | RI at 20 °C | Kw | MW, g/mol | ARI | Sat. | Aromatics |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | HAGO-1 | 0.9504 | 0.9512 | 17.3 | 343 | 397 | 455 | 476 | 398 | 7.3 | 4.6 | 1.5385 | 11.21 | 342 | 2.2 | 38.4 | 61.6 |
| 2 | LVGO-1 | 0.9707 | 0.9715 | 14.1 | 343 | 414 | 493 | 517 | 417 | 12.1 | 7.1 | 1.5509 | 11.07 | 364 | 2.5 | 30.8 | 69.2 |
| 3 | HVGO-1 | 0.9849 | 0.9858 | 12.0 | 426 | 491 | 548 | 562 | 488 | 49.9 | 22.9 | 1.5524 | 11.28 | 462 | 3.0 | 24.4 | 75.6 |
| 4 | HAGO-2 | 0.9582 | 0.9590 | 16.0 | 335 | 395 | 458 | 480 | 396 | 13.6 | 7.9 | 1.5442 | 11.10 | 339 | 2.3 | 35.6 | 64.4 |
| 5 | LVGO-2 | 0.9847 | 0.9856 | 12.1 | 330 | 410 | 488 | 508 | 409 | 15.2 | 8.6 | 1.5612 | 10.88 | 355 | 2.7 | 26.3 | 73.7 |
| 6 | HVGO-2 | 1.0075 | 1.0084 | 8.8 | 430 | 489 | 540 | 554 | 486 | 62.1 | 27.4 | 1.5685 | 11.02 | 458 | 3.4 | 17.0 | 83.0 |
| 7 | HAGO-3 | 0.9506 | 0.9514 | 17.2 | 323 | 377 | 439 | 461 | 380 | 12.9 | 7.5 | 1.5409 | 11.10 | 321 | 2.1 | 38.5 | 61.5 |
| 8 | LVGO-3 | 0.9760 | 0.9768 | 13.4 | 324 | 395 | 482 | 508 | 400 | 16.7 | 9.3 | 1.5567 | 10.92 | 344 | 2.5 | 29.2 | 70.8 |
| 9 | HVGO-3 | 0.9961 | 0.9970 | 10.4 | 405 | 470 | 534 | 551 | 470 | 34.8 | 17.1 | 1.5626 | 11.06 | 434 | 3.1 | 21.1 | 78.9 |
| 10 | FCC SLO-1 | 0.9862 | 0.9871 | 11.9 | 232 | 282 | 412 | 455 | 309 | 3.6 | 2.5 | 1.5763 | 10.30 | 253 | 2.4 | 27.7 | 72.3 |
| 11 | FCC SLO-2 | 1.054 | 1.0549 | 2.6 | 292 | 372 | 475 | 518 | 380 | 9.9 | 6.0 | 1.6140 | 10.01 | 319 | 3.3 | 8.6 | 91.4 |
| 12 | FCC SLO-3 | 1.0564 | 1.0573 | 2.3 | 329 | 392 | 471 | 493 | 397 | 16.2 | 9.1 | 1.6135 | 10.08 | 338 | 3.5 | 7.4 | 92.6 |
| 13 | FCC SLO-4 | 1.0662 | 1.0671 | 1.1 | 337 | 401 | 476 | 498 | 405 | 21.3 | 11.4 | 1.6194 | 10.02 | 345 | 3.6 | 5.1 | 94.9 |
| 14 | FCC SLO-5 | 1.0615 | 1.0624 | 1.7 | 324 | 391 | 471 | 494 | 395 | 17.4 | 9.7 | 1.6172 | 10.02 | 335 | 3.5 | 6.4 | 93.6 |
| 15 | FCC SLO-6 | 1.0943 | 1.0953 | −2.3 | 331 | 400 | 491 | 525 | 407 | 33.8 | 16.7 | 1.6392 | 9.78 | 346 | 3.9 | 0.0 | 100.0 |
| 16 | FCC SLO-7 | 1.0779 | 1.0788 | −0.3 | 326 | 397 | 493 | 531 | 405 | 24.2 | 12.7 | 1.6280 | 9.92 | 345 | 3.7 | 2.7 | 97.3 |
| 17 | FCC SLO-8 | 1.0621 | 1.0630 | 1.6 | 317 | 389 | 484 | 520 | 397 | 18.5 | 10.1 | 1.6178 | 10.02 | 337 | 3.5 | 6.2 | 93.8 |
| 18 | FCC SLO-9 | 1.0826 | 1.0835 | −0.9 | 327 | 401 | 480 | 501 | 403 | 28.5 | 14.5 | 1.6309 | 9.86 | 342 | 3.8 | 2.0 | 98.0 |
| 19 | FCC SLO-10 | 1.1760 | 1.1770 | −11.3 | 371 | 435 | 562 | 634 | 456 | 312.8 | 97.1 | 1.6927 | 9.31 | 395 | 5.1 | 0.0 | 100.0 |
| 20 | FCC SLO-11 | 1.1001 | 1.1011 | −3.0 | 332 | 394 | 482 | 530 | 403 | 21.2 | 11.4 | 1.6440 | 9.70 | 340 | 3.9 | 0.0 | 100.0 |
| 21 | VGO blend | 0.9157 | 0.9165 | 22.9 | 376 | 446 | 525 | 544 | 449 | 14.2 | 8.1 | 1.5088 | 11.92 | 404 | 1.7 | 52.2 | 47.8 |
| 22 | HAGO-4 | 0.9041 | 0.905 | 24.9 | 357 | 425 | 489 | 505 | 424 | 8.0 | 4.9 | 1.5029 | 11.93 | 370 | 1.4 | 46.4 | 53.6 |
| 23 | LVGO-4 | 0.9112 | 0.912 | 23.7 | 322 | 417 | 528 | 550 | 422 | 8.6 | 5.2 | 1.5088 | 11.83 | 369 | 1.6 | 51.0 | 49.0 |
| 24 | HVGO-4 | 0.9211 | 0.922 | 22.0 | 411 | 486 | 552 | 568 | 483 | 27.2 | 13.5 | 1.5082 | 12.03 | 453 | 1.8 | 45.6 | 54.4 |
| Correlation Used | Equation | API | ABP, K | SG |
|---|---|---|---|---|
| Abbott [4] | 10.1°–50.3° | 427.15–889.15 | – | |
| Twu [5] | – | – | – | |
| Fang [14] | | – | 363.15–727.15 | 0.73–0.90 |
| Aboul-Seoud and Moharam [15] | | – | 323.15–773.15 | – |
| AlMulla and Albahri [20] | – | 450.65–883.45 | 0.769–0.952 | |
| Sánchez-Minero [17] | | 12.4°–43° | 303.15–333.15 | – |
| Kotzakoulakis [21] | – | 358–873 | 0.806–1.024 |
| D15 | T10% | T50% | T90% | T95% | ABP, °C | VIS 80 | VIS 98.9 | RI at 20 °C | Kw | MW | ARI | Sat. | Aro | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| D15 | 1.00 | 0.46 | 0.42 | 0.51 | 0.56 | 0.46 | 0.74 | 0.74 | 0.97 | 0.07 | 0.44 | 0.96 | 0.02 | 0.97 |
| T10% | 0.46 | 1.00 | 0.86 | 0.71 | 0.67 | 0.83 | 0.67 | 0.66 | 0.43 | 0.60 | 0.84 | 0.48 | 0.52 | 0.46 |
| T50% | 0.42 | 0.86 | 1.00 | 0.82 | 0.76 | 0.93 | 0.66 | 0.66 | 0.39 | 0.63 | 0.96 | 0.45 | 0.55 | 0.42 |
| T90% | 0.51 | 0.71 | 0.82 | 1.00 | 0.92 | 0.86 | 0.72 | 0.72 | 0.49 | 0.53 | 0.85 | 0.53 | 0.47 | 0.51 |
| T95% | 0.56 | 0.67 | 0.76 | 0.92 | 1.00 | 0.81 | 0.75 | 0.75 | 0.54 | 0.47 | 0.79 | 0.57 | 0.43 | 0.56 |
| ABP °C | 0.46 | 0.83 | 0.93 | 0.86 | 0.81 | 1.00 | 0.70 | 0.69 | 0.43 | 0.59 | 0.96 | 0.49 | 0.51 | 0.46 |
| VIS 80 | 0.74 | 0.67 | 0.66 | 0.72 | 0.75 | 0.70 | 1.00 | 1.00 | 0.71 | 0.33 | 0.68 | 0.74 | 0.26 | 0.72 |
| VIS 98.9 | 0.74 | 0.66 | 0.66 | 0.72 | 0.75 | 0.69 | 1.00 | 1.00 | 0.71 | 0.33 | 0.67 | 0.74 | 0.26 | 0.72 |
| RI at 20 °C | 0.97 | 0.43 | 0.39 | 0.49 | 0.54 | 0.43 | 0.71 | 0.71 | 1.00 | 0.04 | 0.41 | 0.93 | 0.04 | 0.94 |
| Kw | 0.07 | 0.60 | 0.63 | 0.53 | 0.47 | 0.59 | 0.33 | 0.33 | 0.04 | 1.00 | 0.63 | 0.10 | 0.89 | 0.09 |
| MW | 0.44 | 0.84 | 0.96 | 0.85 | 0.79 | 0.96 | 0.68 | 0.67 | 0.41 | 0.63 | 1.00 | 0.46 | 0.54 | 0.44 |
| ARI | 0.96 | 0.48 | 0.45 | 0.53 | 0.57 | 0.49 | 0.74 | 0.74 | 0.93 | 0.10 | 0.46 | 1.00 | 0.03 | 0.96 |
| Sat. | 0.02 | 0.52 | 0.55 | 0.47 | 0.43 | 0.51 | 0.26 | 0.26 | 0.04 | 0.89 | 0.54 | 0.03 | 1.00 | 0.01 |
| Aro | 0.97 | 0.46 | 0.42 | 0.51 | 0.56 | 0.46 | 0.72 | 0.72 | 0.94 | 0.09 | 0.44 | 0.96 | 0.01 | 1.00 |
| D15 | T10% | T50% | T90% | T95% | ABP, °C | VIS 80 | VIS 98.9 | RI at 20 °C | Kw | MW | ARI | Sat. | Aro | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| D15 | 0.00 | 0.53 | 0.57 | 0.48 | 0.43 | 0.52 | 0.26 | 0.26 | 0.03 | 0.91 | 0.55 | 0.02 | 0.97 | 0.02 |
| T10% | 0.53 | 0.00 | 0.12 | 0.27 | 0.32 | 0.15 | 0.33 | 0.33 | 0.55 | 0.38 | 0.14 | 0.49 | 0.46 | 0.52 |
| T50% | 0.57 | 0.12 | 0.00 | 0.16 | 0.22 | 0.04 | 0.33 | 0.33 | 0.59 | 0.34 | 0.03 | 0.53 | 0.42 | 0.55 |
| T90% | 0.48 | 0.27 | 0.16 | 0.00 | 0.06 | 0.11 | 0.27 | 0.27 | 0.50 | 0.45 | 0.13 | 0.45 | 0.51 | 0.47 |
| T95% | 0.43 | 0.32 | 0.22 | 0.06 | 0.00 | 0.17 | 0.25 | 0.25 | 0.45 | 0.51 | 0.20 | 0.41 | 0.56 | 0.43 |
| ABP °C | 0.52 | 0.15 | 0.04 | 0.11 | 0.17 | 0.00 | 0.29 | 0.29 | 0.55 | 0.38 | 0.02 | 0.49 | 0.46 | 0.51 |
| VIS 80 | 0.26 | 0.33 | 0.33 | 0.27 | 0.25 | 0.29 | 0.00 | 0.00 | 0.29 | 0.65 | 0.32 | 0.24 | 0.72 | 0.26 |
| VIS 98.9 | 0.26 | 0.33 | 0.33 | 0.27 | 0.25 | 0.29 | 0.00 | 0.00 | 0.28 | 0.65 | 0.32 | 0.24 | 0.72 | 0.26 |
| RI at 20 °C | 0.03 | 0.55 | 0.59 | 0.50 | 0.45 | 0.55 | 0.29 | 0.28 | 0.00 | 0.94 | 0.58 | 0.05 | 0.94 | 0.04 |
| Kw | 0.91 | 0.38 | 0.34 | 0.45 | 0.51 | 0.38 | 0.65 | 0.65 | 0.94 | 0.00 | 0.35 | 0.88 | 0.09 | 0.89 |
| MW | 0.55 | 0.14 | 0.03 | 0.13 | 0.20 | 0.02 | 0.32 | 0.32 | 0.58 | 0.35 | 0.00 | 0.52 | 0.44 | 0.54 |
| ARI | 0.02 | 0.49 | 0.53 | 0.45 | 0.41 | 0.49 | 0.24 | 0.24 | 0.05 | 0.88 | 0.52 | 0.00 | 0.95 | 0.02 |
| Sat. | 0.97 | 0.46 | 0.42 | 0.51 | 0.56 | 0.46 | 0.72 | 0.72 | 0.94 | 0.09 | 0.44 | 0.95 | 0.00 | 0.99 |
| Aro | 0.02 | 0.52 | 0.55 | 0.47 | 0.43 | 0.51 | 0.26 | 0.26 | 0.04 | 0.89 | 0.54 | 0.02 | 0.99 | 0.00 |
| Abbott | Twu | Al-Mulla | Aboul-Seoud | Sánchez-Minero | Fang | Kotzakoulakis | ||
|---|---|---|---|---|---|---|---|---|
| 1 | MIN E | −1368.7 | −260.5 | −1.41 × 1012 | −65.5 | NA | NA | −22,586.0 |
| 2 | MAX E | 51.3 | 61.8 | 100.0 | 48.9 | NA | NA | 76.9 |
| 3 | SE | 62.9 | 16.0 | 3.42 × 1010 | 6.3 | NA | NA | 15,064.7 |
| 4 | RSE | 190.4 | 110.5 | 1.04 × 1011 | 19.1 | NA | NA | 45,608.1 |
| 5 | SSE | 226.0 | 40.7 | 1.98 × 1020 | 1.9 | NA | NA | 51,789.1 |
| 6 | LNR | −7.8 | −13.8 | −1.60 × 1011 | −13.9 | NA | NA | −70,656.8 |
| 7 | HPR | 291.5 | 60.0 | 97.1 | 14.0 | NA | NA | 24.3 |
| 8 | #R− | 5 | 23 | 23 | 12 | NA | NA | 12 |
| 9 | #R+ | 19 | 1 | 1 | 12 | NA | NA | 12 |
| 10 | Range R | 299.3 | 73.8 | 1.60 × 1011 | 28.0 | NA | NA | 70,681.1 |
| 11 | %AAD | 79.3 | 109.7 | 5.87 × 1010 | 21.7 | NA | NA | 1259.9 |
| Nr | Sample | SG | ABP °C | ARI | Kin. vis. at 80 °C, mm2/s | Predicted Viscosity at 80 °C by Aboud-Seoul and Moharam [15] Empirical Model | Predicted Viscosity at 80 °C by the Empirical Model Developed in This Work | AAD% (Aboul-Seoud and Moharam [15]) | AAD% (This Work) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | HAGO-5 | 0.971 | 397 | 2.4 | 13 | 10.1 | 11.5 | 22.1 | 11.5 |
| 2 | LVGO-5 | 0.986 | 393 | 2.6 | 13 | 9.4 | 11.2 | 28.6 | 15.2 |
| 3 | HVGO-5 | 1.015 | 476 | 3.4 | 57.5 | 45.5 | 53 | 20.8 | 7.8 |
| 4 | FCC SLO-12 | 1.097 | 402 | 3.9 | 22.2 | 33 | 26.2 | 48.5 | 17.7 |
| 5 | VBGO-1 | 0.940 | 439 | 2.1 | 14.7 | 12.0 | 14.6 | 18.2 | 0.4 |
| 6 | VBGO-2 | 0.945 | 431 | 2.1 | 13.5 | 11.3 | 13.7 | 16.2 | 1.3 |
| 7 | FCC SLO-13 | 1.053 | 366 | 3.2 | 14.5 | 12.6 | 12.0 | 13.4 | 17.2 |
| 8 | FCC SLO-14 | 1.077 | 390 | 3.6 | 16.2 | 22.3 | 18.6 | 37.5 | 15.0 |
| 9 | HTVGO-1 | 0.894 | 434 | 1.2 | 10.4 | 7.6 | 10.8 | 27.4 | 3.9 |
| 10 | HTVGO-2 | 0.890 | 431 | 1.2 | 9.6 | 7.1 | 10.4 | 25.9 | 8.2 |
| AAD% | 25.9 | 9.8 |
| Kinematic Viscosity, mm2/s | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| T °C | HAGO-5 | LVGO-5 | HVGO-5 | FCC SLO-12 | VBGO-1 | VBGO-2 | FCC SLO-13 | FCC SLO-14 | HTVGO-1 | HTVGO-2 |
| 40 | 107.8 | 194.0 | ||||||||
| 50 | 29.6 | 30.8 | 109.3 | 46.1 | 39.5 | 46.3 | 85.1 | 28.8 | 25.6 | |
| 60 | 18.7 | 18.5 | 118.6 | 53.7 | 29.8 | 44.8 | 19.6 | 17.7 | ||
| 70 | 15.0 | 14.8 | 95.6 | 31.5 | 20.0 | 25.6 | 14.1 | 13.5 | ||
| 80 | 13.0 | 13.0 | 57.5 | 22.2 | 14.7 | 13.5 | 14.5 | 16.2 | 10.4 | 9.6 |
| 90 | 11.3 | 11.7 | 46.3 | 17.3 | 8.5 | 8.2 | ||||
| 100 | 24.4 | |||||||||
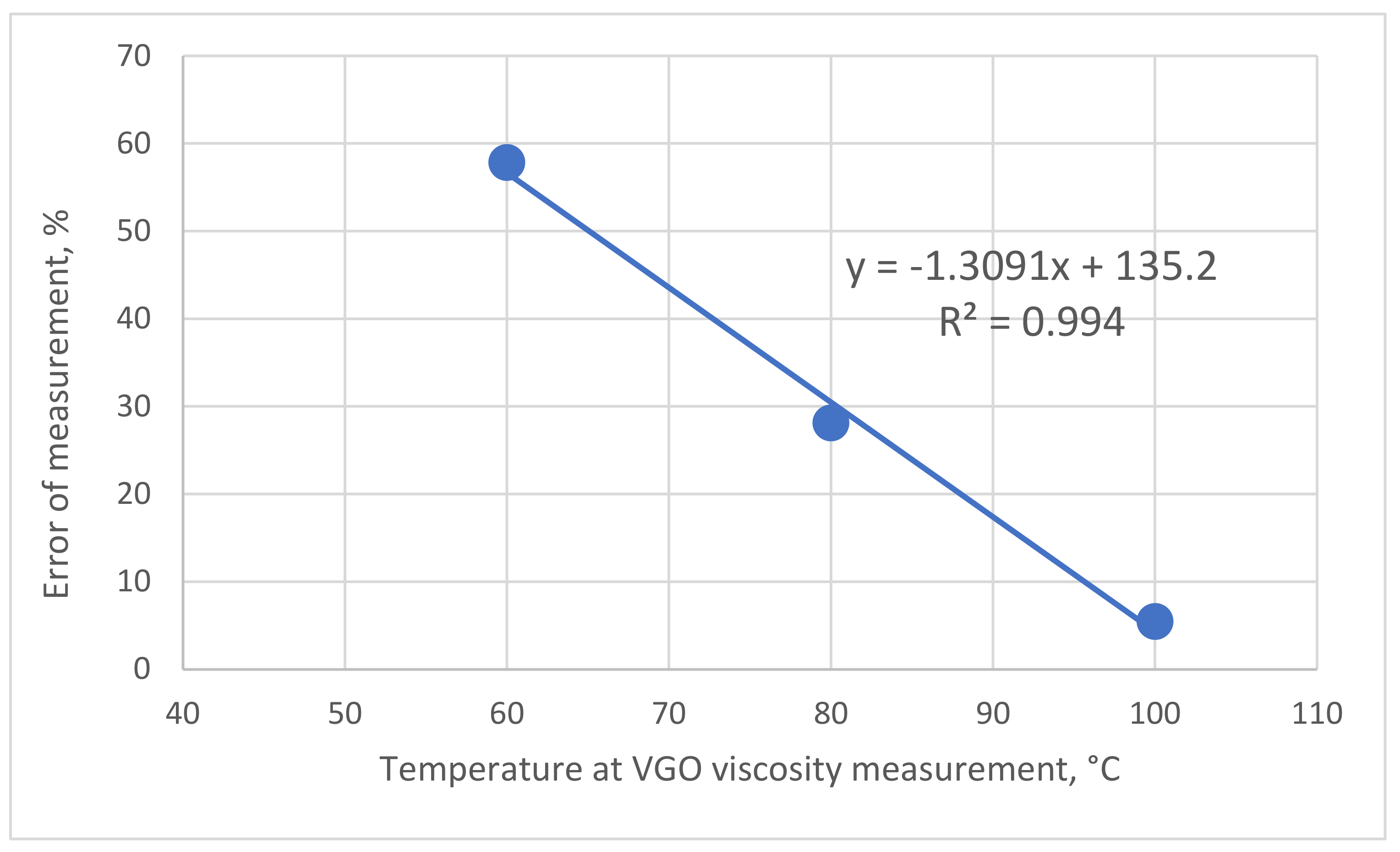
| HVGO-5 | LVGO-5 | ||
|---|---|---|---|
| Temperature °C | 80 | 100 | 60 |
| 1st measurement | 72.6 | 25.7 | 46.7 |
| 2nd measurement | 59.3 | 24.1 | 27.8 |
| 3rd measurement | 57.4 | 24.1 | 25.9 |
| 4th measurement | 51.9 | 24.1 | 25.9 |
| 5th measurement | 51.9 | 24.1 | 27.8 |
| 6th measurement | 51.9 | 24.5 | |
| Average | 57.5 | 24.4 | 30.8 |
| σ2 | 65.4 | 0.4 | 79.4 |
| σ | 8.1 | 0.7 | 8.9 |
| 2 σ, % (error of measurement) | 28.1 | 5.5 | 57.8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stratiev, D.S.; Nenov, S.; Shishkova, I.K.; Dinkov, R.K.; Zlatanov, K.; Yordanov, D.; Sotirov, S.; Sotirova, E.; Atanassova, V.; Atanassov, K.; et al. Comparison of Empirical Models to Predict Viscosity of Secondary Vacuum Gas Oils. Resources 2021, 10, 82. https://doi.org/10.3390/resources10080082
Stratiev DS, Nenov S, Shishkova IK, Dinkov RK, Zlatanov K, Yordanov D, Sotirov S, Sotirova E, Atanassova V, Atanassov K, et al. Comparison of Empirical Models to Predict Viscosity of Secondary Vacuum Gas Oils. Resources. 2021; 10(8):82. https://doi.org/10.3390/resources10080082
Chicago/Turabian StyleStratiev, Dicho S., Svetoslav Nenov, Ivelina K. Shishkova, Rosen K. Dinkov, Kamen Zlatanov, Dobromir Yordanov, Sotir Sotirov, Evdokia Sotirova, Vassia Atanassova, Krassimir Atanassov, and et al. 2021. "Comparison of Empirical Models to Predict Viscosity of Secondary Vacuum Gas Oils" Resources 10, no. 8: 82. https://doi.org/10.3390/resources10080082
APA StyleStratiev, D. S., Nenov, S., Shishkova, I. K., Dinkov, R. K., Zlatanov, K., Yordanov, D., Sotirov, S., Sotirova, E., Atanassova, V., Atanassov, K., Stratiev, D. D., & Todorova-Yankova, L. (2021). Comparison of Empirical Models to Predict Viscosity of Secondary Vacuum Gas Oils. Resources, 10(8), 82. https://doi.org/10.3390/resources10080082










