Charge Pump Phase-Locked Loop-Based Frequency Conditioning of a MEMS Resonator

Abstract

MEMS resonators have attracted attention for their wide applications in highly accurate clock references, sensors, wireless communications, frequency control, etc. Most of the output frequencies of MEMS resonators require post-processing or calibration to be accurate enough. In this paper, a charge pump phase-locked loop-based frequency conditioning method for MEMS resonators is explored. An optimization scheme is proposed to enhance the frequency stability and signal quality of MEMS resonators. The experimental results show that the method significantly improves the resonator performance and achieves effective control of the resonant frequency. This research provides a new technical path for the design of high-performance MEMS oscillators, which has important theoretical significance and practical application value.

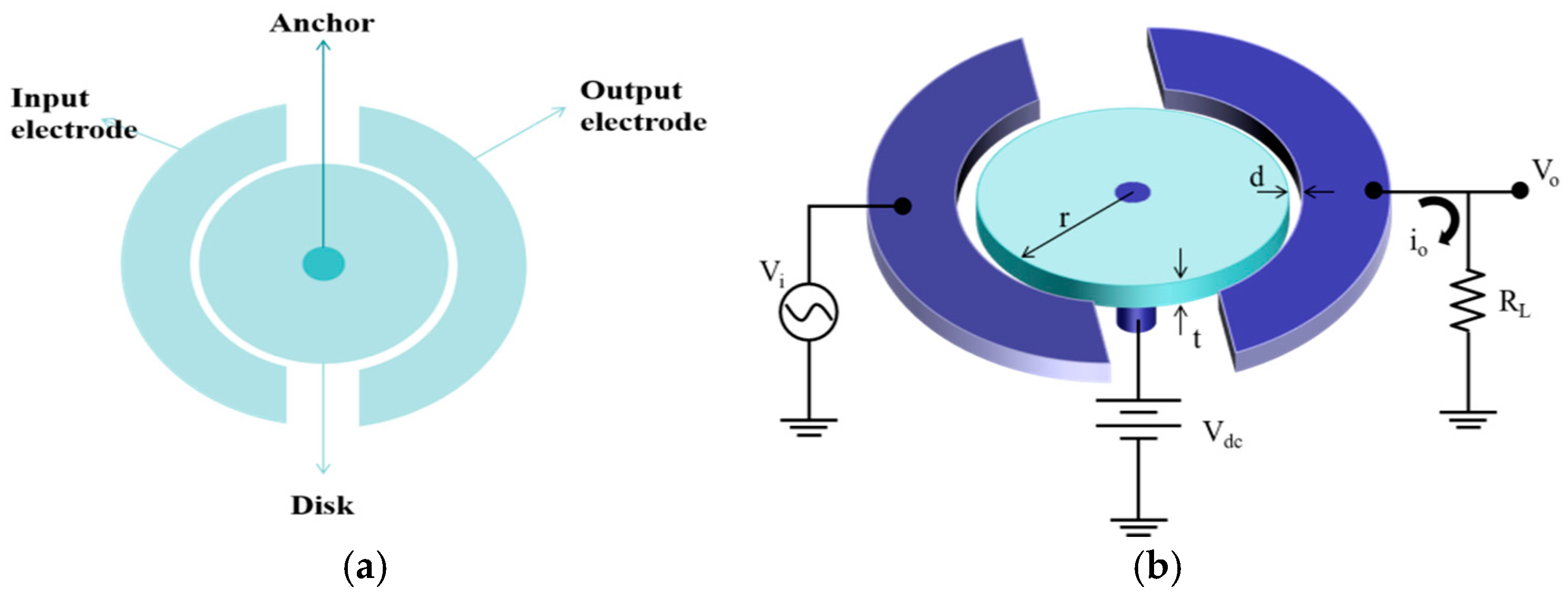
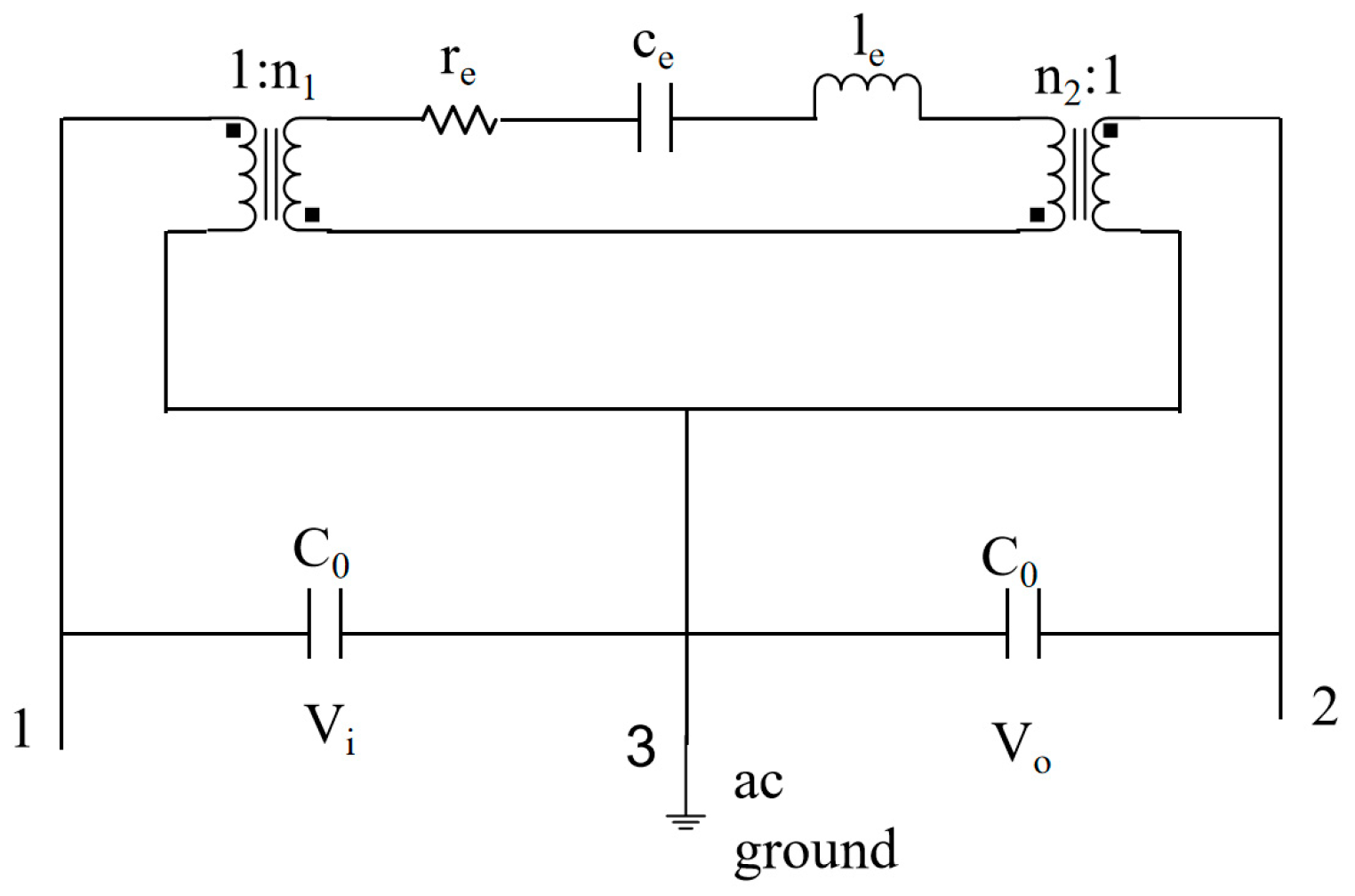
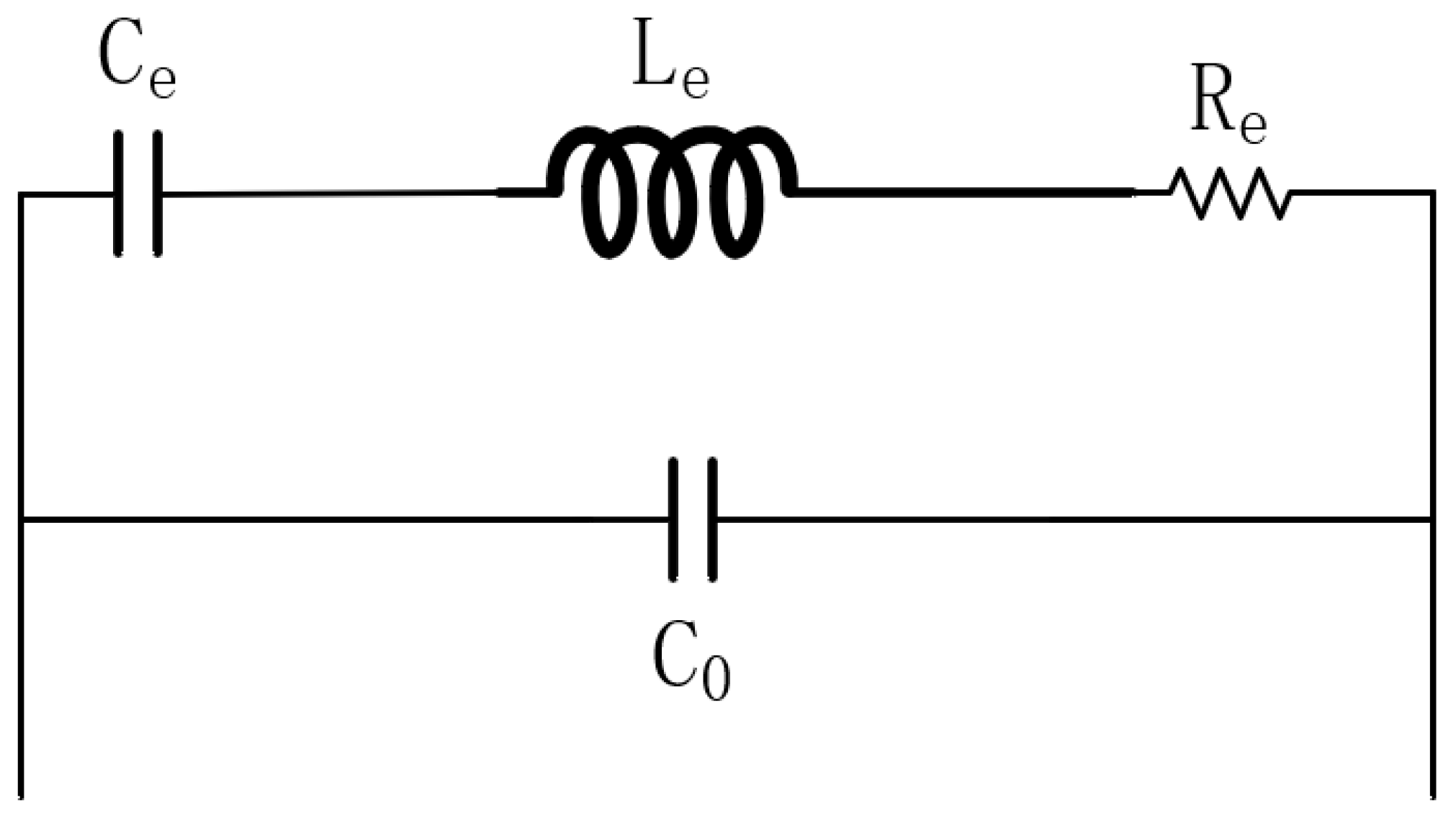
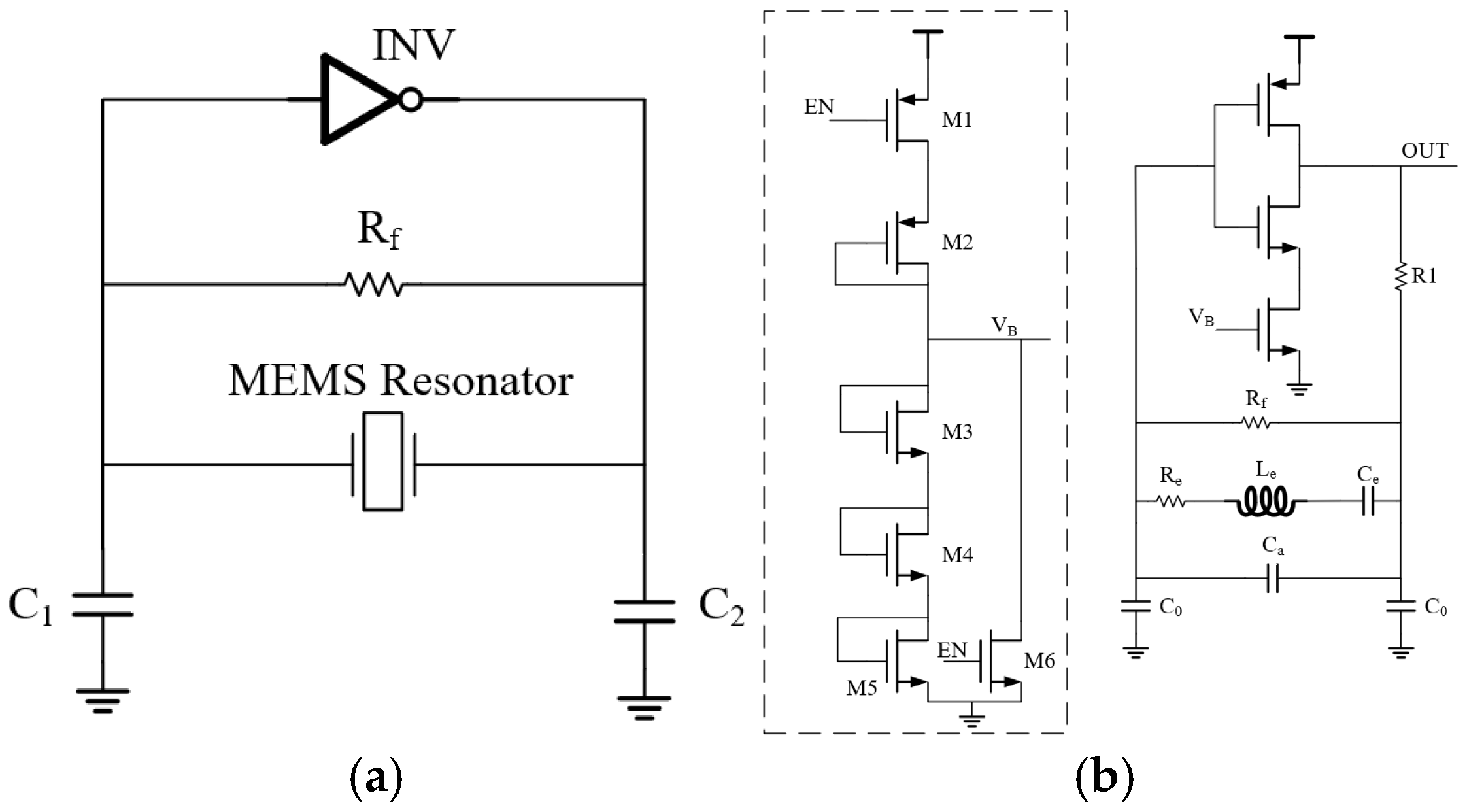
1. Introduction

A resonator is the core device for generating a time–frequency signal, which is the reference for applications such as sensing, positioning, navigation, etc. It is also the “heart” of all electronic systems, in which the stability of the output frequency signals affects the overall system performance [1]. The traditional quartz resonator, with a large size and low accuracy, is very difficult to integrate, becoming one of the bottlenecks limiting the miniaturization of the system and its performance. The silicon MEMS resonator shows much better benefits than those of traditional quartz ones [2]. The silicon MEMS resonator is more than ten times smaller than the traditional quartz resonator [3] and can be integrated with CMOS chips [4], thus bringing disruptive innovations in the related fields [5].

The PLL (phase-locked loop) is an electronic circuit. Its operation principle is based on the comparison of the phase of the reference clock with the phase of the feedback clock signal to achieve a zero-phase output, thus achieving the purpose of phase locking [6]. The PLL is often used as a frequency multiplier, modulation and demodulation circuits, and is always used to generate a clock signal in digital systems [7]. In the clock reference system, the PLL is a key module to help the MEMS resonator realize an efficient and complete clock output [8]. For the MEMS-based resonator, the PLL can be connected to the back stage of the resonator output to process its frequency.

The mechanism by which phase-locked loops (PLLs) enhance the long-term stability of reference clocks can be analyzed through three key aspects: closed-loop control characteristics, noise suppression capabilities, and environmental adaptability.

The PLL continuously monitors the phase error between the local oscillator (LO) output and a high-stability external reference source using a phase detector [6,8]. This error signal, proportional to the phase difference, is converted into a corrective voltage through the loop filter, which drives the voltage-controlled oscillator (VCO) to adjust its output frequency. The dynamic behavior of this process is described by a second-order linearized model:

$${\displaystyle \frac{d^2{\theta }_{out}}{d{t}^2}}+2\zeta {\omega }_n{\displaystyle \frac{d{\theta }_{out}}{dt}}+{\omega }_n^2{\theta }_{out}={\omega }_n^2{\theta }_{ref}$$

(1)

where $\zeta$ is the damping coefficient and ${\omega }_n$ is the natural frequency. By optimizing the loop bandwidth, the PLL effectively suppresses low-frequency phase noise from the LO while tracking the long-term frequency drift of the external reference. PLLs employ multi-mode compensation mechanisms to mitigate environmental disturbances, such as temperature fluctuations and power supply variations. For temperature compensation, high-precision sensors integrated into the LO module collect real-time temperature data. These data are combined with pre-stored frequency–temperature characteristics (typically modeled as polynomials) in a lookup table (LUT) to generate a feedforward compensation voltage, which is superimposed onto the VCO control input. This approach significantly reduces thermally induced frequency drift. In 5G base stations, dual-reference PLL architectures (primary GPS + backup IEEE 1588) achieve inter-base station synchronization errors below ±50 ns when locked to GPS 1PPS signals. During GPS outages, the holdover mode maintains synchronization accuracy within ±100 ns over 24 h, complying with the stringent 3GPP TS 38.104 requirement (<1.5 μs) for TDD air interface synchronization. NASA’s Deep Space Atomic Clock (DSAC) project integrates a miniaturized cesium beam atomic clock with a wide-capture-range PLL, achieving a daily stability of 3 × 10⁻¹⁵ under extreme conditions (−40 °C to +70 °C and high radiation). This enables sub-meter ranging accuracy for deep-space missions. These examples demonstrate that PLLs, through the integration of high-stability references and adaptive compensation algorithms, have become a cornerstone technology for enhancing long-term clock stability in mission-critical systems.

In this paper, firstly, a capacitive disk MEMS resonator is designed. The mechanical modeling of the resonator is mapped to the electrical modeling according to the electromechanical mapping rules to obtain a macro-equivalent resonator electrical model. Secondly, a Pierce oscillator circuit is designed to generate a clock reference signal. Subsequently, a charge pump PLL is designed to connect to the back stage of the oscillator circuit to process its frequency.

2. Disc MEMS Resonator

2.1. Structure and Principle of the Resonator

The designed resonator consists of a disk made of polycrystalline silicon with a cylindrical rod suspended from the center of the disk, the circular rod being the critical point for the vibration of the radial profile mode. At the periphery of the capacitive gap d from the disk, there exist input and output electrodes that act as motor transducers. The principle of the resonator is shown in Figure 1.

By applying a sinusoidal voltage Vi with a frequency equal to the resonant frequency f₀ of the disk to the input electrodes and applying a DC bias voltage Vdc to the rod, the resonator is excited to vibrate in a forced manner, thus generating a time-varying radial electrostatic force Fe on the disk. Due to the symmetrical expansion and contraction of the disk around its edges, the capacitance from the disk to the output electrodes varies in time, thus creating an oscillating force of frequency f₀ in the loads connected to the output ports. A sinusoidal current io with frequency f₀ is generated in the load connected at the output port. The electrical signal at the input port is converted into a mechanical signal (force on the resonator), which is filtered by the mechanical response of the disk to give a sinusoidal displacement of the disk at f₀, which is converted back to the electrical domain by the output electrode.

2.2. Electromechanical Mapping

The dynamic mechanical behavior of the MEMS resonator can be represented by a mass–damper–spring system, as shown in Figure 2, in which the kinetic and potential energies of the MEMS resonator can be continuously converted to each other [9].

The total kinetic energy of disk vibration E_k is as follows:

$$E_k={\displaystyle \frac{1}{2}}\rho t{\int }_0^R{\int }_0^{2\pi }r{v}^2\left(r\right)drd\theta$$

(2)

where $v (r,\theta )$ is the velocity at any point $(r,\theta )$ on the disk, and hence, the expression for the effective mass of the disk vibration is as follows:

$$m_{eff}=\pi \rho t{R}^2\left[1-{\displaystyle \frac{J_0\left(hR\right)J_2\left(hR\right)}{{J_1\left(hR\right)}^2}}\right]$$

(3)

$$\mathrm{h}={\omega }_0\sqrt{{\displaystyle \frac{\rho }{\left(\frac{\mathrm{E}}{1+\sigma }\right)+\left(\frac{\mathrm{E}\sigma }{1-{\sigma }^2}\right)}}}={\displaystyle \frac{{\lambda }_{\mathrm{i}}}{\mathrm{R}}}$$

(4)

The effective spring stiffness $k_{eff}$ of the mechanical model of the resonator is related to the resonant frequency by the following relation:

$${\omega }_0=\sqrt{{\displaystyle \frac{k_{eff}}{m_{eff}}}}$$

(5)

In addition, the damping factor $b_{eff}$ that takes into account the energy loss of the system is given by the following equation [10]:

$$b_{eff}={\displaystyle \frac{{\omega }_0{m}_{eff}}{Q}}={\displaystyle \frac{\sqrt{k_{eff}{m}_{eff}}}{Q}}$$

(6)

A typical MEMS resonator can convert an input electrical signal into a force and apply it to the device, causing the micromechanical structure to be vibrated, which, in turn, is converted into an electrical signal by various transducer techniques. In order to model the MEMS resonator along with other actuator or sensing electronics, an electrical model of the MEMS resonator is required to simulate the entire system at the circuit level. The equivalent circuit of the MEMS resonator is often constructed by an analogy between an electrical and mechanical resonator, as shown in Figure 3. The electromechanical commutation at the two ports is modeled by a transformer with turns ratio n_k, and C₀ denotes the capacitance between each electrode and AC ground [11].

Elements of the model can be related to elements of the mechanistic model by using electromechanical analogies, as shown in

Table 1. Mapping between mechanical domain and electrical domain.

Mechanical Domin	Electrical Domain
Force, f	Voltage, v
$\mathrm{Velocity}, {\displaystyle \frac{\partial x}{\partial t}}$	Current, i
Displacement, x	Charge, q
$\mathrm{Compliance}, {\displaystyle \frac{1}{k_{eff}}}$	Capacitance, ce
$\mathrm{Mass}, m_{eff}$	Inductance, le
$\mathrm{Damping}, b_{eff}$	Resistance, re

.

That is, one can obtain the following equation:

$$l_e=m_{eff},r_e=b_{eff},c_e={\displaystyle \frac{1}{k_{eff}}}$$

(7)

$n_k$ is defined as the electromechanical coupling coefficient at the kth port and given by the following expression:

$$\begin{gathered} n_k=V_{dc}{\displaystyle \frac{\delta C_k}{\delta r}}=V_{dc}{\displaystyle \frac{\delta }{\delta r}}\left({\displaystyle \frac{\epsilon A_k}{d-r}}\right) \\ \approx V_{dc}\left({\displaystyle \frac{\epsilon A_k}{d^2}}\right)\left(r\ll d\right) \end{gathered}$$

(8)

$C_k$ denotes the capacitance between the disk and the kth electrode, $A_k$ is the coupling area given by φ_kRt, φ_k is the magnitude of the angle at which the electrodes overlap the disk, t is the thickness of the disk, d is the initial capacitance gap, ε is the dielectric constant of the material between the disk and the electrodes, and r is the lateral displacement [12].

To simplify the model, it is common practice to remove the transformer and scale the equivalent circuit values accordingly. A simplified equivalent circuit of the resonator can be obtained as shown in Figure 4, with the corresponding component values as follows [13]:

$$L_e={\displaystyle \frac{l_e}{n^2}},R_e={\displaystyle \frac{r_e}{n^2}},C_e=n^2{C}_e$$

(9)

2.3. Pierce Oscillator

The Pierce oscillator has remained one of the most common designs for quartz crystal oscillators, employing the crystal as part of the feedback circuitry to achieve oscillation. This configuration offers distinct advantages in terms of cost, size, complexity, and power consumption. As a result, it has been widely adopted as the preferred architecture for generating stable frequency oscillations in most embedded solutions and devices. For MEMS resonators, the Pierce oscillator circuit also demonstrates strong adaptability, stability, and high performance, making it a well-suited design choice. A typical Pierce oscillator configuration is illustrated in Figure 5a.

The Pierce oscillator topology comprises two core functional modules: an inverting stage and a frequency-selective network [14]. Its operational principle relies on energy transfer mechanisms in nonlinear systems, requiring specific design constraints to achieve sustained oscillation. The phase inversion mechanism is realized through two-stage 180° phase shifts: the first stage employs an inverting amplifier to reverse signal polarity, while the second stage generates additional phase shift through a feedback loop formed by external load capacitors and the crystal resonator. To maintain the inverting amplifier within its linear amplification region, a series feedback resistor Rf is implemented. This resistor forms a voltage divider network with the amplifier’s input impedance, collectively determining the DC operating point.

The oscillation initiation satisfies the Barkhausen criteria: the total loop phase shift must equal integer multiples of 360°, and the loop gain must exceed unity. The crystal resonator, combined with two external matching capacitors C₀, constitutes a bandpass filter. Precise adjustment of these capacitors (typically ranging from several picofarads to tens of picofarads) enables accurate frequency alignment with the nominal resonance. The feedback resistor Rf is optimized for stability and noise performance, typically exceeding 1 MΩ to minimize thermal noise degradation while ensuring sufficient DC bias stability. This architecture ensures energy coupling through impedance-matching networks, with the crystal resonator acting as the frequency-determining element whose equivalent reactance–frequency characteristics govern oscillation mode stability. The implemented Pierce oscillator circuit is illustrated in Figure 5b. The bias voltage VB is generated by the bias circuit. When the EN signal is low and the bias circuit operates normally, VB maintains a fixed voltage level that keeps the NMOS transistor in conduction. This configuration constrains the operating current in the inverting amplifier stage while ensuring sufficient current to sustain its proper functionality. The VB voltage equals the sum of the gate-source voltages (VGS) of transistors M3 through M5. This design effectively reduces the overall power consumption of the Pierce oscillator circuit.

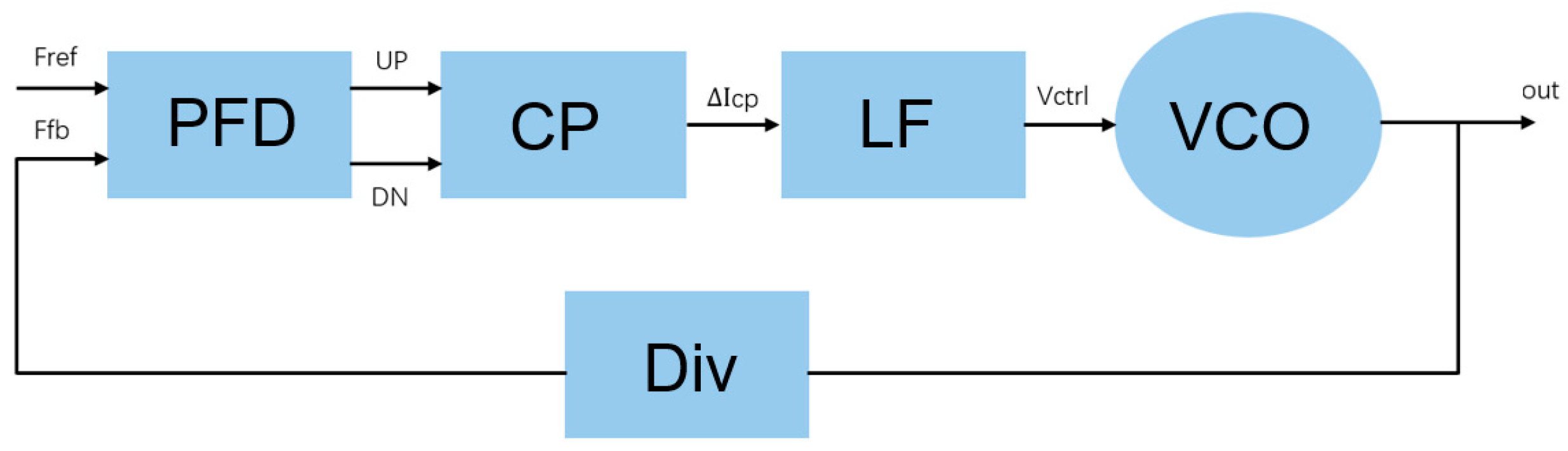
3. Charge Pump Phase-Locked Loop

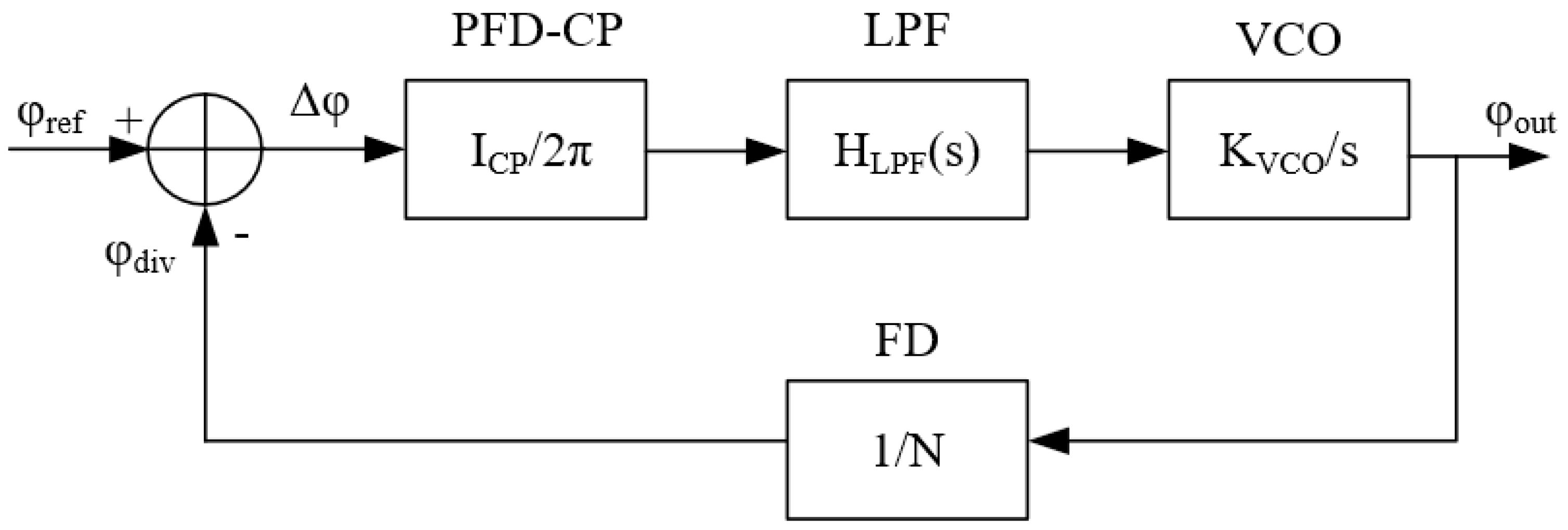
In the MEMS-based resonator, a phase-locked loop can be connected to the back stage of the resonator’s output to condition its frequency. The PLL is a key module to help the MEMS resonator achieve an efficient and complete clock reference. The basic model of the PLL consists of an LF (loop filter), a VCO (voltage-controlled oscillator), a PFD (phase frequency detector), a CP (charge pump), and a divider. These four components form a closed-loop control to lock the phase of the input signals. The PLL is often used as a multiplier, modulator, and demodulator to generate clock signals in communication systems [15]. These four components construct a closed-loop control to lock the phase of the input signal [16]. Figure 6 shows the block diagram of a phase-locked loop of a charge pump.

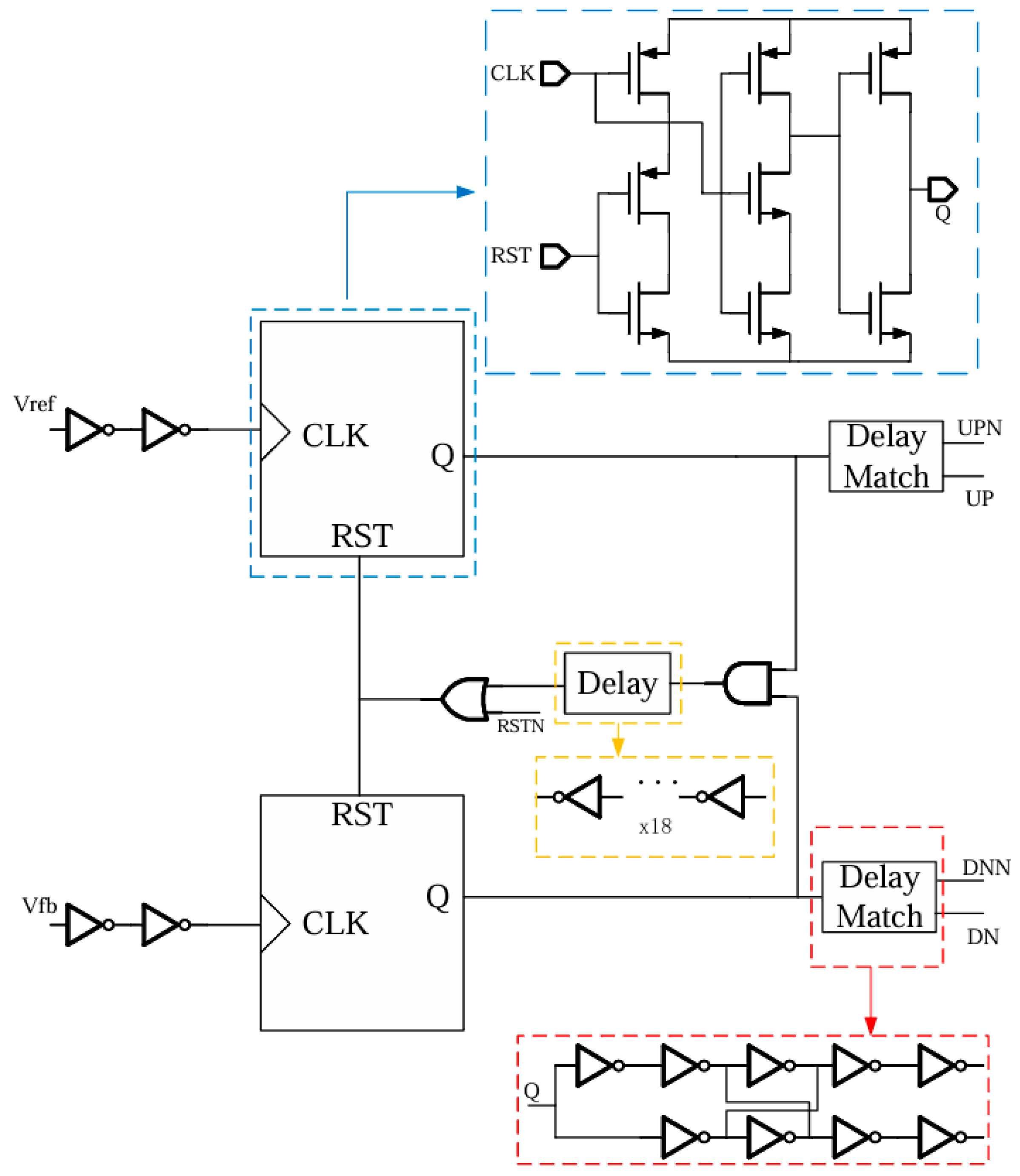
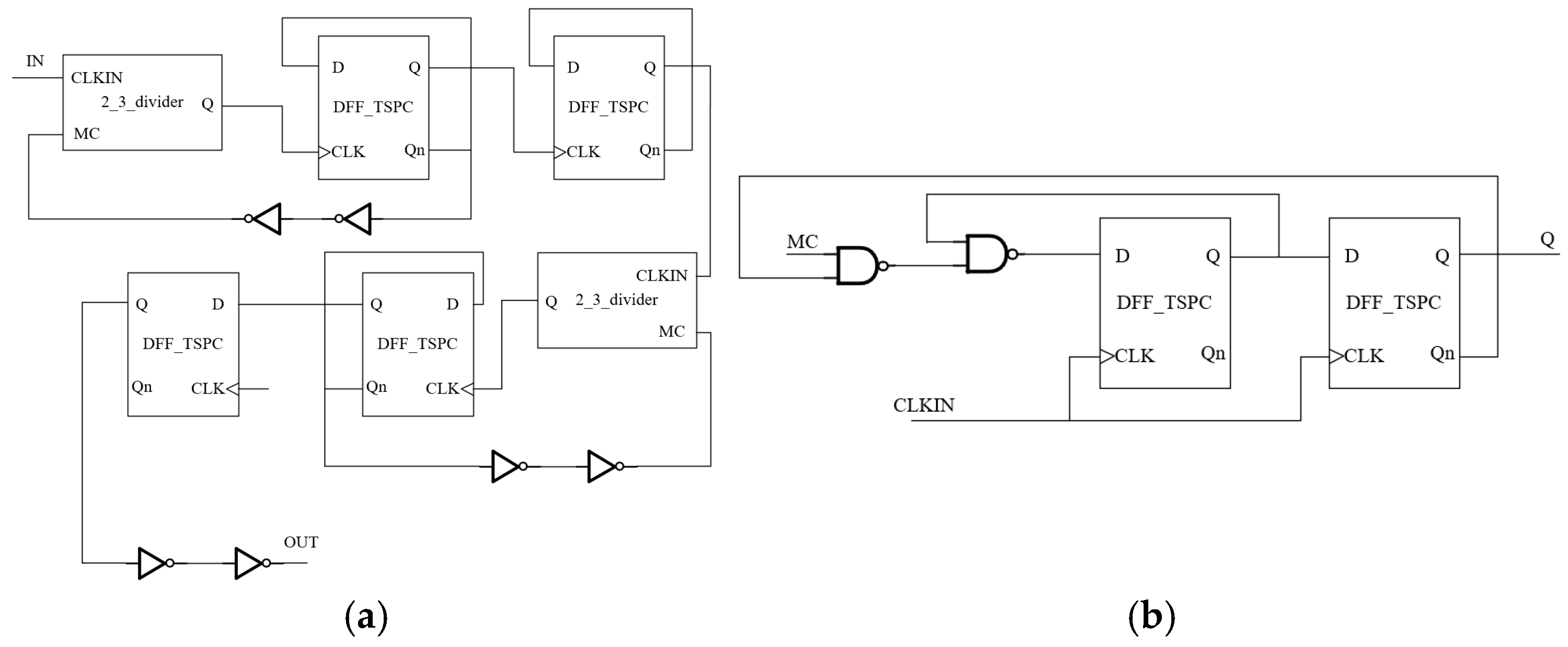
The frequency discriminator is an important module in the charge pump phase-locked loop (CPPLL), which realizes the output of charging and discharging pulse signals by comparing the frequency and phase of the reference signal and the crossover signal in the PLL. Figure 7 shows the circuit structure schematic diagram of the frequency discriminator used in this paper. The frequency discriminator is mainly composed of two TSPC (true single-phase clock) structures; the input reference signal Fref and the feedback input signal Fdiv are connected to the CLK signal terminal of the TSPC structure in the figure, and the output signals are the UP signal and the DN signal, respectively, which are used as inputs to the two reset terminals of the TSPC structure through an output signal after a gate, and the output signals are used as inputs to the two reset terminals of the TSPC structure.

Since the output of the PFD is a digital signal of the phase difference, it cannot directly control the VCO, so the charge pump module is introduced. The main function of the charge pump is to use the digital signal output from the PFD as a switch to control the positive and negative currents and to charge and discharge the capacitors in the loop low-pass filter at the later stage so as to convert the output signal of the PFD into a variable voltage signal and to modulate the frequency of the VCO. In this design, a source level switched charge pump with a common source and common gate and operational amplifier is used, and the circuit results are shown in Figure 8.

The simulation study of the charge pump circuit focused on the current matching characteristics between its charging and discharging branches. A DC sweep analysis was conducted by scanning the output voltage vcpout from 0 V to 1.2 V, with a detailed investigation of the current deviation between the charging current Iup and discharging current Idn within the operational range of 0.2 V to 1 V. The simulation results, presented in Figure 8b, reveal that, across the output voltage range of 0–1.1 V, the relative current mismatch ratio—defined as the difference between Iup and Idn normalized to their average magnitude—measures approximately 0.026%. This quantifies the exceptional charge/discharge current matching performance of the proposed charge pump design, demonstrating its capability to maintain balanced current symmetry under varying output conditions. The sub-0.03% mismatch ratio validates the effectiveness of the circuit topology in minimizing systematic errors inherent to conventional charge pump architectures.

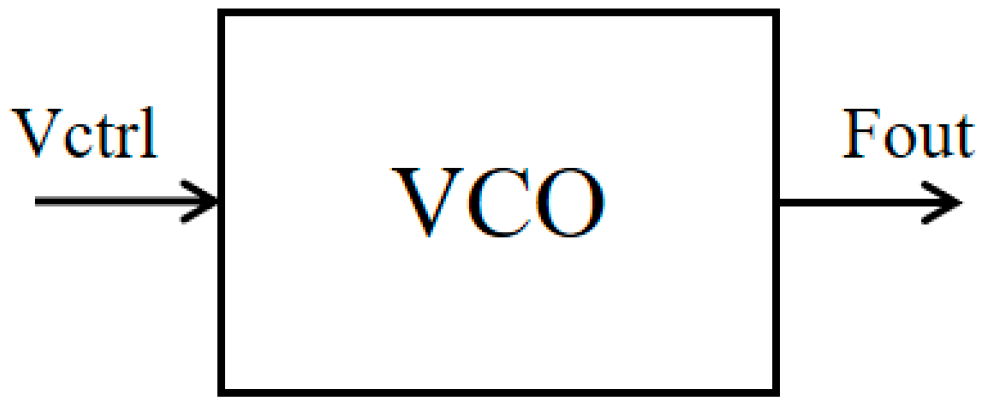
The voltage-controlled oscillator, as a module of the charge pump phase-locked loop to generate the output signal, is shown in Figure 9, and its output, the frequency of the signal Fout, is controlled by the output voltage Vctrl of the previous loop filter circuit.

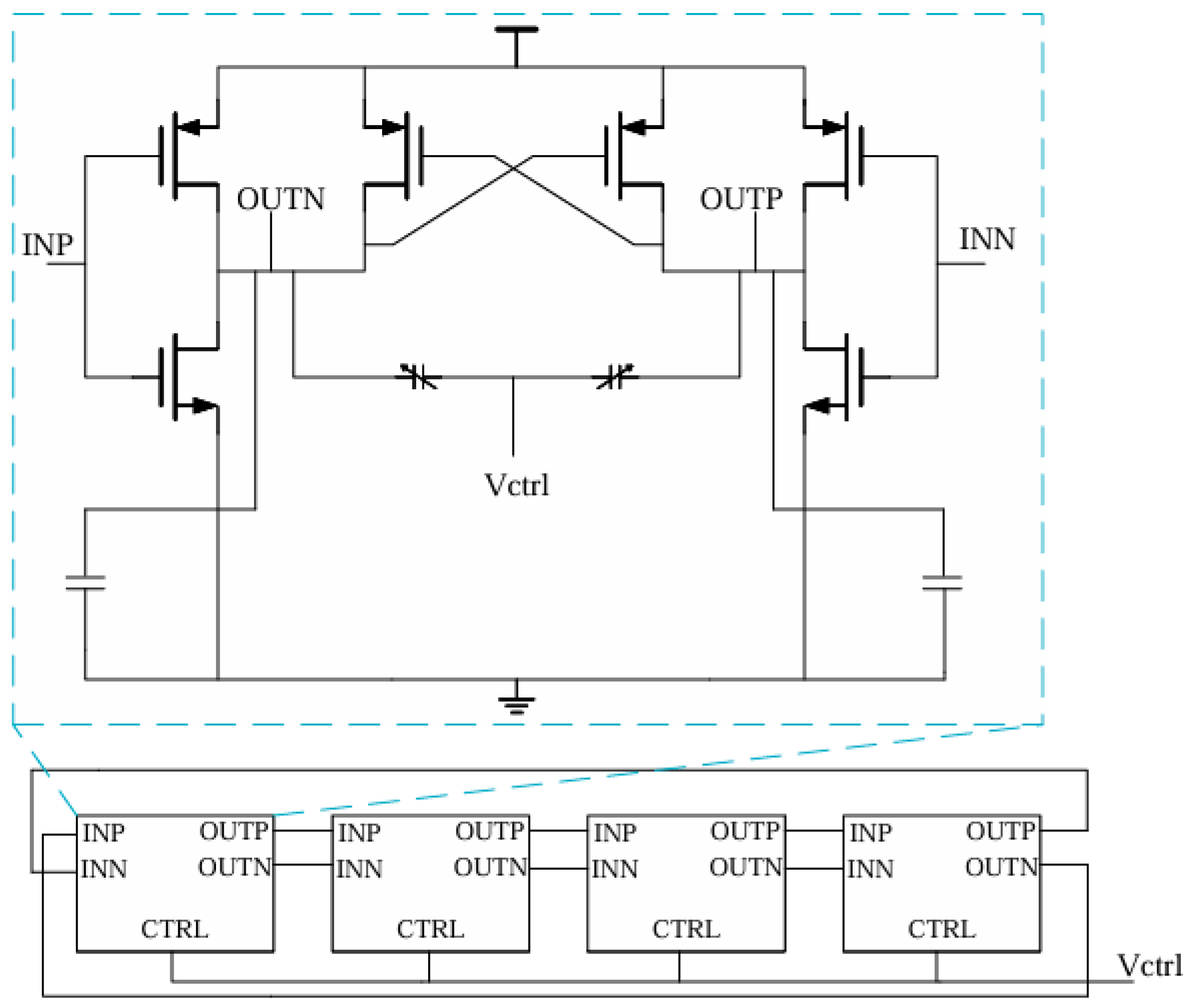
The voltage-controlled oscillator designed in this paper adopts a differential annular voltage-controlled oscillator structure, and the specific circuit is shown in Figure 10.

Figure 11 shows the frequency sweep simulation and the phase noise simulation results of the voltage-controlled oscillator. According to the simulation diagram, the lowest frequency is 695 MHz, and the highest frequency is 1 GHz, that is, the tuning range of the signal frequency is 695 MH–1 GHz, and the linearity of the voltage-controlled oscillator in this paper is good. The phase noise is −98.7 dBc/Hz@1 MHz, and the voltage-controlled oscillator circuit designed in this paper has good noise performance.

In charge pump phase-locked loops, the frequency of the voltage-controlled oscillator output signal Fout is significantly higher than that of the reference signal Fref. Consequently, a frequency divider is employed to down-divide Fout. The divider circuit operates by reducing the frequency of the VCO’s high-speed clock signal and feeding the divided signal back to the phase-frequency detector. This feedback loop continuously adjusts the VCO frequency until the phase difference between the divided clock and the reference clock converges to zero, achieving a phase lock in the PLL system. The closed-loop mechanism ensures precise frequency synchronization through iterative error correction, where the divider ratio directly determines the final locked frequency relationship Fout = N⋅Fref, with N representing the programmable division factor.

The crossover designed in this paper adopts an integer divider based on the 2_3 divider, and the specific structure is shown in Figure 12a. Figure 13 shows the transient simulation results of the frequency divider, which shows that the input frequency of the frequency divider is 800 MHz and the output frequency is 8 MHz, and the design circuit realizes a 100:1 frequency divider.

3.1. Simulation Results of Charge Pump Phase-Locked Loop

Figure 14 illustrates the linearized model of the charge pump phase-locked loop. Based on the linear approximations of individual modules (phase-frequency detector, charge pump, loop filter, and voltage-controlled oscillator), the loop gain transfer function H(s) of the PLL system can be derived as follows:

$$H_{loop}\left(s\right)={\displaystyle \frac{{\phi }_{div}}{{\phi }_{ref}}}={\displaystyle \frac{1}{N}}{\displaystyle \frac{I_{CP}}{2\pi }}{H}_{LPF}\left(s\right){\displaystyle \frac{K_{VCO}}{s}}$$

(10)

The phase-locked loop employs a second-order low-pass loop filter, thereby implementing a third-order charge pump phase-locked loop (CPPLL) architecture. This configuration enables the following:

$$H_{loop}\left(s\right)={\displaystyle \frac{{\phi }_{div}}{{\phi }_{ref}}}={\displaystyle \frac{1}{N}}{\displaystyle \frac{I_{CP}}{2\pi }}{\displaystyle \frac{s+\frac{1}{R_1{C}_1}}{s+\frac{C_1+C_2}{R_1{C}_1{C}_2}}}{\displaystyle \frac{K_{VCO}}{s}}$$

(11)

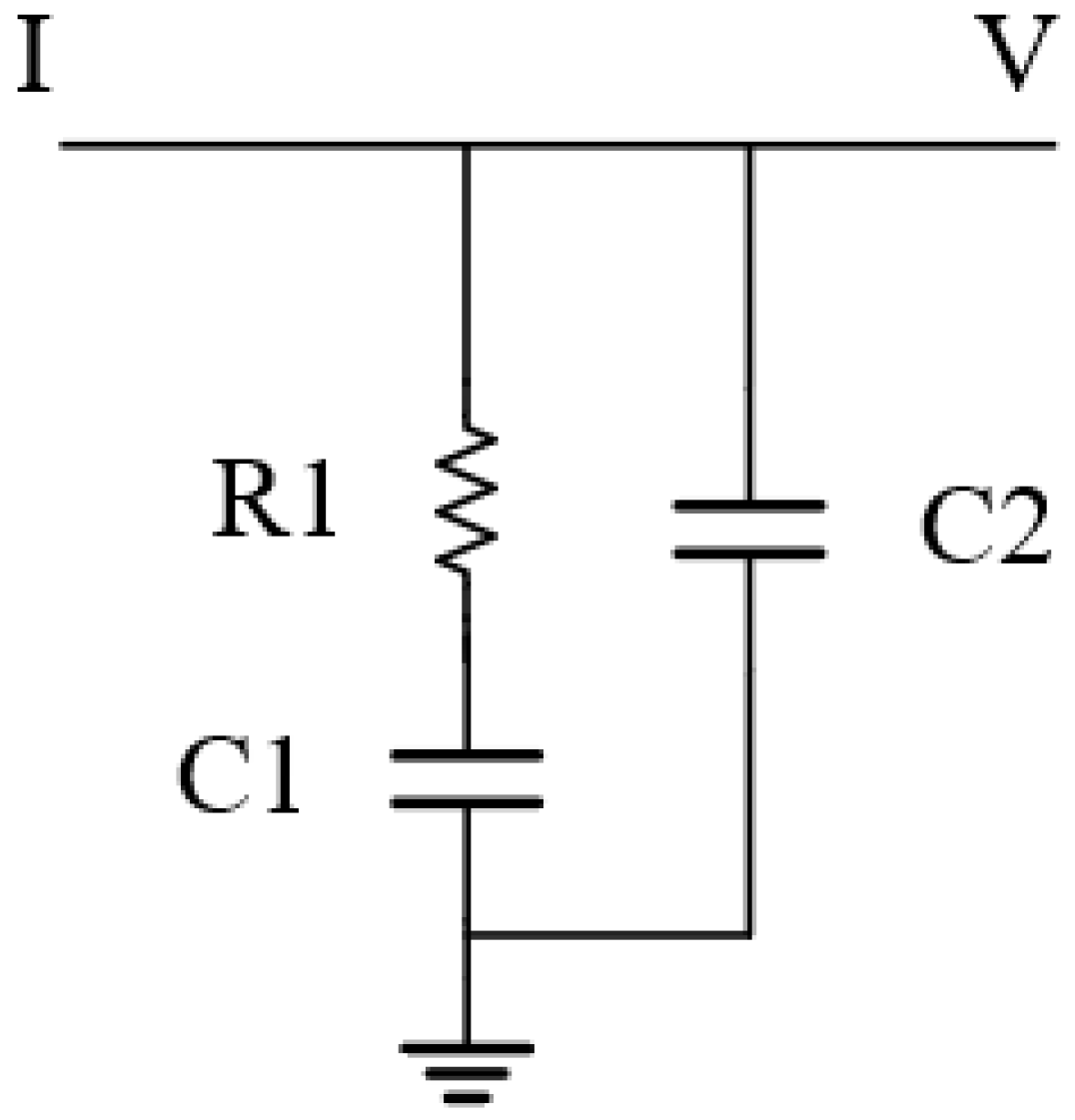
The resistor and capacitor values of the low-pass filter can be determined using the derived pole-zero locations and the maximum phase margin criterion. This method systematically optimizes the filter components to achieve target stability specifications while maintaining the desired closed-loop dynamics in the phase-locked loop system. Figure 15 shows the 2nd order low-pass filter.

$$C_2={\displaystyle \frac{I_{CP}{K}_{VCO}}{N}}{\displaystyle \frac{1}{{{\omega }_c}^2\zeta }}$$

(12)

$$C_1=C_2\left({\zeta }^2-1\right)$$

(13)

$$R_1={\displaystyle \frac{\zeta }{C_2{\omega }_c\left({\zeta }^2-1\right)}}$$

(14)

$$\zeta =\tan \left({∆\varphi }_{MAX}\right)+\sec \left({∆\varphi }_{MAX}\right)$$

(15)

$$\begin{gathered} {∆\varphi }_{MAX}={\left.∆\varphi \right|}_{\omega ={\omega }_c}=\arctan \left({\displaystyle \frac{{\omega }_c}{{\omega }_z}}\right)-\arctan \left({\displaystyle \frac{{\omega }_c}{{\omega }_p}}\right)+180° \\ =\arctan \left({\displaystyle \frac{\frac{{\omega }_c}{{\omega }_z}-\frac{{\omega }_c}{{\omega }_p}}{1+\raisebox{1ex}{${{\omega }_c}^2$}\!\left/ \!\raisebox{-1ex}{${\omega }_z{\omega }_p$}\right.}}\right)+180° \end{gathered}$$

(16)

Among them, ${\omega }_z$, ${\omega }_p$, and ${\omega }_c$ are the zero point, the pole point, and the oop bandwidth separately.

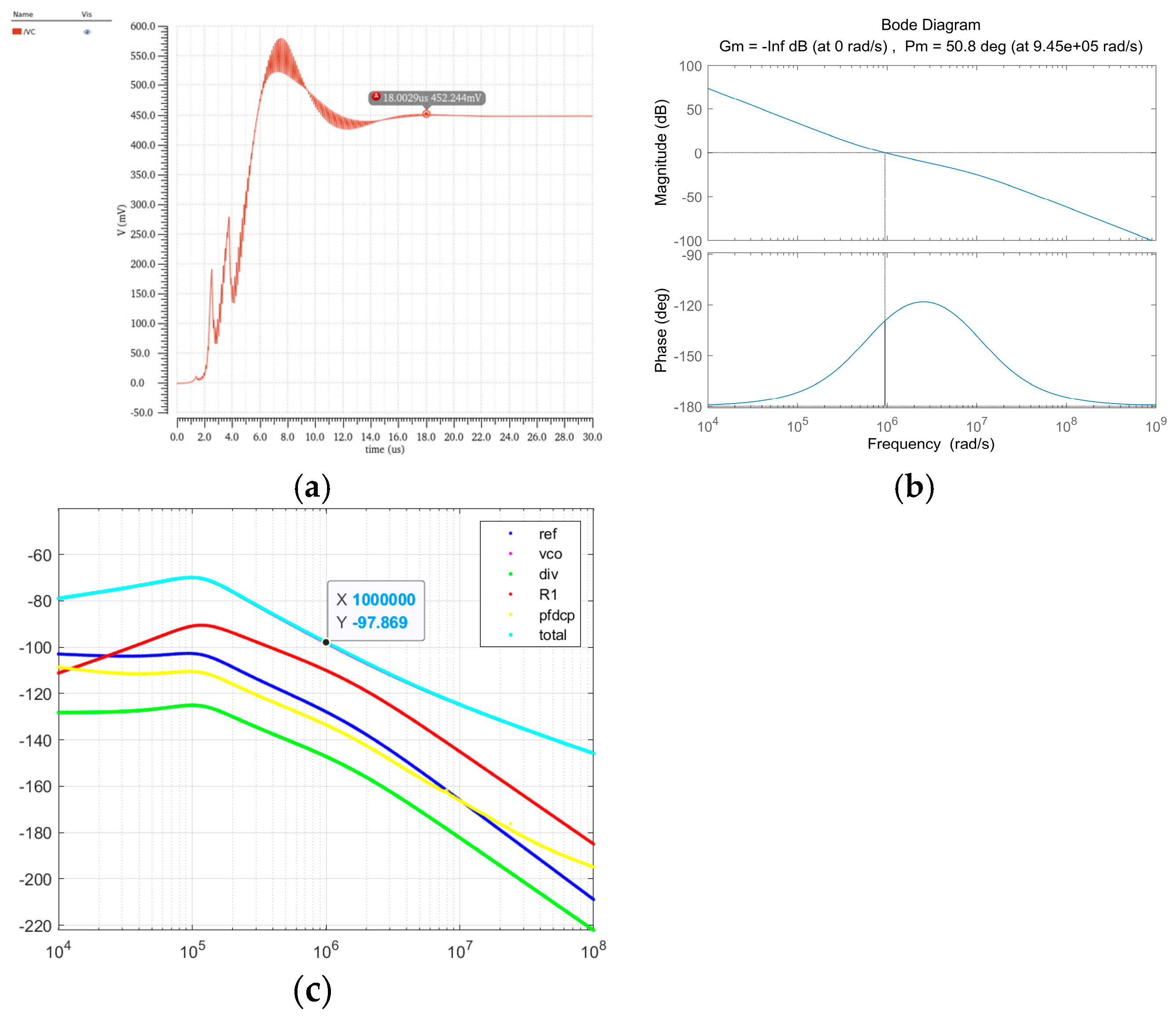
A transient simulation analysis was conducted on the complete charge pump phase-locked loop circuit. Figure 16 presents the simulated locking process, demonstrating that the VCO control voltage Vctrl stabilizes at approximately 16 μs, with the CPPLL achieving phase lock after 18 μs. The system was modeled in MATLAB 2024 with the following parameters: reference frequency Fref = 8 MHz, charge pump current ICP = 20 μA, VCO gain KVCO = 400 MHz/V, and frequency division ratio N = 100. The simulated Bode plot in Figure 16 reveals a loop bandwidth of 9.45 × 10⁵ rad/s with a phase margin of 50.8, meeting stability requirements.

For precise phase noise characterization, systematic simulations were performed to evaluate noise contributions from individual components. Noise profiles of the phase-frequency detector, charge pump, loop filter, and VCO were extracted to construct a comprehensive noise transfer model. Using MATLAB’s phase noise fitting toolkit, the synthesized phase noise spectrum was obtained as shown in Figure 16. The CPPLL exhibits an equivalent output phase noise of −98 dBc/Hz at 1 MHz offset, with detailed noise contributions from each subsystem clearly delineated across the frequency range. This hierarchical analysis methodology enables targeted optimization of dominant noise sources while maintaining loop stability constraints.

3.2. Principle of Charge Pump Phase-Locked Loop Circuit for Frequency Conditioning of MEMS Oscillators

The operational principle of this architecture converts the algorithmically derived frequency compensation quantity into a voltage signal through a frequency-to-voltage converter (FVC). This voltage is then summed with the output of the charge pump phase-locked loop low-pass filter via an analog summation circuit. The resulting composite voltage Vctrl dynamically controls the voltage-controlled oscillator, thereby generating a frequency output signal with precise compensation. This closed-loop configuration ensures continuous frequency stabilization by adaptively adjusting the VCO control voltage through the superimposed compensation component. 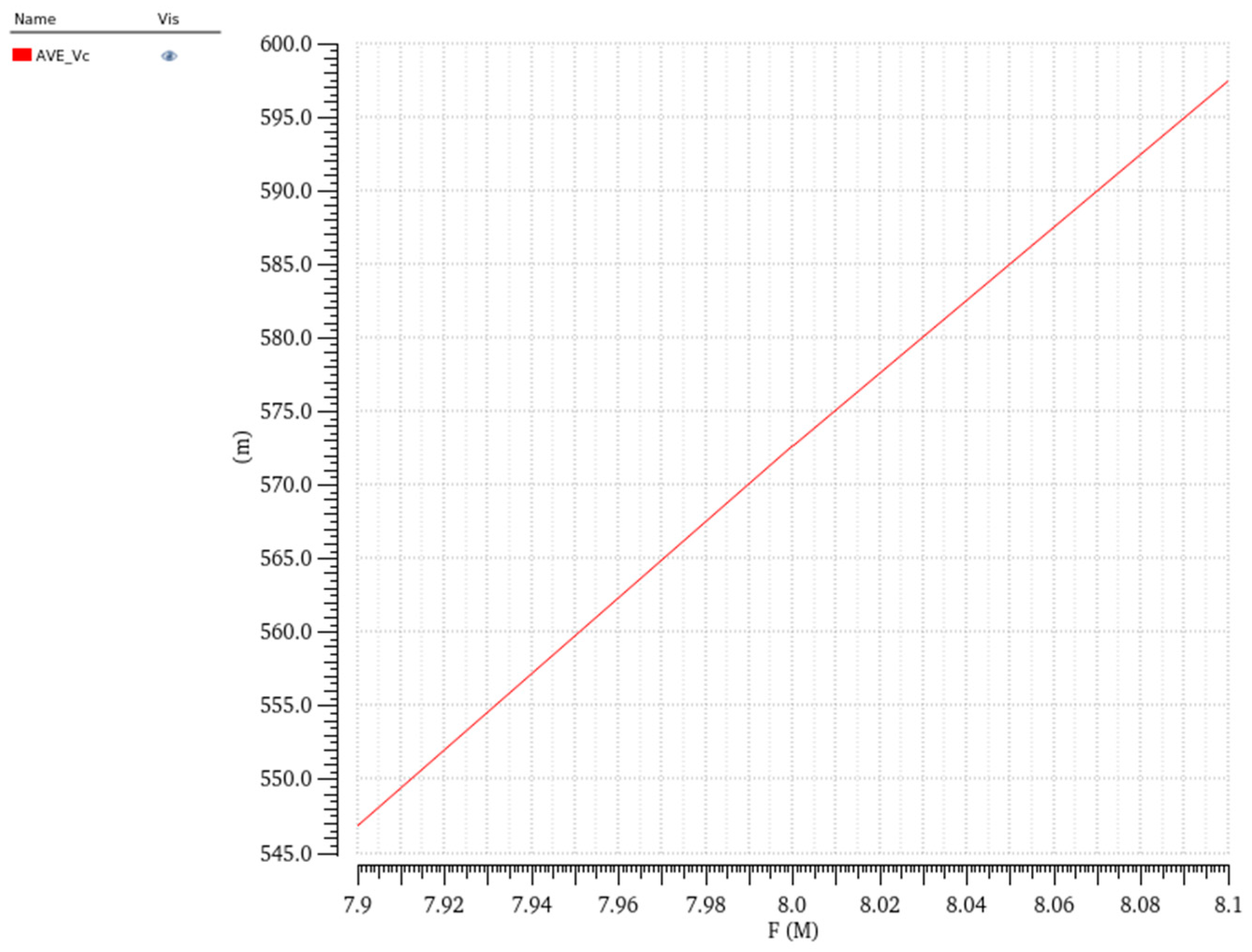The frequency-to-voltage conversion circuit architecture is illustrated in Figure 17b. This design employs an inverse phase-locked loop configuration to implement frequency–voltage conversion. By utilizing the tuning voltage of the voltage-controlled oscillator as the system output, a direct mapping relationship between input frequency and output voltage level is established. A phase-frequency detector monitors the phase difference between the input signal and the VCO feedback signal, generating output pulses whose width is proportional to frequency error, thereby achieving a quantitative measurement of the frequency deviation. These digital pulses from the PFD are subsequently converted into analog voltage signals through a low-pass filter, which attenuates high-frequency components to produce a smoothed tuning voltage. The system employs a closed-loop feedback mechanism to automatically adjust the VCO tuning voltage for frequency error cancellation. When input frequency variations occur, the loop dynamically regulates the output voltage level, ultimately establishing a linear correspondence between input frequency and output voltage at steady-state operation. The simulation results of the FVC are presented in Figure 18. As demonstrated by these results, the circuit architecture exhibits a strong linear relationship between the output voltage and input frequency. This characteristic enables effective conversion of the frequency compensation quantity into corresponding control voltage increments, thereby precisely regulating the final output frequency through closed-loop adjustment. The linear transfer function validates the circuit’s capability to maintain proportional frequency correction across the operational bandwidth.

The effect of temperature changes on the output frequency is shown as follows. Figure 19 shows the Frequency and temperature sweep simulation result of the CPPLL.

Table 2. Output frequencies at different temperatures.

Temperature (°C)	f (MHz)
−25	778.658
−15	782.968
−5	787.246
5	791.154
15	795.514
25	799.603
35	803.874
45	807.654
55	811.984
65	816.289
75	821.564

shows the output frequency results at different temperatures.

Let f_MEMS denote the output frequency of the MEMS resonator, f_CPPLL represent the output frequency of the charge pump phase-locked loop, and T indicate temperature. Under ideal conditions, where the MEMS resonator’s output frequency serves as the CPPLL’s input reference frequency, the relationship between these frequencies is governed by the following:

$$f_{CPPLL}=N·f_{IN}=N·f_{MEMS}$$

(17)

In this phase-locked loop configuration with N = 100, taking 25 °C as the reference temperature, the input reference frequency to the charge pump PLL becomes the temperature-dependent MEMS resonant frequency f_MEMS(T₀) at temperature T₀. The temperature–frequency relationship of the MEMS resonator–PLL system was modeled through MATLAB curve fitting, yielding the following empirical expression:

$$F_{out}\left(T_0\right)=100\left(-237T_0+\mathrm{8,007,000}\right)+\mathrm{418,700}\left(T_0-25\right)=\mathrm{395,000}{T}_0+\mathrm{790,232,500}$$

(18)

The required frequency compensation relative to the reference frequency at 25 °C is given by the following:

$$∆F=F_{out}\left(T_0\right)-F_{out}\left(25\right)$$

(19)

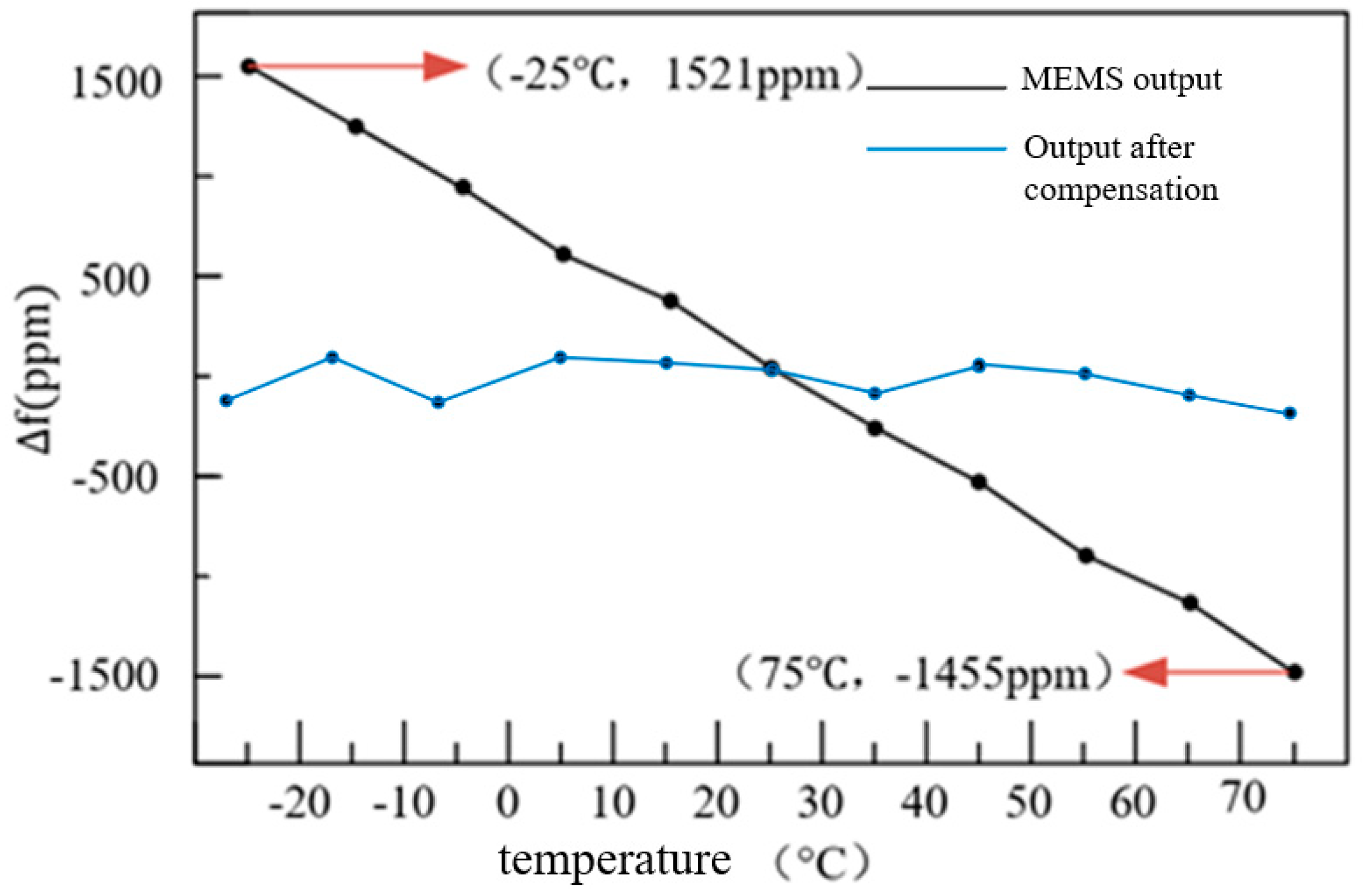
Temperature compensation simulations were conducted across the thermal range of −25 °C to +75 °C using 10 °C increments as test points. At each temperature, the algorithmically determined frequency compensation quantity was applied through the aforementioned circuit architecture. The compensated system output frequencies are tabulated in

Table 3. The results of the compensated circuit simulation data.

Simulation Temperature (°C)	Resonant Frequency (MHz)	Frequency Offset (f0 = f (25 °C)) (ppm)
−25	799.542	−76
−15	799.665	78
−5	799.514	−111
5	799.658	69
15	799.642	49
25	799.603	—
35	799.549	−68
45	799.670	84
55	799.654	64
65	799.585	−23
75	799.556	−59

, while the corresponding post-compensation frequency deviation characteristics are plotted in Figure 20.

The graphical data demonstrate significant improvement in frequency–temperature stability after implementing the algorithmically derived frequency compensation calibration. Compared to the uncompensated MEMS resonator’s frequency drift, the compensated system reduces output frequency deviation to an average of 76 ppm across the operational temperature range of −25 °C to +75 °C.

4. Conclusions

In this paper, a MEMS disk resonator and a charge pump PLL are designed. In the MEMS resonator, it is firstly electromechanically mapped, and then, a Pierce oscillation circuit is designed. A charge pump PLL is designed to condition the output frequency of the MEMS resonator. The experimental results show that the method can enhance the frequency stability of the designed MEMS resonator. A feasible solution for the frequency processing of the MEMS resonator is proposed and verified in this paper.