Abstract
The synergistic interaction between emergency management departments and enterprises constitutes a fundamental mechanism for mitigating the risks of technological accidents caused by natural disasters (Natech). The efficacy of this collaborative approach is impacted by comprehensive risk analyses and the game between government and enterprise. Predicated on these premises, the evolutionary game analysis of government–enterprise collaboration in coping with Natech risk was carried out. Firstly, an evolutionary game model of government–enterprise collaboration in coping with Natech risk was constructed. Secondly, the evolutionary stability strategy (ESS) was developed. Finally, these strategies were substantiated through numerical simulations. The findings revealed that at lower levels of Natech risk, enterprises will choose low investment in coping capacity building, and emergency management departments will choose delayed disposal. Under moderate Natech risk, enterprises will increase their investments if emergency management departments persist with delayed strategies, and emergency management departments will react proactively if enterprises persist with low investment. Under a high Natech risk, a pattern of strategic misalignment emerges between the two entities. This study contributes a theoretical basis for the optimization of government–enterprise collaboration in coping with Natech risks.
1. Introduction
The safety of enterprise production operations is influenced not only by inherent system risks but also by the adverse effects of natural disasters. Technological accidents instigated by natural disasters, known as Natech events, often result in substantial casualties, significant economic damage, environmental pollution, and widespread social disruption [,]. For instance, the 2011 Tohoku earthquake and subsequent tsunami in Japan precipitated catastrophic radiation leaks at the Fukushima Daiichi nuclear power station; in 2019, excessive rainfall led to the rupture of a tailings dam at a Russian gold mine, severely contaminating the Seiba River; and in 2021, a torrential downpour in Zhengzhou, Henan province, inundated the subway system, trapping 967 individuals and culminating in the death of 14 people. Amidst the increasing occurrence of extreme disasters, enhancing the resilience to Natech risks poses a critical contemporary challenge [].
The intricacy and severe implications of Natech incidents necessitate that both governments and enterprises develop effective collaborative strategies [,], underscored by the need for more comprehensive disaster mitigation and response plans []. The formulation of a cooperation strategy enhances the initiative, coordination, and adaptability of both government and enterprises in addressing Natech risks. This strategy promotes resource sharing, complementary advantages, and increased trust, thereby improving overall coping abilities. Given the complexity and impact characteristics of Natech events, it is essential for the government and enterprises to jointly develop cooperation strategies and conduct joint exercises based on risk analysis and policy frameworks. For instance, in China’s emergency management practice, the establishment of various levels and types of emergency plans has laid a solid foundation for the prevention and control of Natech incidents. When a disaster warning is issued, the government and enterprises activate an emergency response according to the emergency plan, maintaining information communication and coordination to jointly manage the uncertainties presented by the disaster scenario. Risk analysis forms the foundation of such efficient collaborative actions between governments and enterprises. However, the consideration of natural disaster risks to technological systems remains inadequately addressed in many regions []. For instance, nearly half of the member states in the European Union failed to sufficiently incorporate Natech risks in their industrial facility risk assessments []; in China’s surveys of natural disaster risks, consideration has been only given to the mining industry. Given Natech events’ low probability yet high-impact nature, they often surpass the disaster preparedness and response capacities of local authorities and enterprises [], leading to suboptimal enterprise responsiveness and delayed governmental action. In addressing these challenges, numerous scholars have conducted risk analyses on Natech, including the exploration of risk evolution factors [,], assessment of probabilities [], risk quantification [,,,], and analysis of historical data [,,], thereby furnishing a scientific basis for enhancing Natech risk reduction and fostering government–enterprise collaboration.
In practice, the challenge of fostering collaboration between government and enterprises in response to Natech risks extends beyond mere risk analysis to encompass the strategic interplay between these entities. So, under this interactive relationship, how can government and enterprises effectively coordinate to deal with Natech risks? Many current studies on emergency collaboration focus on relevant issues from multiple perspectives, such as digital-intelligence technology drive, organizational network, evolutionary game, etc., although the research scenario is not Natech.
- (1)
- Digital-intelligence-driven emergency collaboration aims to improve the multi-agent cooperation ability in responding to emergencies through the integration and application of digital and intelligent technologies. The existing research is conducted through two paradigms of “it is” and “it should be”. For example, based on the general model of safety information cognition, Zhang et al. built a big data-driven emergency information collaboration mechanism with a power mechanism, operation mechanism, and action mechanism as the core []. By analyzing typical cases, Zhang et al. made it clear that the construction of a multi-channel emergency rescue information smooth mechanism could promote the diversification of knowledge transfer methods, and the construction of an emergency rescue information integration mechanism based on a digital platform could enhance the knowledge integration ability, thus improving the performance level of emergency management [].
- (2)
- Organizational network analysis is an important way to reflect the effectiveness of emergency collaboration. Existing studies were carried out through the horizontal comparison of time slices and the longitudinal comparison across cases. For example, Chen et al. found the trend or rule of emergency collaboration by comparing the collaboration network of emergency organizations in Wenchuan, Yushu, Lushan, and Ludian earthquick in China []. Yang et al. analyzed the collaboration network of emergency organizations in different stages of COVID-19 prevention and control in Wuhan to identify the characteristics of collaboration at different stages and the evolutionary mechanism of emergency collaboration [].
- (3)
- The dynamics of government–enterprises interaction have also been explored through the lens of evolutionary game theory, which examines various facets of disaster management collaboration. These aspects include enterprise engagement in disaster management [], governmental mobilization of enterprise resources post-disaster [], corporate involvement in emergency rescue operations [], contributions to post-disaster reconstruction [], government and enterprises’ collaborative governance [,], and the provision of emergency supplies by enterprises [,].
In addition, studies have analyzed the defects of government–enterprise collaboration and proposed optimization measures []. Through the above research, it is found that network analysis is more useful for analyzing the characteristics and rules of emergency collaboration from the overall perspective, and the digital-intelligence technology-driven research aims to optimize emergency collaboration through the innovation of the emergency collaboration mechanism, while the evolutionary game analyzes the interaction mechanism between subjects from a micro-perspective, which can fundamentally promote the optimization of emergency collaboration. This study also aims to reveal the emergency collaboration mechanism between the government and enterprises in coping with Natech risks from the micro-level. While extensive research has been conducted on the government–enterprise collaborative evolutionary game within disaster management frameworks, there remains a gap in understanding the evolutionary mechanisms underpinning such collaborative behaviors specifically from a disaster risk perspective. This study aims to bridge this gap by conducting an evolutionary game analysis of government–enterprise collaboration in coping with Natech risks, thereby providing a theoretical foundation for elucidating the failure phenomenon of government–enterprise collaboration in coping with Natech risks.
Building on the framework established for the evolutionary game model of government–enterprise collaboration in addressing Natech risks (Section 2), this study will delineate the evolutionary stable strategies (ESSs) for such collaborative behaviors (Section 3). Subsequently, numerical simulations will be conducted to test the theoretical model (Section 4). The findings will be thoroughly discussed in Section 5.
2. Establishment of an Evolutionary Game Model of Government–Enterprise Collaboration in Coping with Natech Risks
2.1. Theoretical Model
The impact of natural disasters on the enterprise technology system will induce the occurrence of Natech. To prevent the occurrence of Natech, enterprises and emergency management departments need to coordinate responses based on rigorous risk analysis. Natech risk analysis aims to identify and evaluate the likelihood of damage to technological facilities and the potential accidents that natural disasters such as earthquakes, floods, and hurricanes may provoke, along with their probable impacts [,,,]. The process begins by pinpointing facilities susceptible to technological accidents in the wake of natural disasters, including chemical plants, oil refineries, and nuclear power stations. It then involves assessing the vulnerability of these critical infrastructures to specific natural disasters, examining aspects, such as facility design, maintenance status, safety systems, and emergency preparedness. Furthermore, the analysis extends to evaluating the potential repercussions of technological accidents on human health, the environment, socio-economic factors, and public safety. Finally, by integrating the probabilities of natural disasters and the initiation of technological accidents, along with the extent of damage these accidents might cause, a comprehensive risk assessment is conducted. The significance of Natech risk analysis lies in its capacity to enhance the understanding and preparedness of both government and enterprises for the complex and interconnected impacts of natural disasters, thereby facilitating more effective resource allocation, refining emergency response strategies, minimizing potential economic losses, and safeguarding human lives.
Enterprises are the primary agents responsible for maintaining the safety of technical systems, and they need to invest in developing Natech risk management capabilities. This investment includes forming emergency response teams, stocking emergency supplies, allocating emergency equipment, and devising emergency response plans. The extent of investment in these capabilities directly influences the effectiveness of an enterprise’s response to emergencies. In China’s disaster management framework, the government has stipulated guidelines for building enterprise emergency capacities. For instance, production and business units and other social forces are encouraged to establish emergency rescue teams, equipping them with appropriate emergency rescue equipment and materials and raising the level of specialization in emergency rescue []. The enterprises mentioned above mainly refer to key enterprises such as chemical enterprises, nuclear enterprises, and metro groups, which are the focus and core of emergency management. However, compared with the detailed regulations on production safety, the government’s directives on emergency preparedness for enterprises lack specificity, granting enterprises considerable leeway in their approach. Consequently, the outcomes of Natech risk analysis significantly guide enterprise decisions regarding investments in capacity enhancement.
In the face of natural disasters and potential subsequent Natech incidents, emergency management departments are required to act swiftly and orchestrate a range of emergency response resources. This orchestration entails the distribution of emergency materials and equipment and the deployment of emergency response teams. The timeliness of the emergency management department’s response is critical in preventing or mitigating Natech incidents. Particularly during natural disasters, which typically feature multiple simultaneous outbreaks, emergency management must select the most effective strategy based on the results of Natech risk analysis, opting either for active or delayed disposal []. Active disposal includes preliminary risk assessments, enhancing emergency preparedness, and conducting investigations into potential hazards. Conversely, delayed disposal is initiated upon receiving crisis reports and focuses on managing and recovering from events that have already unfolded. Active measures prioritize prevention and preparedness to lessen the impact of disasters by mitigating risks, whereas delayed measures concentrate on effective post-event management and recovery. Notably, proactive approaches are generally more effective at reducing Natech risk.
Stakeholder’s interests often impede the formation of a consensus between the government and enterprises regarding coordinated responses to Natech incidents. Emergency management departments prioritize public safety, whereas enterprises are typically more concerned with economic efficiency and operational costs. For instance, enterprises may be reluctant to invest in safety infrastructure or implement costly preventative measures due to financial considerations. Furthermore, the delineation of responsibilities and duties in Natech prevention and response is frequently ambiguous []. This lack of clarity can result in parties attributing greater responsibility to one another during the planning and execution of disaster prevention and response strategies.
Drawing on the preceding analysis, a theoretical model of government–enterprise collaborative behavior in response to Natech risks is developed, as depicted in Figure 1. This model serves as the foundation for constructing an interest matrix that underpins the strategic behavior of government–enterprise collaboration aimed at mitigating Natech risks.
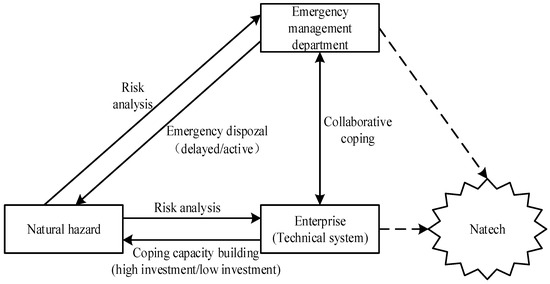
Figure 1.
Theoretical model of government–enterprise collaboration in coping with Natech risk.
2.2. Interest Matrix Construction of Government–Enterprise Collaboration in Coping with Natech Risk
Within the framework of information asymmetry and opportunism, enterprises and emergency management departments are perceived as strategic players possessing bounded rationality []. Enterprises can choose between two strategic options: high investment in coping capability building or low investment. Similarly, emergency management departments can opt for either active or delayed disposal strategies. Given the sporadic nature of disaster risks, technical accidents are more likely to occur when emergency response of emergency management departments is delayed and enterprises have opted for minimal investment in their coping capabilities.
Based on the above assumptions, we assume that the high investment in coping capacity building of the enterprise is , the low investment in coping capacity building is , the investment in emergency management department’s active disposal is , the production income of the enterprise when Natech does not occur is , the social welfare obtained by the emergency management departments when Natech does not occur is , the loss caused to the enterprise by the occurrence of Natech is , the loss caused by the negative impact of Natech on emergency management departments is , the probability of enterprises investing in high coping capacity building is , the probability of emergency management departments taking the initiative to deal with it is , and the probability of Natech occurrence is . The game interest matrix between enterprises and emergency management departments is shown in Table 1.

Table 1.
Interest matrix of government–enterprise collaboration in coping with Natech risk.
3. The Solution of ESS of Government–Enterprise Collaboration in Coping with Natech Risk
3.1. Revenue Expectation Function Construction
According to Table 1, the expected returns and average expected returns of enterprises with high and low investment in coping capacity building are as follows:
The expected returns and average expected returns of active and delayed disposal of emergency management departments are as follows:
Under the replicator dynamic thinking, the replication dynamic equations of government–enterprise collaboration in coping with Natech risk are as follows:
3.2. ESS Analysis
3.2.1. ESS Analysis of Enterprise
Find the first partial derivative with respect to x of the replication dynamic equation F(x,y) of the firm in Equation (7):
Let 0, , < 1. When , , it indicates that all levels are evolutionary stable states, and there is no difference between high and low input of enterprises’ coping ability building. At this time, enterprises’ ESS cannot be determined. To make (), there are two stable points of and . If the probability of an enterprise’s coping behavior selection is in a stable state, the conditions to be met are and . Based on this, different situations are analyzed:
① When ≤ 0, .
Therefore, when , this indicates the ESS point is .
② When .
Therefore, when and , this indicates the ESS point is .
③ When .
Therefore, when and , this indicates the ESS point is .
The phase diagrams of enterprise coping strategies are shown in Figure 2. When the probability of Natech occurrence is less than a certain value and the probability that the emergency management department chooses active disposal is greater than a certain value, enterprises will choose to invest less in coping capacity building. When the probability of Natech occurrence is greater than a certain value and the probability that the emergency management department chooses active disposal is greater than a certain value, enterprises will also choose to invest less in coping capacity building. When the probability of Natech occurrence is greater than a certain value and the probability that the emergency management department chooses active disposal is less than a certain value, enterprises will choose high investment in coping capacity building.
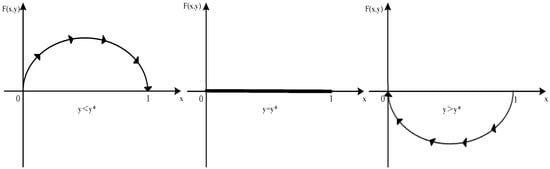
Figure 2.
The coping strategy phase diagram of enterprise.
3.2.2. ESS Analysis of Emergency Management Department
Find the first partial derivative with respect to y of the replication dynamic equation G(x,y) of the firm in Equation (8):
Let 0, < 1. When , , it indicates that all levels are evolutionary stable states, and there is no difference between active disposal and delayed disposal of emergency management departments. At this time, the emergency management department’s ESS cannot be determined. To make (), there are two stable points of and . If the probability of the emergency management department’s coping behavior selection is in a stable state, the conditions to be met are and . Based on this, different situations are analyzed:
① When ≤ 0, .
Therefore, when and , this indicates the ESS point is .
② When , , , , .
Therefore, when and , this indicates the ESS point is .
③ When , , , , .
Therefore, when , and , this indicates the ESS point is .
Coping strategy phase diagrams of the emergency management department are shown in Figure 3. When the probability of Natech occurrence is less than a certain value and the probability that the enterprise chooses high investment in coping capacity building is greater than a certain value, the emergency management department will choose delayed disposal. When the probability of Natech occurrence is greater than a certain value and the probability that the enterprise chooses high investment in coping capacity building is greater than a certain value, the emergency management department will also choose delayed disposal. When the probability of Natech occurrence is greater than a certain value and the probability that the enterprise chooses high investment in coping capacity building is less than a certain value, the emergency management department will choose active disposal.
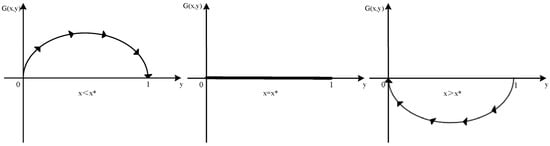
Figure 3.
The coping strategy phase diagram of the emergency management department.
3.2.3. ESS Analysis Based on Natech Risk Level
According to the strategy selection of enterprises and emergency management departments to deal with Natech, the probability p of Natech occurrence is divided into two points of and and three segments (0, 1). In this study, the loss caused by the occurrence of Natech is fixed, so P1 and P2 can be used as the threshold of the Natech risk level. To analyze the evolutionary stability of government and enterprise under different Natech risks, three risk levels are represented on the Natech occurrence probability axis, the respective stable evolution strategies of enterprises and emergency management departments are marked, and the ESSs of government and enterprise under different Natech risk levels are obtained. The shaded part in the figure is the evolutionary strategies of enterprises.
Scenario 1: When , the ESS of the enterprise and emergency management department is shown in Figure 4.
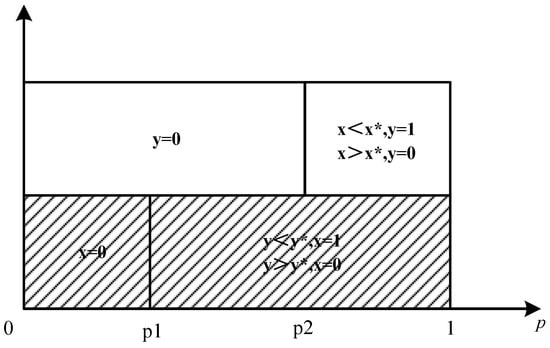
Figure 4.
The ESS of the enterprise and emergency management department in scenario 1.
As can be seen from Figure 4, when , enterprises choose low investment in coping capacity building, while emergency management departments choose delayed disposal. When the emergency management department chooses delayed disposal (). Because of 0, when the emergency management department chooses delayed disposal, the enterprise will choose high investment in coping capacity building (). When , if enterprises chooses high investment in coping capacity building (), the emergency management department will choose delayed disposal (); assuming that the enterprise chooses low investment in coping capacity building (), the emergency management department will choose active disposal (); assuming that the emergency management department chooses active disposal (), the enterprise will choose low investment in emergency capacity building (); assuming that the emergency management department chooses delayed disposal (), the enterprise will choose high investment in coping capacity building (). As a result, the evolution dynamics of enterprises and emergency management departments based on different Natech risks in this scenario are shown in Figure 5.
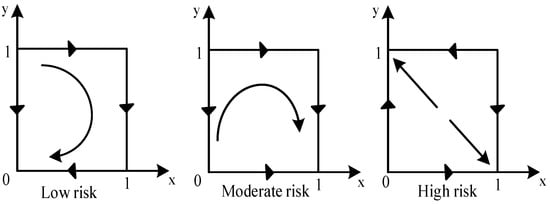
Figure 5.
The evolution game dynamics of enterprises and emergency management departments in scenario 1.
Scenario 2: When , the ESS of the enterprise and emergency management department is shown in Figure 6.

Figure 6.
The ESS of the enterprise and emergency management department in scenario 2.
As can be seen from Figure 6, when , enterprises choose low investment in coping capacity building, while emergency management departments choose delayed disposal. When the enterprise chooses low investment in coping capacity building (). Because of 0, when the enterprise chooses low investment in coping capacity building, the emergency management department will choose active disposal (). When , if the enterprise chooses high investment in coping capacity building (), the emergency management department will choose delayed disposal (); assuming that the enterprise chooses low investment in emergency capacity building (), the emergency management department will choose active disposal (); assuming that the emergency management department chooses active disposal (), the enterprise will choose low investment in emergency capacity building (); assuming that the emergency management department chooses delayed disposal (), the enterprise will choose high investment in coping capacity building (). As a result, the evolution dynamics of enterprises and emergency management departments based on different Natech risks in scenario 2 are shown in Figure 7.
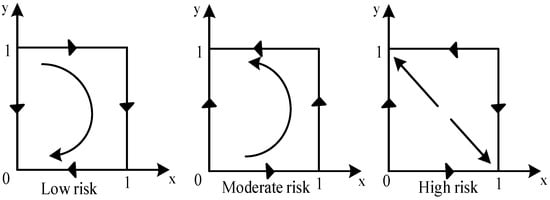
Figure 7.
The evolution game dynamics of enterprises and emergency management departments in scenario 2.
Obviously, there is also a special scenario of , in which there is no moderate risk. In this case, the evolutionary dynamics graph of government and enterprise only includes low Natech risk and high Natech risk. The dynamic nature of evolution is the same as in the above two situations, so we will not delve into details here.
Therefore, faced with low Natech risk, enterprises will opt for minimal investment in coping capacity building, while emergency management departments will choose delayed disposal. In the presence of moderate Natech risk, if the emergency management department still opts for delayed response, enterprises will choose to invest heavily in coping capacity building. Similarly, if the risk of Natech still leads enterprises to choose minimal investment in coping capacity building, the emergency management department will choose active disposal as a response. With high Natech risk, enterprises and governments will evolve based on each other’s behavior, eventually reaching a stable state. For instance, when enterprises opt for significant investment in coping capacity building, the emergency management department will select delayed disposal; conversely, when enterprises choose minimal investment, the emergency management department will select active disposal as the appropriate course of action.
4. Numerical Simulation
4.1. Parameter Assumptions
This study systematically studies the strategic choices of enterprises and emergency management departments considering the probability of Natech occurrence. To further illustrate the validity of the conclusions of this study on the evolution of government and enterprise, numerical simulation is adopted to visually observe the evolution process. It is assumed that the investment of enterprises in building high coping capacity is a = CNY 600,000, while that in building low coping capacity is e = CNY 300,000. The production income of the enterprise when Natech does not occur is c = CNY 1 million, the investment that the government actively disposed of is b = CNY 400,000, , the social welfare obtained by the emergency management departments when Natech does not occur is is d = CNY 600,000, the loss caused by the negative impact of Natech on enterprise is f = CNY 800,000, and the loss caused by the negative impact of Natech on emergency management departments is g = CNY 500,000. The settings of the above parameters are consistent with the basic assumptions in the model and the reality.
Based on the above data, = = 0.2 and = = 0.36 can be obtained, which conforms scenario 1 in the ESS analysis based on Natech risk. Therefore, this study holds that when p ∈ (0, 0.2), Natech risk is low risk; when p ∈ [0.2, 0.36], Natech risk belongs to moderate risk; when p ∈ (0.36, 1), Natech risk belongs to high risk. Therefore, simulation will be performed in the following three cases:
Case 1: Suppose p = 0.1 (p ∈ (0, 0.2)), the behavior strategies of enterprises and emergency management departments in collaborative coping under low Natech risk are studied by dynamic differential equation.
Case 2: Suppose p = 0.3 (p ∈ [0.2, 0.36]), the behavior strategies of enterprises and emergency management departments in collaborative coping under moderate Natech risk are studied by dynamic differential equation.
Case 3: Suppose p = 0.6 (p ∈ (0.36, 1)), the behavior strategies of enterprises and emergency management departments in collaborative coping under high Natech risk are studied by dynamic differential equation.
4.2. Analysis of Evolutionary Results
The probability x of the enterprise choosing high investment in coping ability building and the probability y of the emergency management department choosing active disposal are both constantly changing in (0, 1). The dynamic evolution numerical changes of the enterprise and the emergency management department in case 1 are, respectively, obtained from the dynamic differential equation, as shown in Figure 8.
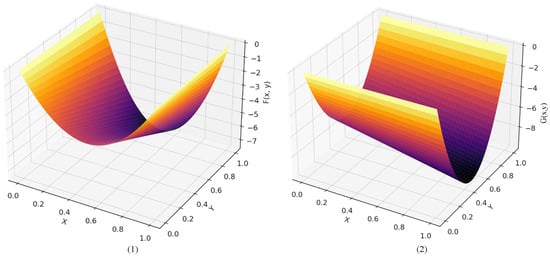
Figure 8.
When p = 0.1, the numerical change of the dynamic evolution of government and enterprise (Among them, (1) shows the numerical change of the enterprise; (2) shows the numerical change of the government).
As can be seen from Figure 8 (1), the dynamic differential equation of the enterprise in case 1 is a concave function (), where x = 0 is the stable point, at which time the enterprise will choose low investment in coping capacity building.
As can be seen from Figure 8 (2), the dynamic differential equation of the emergency management department in case 1 is a concave function (), where y = 0 is the stable point, and the emergency management department will choose the delayed disposal.
When p = 0.3, the numerical change of dynamic evolution of enterprise is shown in Figure 9 (1), where F(x,1/3) = 0 is the critical point in the graph. When y < 1/3, the image is a convex function (), indicating that x = 1 is a stable point. At this time, enterprises will choose high investment in coping capacity building. When y > 1/3, the image is a concave function (), indicating that x = 0 is the stable point, at which time enterprises will choose low investment in coping capacity building.
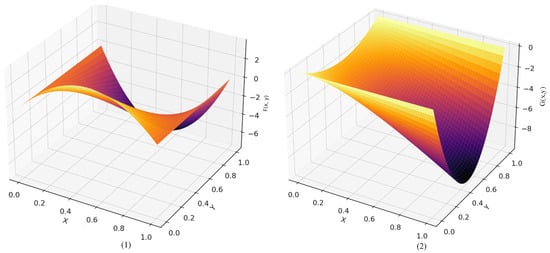
Figure 9.
When p = 0.3, the numerical change of the dynamic evolution of government and enterprise (Among them, (1) shows the numerical change of the enterprise; (2) shows the numerical change of the government).
As can be seen from Figure 9 (2), the dynamic differential equation of the emergency management department in case 2 is a concave function (). In the command, y = 0 indicates the stable point. In this case, the emergency management department chooses delayed disposal. Based on the strategy of the emergency management departments, enterprises will choose to invest in high coping capacity building.
When p = 0.6, the numerical change in the dynamic evolution of enterprise is shown in Figure 10 (1), where F(x,2/3) = 0 is the critical point. When y < 2/3, the image is a convex function (), indicating that x = 1 is a stable point, at which time enterprises will choose high coping ability investment. When y > 2/3, the image is a concave function (), indicating that x = 0 is the stable point, at which time the enterprise will choose low coping capacity investment.
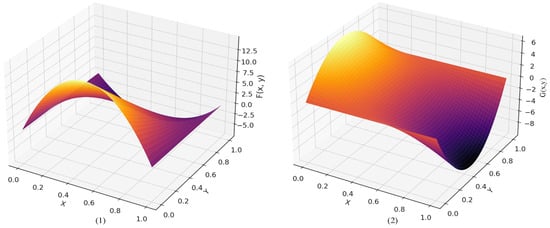
Figure 10.
When p = 0.6, the numerical change of the dynamic evolution of government and enterprise (Among them, (1) shows the numerical change of the enterprise; (2) shows the numerical change of the government).
The numerical change in the dynamic evolution of governance is shown in Figure 10 (2), where G(13/33,y) = 0 is the critical point in the figure. When x < 13/33, the image is a convex function (), indicating that y = 1 is a stable point, and the government will choose to take the active disposal. When x > 13/33, the image is a concave function (), indicating that y = 0 is the stable point, at which time the government will choose the delayed disposal.
5. Results Discussion
5.1. Impact Analysis of the Evolution Game of Government–Enterprise Collaboration in Coping with Natech Risks
From Section 4, it can be seen that the ESS of the government–enterprise collaboration in coping with Natech risks in Section 3 was verified, providing a theoretical basis for analyzing the failure phenomenon of government–enterprise collaboration.
- (1)
- Failure of Natech risk analysis will lead to the failure of government–enterprise collaboration in coping with Natech risks
Under varying levels of Natech risk, the collaborative coping strategies between emergency management departments and enterprises differ. Consequently, the failure of risk analysis can disrupt the collaborative coping strategies between these entities. Specifically, different types and intensities of natural disasters can impact technical facilities differently, underscoring the need for precise risk analysis to ensure that response measures align with potential threats. Firstly, if Natech risk analysis fails to identify all relevant natural disaster risks, emergency management departments and enterprises may overlook developing coping strategies for possible disaster scenarios. Secondly, accurate and up-to-date data are essential for Natech risk analysis. Using inaccurate or outdated data may lead to analysis results that do not reflect the current risk landscape. Thirdly, using inappropriate risk assessment methods or tools for specific types of Natech events can result in underestimating the potential impact of disasters. In such scenarios, the most probable condition of government–enterprise collaboration failure is low investment by enterprises in coping capacity building and delayed response by emergency management departments under low Natech risk.
- (2)
- Insufficient ability of government–enterprise collaboration in coping with Natech risk in extreme disaster situations
In the case of effective risk analysis, there is a higher probability of failure in the collaborative coping strategy of the emergency management departments and enterprises under extreme Natech risk. First, in extreme Natech risks, the interaction of natural disasters and technological systems is more complex, leading to increased uncertainty in response scenarios. This uncertainty can be exacerbated by prediction errors in the scale, intensity, or scope of the disaster. Second, in extreme Natech scenarios, the required emergency resources (such as human, material, technical, and financial) are often far beyond the normal level of preparedness. Emergency management departments and enterprises may face inadequate resources, which can affect the timeliness and effectiveness of disaster response.
5.2. Impact Reduction Strategy for the Evolution Game of Government–Enterprise Collaboration in Coping with Natech Risks
- (1)
- Improve Natech risk analysis ability
The following measures can avoid the failure of government–enterprise coordination caused by the failure of Natech risk analysis: ① Enhance the comprehensiveness of risk identification by adopting systematic risk identification methods and tools to ensure that all possible types of natural disasters are included. ② Use the latest technologies and methods, such as big data analytics and artificial intelligence, to process and analyze risk data and improve the accuracy and timeliness of risk assessment. ③ Risk assessments are regularly updated to ensure that all participants are fully informed of the latest risk situation.
- (2)
- Establish an integrated mechanism of Natech risk information sharing and response
Establish a Natech risk information sharing mechanism so that emergency management departments and enterprises can obtain information about upcoming natural disasters and potential impacts in real time. The following measures can avoid the untimely disposal caused by the mismatch between government and enterprise coordination and actual risks, so as to improve the adaptability to disasters: ① Use advanced monitoring technology and information sharing platforms to ensure that all relevant parties can quickly and accurately obtain disaster information. The above technology obtains data through cameras and identifies risk scenarios through algorithms and can also introduce technologies such as the Internet of Things to strengthen the early warning of Natech risks and the ability of government to obtain emergency information, promote emergency departments to better allocate and coordinate emergency resources, and more effectively promote enterprises to carry out emergency response. ② Develop detailed emergency plans, including response mechanisms at different levels, to ensure that response strategies can be quickly adapted to the scale and nature of the disaster.
- (3)
- Strengthen coping capacity building for Natech
The following measures can not only increase the compatibility of government–enterprise collaboration with risk analysis but also increase the redundancy of disaster preparedness to deal with more high-uncertainty disasters: ① On the basis of risk analysis, the required Natech coping capacity is evaluated to increase the matching degree between risk and capacity building. ② Design redundancy in critical resources and systems to ensure that if one system or resource fails, others can continue to function. ③ Regular emergency response training and multi-party exercises should be conducted to improve the coordination and response ability of all parties in high-pressure environments. ④ More entities should be used as alternatives for emergency collaboration in order to be more capable of responding to extreme disasters.
6. Conclusions and Discussion
The evolutionary game analysis of government–enterprise collaborative coping behavior based on Natech risk provides an important theoretical basis for further improving the efficiency of Natech risk management.
- (1)
- Under Natech’s low risk, enterprises will choose low investment in response capacity building, and emergency management departments will choose delayed disposal.
- (2)
- Under the moderate risk of Natech, when the emergency management department only chooses delayed disposal, the enterprise will choose high response capacity building investment according to the behavioral strategy of the emergency management department. When the enterprise chooses only low response capacity building investment, the emergency management department will choose active disposal according to the behavior strategy of the enterprise.
- (3)
- Under high Natech risk, enterprises and emergency management departments make misplaced choices based on each other’s behavior and eventually form a stable state. When enterprises choose to invest in high coping capacity, emergency management departments will choose delayed disposal; when enterprises choose low coping capacity input, emergency management departments will choose active disposal.
Compared to the existing literature on Natech risk management, this study contributes to knowledge production on the same issue across different disciplines from the perspective of public management. Unlike previous studies on the evolutionary game analysis of government–enterprise emergency collaboration, this research highlights the equal importance of both government and enterprises in Natech risk prevention and control. This contrasts with prior research, where enterprises were merely seen as supplements to government emergency response capabilities. In this study, enterprises are assigned a core responsibility for Natech risk prevention and control and are required to invest appropriate funds to enhance their coping abilities. The development of these behaviors is influenced by risk assessment. Consequently, by incorporating risk assessment and analyzing the interactions and games between government and enterprises, a collaboration mechanism for managing Natech risks is developed. In addition, this study further proposes strategies to reduce the impact of the evolutionary game mechanism, including enhancing Natech risk analysis capabilities, establishing an integrated mechanism for Natech risk information sharing and response, and strengthening Natech coping capacity building. Subsequent research should delve into the above strategies to provide more effective recommendations for Natech risk management.
Author Contributions
Conceptualization, S.G. and W.F.; methodology, S.G. and W.F.; software, S.G. and W.F.; investigation, Y.W. and G.Z.; writing—original draft preparation, S.G. and W.F.; writing—review and editing, G.Z. and Y.W.; visualization, W.F.; supervision, G.Z.; project administration, G.Z. and Y.W.; funding acquisition, G.Z. and Y.W. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by the Hunan Natural Science Foundation Project “Research on Complex System Safety Information Coordination Mechanism and Measurement Method” (Grant Number: 2024JJ5455) and Graduate innovation project of Central South University (2024ZZTS0578).
Data Availability Statement
No new data were created.
Conflicts of Interest
The authors declare no conflicts of interest.
References
- Cruz, A.M. Challenges in Natech Risk Reduction. Rev. Ing. 2012, 79–86. [Google Scholar] [CrossRef]
- Krausmann, E.; Necci, A. Thinking the unthinkable: A perspective on Natech risks and Black Swans. Saf. Sci. 2021, 139, 105255. [Google Scholar] [CrossRef]
- Cruz, A.M.; Suarez-Paba, M.C. Advances in Natech research: An overview. Prog. Disaster Sci. 2019, 1, 100013. [Google Scholar] [CrossRef]
- Li, P.; Wang, T.; Qie, Z. Collaborative Relationship Modeling and Analysis of Natech Emergency Response Organizations Based on Stochastic Petri Net. Nat. Hazards Rev. 2024, 25, 04024009. [Google Scholar] [CrossRef]
- Suarez-Paba, M.C.; Cruz, A.M.; Munoz, F. Emerging Natech risk management in Colombia: A survey of governmental organizations. Saf. Sci. 2020, 128, 104777. [Google Scholar] [CrossRef]
- Kumasaki, M.; King, M. Three cases in Japan occurred by natural hazards and lessons for Natech disaster management. Int. J. Disaster Risk Reduct. 2020, 51, 101855. [Google Scholar] [CrossRef] [PubMed]
- Girgin, S.; Necci, A.; Krausmann, E. Dealing with cascading multi-hazard risks in national risk assessment: The case of Natech accidents. Int. J. Disaster Risk Reduct. 2019, 35, 101072. [Google Scholar] [CrossRef]
- Krausmann, E.; Baranzini, D. Natech risk reduction in the European Union. J. Risk Res. 2012, 15, 1027–1047. [Google Scholar] [CrossRef]
- Pilone, E.; Moreno, V.C.; Cozzani, V.; Demichela, M. Climate change and NaTech events: A step towards local-scale awareness and preparedness. Saf. Sci. 2021, 139, 105264. [Google Scholar] [CrossRef]
- Krausmann, E.; Girgin, S.; Necci, A. Natural hazard impacts on industry and critical infrastructure: Natech risk drivers and risk management performance indicators. Int. J. Disaster Risk Reduct. 2019, 40, 101163. [Google Scholar] [CrossRef]
- Zhang, G.; Feng, W.; Lei, Y.; Wang, S. Generation and evolution mechanism of systemic risk (SR) induced by extreme precipitation in Chinese Urban system: A case study of Zhengzhou “7 20” incident. Int. J. Disaster Risk Reduct. 2022, 83, 103401. [Google Scholar] [CrossRef]
- Santella, N.; Steinberg, L.J.; Aguirra, G.A. Empirical Estimation of the Conditional Probability of Natech Events Within the United States. Risk Anal. 2011, 31, 951–968. [Google Scholar] [CrossRef] [PubMed]
- Khakzad, N.; Cozzani, V. Special issue: Quantitative assessment and risk management of Natech accidents. Reliab. Eng. Syst. Saf. 2020, 203, 107198. [Google Scholar] [CrossRef]
- Damle, S.; Mani, S.K.; Balamurugan, G. Natech guide words: A new approach to assess and manage natech risk to ensure business continuity. J. Loss Prev. Process Ind. 2021, 72, 104564. [Google Scholar] [CrossRef]
- Cai, M.; Marson, S.M. A regional Natech risk assessment based on a Natech-prone facility network for dependent events. Nat. Hazards 2021, 107, 2155–2174. [Google Scholar] [CrossRef]
- O’Reilly, G.J.; Shahnazaryan, D.; Dubini, P.; Brunesi, E.; Rosti, A.; Dacarro, F.; Gotti, A.; Silvestri, D.; Mascetti, S.; Ducci, M.; et al. Risk-aware navigation in industrial plants at risk of NaTech accidents. Int. J. Disaster Risk Reduct. 2023, 88, 103620. [Google Scholar] [CrossRef]
- Luo, X.; Tzioutzios, D.; Tong, Z.; Cruz, A.M. Find-Natech: A GIS-based spatial management system for Natech events. Int. J. Disaster Risk Reduct. 2022, 76, 103028. [Google Scholar] [CrossRef]
- Mesa-Gomez, A.; Casal, J.; Munoz, F. Risk analysis in Natech events: State of the art. J. Loss Prev. Process Ind. 2020, 64, 104071. [Google Scholar] [CrossRef]
- Luo, X.; Cruz, A.M.; Tzioutzios, D. Extracting Natech Reports from Large Databases: Development of a Semi-Intelligent Natech Identification Framework. Int. J. Disaster Risk Sci. 2020, 11, 735–750. [Google Scholar] [CrossRef]
- Guirong, Z.; Yu, L.; Wei, F. Research on coordination mechanism of emergency information driven by big data. J. Intell. 2022, 41, 181–185+201. [Google Scholar]
- Zhang, L.; Wang, J.; Wang, X.; Gao, Y. Research on cross-city emergency management collaboration mechanism in major emergencies: Insights from a knowledge management perspective. J. Knowl. Manag. 2024. ahead-of-print. [Google Scholar] [CrossRef]
- Chen, W.; Zhang, H.; Comfort, L.K.; Tao, Z. Exploring complex adaptive networks in the aftermath of the 2008 Wenchuan earthquake in China. Saf. Sci. 2020, 125, 104607. [Google Scholar] [CrossRef]
- Yang, L.; Lou, J.; Zhou, J.; Zhao, X.; Jiang, Z. Complex network-based research on organization collaboration and cooperation governance responding to COVID-19. Eng. Constr. Archit. Manag. 2023, 30, 3749–3779. [Google Scholar] [CrossRef]
- Wang, L.; Zhao, N.; Liu, D. Complex disaster management: A dynamic game among the government, enterprises, and residents. J. Clean. Prod. 2020, 266, 122091. [Google Scholar] [CrossRef]
- Du, L.; Qian, L. The government’s mobilization strategy following a disaster in the Chinese context: An evolutionary game theory analysis. Nat. Hazards 2016, 80, 1411–1424. [Google Scholar] [CrossRef]
- Sun, W.; Zhu, C.; Li, H. Evolutionary game analysis of emergency rescuer dispatching under bounded rationality. Int. J. Disaster Risk Reduct. 2023, 96, 103927. [Google Scholar] [CrossRef]
- Peng, Y.; Gu, X.; Wang, Z.; Li, Q.; Jiang, S. Behavior of Rural Victims and Local Government during Post-Disaster Reconstruction: Agent-Based Simulation. Nat. Hazards Rev. 2021, 22, 04021051. [Google Scholar] [CrossRef]
- Chen, Y.; Liu, X.; Tadikamalla, P.R.; Qu, M.; Wang, Y. Evolutionary game analysis for multi-level collaborative governance under public crisis in China: From a value perception perspective. Risk Anal. 2024, 44, 582–611. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Chen, H.; Gu, X. A Two-Stage Evolutionary Game Model for Collaborative Emergency Management Between Local Governments and Enterprises. Int. J. Disaster Risk Sci. 2023, 14, 1029–1043. [Google Scholar] [CrossRef]
- Li, C.; Zhang, F.; Cao, C.; Liu, Y.; Qu, T. Organizational coordination in sustainable humanitarian supply chain: An evolutionary game approach. J. Clean. Prod. 2019, 219, 291–303. [Google Scholar] [CrossRef]
- Wu, X.; Yang, M.; Wu, C.; Liang, L. How to avoid source disruption of emergency supplies in emergency supply chains: A subsidy perspective. Int. J. Disaster Risk Reduct. 2024, 102, 104303. [Google Scholar] [CrossRef]
- Ma, X. Research on disaster collaborative management from the perspective of government, market, and society cooperation. Econ. Probl. 2020, 1, 18–22. [Google Scholar] [CrossRef]
- Amaducci, F.; Misuri, A.; Bonvicini, S.; Salzano, E.; Cozzani, V. Quantitative risk assessment of Natech scenarios triggered by earthquakes involving pipelines. Reliab. Eng. Syst. Saf. 2024, 245, 109993. [Google Scholar] [CrossRef]
- Antonioni, G.; Landucci, G.; Necci, A.; Gheorghiu, D.; Cozzani, V. Quantitative assessment of risk due to NaTech scenarios caused by floods. Reliab. Eng. Syst. Saf. 2015, 142, 334–345. [Google Scholar] [CrossRef]
- Cozzani, V.; Antonioni, G.; Landucci, G.; Tugnoli, A.; Bonvicini, S.; Spadoni, G. Quantitative assessment of domino and NaTech scenarios in complex industrial areas. J. Loss Prev. Process Ind. 2014, 28, 10–22. [Google Scholar] [CrossRef]
- Kabir, G.; Suda, H.; Cruz, A.M.; Munoz Giraldo, F.; Tesfamariam, S. Earthquake-related Natech risk assessment using a Bayesian belief network model. Struct. Infrastruct. Eng. 2019, 15, 725–739. [Google Scholar] [CrossRef]
- Congress SCotNPs. Law of the People’s Republic of China on Production Safety; Congress SCotNPs, Ed.; Beijing, China, 2021. [Google Scholar]
- Zhang, H.; Tong, X. Public crisis governance and accountability. Political Stud. 2010, 02, 50–55. [Google Scholar]
- Liu, J.-G.; Wang, J.-J.; Zhou, H.; Zhang, Y. Research on supervision problems of port hazardous chemicals base on security risk level. Syst. Eng. Theory Pract. 2018, 38, 1141–1152. [Google Scholar]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).