A Hybrid Group Decision Approach Based on MARCOS and Regret Theory for Pharmaceutical Enterprises Assessment under a Single-Valued Neutrosophic Scenario
Abstract
:1. Introduction
- ♠
- The prior score functions of SVNS possess several deficiencies in the aspect of ranking SVNNs and can produce ambiguous and inconsistent ranking results.
- ♠
- Considering the complexity and conflict of actual decision and assessment problems, it is necessary for decision makers to predict the combination of the subjective and objective weight of criteria for analyzing the decision issues. In order to enhance the practicability of the designed method in this paper, the combined weight of criteria is employed to acquire a more exact rank of pharmaceutical enterprises.
- ♠
- The extant extensions of MARCOS decision technique fail to consider the psychology factor of decision makers during the decision analysis procedure. Hence, it is essential to fuse the behavioral decision theory to the MARCOS algorithm to achieve more robust results.
- ♠
- There is no research on pharmaceutical enterprises assessment with SHQDA by considering the uncertainty and ambiguity of the assessment procedure.
- ✓
- A novel score function is brought forward and the corresponding elegant properties are taken over;
- ✓
- A synthesize criteria weight determination method is developed based on the BWM approach and improved CRITIC method using the novel score function to ascertain a more rational weight information of criterion;
- ✓
- An integrated assessment framework combining the regret theory-MARCOS method and Heronian mean operator is put forward on the basis of the presented score function;
- ✓
- A pharmaceutical enterprises assessment problem is utilized to elucidate the practicability and robustness of the advanced approach;
- ✓
- An analysis of the contrast and an examination of the parameter discussion demonstrate, respectively, the validity and stability of the suggested method.
2. Preliminaries
2.1. Single Valued Neutrosophic
2.2. Regret Theory
3. An Innovative Single-Valued Neutrosophic Score Function
3.1. Several Prior Single-Valued Neutrosophic Score Functions
3.2. A New SVN Score Function
- ¶
- For the Case 1 and Case 2, the score functions proposed by Smarandache [22] and Sahin [11] is invalid to compare the SVNNs and ; thus, we further compute the corresponding accuracy function and find that the accuracy values of and are still equal, namely, for Case 1 and for Case 1. That means the comparison rules proposed by Smarandache [22] and Sahin [11] cannot rank Case 1 and Case 2. However, the presented score function can rapidly acquire the rank result of Case 1 and Case 2 by a step. Accordingly, the propose score function is more universal and a shortcut to rank SVNNs.
- ¶
- ¶
4. SVN-MARCOS Method Based on the Regret Theory
4.1. Problem Statement
4.2. Obtaining SVN Assessment Information
4.3. Obtaining the Fused SVN Assessment Information
4.4. The Determination of Assessment Criteria Weight
4.5. Ranking by Utilizing the Proposed SVN Regret Theory-MARCOS Approach
4.6. The Decision Procedures of the Propounded Approach
5. Illustrate Example
5.1. Background Introduction
5.2. Decision Analysis
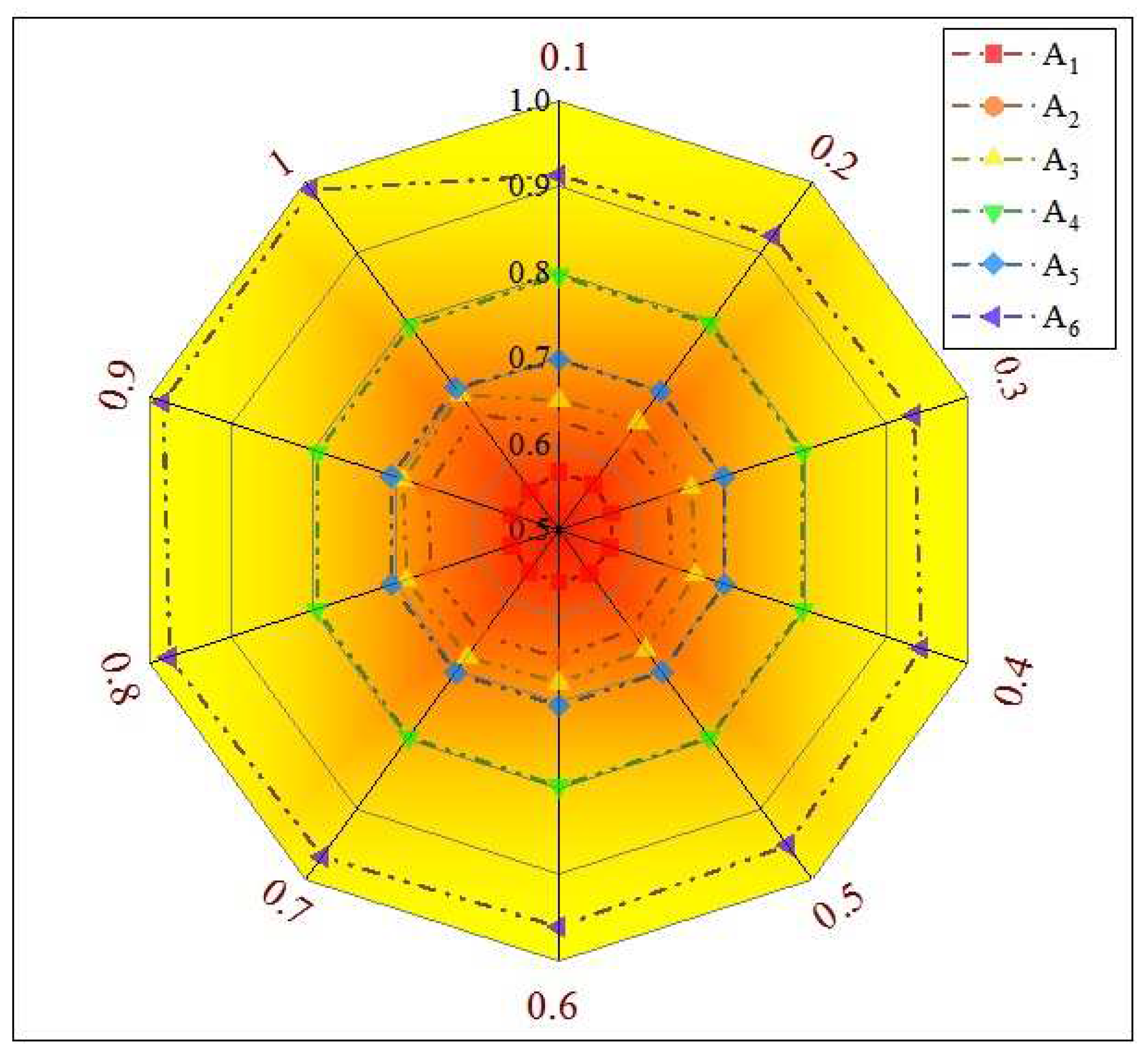
5.3. Sensitivity Analysis
5.4. Comparison Study
- ✠
- The proposed SVNS regret theory-MARCOS approach can effectively cope with the practical uncertain assessment problems when the criteria weight and expert weight are all complete unknown.
- ✠
- The suggested method is built by an innovative SVNS score function, which further provides more credible outcome than other existing score functions.
- ✠
- The weight information of the assessment criteria takes the subjective preference and actual decision information simultaneously into account, which further enhances the reliability and credibility of the ranks in dealing with complex assessment problems.
- ✠
- The propounded SVNS regret theory-MARCOS method takes both the advantages of regret theory and MARCOS method, which comprehensively considers the psychological preference of experts and the utility function theory of decision information to attain a more credible and robust decision outcomes.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
Abbreviations
| Full Name | Abbreviation | |
| Sustainable and High-Quality Development Ability | SHQDA | |
| Measurement Alternatives and Ranking based on the COmpromise Solution | MARCOS | |
| Multi Criteria Group Decision-Making | MCGDM | |
| Single-Valued Neutrosophic Set | SVNS | |
| Single-Valued Neutrosophic Number | SVNN | |
| CRiteria Importance Through Intercriteria Correlation | CRITIC | |
| Best and Worst Method | BWM | |
| Intuitionistic Fuzzy Set | IFS | |
| Single-Valued Neutrosophic | SVN | |
| Multi objective optimization based on the ratio analysis with the full multiplicative form | MULTIMOORA | |
| Technique for Order Preference by Similarity to an Ideal Solution | TOPSIS | |
| VIse KriterijumsaOptimiz acija I Kompromisno Resenje | VIKOR | |
| Mixed Aggregation by COmprehensive Normalization Technique | MACONT | |
| Weighted Aggregated Sum Product ASsessment | WASPAS | |
| LINear programming technique for Multidimensional Analysis of Preference | LINMAP | |
| Single-Valued Neutrosophic weighted averaging operator | SVNWA | |
| Single-Valued Neutrosophic geometric operator | SVNWG | |
| Single-Valued Neutrosophic Einstein weighted averaging operator | SVNEWA | |
| Single-Valued Neutrosophic Einstein geometric operator | SVNEWG |
Appendix A
| Expert | Pharmaceutical Enterprises | |||||||
|---|---|---|---|---|---|---|---|---|
| (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.90, 0.10, 0.10) | (0.80, 0.15, 0.20) | (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | (0.70, 0.25, 0.30) | ||
| (0.60, 0.35, 0.40) | (0.10, 0.90, 0.90) | (0.80, 0.15, 0.20) | (0.60, 0.35, 0.40) | (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.60, 0.35, 0.40) | ||
| (0.70, 0.25, 0.30) | (0.40, 0.65, 0.60) | (0.70, 0.25, 0.30) | (0.40, 0.65, 0.60) | (0.90, 0.10, 0.10) | (0.70, 0.25, 0.30) | (0.40, 0.65, 0.60) | ||
| (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | (0.60, 0.35, 0.40) | ||
| (0.50, 0.50, 0.50) | (0.60, 0.35, 0.40) | (0.40, 0.65, 0.60) | (0.90, 0.10, 0.10) | (0.60, 0.35, 0.40) | (0.90, 0.10, 0.10) | (0.50, 0.50, 0.50) | ||
| (0.60, 0.35, 0.40) | (0.50, 0.50, 0.50) | (0.60, 0.35, 0.40) | (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.40, 0.65, 0.60) | ||
| (0.60, 0.35, 0.40) | (0.60, 0.35, 0.40) | (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.60, 0.35, 0.40) | (0.60, 0.35, 0.40) | ||
| (0.60, 0.35, 0.40) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.60, 0.35, 0.40) | (0.60, 0.35, 0.40) | (0.70, 0.25, 0.30) | (0.40, 0.65, 0.60) | ||
| (0.50, 0.50, 0.50) | (0.30, 0.75, 0.70) | (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.60, 0.35, 0.40) | (0.60, 0.35, 0.40) | (0.50, 0.50, 0.50) | ||
| (0.80, 0.15, 0.20) | (0.10, 0.90, 0.90) | (0.60, 0.35, 0.40) | (0.90, 0.10, 0.10) | (0.50, 0.50, 0.50) | (0.90, 0.10, 0.10) | (0.40, 0.65, 0.60) | ||
| (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.60, 0.35, 0.40) | (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | ||
| (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | (0.80, 0.15, 0.20) | (0.90, 0.10, 0.10) | (0.80, 0.15, 0.20) | (0.60, 0.35, 0.40) | ||
| (0.50, 0.50, 0.50) | (0.50, 0.50, 0.50) | (0.60, 0.35, 0.40) | (0.40, 0.65, 0.60) | (0.30, 0.75, 0.70) | (0.50, 0.50, 0.50) | (0.10, 0.90, 0.90) | ||
| (0.60, 0.35, 0.40) | (0.70, 0.25, 0.30) | (0.50, 0.50, 0.50) | (0.50, 0.50, 0.50) | (0.80, 0.15, 0.20) | (0.40, 0.65, 0.60) | (0.10, 0.90, 0.90) | ||
| (0.50, 0.50, 0.50) | (0.80, 0.15, 0.20) | (0.40, 0.65, 0.60) | (0.70, 0.25, 0.30) | (0.70, 0.25, 0.30) | (0.60, 0.35, 0.40) | (0.60, 0.35, 0.40) | ||
| (0.90, 0.10, 0.10) | (0.70, 0.25, 0.30) | (0.50, 0.50, 0.50) | (0.80, 0.15, 0.20) | (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | (0.40, 0.65, 0.60) | ||
| (0.10, 0.90, 0.90) | (0.80, 0.15, 0.20) | (0.40, 0.65, 0.60) | (0.60, 0.35, 0.40) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.60, 0.35, 0.40) | ||
| (0.60, 0.35, 0.40) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.50, 0.50, 0.50) | (0.70, 0.25, 0.30) | (0.10, 0.90, 0.90) | ||
| (0.60, 0.35, 0.40) | (0.50, 0.50, 0.50) | (0.30, 0.75, 0.70) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | (0.70, 0.25, 0.30) | (0.80, 0.15, 0.20) | ||
| (0.70, 0.25, 0.30) | (0.40, 0.65, 0.60) | (0.40, 0.65, 0.60) | (0.60, 0.35, 0.40) | (0.60, 0.35, 0.40) | (0.80, 0.15, 0.20) | (0.60, 0.35, 0.40) | ||
| (0.80, 0.15, 0.20) | (0.30, 0.75, 0.70) | (0.50, 0.50, 0.50) | (0.70, 0.25, 0.30) | (0.60, 0.35, 0.40) | (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | ||
| (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | (0.40, 0.65, 0.60) | (0.60, 0.35, 0.40) | (0.90, 0.10, 0.10) | (0.60, 0.35, 0.40) | (0.50, 0.50, 0.50) | ||
| (0.60, 0.35, 0.40) | (0.40, 0.65, 0.60) | (0.60, 0.35, 0.40) | (0.90, 0.10, 0.10) | (0.80, 0.15, 0.20) | (0.50, 0.50, 0.50) | (0.80, 0.15, 0.20) | ||
| (0.60, 0.35, 0.40) | (0.50, 0.50, 0.50) | (0.50, 0.50, 0.50) | (0.80, 0.15, 0.20) | (0.80, 0.15, 0.20) | (0.80, 0.15, 0.20) | (0.90, 0.10, 0.10) |
| Pharmaceutical Enterprises | |||||||
|---|---|---|---|---|---|---|---|
| (0.6461, 0.3074, 0.3539) | (0.5850, 0.3836, 0.4150) | (0.6328, 0.3350, 0.3672) | (0.6821, 0.2754,0.3179) | (0.7008, 0.2524, 0.2992) | (0.6241, 0.3390, 0.3759) | (0.5871, 0.3603, 0.4129) | |
| (0.6286, 0.3209, 0.3714) | (0.5001, 0.4743, 0.4999) | (0.6658, 0.2986, 0.3342) | (0.5799, 0.3788,0.4201) | (0.7094, 0.2367, 0.2906) | (0.6823, 0.2752, 0.3177) | (0.5315, 0.4487, 0.4685) | |
| (0.6512, 0.3107, 0.3488) | (0.4685, 0.5392, 0.5315) | (0.6344, 0.3331, 0.3656) | (0.6358, 0.3283,0.3642) | (0.7327, 0.2384, 0.2673) | (0.6888, 0.2585, 0.3112) | (0.4984, 0.4999, 0.5016) | |
| (0.8069, 0.1579, 0.1931) | (0.5678, 0.3905, 0.4322) | (0.5032, 0.4923, 0.4968) | (0.7733, 0.1954,0.2267) | (0.7834, 0.1893, 0.2166) | (0.6990, 0.2796, 0.3010) | (0.4834, 0.5213, 0.5166) | |
| (0.6233, 0.3399, 0.3767) | (0.6376, 0.3241, 0.3624) | (0.5853, 0.3921, 0.4147) | (0.8171, 0.1685,0.1829) | (0.6844, 0.2628, 0.3156) | (0.6695, 0.2913, 0.3305) | (0.6200, 0.3450, 0.3800) | |
| (0.6282, 0.3213, 0.3718) | (0.6413, 0.3227, 0.3587) | (0.6072, 0.3591, 0.3928) | (0.8168, 0.1511,0.1832) | (0.7690, 0.2042, 0.2310) | (0.7815, 0.1677, 0.2185) | (0.6625, 0.3341, 0.3375) |
References
- Dong, J.; Ju, Y.; Dong, P.; Giannakis, M.; Wang, A.; Liang, Y.; Wang, H. Evaluate and select state-owned enterprises with sustainable high-quality development capacity by integrating FAHP-LDA and bidirectional projection methods. J. Clean. Prod. 2021, 329, 129771. [Google Scholar] [CrossRef]
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Haiyun, C.; Zhixiong, H.; Yüksel, S.; Dinçer, H. Analysis of the innovation strategies for green supply chain management in the energy industry using the QFD-based hybrid interval valued intuitionistic fuzzy decision approach. Renew. Sust. Energ. Rev. 2021, 143, 110844. [Google Scholar] [CrossRef]
- Garg, H.; Rani, D. An efficient intuitionistic fuzzy MULTIMOORA approach based on novel aggregation operators for the assessment of solid waste management techniques. Appl. Intell. 2021, 52, 4330–4363. [Google Scholar] [CrossRef]
- Xie, D.; Xiao, F.; Pedrycz, W. Information quality for intuitionistic fuzzy values with its application in decision making. Eng. Appl. Artif. Intell. 2022, 109, 104568. [Google Scholar] [CrossRef]
- Zeng, S.; Zhou, J.; Zhang, C.; Merigó, J.M. Intuitionistic fuzzy social network hybrid MCDM model for an assessment of digital reforms of manufacturing industry in China. Technol. Forecast. Soc. Chang. 2022, 176, 121435. [Google Scholar] [CrossRef]
- Ilbahar, E.; Kahraman, C.; Cebi, S. Risk assessment of renewable energy investments: A modified failure mode and effect analysis based on prospect theory and intuitionistic fuzzy AHP. Energy 2022, 239, 121907. [Google Scholar] [CrossRef]
- Smarandache, F. A unifying field in Logics: Neutrosophic Logic. In Philosophy; American Research Press: Santa Fe, NM, USA, 1999; pp. 1–141. [Google Scholar]
- Wang, H.; Smarandache, F.; Zhang, Y.; Sunderraman, R. Single Valued Neutrosophic Sets. Multispace Multistruct. 2010, Volume 4, pp. 410–413. Available online: http://fs.unm.edu/SingleValuedNeutrosophicSets.pdf (accessed on 1 July 2022).
- Şahin, R. Multi-criteria neutrosophic decision making method based on score and accuracy functions under neutrosophic environment. arXiv 2014, arXiv:1412.5202. [Google Scholar]
- Liu, P. The aggregation operators based on archimedean t-conorm and t-norm for single-valued neutrosophic numbers and their application to decision making. Int. J. Fuzzy Syst. 2016, 18, 849–863. [Google Scholar] [CrossRef]
- Huang, H.L. New distance measure of single-valued neutrosophic sets and its application. Int. J. Intell. Syst. 2016, 31, 1021–1032. [Google Scholar] [CrossRef]
- Garg, H. An improved score function for ranking neutrosophic sets and its application to decision-making process. Int. J. Uncertain Quantif. 2016, 6, 377–385. [Google Scholar]
- Özlü, Ş. Generalized Dice measures of single valued neutrosophic type-2 hesitant fuzzy sets and their application to multi-criteria decision making problems. Int. J. Mach. Learn. Cybern. 2022, 1–30. [Google Scholar] [CrossRef]
- Garg, H. SVNMPR: A new single-valued neutrosophic multiplicative preference relation and their application to decision-making process. Int. J. Intell. Syst. 2022, 37, 2089–2130. [Google Scholar] [CrossRef]
- Garg, H. A novel divergence measure and its based TOPSIS method for multi criteria decision-making under single-valued neutrosophic environment. J. Intell. Fuzzy Syst. 2019, 36, 101–115. [Google Scholar]
- Mishra, A.R.; Rani, P.; Mardani, A.; Pardasani, K.R.; Govindan, K.; Alrasheedi, M. Healthcare evaluation in hazardous waste recycling using novel interval-valued intuitionistic fuzzy information based on complex proportional assessment method. Comput. Ind. Eng. 2020, 139, 106140. [Google Scholar] [CrossRef]
- Pamucar, D.; Deveci, M.; Schitea, D.; Erişkin, L.; Iordache, M.; Iordache, I. Developing a novel fuzzy neutrosophic numbers based decision making analysis for prioritizing the energy storage technologies. Int. J. Hydrogen Energy 2020, 45, 23027–23047. [Google Scholar] [CrossRef]
- Sun, Y.; Cai, Y. A Flexible Decision-Making Method for Green Supplier Selection Integrating TOPSIS and GRA Under the Single-Valued Neutrosophic Environment. IEEE Access 2021, 9, 83025–83040. [Google Scholar] [CrossRef]
- Ye, J. Single valued neutrosophic cross-entropy for multicriteria decision making problems. Appl. Math. Modell. 2014, 38, 1170–1175. [Google Scholar] [CrossRef]
- Smarandache, F. The Score, Accuracy, and Certainty Functions determine a Total Order on the Set of Neutrosophic Triplets (T, I, F). Neutrosophic Sets Syst. 2020, 38, 1–14. [Google Scholar] [CrossRef]
- Nafei, A.; Javadpour, A.; Nasseri, H.; Yuan, W. Optimized score function and its application in group multiattribute decision making based on fuzzy neutrosophic sets. Int. J. Intell. Syst. 2021, 36, 7522–7543. [Google Scholar] [CrossRef]
- Rong, Y.; Liu, Y.; Pei, Z. Generalized single-valued neutrosophic power aggregation operators based on Archimedean copula and co-copula and their application to multi-attribute decision-making. IEEE Access 2020, 8, 35496–35519. [Google Scholar] [CrossRef]
- Mishra, A.R.; Rani, P.; Prajapati, R.S. Multi-criteria weighted aggregated sum product assessment method for sustainable biomass crop selection problem using single-valued neutrosophic sets. Appl. Soft Comput. 2021, 113, 108038. [Google Scholar] [CrossRef]
- Rani, P.; Mishra, A.R.; Krishankumar, R.; Ravichandran, K.S.; Kar, S. Multi-criteria food waste treatment method selection using single-valued neutrosophic-CRITIC-MULTIMOORA framework. Appl. Soft Comput. 2021, 111, 107657. [Google Scholar] [CrossRef]
- Tan, R.; Yang, L.; Chen, S.; Zhang, W. Decision-making method based on game theory and grey theory in a single-value neutrosophic environment and its application to typhoon disaster assessment. Grey Syst. Theory Appl. 2021. [Google Scholar] [CrossRef]
- Pamucar, D.; Ecer, F.; Deveci, M. Assessment of alternative fuel vehicles for sustainable road transportation of United States using integrated fuzzy FUCOM and neutrosophic fuzzy MARCOS methodology. Sci. Total Environ. 2021, 788, 147763. [Google Scholar] [CrossRef] [PubMed]
- Solangi, Y.A.; Longsheng, C.; Shah, S.A.A. Assessing and overcoming the renewable energy barriers for sustainable development in Pakistan: An integrated AHP and fuzzy TOPSIS approach. Renew. Energy 2021, 173, 209–222. [Google Scholar] [CrossRef]
- Lin, M.; Chen, Z.; Xu, Z.; Gou, X.; Herrera, F. Score function based on concentration degree for probabilistic linguistic term sets: An application to TOPSIS and VIKOR. Inf. Sci. 2021, 551, 270–290. [Google Scholar] [CrossRef]
- Wen, Z.; Liao, H. Pension service institution selection by a personalized quantifier-based MACONT method. Int. J. Strateg. Prop. Manag. 2021, 25, 446–458. [Google Scholar] [CrossRef]
- Stević, Ž.; Pamučar, D.; Puška, A.; Chatterjee, P. Sustainable supplier selection in healthcare industries using a new MCDM method: Measurement of alternatives and ranking according to COmpromise solution (MARCOS). Comput. Ind. Eng. 2020, 140, 106231. [Google Scholar] [CrossRef]
- Fan, J.; Wang, S.; Wu, M. An integrated FMEA approach using Best-Worst and MARCOS methods based on D numbers for prioritization of failures. J. Intell. Fuzzy Syst. 2021, 41, 2833–2846. [Google Scholar] [CrossRef]
- Pamucar, D.; Yazdani, M.; Montero-Simo, M.J.; Araque-Padilla, R.A.; Mohammed, A. Multi-criteria decision analysis towards robust service quality measurement. Expert Syst. Appl. 2021, 170, 114508. [Google Scholar] [CrossRef]
- Gong, X.; Yang, M.; Du, P. Renewable energy accommodation potential evaluation of distribution network: A hybrid decision-making framework under interval type-2 fuzzy environment. J. Clean. Prod. 2021, 286, 124918. [Google Scholar] [CrossRef]
- Torkayesh, A.E.; Zolfani, S.H.; Kahvand, M.; Khazaelpour, P. Landfill location selection for healthcare waste of urban areas using hybrid BWM-grey MARCOS model based on GIS. Sustain. Cities Soc. 2021, 67, 102712. [Google Scholar] [CrossRef]
- Ecer, F.; Pamucar, D. MARCOS technique under intuitionistic fuzzy environment for determining the COVID-19 pandemic performance of insurance companies in terms of healthcare services. Appl. Soft Comput. 2021, 104, 107199. [Google Scholar] [CrossRef] [PubMed]
- Kundu, P.; Görçün, Ö.F.; Küçükönder, H. Medical device selection in private hospitals by integrated fuzzy MCGDM methods: A case study in choosing MRI (Magnetic Resonance Imaging) system. J. Oper. Res. Soc. 2021, 1–21. [Google Scholar] [CrossRef]
- Ali, J. A q-rung orthopair fuzzy MARCOS method using novel score function and its application to solid waste management. Appl. Intell. 2021, 1–23. [Google Scholar] [CrossRef]
- Vesković, S.; Stević, Ž.; Nunić, Z.; Milinković, S.; Mladenović, D. A novel integrated large-scale group MCDM model under fuzzy environment for selection of reach stacker in a container terminal. Appl. Intell. 2022, 1–25. [Google Scholar] [CrossRef]
- Darko, A.P.; Liang, D. A heterogeneous opinion-driven decision-support model for tourists’ selection with different travel needs in online reviews. J. Oper. Res. Soc. 2022, 1–18. [Google Scholar] [CrossRef]
- Boral, S.; Chaturvedi, S.K.; Howard, I.M.; McKee, K.; Naikan, V.A. An integrated approach for fuzzy failure mode and effect analysis using fuzzy AHP and fuzzy MARCOS. In Proceedings of the 2020 IEEE International Conference on Industrial Engineering and Engineering Management (IEEM), Singapore, 14–17 December 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 395–400. [Google Scholar]
- Yazdani, M.; Torkayesh, A.E.; Stević, Ž.; Chatterjee, P.; Ahari, S.A.; Hernandez, V.D. An interval valued neutrosophic decision-making structure for sustainable supplier selection. Expert Syst. Appl. 2021, 183, 115354. [Google Scholar] [CrossRef]
- Boral, S.; Chaturvedi, S.K.; Howard, I.; Naikan, V.N.A.; McKee, K. An integrated interval type-2 fuzzy sets and multiplicative half quadratic programming-based MCDM framework for calculating aggregated risk ranking results of failure modes in FMECA. Process Saf. Environ. Prot. 2021, 150, 194–222. [Google Scholar] [CrossRef]
- Mešić, A.; Miškić, S.; Stević, Ž.; Mastilo, Z. Hybrid MCDM solutions for evaluation of the logistics performance index of the western balkan countries. Econ. Innov. Res. J. 2022, 10, 13–34. [Google Scholar]
- Loomes, G.; Sugden, R. Regret theory: An alternative theory of rational choice under uncertainty. Econ. J. 1982, 92, 805–824. [Google Scholar] [CrossRef]
- Tversky, A.; Kahneman, D. Advances in prospect theory: Cumulative representation of uncertainty. J. Risk Uncertain. 1992, 5, 297–323. [Google Scholar] [CrossRef]
- Quiggin, J. Regret theory with general choice sets. J. Risk Uncertain. 1994, 8, 153–165. [Google Scholar] [CrossRef]
- Rezaei, J. Best-worst multi-criteria decision-making method. Omega 2015, 53, 49–57. [Google Scholar] [CrossRef]
- Diakoulaki, D.; Mavrotas, G.; Papayannakis, L. Determining objective weights in multiple criteria problems: The critic method. Comput. Oper. Res. 1995, 22, 763–770. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, Y.; Gong, C.; Dinçer, H.; Yüksel, S. An integrated hesitant 2-tuple Pythagorean fuzzy analysis of QFD-based innovation cost and duration for renewable energy projects. Energy 2022, 248, 123561. [Google Scholar] [CrossRef]
- Rani, P.; Mishra, A.R.; Saha, A.; Hezam, I.M.; Pamucar, D. Fermatean fuzzy Heronian mean operators and MEREC-based additive ratio assessment method: An application to food waste treatment technology selection. Int. J. Intell. Syst. 2022, 37, 2612–2647. [Google Scholar] [CrossRef]
- Mishra, A.R.; Liu, P.; Rani, P. COPRAS method based on interval-valued hesitant Fermatean fuzzy sets and its application in selecting desalination technology. Appl. Soft Comput. 2022, 119, 108570. [Google Scholar] [CrossRef]


| Reference | Score Function | Accuracy Function |
|---|---|---|
| Smarandache [22] | ||
| Sahin [11] | ||
| Garg [14] | ||
| Nafei et al. [23] |
| Reference | Case 1 | Case 2 | Case 3 | Case 4 |
|---|---|---|---|---|
| Smarandache [22] | ||||
| Sahin [11] | ||||
| Garg [14] | ||||
| Nafei et al. [23] | ||||
| Proposed SVN scorefunction |
| Linguistic Term | Abbreviation | SVNNs |
|---|---|---|
| Very Very Low | VVL | (0.00, 0.10, 0.10) |
| Very Low | VL | (0.10, 0.90, 0.90) |
| Low | L | (0.30, 0.75, 0.70) |
| Moderately Low | ML | (0.40, 0.65, 0.60) |
| Middle | M | (0.50, 0.50, 0.50) |
| Moderately High | MH | (0.60, 0.35, 0.40) |
| High | H | (0.70, 0.25, 0.30) |
| Very High | VH | (0.80, 0.15, 0.20) |
| Very Very High | VVH | (0.90, 0.10, 0.10) |
| Extremely High | EH | (1.00, 0.00, 0.00) |
| Criteria | Description | Type |
|---|---|---|
| Effective supply ability () | This refers to the enterprise’s supply capacity of raw materials and instrument and equipment consumables involved in the product production process. It is embodied in whether the supply materials of main products are sufficient, whether the mechanical materials consumed by equipment are sufficient and whether the necessities required by employees in the production process are sufficient. | Benefit |
| Scientific and technological innovation ability () | This refers to the ability of enterprises to continuously innovate and develop new products by paying attention to social development and citizens’ needs in the process of product research and development. It is reflected in the number of international professional papers published in the research and development process, the number of patent projects and the amount of funds for achievement transformation. | Benefit |
| Transnational cooperation ability () | This refers to the ability of enterprises to cooperate with enterprises in other countries to achieve mutual benefit and win-win results in order to accelerate the diversified development of industries. It is comprehensively evaluated from the aspects of the number of cooperation between enterprises and foreign enterprises, the extent of geographical coverage, benefits and so on. | Benefit |
| Efficient operation ability () | This refers to the ability of an enterprise to maximize benefits through resource integration and process optimization by using limited resources, personnel and equipment in the production process. It is embodied by the level of personnel scheduling, the ability of resource optimization and integration and the ability of emergency light in production process optimization. | Benefit |
| market development ability () | This refers to the level at which an enterprise sells its products through various ways and strategies in product sales. It is specifically reflected in the sales scope of the product market, product sales channels and product sales to comprehensively evaluate the market development ability of the enterprise. | Benefit |
| Green development ability () | This refers to the ability of enterprises to follow the concept of green development in the production process and ensure environmental safety while developing the economy. It is specifically reflected in the level of pollutant emissions, the amount of energy consumed and whether there is complete environmental protection equipment and pollutant treatment equipment. | Benefit |
| Social contribution ability () | This refers to the overall impact and comprehensive benefits brought by enterprises to society in the process of production. It is embodied in production safety management, ensuring the harmonious development of society and ensuring the legitimate rights and interests of employees in the production process. | Benefit |
| Expert | ||||||||
|---|---|---|---|---|---|---|---|---|
| Pharmaceutical Enterprises | ||||||||
| VH | H | VH | VH | VH | M | MH | ||
| MH | VL | VH | MH | VH | H | MH | ||
| H | ML | H | ML | VVH | H | ML | ||
| H | VH | M | H | VH | M | MH | ||
| M | MH | ML | VVH | MH | VVH | M | ||
| MH | M | MH | VVH | H | VH | ML | ||
| MH | MH | VH | H | VH | MH | MH | ||
| MH | H | VH | MH | MH | H | ML | ||
| M | L | VH | H | MH | MH | M | ||
| VH | VL | MH | VVH | M | VVH | ML | ||
| VH | H | VH | H | MH | VH | M | ||
| H | VH | M | VH | VVH | VH | MH | ||
| M | M | MH | ML | L | M | VL | ||
| MH | H | M | M | VH | ML | M | ||
| M | VH | ML | H | H | MH | MH | ||
| VVH | H | M | VH | VH | M | ML | ||
| M | VH | ML | MH | H | VH | MH | ||
| MH | H | VH | H | M | H | M | ||
| MH | M | L | H | H | VH | VH | ||
| H | ML | ML | MH | MH | VH | MH | ||
| VH | L | M | H | MH | VH | M | ||
| VH | M | ML | MH | VVH | MH | M | ||
| MH | ML | MH | VH | VH | M | VH | ||
| MH | M | M | VVH | VH | VH | VVH |
| Best-others criteria | |||||||
| 2 | 2 | 8 | 3 | 2 | 1 | 3 | |
| Others-worst criteria | |||||||
| 2 | 3 | 1 | 2 | 4 | 8 | 5 |
| Pharmaceutical Enterprises | |||||||
|---|---|---|---|---|---|---|---|
| 1.3357 | 1.0841 | 1.2734 | 1.4851 | 1.5695 | 1.2406 | 1.1030 | |
| 1.2664 | 0.7668 | 1.4114 | 1.0695 | 1.6129 | 1.4861 | 0.8779 | |
| 1.3521 | 0.6423 | 1.2800 | 1.2875 | 1.7007 | 1.5209 | 0.7511 | |
| 2.0643 | 1.0232 | 0.7695 | 1.8939 | 1.9400 | 1.5450 | 0.6954 | |
| 1.2373 | 1.2963 | 1.0810 | 2.0988 | 1.5017 | 1.4295 | 1.2231 | |
| 1.2650 | 1.3100 | 1.1717 | 2.1126 | 1.8697 | 1.9497 | 1.3784 |
| Pharmaceutical Enterprises | |||||||
|---|---|---|---|---|---|---|---|
| −0.2443 | −0.0701 | −0.0423 | −0.2071 | −0.1176 | −0.2371 | −0.0861 | |
| −0.2705 | −0.1770 | 0.0000 | −0.3674 | −0.1031 | −0.1492 | −0.1620 | |
| −0.2382 | −0.2218 | −0.0402 | −0.2808 | −0.0744 | −0.1373 | −0.2071 | |
| 0.0000 | −0.0898 | −0.2124 | −0.0678 | 0.0000 | −0.1291 | −0.2274 | |
| −0.2816 | −0.0041 | −0.1042 | −0.0041 | −0.1405 | −0.1689 | −0.0477 | |
| −0.2710 | 0.0000 | −0.0746 | 0.0000 | −0.0213 | 0.0000 | 0.0000 |
| Pharmaceutical Enterprises | |||||||
|---|---|---|---|---|---|---|---|
| 1.0915 | 1.0140 | 1.2311 | 1.2780 | 1.4520 | 1.0035 | 1.0168 | |
| 0.9959 | 0.5898 | 1.4114 | 0.7021 | 1.5098 | 1.3368 | 0.7158 | |
| 1.1139 | 0.4206 | 1.2397 | 1.0066 | 1.6262 | 1.3836 | 0.5440 | |
| 2.0643 | 0.9334 | 0.5571 | 1.8261 | 1.9400 | 1.4158 | 0.4680 | |
| 0.9558 | 1.2921 | 0.9768 | 2.0947 | 1.3612 | 1.2605 | 1.1754 | |
| 0.9940 | 1.3100 | 1.0972 | 2.1126 | 1.8483 | 1.9497 | 1.3784 |
| Pharmaceutical Enterprises | |||||||
|---|---|---|---|---|---|---|---|
| AID | 0.9558 | 0.4206 | 0.5571 | 0.7021 | 1.3612 | 1.0035 | 0.4680 |
| 1.0915 | 1.0140 | 1.2311 | 1.2780 | 1.4520 | 1.0035 | 1.0168 | |
| 0.9959 | 0.5898 | 1.4114 | 0.7021 | 1.5098 | 1.3368 | 0.7158 | |
| 1.1139 | 0.4206 | 1.2397 | 1.0066 | 1.6262 | 1.3836 | 0.5440 | |
| 2.0643 | 0.9334 | 0.5571 | 1.8261 | 1.9400 | 1.4158 | 0.4680 | |
| 0.9558 | 1.2921 | 0.9768 | 2.0947 | 1.3612 | 1.2605 | 1.1754 | |
| 0.9940 | 1.3100 | 1.0972 | 2.1126 | 1.8483 | 1.9497 | 1.3784 | |
| ID | 2.0643 | 1.0140 | 1.4114 | 1.8261 | 1.9400 | 1.4158 | 1.0168 |
| Weight Type | Ranking Values | Sorting | |||||
|---|---|---|---|---|---|---|---|
| Objective weight | 0.5566 | 0.6651 | 0.6949 | 0.7938 | 0.7047 | 0.9895 | |
| Subjective weight | 0.5717 | 0.6244 | 0.6474 | 0.7949 | 0.6970 | 0.9022 | |
| Combinative weight | 0.5613 | 0.6426 | 0.6715 | 0.7974 | 0.7032 | 0.9518 | |
| Equal weight | 0.6184 | 0.5865 | 0.6015 | 0.7813 | 0.7220 | 0.8430 | |
| Ranking Values | Rank | |||||||
|---|---|---|---|---|---|---|---|---|
| 0.1 | 0.9 | 0.5689 | 0.6270 | 0.6519 | 0.7962 | 0.6987 | 0.9130 | |
| 0.2 | 0.8 | 0.5665 | 0.6303 | 0.6566 | 0.7970 | 0.7001 | 0.9234 | |
| 0.3 | 0.7 | 0.5645 | 0.6341 | 0.6615 | 0.7975 | 0.7014 | 0.9333 | |
| 0.4 | 0.6 | 0.5627 | 0.6383 | 0.6665 | 0.7976 | 0.7024 | 0.9428 | |
| 0.5 | 0.5 | 0.5613 | 0.6426 | 0.6715 | 0.7974 | 0.7032 | 0.9518 | |
| 0.6 | 0.4 | 0.5600 | 0.6472 | 0.6764 | 0.7970 | 0.7038 | 0.9604 | |
| 0.7 | 0.3 | 0.5590 | 0.6517 | 0.6813 | 0.7964 | 0.7043 | 0.9684 | |
| 0.8 | 0.2 | 0.5581 | 0.6563 | 0.6860 | 0.7956 | 0.7045 | 0.9759 | |
| 0.9 | 0.1 | 0.5573 | 0.6607 | 0.6906 | 0.7948 | 0.7047 | 0.9830 | |
| 1.0 | 0 | 0.5566 | 0.6651 | 0.6949 | 0.7938 | 0.7047 | 0.9895 | |
| Ranking Values | Rank | |||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 1 | 0.6003 | 0.5937 | 0.6184 | 0.7733 | 0.7100 | 0.8831 | |
| 1 | 2 | 0.5768 | 0.6389 | 0.6677 | 0.7874 | 0.7123 | 0.9478 | |
| 1 | 5 | 0.5556 | 0.6972 | 0.7240 | 0.7803 | 0.7021 | 1.0201 | |
| 2 | 1 | 0.5718 | 0.6027 | 0.6295 | 0.7958 | 0.6983 | 0.8963 | |
| 2 | 2 | 0.5613 | 0.6426 | 0.6715 | 0.7974 | 0.7032 | 0.9518 | |
| 2 | 5 | 0.5516 | 0.6927 | 0.7201 | 0.7857 | 0.6994 | 1.0158 | |
| 5 | 5 | 0.5438 | 0.7145 | 0.7399 | 0.7807 | 0.6966 | 1.0446 | |
| 5 | 10 | 0.5458 | 0.7210 | 0.7465 | 0.7773 | 0.6929 | 1.0532 | |
| Approaches | Ranking Values | Sorting | |||||
|---|---|---|---|---|---|---|---|
| SVNWA operator; [12] | 0.7974 | 0.7925 | 0.8027 | 0.8691 | 0.8445 | 0.9011 | |
| SVNWG operator [12] | 0.7939 | 0.7796 | 0.7842 | 0.8359 | 0.8303 | 0.8849 | |
| SVNEWA operator [12] | 0.7969 | 0.7908 | 0.8004 | 0.8655 | 0.8427 | 0.8994 | |
| SVNEWG operator [12] | 0.8642 | 0.8521 | 0.8539 | 0.8934 | 0.8846 | 0.9167 | |
| SVN-WSM [25] | 0.3178 | 0.3110 | 0.3249 | 0.4347 | 0.3917 | 0.5042 | |
| SVN-WPM [25] | 0.3122 | 0.2907 | 0.2942 | 0.3733 | 0.3679 | 0.4693 | |
| SVN-WASPAS [25] | 0.3150 | 0.3008 | 0.3096 | 0.4040 | 0.3798 | 0.4867 | |
| SVN-TOPSIS [17] | 0.0409 | 0.1022 | 0.1445 | 0.3659 | 0.2122 | 0.5299 | |
| Methods | Calculation of Experts Weight | Criteria Weight | Ranking Algorithm | Consider Expert Psychological Factor |
|---|---|---|---|---|
| SVNWA operator [12] | Subjective | Subjective | aggregation | NO |
| SVNWG operator [12] | Subjective | Subjective | aggregation | NO |
| SVNEWA operator [12] | Subjective | Subjective | aggregation | NO |
| SVNEWG operator [12] | Subjective | Subjective | aggregation | NO |
| SVN-WSM method [25] | Subjective | Combined weight | WSM | NO |
| SVN-WPM method [25] | Subjective | Combined weight | WPM | NO |
| SVN-WASPAS method [25] | Subjective | Combined weight | WASPAS | NO |
| SVN-TOPSIS method [17] | Subjective | Subjective | TOPSIS | NO |
| SVN regret theory-MARCOS method | Objective | Combined weight | MARCOS | YES |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rong, Y.; Niu, W.; Garg, H.; Liu, Y.; Yu, L. A Hybrid Group Decision Approach Based on MARCOS and Regret Theory for Pharmaceutical Enterprises Assessment under a Single-Valued Neutrosophic Scenario. Systems 2022, 10, 106. https://doi.org/10.3390/systems10040106
Rong Y, Niu W, Garg H, Liu Y, Yu L. A Hybrid Group Decision Approach Based on MARCOS and Regret Theory for Pharmaceutical Enterprises Assessment under a Single-Valued Neutrosophic Scenario. Systems. 2022; 10(4):106. https://doi.org/10.3390/systems10040106
Chicago/Turabian StyleRong, Yuan, Wenyao Niu, Harish Garg, Yi Liu, and Liying Yu. 2022. "A Hybrid Group Decision Approach Based on MARCOS and Regret Theory for Pharmaceutical Enterprises Assessment under a Single-Valued Neutrosophic Scenario" Systems 10, no. 4: 106. https://doi.org/10.3390/systems10040106
APA StyleRong, Y., Niu, W., Garg, H., Liu, Y., & Yu, L. (2022). A Hybrid Group Decision Approach Based on MARCOS and Regret Theory for Pharmaceutical Enterprises Assessment under a Single-Valued Neutrosophic Scenario. Systems, 10(4), 106. https://doi.org/10.3390/systems10040106







