Depolarization of Light in Optical Fibers: Effects of Diffraction and Spin-Orbit Interaction
Abstract
1. Introduction
2. 2D Polarization
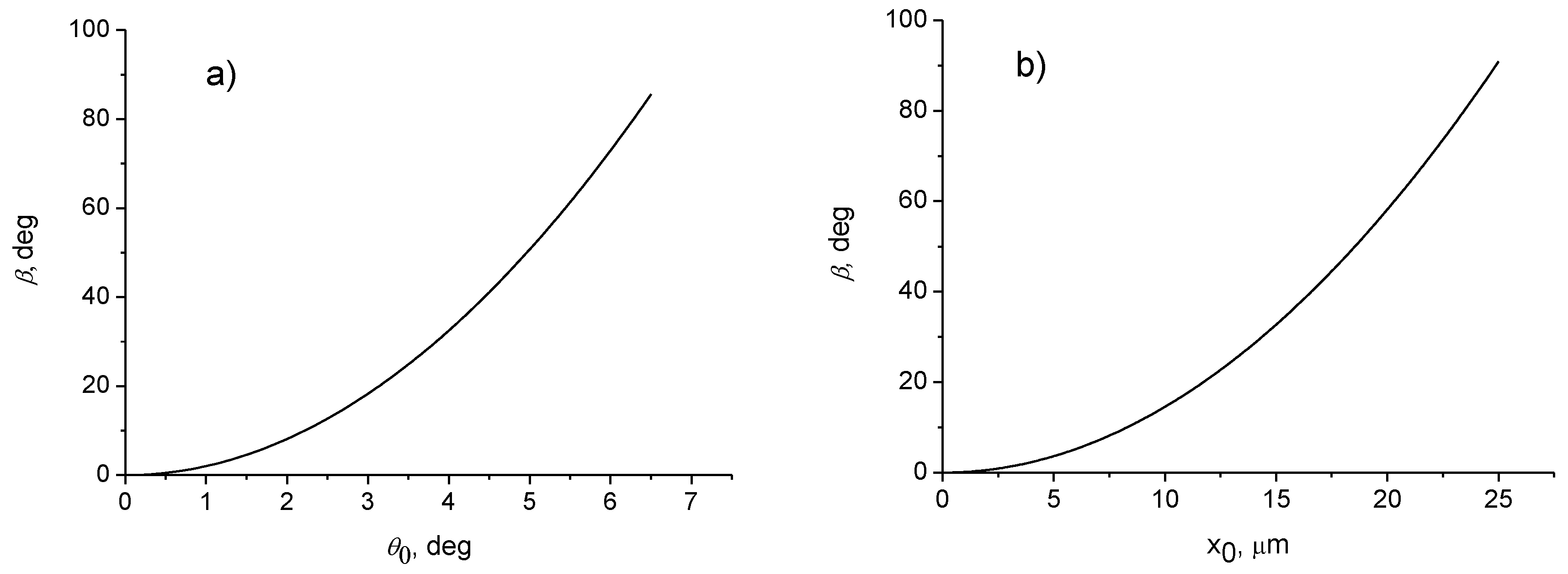
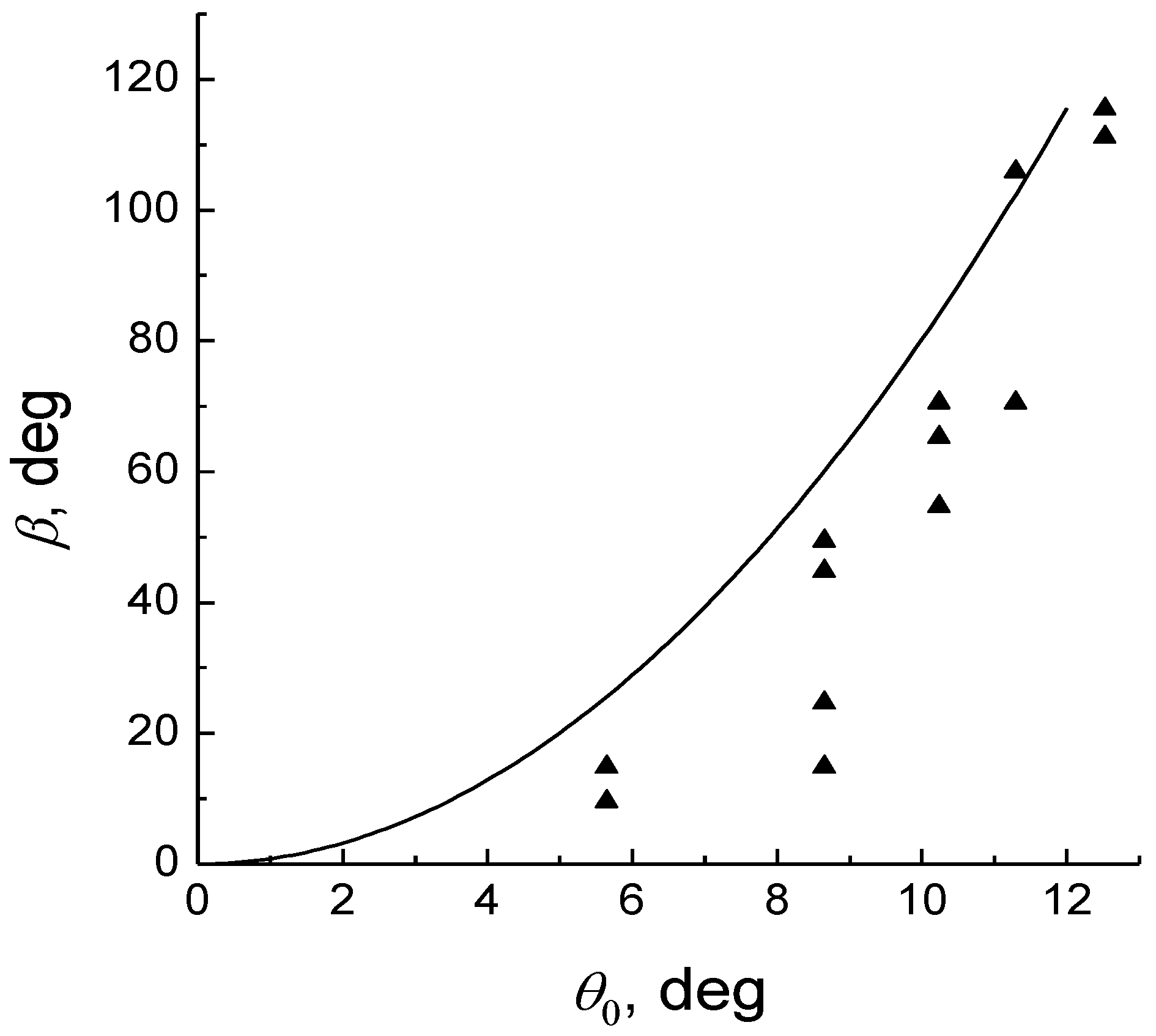
2.1. Rotation of Polarization Plane in a Graded Index Fiber
2.2. Depolarization of Light
2.2.1. Linear Polarization
2.2.2. Circular Polarization
2.3. Berry Phase and Degree of Polarization
3. 3D Polarization
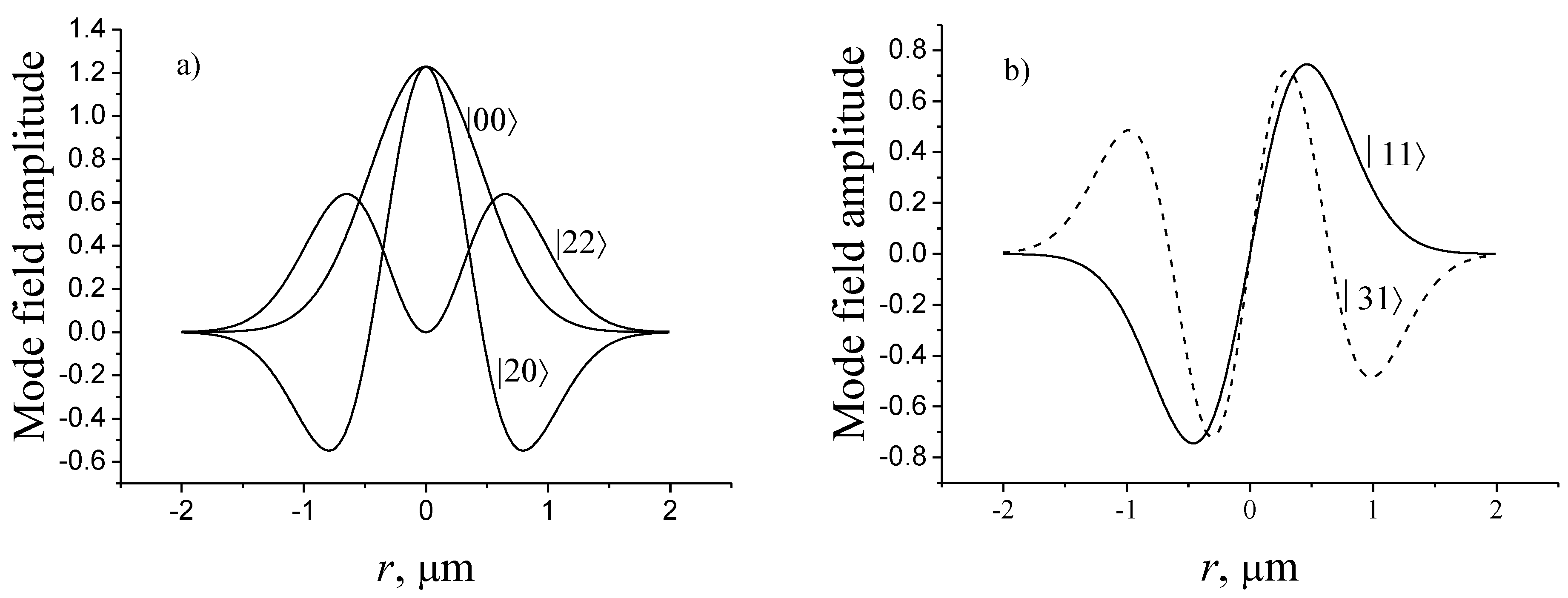
3.1. Vector Laguerre–Gauss (LG) Beams in a Graded-Index Fiber
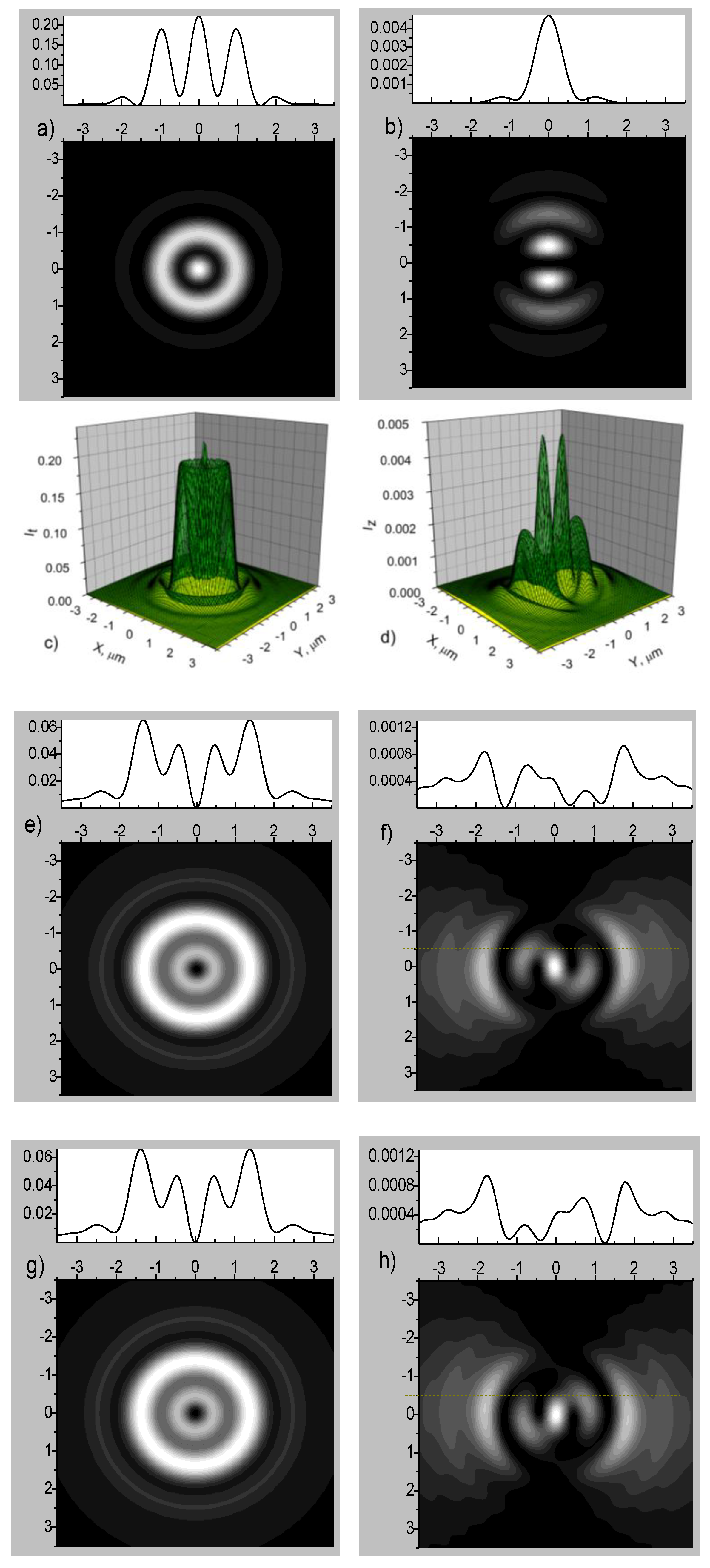
Simulation Results
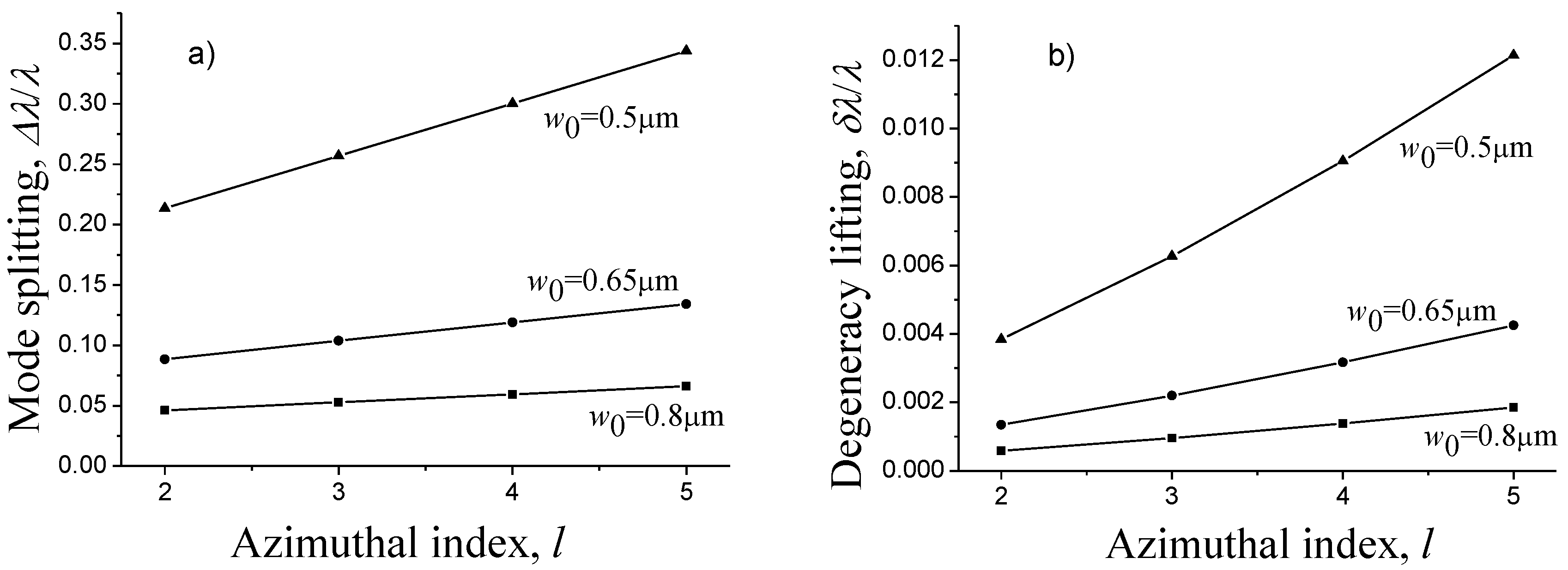
3.2. The Splitting of the Degenerate Modes
3.3. Simulation of Delay Time and Group Delay Splitting
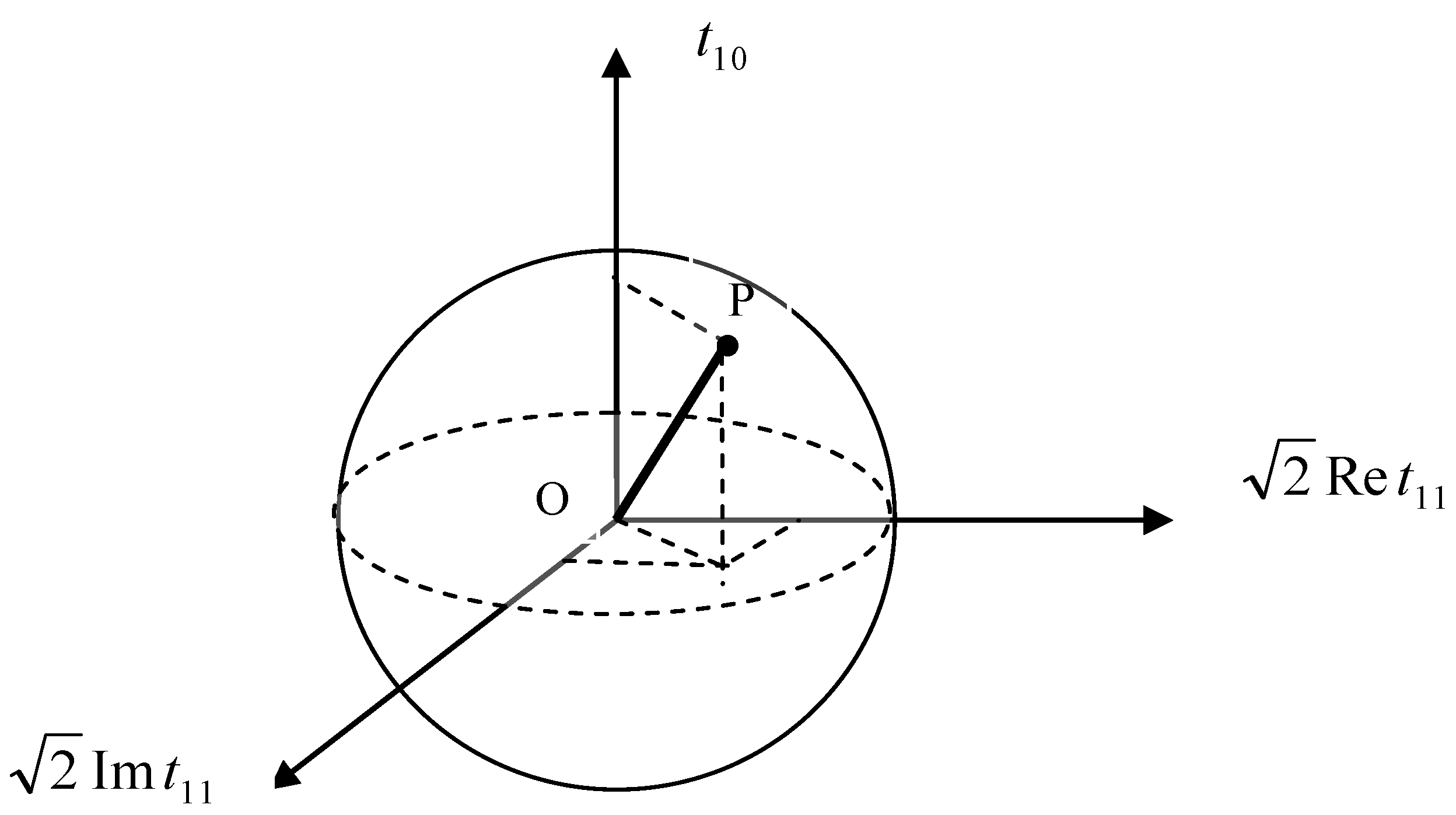
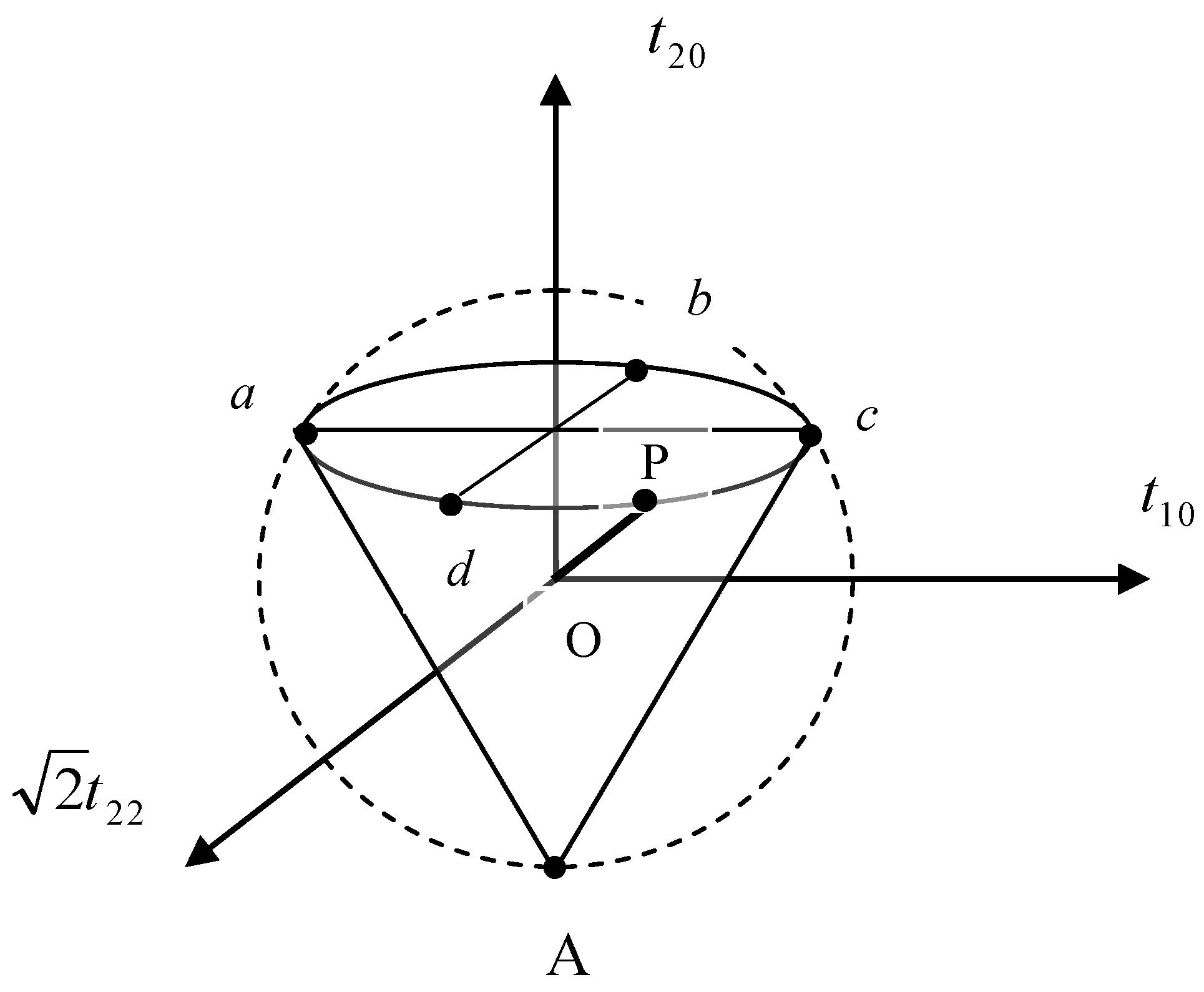
3.4. Vector and Tensor Polarization Degrees of Light
4. Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Azzam, R.M.A.; Bashara, N.M. Ellipsometry and Polarized Light; North-Holland: Amsterdam, The Netherlands, 1977. [Google Scholar]
- Wolf, E. Introduction to the Theory of Coherence and Polarization of Light; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Tatarskii, V.I. Estimation of light depolarization by turbulent inhomogeneities of the atmosphere. Izv. VUZov Radiofiz. 1967, 10, 1762–1765. [Google Scholar]
- Kravtzov, Y.A. Geometrical depolarization of light in a turbulent atmosphere. Izv. VUZov Radiofiz. 1970, 13, 281–284. [Google Scholar]
- Rytov, S.M. On transition from wave to geometrical optics. Dokl. Akad. Nauk USSR 1938, 18, 263–266. [Google Scholar]
- Vladimirsky, V.V. On rotation of polarization plane in twisted ray of light. Dokl. Akad. Nauk USSR 1941, 31, 222–225. [Google Scholar]
- Cohen, L.G. Measured attenuation and depolarization of light transmitted along glass fibers. Bell Syst. Tech. J. 1971, 50, 23–42. [Google Scholar] [CrossRef]
- Smith, A.M. Polarization and magnetooptic properties of single-mode optical fiber. Appl. Opt. 1978, 17, 52–56. [Google Scholar] [CrossRef] [PubMed]
- Kaminov, I.P. Polarization in optical fibers. IEEE J. Quant. Electron. 1981, 17, 15–22. [Google Scholar] [CrossRef]
- Shatrov, A.D. Polarization effects in multimode graded-index fibers. Radiotekh. Elektr. 1988, 26, 505–512. [Google Scholar]
- Esayan, A.A.; Zel’dovich, B.Y. Depolarization of radiation in an ideal multimode graded-index optical fiber. Soviet J. Quantum Electron. 1988, 18, 149–150. [Google Scholar] [CrossRef]
- Kotov, O.I.; Marusov, O.L.; Nikolaev, O.L.; Filippov, V.N. Polarization properties of optical fibers. Modal approach. Opt. Spectr. 1991, 70, 924–927. [Google Scholar]
- Zel’dovich, B.Y.; Kundikova, N.D. Intrafibre rotation of the plane of polarization. Quantum Electron. 1995, 25, 172–174. [Google Scholar] [CrossRef]
- Zel’dovich, B.Y.; Liberman, V.S. Rotation of the plane of a meridional beam in a graded-index waveguide due to the circular nature of the polarization. Soviet J. Quantum Electron. 1990, 20, 427–428. [Google Scholar] [CrossRef]
- Zel’dovich, B.Y.; Kitaevskaya, I.V.; Kundikova, I.D. Inhomogeneity of the optical Magnus effect. Quantum Electron. 1996, 26, 87–88. [Google Scholar] [CrossRef]
- Petrov, N.I. Depolarization of light in a graded-index isotropic medium. J. Mod. Opt. 1996, 43, 2239–2249. [Google Scholar] [CrossRef]
- Petrov, N.I. Evolution of polarization in an inhomogeneous isotropic medium. JETP 1997, 85, 1085–1093. [Google Scholar] [CrossRef]
- Matthews, S.C.; Rockwell, D.A. Correction of phase and depolarization distortions in a multimode fiber at 1.064 μm with stimulated-Brillouin-scattering phase conjugation. Opt. Lett. 1994, 19, 1729–1731. [Google Scholar] [CrossRef] [PubMed]
- Roychowdhury, H.; Agrawal, G.P.; Wolf, E. Changes in the spectrum, in the spectral degree of polarization, and in the spectral degree of coherence of a partially coherent beam propagating through a gradient-index fiber. J. Opt. Soc. Am. A 2006, 23, 940–948. [Google Scholar] [CrossRef] [PubMed]
- Huang, W.; Ponomarenko, S.A.; Cada, M.; Agrawal, G.P. Polarization changes of partially coherent pulses propagating in optical fibers. JOSA A 2007, 24, 3063–3068. [Google Scholar] [CrossRef] [PubMed]
- Bliokh, K.Y.; Desyatnikov, A.S. Spin and orbital Hall effects for diffracting optical beams in gradient-index media. Phys. Rev. A 2009, 79, 011807. [Google Scholar] [CrossRef]
- Bliokh, K.Y. Geometrodynamics of polarized light: Berry phase and spin Hall effect in a gradient-index medium. J. Opt. A Pure Appl. Opt. 2009, 11, 094009. [Google Scholar] [CrossRef]
- Chakravarthy, T.P.; Viswanatham, N.K. Direct and reciprocal spin-orbit interaction effects in a graded-index medium. OSA Contin. 2019, 2, 1576–1589. [Google Scholar] [CrossRef]
- Dugin, A.; Zel’dovich, B.; Kundikova, N.; Liberman, V. Effect of circular polarization on the propagation of light through an optical fiber. J. Exp. Theor. Phys. Lett. 1991, 53, 197–199. [Google Scholar]
- Petrov, N.I. Evolution of 3D Polarization in Inhomogeneous Medium. In Proceedings of the Frontiers in Optics 2007, San Jose, CA, USA, 16–20 September 2007. [Google Scholar] [CrossRef]
- Petrov, N.I. Splitting of levels in a cylindrical dielectric waveguide. Opt. Lett. 2013, 38, 2020–2022. [Google Scholar] [CrossRef] [PubMed]
- Petrov, N.I. Spin-dependent transverse force on a vortex light beam in an inhomogeneous medium. JETP Lett. 2016, 103, 443–448. [Google Scholar] [CrossRef]
- Petrov, N.I. Vector Laguerre–Gauss beams with polarization-orbital angular momentum entanglement in a graded-index medium. J. Opt. Soc. Am. A 2016, 33, 1363–1369. [Google Scholar] [CrossRef] [PubMed]
- Arnaud, J.A. Beam and Fiber Optics; Academic Press: New York, NY, USA, 1976. [Google Scholar]
- Tomita, A.; Chiao, R.Y. Observation of Berry’s Topological Phase by Use of an Optical Fiber. Phys. Rev. Lett. 1986, 57, 937–940. [Google Scholar] [CrossRef] [PubMed]
- Berry, M.V. Quantal phase factors accompanying adiabatic changes. Proc. R. Soc. Lond. A 1984, 392, 45–57. [Google Scholar]
- Imbert, C. Calculation and experimental proof of the transverse shift induced by total internal reflection of a circularly polarized light beam. Phys. Rev. D 1972, 5, 787–796. [Google Scholar] [CrossRef]
- Goos, F.; Hanchen, H. Ein neuer und fundamentaler versuch zur total reflexion. Ann. Phys. 1947, 1, 333–345. [Google Scholar] [CrossRef]
- Petrov, N.I. Reflection and transmission of strongly focused light beams at a dielectric interface. J. Mod. Opt. 2005, 52, 1545–1556. [Google Scholar] [CrossRef]
- Petrov, N.I. The influence of polarization on the trajectory and the width of a radiation beam in an inhomogeneous medium. Las. Phys. 2000, 10, 619–621. [Google Scholar]
- Bliokh, K.Y.; Niv, A.; Kleiner, V.; Hasman, E. Geometrodynamics of spinning light. Nat. Photon. 2008, 2, 748–753. [Google Scholar] [CrossRef]
- Liberman, V.S.; Zel’dovich, B.Y. Spin-orbit interaction of a photon in an inhomogeneous medium. Phys. Rev. A 1992, 46, 5199–5207. [Google Scholar] [CrossRef] [PubMed]
- Onoda, M.; Murakami, S.; Nagaosa, N. Hall Effect of Light. Phys. Rev. Lett. 2004, 93, 083901. [Google Scholar] [CrossRef] [PubMed]
- Kavokin, A.; Malpuech, G.; Glazov, M. Optical Spin Hall Effect. Phys. Rev. Lett. 2005, 95, 136601. [Google Scholar] [CrossRef] [PubMed]
- Snyder, A.W.; Love, J.D. Optical Waveguide Theory; Chapman and Hall: London, UK, 1983. [Google Scholar]
- Sodha, M.S.; Ghatak, A.K. Inhomogeneous Optical Waveguides; Plenum Press: New York, NY, USA, 1977. [Google Scholar]
- Loffler, W.; Van Exter, M.P.; Hooft, G.W.; Eliel, E.R.; Hermans, K.; Broer, D.J.; Woerdman, J.P. Polarization-dependent Goos–Hänchen shift at a graded dielectric interface. Opt. Commun. 2010, 283, 3367–3370. [Google Scholar] [CrossRef]
- Petrov, N.I. Beam shift in a graded-index optical fiber. J. Opt. 2013, 15, 014011. [Google Scholar] [CrossRef]
- Glauber, R.J. Coherent and Incoherent States of the Radiation Field. Phys. Rev. 1963, 131, 2766–2788. [Google Scholar] [CrossRef]
- Schrodinger, E. Der stetige Übergang von der Mikro-zur Makromechanik. Naturwissenschaften 1926, 14, 664–666. [Google Scholar] [CrossRef]
- Krivoshlykov, S.G.; Sissakian, I.N. Coherent states and light propagation in inhomogeneous media. Soviet J. Quantum Electron. 1980, 10, 312–318. [Google Scholar] [CrossRef]
- Krivoshlykov, S.G.; Petrov, N.I.; Sisakyan, I.N. Density-matrix formalism for partially coherent optical fields propagating in slightly inhomogeneous media. Opt. Quant. Electr. 1986, 18, 253–264. [Google Scholar] [CrossRef]
- Petrov, N.I.; Petrova, G.N. Diffraction of partially-coherent light beams by microlens arrays. Opt. Exp. 2017, 25, 22545–22564. [Google Scholar] [CrossRef] [PubMed]
- Hansson, T.; Tonello, A.; Mansuryan, T.; Mangini, F.; Zitelli, M.; Ferraro, M.; Niang, A.; Crescenzi, R.; Wabnitz, S.; Couderc, V. Nonlinear beam self-imaging and self-focusing dynamics in a GRIN multimode optical fiber: Theory and experiments. Opt. Exp. 2020, 16, 24005–24021. [Google Scholar] [CrossRef] [PubMed]
- Mangini, F.; Ferraro, M.; Zitelli, M.; Niang, A.; Tonello, A.; Couderc, V.; Sidelnikov, O.; Frezza, F.; Wabnitz, S. Experimental observation of self-imaging in SMF-28 optical fibers. Opt. Exp. 2021, 8, 12625–12633. [Google Scholar] [CrossRef] [PubMed]
- Merano, M.; Hermosa, N.; Aiello, A.; Woerdman, J.P. Demonstration of a quasi-scalar angular Goos–Hänchen effect. Opt. Lett. 2010, 35, 3562–3564. [Google Scholar] [CrossRef] [PubMed]
- Petrov, N.I.; Danilov, V.A.; Popov, V.V.; Usievich, B.A. Large positive and negative Goos-Hänchen shifts near the surface plasmon resonance in subwavelength grating. Opt. Exp. 2020, 28, 7552–7564. [Google Scholar] [CrossRef] [PubMed]
- Petrov, N.I. Focusing of beams into subwavelength area in an inhomogeneous medium. Opt. Exp. 2001, 9, 658–673. [Google Scholar] [CrossRef] [PubMed]
- Petrov, N.I. Remote focusing of a light beam. Las. Phys. Lett. 2016, 13, 015101. [Google Scholar] [CrossRef]
- Tychinskii, V.P. Microscopy of subwavelength structures. Phys. Uspekhi 1996, 39, 1157–1167. [Google Scholar] [CrossRef]
- Sato, M.; Eto, K.; Masuta, J.; Nishidate, I. Depolarization characteristics of spatial modes in imaging probe using short multimode fiber. Appl. Opt. 2018, 57, 10083–10091. [Google Scholar] [CrossRef] [PubMed]
- Petrov, N.I. Evolution of Berry’s phase in a graded-index medium. Phys. Lett. A 1997, 234, 239–250. [Google Scholar] [CrossRef]
- Carozzi, T.; Karlsson, R.; Bergman, J. Parameters characterizing electromagnetic wave polarization. Phys. Rev. E 2000, 61, 2024–2028. [Google Scholar] [CrossRef] [PubMed]
- Setala, T.; Shevchenko, A.; Kaivola, M.; Friberg, A.T. Degree of polarization for optical near fields. Phys. Rev. E 2002, 66, 016615. [Google Scholar] [CrossRef] [PubMed]
- Dennis, M.R. Geometric interpretation of the three-dimensional coherence matrix for nonparaxial polarization. Pure Appl. Opt. 2004, 6, S26–S31. [Google Scholar] [CrossRef]
- Petrov, N.I. Vector and tensor polarizations of light beams. Laser Phys. 2008, 18, 522–525. [Google Scholar] [CrossRef]
- Petrov, N.I. Spin-orbit and tensor interactions of light in inhomogeneous isotropic media. Phys. Rev. A 2013, 88, 023815. [Google Scholar] [CrossRef]
- Feit, M.D.; Fleck, J.A. Light propagation in graded-index optical fibers. Appl. Opt. 1978, 17, 3990–3998. [Google Scholar] [CrossRef] [PubMed]
- Krivoshlykov, S.G.; Sisakyan, I.N. Coherent states and nonparaxial propagation of light in graded-index media. Soviet J. Quantum Electron. 1983, 13, 455–458. [Google Scholar] [CrossRef]
- Petrov, N.I. Physico-Mathematical Sciences. Ph.D. Thesis, General Physics Institute, Russian Academy of Sciences, Moscow, Russia, 1985. [Google Scholar]
- Krivoshlykov, S.G.; Petrov, N.I.; Sissakian, I.N. Correlated coherent states and propagation of arbitrary Gaussian beams in longitudinally homogeneous quadratic media exhibiting absorption or amplification. Soviet J. Quantum Electron. 1986, 16, 933–941. [Google Scholar] [CrossRef]
- Krivoshlykov, S.G. Non-paraxial propagation of Gaussian beam rays in graded-index waveguides and effect of large-scale initial field revival. J. Mod. Opt. 1992, 39, 723–736. [Google Scholar] [CrossRef]
- Petrov, N.I. Mode structure formation length in an inhomogeneous medium. Laser Phys. 1998, 8, 1245–1248. [Google Scholar]
- Petrov, N.I. Nonparaxial focusing of wave beams in a graded-index medium. Quantum Electron. 1999, 29, 249–255. [Google Scholar] [CrossRef]
- Petrov, N.I. Macroscopic quantum effects for classical light. Phys. Rev. A 2014, 90, 043814. [Google Scholar] [CrossRef]
- Arrizon, V.; Soto-Eguibar, F.; Zuniga-Segundo, A.; Moya-Cessa, H.M. Revival and splitting of a Gaussian beam in gradient index media. JOSA A 2015, 32, 1140. [Google Scholar] [CrossRef] [PubMed]
- Karimi, E.; Santamoto, E. Radial coherent and intelligent states of paraxial wave equation. Opt. Lett. 2012, 37, 2484. [Google Scholar] [CrossRef] [PubMed]
- Hermoza, N.; Aiello, A.; Woerdman, J.P. Radial mode dependence of optical beam shifts. Opt. Lett. 2012, 37, 1044. [Google Scholar] [CrossRef]
- Plick, W.N.; Lapkiewicz, R.; Ramelow, S.; Zeilinger, A. The Forgotten Quantum Number: A short note on the radial modes of Laguerre-Gauss beams. arXiv 2013, arXiv:1306.6517. [Google Scholar]
- Petrov, N.I. Speed of structured light pulses in free space. Sci. Rep. 2019, 9, 18332. [Google Scholar] [CrossRef] [PubMed]
- Petrov, N.I. Evanescent and propagating fields of strongly focused beams. JOSA A 2003, 20, 2385–2389. [Google Scholar] [CrossRef] [PubMed]
- Barreiro, J.T.; Wei, T.C.; Kwiat, P.G. Remote Preparation of Single-Photon “Hybrid” Entangled and Vector-Polarization States. Phys. Rev. Lett. 2010, 105, 030407. [Google Scholar] [CrossRef] [PubMed]
- Bliokh, K.Y.; Frolov, D.Y. Spin-orbit interaction of photons and fine splitting of levels in ring dielectric resonator. Opt. Commun. 2005, 250, 321–327. [Google Scholar] [CrossRef][Green Version]
- Erickson, C.W. High order modes in a spherical Fabry-Perot resonator. IEEE Trans. Microw. Theory Tech. 1975, 23, 218–223. [Google Scholar] [CrossRef]
- Yu, P.K.; Luk, K. Field patterns and resonant frequencies of high-order modes in an open resonator. IEEE Trans. Microw. Theory Tech. 1984, 32, 641–645. [Google Scholar]
- Pennington, R.C.; Alessandro, G.D.; Baumberg, J.J.; Kaczmarek, M. Tracking spatial modes in nearly hemispherical microcavities. Opt. Lett. 2007, 32, 3131–3133. [Google Scholar] [CrossRef] [PubMed]
- Foster, D.H.; Nockel, J.U. Bragg-induced orbital angular-momentum mixing in paraxial high-finesse cavities. Opt. Lett. 2004, 29, 2788–2790. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Brosseau, C. Entropy and Polarization of a Stochastic Radiation Field. Prog. Quantum Electron. 1997, 21, 421–461. [Google Scholar] [CrossRef]
- Ellis, J.; Dogariu, A.; Ponomarenko, S.; Wolf, E. Degree of polarization of statistically stationary electromagnetic fields. Opt. Commun. 2005, 248, 333–337. [Google Scholar] [CrossRef]
- Ellis, J.; Dogariu, A. On the degree of polarization of random electromagnetic fields. Opt. Commun. 2005, 253, 257–265. [Google Scholar] [CrossRef]
- Dennis, M.R. A three-dimensional degree of polarization based on Rayleigh scattering. J. Opt. Soc. Am. A 2007, 24, 2065–2069. [Google Scholar] [CrossRef] [PubMed]
- Petruccelli, J.C.; Moore, N.J.; Alonso, M.A. Two methods for modeling the propagation of the coherence and polarization properties of nonparaxial fields. Opt. Commun. 2010, 283, 4457–4466. [Google Scholar] [CrossRef]
- Sheppard, C.J.R. Jones and Stokes parameters for polarization in three dimensions. Phys. Rev. A 2014, 90, 023809. [Google Scholar] [CrossRef]
- Gil, J.J. Intrinsic Stokes parameters for 3D and 2D polarization states. J. Eur. Opt. Soc. Rapid 2015, 10, 15054. [Google Scholar] [CrossRef]
- Gil, J.J.; Norman, A.; Friberg, A.T.; Setala, T. Polarimetric purity and the concept of degree of polarization. Phys. Rev. A 2018, 97, 023838. [Google Scholar] [CrossRef]
- Arfken, G.B.; Weber, H.J. Mathematical Methods for Physicists; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Richardson, D.J.; Fini, J.M.; Nelson, L.E. Space-division multiplexing in optical fibres. Nat. Photon. 2013, 7, 354–362. [Google Scholar] [CrossRef]
- Stuart, H.R. Dispersive multiplexing in multimode optical fiber. Science 2000, 289, 281–283. [Google Scholar] [CrossRef] [PubMed]
- Tzang, O.; Caravaca-Aguirre, A.M.; Wagner, K.; Piestun, R. Adaptive wavefront shaping for controlling nonlinear multimode interactions in optical fibres. Nat. Photon. 2018, 12, 368–374. [Google Scholar] [CrossRef]
- Wright, L.G.; Renninger, W.H.; Christodoulides, D.N.; Wise, F.W. Spatiotemporal dynamics of multimode optical solitons. Opt. Exp. 2015, 23, 3492–3506. [Google Scholar] [CrossRef] [PubMed]
- Wright, L.G.; Christodoulides, D.N.; Wise, F.W. Controllable spatiotemporal nonlinear effects in multimode fibres. Nat. Photon. 2015, 9, 306–310. [Google Scholar] [CrossRef]
- Fu, W.; Wright, L.G.; Sidorenko, P.; Backus, S.; Wise, F.W. Several new directions for ultrafast fiber lasers [Invited]. Opt. Express 2018, 26, 9432–9463. [Google Scholar] [CrossRef] [PubMed]
- Wright, L.G.; Christodoulides, D.N.; Wise, F.W. Spatiotemporal mode-locking in multimode fiber lasers. Science 2017, 358, 94–97. [Google Scholar] [CrossRef] [PubMed]
- Deng, Z.; Chen, Y.; Liu, J.; Zhao, C.; Fan, D. Graded-index breathing solitons from Airy pulses in multimode fibers. Opt. Exp. 2019, 27, 483–493. [Google Scholar] [CrossRef] [PubMed]
- Krupa, K.; Tonello, A.; Barthélémy, A.; Mansuryan, T.; Couderc, V.; Millot, G.; Grelu, P.; Modotto, D.; Babin, S.A.; Wabnitz, S. Multimode nonlinear fiber optics, a spatiotemporal avenue. APL Photon. 2019, 4, 110901. [Google Scholar] [CrossRef]
- Xiong, W.; Hsu, C.W.; Bromberg, Y.; Antonio-Lopez, J.E.; Correa, R.A.; Cao, H. Complete polarization control in multimode fibers with polarization and mode coupling. Light Sci. Appl. 2018, 7, 54. [Google Scholar] [CrossRef] [PubMed]
- Zou, K.; Miao, L.; Wang, G.; Bi, R.; Chen, K.; She, X.; Shu, X. Light depolarization based on dispersion degree of polarization. Appl. Opt. 2020, 59, 11301–11309. [Google Scholar] [CrossRef] [PubMed]
- Ellis, J.; Dogariu, A. Optical Polarimetry of Random Fields. Phys. Rev. Lett. 2005, 95, 203905. [Google Scholar] [CrossRef] [PubMed]
- Leppänen, A.L.P.; Friberg, T.; Setälä, T. Partial polarization of optical beams and near fields probed with a nanoscatterer. J. Opt. Soc. Am. A 2014, 31, 1627–1635. [Google Scholar] [CrossRef] [PubMed]
- Bliokh, K.Y.; Nori, F. Transverse and longitudinal angular momenta of light. Phys. Rep. 2015, 592, 1–38. [Google Scholar] [CrossRef]
- Petersen, J.V.; Rauschenbeutel, A. Chiral nano-photonic waveguide interface based on spin-orbit interaction of light. Science 2014, 346, 67–71. [Google Scholar] [CrossRef] [PubMed]
- Lefier, Y.; Grosjean, T. Unidirectional sub-diffraction waveguiding based on optical spin-orbit coupling in subwavelength plasmonic waveguides. Opt. Lett. 2015, 40, 2890–2893. [Google Scholar] [CrossRef]
- Eismann, J.S.; Nicholls, L.H.; Roth, D.J.; Alonso, M.A.; Banzer, P.; Rodríguez-Fortuño, F.J.; Zayats, A.V.; Nori, F.; Bliokh, K.Y. Transverse spinning of unpolarized light. Nat. Photon. 2020, 15, 156–161. [Google Scholar] [CrossRef]
- Ketara, M.L.; Kobayashi, H.; Brasselet, E. Sensitive vectorial optomechanical footprint of light in soft condensed matter. Nat. Photon. 2021, 15, 121–124. [Google Scholar] [CrossRef]
- Cardano, F.; Marrucci, L. Longitudinal fields and transverse rotations. Nat. Photon. 2021, 15, 72–74. [Google Scholar] [CrossRef]
- Golat, S.; Lim, E.A.; Rodríguez-Fortuño, F.J. Evanescent Gravitational Waves. arXiv 2020, arXiv:1903.09690. [Google Scholar] [CrossRef]
- Lindfors, K.; Setälä, T.; Kaivola, M.; Friberg, A.T. Degree of polarization in tightly focused optical fields. J. Opt. Soc. Am. A 2005, 22, 561–568. [Google Scholar] [CrossRef] [PubMed]
- Lindfors, K.; Priimagi, A.; Setälä, T.; Shevchenko, A.; Friberg, A.T.; Kaivola, M. Local polarization of tightly focused unpolarized light. Nat. Photon. 2007, 1, 228–231. [Google Scholar] [CrossRef]
- Marrucci, L. Spin gives direction. Nat. Photon. 2014, 11, 9–10. [Google Scholar] [CrossRef]
- Wang, J.; Sciarrino, F.; Laing, A.; Thomson, M.G. Integrated photonic quantum technologies. Nat. Photon. 2020, 14, 273–284. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petrov, N.I. Depolarization of Light in Optical Fibers: Effects of Diffraction and Spin-Orbit Interaction. Fibers 2021, 9, 34. https://doi.org/10.3390/fib9060034
Petrov NI. Depolarization of Light in Optical Fibers: Effects of Diffraction and Spin-Orbit Interaction. Fibers. 2021; 9(6):34. https://doi.org/10.3390/fib9060034
Chicago/Turabian StylePetrov, Nikolai I. 2021. "Depolarization of Light in Optical Fibers: Effects of Diffraction and Spin-Orbit Interaction" Fibers 9, no. 6: 34. https://doi.org/10.3390/fib9060034
APA StylePetrov, N. I. (2021). Depolarization of Light in Optical Fibers: Effects of Diffraction and Spin-Orbit Interaction. Fibers, 9(6), 34. https://doi.org/10.3390/fib9060034







