Determination of Optimum Twist Equation for the Long Staple Combed Cotton Ring-Spun Yarn
Abstract
1. Introduction
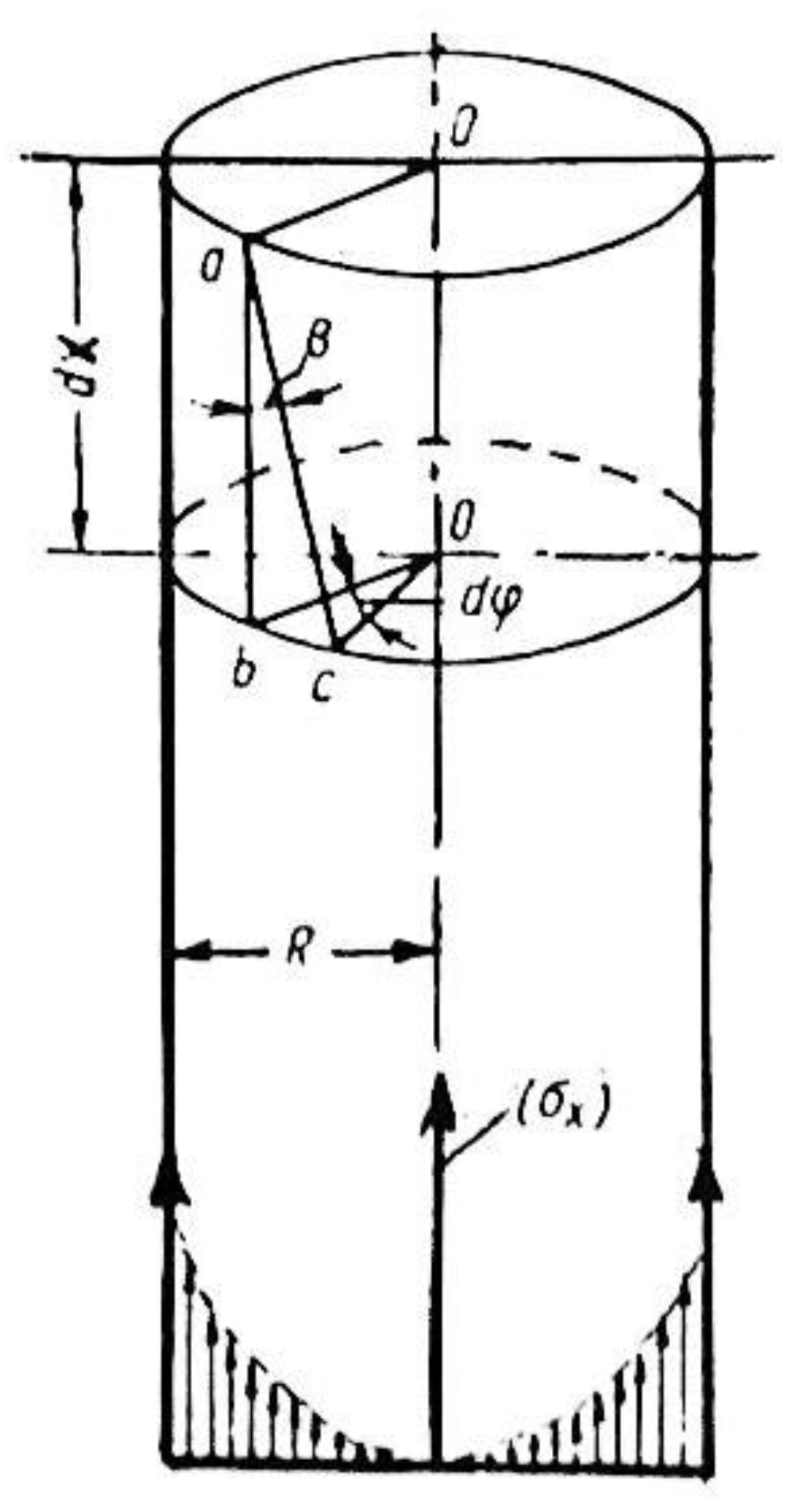
1.1. Theoretical Part
Equations for Calculating Yarn Twist
1.2. Koechlin’s Equation for Calculating Yarn Twist
1.3. Laetsch’s Equation for Calculating the Twist of the Yarn
2. Materials and Methods
2.1. Calculation of the Number of Turns Per Meter in the Yarn
2.2. Production of Yarn on a Ring Spinning Machine
2.3. Method
- Yarn twist and twist direction;
- Tenacity and breaking extension;
- Hairiness, neps and thin and thick places of ring-spun yarn.
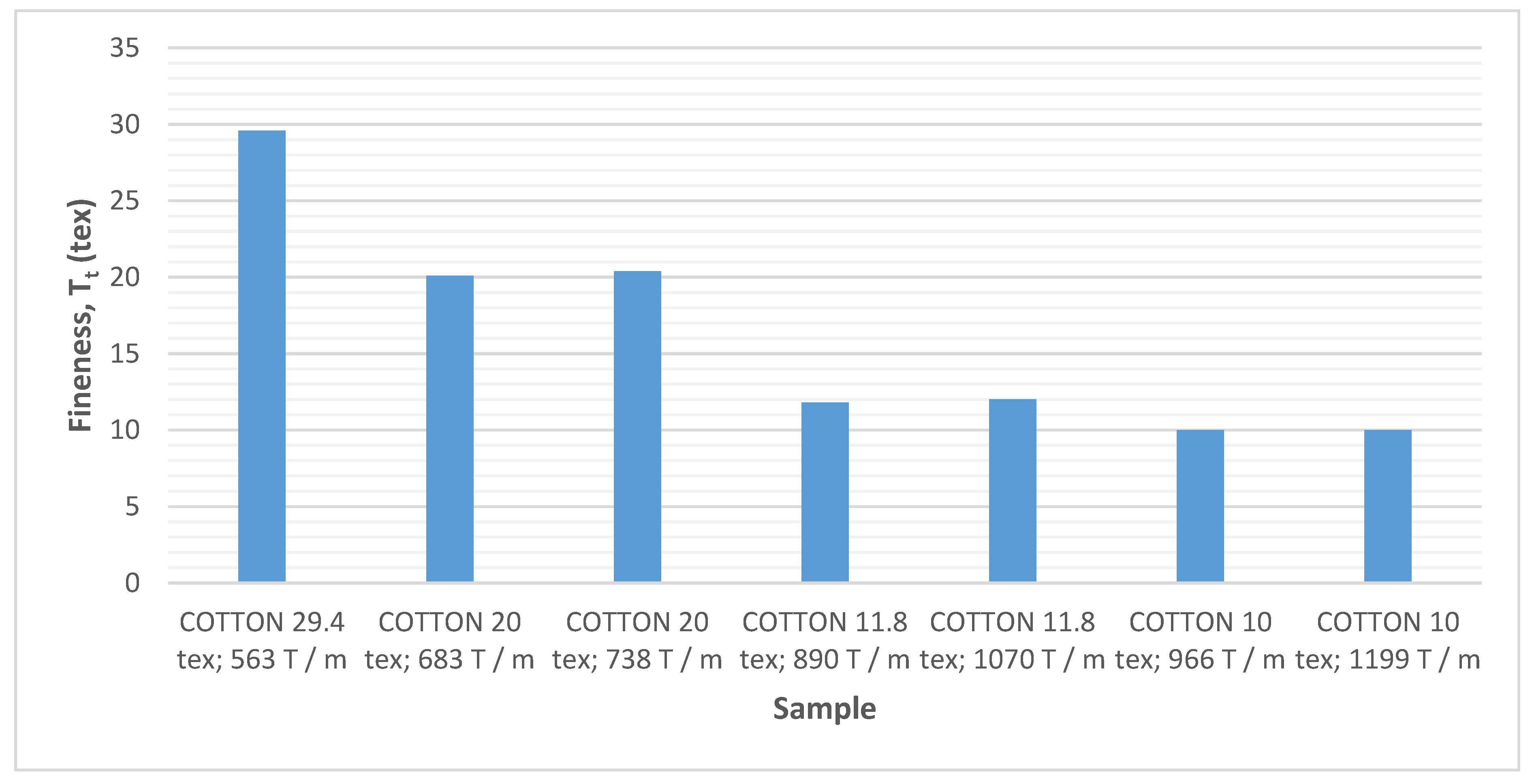
2.4. Fineness of Yarn in Tex and Metric Systems
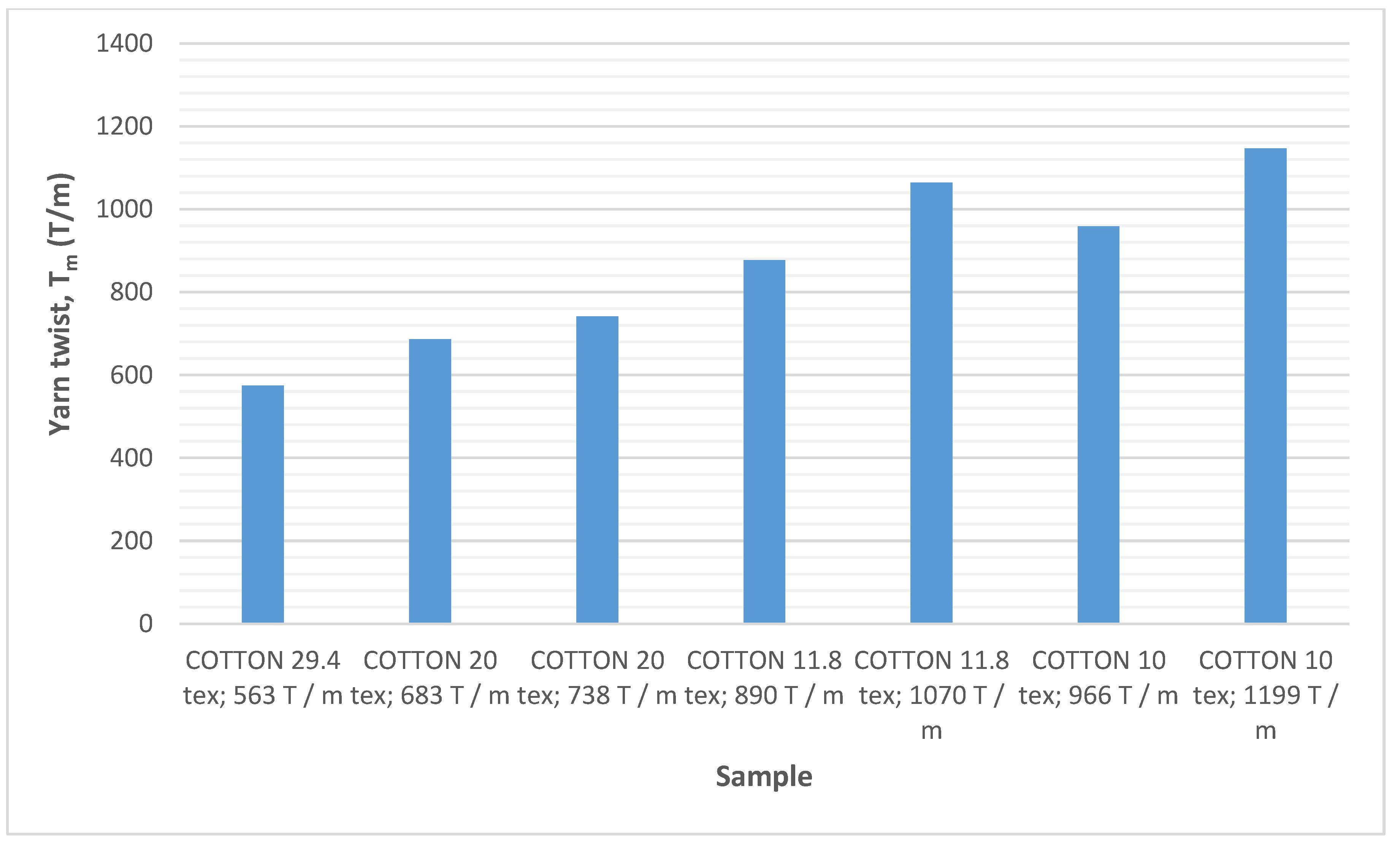
2.5. Number of Turns Per Meter and Direction of Twist
2.6. The Tenacity and the Elongation at Break of the Yarn
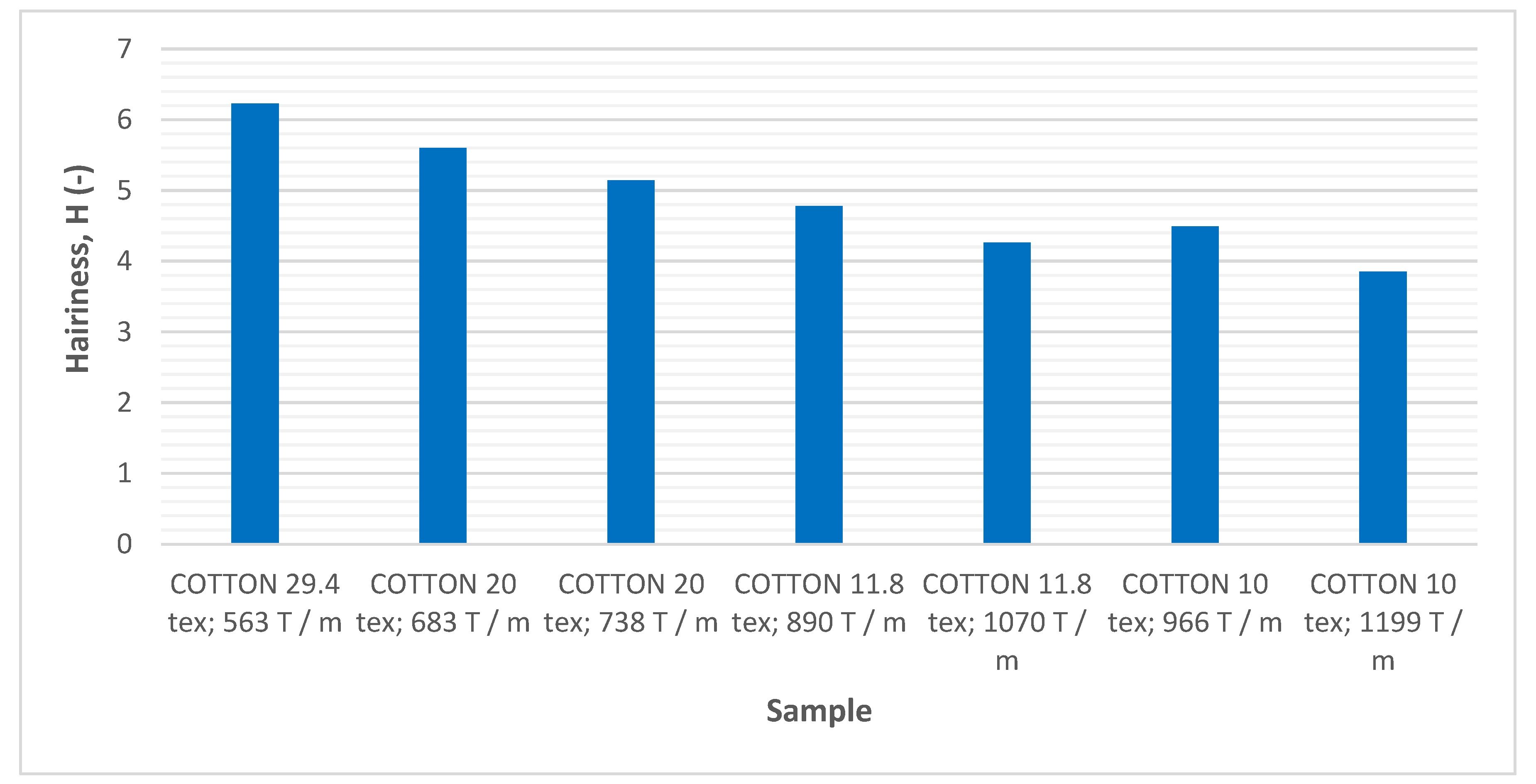
2.7. Hairiness, Neps, Thin and Thick Places of Analyzed Yarn
2.8. Statistical Analysis
3. Discussion of the Results
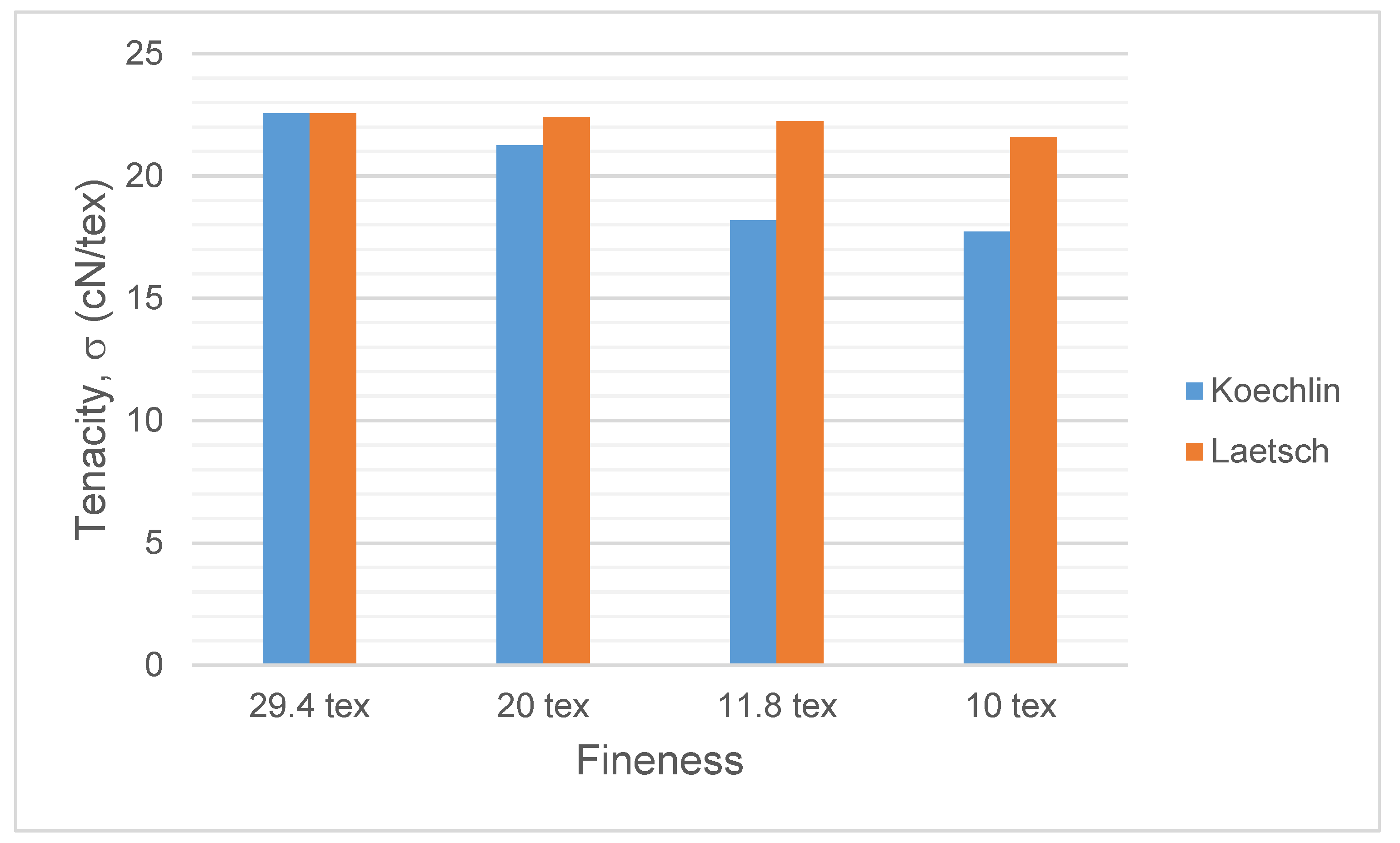
3.1. Tenacity of Analyzed Yarns
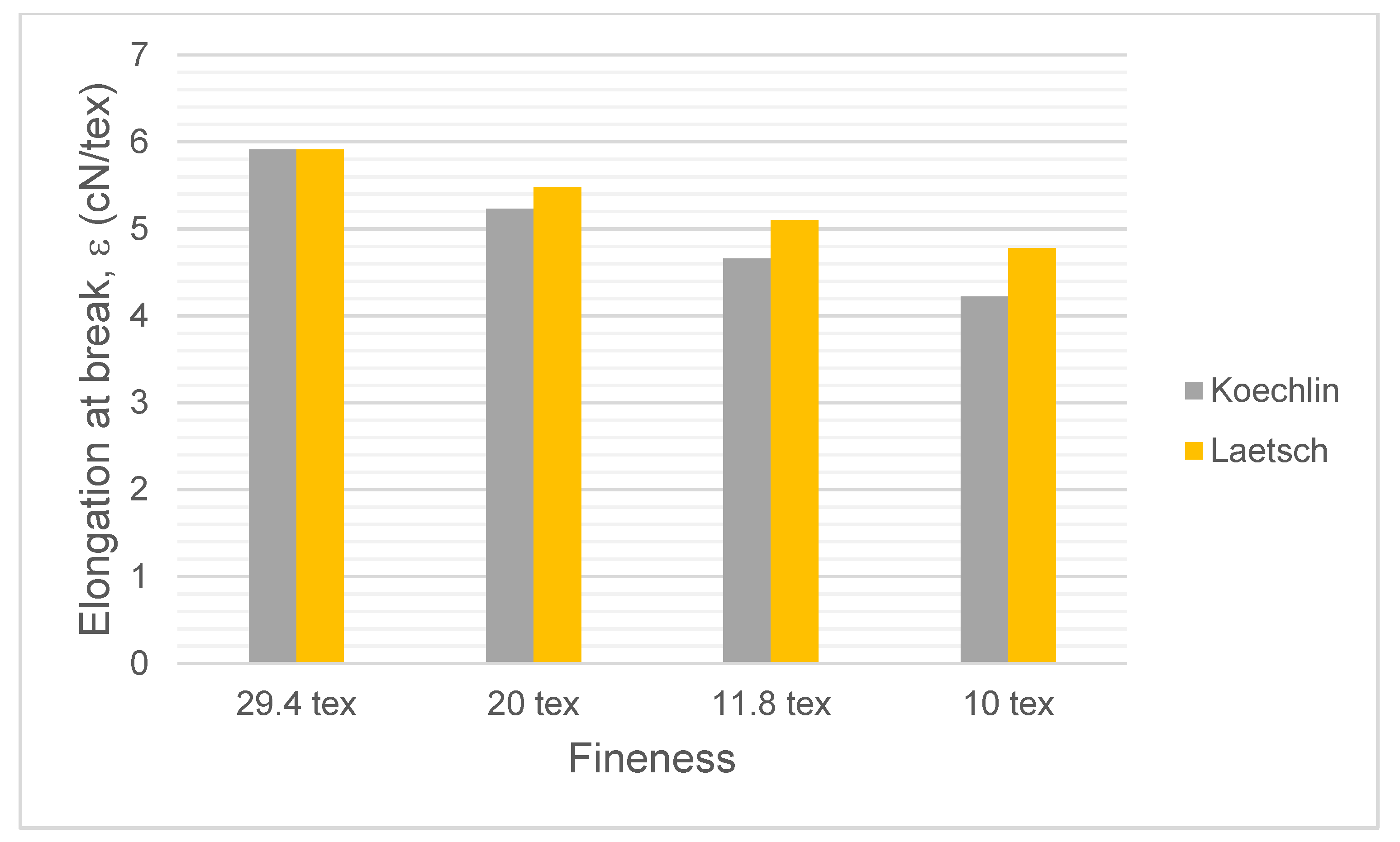
3.2. Elongation at Break of Analyzed Yarns
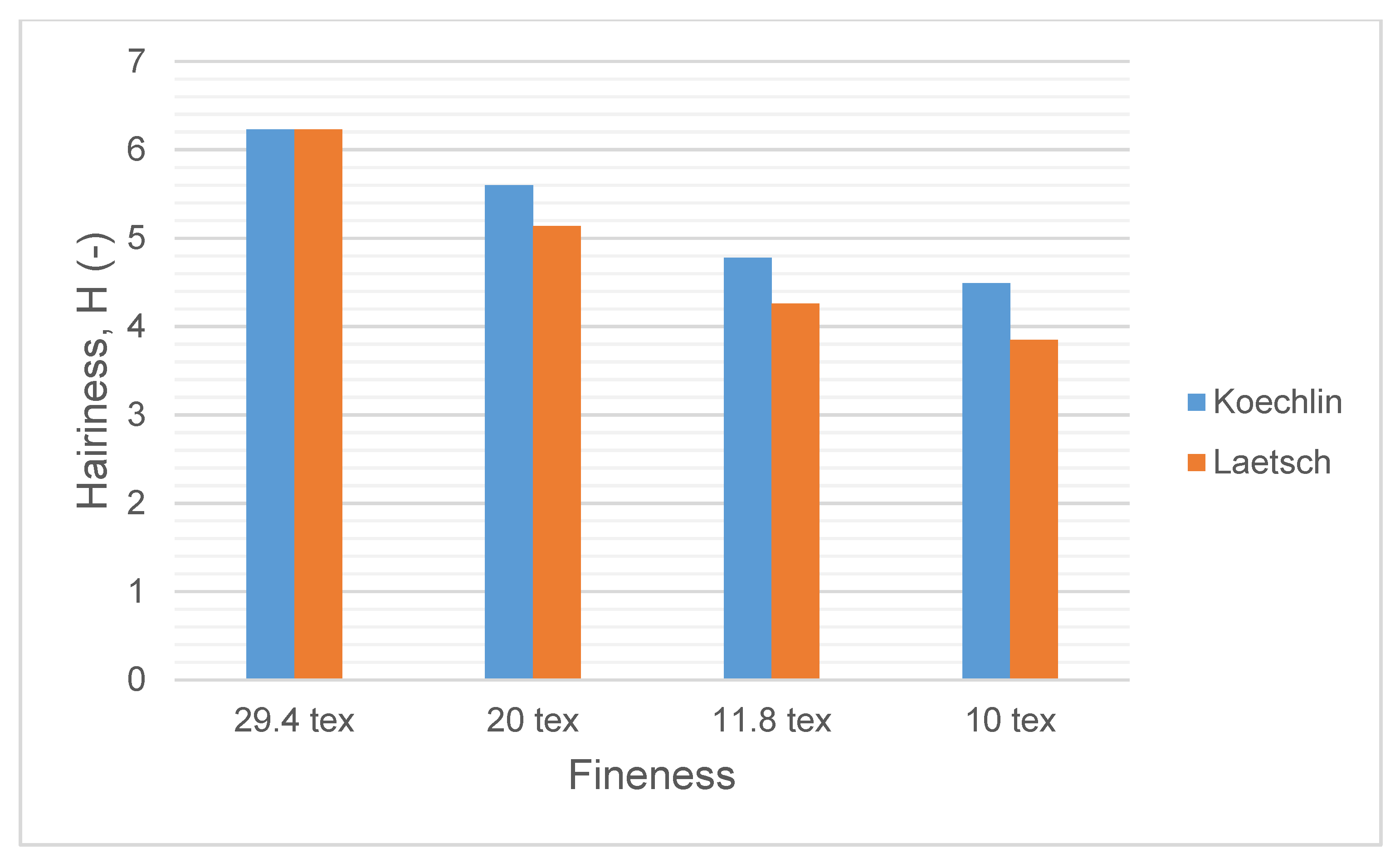
3.3. Yarn Hairiness
3.4. Future Research Perspectives
4. Conclusions
- Ring-spun yarns of combed long staple cotton with a fineness of 10 tex to 20 tex have a higher strength and elongation at break when the twist is calculated according to Laetsch’s equation.
- The decrease in strength and elongation at break as a function of decreasing yarn fineness (from 20 tex to 10 tex) is higher for yarns for which the twist has been calculated using Koechlin’s equation.
- Yarn hairiness showed exactly the opposite in the results for tenacity and elongation, i.e., the hairiness of the analyzed yarns was lower for yarns for which the twist was calculated using the Laetsch equation.
Author Contributions
Funding
Conflicts of Interest
References
- Lawrence, C.A. Advances in Yarn Spinning Technology; Woodhead Publishing: Cambridge, UK, 2010; pp. 42–77. [Google Scholar]
- Neckár, B.; Das, D. Theory of Structure and Mechanics of Yarns; Woodhead Publishing: New Delhi, India, 2018; pp. 24–31. [Google Scholar]
- Gregory, J. Cotton yarn structure; Part 1: Micro yarn structure. J. Text. Inst. 1950, 41, T1–T13. [Google Scholar] [CrossRef]
- Hearle, J.W.S.; Merchant, V.B. Relations between Specific Volume, Count, and Twist of Spun Nylon Yarns. Text. Res. J. 1963, 33, 417–424. [Google Scholar] [CrossRef]
- Hearle, J.W.S.; Grosberg, P.; Backer, S. Structural Mechanics of Fibres, Yarns, and Fabrics; Wiley-Interscience: Hoboken, NJ, USA, 1969; Volume 1, 469p. [Google Scholar]
- Takajima, T. Advanced Fiber Spinning Technology; Woodhead Publishing: Tokio, Japan, 1996; p. 268. [Google Scholar]
- Neckář, B.; Das, D. Computational methodology for determination of yarn twist and diameter. J. Text. Inst. 2019, 110, 1660–1671. [Google Scholar] [CrossRef]
- Mertová, I.; Moučková, E.; Neckář, B.; Vyšanská, M. Influence of twist on selected properties of multifilament yarn. Autex Res. J. 2018, 18, 110–120. [Google Scholar] [CrossRef]
- Lawrence, C.A. Fundamentals of Spun Yarn Technology; CRC Press: London, UK, 2003; p. 32. [Google Scholar]
- Senthil Kumar, R. Process Management in Spinning; CRC Press: London, UK, 2015; pp. 157–216. [Google Scholar]
- Nikolic, M.; Lesjak, F.; Stritof, A. The future of ring spinning = The future of ring spinning. Tekstilec 2000, 43, 188–195. [Google Scholar]
- Saville, B.P. Physical Testing of Textiles; Woodhead Publishing Limited: Manchester, UK, 1999; p. 89. [Google Scholar]
- Klein, W. The Rieter Manual of Spinning-Volume 1: Technology of Short-Staple Spinning; Rieter Holding: Winterthur, Switzerland, 2018; pp. 1–52. [Google Scholar]
- ISO 2060. Textiles–Yarn from Packages—Determination of Linear Density (Mass per unit Length) by the Skein Method; BSI: London, UK, 1996; p. 13. [Google Scholar]
- ISO 2061. Textiles–Determining the Turns per Meter of the Yarn. Direct Counting Method; BSI: London, UK, 2010; p. 12. [Google Scholar]
- ISO 2062. Textiles–Yarns from Windings. Determination of Breaking force and Breaking Elongation on Devices with Constant Expansion Rate (CRE); BSI: London, UK, 2009; p. 3. [Google Scholar]
- Clarke, D.M.; Cooke, D. A Basic Course of Statistics; John Wiley & Sons: London, UK, 2004; pp. 571–593. [Google Scholar]


| The Author of the Equation | Release Year | The Twist Exponent |
|---|---|---|
| Koechlin | 1828 | 0.5 |
| Staub | 1900 | 0.6 |
| Johansen | 1902 | 0.644 |
| Laetch | 1905 1905 1941 | 0.785 warp 0.720 weft 0.62–0.75 |
| Oeser | 1937 1940 | 0.565 0.47 |
| Pluix | 1942 | 0.666 |
| Neckar | 1971 | 0.577 0.6 |
| Salaba | 1975 1975 | 0.518 0.551; 0.570 |
| Type of Yarn | Short Fibers | Medium Fiber | Long Fibers |
|---|---|---|---|
| Knitting | - | 2.5–3.0 | 2.1–2.6 |
| Weft | 3.3–3.8 | 3.0–3.5 | 2.5–3.0 |
| Half-base | 3.7–4.0 | 3.5–3.8 | 3.0–3.4 |
| Warp | 4.0–5.0 | 3.8–4.5 | 3.4–3.9 |
| Tt [tex] | Coefficient of Twist αe | Coefficient of Twist αm | Yarn Twist Koechlin’s Equation (T/m) | Yarn Twist Laetsch’s Equation (T/m) |
|---|---|---|---|---|
| 29.4 | 1.75 | 96.55 | 563 | 563 |
| 20 | 683 | 738 | ||
| 11.8 | 890 | 1070 | ||
| 10 | 966 | 1199 |
| Properties Specified | Set Properties on the Ring Spinner | |||||||
|---|---|---|---|---|---|---|---|---|
| Material | Roving [ktex] | Yarn [tex] | Twist [T/m] | Drawing Length [mm] | Rotational Speed [rpm] | Working Speed [m/min] | Spacer Inserts | Travellers |
| 0.58 | 29.4 | 563 | 19.9–20.6 | 9611 | 17 | 4.0 BLACK | C1 EN No.1 | |
| 0.58 | 20 | 683 | 29.0–29.8 | 10,998 | 16.3 | 3.1 LIGHT BROWN | C1 HR No. 3/0 | |
| Cotton-Long staple | 0.58 | 20 | 738 | 29.1–30.0 | 10,982 | 15 | 3.1 LIGHT BROWN | C1 HR No. 3/0 |
| 0.58 | 11.8 | 890 | 48.4–49.5 | 12,051 | 13.6 | 2.4 ROSES | C1 HR No. 8/0 | |
| 0.58 | 11.8 | 1070 | 48.4–49.3 | 12,000 | 11.2 | 2.4 ROSES | C1 HR No. 8/0 | |
| 0.58 | 10 | 966 | 57.6–59.9 | 12,017 | 12.5 | 2.2 YELLOW | C1 HR No. 10/0 | |
| 0.58 | 10 | 1199 | 57.8–59.4 | 11,817 | 9.8 | 2.2 YELLOW | C1 HR No. 10/0 | |
| Sample | Cotton 563 T/m | Cotton 683 T/m | Cotton 738 T/m | Cotton 890 T/m | Cotton 1070 T/m | Cotton 966 T/m | Cotton 1199 T/m |
|---|---|---|---|---|---|---|---|
| Fineness | Tt [tex] | ||||||
| 1 | 29.9 | 20.2 | 20.2 | 11.8 | 12.0 | 9.9 | 10.1 |
| 2 | 29.6 | 20.3 | 20.4 | 11.9 | 12.0 | 9.9 | 9.9 |
| 3 | 29.4 | 19.9 | 20.6 | 12.0 | 12.0 | 10.1 | 10.0 |
| 4 | 29.5 | 20.3 | 20.4 | 11.8 | 11.9 | 10.0 | 10.2 |
| 5 | 29.7 | 19.8 | 20.4 | 11.7 | 11.9 | 10.0 | 10.0 |
| Average | 29.6 | 20.1 | 20.4 | 11.8 | 12.0 | 10.0 | 10.0 |
| CV [%] | 0.16 | 0.27 | 0.09 | 0.09 | 0.05 | 0.08 | 0.17 |
| Sx | 0.22 | 0.23 | 0.13 | 0.101 | 0.079 | 0.088 | 0.13 1 |
| Sample | CO 29.4 tex; 563 T/m | |||||
|---|---|---|---|---|---|---|
| No. | Average | Sx | CV [%] | |||
| Cop 1 | 570 | 17.15 | 3.01 | |||
| Cop 2 | 581 | 11.95 | 2.06 | |||
| Cop 3 | 584 | 12.19 | 2.09 | |||
| Cop 4 | 580 | 15.26 | 2.63 | |||
| Cop 5 | 556 | 10.78 | 1.94 | |||
| Average | 574 | |||||
| Sample | CO 20 tex; 683 T/m | CO 20 tex; 738 T/m | ||||
| No. | Average | Sx | CV [%] | Average | Sx | CV [%] |
| Cop 1 | 678 | 7.4 | 1.09 | 734 | 14.741 | 2.01 |
| Cop 2 | 678 | 21.42 | 3.16 | 743 | 32.668 | 4.4 |
| Cop 3 | 701 | 15.02 | 2.14 | 725 | 12.988 | 1,79 |
| Cop 4 | 686 | 8.88 | 1.29 | 748 | 21.824 | 2.92 |
| Cop 5 | 690 | 13.58 | 1.97 | 755 | 16.416 | 2.17 |
| Average | 686 | 741 | ||||
| Sample | CO 11.8 tex; 890 T/m | CO 11.8 tex; 1070 T/m | ||||
| No. | Average | Sx | CV [%] | Average | Sx | CV [%] |
| Cop 1 | 882 | 21.47 | 2.43 | 1062 | 14.17 | 1.33 |
| Cop 2 | 894 | 18.93 | 2.12 | 1087 | 26.79 | 2.47 |
| Cop 3 | 886 | 30.18 | 3.41 | 1061 | 25.48 | 2.4 |
| Cop 4 | 874 | 25.95 | 2.97 | 1074 | 25.96 | 2.42 |
| Cop 5 | 851 | 22.76 | 2.67 | 1039 | 24.17 | 2.33 |
| Average | 877 | 1064 | ||||
| Sample | CO 10 tex; 966 T/m | CO 10 tex; 1199 T/m | ||||
| No. | Average | Sx | CV [%] | Average | Sx | CV [%] |
| Cop 1 | 944 | 25.7 | 2.72 | 1164 | 29.55 | 2.54 |
| Cop 2 | 965 | 38.24 | 3.96 | 1130 | 21.11 | 1.87 |
| Cop 3 | 959 | 23.12 | 2.41 | 1144 | 24.29 | 2.12 |
| Cop 4 | 973 | 26.16 | 2.69 | 1157 | 39.63 | 3.42 |
| Cop 5 | 954 | 12.09 | 1.27 | 1139 | 24.23 | 2.13 |
| Average | 959 | 1147 | ||||
| Sample | CO; 29.4 tex | CO; 20 tex | ||||||
|---|---|---|---|---|---|---|---|---|
| Turns Per Meter | 563 T/m | 683 T/m | 738 T/m | |||||
| Measurement | Tenacity [cN/tex] | Breaking Extension εpr [%] | Tenacity σpr [cN/tex] | Breaking Extension εpr [%] | Tenacity σpr [cN/tex] | Breaking Extension εpr [%] | ||
| 1 | 23.14 | 5.83 | 20.74 | 4.75 | 23.25 | 5.27 | ||
| 2 | 22.14 | 5.68 | 20.8 | 5.41 | 22.06 | 5.56 | ||
| 3 | 21.93 | 6.02 | 22.11 | 5.21 | 21.91 | 5.37 | ||
| 4 | 24.04 | 6.08 | 21.44 | 5.42 | 22.29 | 5.64 | ||
| 5 | 21.56 | 5.96 | 21.18 | 5.38 | 22.56 | 5.54 | ||
| Average | 22.56 | 5.91 | 21.25 | 5.23 | 22.41 | 5.48 | ||
| CV [%] | 4.49 | 2.71 | 2.62 | 5.42 | 2.36 | 2.77 | ||
| Sx | 1.01 | 0.16 | 0.557 | 0.284 | 0.528 | 0.151 | ||
| Sample | CO; 11.8 tex | CO; 10 tex | ||||||
| Turns per Meter | 890 T/m | 1070 T/m | 966 T/m | 1199 T/m | ||||
| Measurement | Tenacity σpr [cN/tex] | Breaking Extension εpr [%] | Tenacity σpr [cN/tex] | Breaking Extension εpr [%] | Tenacity σpr [cN/tex] | Breaking Extension εpr [%] | Tenacity σpr [cN/tex] | Elongation at Break εpr [%] |
| 1 | 18.7 | 4.70 | 23.55 | 5.51 | 18.42 | 4.44 | 22.23 | 5.00 |
| 2 | 19.51 | 4.77 | 22.28 | 5.08 | 19.15 | 4.45 | 22.40 | 4.68 |
| 3 | 19.47 | 4.77 | 22.38 | 5.12 | 17.38 | 4.28 | 20.32 | 4.66 |
| 4 | 17.54 | 4.50 | 20.72 | 4.80 | 15.45 | 4.07 | 21.72 | 4.72 |
| 5 | 19.19 | 4.55 | 22.21 | 5.00 | 18.22 | 3.88 | 21.22 | 4.83 |
| Average | 18.88 | 4.66 | 22.23 | 5.10 | 17.72 | 4.22 | 21.58 | 4.78 |
| CV [%] | 4.33 | 2.70 | 4,53 | 5.08 | 8.01 | 5.83 | 3.90 | 2.94 |
| Sx | 0.82 | 0.13 | 1.01 | 0.26 | 1.42 | 0.25 | 0.84 | 0.14 |
| Sample | CO 29.4 tex; 563 T/m | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| No. | CV% | Thin Places | Thick Places | Neps | Hairiness | |||||||||
| 1 | 8.92 | 0 | 0 | 0 | 6.33 | |||||||||
| 2 | 9.03 | 0 | 5 | 5 | 6.28 | |||||||||
| 3 | 8.96 | 0 | 5 | 0 | 6.28 | |||||||||
| 4 | 9.46 | 0 | 5 | 5 | 6.03 | |||||||||
| 5 | 8.86 | 0 | 0 | 0 | 6.22 | |||||||||
| Average | 9.05 | 0 | 3 | 2 | 6.23 | |||||||||
| Sample | CO 20 tex; 683 T/m | CO 20 tex; 738 T/m | ||||||||||||
| No. | CV% | Thin Places | Thick Places | Neps | Hairiness | CV% | Thin Places | Thick Places | Neps | Hairiness | ||||
| 1 | 10.66 | 0 | 10 | 35 | 5.68 | 10.32 | 0 | 5 | 5 | 5.1 | ||||
| 2 | 10.81 | 0 | 10 | 10 | 5.7 | 10.51 | 0 | 0 | 10 | 5.11 | ||||
| 3 | 10.41 | 0 | 0 | 0 | 5.59 | 10.44 | 0 | 0 | 5 | 5.19 | ||||
| 4 | 10.76 | 0 | 10 | 0 | 5.45 | 10.80 | 0 | 0 | 15 | 5.1 | ||||
| 5 | 10.71 | 0 | 5 | 15 | 5.59 | 10.49 | 0 | 5 | 5 | 5.19 | ||||
| Average | 10.67 | 0 | 7 | 12 | 5.60 | 10.51 | 0 | 2 | 8 | 5.14 | ||||
| Sample | CO 11.8 tex; 890 T/m | CO 11.8 tex; 1070 T/m | ||||||||||||
| No. | CV% | Thin Places | Thick Places | Neps | Hairiness | CV% | Thin Places | Thick Places | Neps | Hairiness | ||||
| 1 | 12.83 | 0 | 20 | 45 | 4.78 | 12.71 | 5 | 35 | 30 | 4.23 | ||||
| 2 | 12.66 | 0 | 10 | 35 | 4.58 | 12.54 | 5 | 15 | 30 | 4.07 | ||||
| 3 | 13.05 | 0 | 35 | 45 | 4.84 | 12.69 | 0 | 25 | 85 | 4.33 | ||||
| 4 | 12.74 | 0 | 45 | 70 | 4.94 | 12.81 | 5 | 25 | 40 | 4.33 | ||||
| 5 | 13.27 | 0 | 15 | 35 | 4.74 | 12.61 | 0 | 25 | 45 | 4.36 | ||||
| Average | 12.91 | 0 | 25 | 46 | 4.78 | 12.67 | 3 | 25 | 46 | 4.26 | ||||
| Sample | CO 10 tex; 966 T/m | CO 10 tex; 1199 T/m | ||||||||||||
| No. | CV% | Thin Places | Thick Places | Neps | Hairiness | CV% | Thin Places | Thick Places | Neps | Hairiness | ||||
| 1 | 13.51 | 10 | 35 | 90 | 4.37 | 13.27 | 5 | 35 | 80 | 3.90 | ||||
| 2 | 13.72 | 15 | 55 | 60 | 4.48 | 13.03 | 0 | 5 | 70 | 3.81 | ||||
| 3 | 13.57 | 10 | 20 | 45 | 4.43 | 13.61 | 10 | 40 | 60 | 3.79 | ||||
| 4 | 13.80 | 0 | 40 | 75 | 4.49 | 13.24 | 5 | 30 | 45 | 3.82 | ||||
| 5 | 13.48 | 5 | 15 | 65 | 4.71 | 13.38 | 0 | 25 | 40 | 3.94 | ||||
| Average | 13.60 | 8 | 33 | 67 | 4.49 | 13.31 | 4 | 27 | 59 | 3.85 | ||||
| Sample CO; 20 tex | ||||||
|---|---|---|---|---|---|---|
| Source of Variation | SS | df | MS | F | p-Value | F crit |
| Between Groups | 3.36 | 1 | 3.36 | 11.41 | 0.0097 | 5.32 |
| Within Groups | 2.36 | 8 | 0.29 | |||
| Total | 5.72 | 9 | ||||
| Sample CO; 11.8 tex | ||||||
| Source of Variation | SS | df | MS | F | p-Value | F crit |
| Between Groups | 27.99 | 1 | 27.99 | 33.34 | 0.00042 | 5.32 |
| Within Groups | 6.72 | 8 | 0.84 | |||
| Total | 34.71 | 9 | ||||
| Sample CO; 10 tex | ||||||
| Source of Variation | SS | df | MS | F | p-Value | F crit |
| Between Groups | 37.13 | 1 | 37.13 | 27.29 | 0.00079 | 5.32 |
| Within Groups | 10.89 | 8 | 1.36 | |||
| Total | 48.02 | 9 |
| Sample CO; 20 tex | ||||||
|---|---|---|---|---|---|---|
| Source of Variation | SS | df | MS | F | p-Value | F crit |
| Between Groups | 0.54 | 1 | 0.54 | 89.41 | 1.29E-05 | 5.32 |
| Within Groups | 0.05 | 8 | 0.0060 | |||
| Total | 0.59 | 9 | ||||
| Sample CO; 11.8 tex | ||||||
| Source of Variation | SS | df | MS | F | p-Value | F crit |
| Between Groups | 0.66 | 2 | 0.33 | 17.99 | 0.0017 | 4.74 |
| Within Groups | 0.13 | 7 | 0.02 | |||
| Total | 0.78 | 9 | ||||
| Sample CO; 10 tex | ||||||
| Source of Variation | SS | df | MS | F | p-Value | F crit |
| Between Groups | 1.04 | 2 | 0.52 | 43.72 | 0.00011 | 4.74 |
| Within Groups | 0.08 | 7 | 0.012 | |||
| Total | 1.12 | 9 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gorjanc, D.Š.; Sukič, N. Determination of Optimum Twist Equation for the Long Staple Combed Cotton Ring-Spun Yarn. Fibers 2020, 8, 59. https://doi.org/10.3390/fib8090059
Gorjanc DŠ, Sukič N. Determination of Optimum Twist Equation for the Long Staple Combed Cotton Ring-Spun Yarn. Fibers. 2020; 8(9):59. https://doi.org/10.3390/fib8090059
Chicago/Turabian StyleGorjanc, Dunja Šajn, and Neža Sukič. 2020. "Determination of Optimum Twist Equation for the Long Staple Combed Cotton Ring-Spun Yarn" Fibers 8, no. 9: 59. https://doi.org/10.3390/fib8090059
APA StyleGorjanc, D. Š., & Sukič, N. (2020). Determination of Optimum Twist Equation for the Long Staple Combed Cotton Ring-Spun Yarn. Fibers, 8(9), 59. https://doi.org/10.3390/fib8090059





