Large Normal Dispersion Mode-Locked Erbium-Doped Fiber Laser
Abstract
1. Introduction
2. Materials and Methods
2.1. Fiber Structure and Dispersion Properties
2.2. Laser Experimental Setup
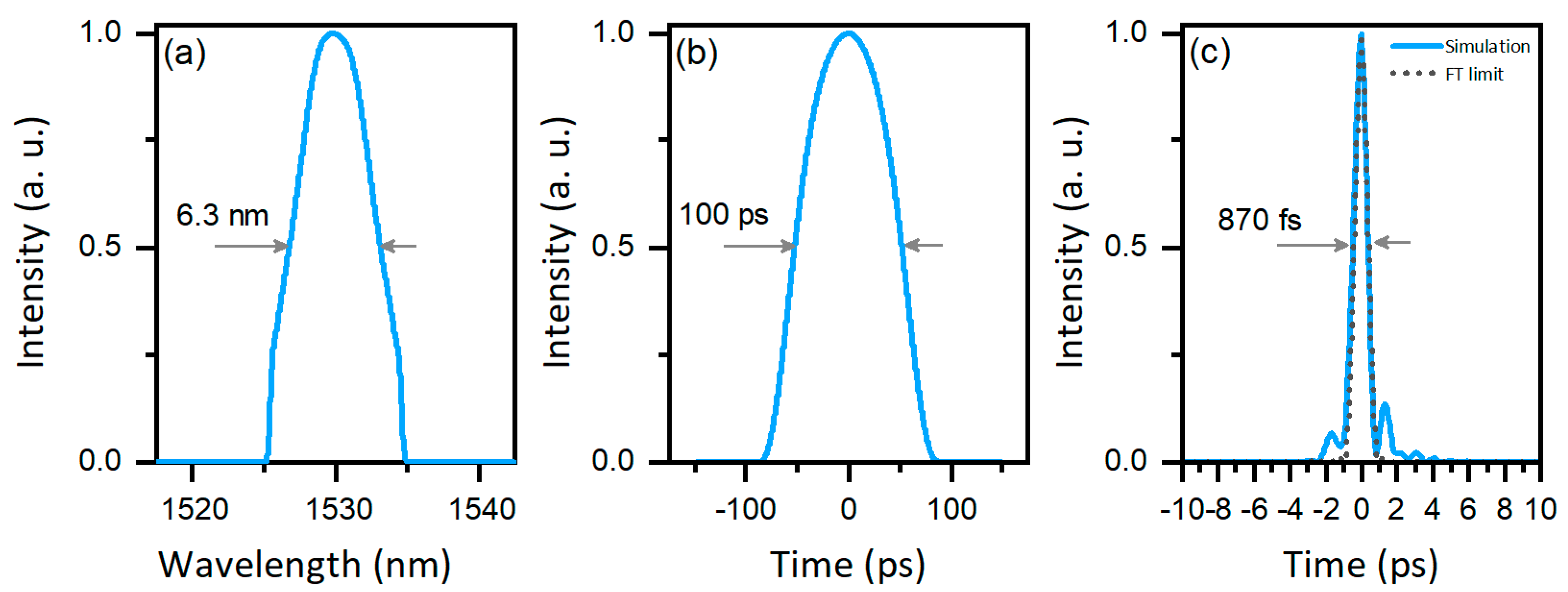
3. Experimental and Numerical Results
4. Discussion
Author Contributions
Funding
Conflicts of Interest
References
- Ilday, F.Ö.; Buckley, J.R.; Clark, W.G.; Wise, F.W. Self-Similar Evolution of Parabolic Pulses in a Laser. Phys. Rev. Lett. 2004, 92, 213902. [Google Scholar] [CrossRef] [PubMed]
- Chong, A.; Renninger, W.H.; Wise, F.W. All-Normal-Dispersion femtosecond fiber laser with pulse energy above 20 nJ. Opt. Lett. 2007, 32, 2408. [Google Scholar] [CrossRef] [PubMed]
- Nie, B.; Pestov, D.; Wise, F.W.; Dantus, M. Generation of 42-fs and 10-nJ pulses from a fiber laser with self-similar evolution in the gain segment. Opt. Express 2011, 19, 12074. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Ziegler, Z.M.; Wright, L.G.; Wise, F.W. Megawatt peak power from a Mamyshev oscillator. Optica 2017, 4, 649–654. [Google Scholar] [CrossRef] [PubMed]
- Lefrançois, S.; Kieu, K.; Deng, Y.; Kafka, J.D.; Wise, F.W. Scaling of dissipative soliton fiber lasers to megawatt peak powers by use of large-area photonic crystal fiber. Opt. Lett. 2010, 35, 1569–1571. [Google Scholar] [CrossRef]
- Lecaplain, C.; Baumgartl, M.; Schreiber, T.; Hideur, A. On the mode-locking mechanism of a dissipative- soliton fiber oscillator. Opt. Express 2011, 19, 26742–26751. [Google Scholar] [CrossRef]
- Baumgartl, M.; Lecaplain, C.; Hideur, A.; Limpert, J.; Tünnermann, A. 66 W average power from a microjoule-class sub-100 fs fiber oscillator. Opt. Lett. 2012, 37, 1640–1642. [Google Scholar] [CrossRef]
- Liu, W.; Liao, R.; Zhao, J.; Cui, J.; Song, Y.; Wang, C.; Hu, M. Femtosecond Mamyshev oscillator with 10-MW-level peak power. Optica 2019, 6, 194–197. [Google Scholar] [CrossRef]
- Cabasse, A.; Ortaç, B.; Martel, G.; Hideur, A.; Limpert, J. Dissipative solitons in a passively mode-locked Er-doped fiber with strong normal dispersion. Opt. Express 2008, 16, 19322. [Google Scholar] [CrossRef]
- Chichkov, N.B.; Hausmann, K.; Wandt, D.; Morgner, U.; Neumann, J.; Kracht, D. High-Power dissipative solitons from an all-normal dispersion erbium fiber oscillator. Opt. Lett. 2010, 35, 2807. [Google Scholar] [CrossRef]
- Liu, H.; Liu, Z.; Lamb, E.S.; Wise, F. Self-Similar erbium-doped fiber laser with large normal dispersion. Opt. Lett. 2014, 39, 1019. [Google Scholar] [CrossRef] [PubMed]
- Tang, M.; Wang, H.; Becheker, A.; Gaponov, D.; Oudar, J.-L.; Godin, T.; Hideur, A. High-Energy dissipative solitons generation from a large normal dispersion Er-fiber laser. Opt. Lett. 2015, 40, 1414. [Google Scholar] [CrossRef] [PubMed]
- Kharenko, D.S.; Zhdanov, I.S.; Bednyakova, A.E.; Podivilov, E.V.; Fedoruk, M.P.; Apolonski, A.; Turitsyn, S.K.; Babin, S.A. All-Fiber highly chirped dissipative soliton generation in the telecom range. Opt. Lett. 2017, 42, 3221–3224. [Google Scholar] [CrossRef] [PubMed]
- Olivier, M.; Boulanger, V.; Guilbert-Savary, F.; Sidorenko, P.; Wise, F.W.; Piché, M. Femtosecond fiber Mamyshev oscillator at 1550 nm. Opt. Lett. 2019, 44, 851–854. [Google Scholar] [CrossRef]
- Kruglov, V.I.; Peacock, A.C.; Harvey, J.D.; Dudley, J.M. Self-Similar propagation of parabolic pulses in normal-dispersion fiber amplifiers. J. Opt. Soc. Am. B 2002, 19, 461–469. [Google Scholar] [CrossRef]
- Deng, Y.; Chien, C.-Y.; Fidric, B.G.; Kafka, J.D. Generation of sub-50 fs pulses from a high-power Yb-doped fiber amplifier. Opt. Lett. 2009, 34, 3469–3471. [Google Scholar] [CrossRef]
- Nishida, S.K.S. Characteristics of a double clad optical fiber with a low-index inner cladding. IEEE J. Quantum Electron. 1974, 10, 879–887. [Google Scholar]
- Monerie, M. Propagation in doubly clad single-mode fibers. IEEE J. Quantum Electron. 1982, 18, 535–542. [Google Scholar] [CrossRef]
- Soh, D.; Yoo, S.; Nilsson, J.; Sahu, J.; Oh, K.; Baek, S.; Jeong, Y.; Codemard, C.; Dupriez, P.; Kim, J.; et al. Neodymiumdoped cladding-pumped aluminosilicate fiber laser tunable in the 0.9-µm wavelength range. IEEE J. Quantum Electron. 2004, 40, 1275–1282. [Google Scholar] [CrossRef]
- Yang, L.-G.; Jyu, S.-S.; Chow, C.-W.; Yeh, C.-H.; Lai, Y. S-Band pulse generation by polarization additive-pulse mode-locking in an erbium-doped all-fiber ring laser. Las. Phys. Lett. 2013, 11, 015105. [Google Scholar] [CrossRef]
- Grüner-Nielsen, L.; Knudsen, N.; Edvold, B.; Veng, T.; Magnussen, D.; Larsen, C.; Damsgaard, H. Dispersion Compensating Fibers. Opt. Fiber Technol. 2000, 6, 164–180. [Google Scholar] [CrossRef]
- Ramachandran, S.; Ghalmi, S.; Nicholson, J.W.; Yan, M.F.; Wisk, P.; Monberg, E.; Dimarcello, F.V. Anomalous dispersion in a solid, silica-based fiber. Opt. Lett. 2006, 31, 2532–2534. [Google Scholar] [CrossRef] [PubMed]
- Auguste, J.-L.; Jindal, R.; Blondy, J.-M.; Clapeau, M.; Marcou, J.; Dussardier, B.; Monnom, G.; Ostrowsky, D.; Pal, B.; Thyagarajan, K. −1800 ps nm km chromatic dispersion at 1 55 µm in a dual concentric core fibre. Electron. Lett. 2000, 36, 1689. [Google Scholar] [CrossRef]
- Gérôme, F.; Auguste, J.L.; Février, S.; Maury, J.; Blondy, J.M.; Gasca, L.; Provost, L. Dual concentric core dispersion compensating fibre optimized for WDM application. Electron. Lett. 2005, 41, 116. [Google Scholar] [CrossRef]
- Gérôme, F.; Auguste, J.-L.; Maury, J.; Blondy, J.-M.; Marcou, J. Theoretical and Experimental Analysis of a Chromatic Dispersion Compensating Module Using a Dual Concentric Core Fiber. J. Lightwave Technol. 2006, 24, 442. [Google Scholar] [CrossRef]
- Hamel, P.; Jaouën, Y.; Gabet, R.; Ramachandran, S. Optical low-coherence reflectometry for complete chromatic dispersion characterization of few-mode fibers. Opt. Lett. 2007, 32, 1029. [Google Scholar] [CrossRef]
- Cabasse, A.; Gaponov, D.; Ndao, K.; Khadour, A.; Oudar, J.-L.; Martel, G. 130 mW average power, 4.6 nJ pulse energy, 10.2 ps pulse duration from an Er3+ fiber oscillator passively mode locked by a resonant saturable absorber mirror. Opt. Lett. 2011, 36, 2620–2622. [Google Scholar] [CrossRef]
- Ankiewicz, N.N.A.A.; Luther-Davies, M.J.L.B. Ultrashort pulses generated by mode-locked lasers with either a slow or a fast saturable-absorber response. Opt. Lett. 1998, 23, 280. [Google Scholar]
- Schreiber, T.; Ortaç, B.; Limpert, J.; Tünnermann, A. On the study of pulse evolution in ultra-short pulse mode-locked fiber lasers by numerical simulations. Opt. Express 2007, 15, 8252–8262. [Google Scholar] [CrossRef]
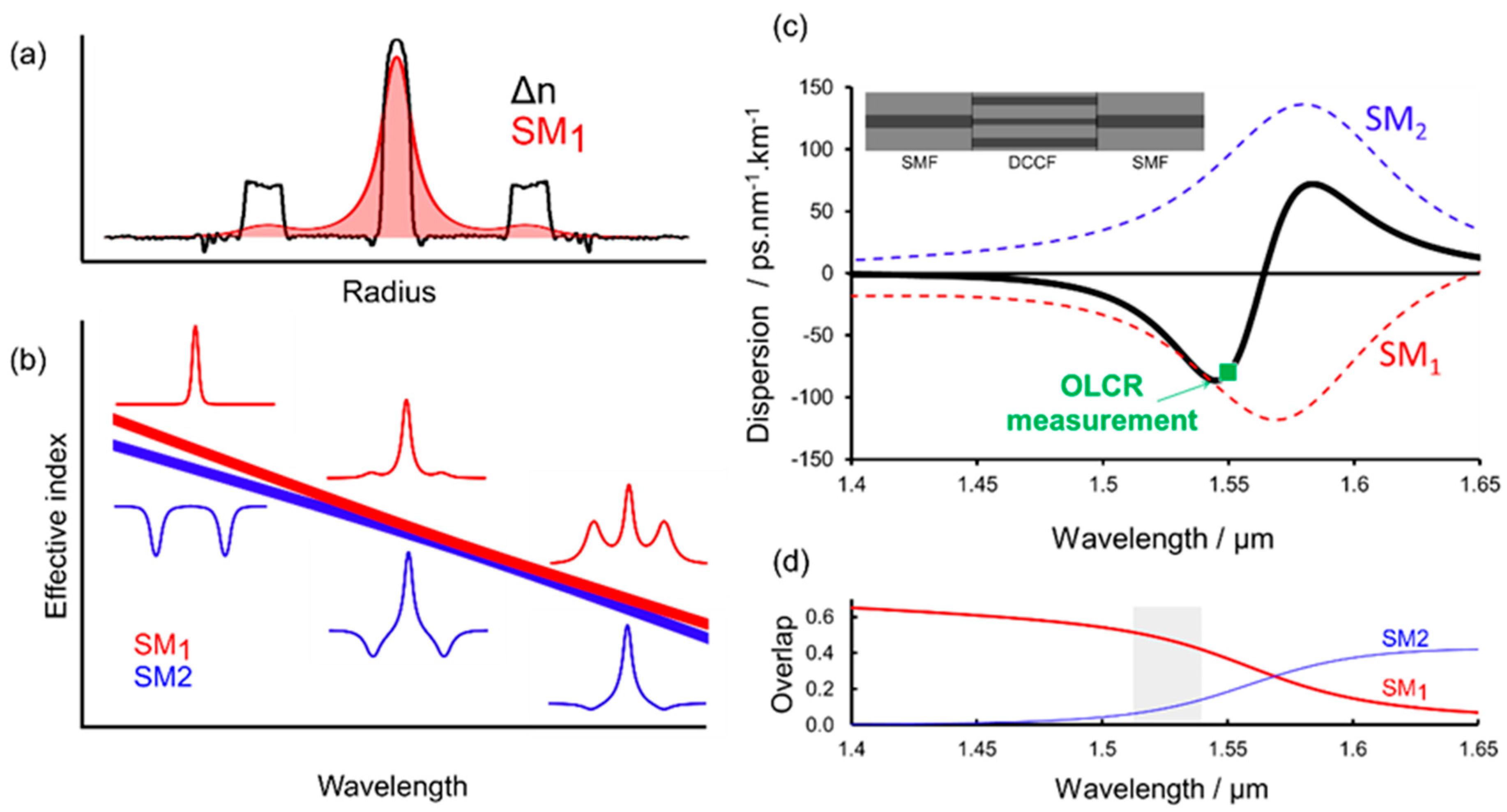
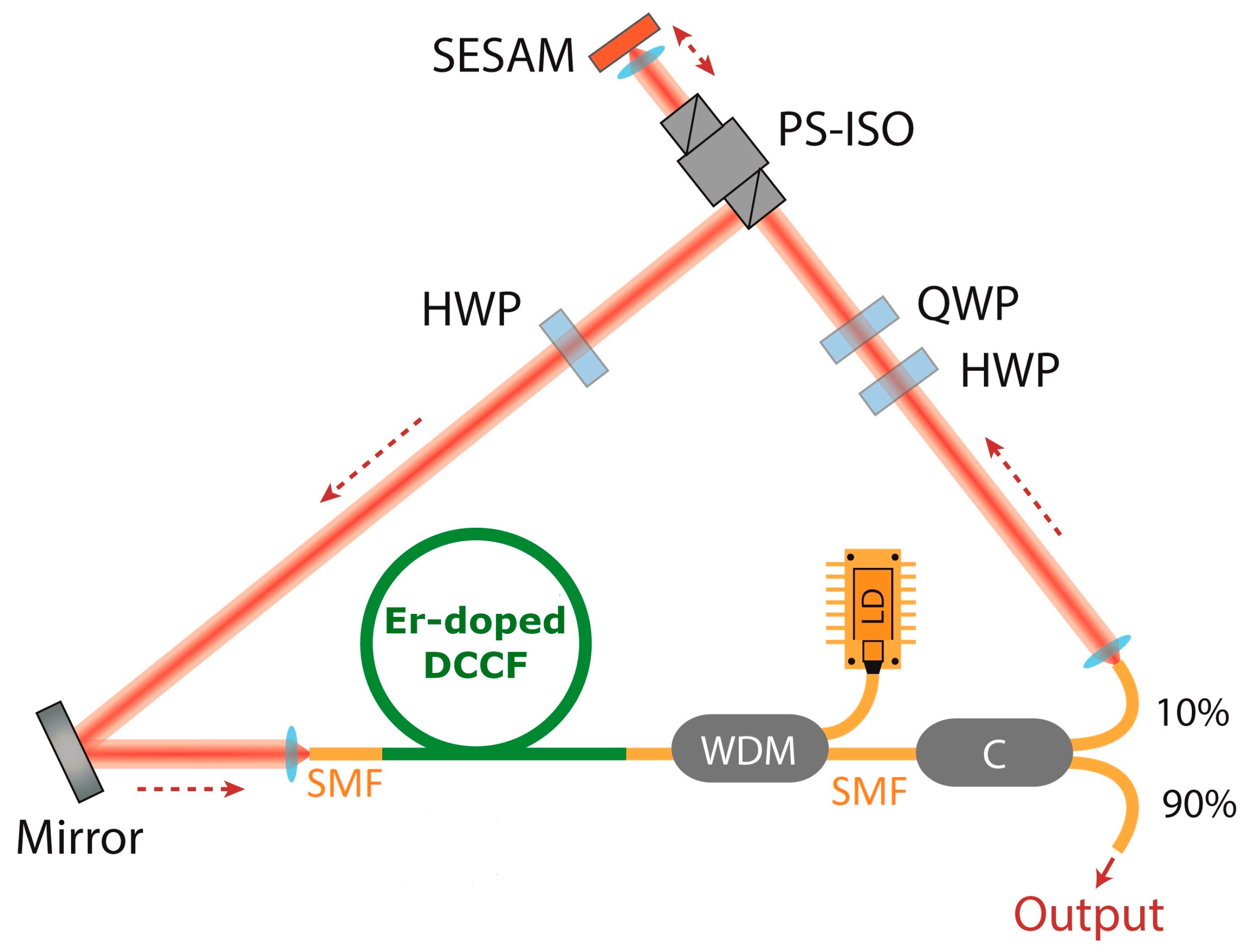
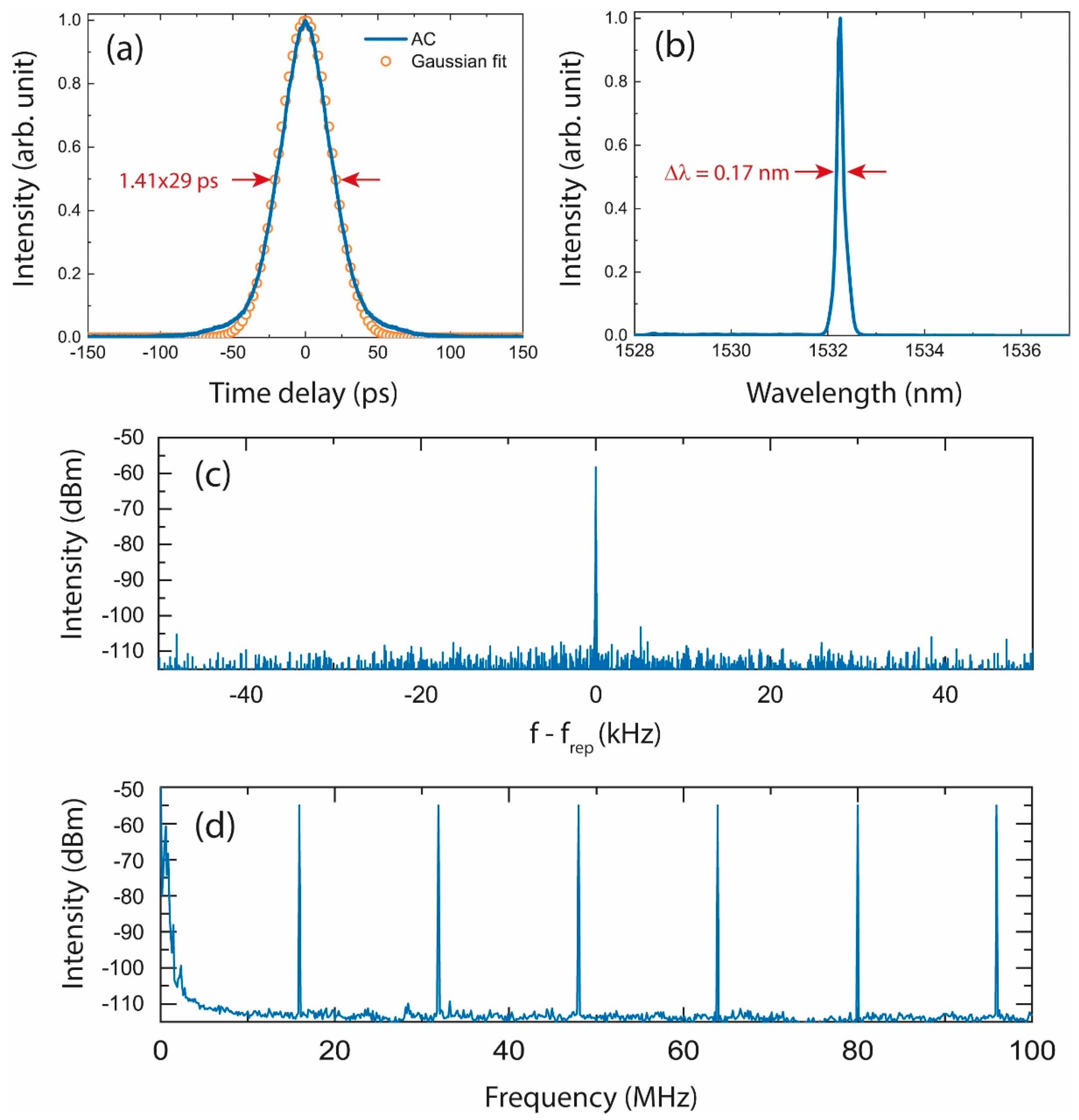

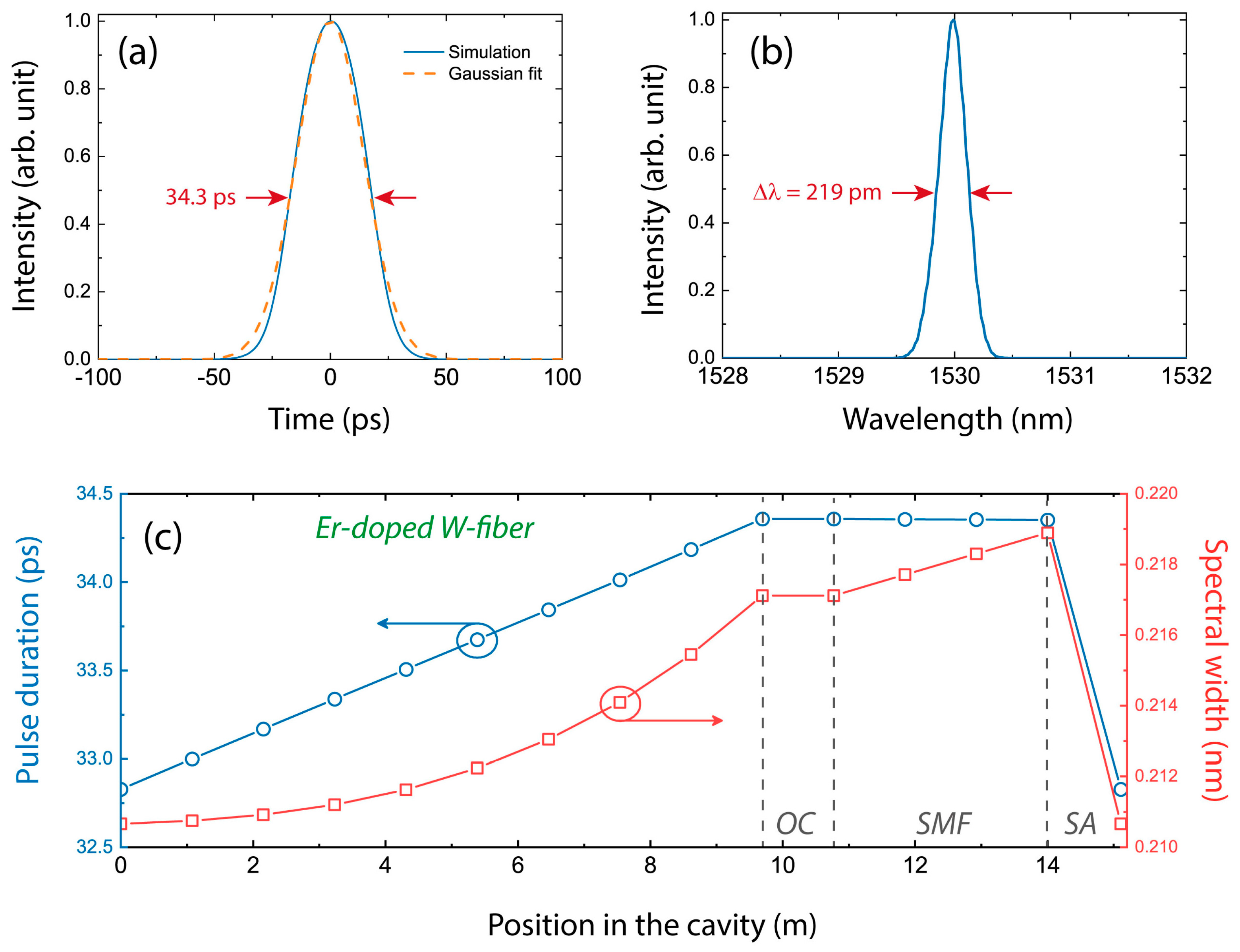
| Parameter | Units | Er-DCCF | SMF |
|---|---|---|---|
| Length | m | 10 | 1.5 |
| GVD (β2@1530 nm) | ps2/km | 100 | −20 |
| Dispersion slope (β3) | ps3/km | −2 | −0.13 |
| Nonlinear coefficient (γ) | W−1 km−1 | 1.7 | 1.6 |
| Small signal gain (g0) | m−1 | 2 | - |
| Gain bandwidth (Δλg) | nm | 30 | - |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, M.; Granger, G.; Lesparre, F.; Wang, H.; Qian, K.; Lecaplain, C.; Oudar, J.-L.; Jaouen, Y.; Gabet, R.; Gaponov, D.; et al. Large Normal Dispersion Mode-Locked Erbium-Doped Fiber Laser. Fibers 2019, 7, 97. https://doi.org/10.3390/fib7110097
Tang M, Granger G, Lesparre F, Wang H, Qian K, Lecaplain C, Oudar J-L, Jaouen Y, Gabet R, Gaponov D, et al. Large Normal Dispersion Mode-Locked Erbium-Doped Fiber Laser. Fibers. 2019; 7(11):97. https://doi.org/10.3390/fib7110097
Chicago/Turabian StyleTang, Mincheng, Geoffroy Granger, Fabien Lesparre, Hongjie Wang, Kai Qian, Caroline Lecaplain, Jean-Louis Oudar, Yves Jaouen, Renaud Gabet, Dmitry Gaponov, and et al. 2019. "Large Normal Dispersion Mode-Locked Erbium-Doped Fiber Laser" Fibers 7, no. 11: 97. https://doi.org/10.3390/fib7110097
APA StyleTang, M., Granger, G., Lesparre, F., Wang, H., Qian, K., Lecaplain, C., Oudar, J.-L., Jaouen, Y., Gabet, R., Gaponov, D., Likhachev, M., Godin, T., Février, S., & Hideur, A. (2019). Large Normal Dispersion Mode-Locked Erbium-Doped Fiber Laser. Fibers, 7(11), 97. https://doi.org/10.3390/fib7110097





