3D FE Analysis of RC Beams Externally Strengthened with SRG/SRP Systems
Abstract
:1. Introduction
2. Experimental Program
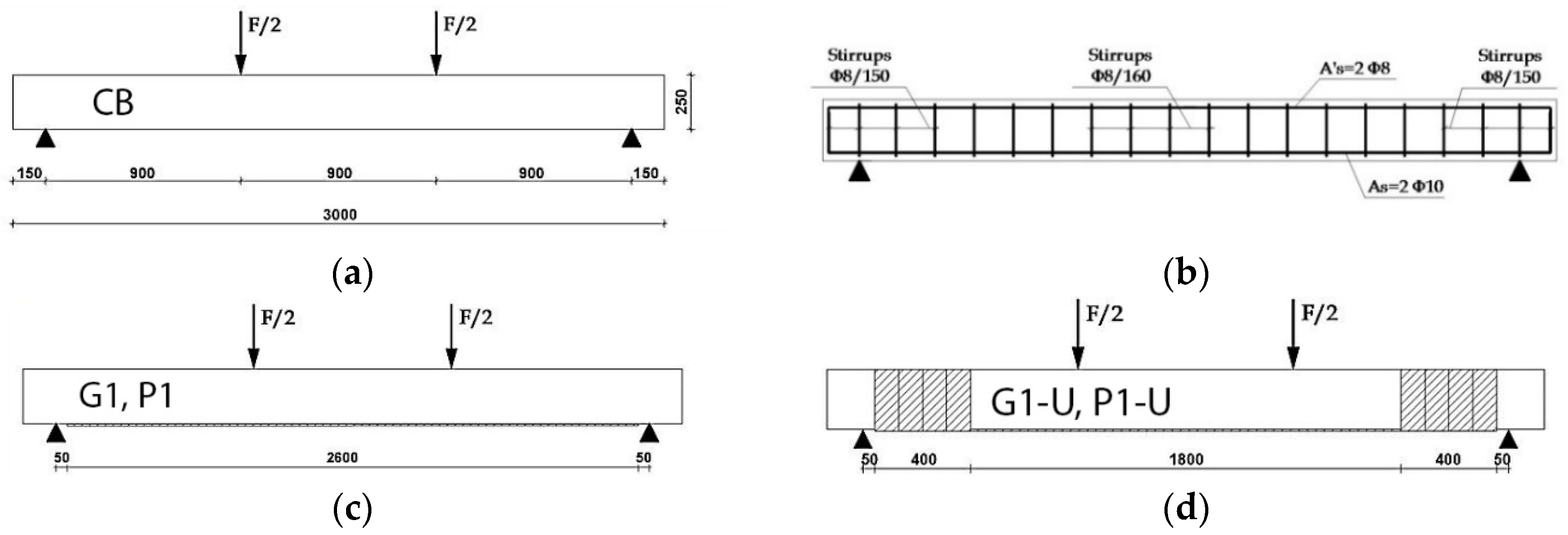
2.1. Beam Prototypes
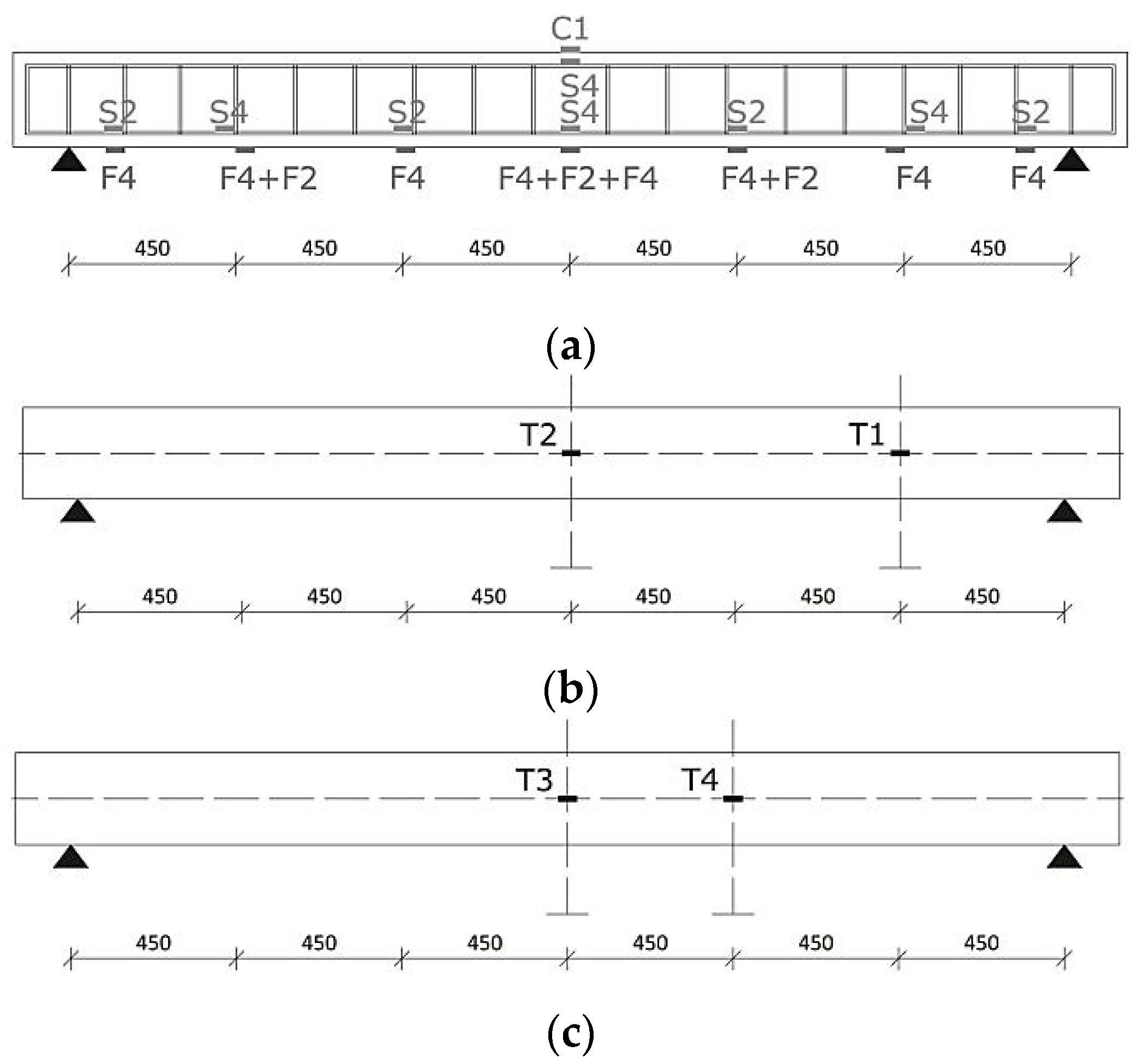
2.2. Test Setup and Instrumentation
2.3. Material Properties
2.4. Surface Preparation, Matrix, and Bonding Procedure
3. FE Modeling
3.1. Geometry
3.2. Material Models
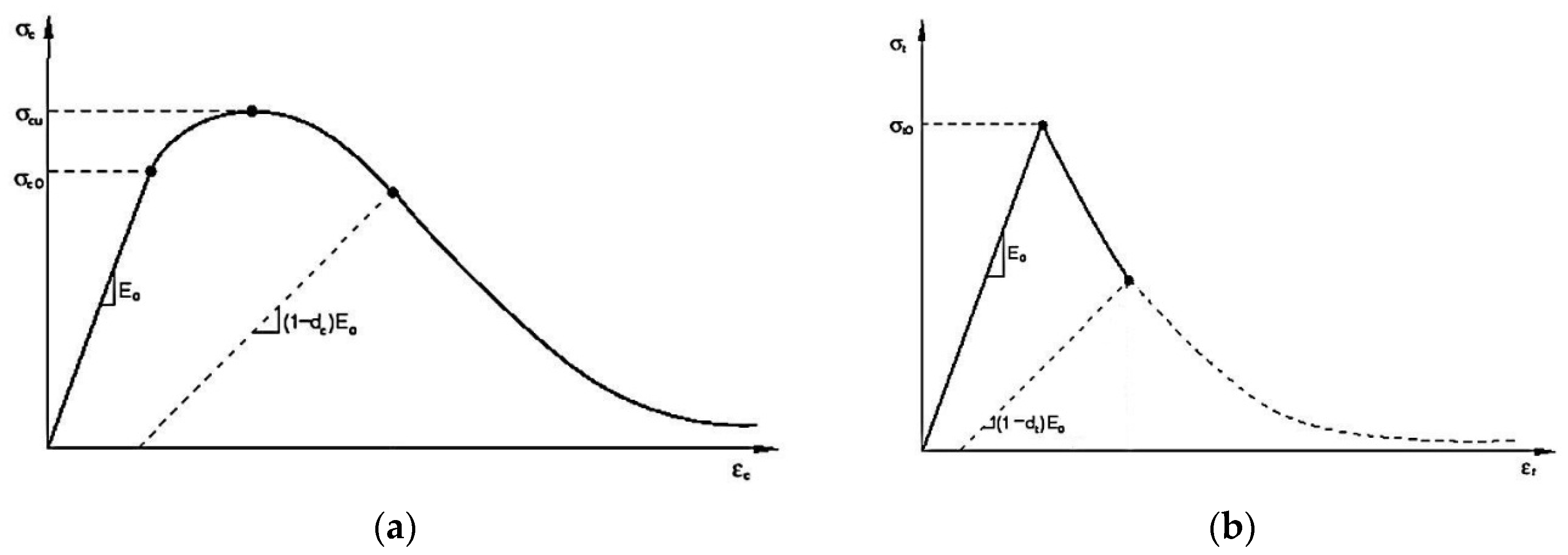
3.2.1. Concrete, Steel, SRG, and SRP Systems
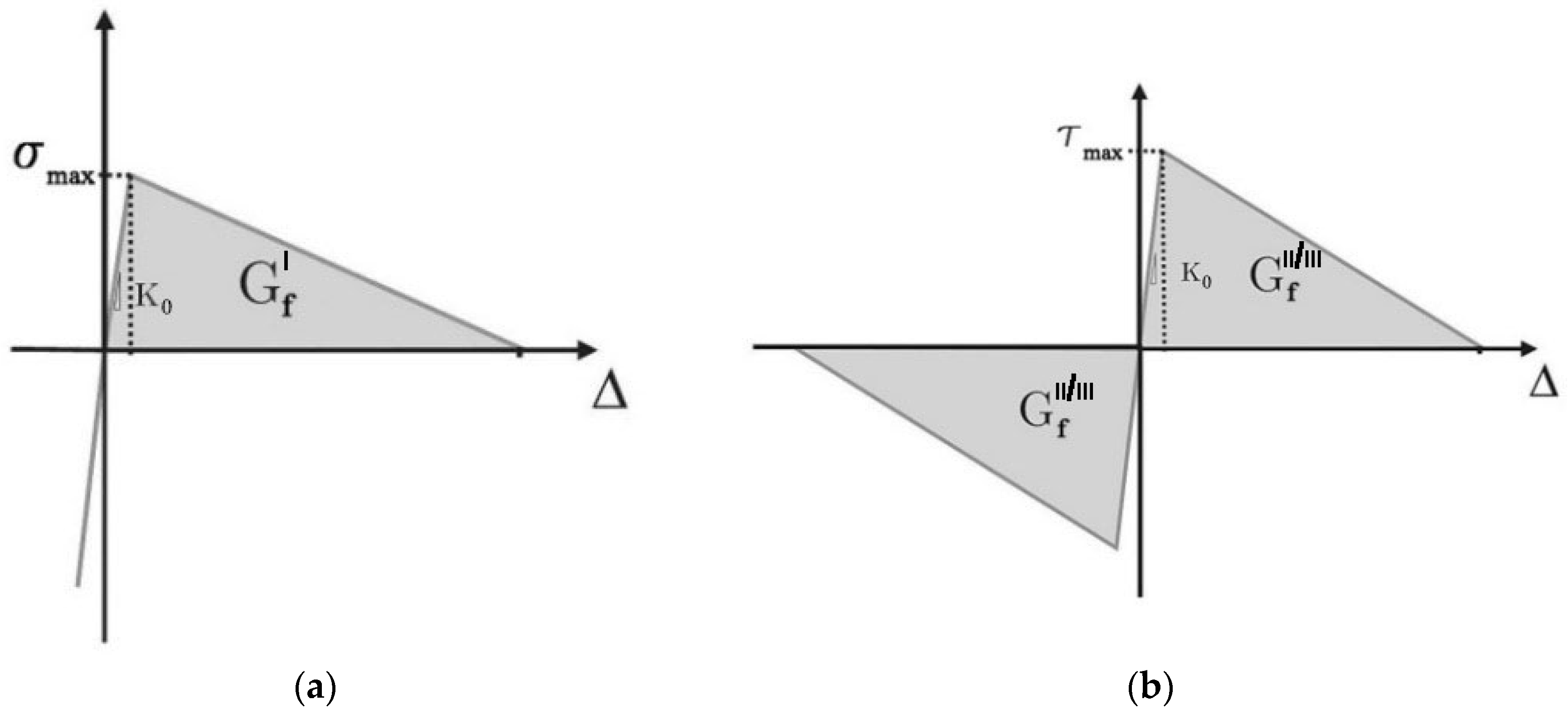
3.2.2. Interface Model and Bond-Slip Laws
3.3. Solution Technique
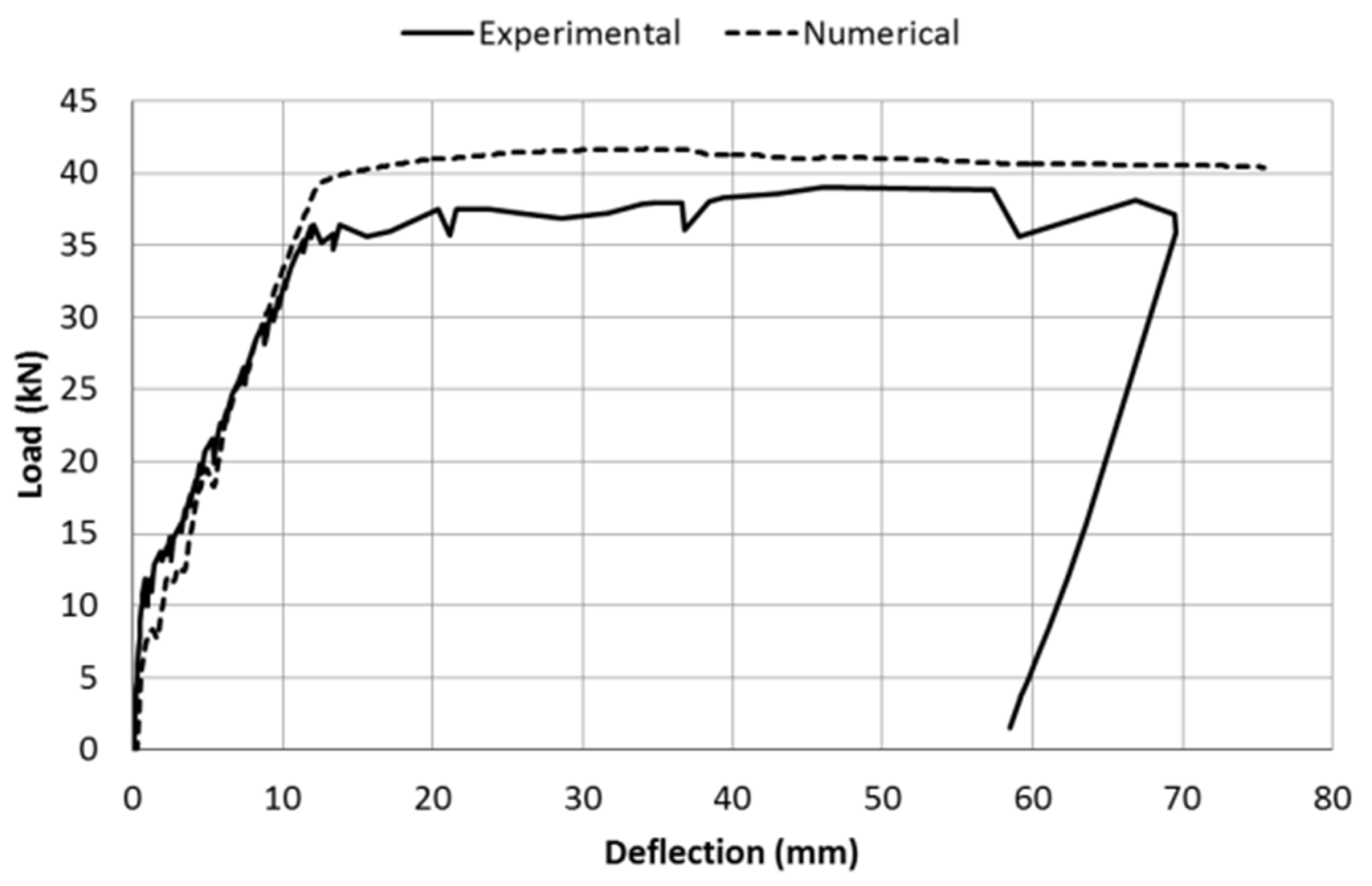
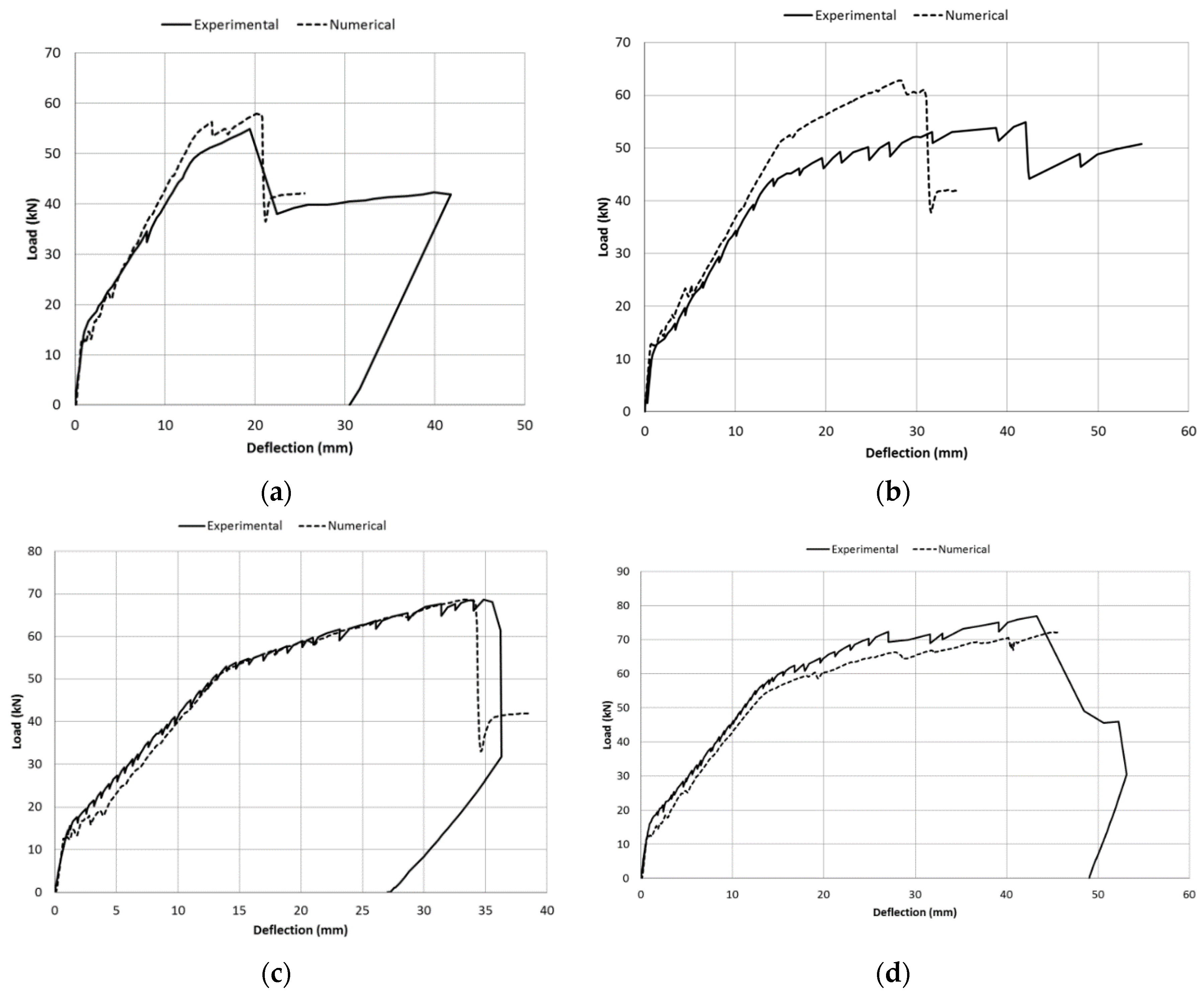
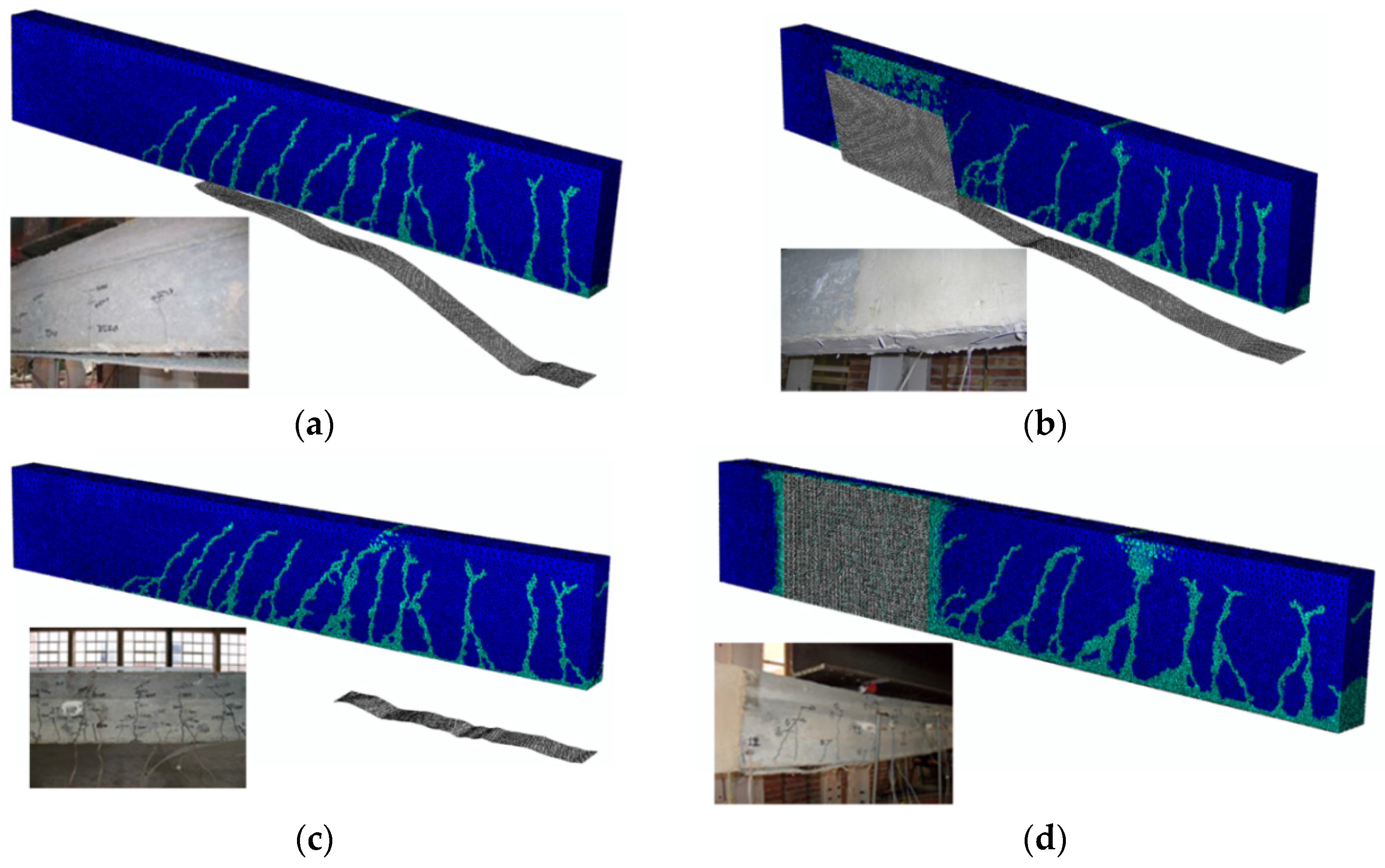
4. Numerical/Experimental Comparisons
5. Conclusions
- The SRG/SRP-concrete bond-slip laws calibrated and validated by Bencardino and Condello [23] provide a good prediction of the structural behavior of SRG/SRP strengthened RC beams with U-wrap end anchorages as well.
- Numerical failure modes are, in general, coherent with the experimental ones.
- The average percentage error on the ultimate load value is about 6.5% and it is 4.6% excluding the beam G1-U. This highlights the accuracy of the FE model.
- The explicit dynamic method, used for the nonlinear analysis, can efficiently overcome convergence difficulties due to strain softening. It can be a good alternative to various static solution methods to solve quasi-static structural problems which involve concrete cracking and debonding phenomena.
- Engineers and researchers can use the proposed FE model as a numerical tool to investigate the performance of similar beams without carrying out experimental tests.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Bakis, C.E.; Bank, L.C.; Brown, V.L.; Cosenza, E.; Davalos, J.F.; Lesko, J.J.; Machida, A.; Rizkalla, S.H.; Triantafillou, T.C. Fiber-reinforced polymer composites for construction-state-of-the-art review. J. Compos. Constr. 2002, 6, 73–87. [Google Scholar] [CrossRef]
- Bencardino, F.; Colotti, V.; Spadea, G.; Swamy, R.N. Holistic design of RC beams and slabs strengthened with externally bonded FRP laminates. Cem. Concr. Compos. 2006, 28, 832–844. [Google Scholar] [CrossRef]
- Demakos, C.B.; Repapis, C.C.; Drivas, D. Investigation of structural response of reinforced concrete beams strengthened with anchored FRPs. Open Constr. Build. Technol. J. 2013, 7, 146–157. [Google Scholar] [CrossRef]
- Jumaat, M.Z.; Ashraful Alam, M.D. Experimental and numerical analysis of end anchored steel plate and CFRP laminate flexurally strengthened reinforced concrete (r.c.) beams. Int. J. Phys. Sci. 2010, 5, 132–144. [Google Scholar]
- Lu, X.Z.; Ye, L.P.; Teng, J.G.; Jiang, J.J. Meso-scale finite element model for FRP sheets/plates bonded to concrete. Eng. Struct. 2005, 27, 564–575. [Google Scholar] [CrossRef]
- Obaidat, Y.T.; Heyden, S.; Dahlblom, O. The Effect of CFRP and CFRP/Concrete Interface Models when modelling Retrofitted RC Beams with FEM. Compos. Struct. 2010, 92, 1391–1398. [Google Scholar] [CrossRef]
- Camata, G.; Spacone, E.; Zarnic, R. Experimental and nonlinear finite element studies of RC beams strengthened with FRP plates. Compos. B Eng. 2007, 38, 277–288. [Google Scholar] [CrossRef]
- Zhang, L.; Teng, J.G. Finite element prediction of interfacial stresses in structural members bonded with a thin plate. Eng. Struct. 2010, 32, 459–471. [Google Scholar] [CrossRef]
- Figeys, W.; Schueremans, L.; van Gemert, D.; Brosens, K. A new composite for external reinforcement: Steel cord reinforced polymer. Constr. Build. Mater. 2008, 22, 1929–1938. [Google Scholar] [CrossRef]
- Huang, X.; Birman, V.; Nanni, A.; Tunis, G. Properties and potential for application of steel reinforced polymer and steel reinforced grout composites. Compos. B Eng. 2004, 36, 73–82. [Google Scholar] [CrossRef]
- Abdelrahman, K.; El-Hacha, R. Behavior of large-scale concrete columns wrapped with CFRP and SFRP sheets. J. Compos. Constr. 2012, 16, 430–439. [Google Scholar] [CrossRef]
- Bencardino, F.; Condello, A. Structural behaviour of RC beams externally strengthened in flexure with SRG and SRP systems. Int. J. Struct. Eng. 2014, 5, 346–368. [Google Scholar] [CrossRef]
- Bencardino, F.; Ombres, L. Structural performance of RC beams strengthened by SRG and FRCM system. In Large Structures Infrastructures Environmentally Constrained and Urbanised Areas; ISBSE: Zurich, Switzerland, 2010; pp. 488–489. [Google Scholar]
- Barton, B.; Wobbe, E.; Dharani, L.R.; Silva, P.; Birman, V.; Nanni, A.; Alkhrdaji, T.; Thomas, J.; Tunis, G. Characterization of reinforced concrete beams strengthened by steel reinforced polymer and grout (SRP and SRG) composites. Mater. Sci. Eng. 2005, 412, 129–136. [Google Scholar] [CrossRef]
- Balsamo, A.; Nardone, F.; Iovinella, I.; Ceroni, F.; Pecce, M. Flexural strengthening of concrete beams with EB-FRP, SRP and SRCM: Experimental investigation. Compos. B Eng. 2013, 46, 91–101. [Google Scholar] [CrossRef]
- El-Hacha, R.; Mashrik, M.A. Effect of SFRP confinement on circular and square concrete columns. Eng. Struct. 2012, 36, 379–393. [Google Scholar] [CrossRef]
- Napoli, A.; Realfonzo, R. Compressive behavior of concrete confined by SRP wraps. Constr. Build. Mater. 2015. [Google Scholar] [CrossRef]
- Ombres, L. Flexural analysis of reinforced concrete beams strengthened with a cement based high strength composite material. Compos. Struct. 2011, 94, 143–155. [Google Scholar] [CrossRef]
- Pecce, M.; Ceroni, F.; Prota, A.; Manfredi, G. Response prediction of RC beams externally bonded with steel-reinforced polymers. J. Compos. Constr. 2006, 10, 195–203. [Google Scholar] [CrossRef]
- Thermou, G.E.; Katakalos, K.; Manos, G. Influence of the cross section shape on the behaviour of SRG-confined prismatic concrete specimens. Mater. Struct. 2016, 49, 869–887. [Google Scholar] [CrossRef]
- Thermou, G.E.; Katakalos, K.; Manos, G. Concrete confinement with steel-reinforced grout jackets. Mater. Struct. 2015, 48, 1355–1376. [Google Scholar] [CrossRef]
- Thermou, G.E.; Pantazopoulou, S.J. Metallic fabric jackets: An innovative method for seismic retrofitting of substandard RC prismatic members. Struct. Concr. 2007, 8, 35–46. [Google Scholar] [CrossRef]
- Bencardino, F.; Condello, A. SRG/SRP–concrete bond–slip laws for externally strengthened RC beams. Compos. Struct. 2015, 132, 804–815. [Google Scholar] [CrossRef]
- Bencardino, F.; Condello, A. Reliability and adaptability of the analytical models proposed for the FRP systems to the Steel Reinforced Polymer and Steel Reinforced Grout strengthening systems. Compos. B Eng. 2015, 76, 249–259. [Google Scholar] [CrossRef]
- Technical data sheets Kimia S.p.A. Unidirectional high-strength carbon steel fibre reinforcing mesh, Kimisteel 1500, ST2-0610; Ready-to-use fine granulometry mortar for SRG and SRP systems, Kimisteel LM, ST2-0610; Synthetic resin used to improve the chemical and physical properties of single-component mortars (SRG), Kimitech B2, ST9-0607; Bicomponent epoxy resin used to improve the chemical and physical properties of single-component mortars (SRP), Kimicover FIX, ST3-0503, 2009. Available online: http://www.kimia.it/en/prodotti/categorie-merceologiche/83 (accessed on 22 September 2009).
- Hibbitt, Karlsson and Sorensen, Inc. ABAQUS Finite Element Code (2011); Hibbitt, Karlsson & Sorensen: Pawtucket, RI, USA, 2011. [Google Scholar]
- Lee, J.; Fenves, G. Plastic-Damage Model for Cyclic Loading of Concrete Structures. J. Eng. Mech. 1998, 124, 892–900. [Google Scholar] [CrossRef]
- Lubliner, J.; Oliver, J.; Oller, S.; Oñate, E. A Plastic-Damage Model for Concrete. Int. J. Solids Struct. 1989, 25, 299–329. [Google Scholar] [CrossRef]
- European Committee for Standardization. Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings EN 1992-1-1; Eurocode 2; European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- Hognestad, E. A Study of Combined Bending and Axial Load in Reinforced Concrete Members. In Bulletin Series No. 399, Engineering Experiment Station; University of Illinois: Champaign, IL, USA, 1951; Volume 49. [Google Scholar]
- Alfano, G.; Crisfield, M.A. Finite element interface models for the delamination analysis of laminated composites: Mechanical and computational issues. Int. J. Numer. Methods Eng. 2001, 50, 1701–1736. [Google Scholar] [CrossRef]
- Turon, A.; Davila, C.G.; Camanho, P.P.; Costa, J. An engineering solution for mesh size effects in the simulation of delamination using cohesive zone models. Eng. Fract. Mech. 2007, 74, 1665–1682. [Google Scholar] [CrossRef]
- Obaidat, Y.T.; Heyden, S.; Dahlblom, O. Evaluation of parameters of bond action between FRP and concrete. J. Compos. Constr. 2013, 17, 626–635. [Google Scholar] [CrossRef]
- Monti, M.; Renzelli, M.; Luciani, P. FRP adhesion in uncracked and cracked concrete zones. In Proceedings of the 6th International Symposium on FRP Reinforcement for Concrete Structures, Singapore, 8–10 July 2003.
- Lu, X.Z.; Teng, J.G.; Yea, L.P.; Jiang, J.J. Bond–slip models for FRP sheets/plates bonded to concrete. Eng. Struct. 2005, 27, 920–937. [Google Scholar] [CrossRef]
- Guide for the Design and Construction of Externally Bonded FRP Systems for Strengthening Existing Structures—Materials, RC and PC Structures, Masonry Structures; CNR-DT 200 R1/2013; Italian National Research Council: Rome, Italy, 2013.
- Chen, G.M.; Teng, J.G.; Chen, J.F.; Xiao, Q.G. Finite element modeling of debonding failures in FRP-strengthened RC beams: A dynamic approach. Comput. Struct. 2015, 158, 167–183. [Google Scholar] [CrossRef]


| Diameter of the Steel Bars | Yield Strength (MPa) | Tensile Strength (MPa) |
|---|---|---|
| Longitudinal 10 mm | 604.2 | 717.3 |
| Longitudinal 8 mm | 380.1 | 517.5 |
| Transversal 8 mm | 496.0 | 644.9 |
| Properties | Value |
|---|---|
| Total weight of fabric | 1528 g/m2 |
| Fiber direction (Warp–Steel) | 99% |
| Fiber direction (weft) | 1% |
| Diameter steel chord (plait/braid) | 1.07 mm |
| Equivalent dry fabric thickness (steel only) | 0.19 mm |
| Fiber tensile breaking stress | 2950 MPa |
| Unitary tensile strength (of the fabric) | 570 N/mm |
| Elastic tensile stress modulus (tangent modulus) | 206 GPa |
| Maximum tensile strain of the steel (εfu) | 2.3% |
| Properties | SRG | SRP |
|---|---|---|
| Appearance | powder | |
| Color | grey | |
| Apparent volumetric mass of wet mortar | 1750 kg/m3 | 1700 kg/m3 |
| Consistency | 170% | 180% |
| Setting time (start), EN 196-3 | 72 min | 135 min |
| Setting time (end), EN 196-3 | 95 min | 186 min |
| Compression strength at 28 days, EN 1015-12 | >45 MPa | |
| Flexural strength at 28 days, EN 1015-11 | >8 MPa | |
| Concrete adhesion, EN 1542 | >2 MPa | |
| System | Bond-Slip Law | K0 (N/mm3) | τmax (N/mm2) | Gf (N/mm) |
|---|---|---|---|---|
| SRG | Lu et al. [35] | 76.92 | 3.91 | 0.32 |
| SRP | Proposed [23] | 76.92 | 5.00 | 0.70 |
| Beam | Value | Failure Mode | Load (kN) | Ultimate Strain (‰) | Err (%) | ||||
|---|---|---|---|---|---|---|---|---|---|
| Fcr | Fy | Fu | εc | εs | εf | ||||
| CB | Exp. | Concrete crushing | 9.89 | 35.30 | 38.98 | 3.53 | 25.29 | - | 6.90 |
| FEA | Concrete crushing | 11.01 | 39.06 | 41.67 | 3.50 | 21.76 | |||
| G1 | Exp. | End debonding | 13.71 | 48.07 | 54.93 | 2.17 | 3.04 | 4.95 | 5.39 |
| FEA | Intermediate debonding | 12.68 | 50.74 | 57.89 | 1.61 | 2.87 | 5.54 | ||
| G1-U | Exp. | U-wrap debonding | 11.92 | 44.16 | 54.93 | 2.83 | 3.23 | 7.36 | 13.95 |
| FEA | U-wrap debonding | 12.85 | 52.39 | 62.59 | 1.92 | 2.98 | 6.63 | ||
| P1 | Exp. | Intermediate debonding | 12.80 | 51.04 | 68.64 | 2.76 | 21.62 | 10.42 | 0.04 |
| FEA | Intermediate debonding | 12.63 | 50.89 | 68.67 | 2.37 | 6.35 | 9.89 | ||
| P1-U | Exp. | UHTSS rupture | 14.65 | 55.93 | 76.88 | 3.07 | 23.75 | 11.48 | 6.13 |
| FEA | UHTSS rupture | 12.86 | 52.74 | 72.17 | 2.72 | 7.02 | 10.67 | ||
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bencardino, F.; Condello, A. 3D FE Analysis of RC Beams Externally Strengthened with SRG/SRP Systems. Fibers 2016, 4, 19. https://doi.org/10.3390/fib4020019
Bencardino F, Condello A. 3D FE Analysis of RC Beams Externally Strengthened with SRG/SRP Systems. Fibers. 2016; 4(2):19. https://doi.org/10.3390/fib4020019
Chicago/Turabian StyleBencardino, Francesco, and Antonio Condello. 2016. "3D FE Analysis of RC Beams Externally Strengthened with SRG/SRP Systems" Fibers 4, no. 2: 19. https://doi.org/10.3390/fib4020019
APA StyleBencardino, F., & Condello, A. (2016). 3D FE Analysis of RC Beams Externally Strengthened with SRG/SRP Systems. Fibers, 4(2), 19. https://doi.org/10.3390/fib4020019






