Numerical Simulation of Engineering Cementitious Composite Beams Strengthened with Fiber-Reinforced Polymer and Steel Bars
Abstract
1. Introduction
2. Materials and Methods
2.1. Material Properties
2.1.1. Concrete
2.1.2. ECC Material
2.1.3. Steel Reinforcement and Steel Plates
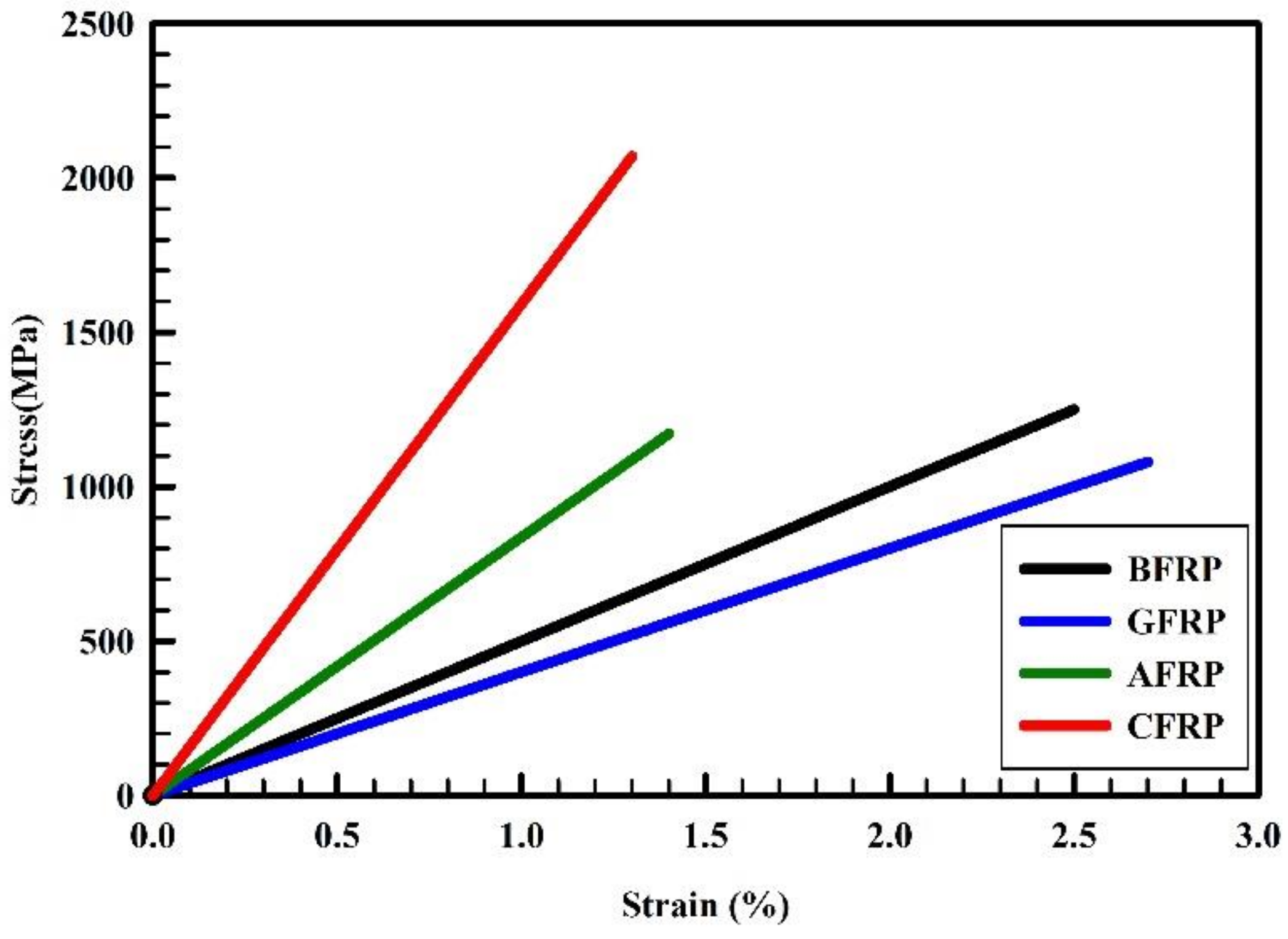
2.1.4. FRP Reinforcement
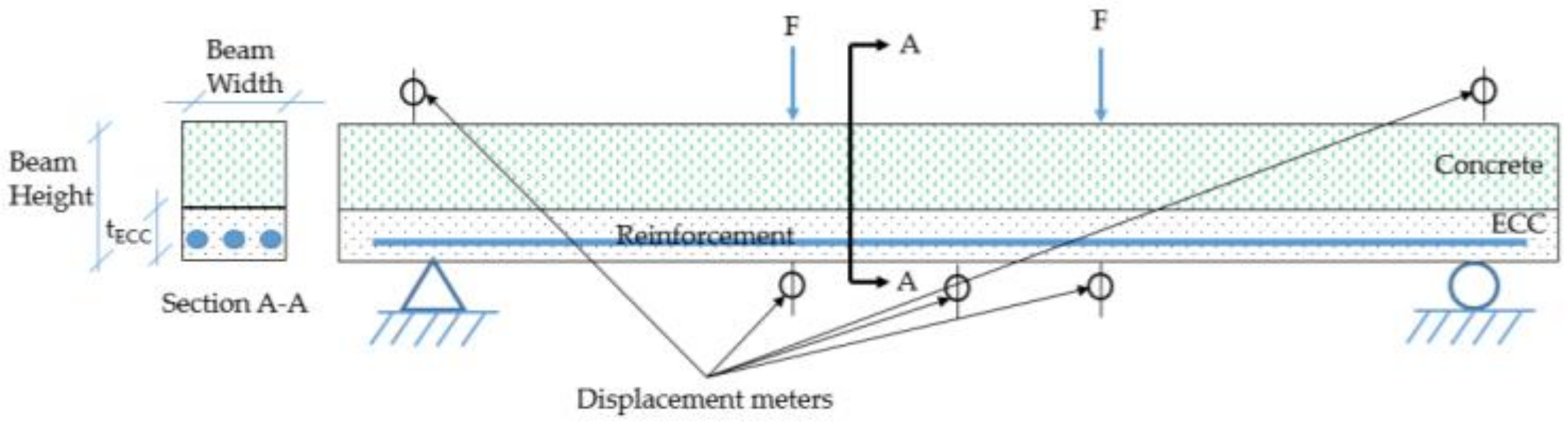
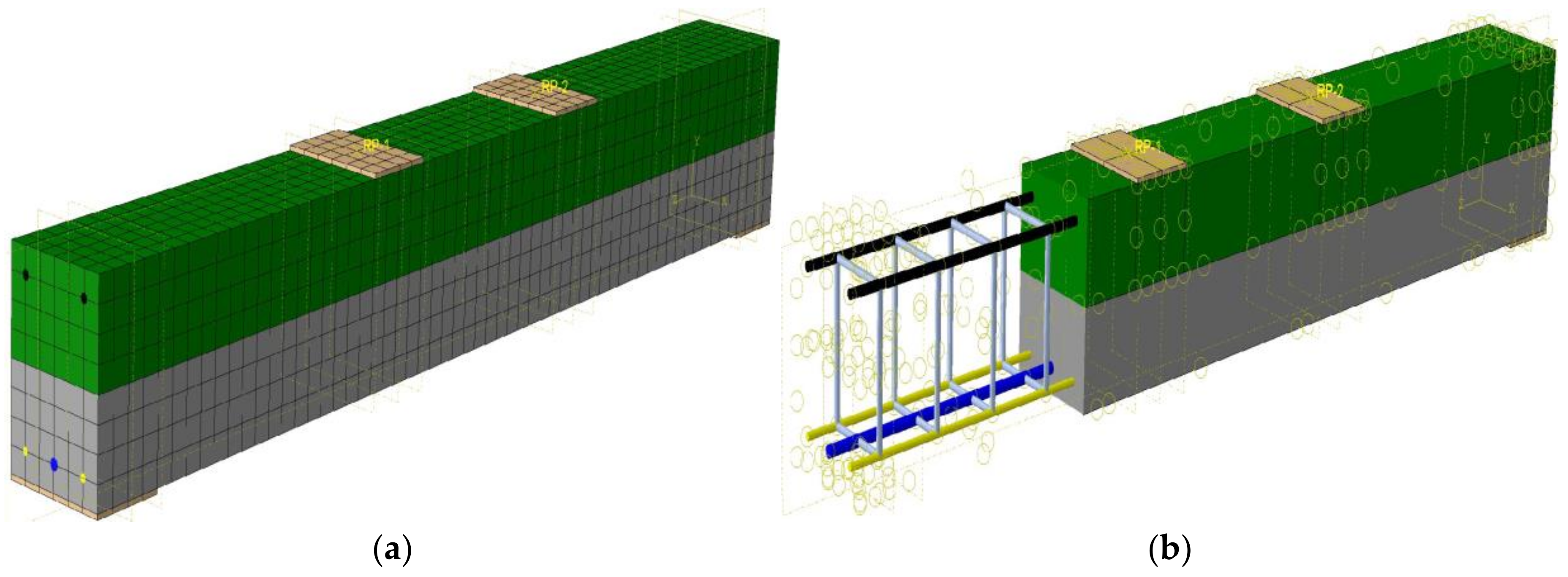
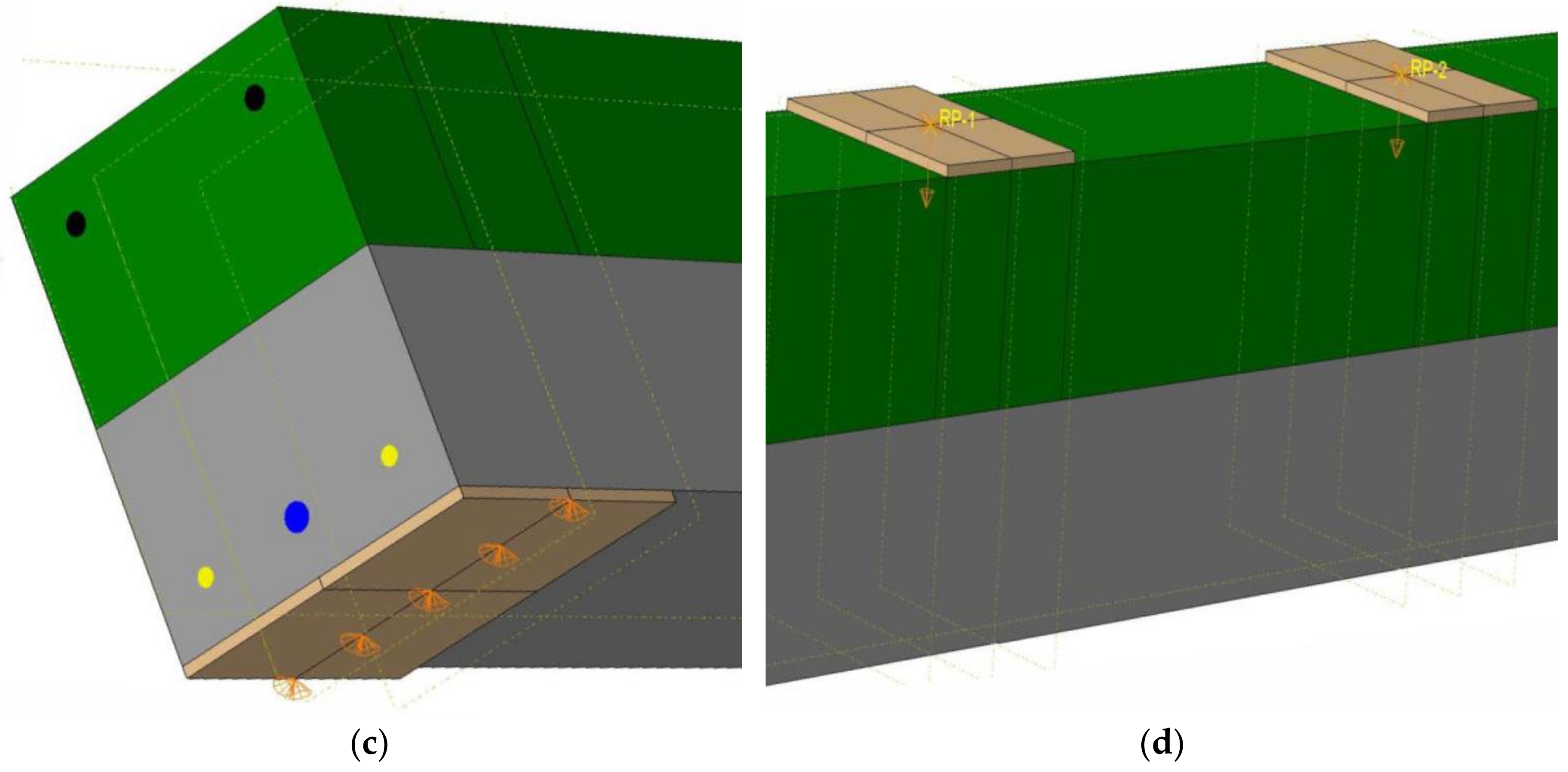
2.2. Nonlinear Finite Element Model (NLFEM)
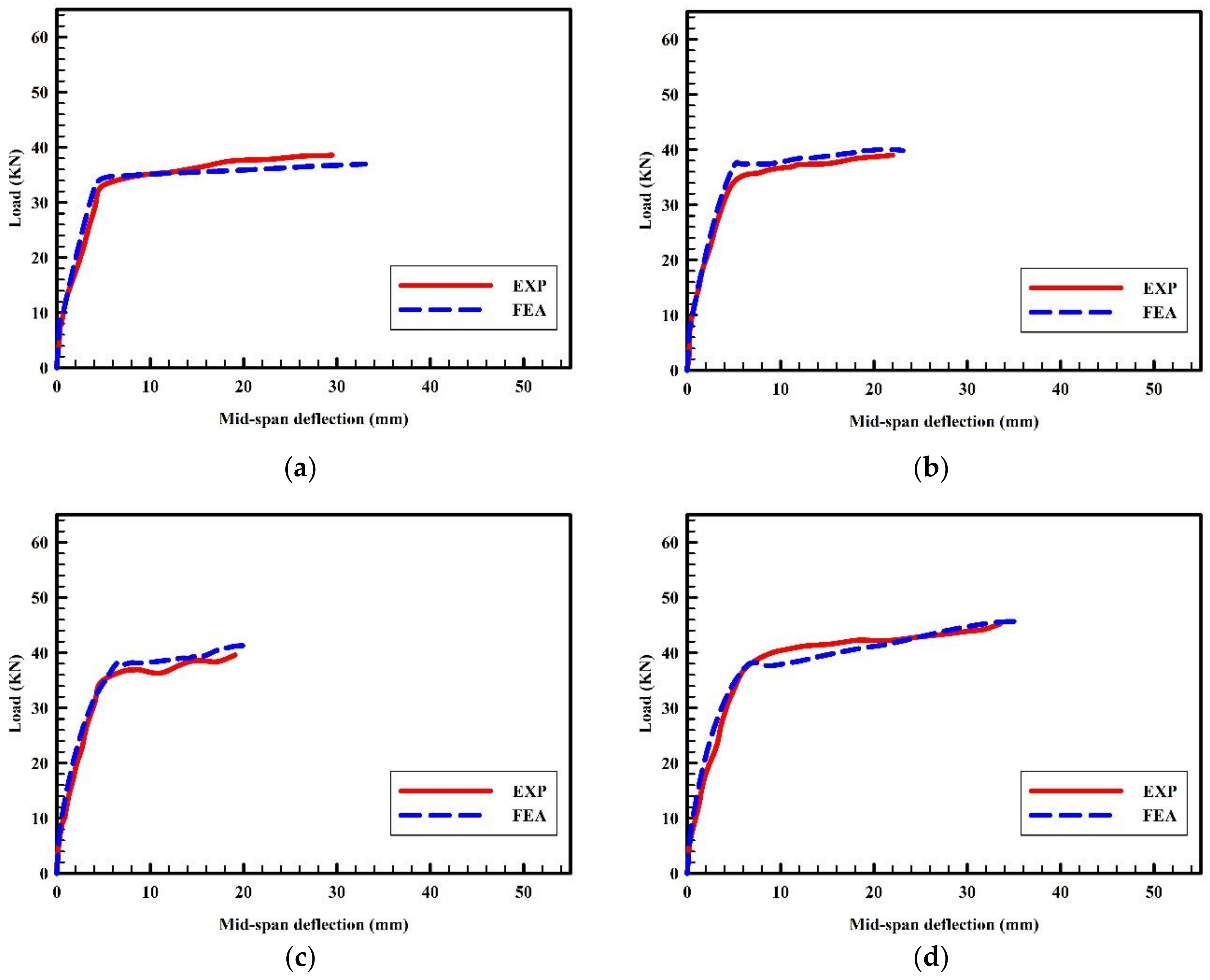
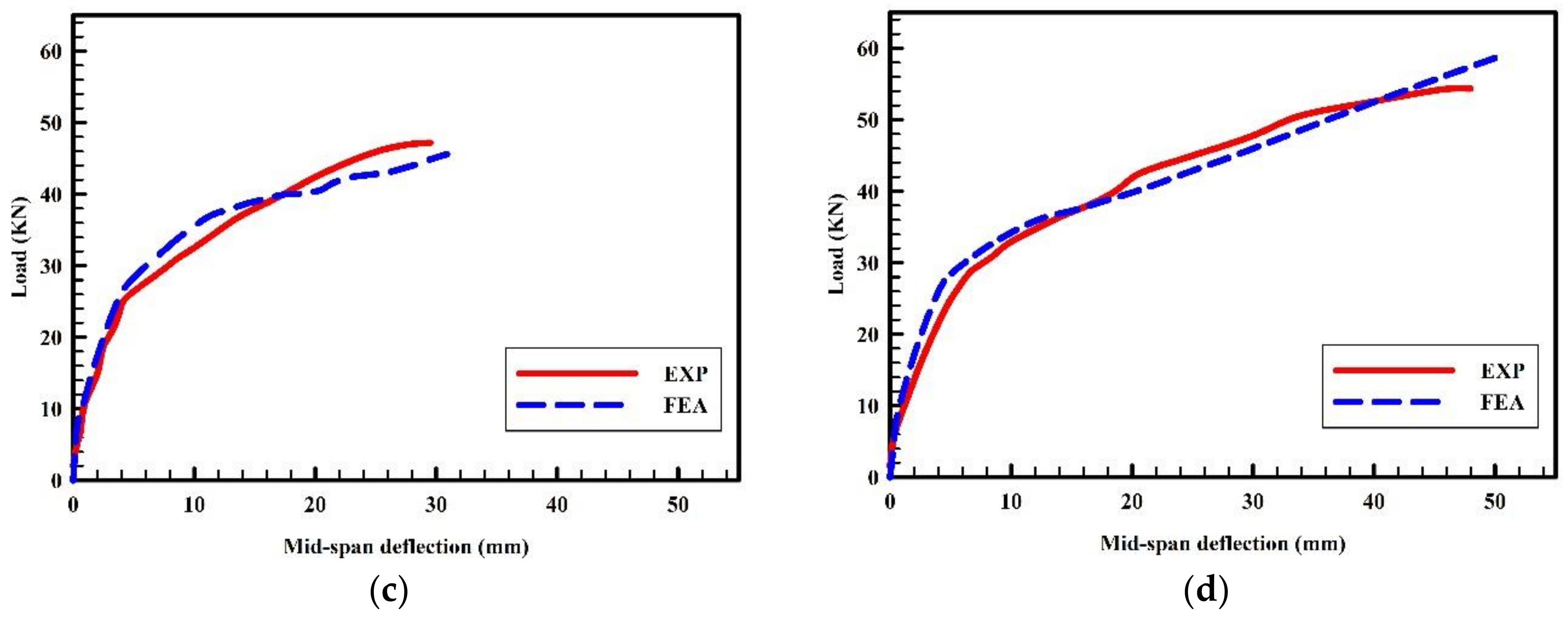
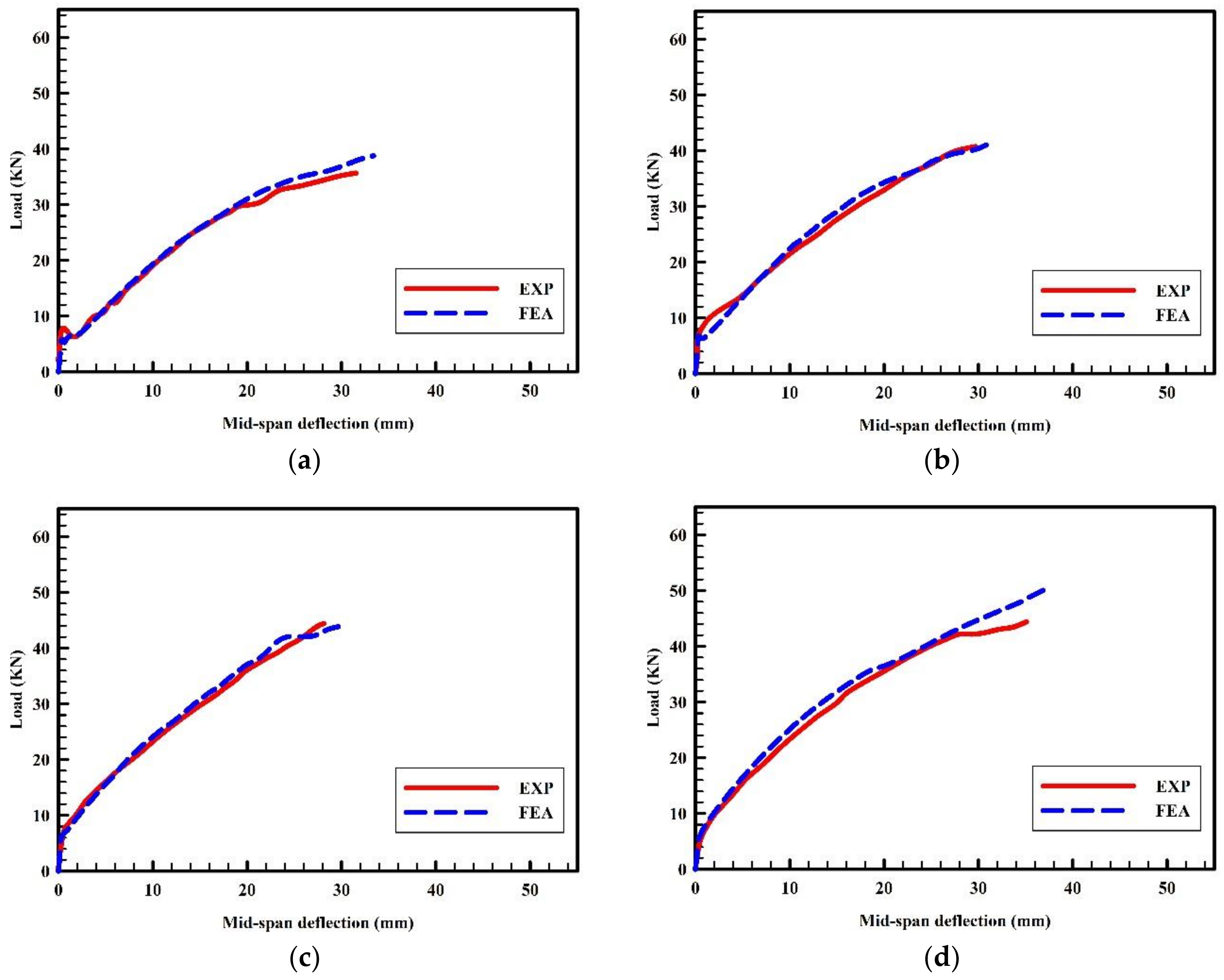
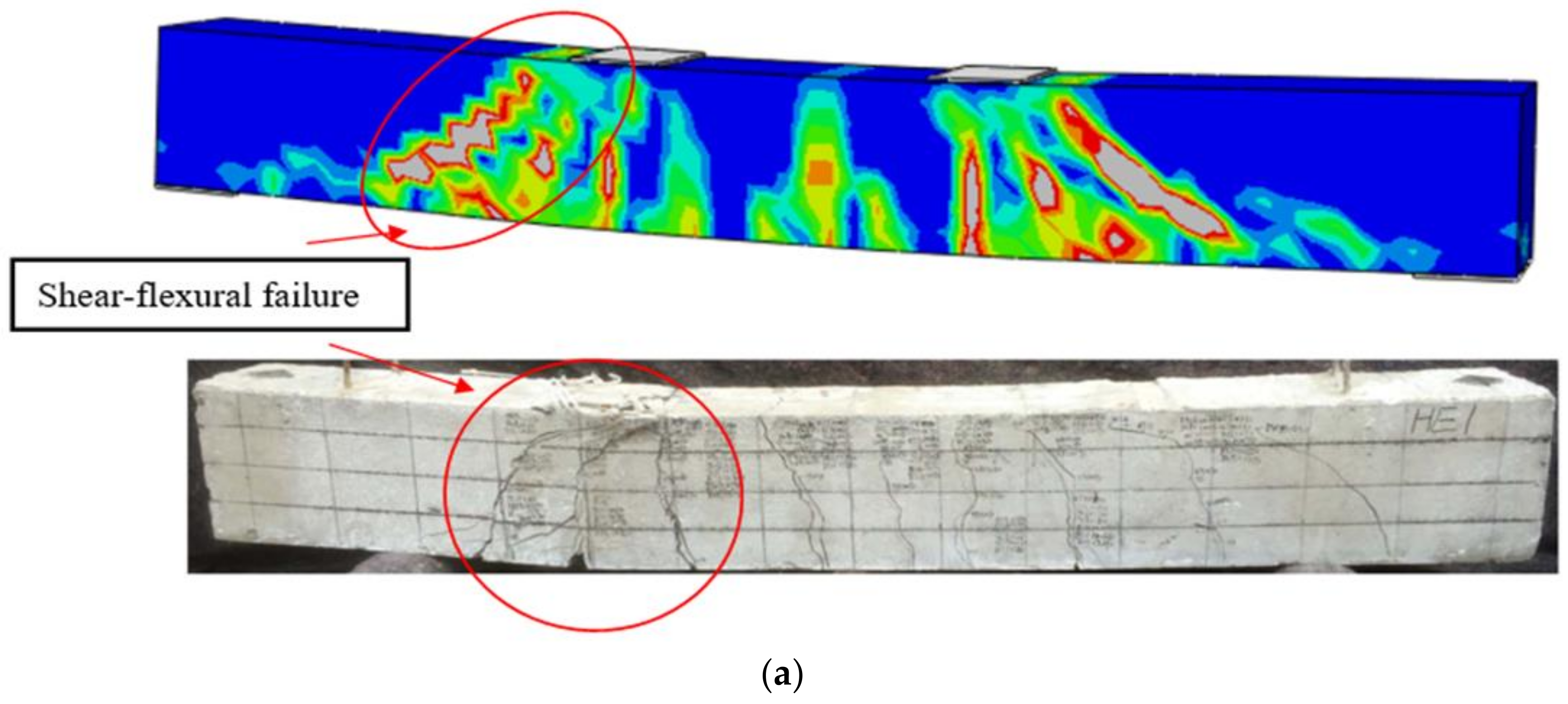
2.3. Model Verification
3. Results and Discussion
3.1. Parametric Study Parameters
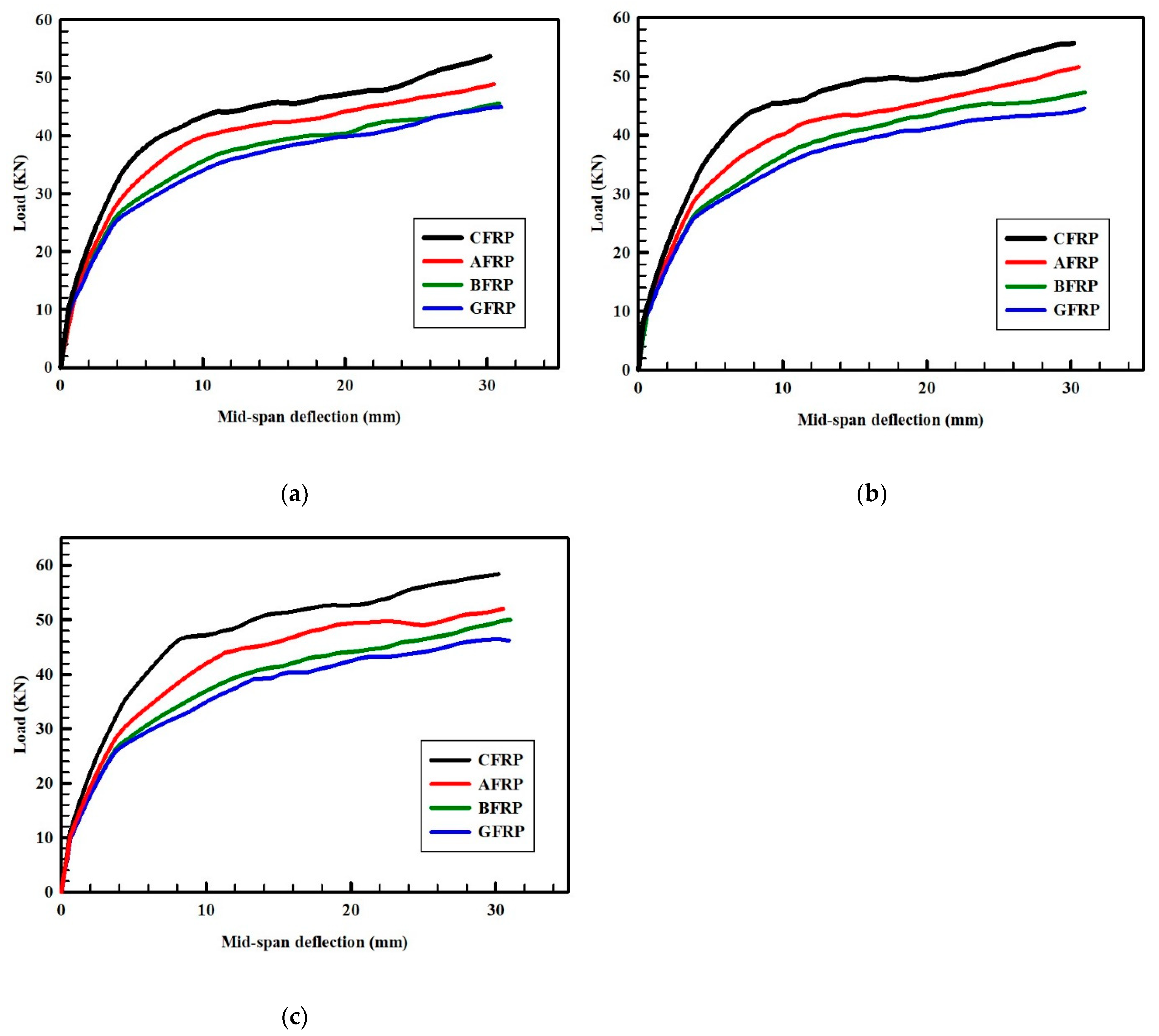
3.2. Effect of FRP Type and Concrete Compressive Strength
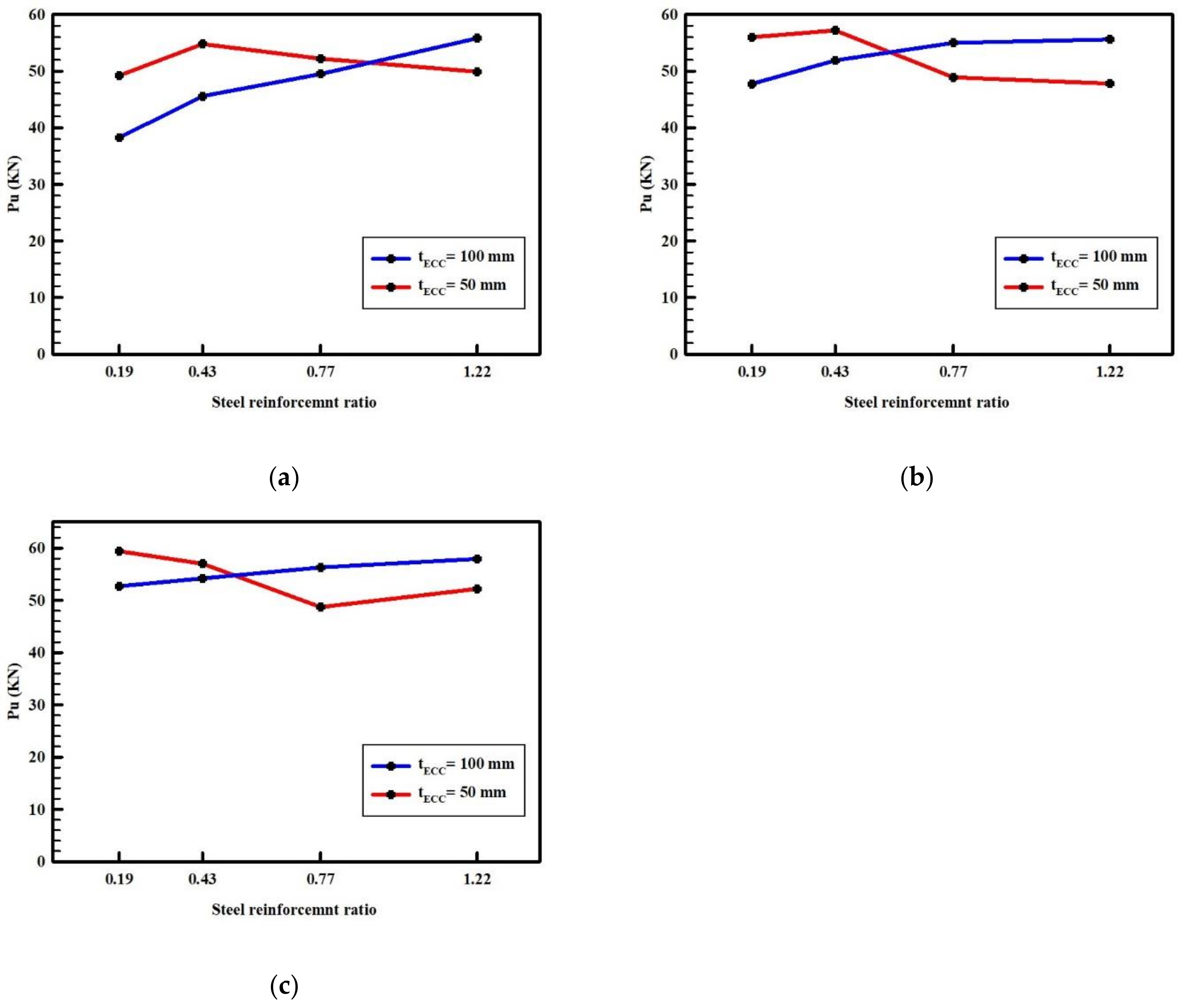
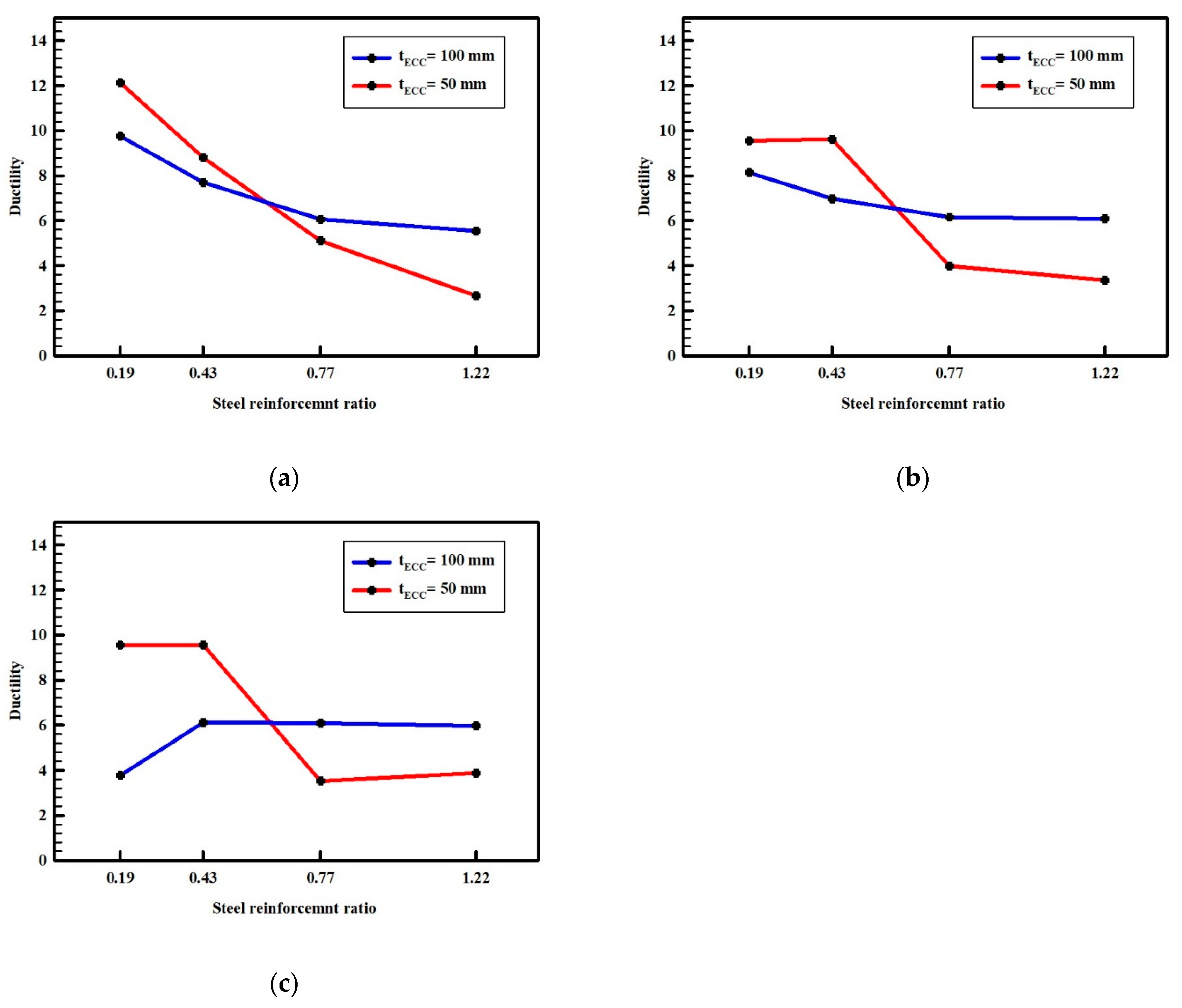
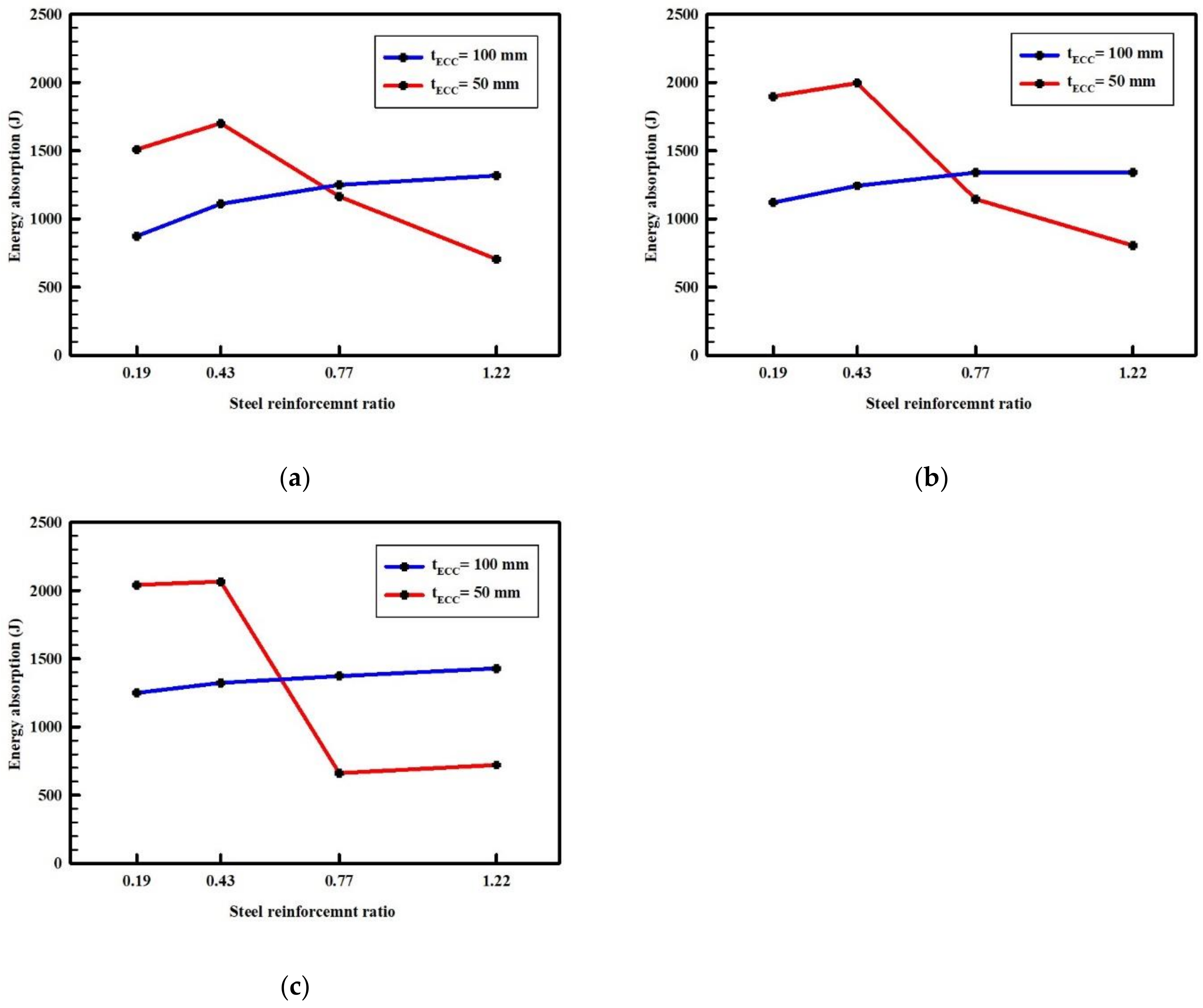
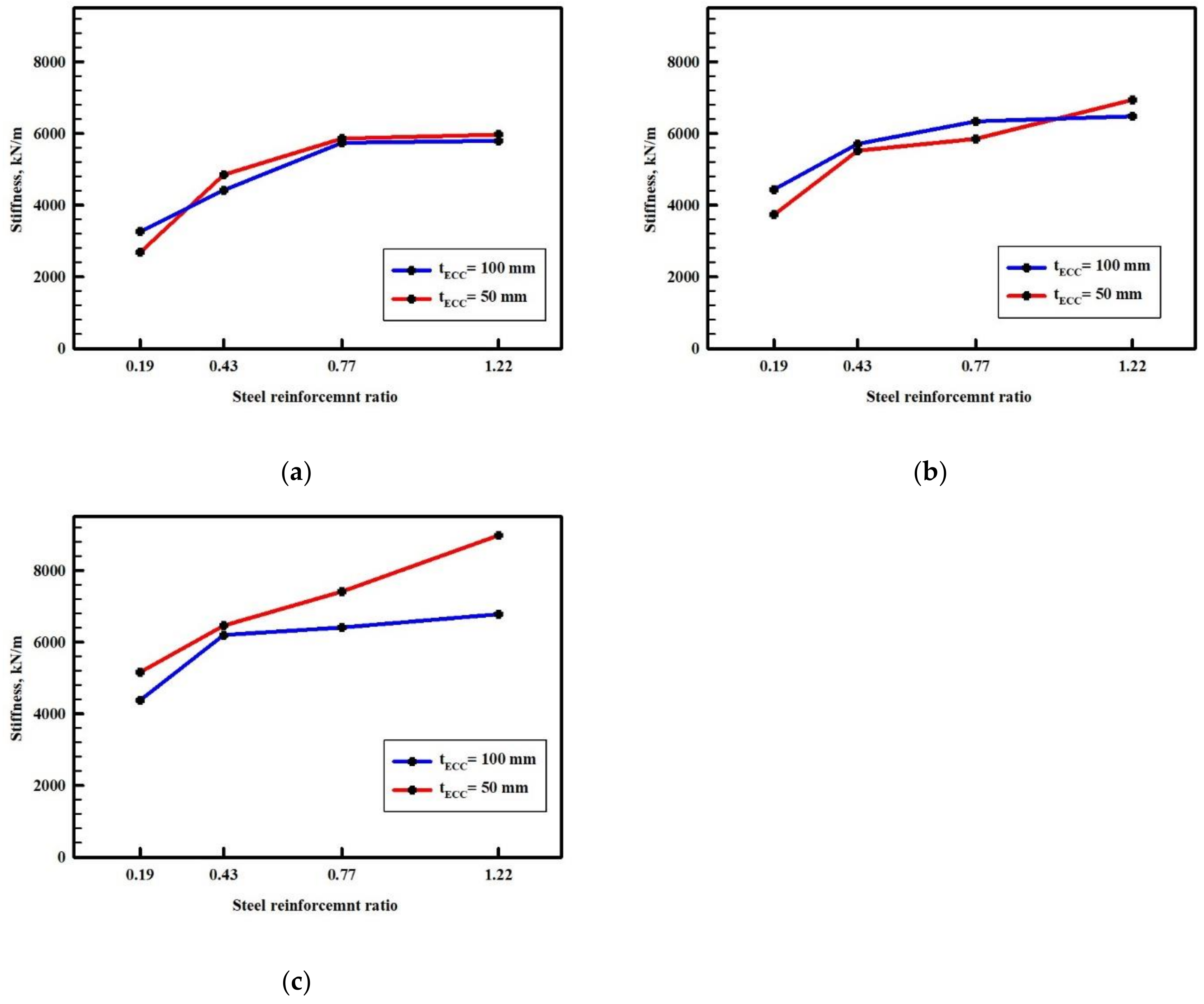
3.3. Effect of Hybrid Tensile Reinforcement Ratio and tECC
4. Conclusions
- The numerical simulation models accurately predict the load-deflection response with an error of less than 10% of the ultimate load, ultimate deflection, and stiffness. This provides researchers with a valuable tool for studying the behavior of ECC-concrete hybrid reinforced beams, particularly considering the higher costs associated with ECC material and FRP bars.
- In hybrid composite beams (using 50 mm ECC), when the BFRP reinforcement ratio is kept constant, utilizing a higher steel reinforcement ratio results in a reduction in ultimate load capacity, toughness, and ultimate deflection, along with insufficient ductility (less than 3), leading to early failure immediately after yielding of steel rebars due to high stress concentration in the ECC layer.
- In hybrid composite beams (using 100 mm ECC), when the BFRP reinforcement ratio is kept constant, using a higher steel reinforcement ratio leads to improvements in ultimate load, toughness, and flexural stiffness, but reduces beam ductility and shows a slight decrease in maximum deflection.
- With the remaining steel reinforcement ratio constant in the hybrid reinforcement system, an increase in the ratio of BFRP enhances ultimate load capacity, toughness, and flexural stiffness, but causes a decline in maximum deflection and ductility.
- The BFRP/steel tensile reinforcement ratio should be carefully designed to achieve the required strength, acceptable ductile performance, and improved load-carrying capacity of the composite beam.
- Strengthening composite hybrid beams with CFRP bars instead of BFRP results in better mechanical behavior in terms of ultimate load, toughness, flexural stiffness, and plastic stiffness, albeit with a reduction in ductility index. Conversely, replacing BFRP with GFRP bars exhibits a higher enhancement in ductility performance but a reduction in other mechanical properties. Additionally, the use of AFRP bars provides moderate mechanical behavior, performing better than BFRP but still inferior to CFRP bars.
- Using a higher grade of concrete strength in composite hybrid beams contributes minimally to improving mechanical behavior, with no noticeable effect on enhancement or reduction when using different types of FRP bars.
- In composite hybrid reinforced beams, using a 100 mm ECC layer in the tension zone significantly impacts the load-deflection response and greatly improves mechanical performance with increasing effective beam depth.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cosenza, E.; Manfredi, G.; Realfonzo, R. Behavior and modeling of bond of FRP rebars to concrete. J. Compos. Constr. 1997, 1, 40–51. [Google Scholar] [CrossRef]
- Gravina, R.J.; Smith, S.T. Flexural behaviour of indeterminate concrete beams reinforced with FRP bars. Eng. Struct. 2008, 30, 2370–2380. [Google Scholar] [CrossRef]
- Soric, Z.; Kisicek, T.; Galic, J. Deflections of concrete beams reinforced with FRP bars. Mater. Struct. 2010, 43, 73–90. [Google Scholar] [CrossRef]
- Qin, W.; Fernand, M.; Yang, Z.-J.; Georges, I.; Zakari, M.D. Effect of fiber volume ratio on flexural behavior of RC beam with novel HFRP rebar and steel rebar. Structures 2022, 43, 1882–1900. [Google Scholar] [CrossRef]
- Harris, H.G.; Somboonsong, W.; Ko, F.K. New ductile hybrid FRP reinforcing bar for concrete structures. J. Compos. Constr. 1998, 2, 28–37. [Google Scholar] [CrossRef]
- Aiello, M.A.; Ombres, L. Structural performances of concrete beams with hybrid (fiber-reinforced polymer-steel) reinforcements. J. Compos. Constr. 2002, 6, 133–140. [Google Scholar] [CrossRef]
- Leung, H.Y.; Balendran, R.V. Flexural behaviour of concrete beams internally reinforced with GFRP rods and steel rebars. Struct. Surv. 2003, 21, 146–157. [Google Scholar] [CrossRef]
- Lau, D.; Pam, H.J. Experimental study of hybrid FRP reinforced concrete beams. Eng. Struct. 2010, 32, 3857–3865. [Google Scholar] [CrossRef]
- Kara, I.F.; Ashour, A.F.; Köroğlu, M.A. Flexural behavior of hybrid FRP/steel reinforced concrete beams. Compos. Struct. 2015, 129, 111–121. [Google Scholar] [CrossRef]
- Maalej, M.; Li, V.C. Introduction of strain-hardening engineered cementitious composites in design of reinforced concrete flexural members for improved durability. Struct. J. 1995, 92, 167–176. [Google Scholar]
- Şahmaran, M.; Özbay, E.; Yücel, H.E.; Lachemi, M.; Li, V.C. Frost resistance and microstructure of Engineered Cementitious Composites: Influence of fly ash and micro poly-vinyl-alcohol fiber. Cem. Concr. Compos. 2012, 34, 156–165. [Google Scholar] [CrossRef]
- Özbay, E.; Karahan, O.; Lachemi, M.; Hossain, K.M.; Atis, C.D. Dual effectiveness of freezing–thawing and sulfate attack on high-volume slag-incorporated ECC. Compos. Part B Eng. 2013, 45, 1384–1390. [Google Scholar] [CrossRef]
- Shang, X.Y.; Yu, J.T.; Li, L.Z.; Lu, Z.D. Strengthening of RC structures by using engineered cementitious composites: A review. Sustainability 2019, 11, 3384. [Google Scholar] [CrossRef]
- Li, V.C. Advances in ECC research. ACI Spec. Publ. 2002, 206, 373–400. [Google Scholar]
- Li, V.C. On engineered cementitious composites (ECC) a review of the material and its applications. J. Adv. Concr. Technol. 2003, 1, 215–230. [Google Scholar] [CrossRef]
- Lim, Y.M.; Li, V.C. Durable repair of aged infrastructures using trapping mechanism of engineered cementitious composites. Cem. Concr. Compos. 1997, 19, 373–385. [Google Scholar] [CrossRef]
- Kesner, K.; Billington, S. Investigation of ductile cement-based composites for seismic strengthening and retrofit. In Fracture Mechanics of Concrete Structures; Swets & Zeitlinger: Lisse, The Netherlands, 2001; pp. 65–72. [Google Scholar]
- Ge, W.; Zhang, J.; Cao, D.; Tu, Y. Flexural behaviors of hybrid concrete beams reinforced with BFRP bars and steel bars. Constr. Build. Mater. 2015, 87, 28–37. [Google Scholar] [CrossRef]
- Zhang, J.; Leung, C.K.; Cheung, Y.N. Flexural performance of layered ECC-concrete composite beam. Compos. Sci. Technol. 2006, 66, 1501–1512. [Google Scholar] [CrossRef]
- Yuan, F.; Pan, J.; Leung, C.K.Y. Flexural behaviors of ECC and concrete/ECC composite beams reinforced with basalt fiber-reinforced polymer. J. Compos. Constr. 2013, 17, 591–602. [Google Scholar] [CrossRef]
- Ge, W.; Ashour, A.F.; Cao, D.; Lu, W.; Gao, P.; Yu, J.; Ji, X.; Cai, C. Experimental study on flexural behavior of ECC-concrete composite beams reinforced with FRP bars. Compos. Struct. 2019, 208, 454–465. [Google Scholar] [CrossRef]
- Ge, W.J.; Ashour, A.F.; Yu, J.; Gao, P.; Cao, D.F.; Cai, C.; Ji, X. Flexural behavior of ECC–concrete hybrid composite beams reinforced with FRP and steel bars. J. Compos. Constr. 2019, 23, 04018069. [Google Scholar] [CrossRef]
- Carreira, D.J.; Chu, K.-H. Stress-strain relationship for plain concrete in compression. J. Proc. 1985, 82, 797–804. [Google Scholar]
- Aslani, F.; Jowkarmeimandi, R. Stress–strain model for concrete under cyclic loading. Mag. Concr. Res. 2012, 64, 673–685. [Google Scholar] [CrossRef]
- Elwell, D.J.; Fu, G. Compression testing of concrete: Cylinders vs. cubes, Report FHWA/NY/SR-95/119, special report 119, Transportation research and development bureau New York state department of transportation, 1995.
- Lubliner, J.; Oliver, J.; Oller, S.; Oñate, E. A plastic-damage model for concrete. Int. J. Solids Struct. 1989, 25, 299–326. [Google Scholar] [CrossRef]
- Smith, M. ABAQUS/Standard User’s Manual; Version 6.9; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2009. [Google Scholar]
- Yi, S.-T.; Yang, E.-I.; Choi, J.-C. Effect of specimen sizes, specimen shapes, and placement directions on compressive strength of concrete. Nucl. Eng. Des. 2006, 236, 115–127. [Google Scholar] [CrossRef]
- Hosen, M.A.; Alengaram, U.J.; Jumaat, M.Z.; Sulong, N.H.R.; Darain, K.M.U. Glass Fiber Reinforced Polymer (GFRP) Bars for Enhancing the Flexural Performance of RC Beams Using Side-NSM Technique. Polymers 2017, 9, 180. [Google Scholar] [CrossRef]
- Zhou, F.; Zhang, J.; Song, S.; Yang, D.; Wang, C. Effect of Temperature on Material Properties of Carbon Fiber Reinforced Polymer (CFRP) Tendons: Experiments and Model Assessment. Materials 2019, 12, 1025. [Google Scholar] [CrossRef]
- Paegle, I.; Fischer, G. Phenomenological interpretation of the shear behavior of reinforced Engineered Cementitious Composite beams. Cem. Concr. Compos. 2016, 73, 213–225. [Google Scholar] [CrossRef]
- Meng, D.; Lee, C.; Zhang, Y. Flexural and shear behaviours of plain and reinforced polyvinyl alcohol-engineered cementitious composite beams. Eng. Struct. 2017, 151, 261–272. [Google Scholar] [CrossRef]
- Ding, Y.; Yu, K.Q.; Yu, J.T.; Xu, S.L. Structural behaviors of ultra-high performance engineered cementitious composites (UHP-ECC) beams subjected to bending-experimental study. Constr. Build. Mater. 2018, 177, 102–115. [Google Scholar] [CrossRef]
- OEl-Mahdy, O.; AHamdy, G.; HEl-Diasity, M.; Shalaby, Y. Performance of Reinforced Engineered Cementitious Composite Beams. Eng. Res. J.-Fac. Eng. 2022, 51, 83–101. [Google Scholar] [CrossRef]

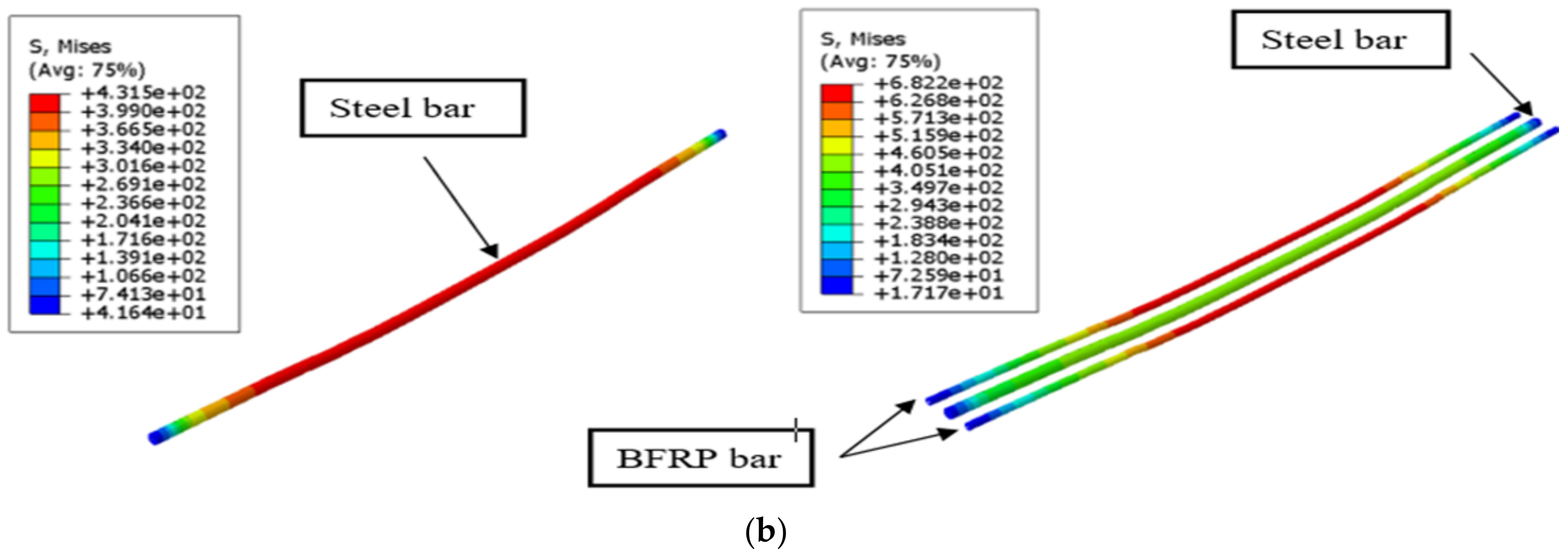
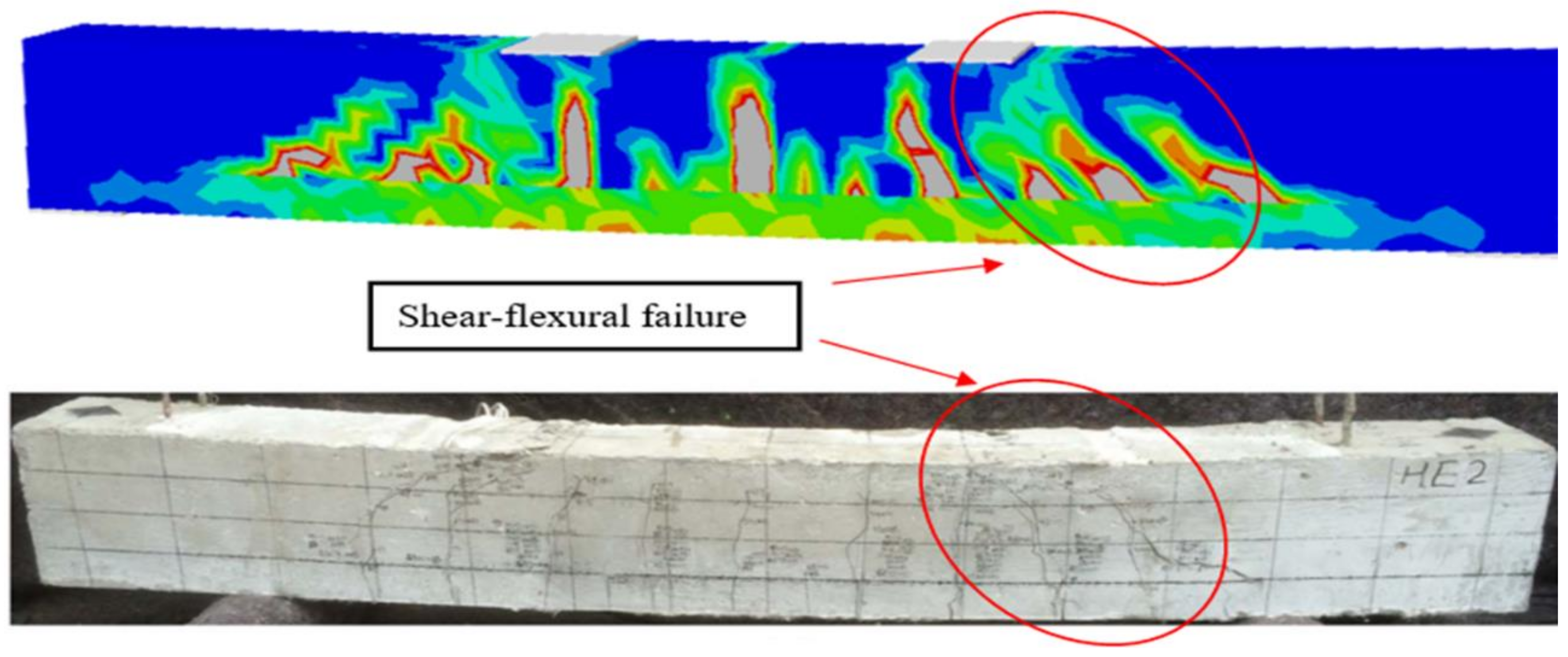
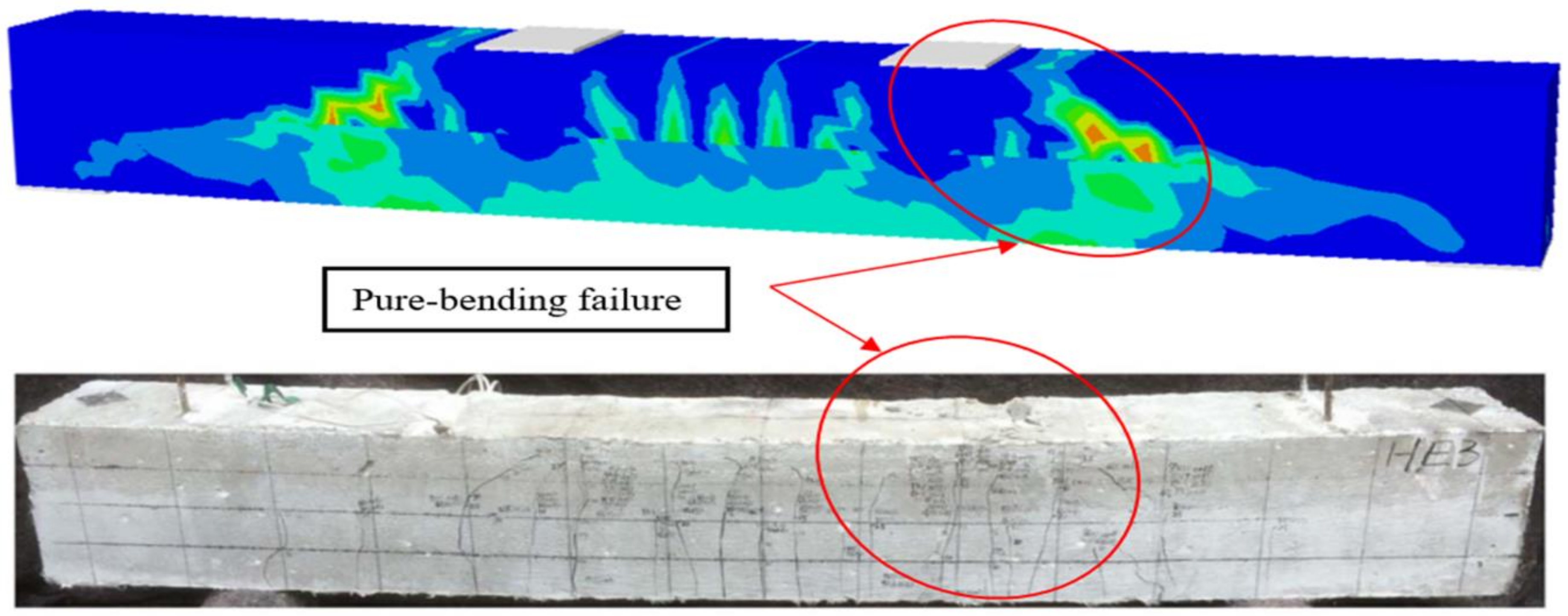
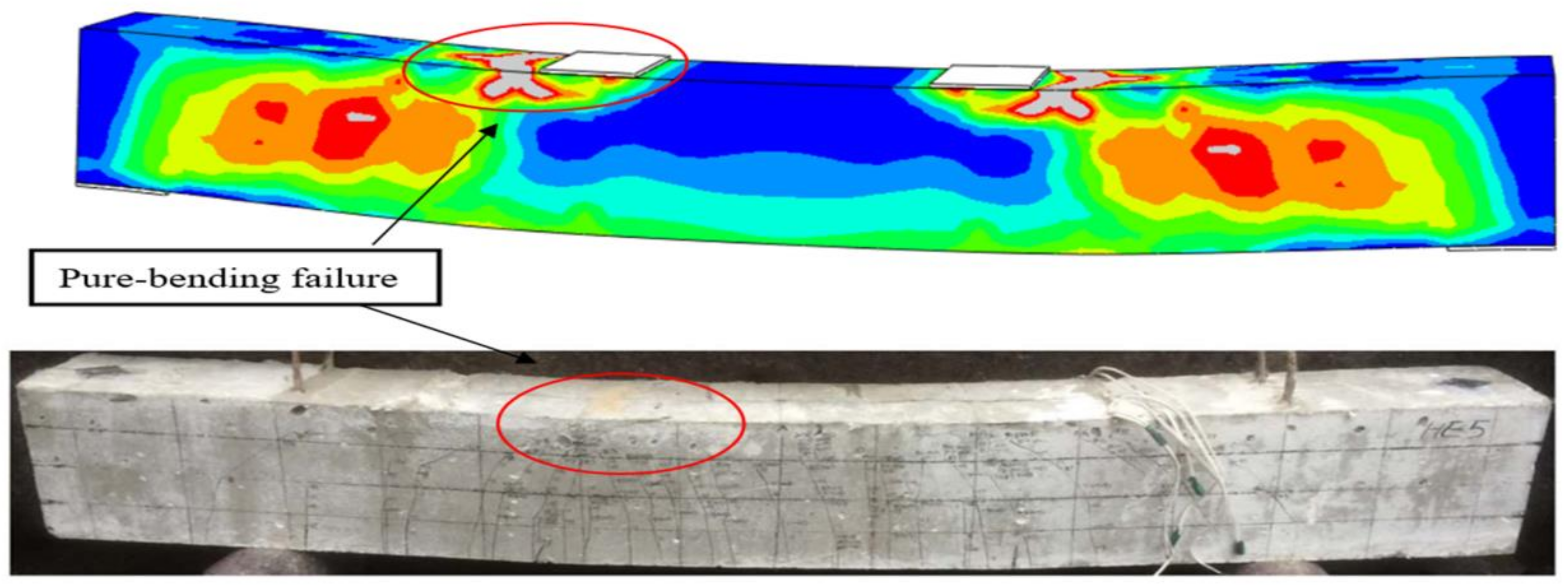


| Parameter | Value |
|---|---|
| Flow potential eccentricity (ϵ) | 0.1 |
| Dilation angle (ψ) | 30 |
| Compressive meridian at in initial yield (k) | 2/3 |
| Viscosity (μ) | 0.0005 |
| Initial biaxial compressive yield stress to initial uniaxial compressive yield stress (fb0/fc0) | 1.16 |
| Bars Type | Diameter (mm) | Elastic Modulus (GPa) | fy (MPa) | fu (MPa) | Ultimate Strain |
|---|---|---|---|---|---|
| Top steel bar | 10 | 198 | 403 | 495 | 0.051 |
| Bottom steel bar | 12 | 199 | 408 | 503 | 0.0675 |
| Stirrups | 8 | 198 | 406 | 485 | 0.075 |
| Bars Type | Ultimate Tensile Stress (MPa) | Elastic Modulus (GPa) |
|---|---|---|
| CFRP | 2070 | 156 |
| AFRP | 1172 | 83 |
| BFRP | 1250 | 50 |
| GFRP | 1080 | 40 |
| Group | Beam Notation | As (Steel) | Afrp (BFRP) | ECC Layer Thickness (mm) |
|---|---|---|---|---|
| I | B1 | 2ϕ12 (226.2 mm2) | _ | 0 |
| B2 | 2ϕ12 (226.2 mm2) | _ | 50 | |
| B3 | 2ϕ12 (226.2 mm2) | _ | 100 | |
| B4 | 2ϕ12 (226.2 mm2) | _ | 200 | |
| II | B5 | 1 ϕ12 (113.1 mm2) | 2ϕ8 (100.53 mm2) | 0 |
| B6 | 1 ϕ12 (113.1 mm2) | 2ϕ8 (100.53 mm2) | 50 | |
| B7 | 1 ϕ12 (113.1 mm2) | 2ϕ8 (100.53 mm2) | 100 | |
| B8 | 1 ϕ12 (113.1 mm2) | 2ϕ8 (100.53 mm2) | 200 | |
| III | B9 | _ | 3ϕ8 (150.80 mm2) | 0 |
| B10 | _ | 3ϕ8 (150.80 mm2) | 50 | |
| B11 | _ | 3ϕ8 (150.80 mm2) | 100 | |
| B12 | _ | 3ϕ8 (150.80 mm2) | 200 |
| Group | Beam Notation | Ultimate Deflection (mm) | Ultimate Load (kN) | ||||
|---|---|---|---|---|---|---|---|
| Experiment [22] | NLFEA | %Error | Experiment [22] | NLFEA | %Error | ||
| I | B1 | 29.5 | 32.05 | 8.64 | 38.6 | 36.98 | 4.2 |
| B2 | 22.0 | 23.09 | 4.95 | 39 | 39.83 | 2.13 | |
| B3 | 19.1 | 19.99 | 4.66 | 39.6 | 41.28 | 4.24 | |
| B4 | 33.3 | 35.15 | 5.56 | 45 | 45.68 | 1.51 | |
| II | B5 | 31.1 | 33.29 | 7.04 | 42.4 | 42.61 | 0.5 |
| B6 | 41.5 | 41.47 | 0.07 | 53.6 | 54.79 | 2.22 | |
| B7 | 29.5 | 30.89 | 4.51 | 47.2 | 45.59 | 3.41 | |
| B8 | 47.9 | 50.14 | 4.68 | 54.2 | 58.73 | 8.36 | |
| III | B9 | 31.5 | 33.41 | 6.06 | 35.6 | 38.79 | 8.96 |
| B10 | 29.7 | 31.36 | 5.59 | 40.8 | 41.39 | 1.45 | |
| B11 | 28.1 | 29.67 | 5.59 | 44.6 | 43.88 | 1.61 | |
| B12 | 35.0 | 36.92 | 5.49 | 45 | 49.0 | 8.89 | |
| Overall | 5.25 | 3.96 | |||||
| Model | fʹc (MPa) | Beam Height (mm) | ρs(%) | FRP Type | ρfrp (%) | tECC (mm) |
|---|---|---|---|---|---|---|
| M1 | 24 | 200 | 0.43 | BFRP | 0.38 | 100 |
| M2 | 24 | 200 | 0.43 | CFRP | 0.38 | 100 |
| M3 | 24 | 200 | 0.43 | AFRP | 0.38 | 100 |
| M4 | 24 | 200 | 0.43 | GFRP | 0.38 | 100 |
| M5 | 30 | 200 | 0.43 | BFRP | 0.38 | 100 |
| M6 | 30 | 200 | 0.43 | CFRP | 0.38 | 100 |
| M7 | 30 | 200 | 0.43 | AFRP | 0.38 | 100 |
| M8 | 30 | 200 | 0.43 | GFRP | 0.38 | 100 |
| M9 | 40 | 200 | 0.43 | BFRP | 0.38 | 100 |
| M10 | 40 | 200 | 0.43 | CFRP | 0.38 | 100 |
| M11 | 40 | 200 | 0.43 | AFRP | 0.38 | 100 |
| M12 | 40 | 200 | 0.43 | GFRP | 0.38 | 100 |
| M13 | 24 | 250 | 0.43 | BFRP | 0.38 | 100 |
| M14 | 24 | 300 | 0.43 | BFRP | 0.38 | 100 |
| M15 | 24 | 200 | 0.19 | BFRP | 0.38 | 50 |
| M16 | 24 | 200 | 0.19 | BFRP | 0.86 | 50 |
| M17 | 24 | 200 | 0.19 | BFRP | 1.53 | 50 |
| M18 | 24 | 200 | 0.43 | BFRP | 0.38 | 50 |
| M19 | 24 | 200 | 0.43 | BFRP | 0.86 | 50 |
| M20 | 24 | 200 | 0.43 | BFRP | 1.53 | 50 |
| M21 | 24 | 200 | 0.77 | BFRP | 0.38 | 50 |
| M22 | 24 | 200 | 0.77 | BFRP | 0.86 | 50 |
| M23 | 24 | 200 | 0.77 | BFRP | 1.53 | 50 |
| M24 | 24 | 200 | 1.22 | BFRP | 0.38 | 50 |
| M25 | 24 | 200 | 1.22 | BFRP | 0.86 | 50 |
| M26 | 24 | 200 | 1.22 | BFRP | 1.53 | 50 |
| M27 | 24 | 200 | 0.19 | BFRP | 0.38 | 100 |
| M28 | 24 | 200 | 0.19 | BFRP | 0.86 | 100 |
| M29 | 24 | 200 | 0.19 | BFRP | 1.53 | 100 |
| M30 | 24 | 200 | 0.43 | BFRP | 0.86 | 100 |
| M31 | 24 | 200 | 0.43 | BFRP | 1.53 | 100 |
| M32 | 24 | 200 | 0.77 | BFRP | 0.38 | 100 |
| M33 | 24 | 200 | 0.77 | BFPR | 0.86 | 100 |
| M34 | 24 | 200 | 0.77 | BFPR | 1.53 | 100 |
| M35 | 24 | 200 | 1.22 | BFPR | 0.38 | 100 |
| M36 | 24 | 200 | 1.22 | BFPR | 0.86 | 100 |
| M37 | 24 | 200 | 1.22 | BFPR | 1.53 | 100 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shbeeb, N.I.; Barham, W.S.; Alyahya, W. Numerical Simulation of Engineering Cementitious Composite Beams Strengthened with Fiber-Reinforced Polymer and Steel Bars. Fibers 2024, 12, 49. https://doi.org/10.3390/fib12060049
Shbeeb NI, Barham WS, Alyahya W. Numerical Simulation of Engineering Cementitious Composite Beams Strengthened with Fiber-Reinforced Polymer and Steel Bars. Fibers. 2024; 12(6):49. https://doi.org/10.3390/fib12060049
Chicago/Turabian StyleShbeeb, Nadim I., Wasim S. Barham, and Wala’a Alyahya. 2024. "Numerical Simulation of Engineering Cementitious Composite Beams Strengthened with Fiber-Reinforced Polymer and Steel Bars" Fibers 12, no. 6: 49. https://doi.org/10.3390/fib12060049
APA StyleShbeeb, N. I., Barham, W. S., & Alyahya, W. (2024). Numerical Simulation of Engineering Cementitious Composite Beams Strengthened with Fiber-Reinforced Polymer and Steel Bars. Fibers, 12(6), 49. https://doi.org/10.3390/fib12060049