CFD Comparison of the Influence of Casting of Samples on the Fiber Orientation Distribution
Abstract
1. Introduction
2. Materials and Methods
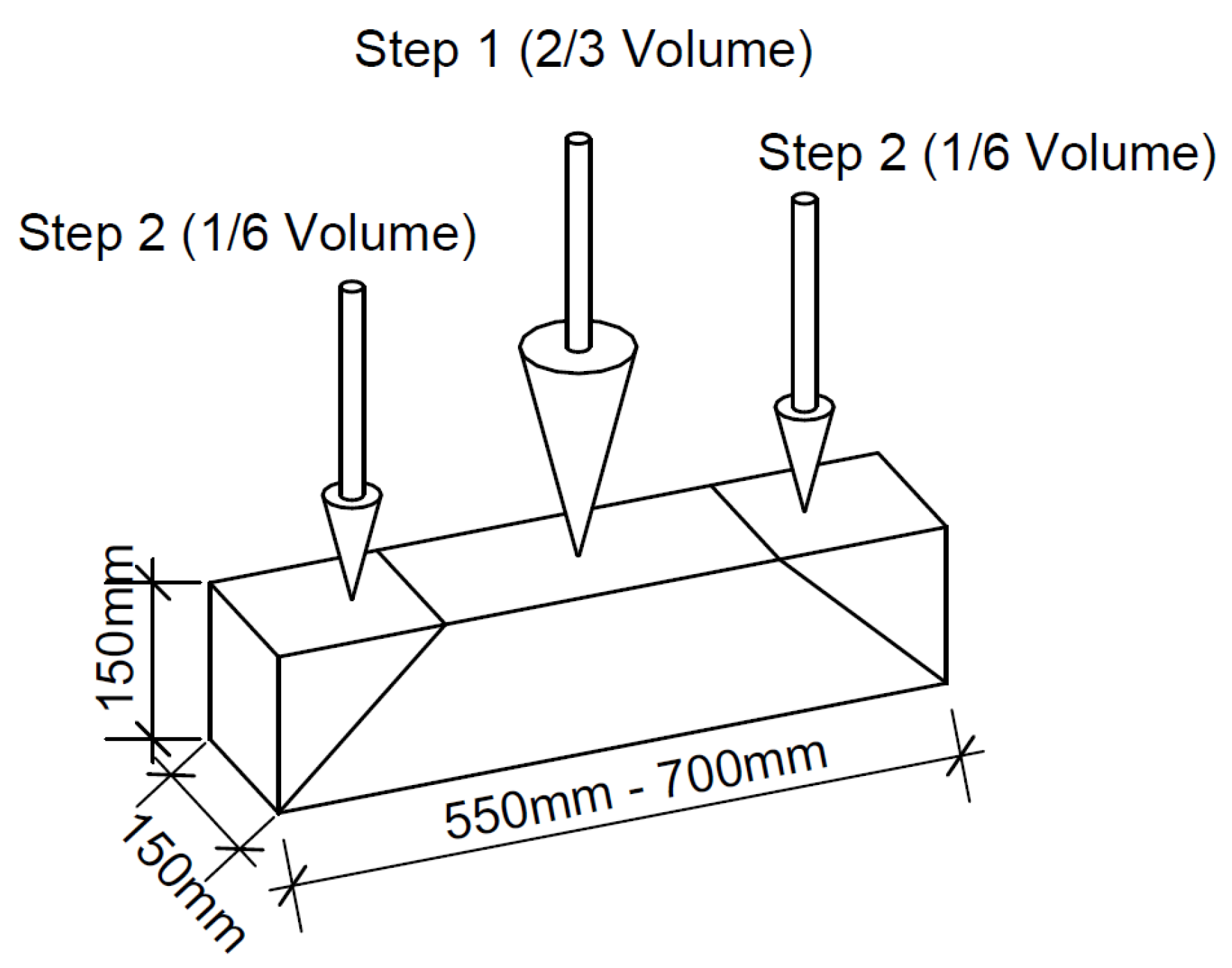
2.1. Sample Preparation and Testing According to EN 14651
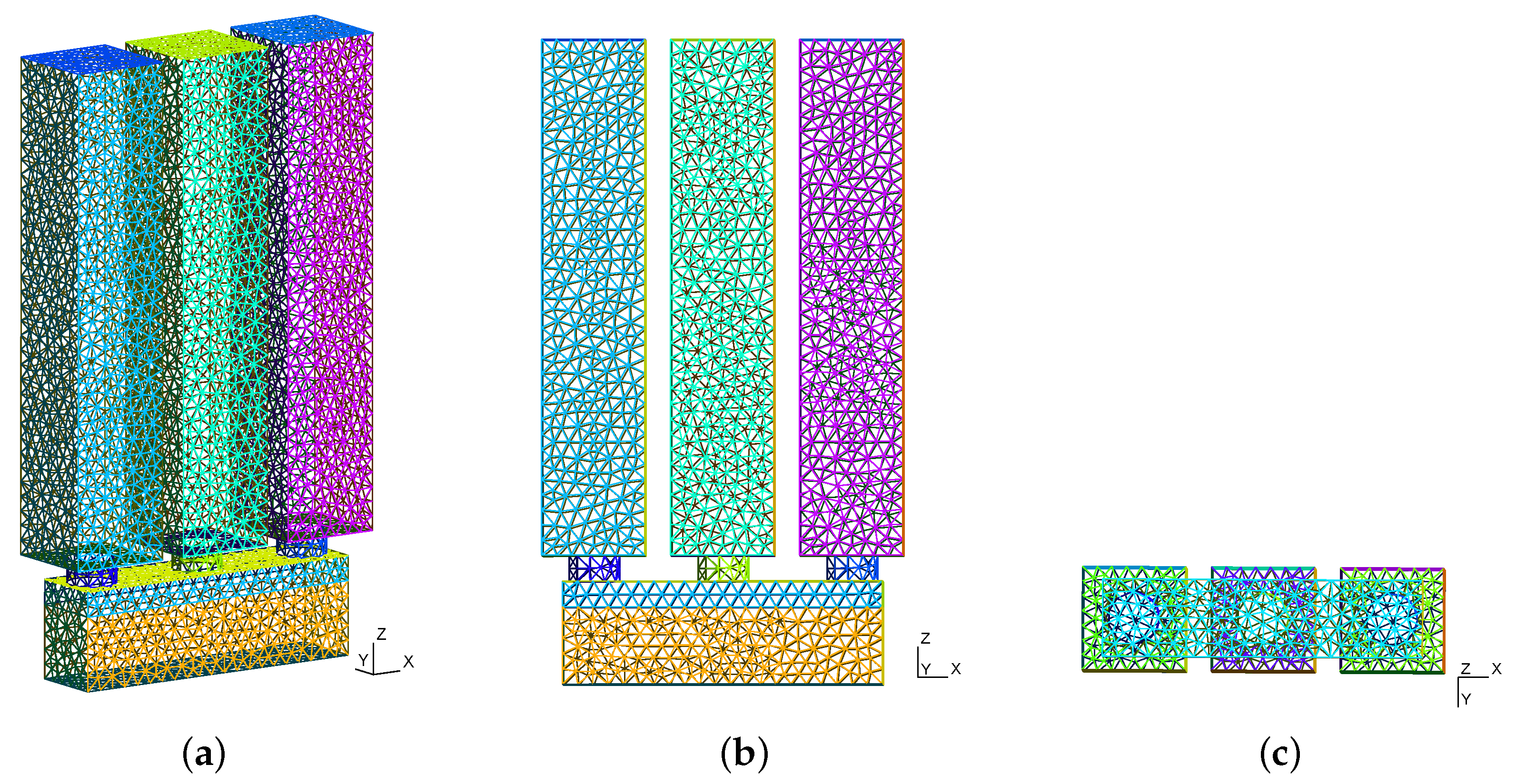
2.2. Computational Fluid Dynamics Simulations
3. Results
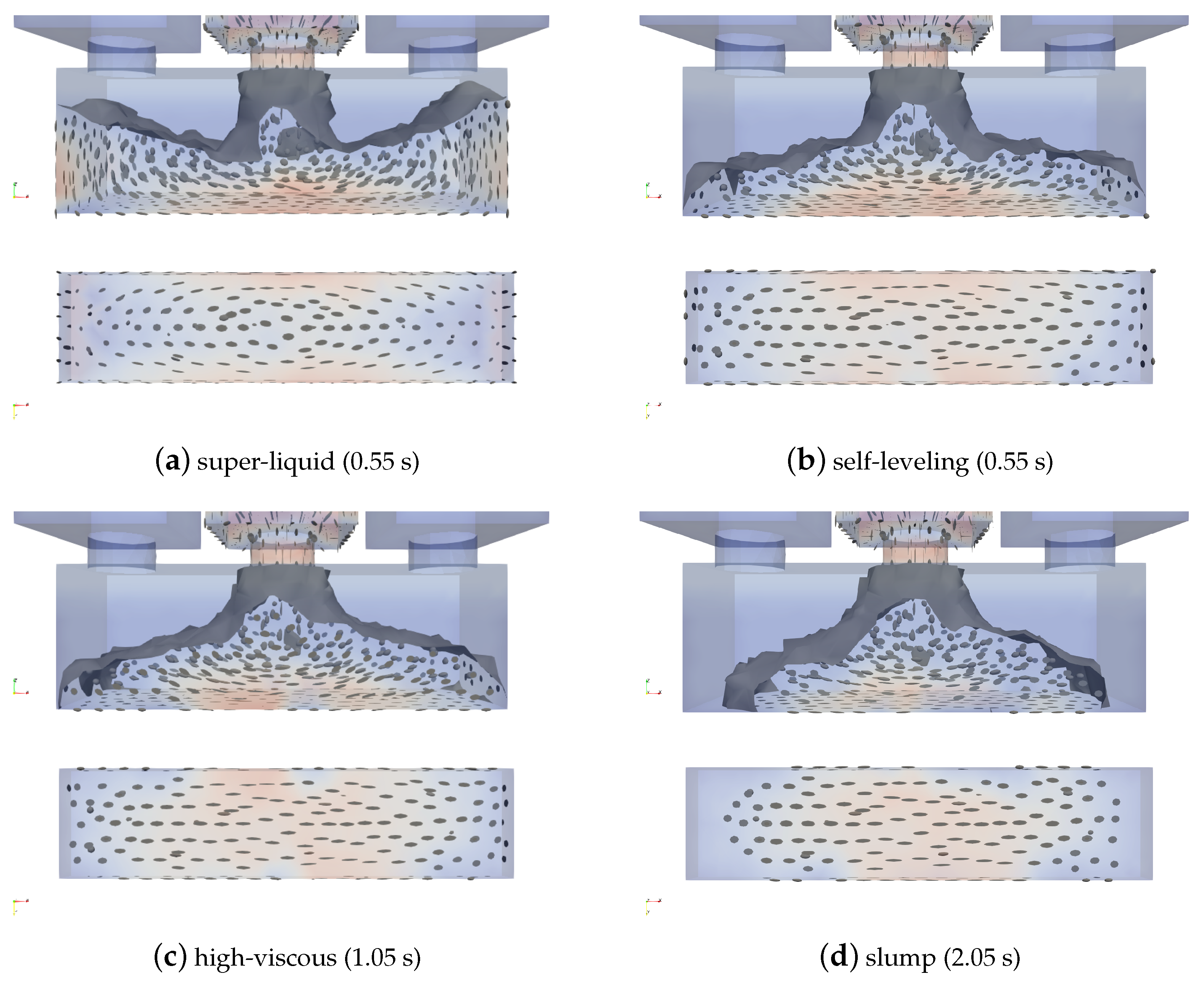
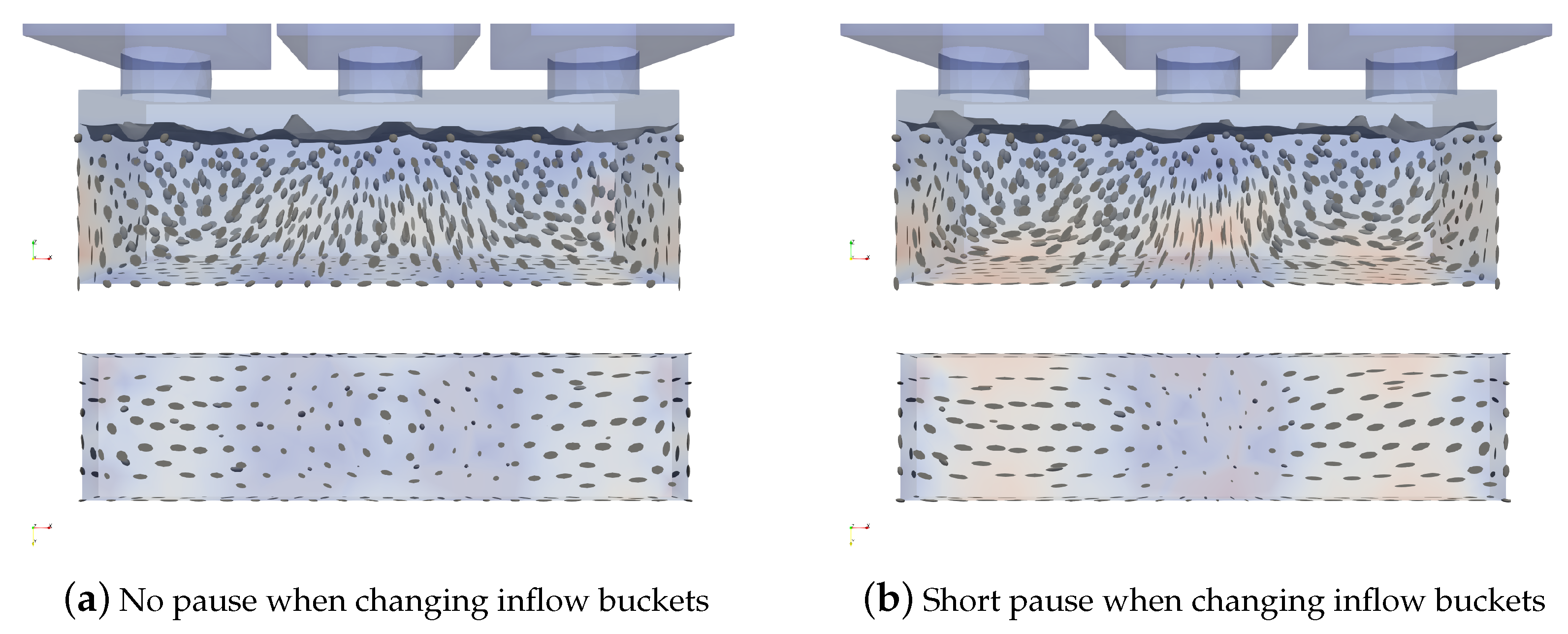
3.1. Dynamics of Filling the form
3.1.1. Super Liquid
3.1.2. Self-Leveling
3.1.3. High Viscous
3.1.4. Slump
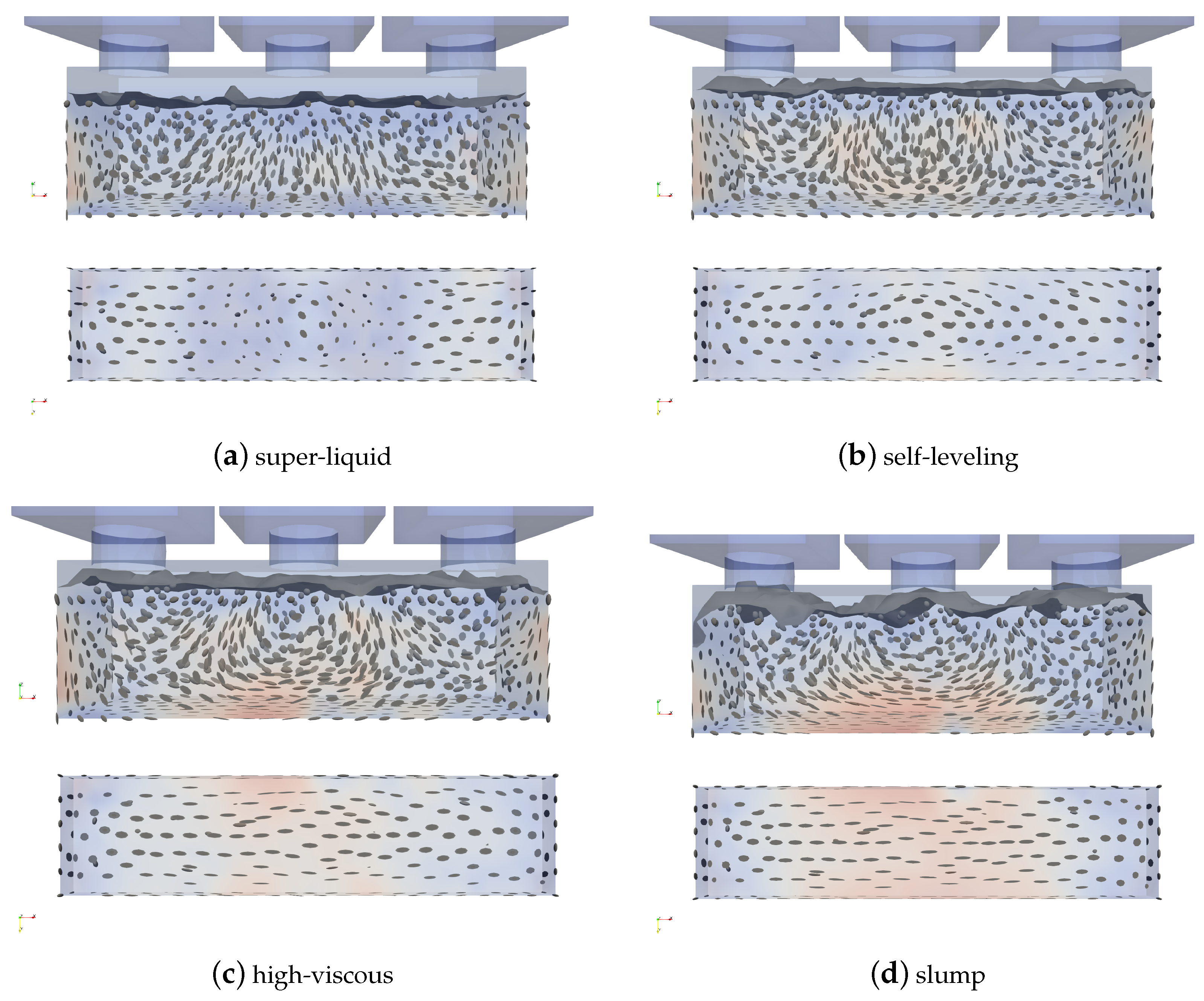
3.2. Obtained Final Fiber Orientation Distributions
3.2.1. Super-Liquid
3.2.2. Self-Leveling
3.2.3. High Viscous
3.2.4. Slump
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CFD | Computational fluid dynamics |
| FVE | Finite-volume elements |
| SFRC | Short-fiber reinforced concrete |
| VOF | Volume of fluid |
| IBOF | Invariant-based optimal fitting |
References
- Roussel, N. Rheology of fresh concrete: From measurements to predictions of casting processes. Mater. Struct. 2007, 40, 1001–1012. [Google Scholar] [CrossRef]
- Hu, J.; Wang, K. Effect of coarse aggregate characteristics on concrete rheology. Constr. Build. Mater. 2011, 25, 1196–1204. [Google Scholar] [CrossRef]
- Kostrzanowska-Siedlarz, A.; Gołaszewski, J. Rheological properties of high performance self-compacting concrete: Effects of composition and time. Constr. Build. Mater. 2016, 115, 705–715. [Google Scholar] [CrossRef]
- Jiao, D.; Shi, C.; Yuan, Q.; An, X.; Liu, Y.; Li, H. Effect of constituents on rheological properties of fresh concrete-A review. Cem. Concr. Compos. 2017, 83, 146–159. [Google Scholar] [CrossRef]
- Boulekbache, B.; Hamrat, M.; Chemrouk, M.; Amziane, S. Flowability of fibre-reinforced concrete and its effect on the mechanical properties of the material. Constr. Build. Mater. 2010, 24, 1664–1671. [Google Scholar] [CrossRef]
- Ponikiewski, T.; Gołaszewski, J. Properties of Steel Fibre Reinforced Self-compacting Concrete for Optimal Rheological and Mechanical Properties in Precast Beams. Procedia Eng. 2013, 65, 290–295. [Google Scholar] [CrossRef]
- Khaloo, A.; Raisi, E.M.; Hosseini, P.; Tahsiri, H. Mechanical performance of self-compacting concrete reinforced with steel fibers. Constr. Build. Mater. 2014, 51, 179–186. [Google Scholar] [CrossRef]
- Torrijos, M.; Tobes, J.; Barragán, B.; Zerbino, R. Orientation and distribution of steel fibres in self-compacting concrete. In Proceedings of the 7th RILEM Symposium on Fiber Reinforced Concrete: Design and Applications (BEFIB 2008), Chennai, India, 17–19 September 2008; p. 729e38. [Google Scholar]
- Torrijos, M.C.; Barragán, B.E.; Zerbino, R.L. Placing conditions, mesostructural characteristics and post-cracking response of fibre reinforced self-compacting concretes. Constr. Build. Mater. 2010, 24, 1078–1085. [Google Scholar] [CrossRef]
- Ponikiewski, T.; Katzer, J. X-ray computed tomography of fibre reinforced self-compacting concrete as a tool of assessing its flexural behaviour. Mater. Struct. 2016, 49, 2131–2140. [Google Scholar] [CrossRef]
- Sucharda, O.; Lehner, P.; Konečnỳ, P.; Ponikiewski, T. Investigation of fracture properties by inverse analysis on selected SCC concrete beams with different amount of fibres. Procedia Struct. Integr. 2018, 13, 1533–1538. [Google Scholar] [CrossRef]
- Vicente, M.A.; Mínguez, J.; González, D.C. Computed tomography scanning of the internal microstructure, crack mechanisms, and structural behavior of fiber-reinforced concrete under static and cyclic bending tests. Int. J. Fatigue 2019, 121, 9–19. [Google Scholar] [CrossRef]
- Voutetaki, M.E.; Naoum, M.C.; Papadopoulos, N.A.; Chalioris, C.E. Cracking Diagnosis in Fiber-Reinforced Concrete with Synthetic Fibers Using Piezoelectric Transducers. Fibers 2022, 10, 5. [Google Scholar] [CrossRef]
- Barnett, S.J.; Lataste, J.F.; Parry, T.; Millard, S.G.; Soutsos, M.N. Assessment of fibre orientation in ultra high performance fibre reinforced concrete and its effect on flexural strength. Mater. Struct. 2010, 43, 1009–1023. [Google Scholar] [CrossRef]
- Ferrara, L.; Ozyurt, N.; Di Prisco, M. High mechanical performance of fibre reinforced cementitious composites: The role of “casting-flow induced” fibre orientation. Mater. Struct. 2011, 44, 109–128. [Google Scholar] [CrossRef]
- Zhou, B.; Uchida, Y. Fiber orientation in ultra high performance fiber reinforced concrete and its visualization. In Proceedings of the Eighth International Conference on Fracture Mechanics of Concrete and Concrete Structures, Toledo, Spain, 10–14 March 2013. [Google Scholar]
- Zhou, B.; Uchida, Y. Influence of flowability, casting time and formwork geometry on fiber orientation and mechanical properties of UHPFRC. Cem. Concr. Res. 2017, 95, 164–177. [Google Scholar] [CrossRef]
- Yoo, D.Y.; Kang, S.T.; Yoon, Y.S. Effect of fiber length and placement method on flexural behavior, tension-softening curve, and fiber distribution characteristics of UHPFRC. Constr. Build. Mater. 2014, 64, 67–81. [Google Scholar] [CrossRef]
- EN 14651; Test Method for Metallic Fibrered Concrete—Measuring the Flexural Tensile Strength (Limit of Proportionality (LOP), Residual). European Committee for Standardization: Brussels, Belgium, 2005.
- Herrmann, H.; Eik, M.; Berg, V.; Puttonen, J. Phenomenological and numerical modelling of short fibre reinforced cementitious composites. Meccanica 2014, 49, 1985–2000. [Google Scholar] [CrossRef]
- Eik, M.; Puttonen, J.; Herrmann, H. An orthotropic material model for steel fibre reinforced concrete based on the orientation distribution of fibres. Compos. Struct. 2015, 121, 324–336. [Google Scholar] [CrossRef]
- Herrmann, H. Chapter: An Improved Constitutive Model for Short Fibre Reinforced Cementitious Composites (SFRC) Based on the Orientation Tensor. In Generalized Continua as Models for Classical and Advanced Materials; Springer International Publishing: Cham, Switzerland, 2016; pp. 213–227. [Google Scholar] [CrossRef]
- Mishurova, T.; Rachmatulin, N.; Fontana, P.; Oesch, T.; Bruno, G.; Radi, E.; Sevostianov, I. Evaluation of the probability density of inhomogeneous fiber orientations by computed tomography and its application to the calculation of the effective properties of a fiber-reinforced composite. Int. J. Eng. Sci. 2018, 122, 14–29. [Google Scholar] [CrossRef]
- Herrmann, H. A constitutive model for linear hyperelastic materials with orthotropic inclusions by use of quaternions. Contin. Mech. Thermodyn. 2021, 33, 1375–1384. [Google Scholar] [CrossRef]
- Kartofelev, D.; Goidyk, O.; Herrmann, H. A case study on the spatial variability of strength in a SFRSCC slab and its correlation with fibre orientation. Proc. Est. Acad. Sci. 2020, 69, 298–310. [Google Scholar] [CrossRef]
- Gram, A. Modelling Bingham Suspensional Flow. Ph.D. Thesis, Royal Institute of Technology (KTH), Stockholm, Sweden, 2015. [Google Scholar]
- Herrmann, H.; Lees, A. On the Influence of the Rheological Boundary Conditions on the Fibre Orientations in the Production of Steel Fibre Reinforced Concrete Elements. Proc. Est. Acad. Sci. 2016, 65, 408–413. [Google Scholar] [CrossRef]
- Eiduks, M.; Krasnikovs, A.; Dunskis, E.; Kononova, O. Investigation of Fiber Orientation in Viscous Fluid. Sci. J. Riga Tech. Univ. 2010, 33, 98–102. [Google Scholar]
- Kononova, O.; Krasnikovs, A.; Lapsa, V.; Kalinka, J.; Galushchak, A. Internal Structure Formation in High Strength Fiber Concrete during Casting. Int. J. Mech. Aerosp. Ind. Mechatron. Manuf. Eng. 2011, 5, 2489–2492. [Google Scholar]
- Roussel, N. Correlation between yield stress and slump: Comparison between numerical simulations and concrete rheometers results. Mater. Struct. 2006, 39, 501–509. [Google Scholar] [CrossRef]
- Thrane, L.N.; Szabo, P.; Geiker, M.; Glavind, M.; Stang, H. Simulation of the test method “L-Box” for self-compacting concrete. Annu. Trans. NORDIC Rheol. Soc. 2004, 12, 47–54. [Google Scholar]
- Krenzer, K.; Mechtcherine, V.; Palzer, U. Simulating mixing processes of fresh concrete using the discrete element method (DEM) under consideration of water addition and changes in moisture distribution. Cem. Concr. Res. 2019, 115, 274–282. [Google Scholar] [CrossRef]
- Wallevik, J.E.; Wallevik, O.H. Concrete mixing truck as a rheometer. Cem. Concr. Res. 2020, 127, 105930. [Google Scholar] [CrossRef]
- Vasilic, K.; Schmidt, W.; Kühne, H.C.; Haamkens, F.; Mechtcherine, V.; Roussel, N. Flow of fresh concrete through reinforced elements: Experimental validation of the porous analogy numerical method. Cem. Concr. Res. 2016, 88, 1–6. [Google Scholar] [CrossRef]
- Marcalikova, Z.; Bujdos, D.; Cajka, R. Approach to numerical modelling of fiber reinforced concrete. Procedia Struct. Integr. 2020, 25, 27–32. [Google Scholar] [CrossRef]
- Vasilic, K.; Gram, A.; Wallevik, J.E. Numerical simulation of fresh concrete flow: Insight and challenges. RILEM Tech. Lett. 2019, 4, 57–66. [Google Scholar] [CrossRef]
- Roussel, N.; Spangenberg, J.; Wallevik, J.; Wolfs, R. Numerical simulations of concrete processing: From standard formative casting to additive manufacturing. Cem. Concr. Res. 2020, 135, 106075. [Google Scholar] [CrossRef]
- Roussel, N.; Gram, A.; Cremonesi, M.; Ferrara, L.; Krenzer, K.; Mechtcherine, V.; Shyshko, S.; Skocec, J.; Spangenberg, J.; Svec, O.; et al. Numerical simulations of concrete flow: A benchmark comparison. Cem. Concr. Res. 2016, 79, 265–271. [Google Scholar] [CrossRef]
- Kulasegaram, S.; Karihaloo, B.L.; Ghanbari, A. Modelling the flow of self-compacting concrete. Int. J. Numer. Anal. Methods Geomech. 2011, 35, 713–723. [Google Scholar] [CrossRef]
- Oesch, T.S. Investigation of Fiber and Cracking Behavior for Conventional and Ultra-High Performance Concretes Using X-ray Computed Tomography. Ph.D. Thesis, University of Illinois at Urbana, Champaign, IL, USA, 2015. [Google Scholar]
- di Prisco, M.; Ferrara, L.; Lamperti, M.G.L. Double edge wedge splitting (DEWS): An indirect tension test to identify post-cracking behaviour of fibre reinforced cementitious composites. Mater. Struct. 2013, 46, 1893–1918. [Google Scholar] [CrossRef]
- Pujadas, P.; Blanco, A.; Cavalaro, S.; de la Fuente, A.; Aguado, A. Fibre distribution in macro-plastic fibre reinforced concrete slab-panels. Constr. Build. Mater. 2014, 64, 496–503. [Google Scholar] [CrossRef]
- Herrmann, H.; Pastorelli, E.; Kallonen, A.; Suuronen, J.P. Methods for Fibre Orientation Analysis of X-ray Tomography Images of Steel Fibre Reinforced Concrete (SFRC). J. Mater. Sci. 2016, 51, 3772–3783. [Google Scholar] [CrossRef]
- Banthia, N.; Bindiganavile, V.; Jones, J.; Novak, J. Fiber-reinforced concrete in precast concrete applications: Research leads to innovative products. PCI J. 2012, 57, 33–46. [Google Scholar] [CrossRef]
- Hess, S.; Köhler, W. Formeln zur Tensor-Rechnung; Palm & Enke: Erlangen, Germany, 1980. [Google Scholar]
- Ehrentraut, H.; Muschik, W. On Symmetric irreducible tensors in d-dimensions. ARI-Int. J. Phys. Eng. Sci. 1998, 51, 149–159. [Google Scholar] [CrossRef]
- Herrmann, H.; Beddig, M. Tensor series expansion of a spherical function for use in constitutive theory of materials containing orientable particles. Proc. Est. Acad. Sci. 2018, 67, 73–92. [Google Scholar] [CrossRef]
- Advani, S.G.; Tucker, C.L., III. The Use of Tensors to Describe and Predict Fiber Orientation in Short Fiber Composites. J. Rheol. 1987, 31, 751–784. [Google Scholar] [CrossRef]
- Heinen, K. Mikrostrukturelle Orientierungszustände Strömender Polymerlösungen und Fasersuspensionen. Ph.D. Thesis, Universität Dortmund, Dortmund, Germany, 2007. [Google Scholar]
- Folgar, F.; Tucker, C.L. Orientation Behavior of Fibers in Concentrated Suspensions. J. Reinf. Plast. Compos. 1984, 3, 98–119. [Google Scholar] [CrossRef]
- Chung, D.H.; Kwon, T.H. Invariant-based optimal fitting closure approximation for the numerical prediction of flow-induced fiber orientation. J. Rheol. 2002, 46, 169–194. [Google Scholar] [CrossRef]
- The OpenFOAM Foundation. OpenFOAM. v.2.3.0. 2014. Available online: http://www.openfoam.org (accessed on 5 March 2022).
- Damián, S.M. An Extended Mixture Model for the Simultaneous Treatment of Short and Long Scale Interfaces. Ph.D. Thesis, Universidad Nacional del Litoral, Santa Fe, Argentina, 2013. [Google Scholar]
- Geuzaine, C.; Remacle, J.F. Gmsh: A 3-D finite element mesh generator with built-in pre- and post-processing facilities. Int. J. Numer. Methods Eng. 2009, 79, 1309–1331. [Google Scholar] [CrossRef]
- Henderson, A.; Ahrens, J.; Law, C. The ParaView Guide; Kitware Inc.: Clifton Park, NY, USA, 2004. [Google Scholar]
- de Larrard, F.; Ferraris, C.F.; Sedran, T. Fresh concrete: A Herschel-Bulkley material. Mater. Struct. 1998, 31, 494–498. [Google Scholar] [CrossRef]
- Lashkarbolouk, H.; Halabian, A.M.; Chamani, M.R. Simulation of concrete flow in V-funnel test and the proper range of viscosity and yield stress for SCC. Mater. Struct. 2014, 47, 1729–1743. [Google Scholar] [CrossRef]
- Herrmann, H.; Eik, M. Some comments on the theory of short fibre reinforced material. Proc. Est. Acad. Sci. 2011, 60, 179–183. [Google Scholar] [CrossRef]
- Jankun-Kelly, T.J.; Mehta, K. Superellipsoid-based, real symmetric traceless tensor glyphs motivated by nematic liquid crystal alignment visualization. IEEE Trans. Vis. Comput. Graph. 2006, 12, 1197–1204. [Google Scholar] [CrossRef]


| Case | OpenFOAM Value | Physical Value | |
|---|---|---|---|
| all cases | 2300 | 2300 | |
| all cases | 1000 | 1000 | |
| n | all cases | 1 | 1 |
| super-liquid | 0.017391 | 40 Pa | |
| self-leveling | 0.0347 | 79.810 Pa | |
| high-viscous | 0.0547 | 125.81 Pa | |
| slump | 0.0947 | 217.81 Pa | |
| super-liquid | 0.010870 | 25 Pa·sn | |
| self-leveling | 0.0217 | 49.910 Pa·sn | |
| high-viscous | 0.0417 | 95.910 Pa·sn | |
| slump | 0.0917 | 210.91 Pa·sn |
| Case | Funnel Outflow Time | Flow Table Diameter |
|---|---|---|
| super-liquid | 1 s | – |
| self-leveling | 2.5–3.2 s | 108 cm |
| high-viscous | 5–7 s | 78 cm |
| slump | 16 s | 62 cm |
| According to EN Standard | Only Center Casting | Only Edge Casting | |
|---|---|---|---|
| super-liquid |  |  |  |
| self-leveling |  | 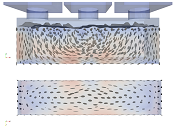 |  |
| high-viscous | 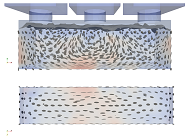 | 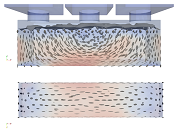 | 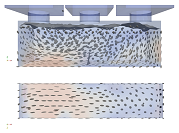 |
| slump |  | 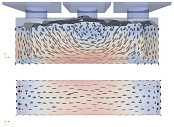 | 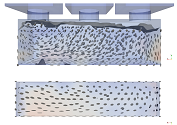 |
 | |||
| Cast Position | Flowability | Position | S | b | EV() |
|---|---|---|---|---|---|
| Norm | super-liquid | bottom 1 | 0.09 | 0.03 | (−0.41, −0.91, −0.061) |
| Norm | super-liquid | side 2 | 0.44 | 0.02 | (−0.015, 0.24, 0.97) |
| Norm rest | super-liquid | bottom 1 | 0.36 | 0.05 | (−0.099, 0.099, −0.99) |
| Norm rest | super-liquid | side 2 | 0.53 | 0.03 | (0.001, 0.17, 0.98) |
| Center | super-liquid | bottom 1 | −0.12 | 0.03 | (0.208, 0.007, 0.98) |
| Center | super-liquid | side 2 | 0.42 | 0.06 | (0.044, 0.049, 0.99) |
| Side | super-liquid | bottom 1 | −0.19 | 0.04 | (−0.75, −0.075, 0.65) |
| Side | super-liquid | side 2 | 0.37 | 0.11 | (0.21, 0.085, 0.97) |
| Norm | self-leveling | bottom 1 | −0.25 | 0.08 | (0.045, 0.042, −0.99) |
| Norm | self-leveling | side 2 | 0.38 | 0.09 | (−0.26, −0.101, −0.96) |
| Center | self-leveling | bottom 1 | 0.37 | 0.10 | (0.99, 0.15, 0.034) |
| Center | self-leveling | side 2 | −0.33 | 0.10 | (0.078, −0.99, 0.15) |
| Side | self-leveling | bottom 1 | 0.38 | 0.08 | (0.98, 0.031, 0.18) |
| Side | self-leveling | side 2 | −0.37 | 0.12 | (0.19, 0.96, −0.22) |
| Norm | high-viscous | bottom 1 | 0.35 | 0.07 | (0.99, −0.002, 0.007) |
| Norm | high-viscous | side 2 | 0.37 | 0.10 | (−0.17, −0.14, −0.97) |
| Center | high-viscous | bottom 1 | 0.63 | 0.05 | (0.99, 0.05, −0.008) |
| Center | high-viscous | side 2 | 0.42 | 0.10 | (0.99, 0.05, 0.073) |
| Side | high-viscous | bottom 1 | 0.51 | 0.05 | (0.99, 0.074, 0.11) |
| Side | high-viscous | side 2 | 0.41 | 0.09 | (0.84, −0.045, 0.54) |
| Norm | slump | bottom 1 | 0.59 | 0.05 | (0.99, 0.007, −0.009) |
| Norm | slump | side 2 | −0.28 | 0.09 | (0.024, −0.96, 0.27) |
| Center | slump | bottom 1 | 0.65 | 0.04 | (1.0, 0.01, −0.01) |
| Center | slump | side 2 | 0.40 | 0.07 | (0.99, −0.04, −0.12) |
| Side | slump | bottom 1 | 0.38 | 0.08 | (0.98, 0.007, −0.19) |
| Side | slump | side 2 | 0.29 | 0.09 | (0.83, 0.054, −0.56) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Goidyk, O.; Heinštein, M.; Herrmann, H. CFD Comparison of the Influence of Casting of Samples on the Fiber Orientation Distribution. Fibers 2023, 11, 6. https://doi.org/10.3390/fib11010006
Goidyk O, Heinštein M, Herrmann H. CFD Comparison of the Influence of Casting of Samples on the Fiber Orientation Distribution. Fibers. 2023; 11(1):6. https://doi.org/10.3390/fib11010006
Chicago/Turabian StyleGoidyk, Oksana, Mark Heinštein, and Heiko Herrmann. 2023. "CFD Comparison of the Influence of Casting of Samples on the Fiber Orientation Distribution" Fibers 11, no. 1: 6. https://doi.org/10.3390/fib11010006
APA StyleGoidyk, O., Heinštein, M., & Herrmann, H. (2023). CFD Comparison of the Influence of Casting of Samples on the Fiber Orientation Distribution. Fibers, 11(1), 6. https://doi.org/10.3390/fib11010006







