Experiment Study of Rapid Laser Polishing of Freeform Steel Surface by Dual-Beam
Abstract
1. Introduction
2. CW Laser Polishing
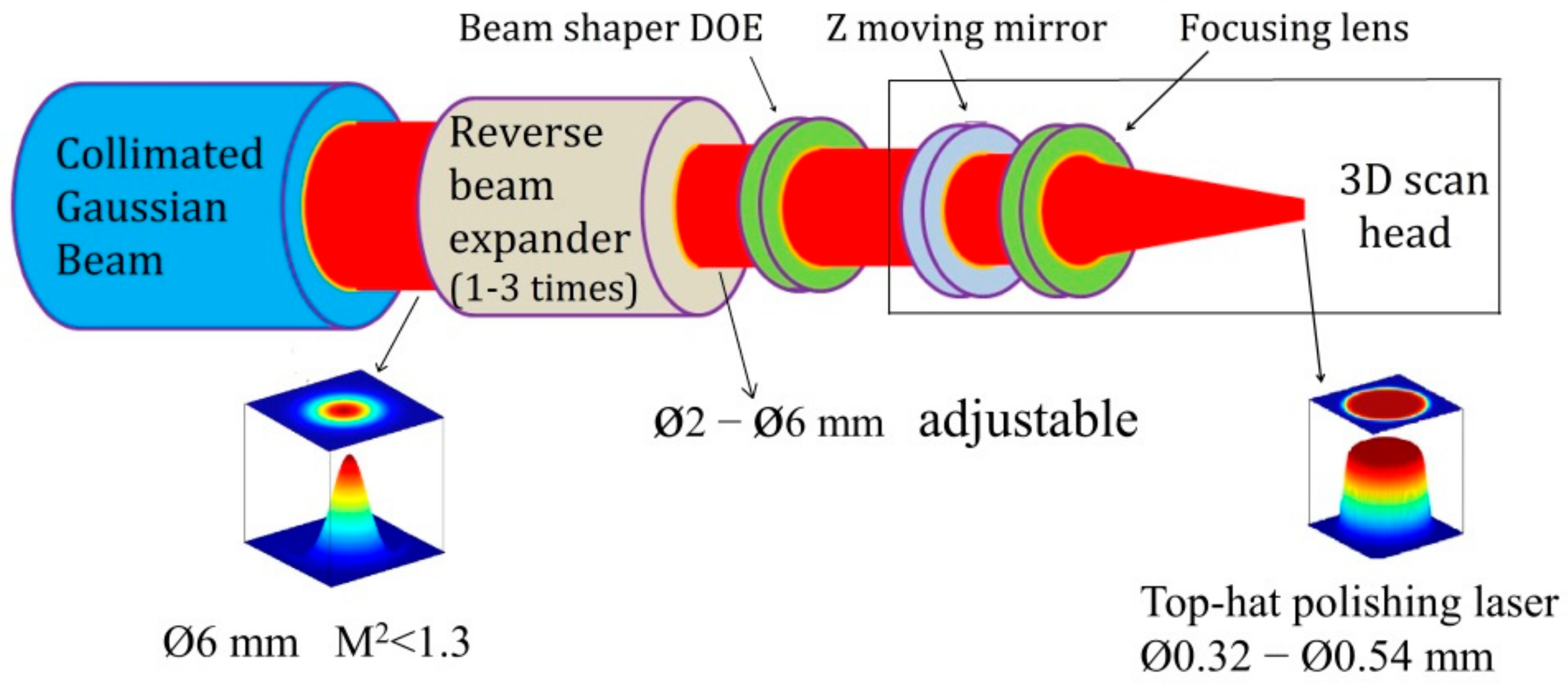
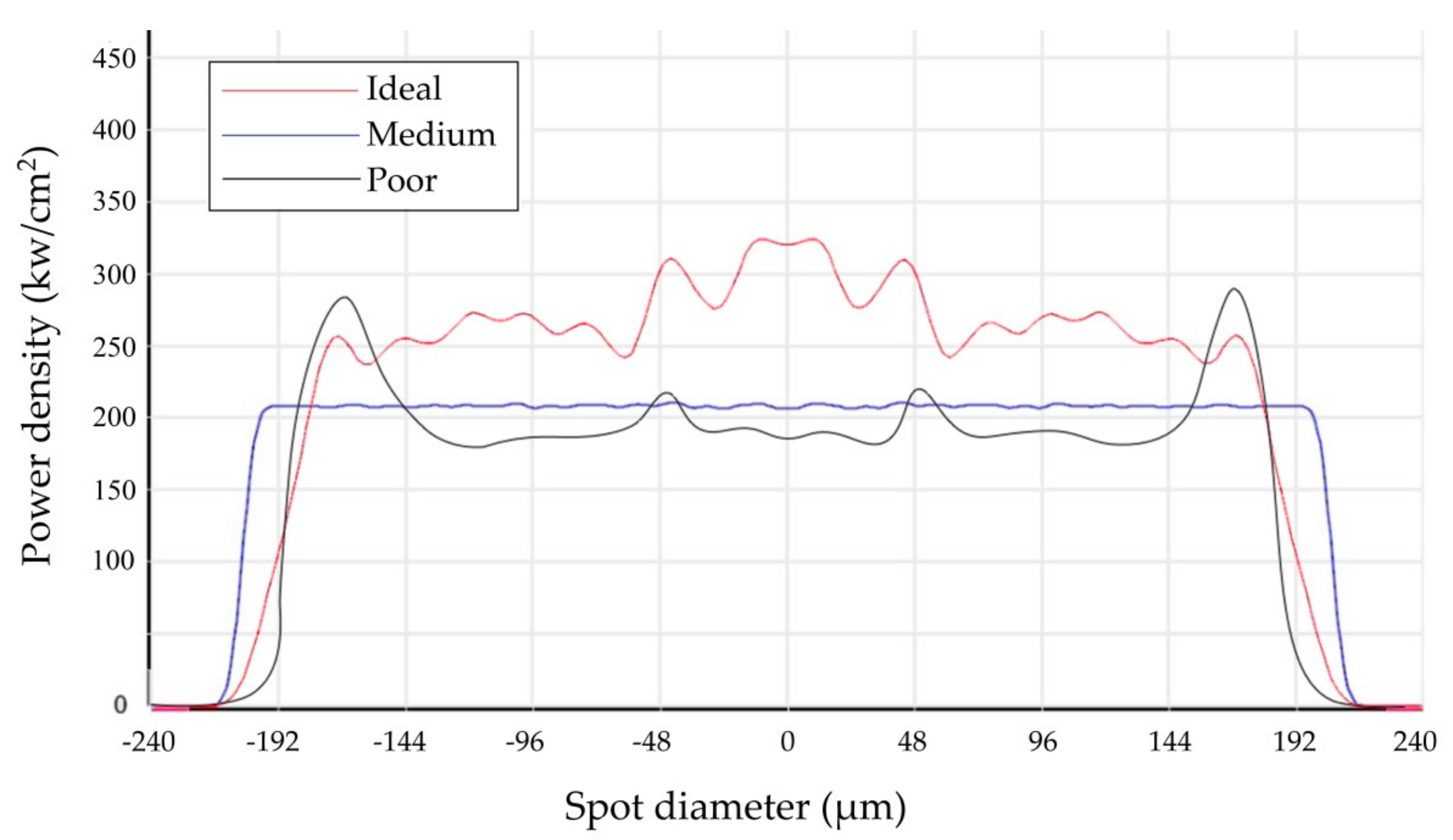
2.1. CW Laser Beam Profile Pattern
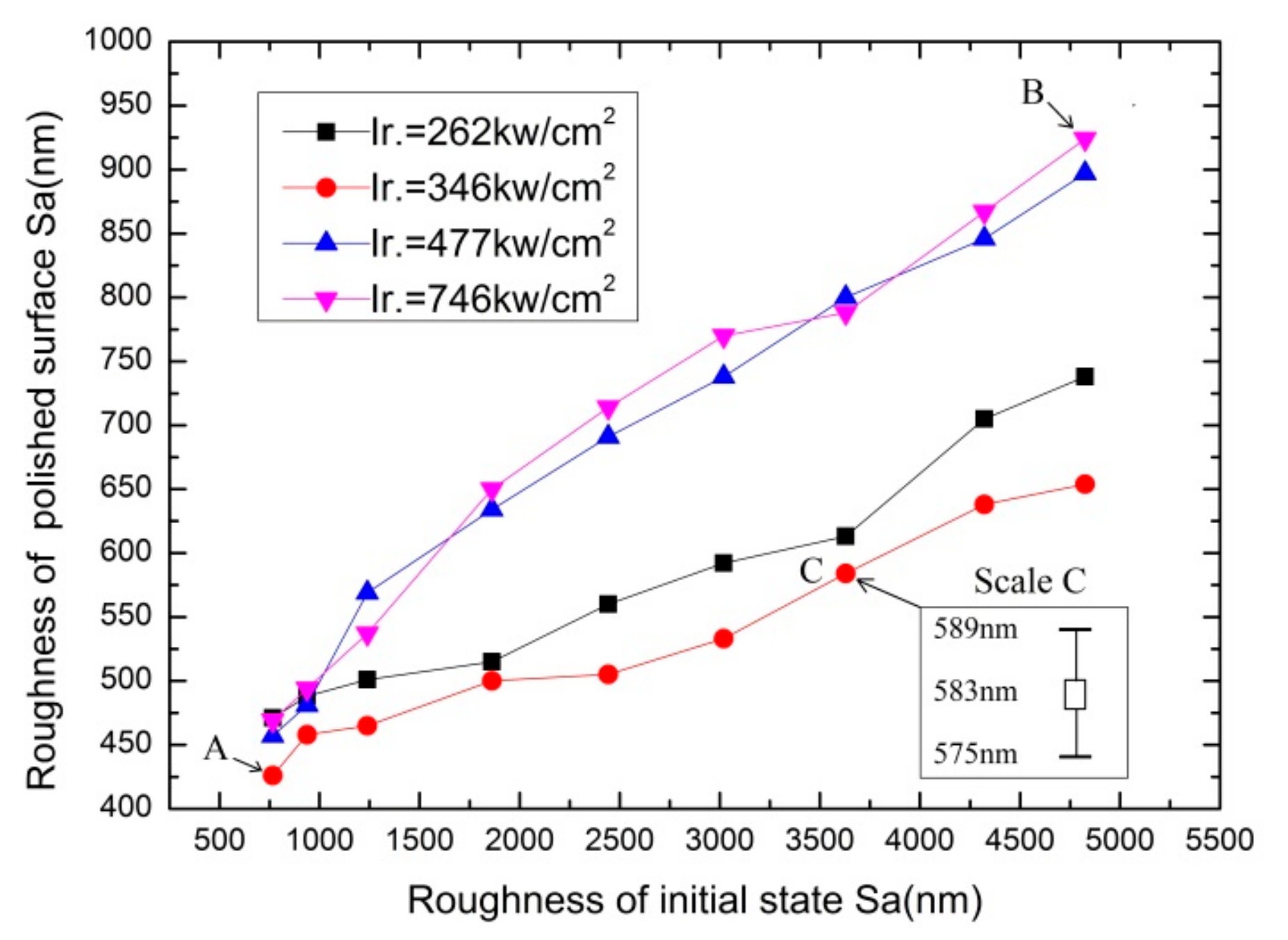
2.2. CW Laser Polishing Using Different Sopt Diameters
3. Experiment of Dual-Beam Laser Polishing
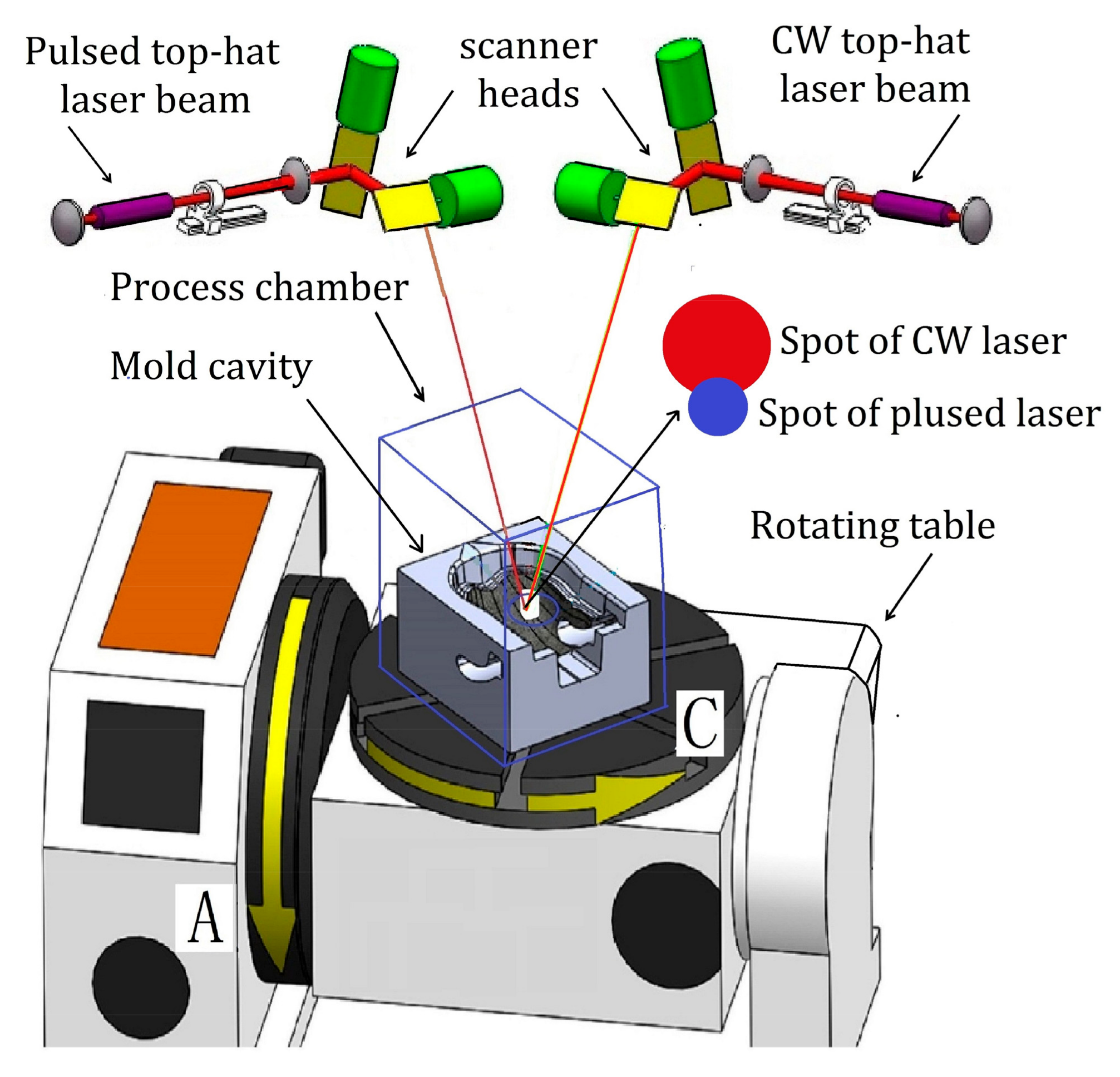
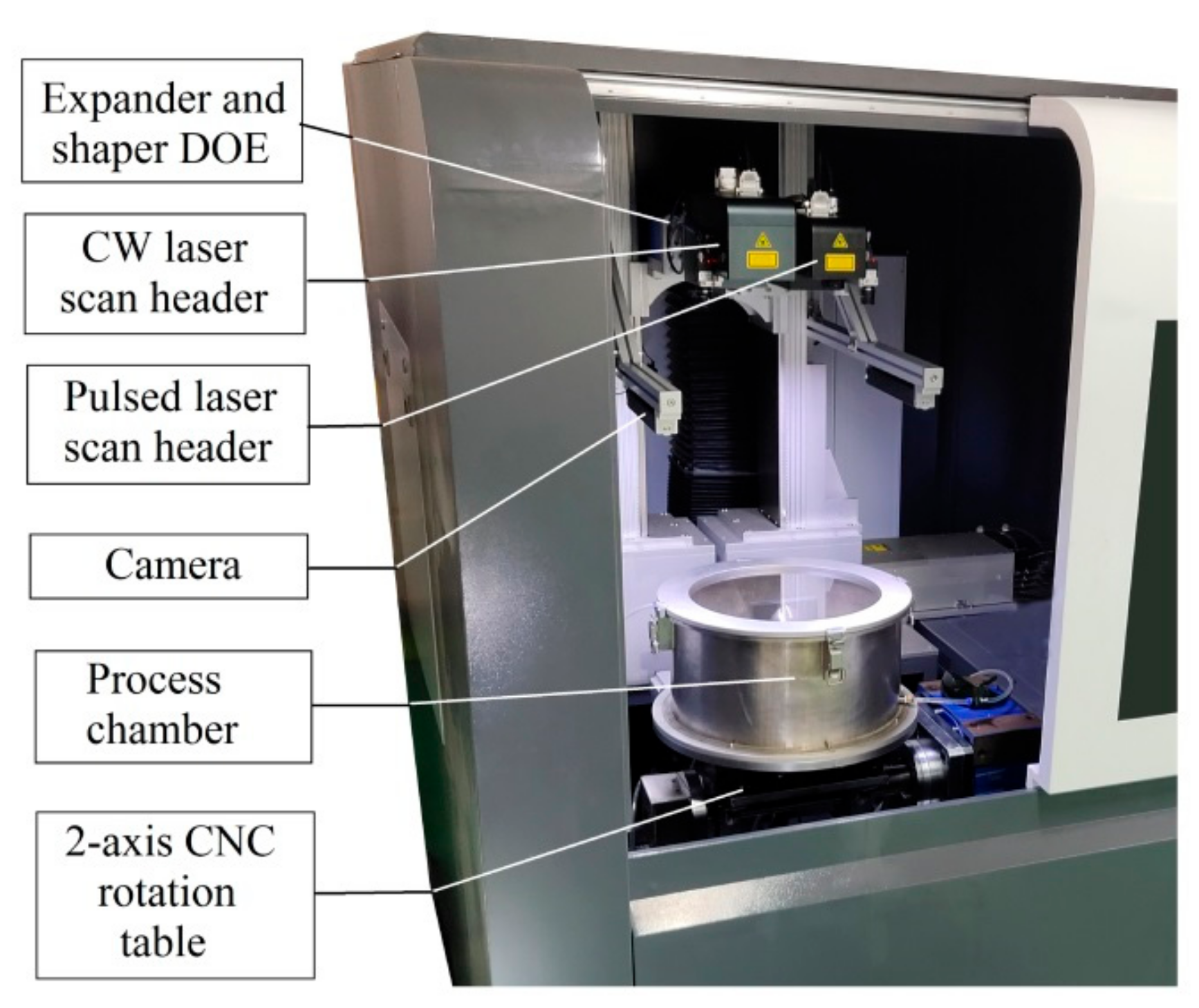
3.1. Dual-Beam Laser Polishing Device
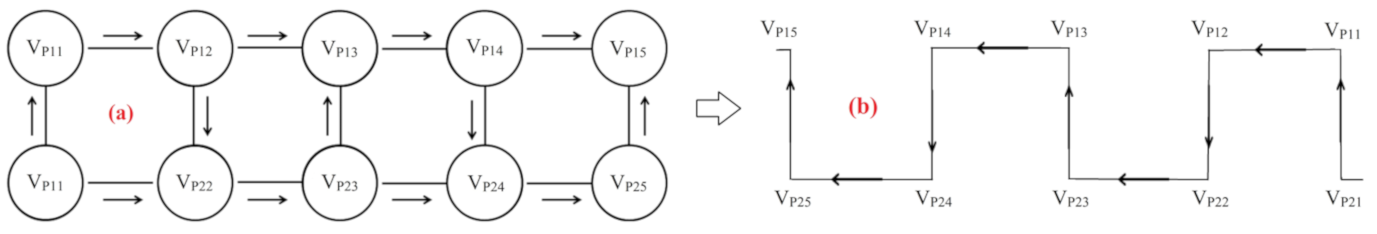
3.2. Trajectory of Zigzag-Square Wave of Dual-Beam
3.3. Experiment of Dual-Laser Beam Polishing
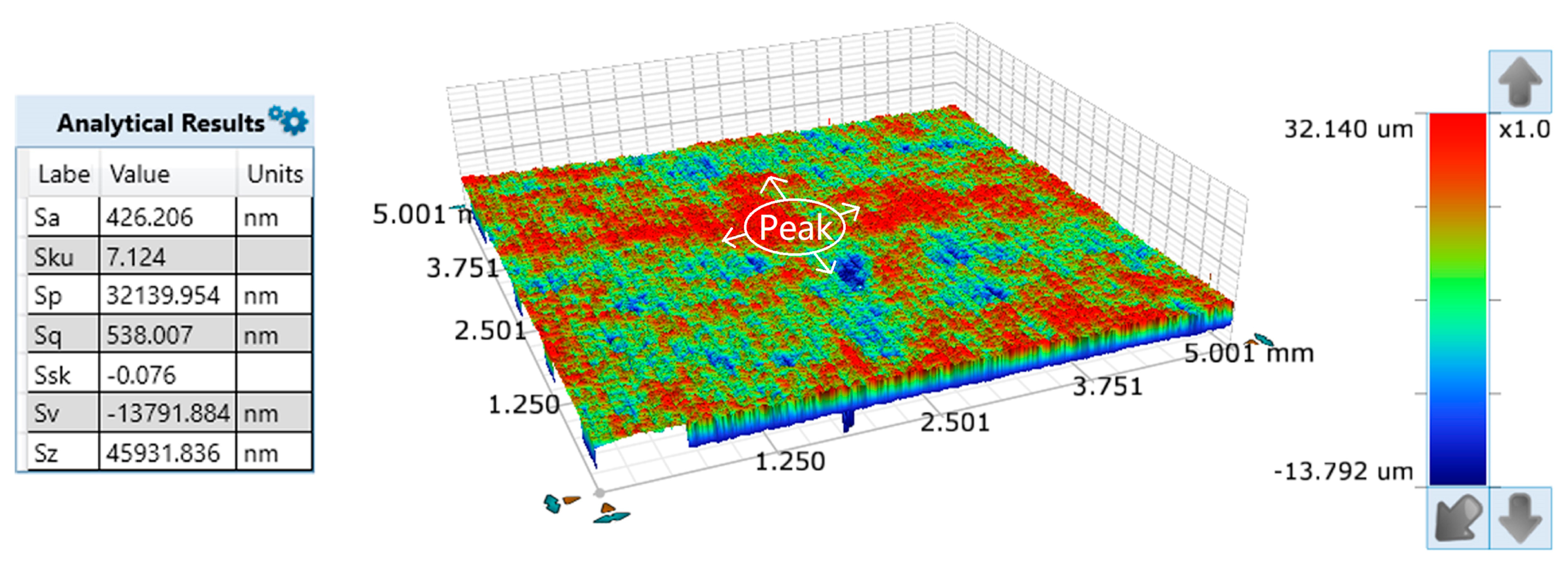
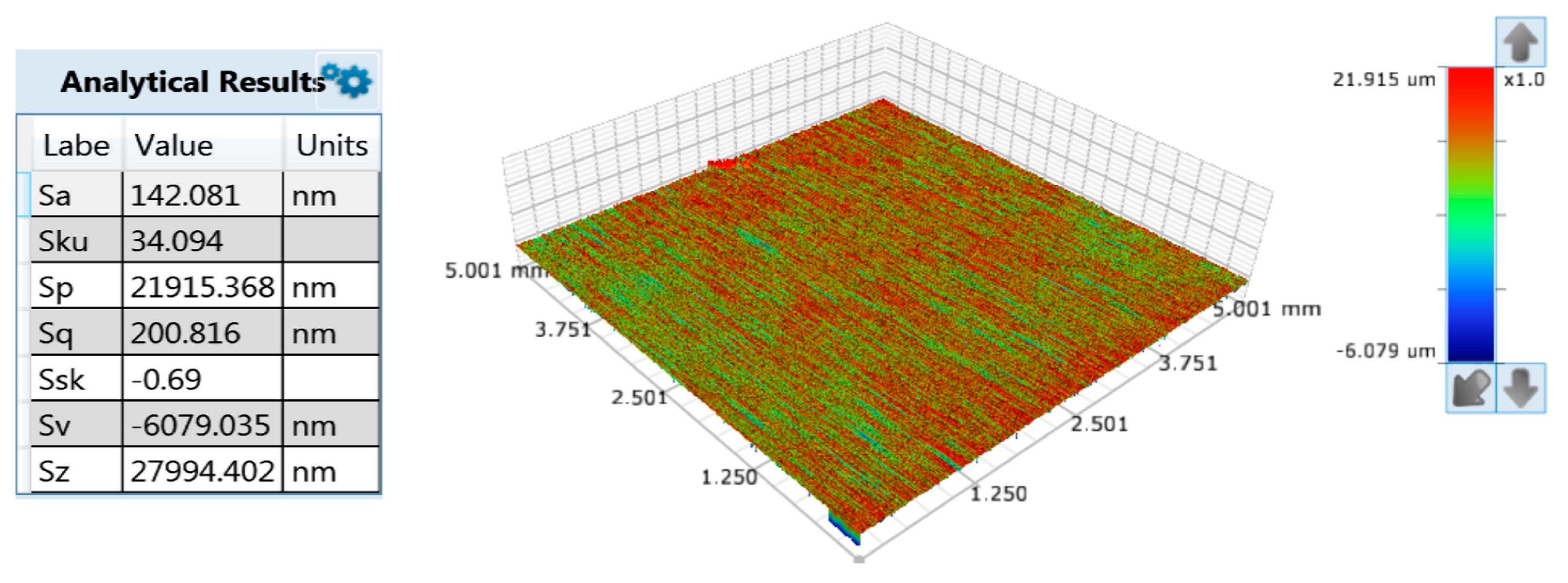
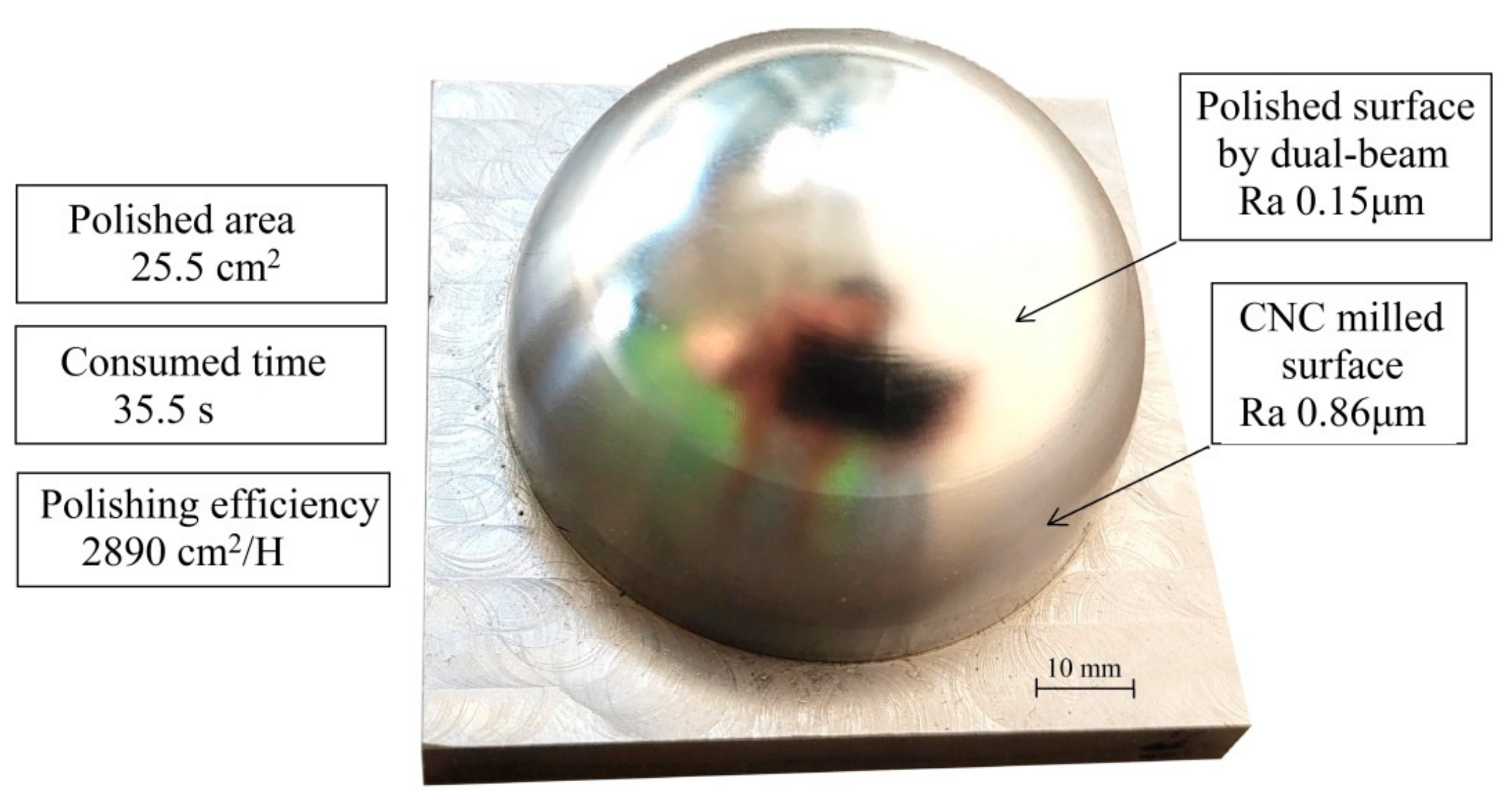
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Burzic, B.; Hofele, M.; Mürdter, S.; Riegel, H. Laser polishing of ground aluminum surfaces with high energy continuous wave laser. J. Laser Appl. 2017, 29, 011701. [Google Scholar] [CrossRef]
- Perry, T.L.; Werschmoeller, D.; Duffie, N.A.; Li, X.; Pfefferkorn, F.E. Examination of selective pulsed laser micropolishing on microfabricated nickel samples using spatial frequency analysis. J. Manuf. Sci. Eng. 2009, 131, 021002. [Google Scholar] [CrossRef]
- Miller, J.D.; Tutunea-Fatan, O.R.; Bordatchev, E.V. Experimental analysis of laser and scanner control parameters during laser polishing of h13 steel. Procedia Manuf. 2017, 10, 720–729. [Google Scholar] [CrossRef]
- Athanasiou, C.-E.; Bellouard, Y. A monolithic micro-tensile tester for investigating silicon dioxide polymorph micromechanics, fabricated and operated using a femtosecond laser. Micromachines 2015, 6, 1365–1386. [Google Scholar] [CrossRef]
- Drs, J.; Kishi, T.; Bellouard, Y. Laser-assisted morphing of complex three dimensional objects. Opt. Express 2015, 23, 17355–17366. [Google Scholar] [CrossRef]
- Bordatchev, E.V.; Hafiz, A.M.; Tutunea-Fatan, O.R. Performance of laser polishing in finishing of metallic surfaces. Int. J. Adv. Manuf. Technol. 2014, 73, 35–52. [Google Scholar] [CrossRef]
- Goffin, N.; Tyrer, J.; Woolley, E. Complex beam profiles for laser annealing of thin-film cdte photovoltaics. J. Laser Appl. 2018, 30, 042006. [Google Scholar] [CrossRef]
- Hafiz, A.M.K.; Bordatchev, E.V.; Tutunea-Fatan, R.O. Influence of overlap between the laser beam tracks on surface quality in laser polishing of AISI H13 tool steel. J. Manuf. Process. 2012, 14, 425–434. [Google Scholar] [CrossRef]
- Bhaduri, D.; Penchev, P.; Batal, A.; Dimov, S.; Soo, S.L.; Sten, S.; Harrysson, U.; Zhang, Z.; Dong, H. Laser polishing of 3D printed mesoscale components. Appl. Surf. Sci. 2017, 405, 29–46. [Google Scholar] [CrossRef]
- Beam Shaper/Top-Hat. Available online: https://www.holoor.co.il (accessed on 9 May 2019).
- Kumstel, J.; Kirsch, B. Polishing titanium-and nickel-based alloys using cw-laser radiation. Phys. Procedia 2013, 41, 362–371. [Google Scholar] [CrossRef]
- Chang, C.-S.; Chen, T.-H.; Li, T.-C.; Lin, S.-L.; Liu, S.-H.; Lin, J.-F. Influence of laser beam fluence on surface quality, microstructure, mechanical properties, and tribological results for laser polishing of SKD61 tool steel. J. Mater. Process. Technol. 2016, 229, 22–35. [Google Scholar] [CrossRef]
- Vaithilingam, J.; Goodridge, R.D.; Hague, R.J.; Christie, S.D.; Edmondson, S. The effect of laser remelting on the surface chemistry of Ti6Al4v components fabricated by selective laser melting. J. Mater. Proc. Technol. 2016, 232, 1–8. [Google Scholar] [CrossRef]
- Richter, B.; Blanke, N.; Werner, C.; Vollertsen, F.; Pfefferkorn, F. Effect of initial surface features on laser polishing of Co-Cr-Mo alloy made by powder-bed fusion. JOM 2019, 71, 912–919. [Google Scholar] [CrossRef]
- Nüsser, C.; Sändker, H.; Willenborg, E. Pulsed laser micro polishing of metals using dual-beam technology. Phys. Procedia 2013, 41, 346–355. [Google Scholar] [CrossRef]
- Ukar, E.; Lamikiz, A.; Martínez, S.; Tabernero, I.; de Lacalle, L.L. Roughness prediction on laser polished surfaces. J. Mater. Process. Technol. 2012, 212, 1305–1313. [Google Scholar] [CrossRef]
- Pfefferkorn, F.E.; Duffie, N.A.; Morrow, J.D.; Wang, Q. Effect of beam diameter on pulsed laser polishing of S7 tool steel. CIRP Ann. 2014, 63, 237–240. [Google Scholar] [CrossRef]
- Liu, R.; Wang, Z.; Zhang, Y.; Sparks, T.; Liou, F. A smooth toolpath generation method for laser metal deposition. In Proceedings of the 27th Annual International Solid Freeform Fabrication Symposium, Austin, TX, USA, 8–10 August 2016; pp. 1038–1046. [Google Scholar]
- Roghanian, E.; Shakeri Kebria, Z. The combination of topsis method and Dijkstra’s algorithm in multi-attribute routing. Sci. Iran. 2017, 24, 2540–2549. [Google Scholar] [CrossRef][Green Version]
- Rodríguez-Puente, R.; Lazo-Cortés, M.S. Algorithm for shortest path search in geographic information systems by using reduced graphs. SpringerPlus 2013, 2, 291. [Google Scholar] [CrossRef]
- Ahmadi, S.M.; Kebriaei, H.; Moradi, H. Constrained coverage path planning: Evolutionary and classical approaches. Robotica 2018, 36, 904–924. [Google Scholar] [CrossRef]
- Kapil, S.; Joshi, P.; Yagani, H.V.; Rana, D.; Kulkarni, P.M.; Kumar, R.; Karunakaran, K. Optimal space filling for additive manufacturing. Rapid Prototyp. J. 2016, 22, 660–675. [Google Scholar] [CrossRef]
- Selvaraj, P.; Radhakrishnan, P. Algorithm for pocket milling using zig-zag tool path. Def. Sci. J. 2006, 56, 117–127. [Google Scholar] [CrossRef]




| Group # | I | II | III | IV |
|---|---|---|---|---|
| Spot diameter Ds (mm) | 0.54 | 0.47 | 0.40 | 0.32 |
| Spot Irradiation Ir. (kw/cm2) | 262 | 346 | 477 | 746 |
| Factor Name | Optimized Value or Feature | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Roughness of initial state Sa (nm) | 768 | 983 | 1293 | 1862 | 2443 | 3020 | 3612 | 4361 | 4826 |
| Scanning speed (mm/s) | 800 | 800 | 750 | 750 | 700 | 700 | 650 | 650 | 600 |
| Top-hat beam profile | Between Medium and Ideal | ||||||||
| Polishing parameters | Wavelength: 1080 nm, Power: 600 W, Step-over: 0.1 mm | ||||||||
| Set up Laser Parameters | CW Laser | Pulsed Laser |
|---|---|---|
| Power | 600 W | 80 W |
| Wavelength | 1080 nm | 1064 nm |
| Beam profile pattern | Top-hat (shaped) | Top-hat |
| Pulse duration | N/A | 1.3 μs |
| Spot diameter | 0.47 mm | 0.32 mm |
| Scanning speed | 800 mm/s | 2000 mm/s |
| Step-over | 0.1 mm | 0.1 mm |
| Scanning route | Zigzag | Zigzag-square wave |
| Top-hat beam profile | Between Medium and Ideal | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Y.; Zhao, Z.; Zhang, W.; Xiao, H.; Xu, X. Experiment Study of Rapid Laser Polishing of Freeform Steel Surface by Dual-Beam. Coatings 2019, 9, 324. https://doi.org/10.3390/coatings9050324
Zhou Y, Zhao Z, Zhang W, Xiao H, Xu X. Experiment Study of Rapid Laser Polishing of Freeform Steel Surface by Dual-Beam. Coatings. 2019; 9(5):324. https://doi.org/10.3390/coatings9050324
Chicago/Turabian StyleZhou, Yongquan, Zhenyu Zhao, Wei Zhang, Haibing Xiao, and Xiaomei Xu. 2019. "Experiment Study of Rapid Laser Polishing of Freeform Steel Surface by Dual-Beam" Coatings 9, no. 5: 324. https://doi.org/10.3390/coatings9050324
APA StyleZhou, Y., Zhao, Z., Zhang, W., Xiao, H., & Xu, X. (2019). Experiment Study of Rapid Laser Polishing of Freeform Steel Surface by Dual-Beam. Coatings, 9(5), 324. https://doi.org/10.3390/coatings9050324





