Analysis of Unsteady Flow and Heat Transfer of Nanofluid Using Blasius–Rayleigh–Stokes Variable
Abstract
:1. Introduction
2. Mathematical Formulation
3. Results and Discussions
4. Conclusions
- With the increase in the value of Brownian diffusion parameter , the temperature enhances while the nanoparticles volume fraction decreases.
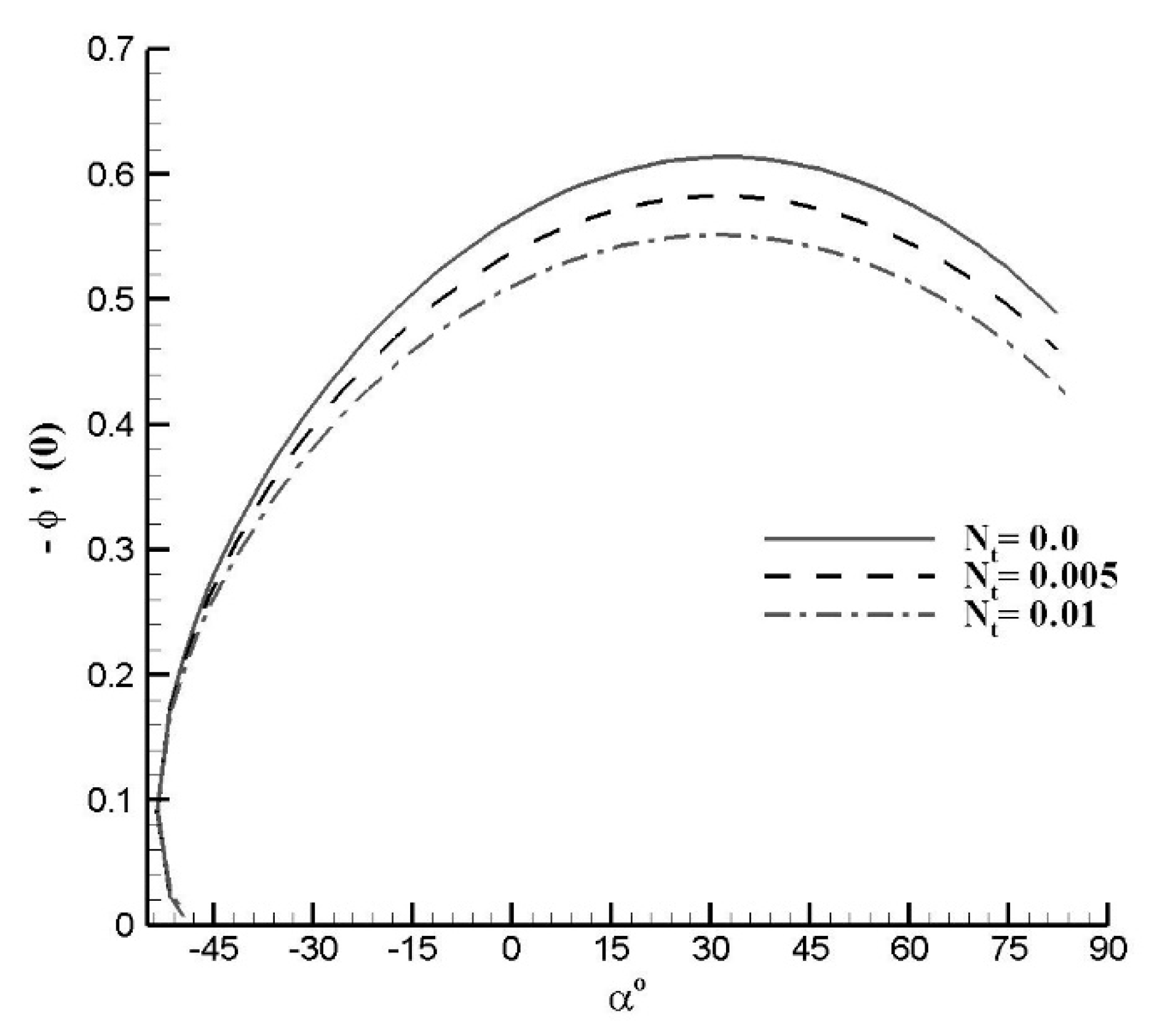
- By increasing the thermophoretic diffusion parameter , both temperature and nanoparticles concentration are increased.
- Concentration of nanoparticles reduces with the enhancement of Lewis number .
- Dual solutions exist for both thermal and concentration boundary layers. The mass flux rate attains the maximum value of slot moving parameter , as the Lewis number is increased.
- Heat flux at the surface reduces with the increase of and in the upper solution branch. The reduced Sherwood number is enhanced when is increased, whereas it reduces with increasing .
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclatures
| U | Velocity component in X direction |
| V | Velocity component in Y direction |
| Uw | Plate velocity |
| ψ | Stream function |
| T | Temperature |
| T∞ | Ambient temperature |
| TW | Wall temperature |
| C | Nanoparticles concentration |
| C∞ | Ambient nanoparticles concentration |
| CW | Wall nanoparticles concentration |
| η | Similarity variable |
| α | Moving slot parameter |
| DB | Brownian diffusion coefficient |
| DT | Thermophoretic diffusion coefficient |
| Le | Lewis number |
| Nb | Brownian diffusion parameter |
| Nt | Thermophoretic diffusion parameter |
| Pr | Prandtl number |
| φ | Nondimensional nanoparticles concentration |
| θ | Nondimensional temperature |
| ν | Kinematic viscosity |
| σ | Thermal diffusivity |
| ε | Ratio of heat capacities of the nanoparticles |
| Heat capacity of fluid | |
| Heat capacity of nanoparticles |
Appendix A
References
- Singh, R.P. “Drag Reduction”. In Encyclopedia of Polymer Science and Technology; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2004. [Google Scholar]
- Zhao, H.; Wu, J.Z.; Luo, J.S. Turbulent drag reduction by traveling wave of flexible wall. Fluid Dyn. Res. 2004, 34, 175–198. [Google Scholar] [CrossRef]
- Gyr, A.; Bewersdorff, H.W. Drag Reduction of Turbulent Flow by Additives; Academic Publishers: Dordrecht, The Netherlands, 1995. [Google Scholar]
- Buongiorno, J. Convective transport in nanofluids. J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Khan, W.A.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Transf. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]
- Das, K. Nanofluid flow over a shrinking sheet with surface slip. Microfluid. Nanofluid. 2014, 16, 391–401. [Google Scholar] [CrossRef]
- Bashirnezhad, K.; Bazri, S.; Safaei, M.R. Viscosity of nanofluids: A review of recent experimental studies. Int. Commun. Heat Mass Transf. 2016, 73, 114–123. [Google Scholar] [CrossRef]
- Ahmad, A. Flow of ReinerPhilippoff based nano-fluid past a stretching sheet. J. Mol. Liquids 2016, 219, 643–646. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Groşanc, T.; Pop, I. Steady-state free convection in right-angle porous trapezoidal cavity filled by a nanofluid: Buongiorno’s mathematical model. Eur. J. Mech. B/Fluids 2015, 53, 241–250. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Pop, I. Free convection in a porous horizontal cylindrical annulus with a nanofluid using Buongiorno’s model. Comput. Fluids 2015, 118, 182–190. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Cimpean, D.S.; Pop, I. Free convection in a partially heated wavy porous cavity filled with a nanofluid under the effects of Brownian diffusion and thermophoresis. Appl. Therm. Eng. 2017, 113, 413–418. [Google Scholar] [CrossRef]
- Afridi, M.I.; Qasim, M.; Wakif, A.; Hussanan, A. Second law analysis of dissipative nanofluid flow over a curved surface in the presence of Lorentz force: Utilization of the Chebyshev–Gauss–Lobatto spectral method. Nanomaterials 2019, 9, 195. [Google Scholar] [CrossRef] [PubMed]
- Crane, L.J. Flow past a stretching plate. Zeitschrift für angewandte Mathematik und Physik ZAMP 1970, 21, 645–647. [Google Scholar] [CrossRef]
- Magyari, E.; Keller, B. Heat and mass transfer in the boundary layers on an exponentially stretching continuous surface. J. Phys. D Appl. Phys. 1999, 32, 577. [Google Scholar] [CrossRef]
- Liao, S. A new branch of solutions of boundary-layer flows over an impermeable stretched plate. Int. J. Heat Mass Transf. 2005, 48, 2529–2539. [Google Scholar] [CrossRef]
- Fang, T. Flow over a stretchable disk. Phys. Fluids 2007, 19, 128105. [Google Scholar] [CrossRef]
- Ramesh, G.K.; Gireesha, B.J.; Bagewadi, C.S. Heat transfer in MHD dusty boundary layer flow over an inclined stretching sheet with non-uniform heat source/sink. Adv. Math. Phys. 2012, 2012, 657805. [Google Scholar] [CrossRef]
- Imran, S.M.; Asghar, S.; Mushtaq, M. Mixed Convection Flow over an Unsteady Stretching Surface in a Porous Medium with Heat Source. Math. Probl. Eng. 2012, 2012, 485418. [Google Scholar] [CrossRef]
- Todd, L. A family of laminar boundary layers along a semi-infinite flat plate. Fluid Dyn. Res. 1997, 19, 235–249. [Google Scholar] [CrossRef]
- Fang, T.; Zhang, J.; Yao, S. A new family of unsteady boundary layers over a stretching surface. Appl. Math. Comput. 2010, 217, 3747–3755. [Google Scholar] [CrossRef]
- Pritchard, P.J.; Mitchell, J.W. Fox and McDonald’s Introduction to Fluid Mechanics, 9th ed.; Wiley: Hoboken, NJ, USA, 2011. [Google Scholar]
- Sakiadis, B.C. Boundary layer behavior on continuous solid surfaces: I. Boundary-layer equations for two-dimensional and axisymmetric flow. AIChE J. 1961, 7, 26–28. [Google Scholar] [CrossRef]

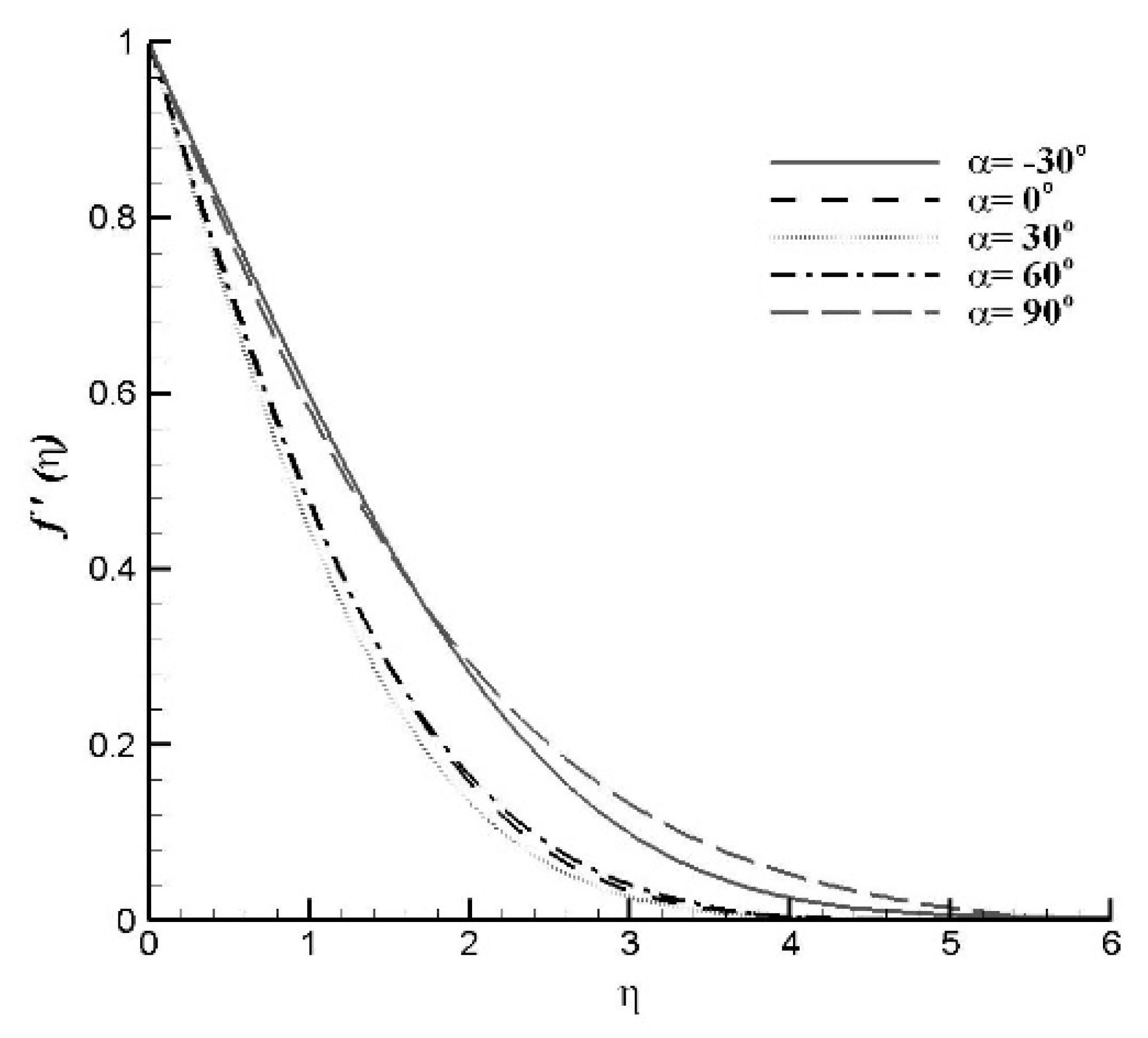
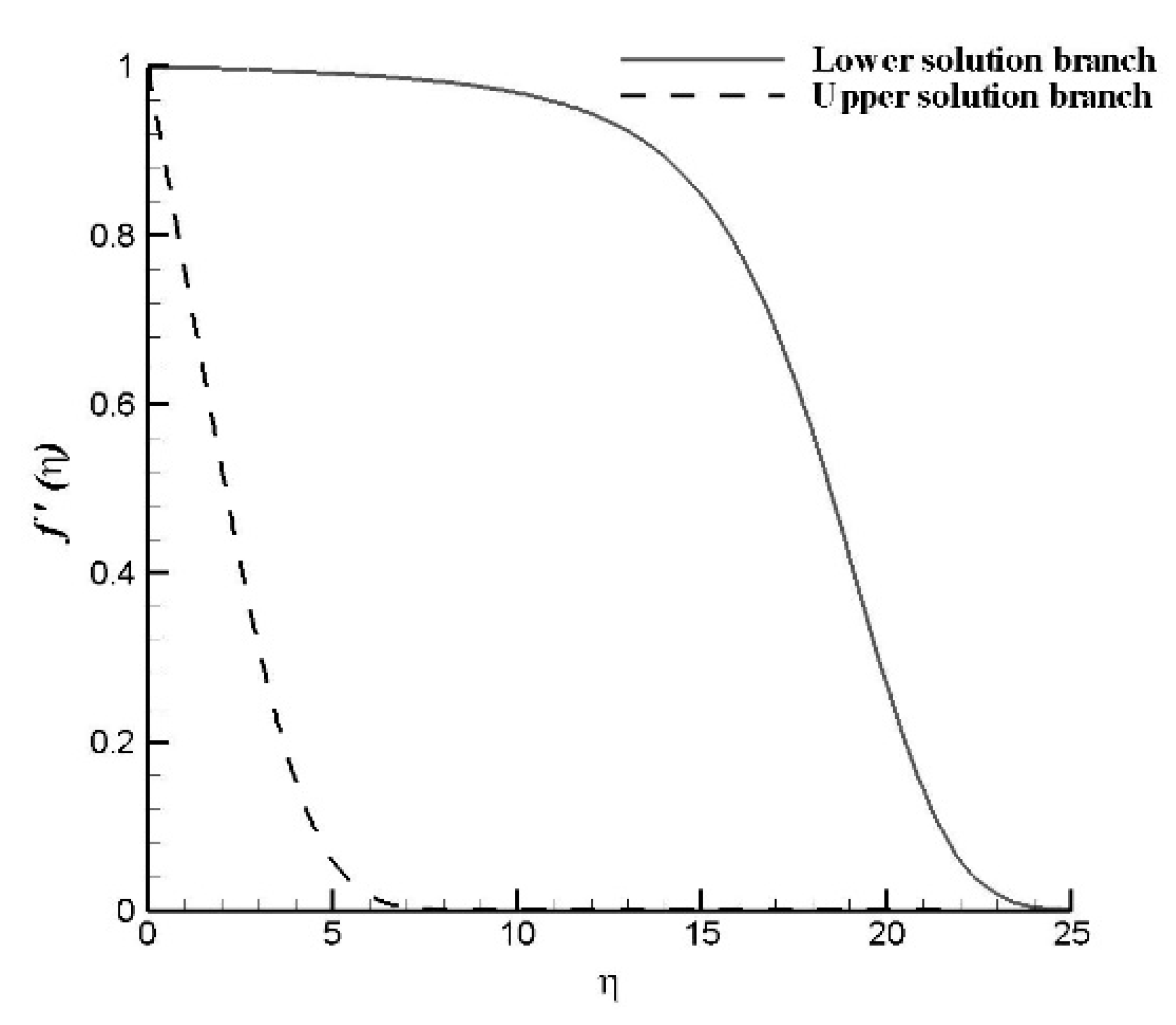
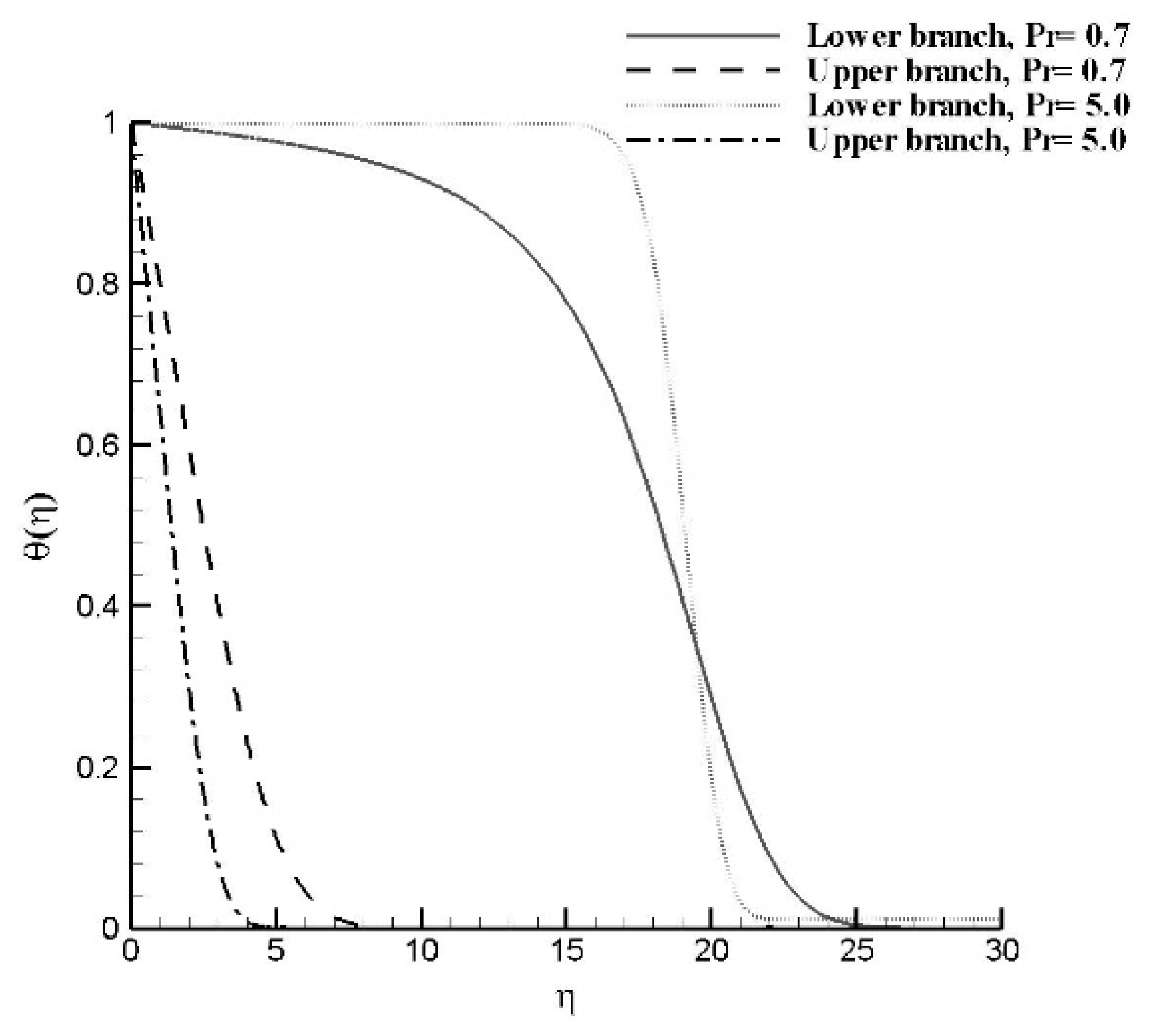
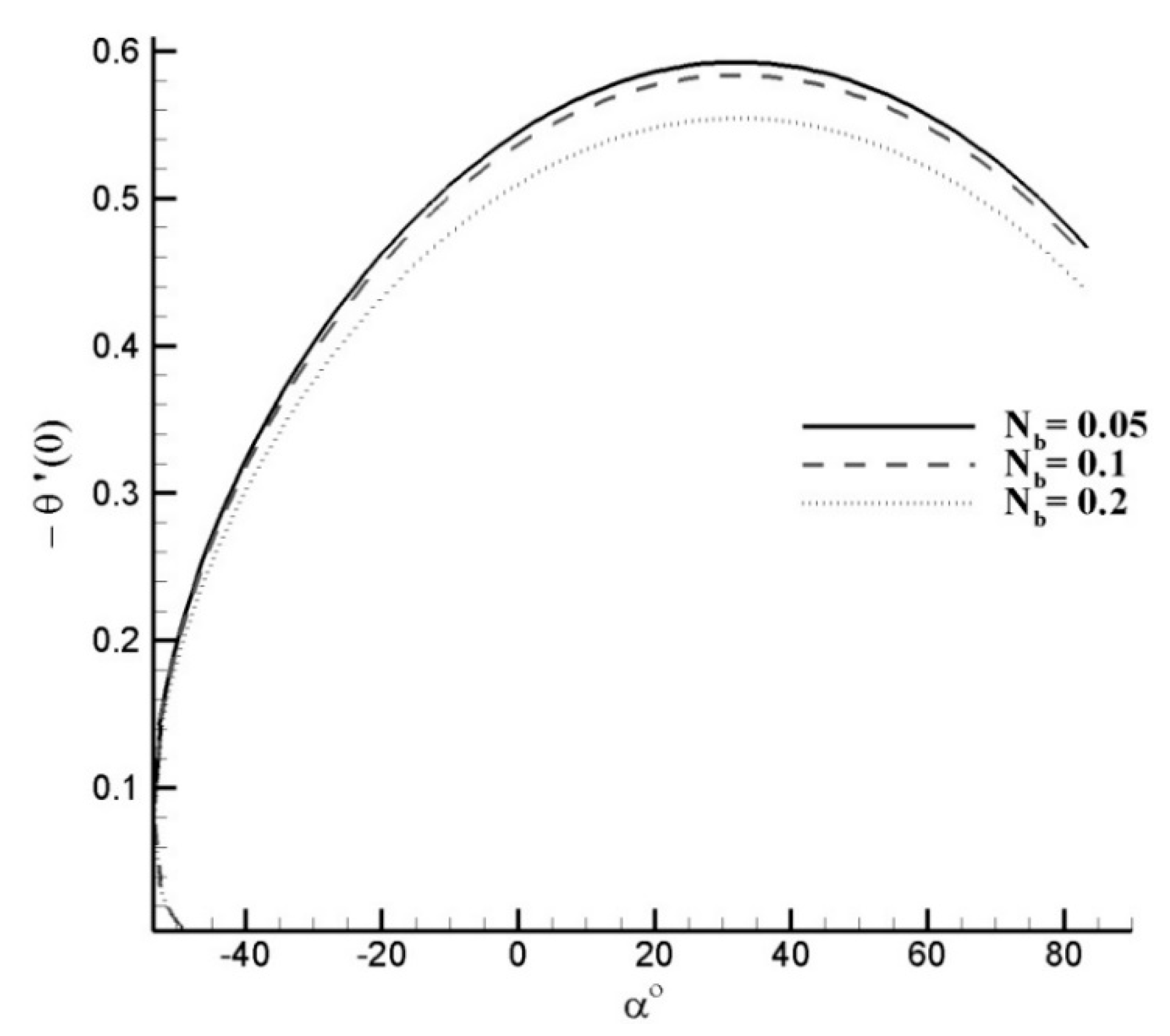
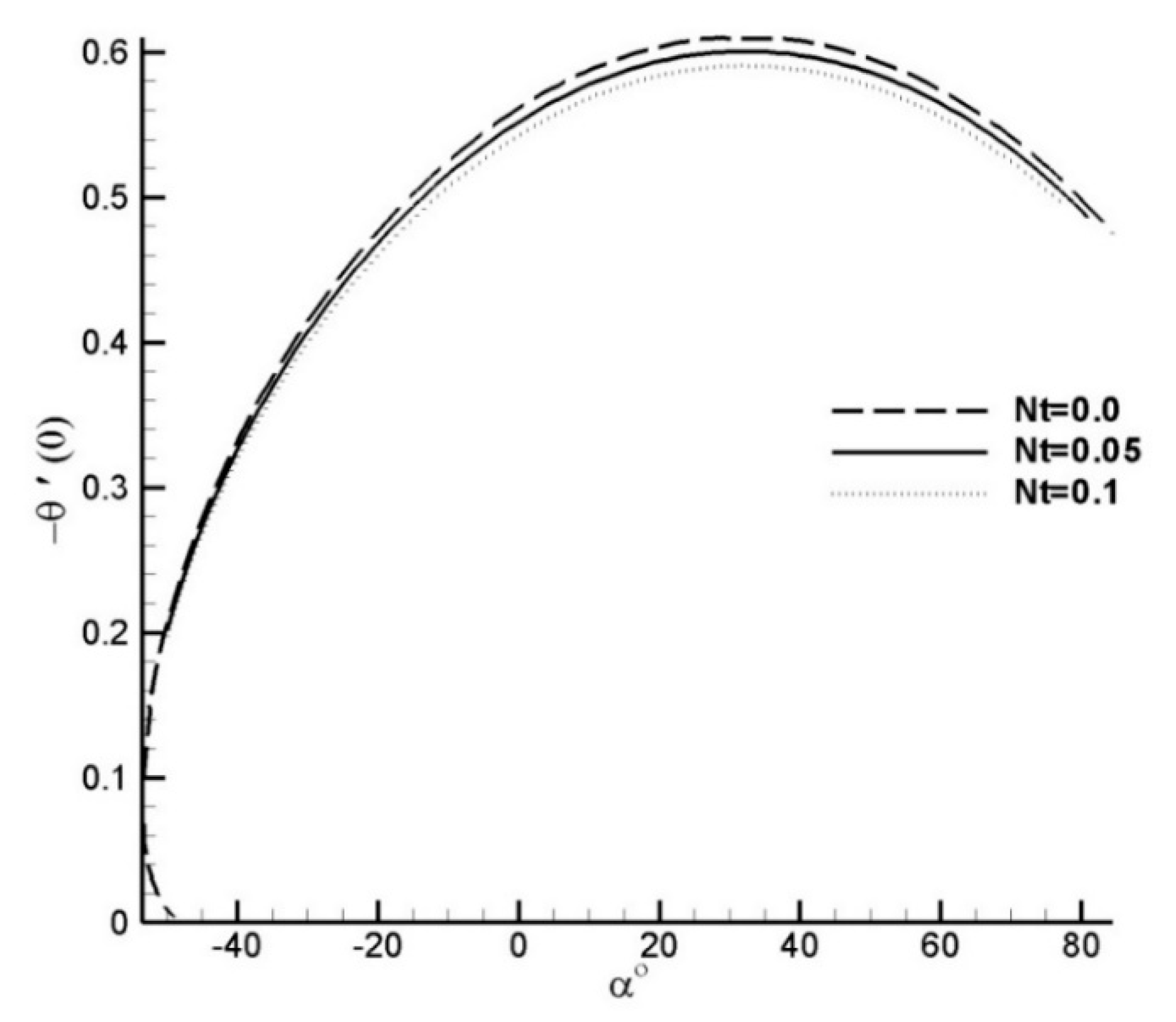
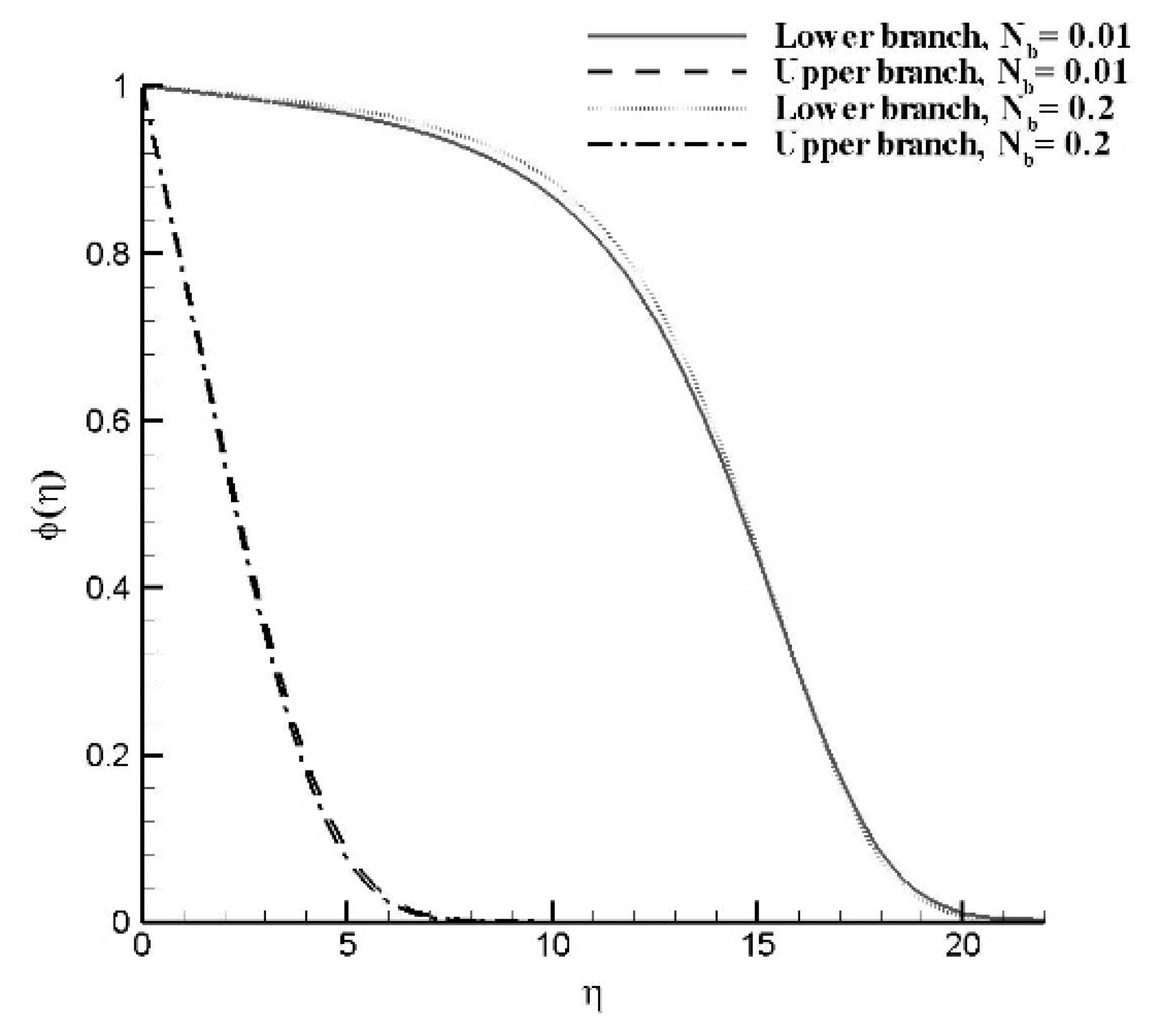
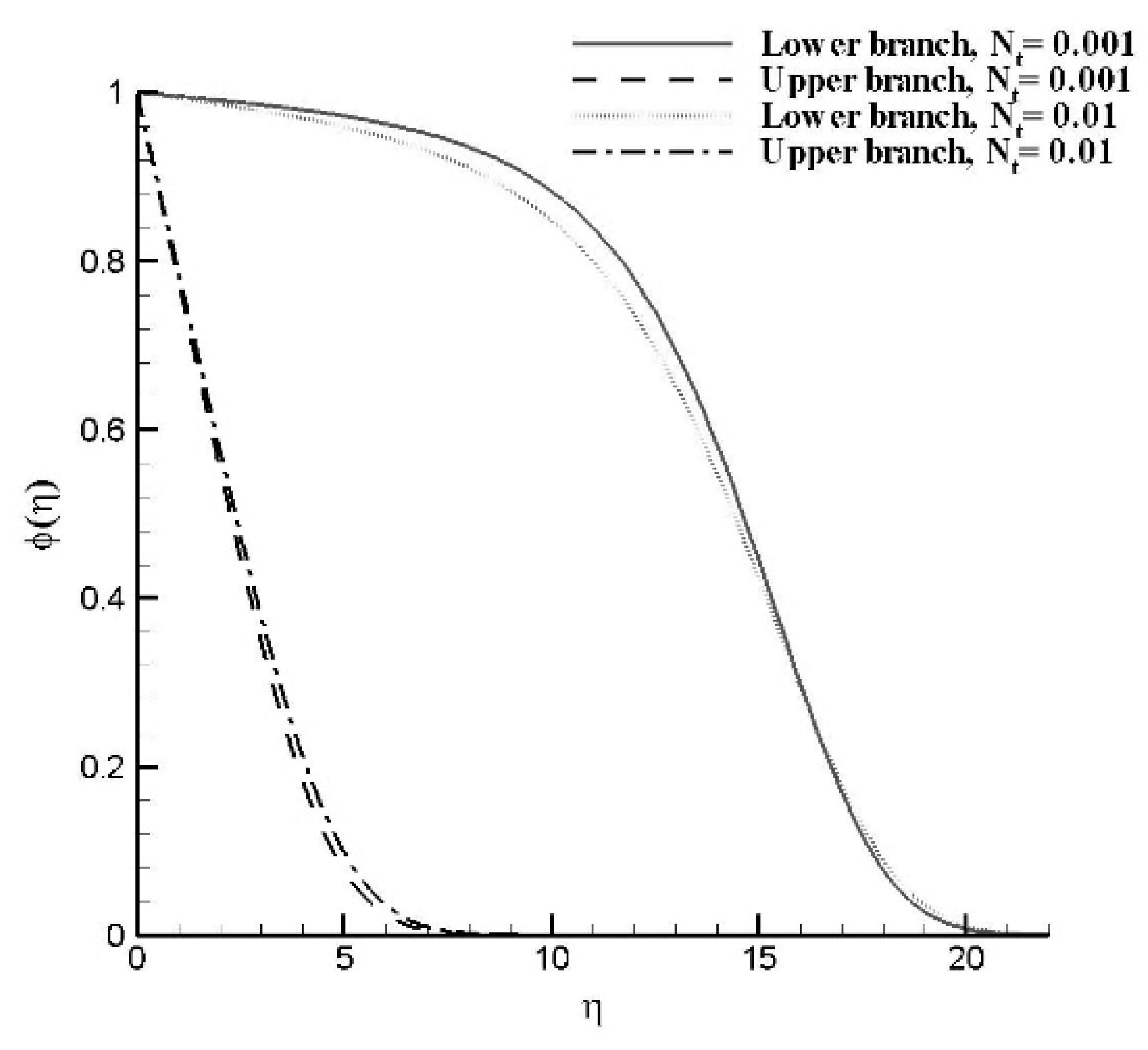
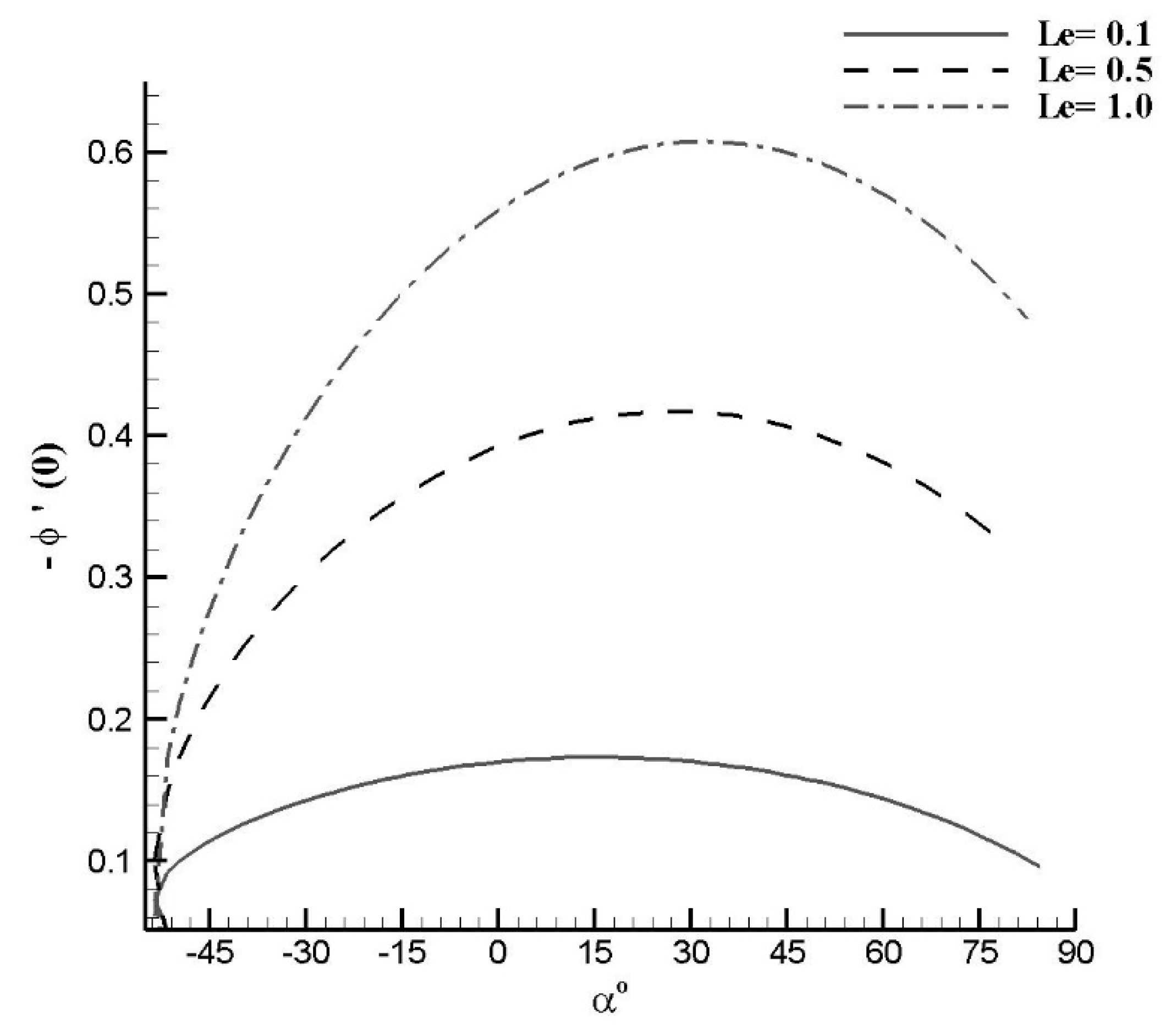

| (Fang [17]) | Present Study | |
|---|---|---|
| 90° | 0.443748 | 0.443872 |
| 60° | 0.576684 | 0.576685 |
| 30° | 0.613527 | 0.613526 |
| 0° | 0.564190 | 0.564189 |
| −30° | 0.416304 | 0.416303 |
| −48° (upper solution) | 0.239052 | 0.239055 |
| −48° (lower solution) | 0.00150569 | 0.00149961 |
| Parameters Values | (Analytical) | (Numerical) | (Analytical) | (Numerical) |
|---|---|---|---|---|
| Pr = 1, Le = 0.5, Nb = 0.01, Nt = 0.01 | 0.5541896 | 0.5603877 | 0.0884477 | 0.0718526 |
| Pr = 1, Le = 0.5, Nb = 0.05, Nt = 0.0 | 0.5541896 | 0.5532004 | 0.39894228 | 0.39894228 |
| Pr = 1, Le = 1.0, Nb = 0.05, Nt = 0.0 | 0.55418958 | 0.5502023 | 0.56418958 | 0.56418958 |
| Pr = 1, Le = 2.0, Nb = 0.02, Nt = 0.01 | 0.55418958 | 0.5551755 | 0.67603707 | 0.68467843 |
| Pr | Le | α | C | CB | CT | Max. % Error | Solution Curve |
|---|---|---|---|---|---|---|---|
| 0.5 | 1.5 | 30 | 0413 | −0.226 | −0.132 | 2.60% | – |
| 1.0 | 1.0 | 30 | 0.605 | −0.261 | −0.168 | 1.760% | – |
| 1.0 | 1.0 | 0.0 | 0.601 | −0.253 | −0.161 | 2.650% | – |
| 2.0 | 1.5 | 30 | 0.877 | −0.305 | −0.21 | 0.986% | – |
| 1.0 | 1.0 | −49 | 0.235 | −0.101 | −0.075 | 2.430% | Upper Solution |
| 1.0 | 1.0 | −49 | 0.004 | −0.002 | −0.002 | 7.970% | Lower Solution |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lu, D.; Mumtaz, S.; Farooq, U.; Ahmad, A. Analysis of Unsteady Flow and Heat Transfer of Nanofluid Using Blasius–Rayleigh–Stokes Variable. Coatings 2019, 9, 211. https://doi.org/10.3390/coatings9030211
Lu D, Mumtaz S, Farooq U, Ahmad A. Analysis of Unsteady Flow and Heat Transfer of Nanofluid Using Blasius–Rayleigh–Stokes Variable. Coatings. 2019; 9(3):211. https://doi.org/10.3390/coatings9030211
Chicago/Turabian StyleLu, Dianchen, Sumayya Mumtaz, Umer Farooq, and Adeel Ahmad. 2019. "Analysis of Unsteady Flow and Heat Transfer of Nanofluid Using Blasius–Rayleigh–Stokes Variable" Coatings 9, no. 3: 211. https://doi.org/10.3390/coatings9030211
APA StyleLu, D., Mumtaz, S., Farooq, U., & Ahmad, A. (2019). Analysis of Unsteady Flow and Heat Transfer of Nanofluid Using Blasius–Rayleigh–Stokes Variable. Coatings, 9(3), 211. https://doi.org/10.3390/coatings9030211






