The Development and Experimental Validation of a Surface Roughness Prediction Model for the Vertical Vibratory Finishing of Blisks
Abstract
1. Introduction
2. Vertical Vibratory Finishing of Blisks
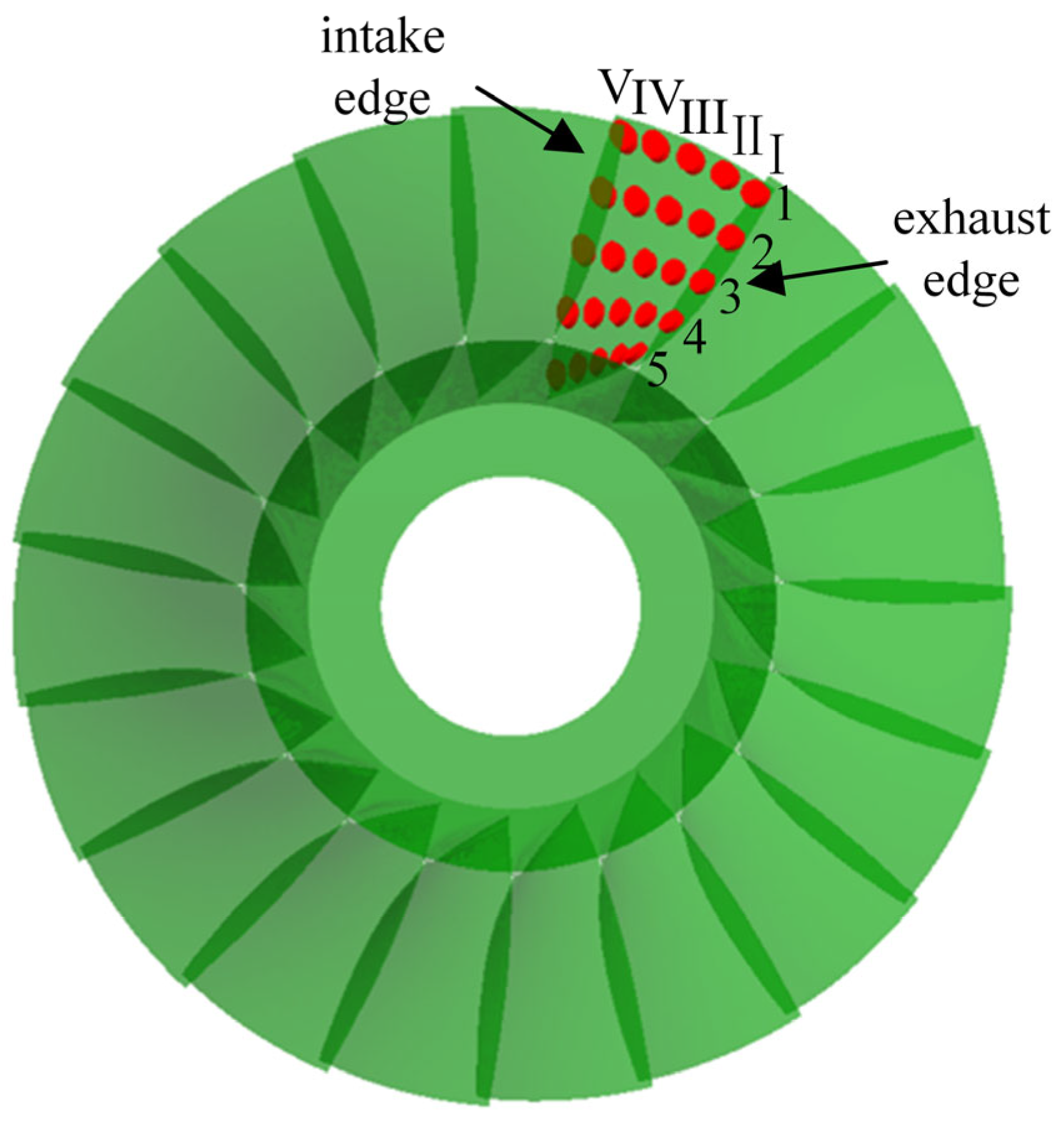
2.1. Processing Principle and Simulation Parameters
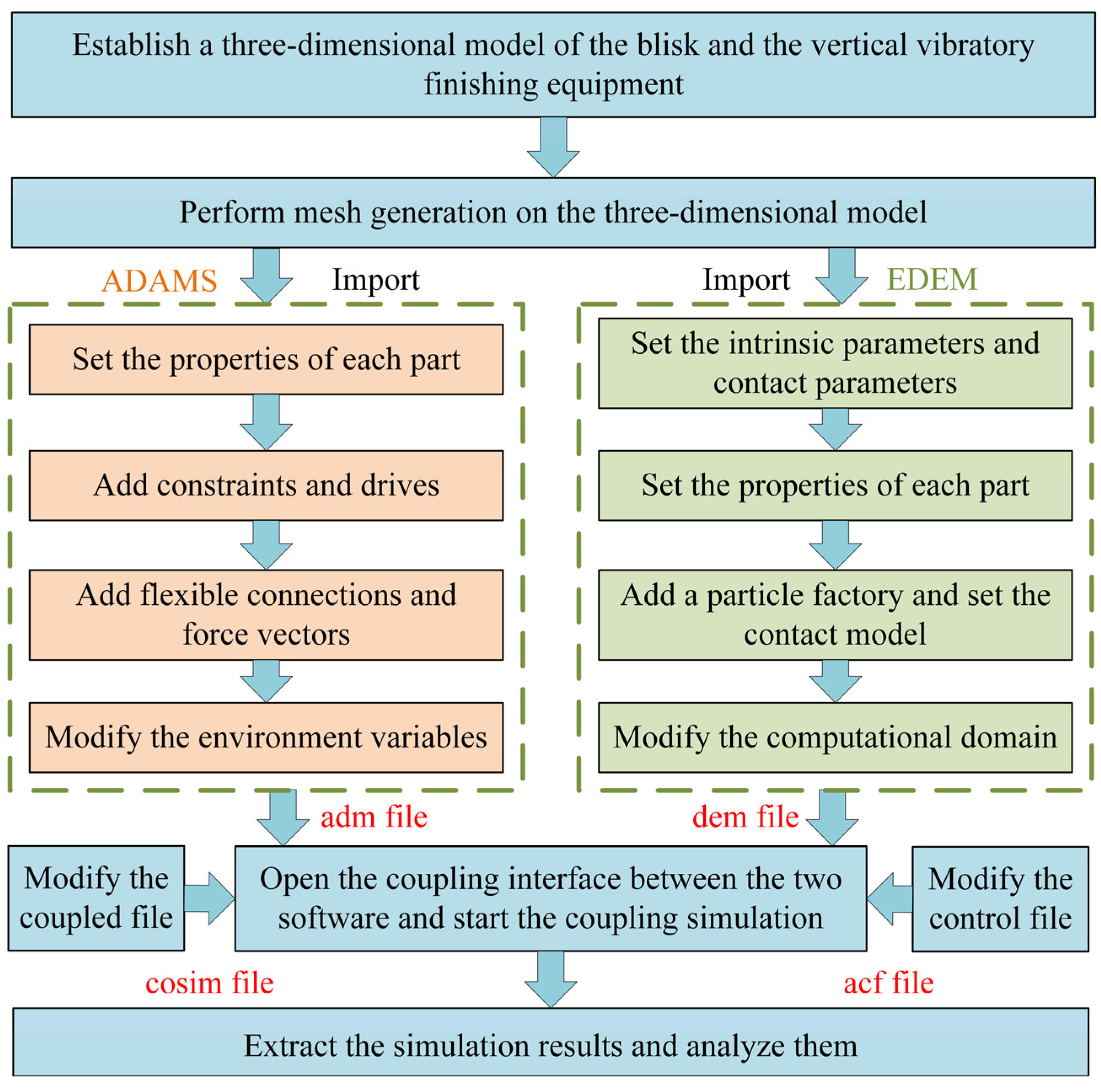
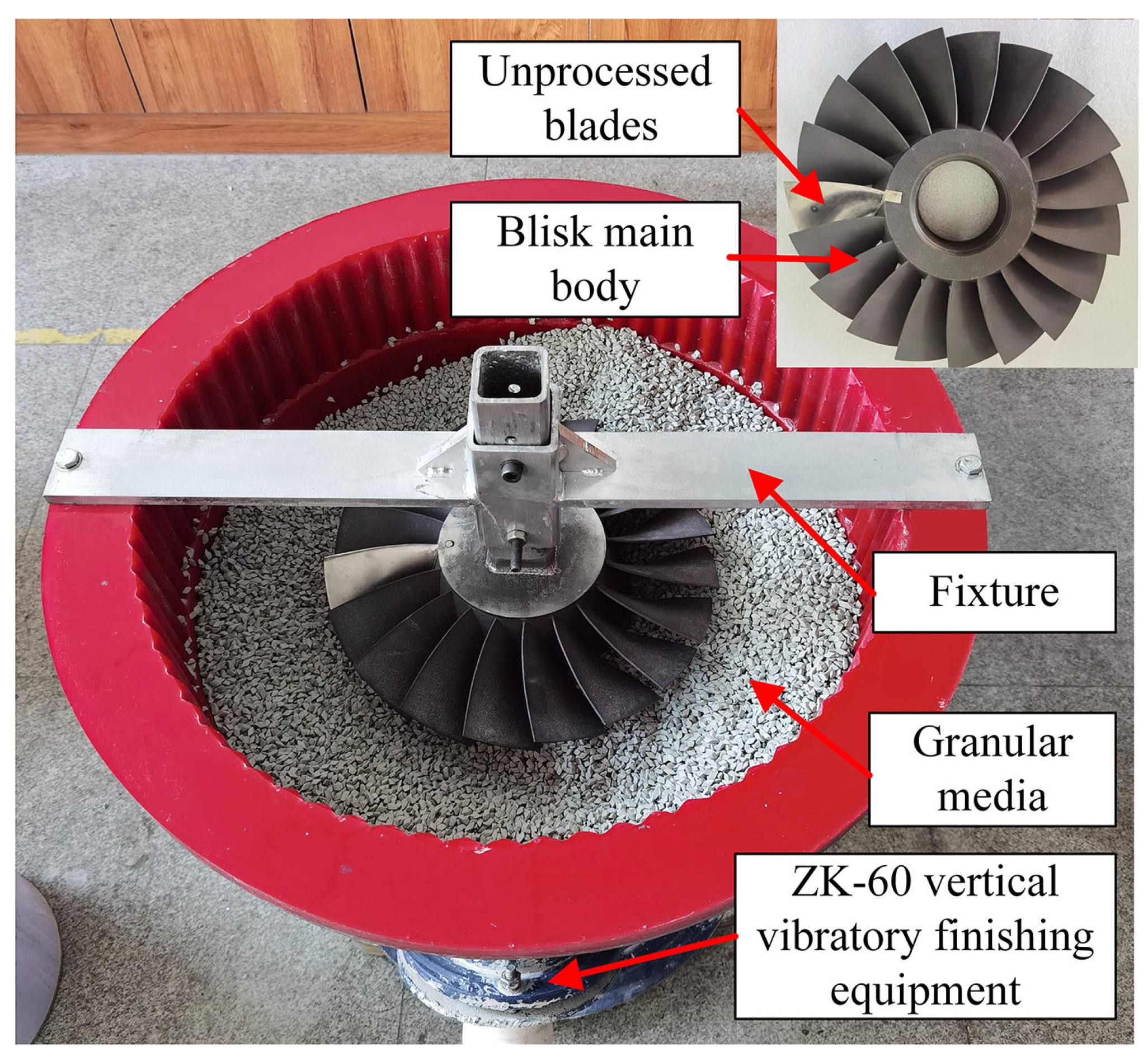
2.2. Experimental Device and Testing Method
3. The Construction of the Theoretical Model of Surface Roughness
- There is a limit value for the surface roughness of the parts in vibratory finishing.
- The greater the difference between the surface roughness and the limit value of the surface roughness, the faster the change rate of the roughness.
- Vibratory finishing has a constant material removal rate during the steady-state process.
3.1. The Steady-State Stage
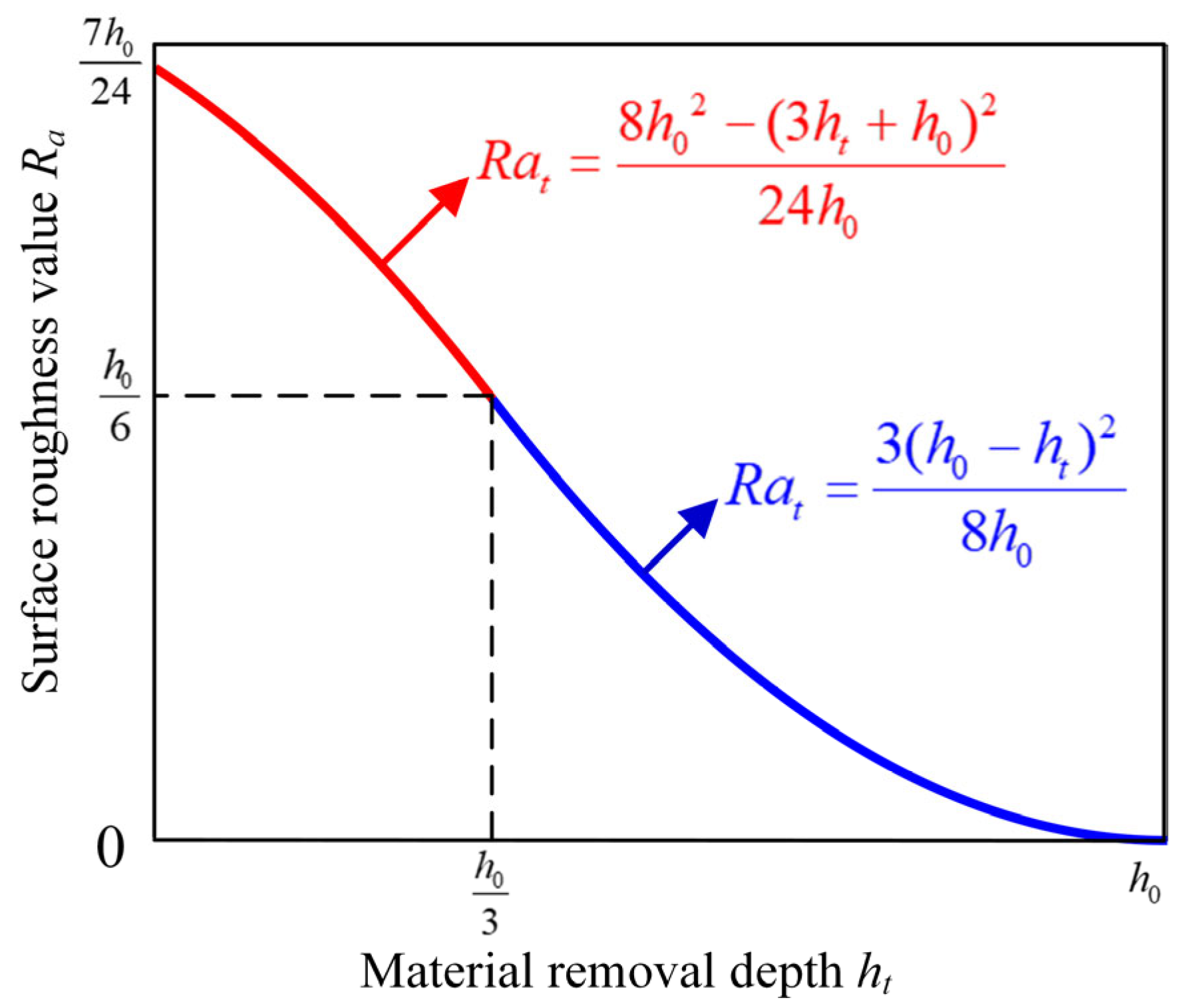
3.2. The Transient Stage Model
3.3. Surface Roughness Model
4. The Verification of the Surface Roughness Model
4.1. Solution of Model Coefficients
4.2. Model Validation
5. Conclusions
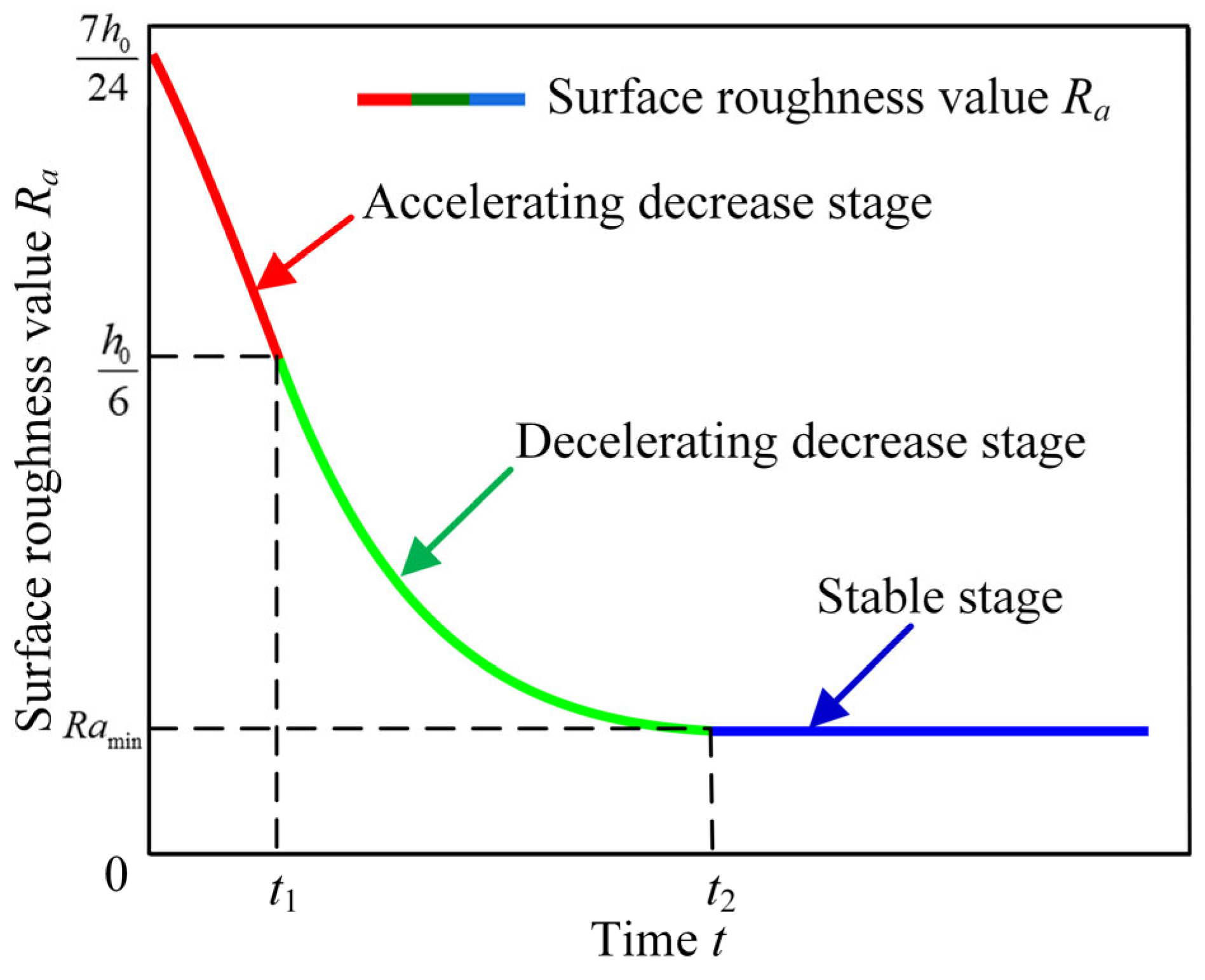
- The change in the surface roughness during the vertical vibratory finishing and grinding of the blisk is divided into a transient stage and a steady-state stage. In the transient stage, as the depth of the material removal increases, the surface roughness decreases first at an accelerating rate and then at a decelerating rate. When the depth of the material removal is h0/3, the rate of the decrease in the surface roughness is the fastest. In the steady-state stage, the depth of the material removal increases linearly, and the surface roughness value does not change.
- In order to improve the accuracy of the prediction model, a simplified two-dimensional surface profile of a trapezoidal array is established based on the initial surface profile of the blisk. The machining marks caused by the granular media on the parts are the main factors affecting the machining limit of the surface roughness. A formula for the influence of scratches is introduced, and a theoretical model of surface roughness is established. The surface roughness value Ra, with the increase in the machining time, can be divided into three stages: the stage of the decreasing growth rate, the stage of decreasing at a decelerated speed, and the stable stage.
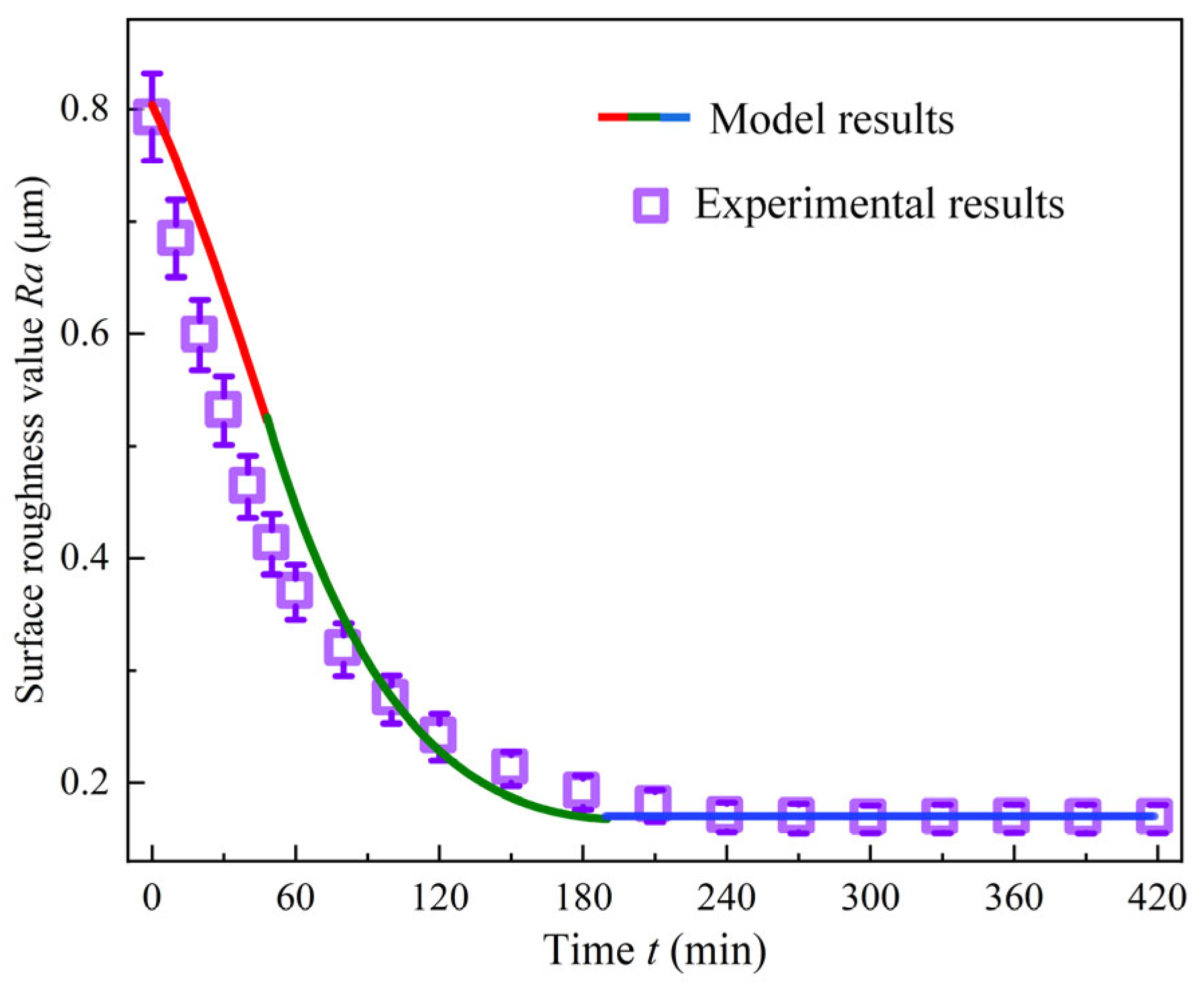
- The vertical vibratory finishing and grinding experiment of the blisk was carried out. In combination with the results of the normal force and relative tangential velocity from the simulation, the steady-state material removal coefficient, ks = 3.37 × 10−5, and the transient material removal coefficient, kt = 1.35 × 10−4, were solved. As the machining time increases, the results of the prediction model for the vertical vibratory finishing and grinding of the blisk and the experimental results both decrease. The average error between the two is 8.40%, which verifies the correctness of the model.
- The normal force acting on the blade tip area of the blade is greater than that on the blade root area, and the relative tangential velocity between the edge area and the granular media is greater than that in the profile area. After 100 min of machining, the model results are 0.169~0.472 μm, and the experimental results are 0.162~0.493 μm. The average error between the two is 11.8%, which further demonstrates the effectiveness of the constructed surface roughness model.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Luo, S.; Liao, L.; Wang, Y. A Novel Process of Chemical-Mechanical Fluid-Solid Coupling Polishing with High-Quality and High-Efficiency for Turbine Blisk. J. Manuf. Process. 2024, 131, 494–506. [Google Scholar] [CrossRef]
- Uluğ, D.; Katmer, Ş.; Yavaş, Ç.; Şeker, U.; Kurt, M. Effects of Cutting Parameters in Fine Milling Processes of Blisk. Int. J. Precis. Eng. Manuf. 2024, 26, 905–918. [Google Scholar] [CrossRef]
- Ye, H.; Chen, Z.; Si, C.; Fang, Z. A Novel Shape-Curved Tool Design Aiming at High-Quality Blisk Root-Fillet Surface Polishing. J. Manuf. Process. 2024, 127, 304–313. [Google Scholar] [CrossRef]
- Gillespie, L.K. Mass Finishing Handbook, 1st ed.; Industrial Press: New York, NY, USA, 2007; ISBN 978-0-8311-3257-6. [Google Scholar]
- Da Silva Maciel, L.; Spelt, J.K. Measurements of Wall-Media Contact Forces and Work in a Vibratory Finisher. Powder Technol. 2020, 360, 911–920. [Google Scholar] [CrossRef]
- Wang, J.; Xu, Z.; Zhu, D. Improving Profile Accuracy and Surface Quality of Blisk by Electrochemical Machining with a Micro Inter-Electrode Gap. Chin. J. Aeronaut. 2023, 36, 523–537. [Google Scholar] [CrossRef]
- Kang, Y.S.; Hashimoto, F.; Johnson, S.P.; Rhodes, J.P. Discrete Element Modeling of 3D Media Motion in Vibratory Finishing Process. CIRP Ann. 2017, 66, 313–316. [Google Scholar] [CrossRef]
- Zhang, C.; Liu, W.; Wang, S.; Liu, Z.; Morgan, M.; Liu, X. Dynamic Modeling and Trajectory Measurement on Vibratory Finishing. Int. J. Adv. Manuf. Technol. 2020, 106, 253–263. [Google Scholar] [CrossRef]
- Yabuki, A.; Baghbanan, M.R.; Spelt, J.K. Contact Forces and Mechanisms in a Vibratory Finisher. Wear 2002, 252, 635–643. [Google Scholar] [CrossRef]
- Wang, J.; Li, X.; Li, W.; Yang, S. Research of Horizontal Vibratory Finishing for Aero-Engine Blades: Movement Characteristics and Action Behavior of Media. Int. J. Adv. Manuf. Technol. 2023, 126, 2065–2081. [Google Scholar] [CrossRef]
- Han, J.; Zhu, J.; Zheng, W.; Wang, G. Influence of Metal Forming Parameters on Surface Roughness and Establishment of Surface Roughness Prediction Model. Int. J. Mech. Sci. 2019, 163, 105093. [Google Scholar] [CrossRef]
- Otsuki, T.; Okita, K.; Sasahara, H. Evaluating Surface Quality by Luminance and Surface Roughness. Precis. Eng. 2022, 74, 147–162. [Google Scholar] [CrossRef]
- Xia, Z.; Wu, D.; Zhang, X.; Wang, J.; Han, E.-H. Rolling Contact Fatigue Failure Mechanism of Bearing Steel on Different Surface Roughness Levels under Heavy Load. Int. J. Fatigue 2024, 179, 108042. [Google Scholar] [CrossRef]
- Hao, Y.; Yang, S.; Chen, H.; Li, X. Evolution Mechanism of the Rough Surface in Vibratory Finishing within Restricted Space. Mater. Manuf. Process. 2024, 39, 825–833. [Google Scholar] [CrossRef]
- Deng, J.; Zhang, Q.; Lu, J.; Yan, Q.; Pan, J.; Chen, R. Prediction of the Surface Roughness and Material Removal Rate in Chemical Mechanical Polishing of Single-Crystal SiC via a Back-Propagation Neural Network. Precis. Eng. 2021, 72, 102–110. [Google Scholar] [CrossRef]
- Yang, K.; Huang, N.; Di, H.; Zhou, P. Modeling of Surface Microtopography Evolution in Chemical Mechanical Polishing Considering Chemical-Mechanical Synergy. Tribol. Int. 2025, 201, 110206. [Google Scholar] [CrossRef]
- Peng, W.; Jiang, L.; Huang, C.; Chen, Y.; Tian, Y.; Han, Y.; Zhang, S.; Qian, L. Surface Roughness Evolution Law in Full-Aperture Chemical Mechanical Polishing. Int. J. Mech. Sci. 2024, 277, 109387. [Google Scholar] [CrossRef]
- Liu, G.; Xu, W.; Zhou, Q. DEM Contact Model for Spherical and Polyhedral Particles Based on Energy Conservation. Comput. Geotech. 2023, 153, 105072. [Google Scholar] [CrossRef]
- Wang, J.; Li, X.; Li, W.; Cheng, S.; Li, H.; Yang, S. Convection and Motion Characteristics of Granular Media in Horizontal Vibratory Finishing. Granul. Matter 2023, 25, 76. [Google Scholar] [CrossRef]
- Li, X.; Li, Z.; He, D.; Ren, J.; Feng, Q.; Tang, K. Efficient Machining of a Complex Blisk Channel Using a Disc Cutter. Chin. J. Aeronaut. 2024, 37, 414–437. [Google Scholar] [CrossRef]
- Tu, M.; Yang, J.; Qi, Q.; Ding, H. Efficient Construction of an Interference-Free Region and Tool Orientation Planning for the Robotic Grinding of Blisks. J. Manuf. Process. 2024, 131, 356–368. [Google Scholar] [CrossRef]
- Hashinioto, F.; DeBra, D. Modelling and Optimization of Vibratory Finishing Process. Ann. ClRP 1996, 45, 303–306. [Google Scholar] [CrossRef]
- Archard, J. Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Misra, A.; Pandey, P.; Dixit, U. Modeling of Material Removal in Ultrasonic Assisted Magnetic Abrasive Finishing Process. Int. J. Mech. Sci. 2017, 131, 853–867. [Google Scholar] [CrossRef]
- Wan, S.; Liu, Y.; Woon, K.S.; Tnay, G.L. A Material Removal and Surface Roughness Evolution Model for Loose Abrasive Polishing of Free Form Surfaces. IJAT 2014, 6, 269. [Google Scholar] [CrossRef]
- Preston, F. The Theory and Design of Plate Galss Polishing Machines. J. Soc. Glass Technol. 1927, 11, 214–256. [Google Scholar]
- Hashimoto, F.; Johnson, S.P. Modeling of Vibratory Finishing Machines. CIRP Ann. 2015, 64, 345–348. [Google Scholar] [CrossRef]
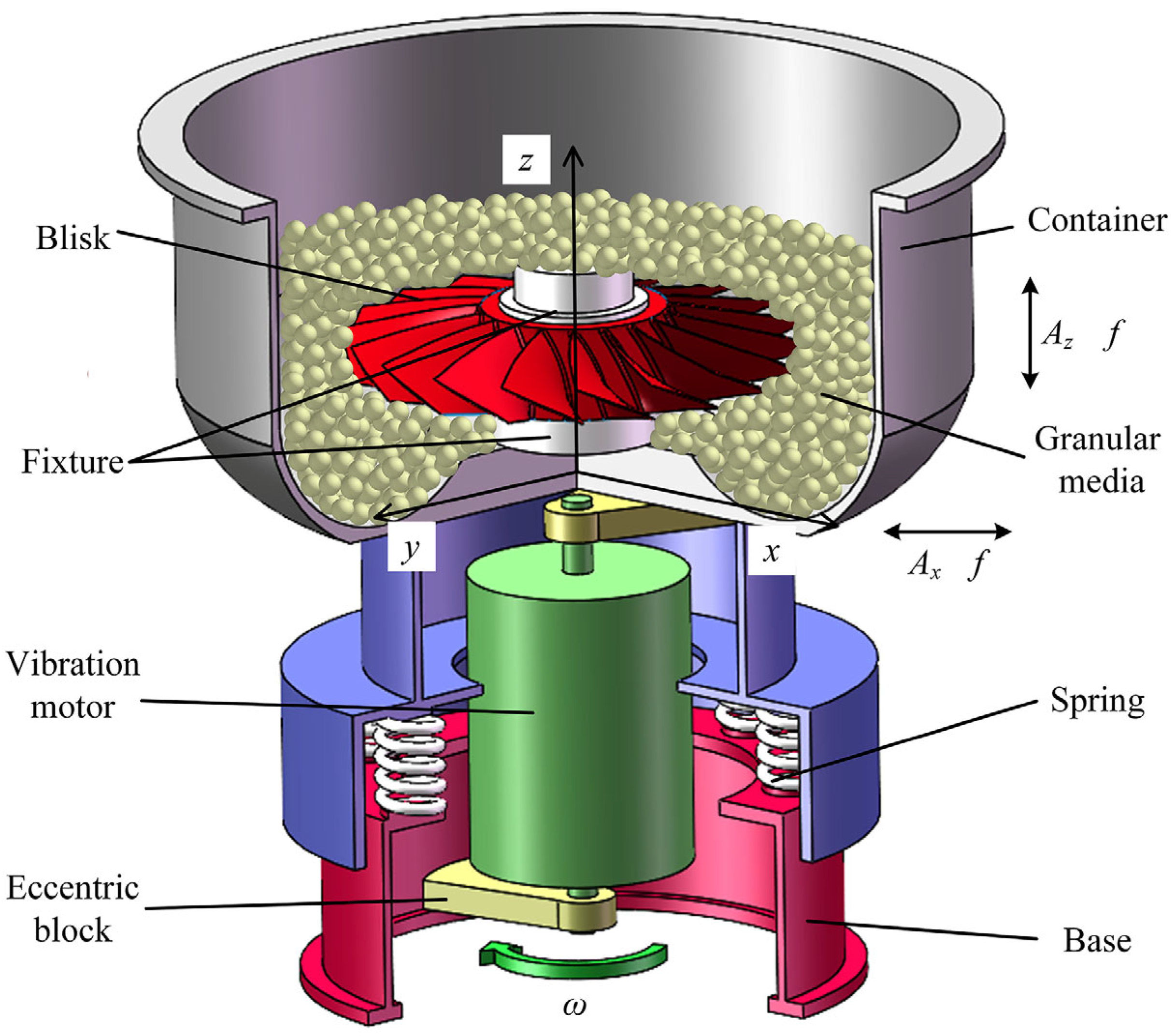





| Conditions | Parameters |
|---|---|
| Motor rotational speed (r/min) | 1440 |
| Vibratory body mass (kg) | 165 |
| Spring horizontal stiffness coefficient (N/m) | 22,450 |
| Spring vertical stiffness coefficient (N/m) | 44,630 |
| Spring horizontal damping coefficient (Ns/m) | 34 |
| Spring vertical damping coefficient (Ns/m) | 75 |
| Eccentric block mass (kg) | 1.4 |
| Eccentric block angle (°) | 90 |
| Material Parameters | Density (kg·m⁻³) | Poisson’s Ratio | Shear Modulus (Pa) |
|---|---|---|---|
| Container | 2675 | 0.28 | 1.24 × 1011 |
| Particles | 1150 | 0.21 | 3.2 × 109 |
| Blisk | 4500 | 0.33 | 4.5 × 1010 |
| Interaction | Coefficient of Restitution for Collision | Coefficient of Static Friction | Coefficient of Rolling Friction |
|---|---|---|---|
| Particle–particle | 0.75 | 0.3 | 0.03 |
| Particle–container | 0.50 | 0.26 | 0.10 |
| Particle–blisk | 0.75 | 0.30 | 0.05 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Zhang, Y.; Zhang, L.; Li, W.; Li, X.; Shan, K. The Development and Experimental Validation of a Surface Roughness Prediction Model for the Vertical Vibratory Finishing of Blisks. Coatings 2025, 15, 634. https://doi.org/10.3390/coatings15060634
Zhang Y, Zhang Y, Zhang L, Li W, Li X, Shan K. The Development and Experimental Validation of a Surface Roughness Prediction Model for the Vertical Vibratory Finishing of Blisks. Coatings. 2025; 15(6):634. https://doi.org/10.3390/coatings15060634
Chicago/Turabian StyleZhang, Yan, Yashuang Zhang, Liaoyuan Zhang, Wenhui Li, Xiuhong Li, and Kun Shan. 2025. "The Development and Experimental Validation of a Surface Roughness Prediction Model for the Vertical Vibratory Finishing of Blisks" Coatings 15, no. 6: 634. https://doi.org/10.3390/coatings15060634
APA StyleZhang, Y., Zhang, Y., Zhang, L., Li, W., Li, X., & Shan, K. (2025). The Development and Experimental Validation of a Surface Roughness Prediction Model for the Vertical Vibratory Finishing of Blisks. Coatings, 15(6), 634. https://doi.org/10.3390/coatings15060634






