Dual Modulation Polarization-Independent Terahertz BIC Metasurface for Multi-Wavelength Sensing
Abstract
1. Introduction
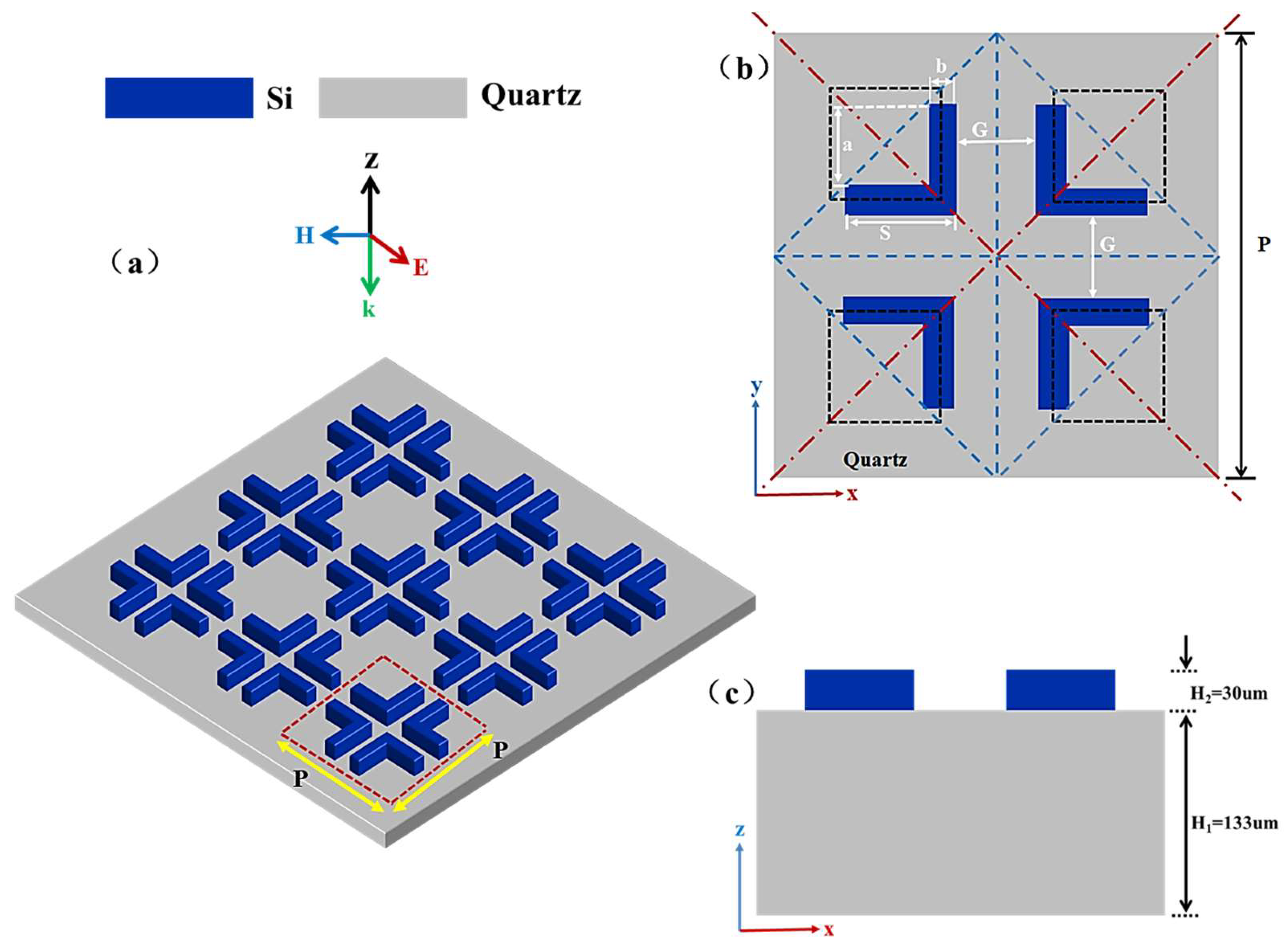
2. Model and Method
3. Results and Discussion
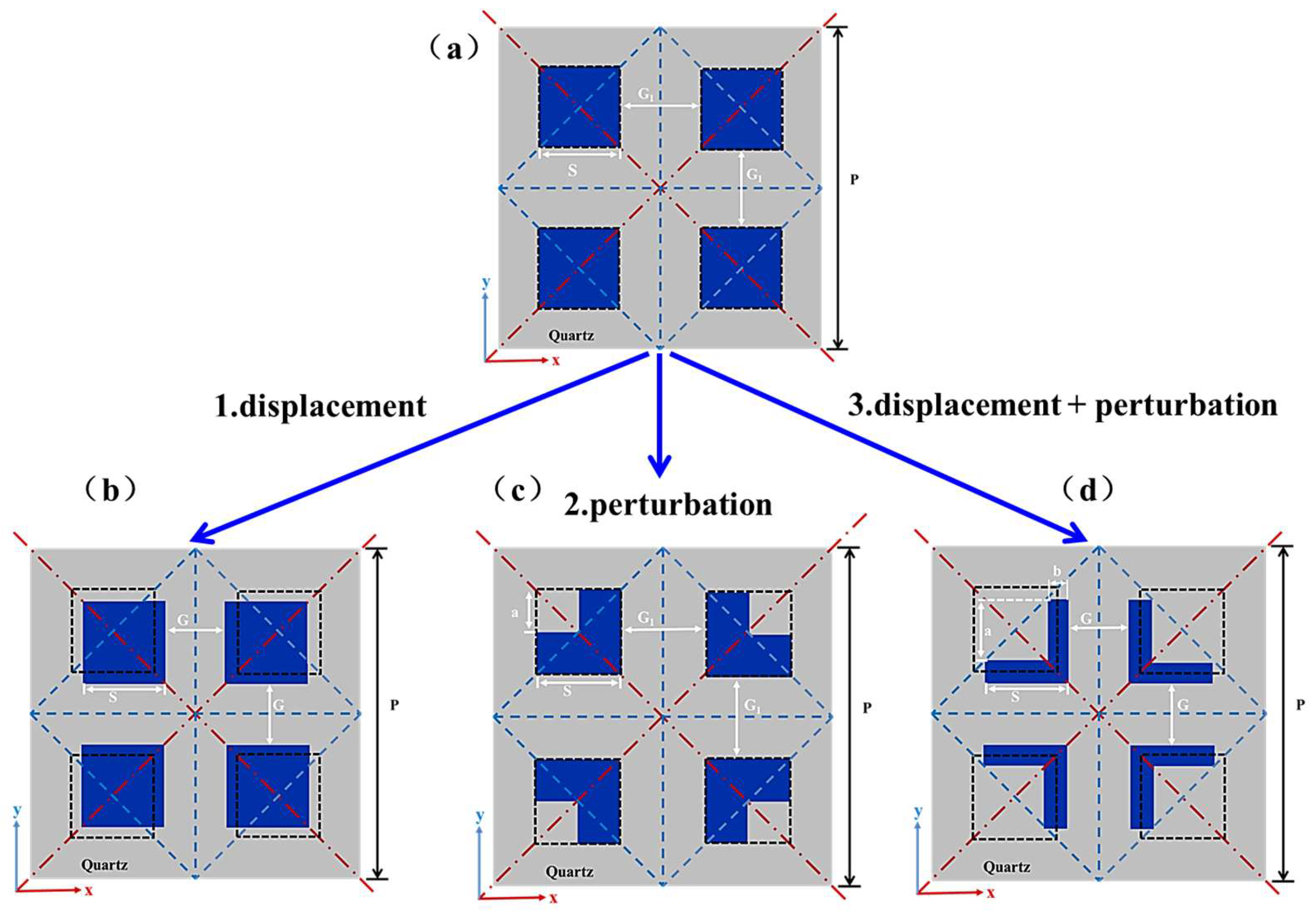
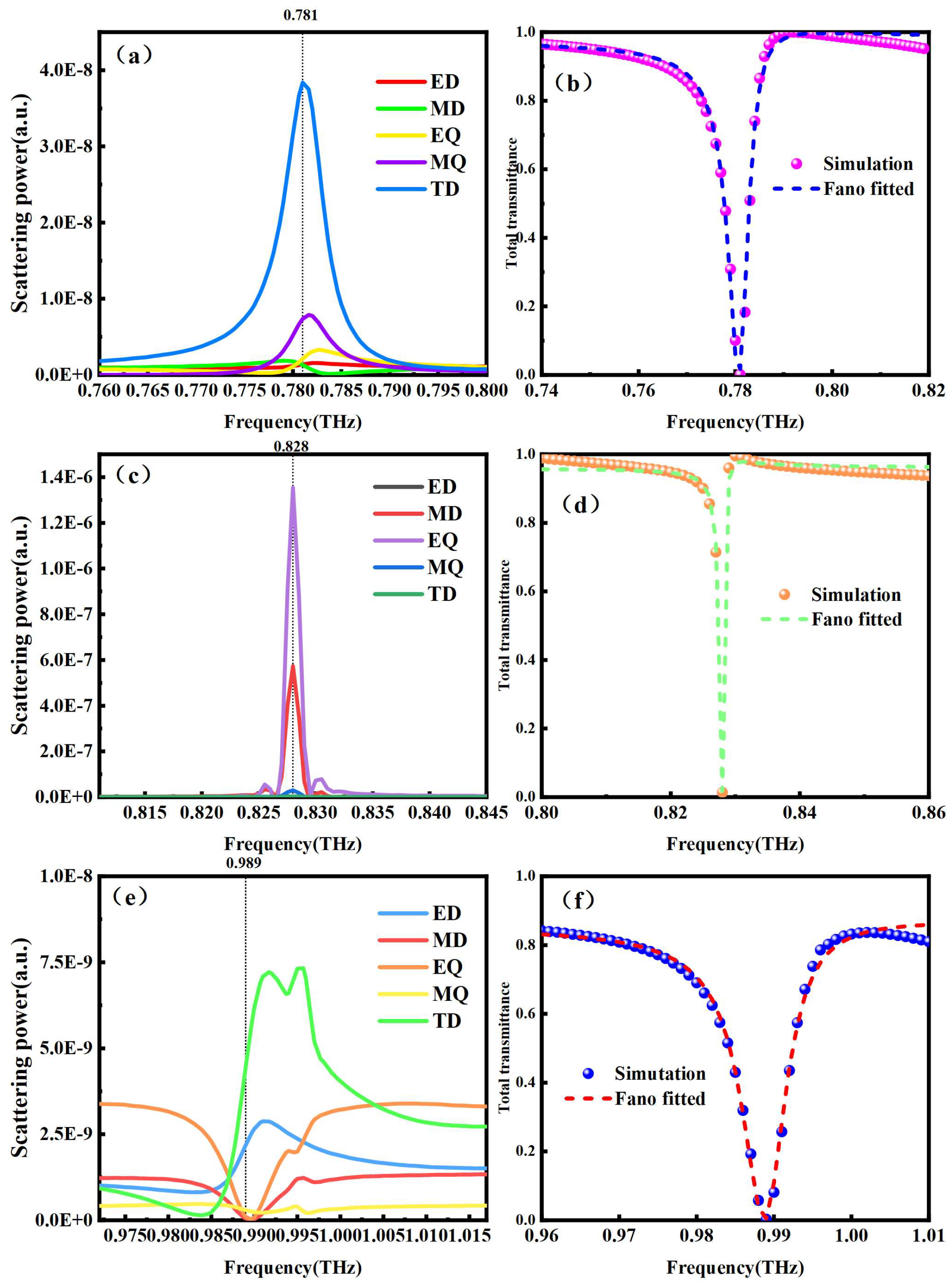
3.1. The Generation of Tri-Band Quasi-BICs
3.1.1. Characteristics of the Shift from BICs to Tri-Band Quasi-BICs Produced by Particular Displacements
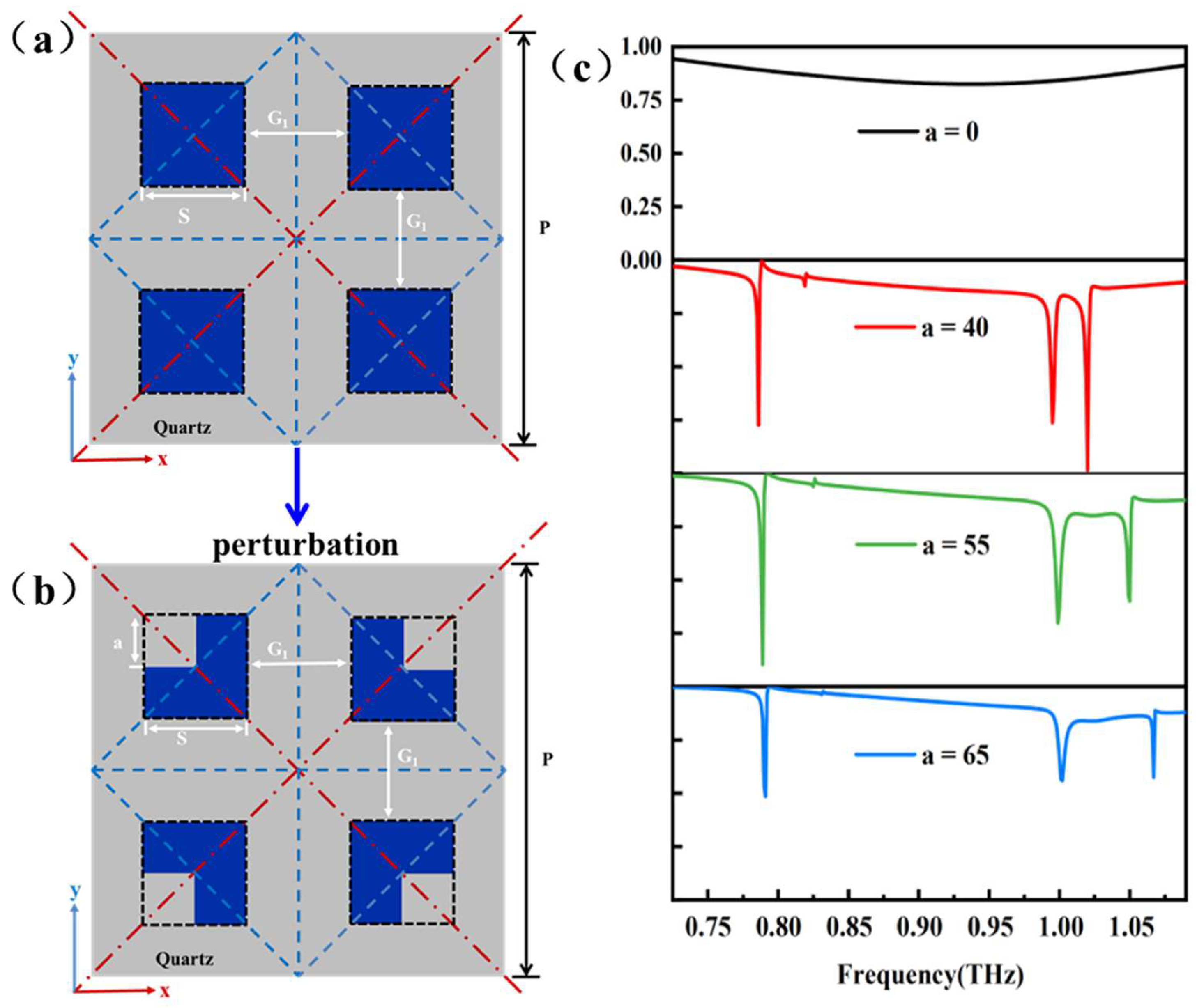
3.1.2. Characteristics of Transformation from BICs to Tri-Band Quasi-BICs Caused by Collective Perturbation
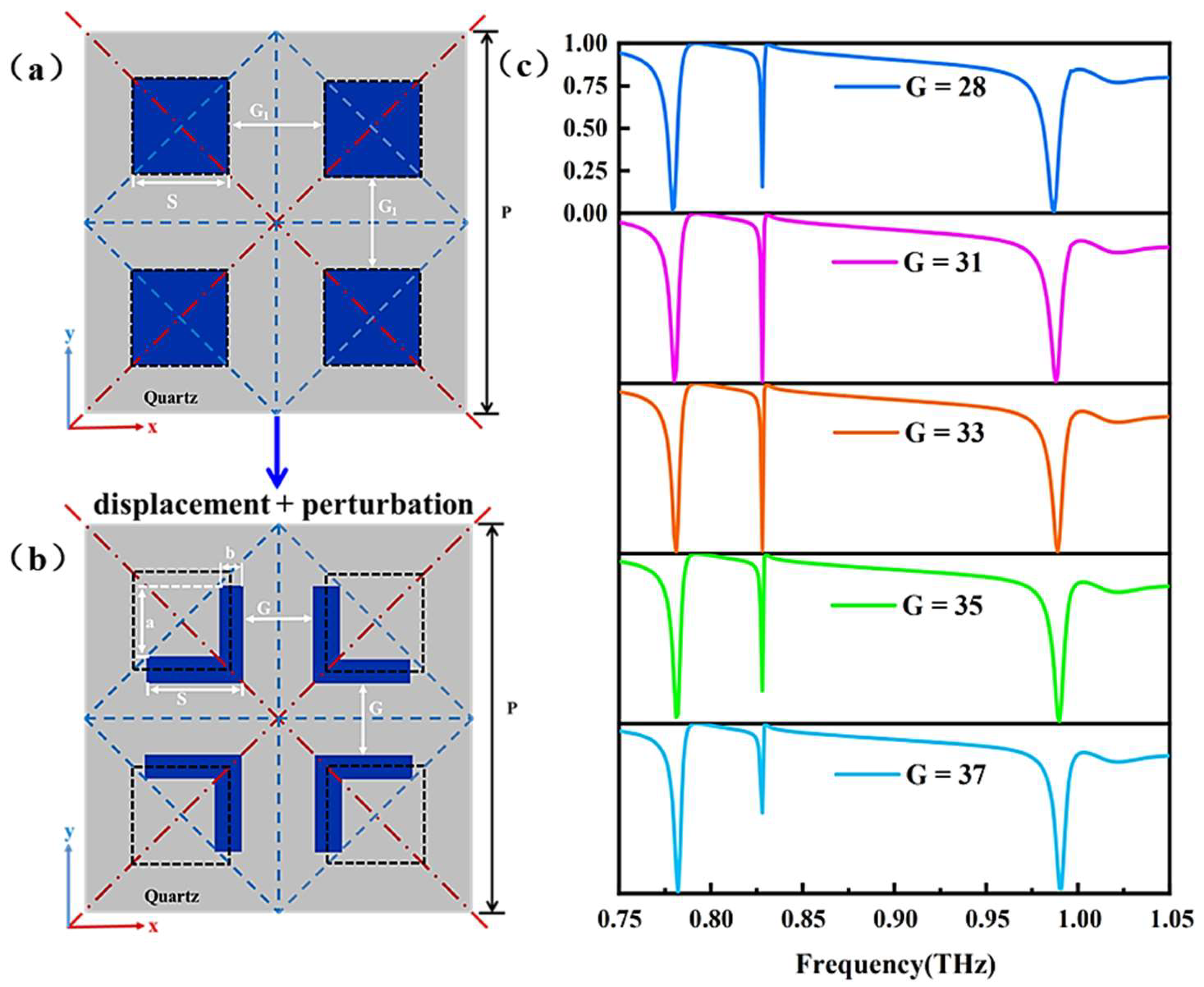
3.1.3. Tri-Band Quasi-BICs Generated by the Combination of Particular Displacement and Collective Perturbation
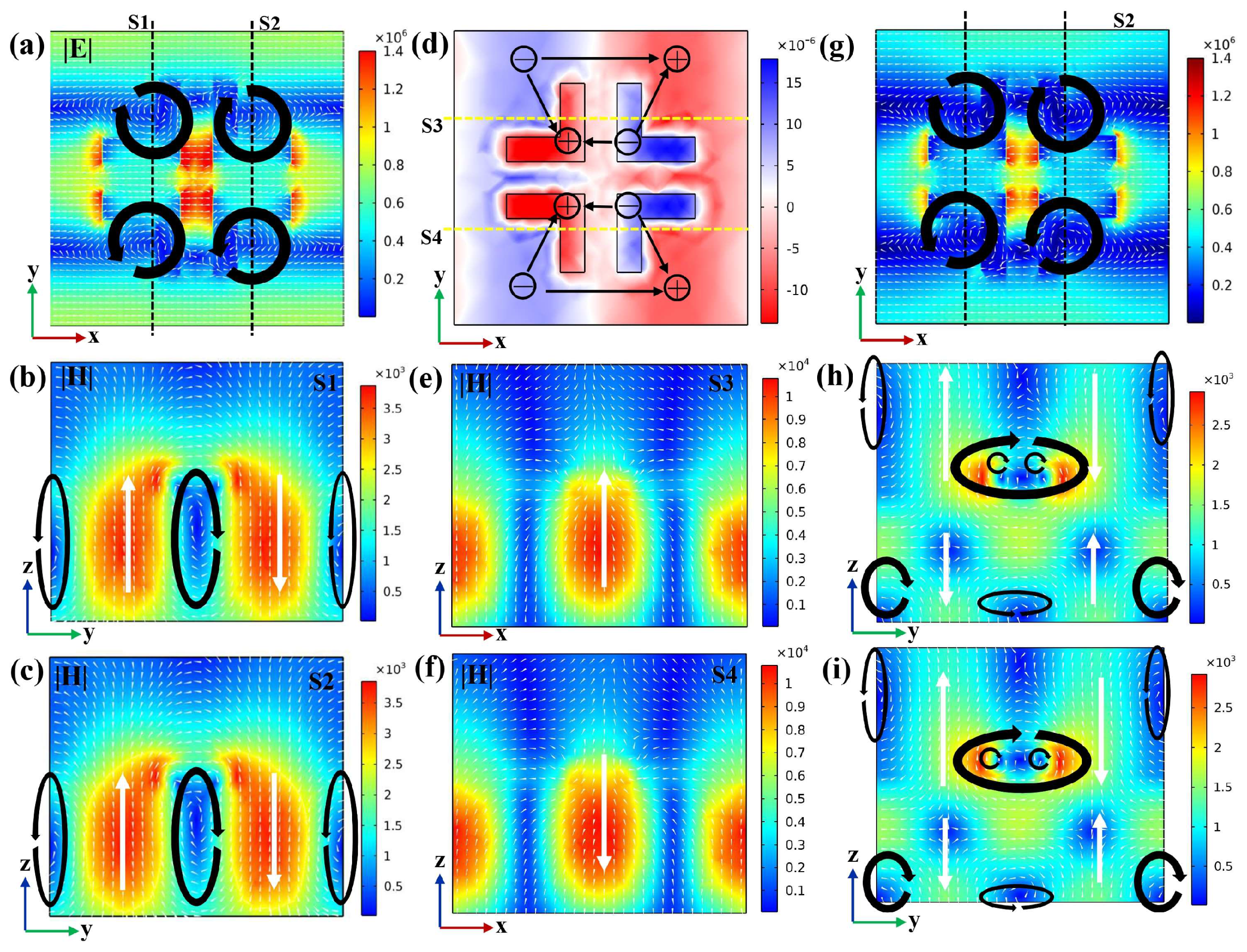
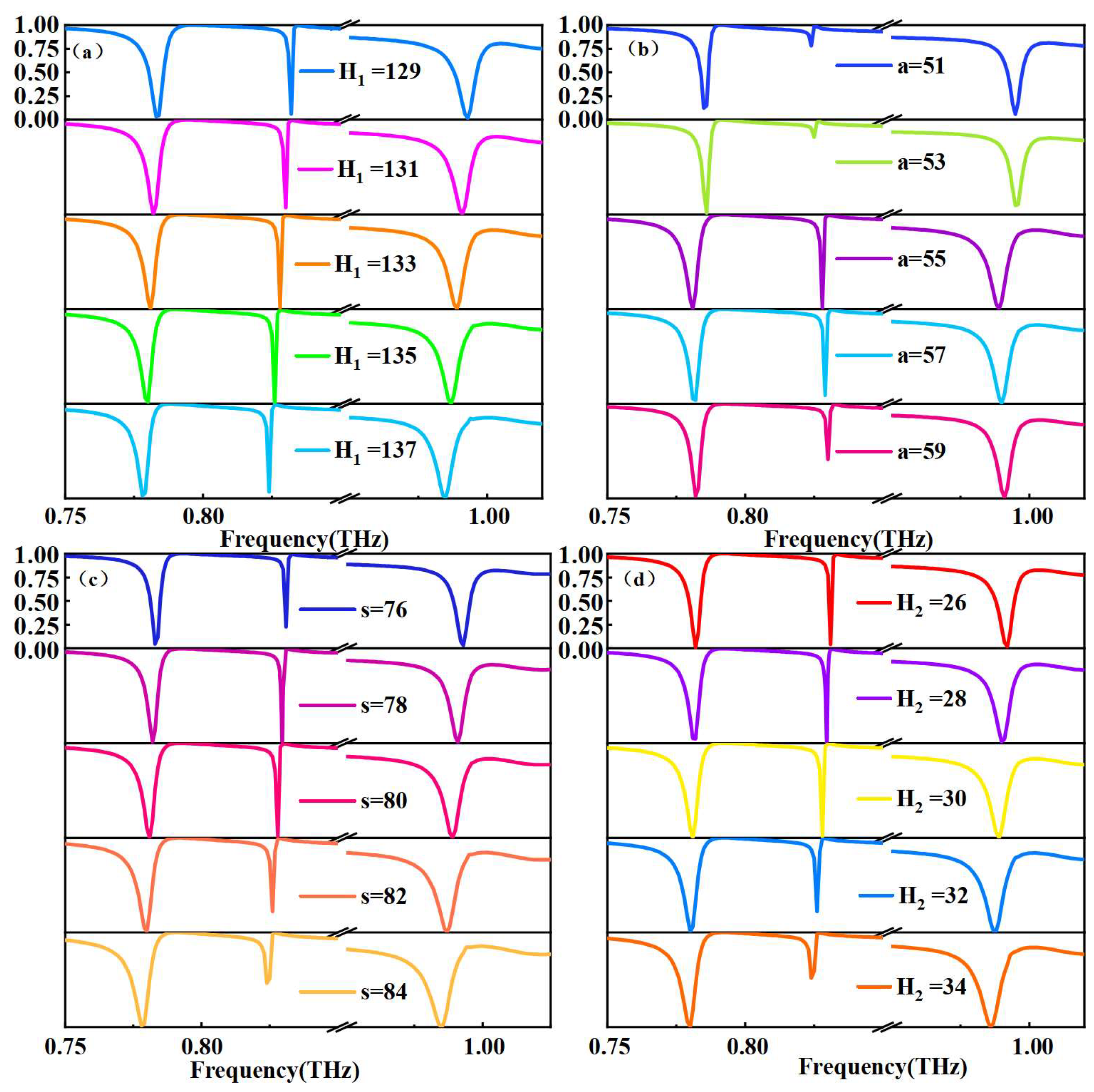
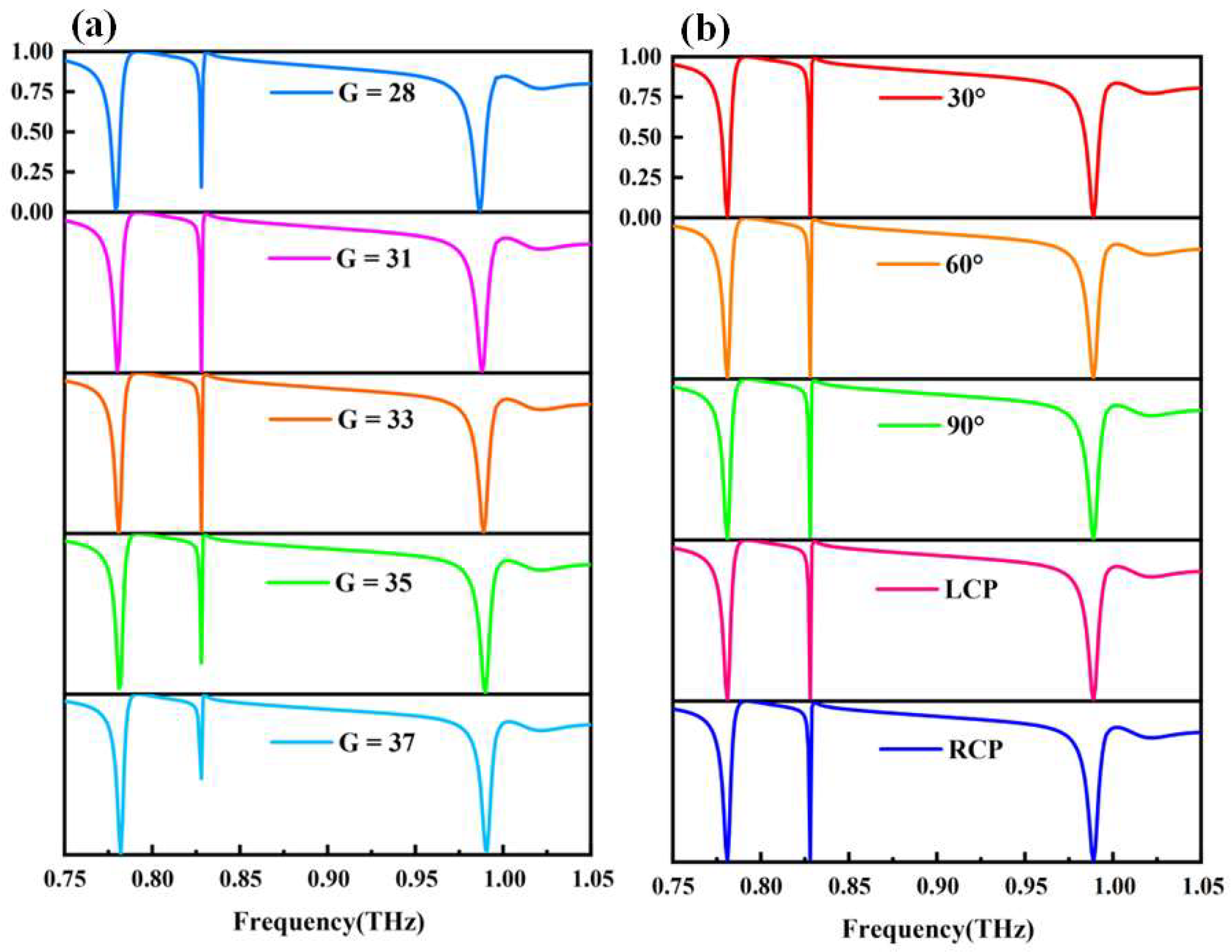
3.2. Mechanisms: Near-Field Properties and Effects of Structural Parameters
3.3. Application of L-Type Metasurface
3.3.1. Tri-Band Quasi-BICs in Terahertz Biosensing
3.3.2. Polarization Characteristics of Tri-Band Quasi-BICs
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, R.; Xu, L.; Huang, L.; Zhang, X.; Ruan, H.; Yang, X.; Lou, J.; Chang, C.; Du, X. Ultrasensitive Terahertz Biodetection Enabled by Quasi-BIC-Based Metasensors. Small 2023, 19, 2301165. [Google Scholar]
- Sun, W.; Liang, Z.; Shi, X.; Yang, F.; Dong, Y.; Dai, R.; Jia, Y.; Xin, W.; Hou, E.; Wu, Z. Potential of high Q dual band Mid-Infrared metasurfaces with Quasi-BIC for refractive index sensing. Opt. Laser Technol. 2024, 174, 110631. [Google Scholar] [CrossRef]
- Chen, X.; Fan, W.; Jiang, X.; Yan, H. High-Q toroidal dipole metasurfaces driven by bound states in the continuum for ultra-sensitive terahertz sensing. J. Light. Technol. 2021, 40, 2181–2190. [Google Scholar] [CrossRef]
- Algorri, J.; Dmitriev, V.; Hernández-Figueroa, H.; Rodríguez-Cobo, L.; Dell’olio, F.; Cusano, A.; López-Higuera, J.; Zografopoulos, D. Polarization-independent hollow nanocuboid metasurfaces with robust quasi-bound states in the continuum. Opt. Mater. 2023, 147, 114631. [Google Scholar] [CrossRef]
- Algorri, J.; Dell’olio, F.; Ding, Y.; Labbé, F.; Dmitriev, V.; López-Higuera, J.; Sánchez-Pena, J.; Andreani, L.; Galli, M.; Zografopoulos, D. Experimental demonstration of a silicon-slot quasi-bound state in the continuum in near-infrared all-dielectric metasurfaces. Opt. Laser Technol. 2023, 161, 109199. [Google Scholar] [CrossRef]
- Hsiao, H.H.; Hsu, Y.C.; Liu, A.Y.; Hsieh, J.C.; Lin, Y.H. Ultrasensitive refractive index sensing based on the quasi-bound states in the con-tinuum of all-dielectric metasurfaces. Adv. Opt. Mater 2022, 10, 2200812. [Google Scholar]
- Srivastava, Y.K.; Ako, R.T.; Gupta, M.; Bhaskaran, M.; Sriram, S.; Singh, R. Terahertz sensing of 7 nm dielectric film with bound states in the continuum metasurfaces. Appl. Phys. Lett. 2019, 115, 151105. [Google Scholar] [CrossRef]
- Chen, X.; Fan, W.-H.; Yan, H. Toroidal dipole bound states in the continuum metasurfaces for terahertz nanofilm sensing. Opt. Express 2020, 28, 17102–17112. [Google Scholar] [CrossRef]
- Ouimet, M.; Cortés, J. Collective estimation of ocean nonlinear internal waves using robotic underwater drifters. IEEE Access 2013, 1, 418–427. [Google Scholar] [CrossRef]
- Wang, T.; Liu, S.; Zhang, J.; Xu, L.; Yang, M.; Ma, D.; Jiang, S.; Jiao, Q.; Tan, X. Dual high-Q Fano resonances metasurfaces excited by asymmetric dielectric rods for refractive index sensing. Nanophotonics 2024, 13, 463–475. [Google Scholar] [CrossRef]
- Lin, H.; Ye, X.; Chen, X.; Zhou, Z.; Yi, Z.; Niu, G.; Yi, Y.; Hua, Y.; Hua, J.; Xiao, S. Plasmonic absorption enhancement in graphene circular and elliptical disk arrays. Mater. Res. Express 2019, 6, 045807. [Google Scholar] [CrossRef]
- Cong, L.; Singh, R. Symmetry-protected dual bound states in the continuum in metamaterials. Adv. Opt. Mater. 2019, 7, 1900383. [Google Scholar] [CrossRef]
- Wu, C.; Arju, N.; Kelp, G.; Fan, J.A.; Dominguez, J.; Gonzales, E.; Tutuc, E.; Brener, I.; Shvets, G. Spectrally selective chiral silicon metasurfaces based on infrared Fano resonances. Nat. Commun. 2014, 5, 3892. [Google Scholar] [CrossRef] [PubMed]
- Koshelev, K.; Lepeshov, S.; Liu, M.; Bogdanov, A.; Kivshar, Y. Asymmetric metasurfaces with high-Q resonances governed by bound states in the continuum. Phys. Rev. Lett. 2018, 121, 193903. [Google Scholar] [CrossRef]
- Wang, M.; Li, B.; Wang, W. Symmetry-protected dual quasi-bound states in the continuum with high tunability in metasur-face. J. Opt. 2020, 22, 125102. [Google Scholar] [CrossRef]
- Kupriianov, A.S.; Xu, Y.; Sayanskiy, A.; Dmitriev, V.; Kivshar, Y.S.; Tuz, V.R. Metasurface engineering through bound states in the continuum. Phys. Rev. Appl. 2019, 12, 014024. [Google Scholar] [CrossRef]
- Zhou, C.; Pu, T.; Huang, J.; Fan, M.; Huang, L. Manipulating optical scattering of quasi-bic in dielectric metasurface with off-center hole. Nanomaterials 2021, 12, 54. [Google Scholar] [CrossRef]
- Chen, X.; Fan, W. Ultrahigh-Q toroidal dipole resonance in all-dielectric metamaterials for terahertz sensing. Opt. Lett. 2019, 44, 5876–5879. [Google Scholar] [CrossRef]
- Meng, B.; Wang, J.; Zhou, C.; Huang, L. Bound states in the continuum supported by silicon oligomer metasurfaces. Opt. Lett. 2022, 47, 1549–1552. [Google Scholar] [CrossRef]
- Chen, Y.; Li, M.; Zhao, M.; Wang, J. Multiple quasibound states in the continuum of permittivity-asymmetric all-dielectric metasurface: Group-theoretical description. Opt. Mater. 2023, 138, 113693. [Google Scholar] [CrossRef]
- Kühne, J.; Wang, J.; Weber, T.; Kühner, L.; Maier, S.A.; Tittl, A. Fabrication robustness in BIC metasurfaces. Nanophotonics 2021, 10, 4305–4312. [Google Scholar] [CrossRef]
- Shi, T.; Deng, Z.-L.; Tu, Q.-A.; Cao, Y.; Li, X. Displacement-mediated bound states in the continuum in all-dielectric superlattice metasurfaces. PhotoniX 2021, 2, 7. [Google Scholar] [CrossRef]
- van Hoof, N.J.; Abujetas, D.R.; ter Huurne, S.E.; Verdelli, F.; Timmermans, G.C.; Sánchez-Gil, J.A.; Rivas, J.G. Unveiling the symmetry protection of bound states in the continuum with terahertz near-field imaging. ACS Photonics 2021, 8, 3010–3016. [Google Scholar] [CrossRef] [PubMed]
- Biswas, S.K.; Adi, W.; Beisenova, A.; Rosas, S.; Arvelo, E.R.; Yesilkoy, F. From weak to strong coupling: Quasi-BIC metasurfaces for mid-infrared light–matter interactions. Nanophotonics 2024, 13, 2937–2949. [Google Scholar] [CrossRef]
- Liu, Q.K.; Luo, X.Q.; Xu, X.; Li, Y.; Zhu, W.; Chen, Z.; Liu, W.M.; Wang, X.L. Interplay of bound states in the continuum empowers spectral-lineshape manipulation in all-dielectric metasurfaces. Phys. Rev. B 2023, 107, 205422. [Google Scholar] [CrossRef]
- Wang, M.; Zhao, X.; Zhao, R.; Lu, G. Dual resonance based on quasi-bound states in continuum in the all-dielectric terahertz metasurface and its application in sensing. Results Phys. 2023, 49, 106518. [Google Scholar] [CrossRef]
- Labbé-Lavigne, S.; Barret, S.; Garet, F.; Duvillaret, L.; Coutaz, J.-L. Far-infrared dielectric constant of porous silicon layers measured by terahertz time-domain spectroscopy. J. Appl. Phys. 1998, 83, 6007–6010. [Google Scholar] [CrossRef]
- Hsiao, H.; Chu, C.H.; Tsai, D.P. Fundamentals and Applications of Metasurfaces. Small Methods 2017, 1, 1600064. [Google Scholar] [CrossRef]
- Hsu, W.L.; Chen, Y.C.; Yeh, S.P.; Zeng, Q.C.; Huang, Y.W.; Wang, C.M. Review of metasurfaces and metadevices: Advantages of different materials and fab-rications. Nanomaterials 2022, 12, 1973. [Google Scholar] [CrossRef]
- Shanei, M.M.; Fathi, D.; Ghasemifard, F.; Quevedo-Teruel, O. All-silicon reconfigurable metasurfaces for multifunction and tunable performance at optical frequencies based on glide symmetry. Sci. Rep. 2019, 9, 13641. [Google Scholar] [CrossRef]
- Han, S.; Cong, L.; Srivastava, Y.K.; Qiang, B.; Rybin, M.V.; Kumar, A.; Jain, R.; Lim, W.X.; Achanta, V.G.; Prabhu, S.S.; et al. All-Dielectric active terahertz photonics driven by bound states in the continuum. Adv. Mater. 2019, 31, e1901921. [Google Scholar] [CrossRef] [PubMed]
- Mahmood, N.; Kim, I.; Mehmood, M.Q.; Jeong, H.; Akbar, A.; Lee, D.; Saleem, M.; Zubair, M.; Anwar, M.S.; Tahir, F.A.; et al. Polarisation insensitive multifunctional metasurfaces based on all-dielectric nanowaveguides. Nanoscale 2018, 10, 18323–18330. [Google Scholar] [CrossRef] [PubMed]
- Qiu, J.; Liu, X.; Liang, Z.; Zhu, J. Ultra-wideband perfect reflection and tunneling by all-dielectric metamaterials. Opt. Lett. 2021, 46, 849–852. [Google Scholar] [CrossRef] [PubMed]
- Luo, J.; Zeng, B.; Wang, C.; Gao, P.; Liu, K.; Pu, M.; Jin, J.; Zhao, Z.; Li, X.; Yu, H.; et al. Fabrication of anisotropically arrayed nano-slots metasurfaces using reflective plasmonic lithography. Nanoscale 2015, 7, 18805–18812. [Google Scholar] [CrossRef]
- Saadabad, R.M.; Huang, L.; Miroshnichenko, A.E. Polarization-independent perfect absorber enabled by quasi-bound states in the continuum. Phys. Rev. B 2021, 104, 235405. [Google Scholar]
- Azzam, S.I.; Kildishev, A.V. Photonic bound states in the continuum: From basics to applications. Adv. Opt. Mater. 2020, 9. [Google Scholar] [CrossRef]
- Hsu, C.W.; Zhen, B.; Stone, A.D.; Joannopoulos, J.D.; Soljačić, M. Bound states in the continuum. Nat. Rev. Mater. 2016, 1, 16048. [Google Scholar] [CrossRef]
- Rybin, M.V.; Koshelev, K.L.; Sadrieva, Z.F.; Samusev, K.B.; Bogdanov, A.A.; Limonov, M.F.; Kivshar, Y.S. High-Q supercavity modes in subwavelength dielectric resonators. Phys. Rev. Lett. 2017, 119, 243901. [Google Scholar]
- Tang, C.; Yan, B.; Wang, Q.; Chen, J.; Yan, Z.; Liu, F.; Chen, N.; Sui, C. Toroidal dipolar excitation in metamaterials consisting of metal nanodisks and a dielectrc spacer on metal substrate. Sci. Rep. 2017, 7, 582. [Google Scholar] [CrossRef]
- Yang, B.; Liu, W.; Li, Z.; Cheng, H.; Choi, D.-Y.; Chen, S.; Tian, J. Ultrahighly saturated structural colors enhanced by multipolar-modulated metasurfaces. Nano Lett. 2019, 19, 4221–4228. [Google Scholar] [CrossRef]
- Prokhorov, A.V.; Gubin, M.Y.; Shesterikov, A.V.; Arsenin, A.V.; Volkov, V.S.; Evlyukhin, A.B. Lasing Effect in Symmetrical van der Waals Heterostructured Metasurfaces Due to Lattice-Induced Multipole Coupling. Nano Lett. 2023, 23, 11105–11111. [Google Scholar] [CrossRef] [PubMed]
- Zhou, C.; Huang, L.; Jin, R.; Xu, L.; Li, G.; Rahmani, M.; Chen, X.; Lu, W.; Miroshnichenko, A.E. Bound states in the continuum in asymmetric dielectric metasurfaces. Laser Photonics Rev. 2022, 17, 2200564. [Google Scholar] [CrossRef]
- Terekhov, P.D.; Babicheva, V.E.; Baryshnikova, K.V.; Shalin, A.S.; Karabchevsky, A.; Evlyukhin, A.B. Multipole analysis of dielectric metasurfaces composed of non-spherical nanoparticles and lattice invisibility effect. Phys. Rev. B 2019, 99, 045424. [Google Scholar]
- Evlyukhin, A.B.; Reinhardt, C.; Seidel, A.; Luk’yanchuk, B.S.; Chichkov, B.N. Optical response features of Si-nanoparticle arrays. Phys. Rev. B 2010, 82, 045404. [Google Scholar] [CrossRef]
- Zhang, D.; Wang, Y.; Zhu, Y.; Cui, Z.; Sun, G.; Zhang, X.; Yao, Z.; Zhang, X.; Zhang, K. Ultra-high Q resonances governed by quasi-bound states in the continuum in all-dielectric THz metamaterials. Opt. Commun. 2022, 520, 128555. [Google Scholar] [CrossRef]
- Wu, J.; Jiang, H.T.; Guo, Z.; Sun, Y.; Li, Y.; Chen, H. Giant optical chirality in dielectric metasurfaces induced by toroidal dipole resonances. Opt. Lett. 2023, 48, 916–919. [Google Scholar] [CrossRef]
- Maleki, J.; Fathi, D. Refractive index sensor based on fano-magnetic toroidal quadrupole resonance enabled by bound state in the continuum in all-dielectric metasurface. Sci. Rep. 2024, 14, 4110. [Google Scholar] [CrossRef]
- Wang, T.; Liu, S.; Zhang, J.; Xu, L.; Yang, M.; Han, B.; Ma, D.; Jiang, S.; Jiao, Q.; Tan, X. Highly sensitive polarization-tunable Fano resonant metasurface excited by BICs for re-fractive index detection. Results Phys. 2024, 58, 107451. [Google Scholar]
- Yanik, A.A.; Cetin, A.E.; Huang, M.; Artar, A.; Mousavi, S.H.; Khanikaev, A.; Connor, J.H.; Shvets, G.; Altug, H. Seeing protein monolayers with naked eye through plasmonic Fano resonances. Proc. Natl. Acad. Sci. USA 2011, 108, 11784–11789. [Google Scholar] [CrossRef]
- Sherry, L.J.; Chang, S.-H.; Schatz, G.C.; Van Duyne, R.P.; Wiley, B.J.; Xia, Y. Localized surface plasmon resonance spectroscopy of single silver nanocubes. Nano Lett. 2005, 5, 2034–2038. [Google Scholar] [CrossRef]
- Pan, W.; Yan, Y.; Ma, Y.; Shen, D. A terahertz metamaterial based on electromagnetically induced transparency effect and its sensing performance. Opt. Commun. 2019, 431, 115–119. [Google Scholar] [CrossRef]
- Yahiaoui, R.; Tan, S.; Cong, L.; Singh, R.; Yan, F.; Zhang, W. Multispectral terahertz sensing with highly flexible ultrathin metamaterial absorber. J. Appl. Phys. 2015, 118, 083103. [Google Scholar] [CrossRef]
- Zhang, Z.; Ding, H.; Yan, X.; Liang, L.; Wei, D.; Wang, M.; Yang, Q.; Yao, J. Sensitive detection of cancer cell apoptosis based on the non-bianisotropic metamaterials biosensors in terahertz frequency. Opt. Mater. Express 2018, 8, 659–667. [Google Scholar] [CrossRef]
- Gupta, M.; Srivastava, Y.K.; Manjappa, M.; Singh, R. Sensing with toroidal metamaterial. Appl. Phys. Lett. 2017, 110, 121108. [Google Scholar] [CrossRef]
- Ma, S.; Li, F.; Su, Y.; Chen, L.; Song, Y.; Ye, J. Detection of benzoic acid additive based on a terahertz metasurface sensor. Photonics 2023, 10, 663. [Google Scholar] [CrossRef]
- Kameshkov, O.; Gerasimov, V.; Kuznetsov, S. Sensing performance analysis of spiral metasurface utilizing phase spectra measurement technique. Photonics 2023, 10, 243. [Google Scholar] [CrossRef]
- Janneh, M.; De Marcellis, A.; Palange, E.; Tenggara, A.T.; Byun, D. Design of a metasurface-based dual-band Terahertz perfect absorber with very high Q-factors for sensing applications. Opt. Commun. 2018, 416, 152–159. [Google Scholar]
- Wang, X.; Li, S.; Zhou, C. Polarization-independent toroidal dipole resonances driven by symmetry-protected BIC in ultra-violet region. Opt. Express 2020, 28, 11983–11989. [Google Scholar]
- Ding, J.; Huang, L.; Luo, Y.; Wang, T.; Hu, J.; Li, R.; Xiao, S. Multi-Band Polarization-Independent Quasi-Bound States in the Continuum Based on Te-tramer-Based Metasurfaces and Their Potential Application in Terahertz Microfluidic Biosensing. Adv. Opt. Mater. 2023, 11, 2300685. [Google Scholar]
- Liu, D.; Yu, X.; Wu, F.; Xiao, S.; Itoigawa, F.; Ono, S. Terahertz high-Q quasi-bound states in the continuum in laser-fabricated metallic double-slit arrays. Opt. Express 2021, 29, 24779–24791. [Google Scholar] [CrossRef]
- Zheng, Z.; Rocco, D.; Ren, H.; Sergaeva, O.; Zhang, Y.; Whaley, K.B.; Ying, C.; de Ceglia, D.; De-Angelis, C.; Rahmani, M.; et al. Advances in nonlinear metasurfaces for imaging, quantum, and sensing applications. Nanophotonics 2023, 12, 4255–4281. [Google Scholar] [CrossRef] [PubMed]
- Zou, X.; Lin, R.; Fu, Y.; Gong, G.; Zhou, X.; Wang, S.; Zhu, S.; Wang, Z. Advanced optical imaging based on metasurfaces. Adv. Opt. Mater. 2023, 12, 2203149. [Google Scholar] [CrossRef]
- Chen, Y.; Gao, J.; Yang, X. Chiral grayscale imaging with plasmonic metasurfaces of stepped nanoapertures. Adv. Opt. Mater. 2019, 7, 201801467. [Google Scholar] [CrossRef]
- Mishra, K.V.; Hodge, J.A.; Zaghloul, A.I. Reconfigurable metasurfaces for radar and communications systems. In Proceedings of the 2019 URSI Asia-Pacific Radio Science Conference (AP-RASC), New Delhi, India, 9–15 March 2019; pp. 1–4. [Google Scholar]
- Zeng, B.; Huang, Z.; Singh, A.; Yao, Y.; Azad, A.K.; Mohite, A.D.; Taylor, A.J.; Smith, D.R.; Chen, H.T. Hybrid graphene metasurfaces for high-speed mid-infrared light modulation and sin-gle-pixel imaging. Light Sci. Appl. 2018, 7, 51. [Google Scholar] [CrossRef]
- Keren-Zur, S.; Tal, M.; Fleischer, S.; Mittleman, D.M.; Ellenbogen, T. Generation of spatiotemporally tailored terahertz wavepackets by nonlinear metasurfaces. Nat. Commun. 2019, 10, 1–6. [Google Scholar] [CrossRef]
- Ahmed, H.; Kim, H.; Zhang, Y.; Intaravanne, Y.; Jang, J.; Rho, J.; Chen, S.; Chen, X. Optical metasurfaces for generating and manipulating optical vortex beams. Nanophotonics 2022, 11, 941–956. [Google Scholar] [CrossRef]
- Huang, Y.-W.; Lee, H.W.H.; Sokhoyan, R.; Pala, R.A.; Thyagarajan, K.; Han, S.; Tsai, D.P.; Atwater, H.A. Gate-tunable conducting oxide metasurfaces. Nano Lett. 2016, 16, 5319–5325. [Google Scholar] [CrossRef]
- Gladyshev, S.; Karamanos, T.D.; Kuhn, L.; Beutel, D.; Weiss, T.; Rockstuhl, C.; Bogdanov, A. Inverse design of all-dielectric metasurfaces with accidental bound states in the continuum. Nanophotonics 2023, 12, 3767–3779. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, Q.; Xing, X.; Zou, D.; Mao, B.; Yao, J.-Q.; Ouyang, C.; Wang, Z.; Wu, L. Terahertz narrowband filter metasurfaces based on bound states in the continuum. Opt. Express 2023, 31, 35272–35281. [Google Scholar] [CrossRef]
- Yang, J.; Huang, Z.; Maksimov, D.N.; Pankin, P.S.; Timofeev, I.V.; Hong, K.; Li, H.; Chen, J.; Hsu, C.; Liu, Y.; et al. Low-threshold bound state in the continuum lasers in hybrid lattice resonance metasurfaces. Laser Photonics Rev. 2021, 15, 2100118. [Google Scholar] [CrossRef]


| Resonance Type | Sensitivity | FOM | Reference |
|---|---|---|---|
| EIT effect | 96.2 GHz/RIU | 7.8 | [51] |
| Highly flexible, ultrathin metamaterial absorber | 139.2 GHz/RIU | / | [52] |
| Non-bianisotropic metamaterial | 182 GHz/RIU | / | [53] |
| Toroidal moment-based metamaterial | 23.7 GHz/RIU | / | [54] |
| A cross-shaped aluminum resonator | 247 GHz/RIU | 3.927 | [55] |
| An Archimedean spiral with a C-shaped resonator | 78.7 GHz/RIU | 14.4 | [56] |
| Two concentric square rings and a cylinder | 187.5 GHz/RIU | 19.1 | [57] |
| L-shaped structure | 300 GHZ/RIU | 5367.8 | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, Y.; Lv, J.; Liu, C.; Wang, D.; Li, R.; Li, L.; Lu, X.; Liu, Q.; Wang, J.; Liu, W.; et al. Dual Modulation Polarization-Independent Terahertz BIC Metasurface for Multi-Wavelength Sensing. Coatings 2025, 15, 363. https://doi.org/10.3390/coatings15030363
Ren Y, Lv J, Liu C, Wang D, Li R, Li L, Lu X, Liu Q, Wang J, Liu W, et al. Dual Modulation Polarization-Independent Terahertz BIC Metasurface for Multi-Wavelength Sensing. Coatings. 2025; 15(3):363. https://doi.org/10.3390/coatings15030363
Chicago/Turabian StyleRen, Yanru, Jingwei Lv, Chao Liu, Debao Wang, Renfeng Li, Liangliang Li, Xili Lu, Qiang Liu, Jianxin Wang, Wei Liu, and et al. 2025. "Dual Modulation Polarization-Independent Terahertz BIC Metasurface for Multi-Wavelength Sensing" Coatings 15, no. 3: 363. https://doi.org/10.3390/coatings15030363
APA StyleRen, Y., Lv, J., Liu, C., Wang, D., Li, R., Li, L., Lu, X., Liu, Q., Wang, J., Liu, W., & Chu, P. K. (2025). Dual Modulation Polarization-Independent Terahertz BIC Metasurface for Multi-Wavelength Sensing. Coatings, 15(3), 363. https://doi.org/10.3390/coatings15030363







