1. Introduction
Hard and soft magnetic materials are two distinct yet crucial types of magnetic materials. Hard magnetic materials exhibit significant magnetic anisotropy, in contrast to soft magnetic materials, which possess lower anisotropy. When these two materials are combined to form nanocomposite magnetic materials, novel phenomena and properties emerge due to the magnetic exchange coupling effects between the phases [
1,
2,
3,
4,
5,
6,
7]. Hard–soft systems have been extensively studied because of their important applications in permanent magnetic materials, high-density magnetic recording media, giant magnetostrictive materials, etc. [
8,
9,
10,
11,
12,
13,
14,
15,
16,
17]. For example, the anisotropic nanocomposite permanent magnetic material which contains the coupled and aligned hard and soft phases has the theoretically predicted giant maximum energy product (BH)
max. It is expected to be a good candidate for the next generation of permanent magnetic materials. Isotropic nanocomposite magnets, containing randomly oriented hard magnetic grains, have been widely employed in magnetic devices due to their enhanced remanence. The hard–soft exchange coupled system has also been utilized in state-of-the-art high-density magnetic recording media to tune their coercive force H
c or irreversible field H
irr [
10,
11].
One key and basic feature in the hard–soft exchange coupling nanocomposite system is the significantly modified nucleation field (reversal field) of soft-phase H
ns relative to that of the bulk single soft phase. H
ns can be greatly enhanced by reducing ds, particularly in the nanometric scale. The value of H
ns is influenced by both the size of the soft phase and the strength of the exchange coupling between the hard and soft phases [
1,
2]. quantitatively characterizing the dependence of H
ns on ds forms a crucial basis for understanding the magnetic properties and reversal mechanisms in hard–soft magnetic materials. For instance, when designing a hard–soft nanocomposite permanent magnetic material with a specific coercive force or a single-phase hysteresis loop, the relationship between H
ns and ds can essentially guide us in determining the appropriate size of the soft phase to ensure the desired properties.
H
ns, as a function of ds, has been studied theoretically in hard–soft exchange coupled systems [
2,
18,
19,
20,
21]. However, there is currently no unified theoretical formula that applies to a wide range of sizes of soft magnetic phases and different theoretical formulas are only applicable to specific size ranges.
The dependence of H
ns on d
s has been also experimentally studied. S.S. Yan et al. successfully fabricated well-textured SmFe/NiFe bilayer films and obtained the accurate value of H
ns when d
s varied from 34 to 170 nm [
8]. To quantitatively describe the relationship between H
ns and d
s, a fitting formula,
, was used to match the experimental data, yielding a satisfactory result [
8]. S. Sawatzki et al. fabricated SmCo
5/Fe/SmCo
5 tri-layer films and determined the value of H
ns for smaller d
s but in a narrow range: d
s = 6.3~12 nm [
9]. Their tri-layer films, however, were fabricated at a high temperature. Diffusion between the Sm-Co and Fe layers was inevitable and an Fe-Co intermixed phase was introduced. So, the dependence of H
ns on d
s could not be determined strictly. Obtaining the precise value of H
ns for small d
s is typically difficult, as it requires achieving both a large coercive force and excellent texture for the hard phase. The quantitative relationship between H
ns and d
s in the sub-tens nanometer range remains significantly under-explored in experimental investigations.
A high-quality sample is a necessary prerequisite to determine accurately the quantitative dependence of Hns on ds. An ideal sample should have the following characteristics: (i) no diffusion between the hard and soft magnetic phases; (ii) the hard magnetic phase has a high coercive force considering that the value of Hns increases as the size of the soft phase decreases; (iii) the hard phase should be well textured.
To address these issues, high-quality nanostructured SmCo5/Fe double layers with different soft-phase sizes with large coercive forces and strong textures were successfully fabricated through strict control of the preparation process in the present work. We conducted a quantitative analysis of the relationship between Hns and ds within the range of 2–40 nm. Based on the experimental results, we propose a new theoretical simulation formula that enhances the understanding of the characteristics at the interface between the soft magnetic and hard magnetic phases. These results not only contribute to the understanding of the magnetic mechanism of nano-composite magnets but also provide important guidance for the design of high-performance nano-composite magnets.
3. Results and Discussion
We tried many experimental parameters in an effort to achieve a good texture and high coercive force of the Sm-Co hard-layer films. We found that the high sputtering power and deposition temperature play important roles in developing the strong texture on the MgO (110)/Cr substrate, possibly due to the induced high kinetic energy of sputtered atoms conductive to make SmCo grow along the (110) as much as possible. Finally, the Sm-Co layer film with an excellent texture and high coercive force was achieved.
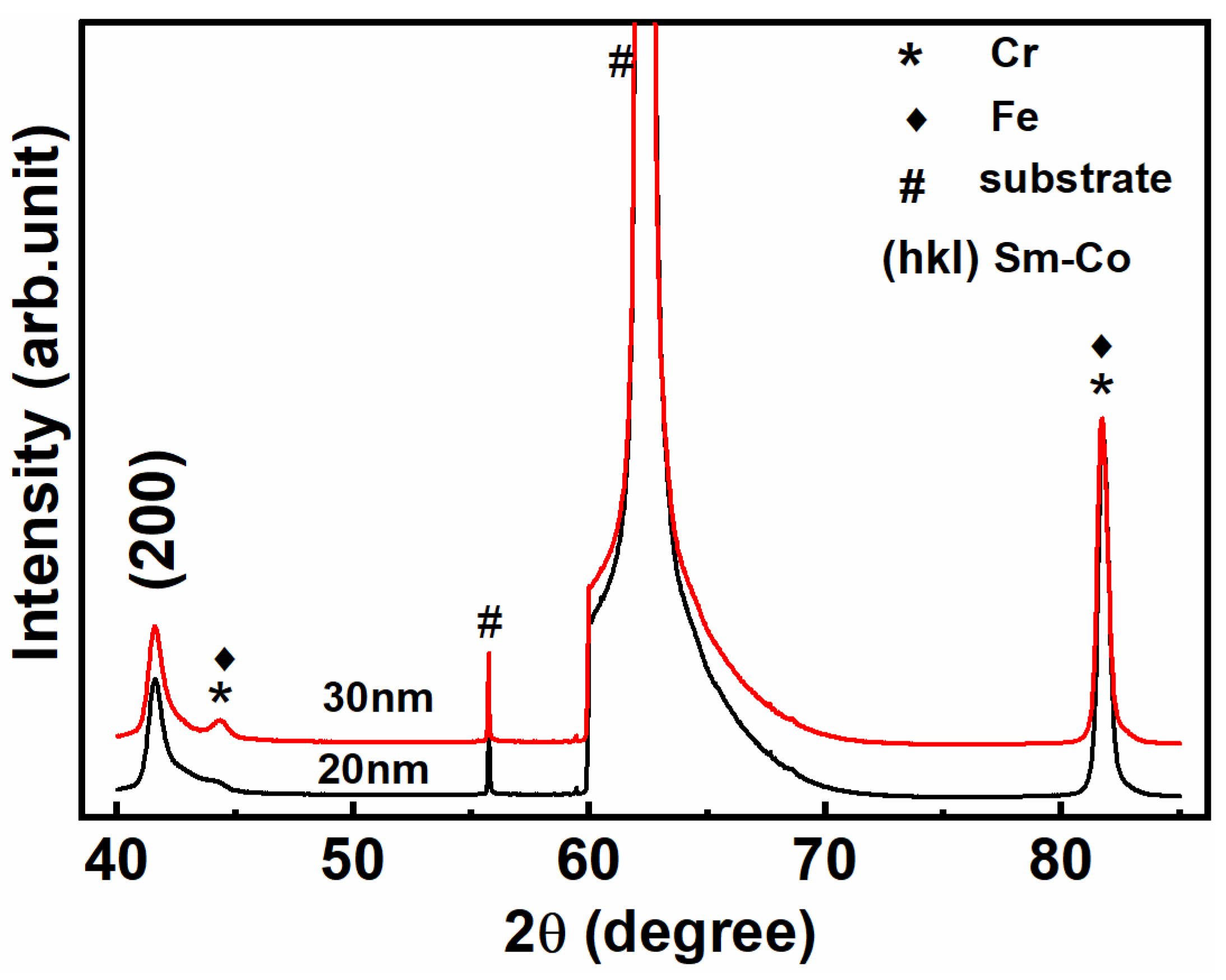
Figure 1 shows the XRD patterns of the Cr (50 nm)/SmCo (50 nm)/Fe (x nm)/Cr (50 nm) films with x as 20 and 30 nm. Only (200) diffraction peak of SmCo
5 was detected at 41.6030 and 41.6001 degrees and the full width at half maximum (FWHM) of the (200) diffraction peaks was 0.7049 and 0.7006 degrees for films with x as 20 and 30 nm, respectively. The grain size of SmCo was estimated to be around 2.6 nanometers based on the following Scherrer formula using the above data:
where
K is the Scherrer factor, which is chosen as 0.89 here,
λ is the wavelength of the incident light;
β is the full width at half maximum (FWHM) of the diffraction peaks and
θ is the diffraction angle. Specially, no (111) peaks exist, further demonstrating the excellent texture, which is much better than the Sm-Co film deposited on the SiO
2/Cr substrate [
14]. This indicates that the hard Sm-Co monolayer successfully achieves an excellent texture on the MgO (110) substrate via optimizing the sputtering fabrication parameters.
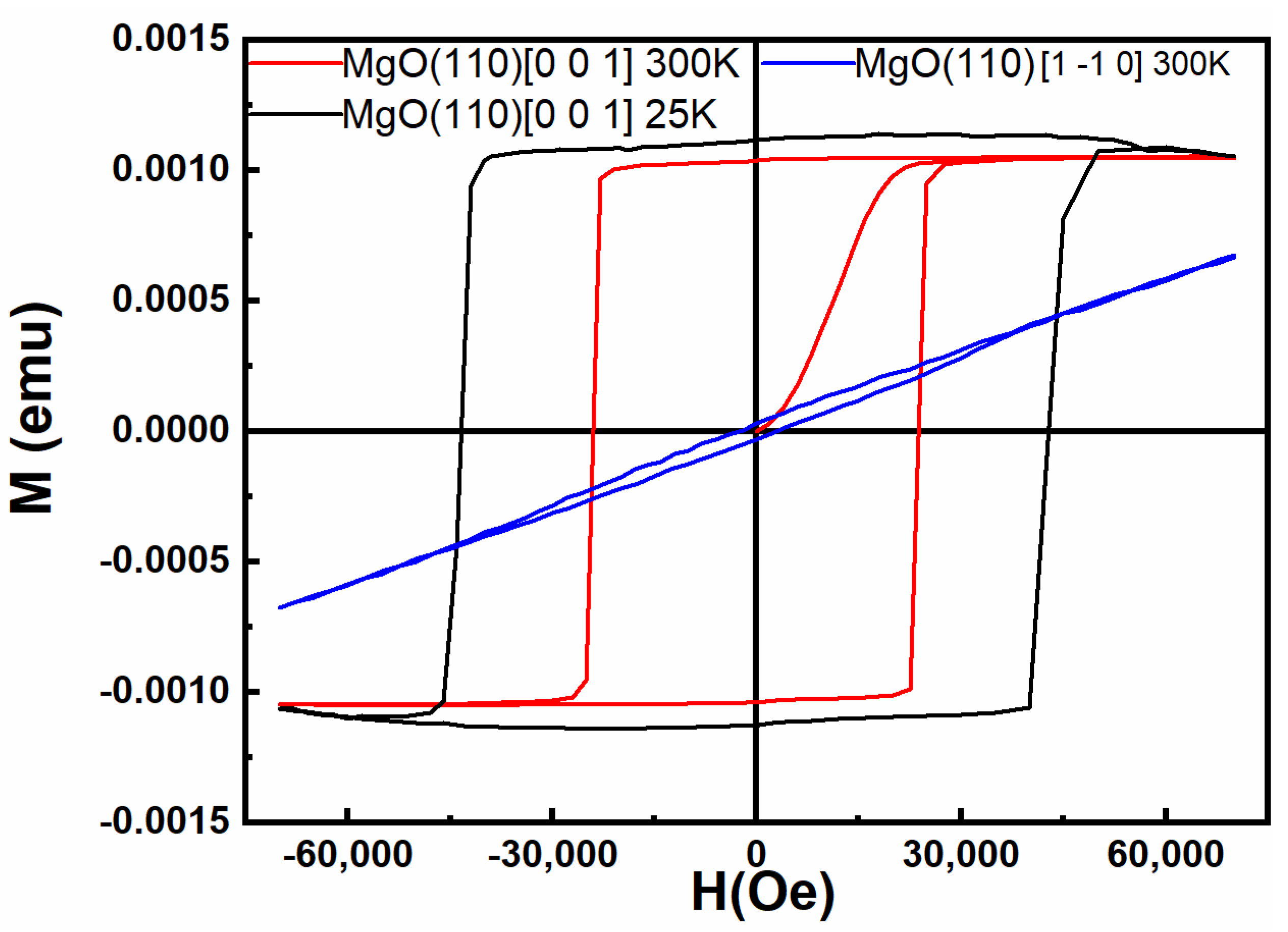
The magnetic hysteresis loops of the Sm-Co monolayer measured at 300 and 25 K shown in
Figure 2 exhibit a very good rectangular shape along the easy axis (MgO [0 0 1]) and extremely low remanence along the hard axis (MgO [1 −1 0]), further confirming the strongly textured Sm-Co phase. The coercive forces derived from the hysteresis loops measured at 300 and 25 K are 24.0 and 43.4 kOe, respectively. The increase in coercive force from 300 to 25 K should arise from the enhancement of Sm-Co magnetocrystalline anisotropy.
Figure 3a,b present the magnetic hysteresis loops of SmCo/Fe bi-layer films with different thicknesses of soft phase d
s from 2 to 40 nm measured at 25 and 300 K, respectively. For nanocomposite permanent magnetic materials, the exchange coupling between the hard and soft phase is dominated by the direct exchange coupling, a kind of short-range interaction effective within one atomic distance. During the demagnetization process, atom spins of the hard phase can have an influence on the rotation of those of the soft phase with a maximum distance of domain wall width through a cascade of interactions between neighbor atom spins. For the very large size of soft phase, e.g., 40 nm, the spins far from the hard phase very easily rotate, meaning that the H
ns of the soft phase is very low. During the demagnetization process, that part of soft phase first rotates, while the direction of the hard-phase spins still persists until the applied magnetic field reaches the H
irr, where the hard phase starts to reverse. Therefore, a two-step demagnetization curve is observed. We can see that the H
irr of the hard phase in the hard–soft bi-layer film is much lower than the H
c (or H
irr) of the single hard-layer film, which should be attributed to the formation of a domain wall upon the rotation of the soft phase, prompting the rotation of the hard phase [
22]. With the decrease in d
s, the total soft-phase spins become closer to the hard phase and their rotations turn out to be harder; therefore, H
ns increases. As d
s further decreases, H
ns becomes very large. At a critical size of soft phase d
sc, H
ns is identical to H
irr, i.e., the rotation of the soft phase immediately leads to the reverse of the hard phase, signifying that the hard and soft phases start to reverse together and a single-phase behavior in the demagnetization curve is observed.
The critical size of soft phase d
sc at which the demagnetization curve changes from the single-phase behavior to two-step behavior is about 6 nm at 25 K and 8 nm at 300 K (
Figure 3). In the hard–soft nanocomposite system, d
sc is usually calculated in terms of the theoretical model invoked by Kneller and Hawig: d
sc =
, where A
s is the exchange energy of the soft phase and K
k is the magnetocrystalline anisotropy constant of the hard phase [
1]. d
sc was calculated for the Sm-Co/Fe hard–soft system (A
s = 2.53 × 10
−11 J/m and K
k = 1.71 × 10
7 J/m
3) [
23] using the above formula and the value of d
sc was 2.7 nm, which is inconsistent with our experimental results. This inconsistency suggests that d
sc is not determined only by A
s and K
k. H
irr or H
c of the initial single hard phase (
Figure 3) may also have an influence on d
sc. A higher H
irr or H
c will lead to a smaller d
sc [
23].
We can determine the reversal field (nucleation field) of the soft phase H
ns, the irreversible field of the hard phase H
irr and the coercive force H
c for different d
s based on the experimentally measured demagnetization curves (
Figure 3), which are shown in
Figure 4. It can be seen that H
ns gradually increases with the decrease in d
s for d
s ≥ 20 nm. The increase in H
ns becomes more rapid when d
s ≤ 20 nm. It rises up sharply for d
s ≤ 10 nm. The value of H
irr was fixed at 17.6 ± 0.1 kOe at 25 K and 9.0 ± 0.1 kOe at 300 K for a relatively large size d
s (15 nm ≤ d
s ≤ 40 nm). In this d
s range, the Bloch domain wall could be formed in the demagnetization process due to the 180° rotation of the soft-phase spins far from the hard phase, which will exert equal force on the hard phase, giving rise to the identical H
irr. It is interesting to observe that H
irr starts to increase with the decrease in d
s for d
s ≥ 15 nm, which is highly likely attributed to the incomplete Bloch domain wall formed at the same magnetic field exerting the reduced force on the reverse of the hard phase. Anyhow, we strictly and accurately obtained the dependence of H
ns and H
irr on d
s in experiments (
Figure 4). The results can serve as an important basis for research on understanding the mechanism and practical design of a hard–soft system for different applications. For example, the nanocomposite magnet usually needs high H
c and H
irr. In this case, the preferred size of soft phase d
s should be lower than 10 nm. The hard–soft exchange coupling system utilized in high-density magnetic recording media, however, requires a lower H
irr. In this case, a designed d
s larger than 10 nm is favored. In addition, for the nanocomposite magnet with high (BH)
max, a hysteresis loop with characteristics of single-phase behavior is necessary, the determined relationship between H
ns and d
s can tell us how much size of soft phase can be adopted in order to obtain the desired coercive force.
There have been some important theories and simulations on the relationship between H
ns and d
s. E. Goto et al. hypothesized an extremely hard magnetic layer and its magnetization during the demagnetization process was immobile before reaching its irreversible field in the hard and soft magnetic bi-layer film system [
18]. Based on this model, an analytic solution was resolved as the following formula:
where A and M
s are the exchange coupling constant and saturation magnetization, respectively, and N is a constant and derived to be 2. T. Leineweber and H. Kronmüller analyzed the relationship between H
ns and d
s using the micromagnetic method [
19]. They found that H
ns was related to the width of the Bloch wall of the hard magnetic layer, and H
ns~d
s were divided into three regions:
where πδ
h is the width of the Bloch wall and h denotes the hard magnetic layer. It can be seen from Formula (3) that H
ns keeps constant when 2d
s is less than πδ
h and goes through a rapid descent process until 2d
s reaches 8πδ
h. With further increasing d
s, the relationship between H
ns and d
s is similar to Formula (1), with N chosen as 1.75.
When d
s is large, H
ns is small and relatively easy to determine experimentally. S.S Yan et al. experimentally measured H
ns of SmFe/NiFe bi-layer films when d
s was in a range of 34~170 nm [
8]. Since d
s is large, they used the formula
in Formula (3) to fit the dependence of H
ns on d
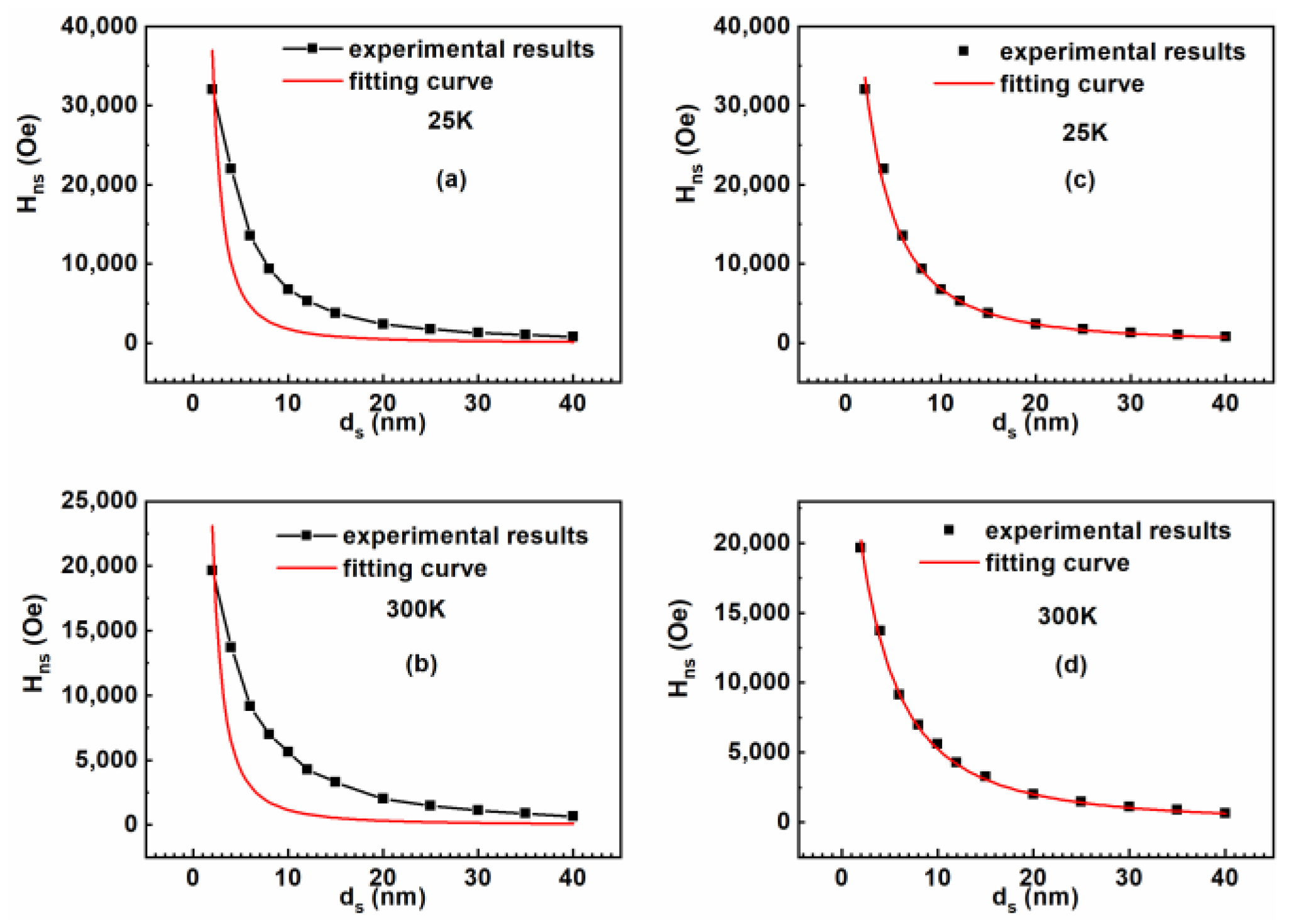
s, and a good fitting was achieved for N = 1.73, which is close to the N value of Formula (3). Usually, it is difficult to obtain the accurate value of H
ns for small d
s in experiments because it needs a high-quality sample with good texture and high H
irr of the hard phase. We successfully determined the experimental value of H
ns for very small d
s = 2–40 nm. We used Formula (2) to fit the dependence of H
ns on d
s. However, no ideal result was achieved, as indicated in
Figure 5a,b. Although the values of N obtained are 2.05 and 2.14 at 300 and 25 K, respectively, close to the values obtained by E. Goto et al. using Formula (1) [
18], the fitting curves do not conform to the experimental data very well.
In hard–soft nanocomposite magnetic systems, due to the strong exchange coupling between the hard and soft magnetic layers, the magnetic moment of the soft magnetic layer will not reverse during the demagnetization process until the external magnetic field increases to the nucleation field H
ns of the soft phase. When the external magnetic field continues to increase and lies between the soft magnetic nucleation field H
ns and the hard magnetic irreversible field H
irr, the magnetic moment of the soft magnetic layer reverses, while that of the hard magnetic layer is theoretically supposed to persist completely [
18]. When the external magnetic field is larger than H
irr, the magnetic moment of the hard magnetic layer starts to rotate. The theoretical expectation that the hard magnetic layer is totally immobile during the reverse of the soft layer is just the ideal case. In real hard–soft systems, the magnetic anisotropy of the hard phase is not infinite. Moreover, an interfacial region between the hard and soft phases should exist, which would give rise to the softening of partial thickness of the hard layer close to the soft layer. This thin softened hard layer should also concurrently reverse during the soft magnetic layer reversal process, which means that a certain thin hard layer will become the soft layer, causing an increase in d
s. Actually, a thin hard layer in the interfacial region rotating much easier concurrently with the reversal of the soft layer has been experimentally demonstrated [
24,
25,
26]. Based on the analysis above, a modified formula is obtained:
where a is a parameter representing the extra soft magnetic layer from the softened thin hard layer in the interfacial region. The dependence of H
ns on d
s determined by our experimental SmCo/Fe nanocomposite bi-layer films is fitted by Formula (4). A perfect fitting is achieved, as indicated in
Figure 5c,d. The values of N and parameter a produced from the fittings are shown in
Table 1. The obtained N is very close to what has been achieved by E. Goto et al. using Formula (2) [
16]. The values imply that 4.8 nm at 25 K and 6.6 nm at 300 K of the hard magnetic layer easily reverse, accompanying the reversal of the soft layer during the demagnetization process. The derived value of a at 25 K is smaller than that at 300 K, which is reasonable because the much-enhanced magnetocrystalline anisotropy of the hard layer at low temperatures makes its softening part become hard. Our results have important practical significance in designing a high-performance nanocomposite magnet at room temperature. In order to effectively pin the magnetic moment of the soft phase, the size of the hard phase should be larger than a certain thickness, e.g., about 6.6 nm in a Sm-Co/Fe bi-layer system, otherwise the total hard phase will reverse together with the soft phase, which will result in a very low coercive force of the whole nanocomposite magnet.