Temperature Field Analytical Solution for OGFC Asphalt Pavement Structure
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Determination of Thermal Conductivity
3. Theory
3.1. Basic Temperature-Field Equation
- The structure of each layer is uniform and homogeneous, showing no obvious difference in either appearance or physical properties at each point.
- The cross-sectional temperature at the same depth is the same at different pavement locations, and the heat transfer is only in one dimension, along the longitudinal direction, without considering the transverse distribution of the pavement-structure temperature field and transverse transfer of heat flow.
- The materials of each layer of pavement are closely combined, and there is no temperature-field fault; the interlayer temperature and heat flow are continuous; and the heat accumulation phenomenon is not evident and can be ignored.
3.2. Boundary Conditions
3.3. Initial Conditions and Simultaneous Equations
4. Determining the Temperature Field
4.1. Temperature Field Caused by the Initial Conditions
4.2. Temperature Field Caused by the Forced Conditions
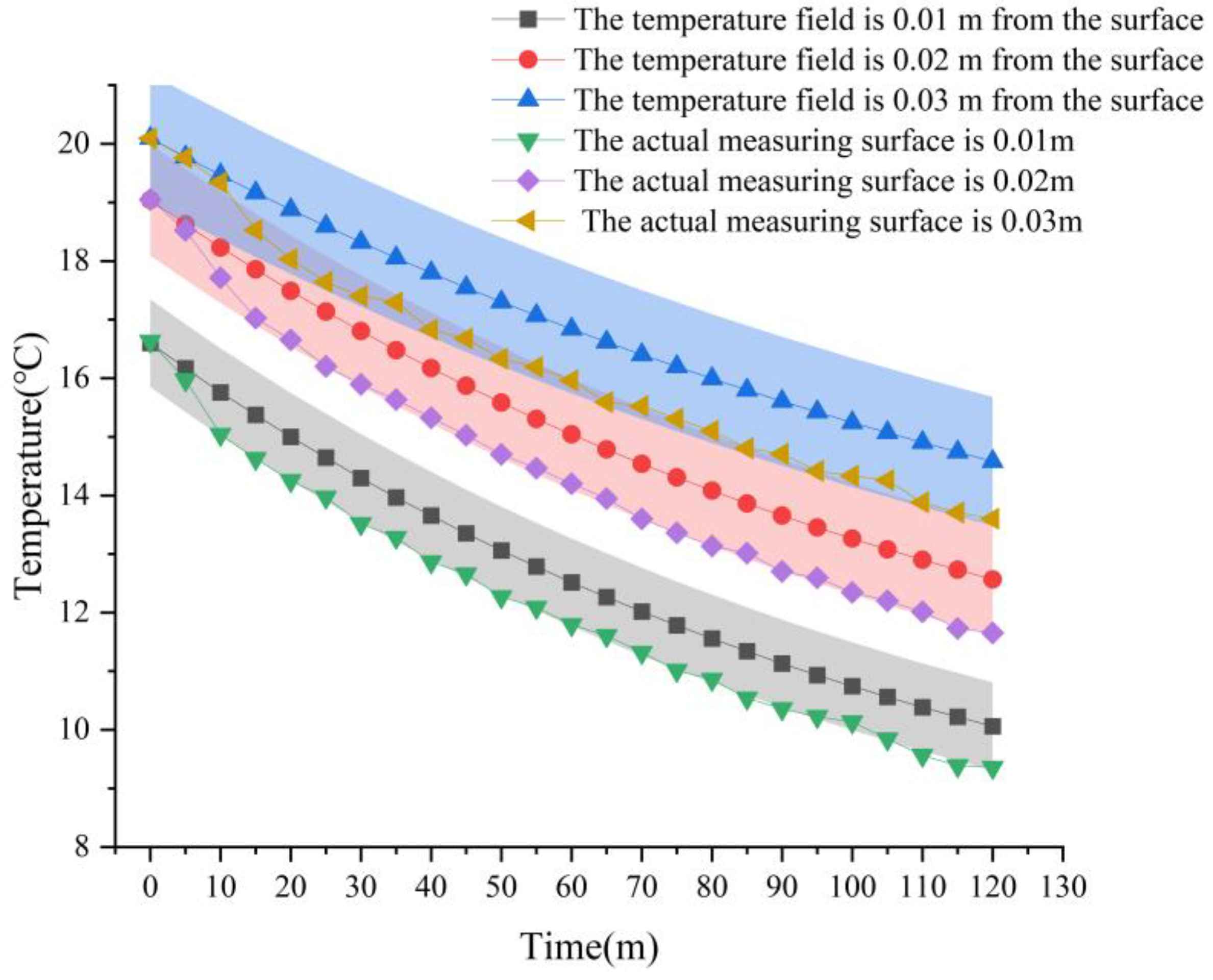
5. Verification of the Analytical Solution
6. Conclusions
- The relationship between the oil–stone ratio and thermal conductivity of the OGFC asphalt mixture was determined, and a quadratic function was fit to the resulting equation.
- Mathematical and physical methods—including the separation of variables and the homogenization principle—were used to solve the temperature field caused by the forced and initial conditions. An analytical solution of the temperature field of the OGFC asphalt pavement structure in the form of a Fourier series was then obtained. The analytical solution of the OGFC asphalt pavement temperature field contained three independent variables: time, depth, and oil–stone ratio.
- The analytical solution of the OGFC asphalt pavement temperature field was a composite polynomial comprising exponential and trigonometric functions in the form of a Fourier-series expansion. There were many physical and meteorological parameters in the analytical solution to describe radiation, sunshine, and other changes in the external environment as well as the properties of the pavement structure.
- Large Marshall specimens were used as the research objects for the outdoor test. The experimental results were then compared with the analytical-solution prediction model, with the calculated results being the same as those of the actual temperature field.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Corlew, J.S.; Dickson, P.F. Methods for Calculating Temperature Profiles of Hot-Mix Asphalt Concrete as Related to the Construction of Asphalt Pavements; ProQuest: Ann Arbor, MI, USA, 1968. [Google Scholar]
- Putman, B.J.; Kline, L.C. Comparison of Mix Design Methods for Porous Asphalt Mixtures. J. Mater. Civ. Eng. 2012, 24, 1359–1367. [Google Scholar] [CrossRef]
- Huber, G. Performance Survey on Open-Graded Friction Course Mixes; NCHRP Synthesis of Highway Practice; Transportation Research Board, National Research Council: Washington, DC, USA, 2000. [Google Scholar]
- Bérengier, M.; Stinson, M.R.; Daigle, G.A.; Hamet, J.F. Porous Road Pavements: Acoustical Characterization and Propagation Effects. J. Acoust. Soc. Am. 1997, 101, 155–162. [Google Scholar] [CrossRef]
- Shi, X.; Rew, Y.; Ivers, E.; Shon, C.-S.; Stenger, E.M.; Park, P. Effects of thermally modified asphalt concrete on pavement temperature. Int. J. Pavement Eng. 2019, 20, 669–681. [Google Scholar] [CrossRef]
- Tian, X.; Huang, X.; Yu, B. Experimental study on the durability of open graded friction course mixtures. In Pavements and Materials: Recent Advances in Design, Testing and Construction; ASCE: Reston, VA, USA, 2011; pp. 71–77. [Google Scholar]
- Hamzah, M.O.; Hasan, M.R.M.; van de Ven, M. Permeability loss in porous asphalt due to binder creep. Constr. Build. Mater. 2012, 30, 10–15. [Google Scholar] [CrossRef]
- Chen, J.; Li, H.; Huang, X.; Wu, J. Permeability Loss of Open-Graded Friction Course Mixtures due to Deformation-Related and Particle-Related Clogging: Understanding from a Laboratory Investigation. J. Mater. Civ. Eng. 2015, 27, 04015023. [Google Scholar] [CrossRef]
- Wang, D. Analytical Solutions for Temperature Profile Prediction in Multi-Layered Pavement Systems. Ph.D. Thesis, The University of Illinois at Urbana-Champaign, Champaign, IL, USA, 2010. [Google Scholar]
- Yan, L. Regression Analysis of Actual Measurement of Temperature Field Distribution Rules of Asphalt Pavement. China J. Highw. Transp. 2007, 20, 13–18. [Google Scholar]
- Barber, E.S. Calculation of Maximum Pavement Temperatures from Weather Reports; Highway Research Board Bulletin; Highway Research Board: Washington, DC, USA, 1957. [Google Scholar]
- Dempsey, B.J.; Thompson, M.R. A Heat Transfer Model for Evaluating Frost Action and Temperature-Related Effects in Multilayered Pavement Systems; Highway Research Record. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Champaign, IL, USA, 1970. [Google Scholar]
- Christison, J.T.; Anderson, K.O. The Response of Asphalt Pavements to Low Temperature Climatic Environments; Grosvenor House: London, UK, 1972. [Google Scholar]
- Lytton, R.L.; Pufahl, D.E.; Michalak, C.H.; Liang, H.S.; Dempsey, B.J. An Integrated Model of the Climatic Effects on Pavements. Final Report; National Research Council: Washington, DC, USA, 1993. [Google Scholar]
- Solaimanian, M.; Kennedy, T.W. Predicting Maximum Pavement Surface Temperature Using Maximum Air Temperature and Hourly Solar Radiation. In Transportation Research Record; National Academy Press: Washington, DC, USA, 1993; pp. 1–11. [Google Scholar]
- Liang, R.Y.; Niu, Y.-Z. Temperature and Curling Stress in Concrete Pavements: Analytical Solutions. J. Transp. Eng. ASCE 1998, 124, 91–100. [Google Scholar] [CrossRef]
- Hermansson, Å. Simulation Model for Calculating Pavement Temperatures Including Maximum Temperature. Transp. Res. Rec. 2000, 1699, 134–141. [Google Scholar] [CrossRef]
- Mrawira, D.; Luca, J.A.D. Thermal Properties and Transient Temperature Response of Full-Depth Asphalt Pavements. Transp. Res. Rec. 2002, 1809, 160–171. [Google Scholar] [CrossRef]
- Diefenderfer, B.K.; Al-Qadi, I.L.; Diefenderfer, S.D. Model to Predict Pavement Temperature Profile: Development and Validation. J. Transp. Eng. ASCE 2006, 132, 162–167. [Google Scholar] [CrossRef]
- Wang, D. Analytical Approach to Predict Temperature Profile in a Multilayered Pavement System Based on Measured Surface Temperature Data. J. Transp. Eng. ASCE 2012, 138, 674–679. [Google Scholar] [CrossRef]
- Wang, D.; Roesler, J.R. One-Dimensional Rigid Pavement Temperature Prediction Using Laplace Transformation. J. Transp. Eng. ASCE 2012, 138, 1171–1177. [Google Scholar] [CrossRef]
- Alawi, M.H.; Helal, M.M. Mathematical modelling for solving nonlinear heat diffusion problems of pavement spherical roads in Makkah. Int. J. Pavement Eng. 2012, 13, 137–151. [Google Scholar] [CrossRef]
- Li, Z.; Tan, Y. Temperature Field of Asphalt Pavement in Seasonal Frozen Region Suffered by Freeze-Thaw Cycles. In Proceedings of the ICTE 2013: Safety, Speediness, Intelligence, Low-Carbon, Innovation, Chengdu, China, 19–20 October 2013. [Google Scholar]
- Wang, D. Prediction of Asphalt Pavement Temperature Profile during FWD Testing: Simplified Analytical Solution with Model Validation Based on LTPP Data. J. Transp. Eng. ASCE 2013, 139, 109–113. [Google Scholar] [CrossRef]
- Wang, D. Prediction of Time-Dependent Temperature Distribution within the Pavement Surface Layer during FWD Testing. J. Transp. Eng. ASCE 2016, 142, 06016002. [Google Scholar] [CrossRef]
- Chen, J.; Li, L.; Zhao, L.-h.; Dan, H.-c.; Yao, H. Solution of pavement temperature field in “Environment-Surface” system through Green’s function. J. Cent. South Univ. 2014, 21, 2108–2116. [Google Scholar] [CrossRef]
- Chen, J.; Wang, H.; Zhu, H. Analytical approach for evaluating temperature field of thermal modified asphalt pavement and urban heat island effect. Appl. Therm. Eng. 2017, 113, 739–748. [Google Scholar] [CrossRef]
- Qin, Y. Pavement surface maximum temperature increases linearly with solar absorption and reciprocal thermal inertial. Int. J. Heat Mass Transf. 2016, 97, 391–399. [Google Scholar] [CrossRef]
- Dumais, S.; Doré, G. An albedo based model for the calculation of pavement surface temperatures in permafrost regions. Cold Reg. Sci. Technol. 2016, 123, 44–52. [Google Scholar] [CrossRef]
- Zhang, L.; Huang, J.; Li, P. Prediction of Temperature Distribution for Previous Cement Concrete Pavement with Asphalt Overlay. Appl. Sci. 2020, 10, 3697. [Google Scholar] [CrossRef]
- Yu, B.; Sun, Z.; Qi, L. Formation And Failure Behavior Of Open-Graded Friction Course At Low Temperatures. Stavební Obz.-Civ. Eng. J. 2022, 20, 260–273. [Google Scholar] [CrossRef]
- Press, C.C. Technical Specification for Permeable Asphalt Pavement; China Communications Press: Beijing, China, 2012. [Google Scholar]
- Yu, X.; Zadshir, M.; Yan, J.R.; Yin, H. Morphological, Thermal, and Mechanical Properties of Asphalt Binders Modified by Graphene and Carbon Nanotube. J. Mater. Civ. Eng. 2022, 34, 04022047. [Google Scholar] [CrossRef]
- Zhang, Y.; Dong, X.F.; Wang, J.; Li, N. Study on Temperature Field of Asphalt Pavement in Tongliao, Inner Mongolia. IOP Conf. Ser. Earth Environ. Sci. 2020, 587, 012026. [Google Scholar] [CrossRef]
- Jianguang, X.; Jia, S.; Li, H.; Lei, G.H. Study on the Influence of Clogging on the Cooling Performance of Permeable Pavement. Water 2018, 10, 299. [Google Scholar]
- Mühlich, U.; Pipintakos, G.; Tsakalidis, C. Mechanism based diffusion-reaction modelling for predicting the influence of SARA composition and ageing stage on spurt completion time and diffusivity in bitumen. Constr. Build. Mater. 2021, 267, 120592. [Google Scholar] [CrossRef]
- Zuoren, Y. Analysis of the temperature field in layered pavement system. J. Tongji Univ. 1984, 3, 79–88. [Google Scholar]
- Li-kui, H.; Jian-ping, W. Numerical analysis and experimental validation of high temperature fields in asphalt pavements. Rock Soil Mech. 2006, 27, 40–45. [Google Scholar]
- Minhoto, M.J.C.; Pais, J.C.; Pereira, P.; Picado-Santos, L.G. Predicting Asphalt Pavement Temperature with a Three-Dimensional Finite Element Method. Transp. Res. Rec. 2005, 1919, 110–196. [Google Scholar] [CrossRef]
- Ganchang, W.U. The analytic theory of the temperature fields of bituminous pavement over semi-rigid roadbase. Appl. Math. Mech. 1997, 18, 181–190. [Google Scholar] [CrossRef]
- Wang, Y.; Leng, Z.; Wang, G. Structural contribution of open-graded friction course mixes in mechanistic–empirical pavement design. Int. J. Pavement Eng. 2014, 15, 731–741. [Google Scholar] [CrossRef]
- Mason, R.B. Thermal Insulation with Aluminum Foil. Ind. Eng. Chem. 1933, 25, 245–255. [Google Scholar] [CrossRef]
- Qian, G.; He, Z.G.; Yu, H.; Gong, X.; Sun, J. Research on the affecting factors and characteristic of asphalt mixture temperature field during compaction. Constr. Build. Mater. 2020, 257, 119509. [Google Scholar] [CrossRef]
- JTG E20-2011; Standard Test Methods of Bitumen and Bituminous Mixtures for Highway Engineering. China Communications Press: Beijing, China, 2011.
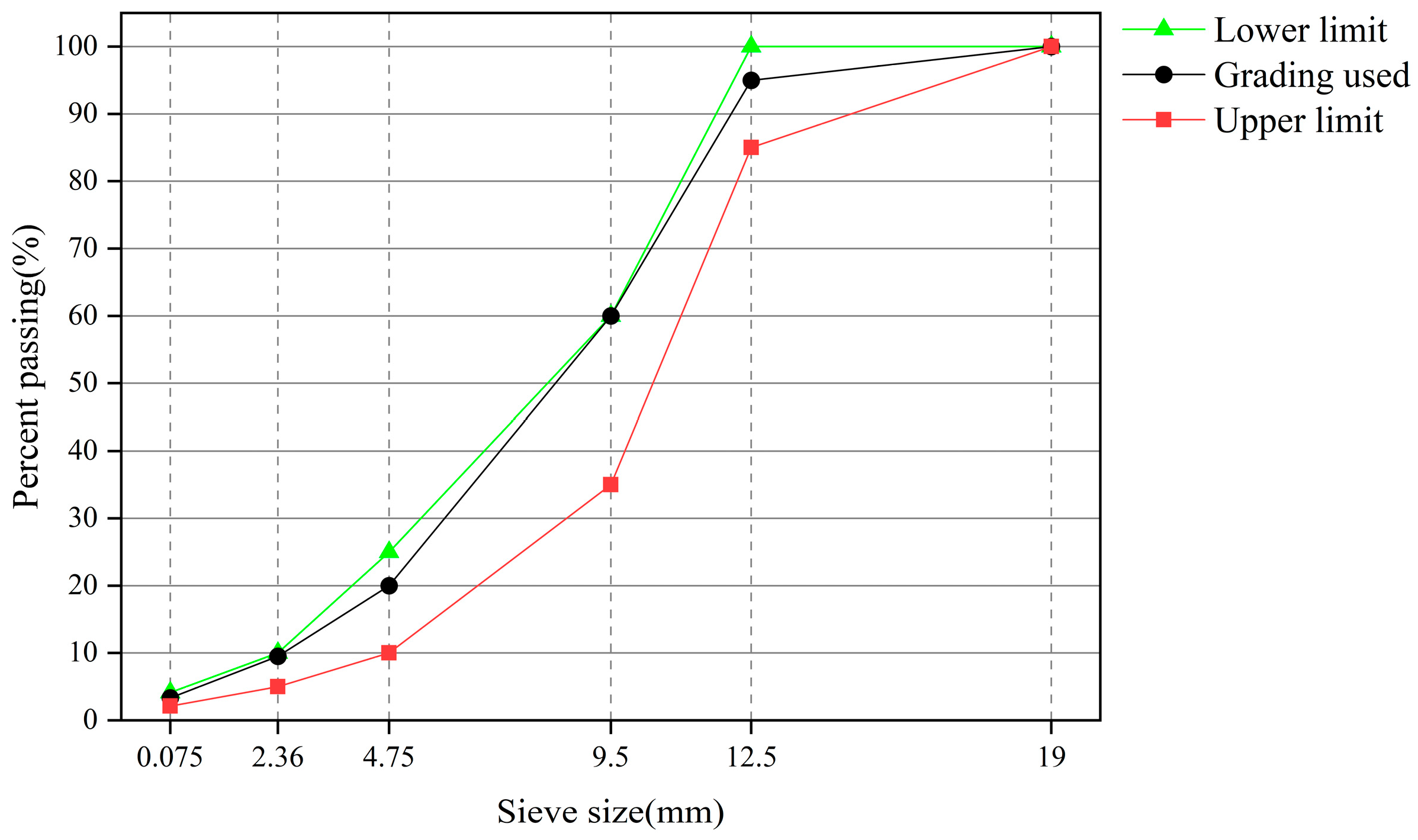

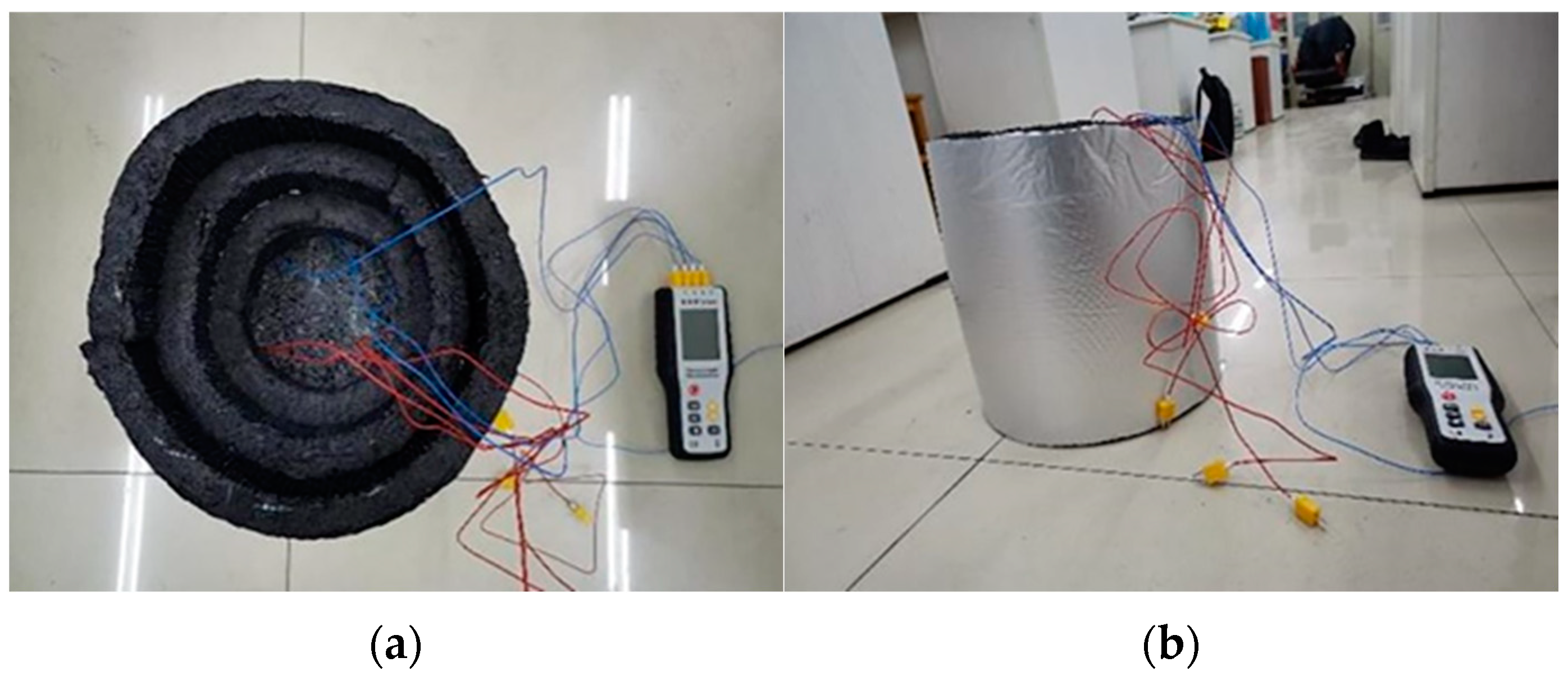
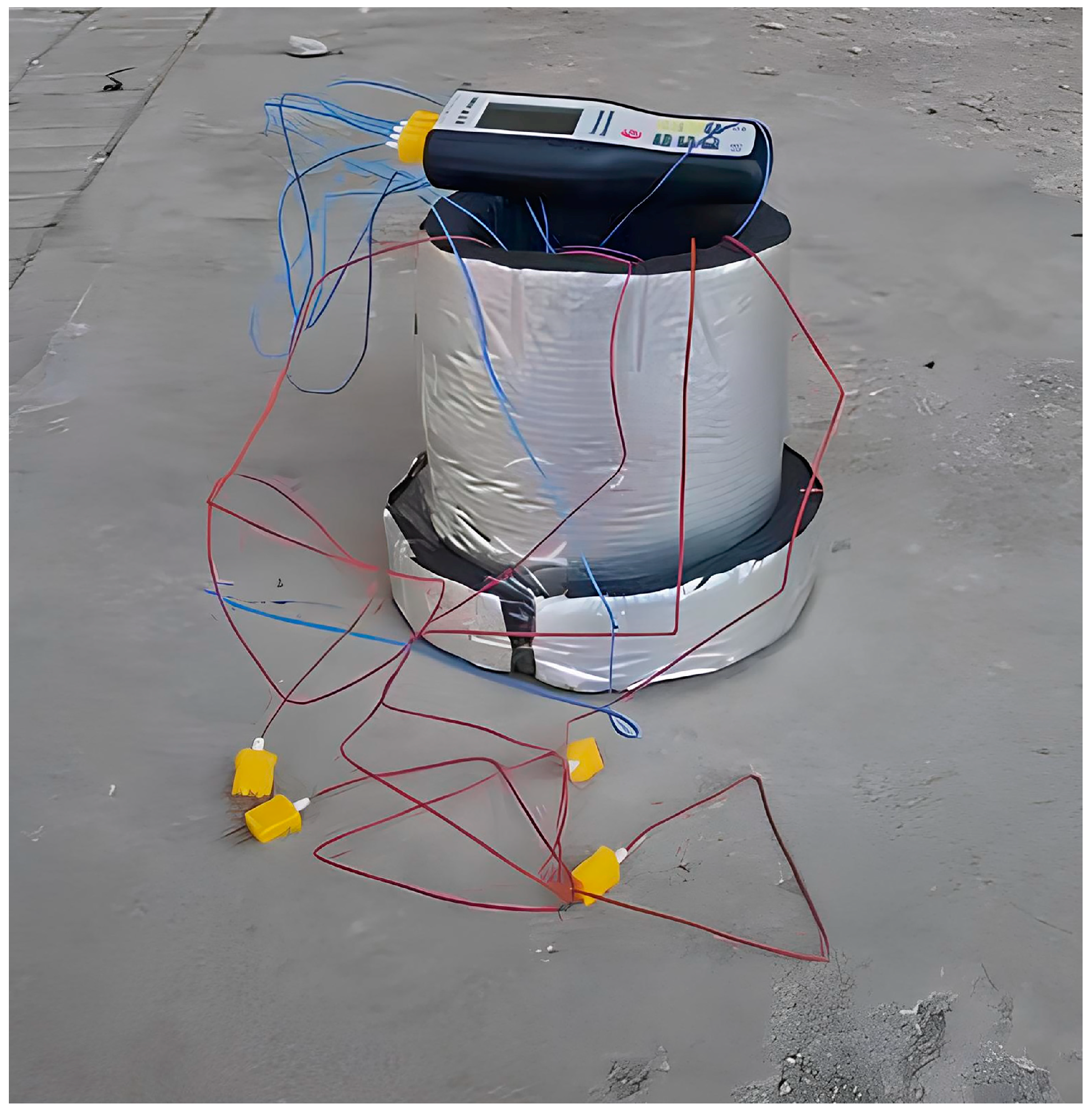
| Index | Unit | Test Result |
|---|---|---|
| 25 °C penetration | 0.1 mm | 88.2 |
| Softening point | °C | 49.2 |
| 15 °C ductility | cm | >150 |
| 60 °C viscosity | Pa·S | 228.5 |
| 35 °C viscosity | Pa·S | 0.334 |
| Flash point | °C | 323 |
| Temperature (°C) | Curve Fit of Thermal Conductivity to Oil–Stone Ratio | R2 |
|---|---|---|
| 20 | 0.96826 | |
| 40 | 0.97849 | |
| 60 | 0.98461 |
| Parameters | Unit | Numerical Value |
|---|---|---|
| Convective heat-transfer coefficient B | kJ/m2·h·°C | 46.024 |
| Density of OGFC asphalt concrete | kg/m3 | 2100 |
| Specific heat capacity of OGFC asphalt concrete c | J/(kg·°C) | 1.0985 |
| Wind speed v | km/h | 1.325 |
| Daily maximum temperature in winter | °C | 4 |
| Daily minimum temperature in winter | °C | −21 |
| Total daily radiation in winter | kJ/m3 | 7600 |
| Initial phase | h | 9 |
| Maximum sunshine hours in winter months | h | 5.2 |
| Maximum sunshine hours in the longest month | h | 11.4 |
| Radiation absorptivity | % | 0.87 |
| Underground constant temperature in winter | °C | 5 |
| Initial conditions of temperature field in winter | - | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, L.; Yu, B.; Zhao, Z.; Zhang, C. Temperature Field Analytical Solution for OGFC Asphalt Pavement Structure. Coatings 2023, 13, 1172. https://doi.org/10.3390/coatings13071172
Qi L, Yu B, Zhao Z, Zhang C. Temperature Field Analytical Solution for OGFC Asphalt Pavement Structure. Coatings. 2023; 13(7):1172. https://doi.org/10.3390/coatings13071172
Chicago/Turabian StyleQi, Lin, Baoyang Yu, Zhonghua Zhao, and Chunshuai Zhang. 2023. "Temperature Field Analytical Solution for OGFC Asphalt Pavement Structure" Coatings 13, no. 7: 1172. https://doi.org/10.3390/coatings13071172
APA StyleQi, L., Yu, B., Zhao, Z., & Zhang, C. (2023). Temperature Field Analytical Solution for OGFC Asphalt Pavement Structure. Coatings, 13(7), 1172. https://doi.org/10.3390/coatings13071172





