Research on the Interfacial Instability of Non-Newtonian Fluid Displacement Using Flow Geometry
Abstract
:1. Introduction
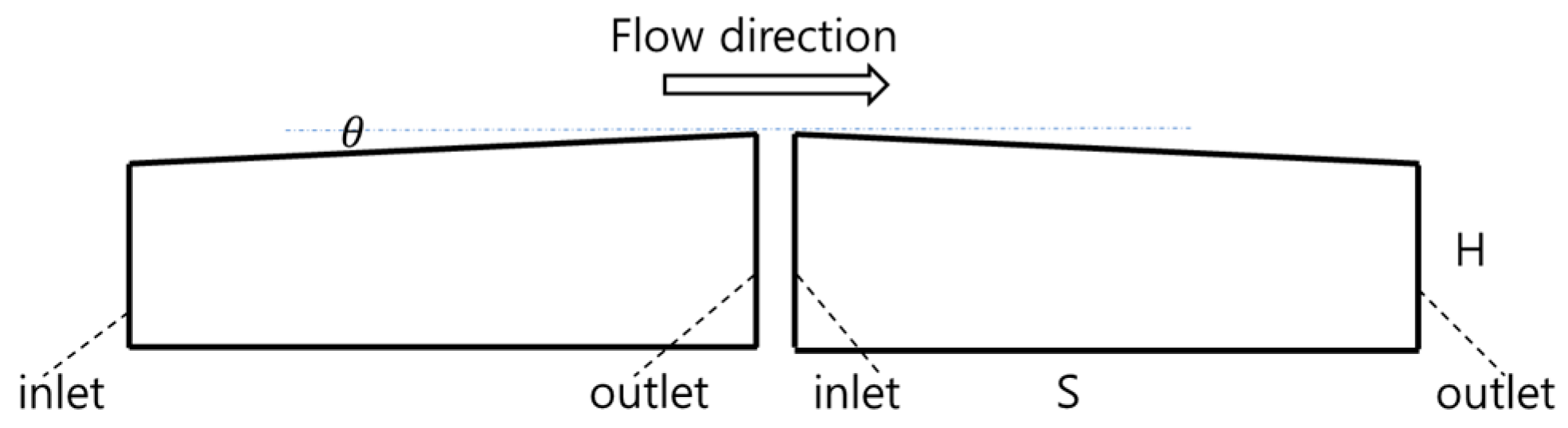
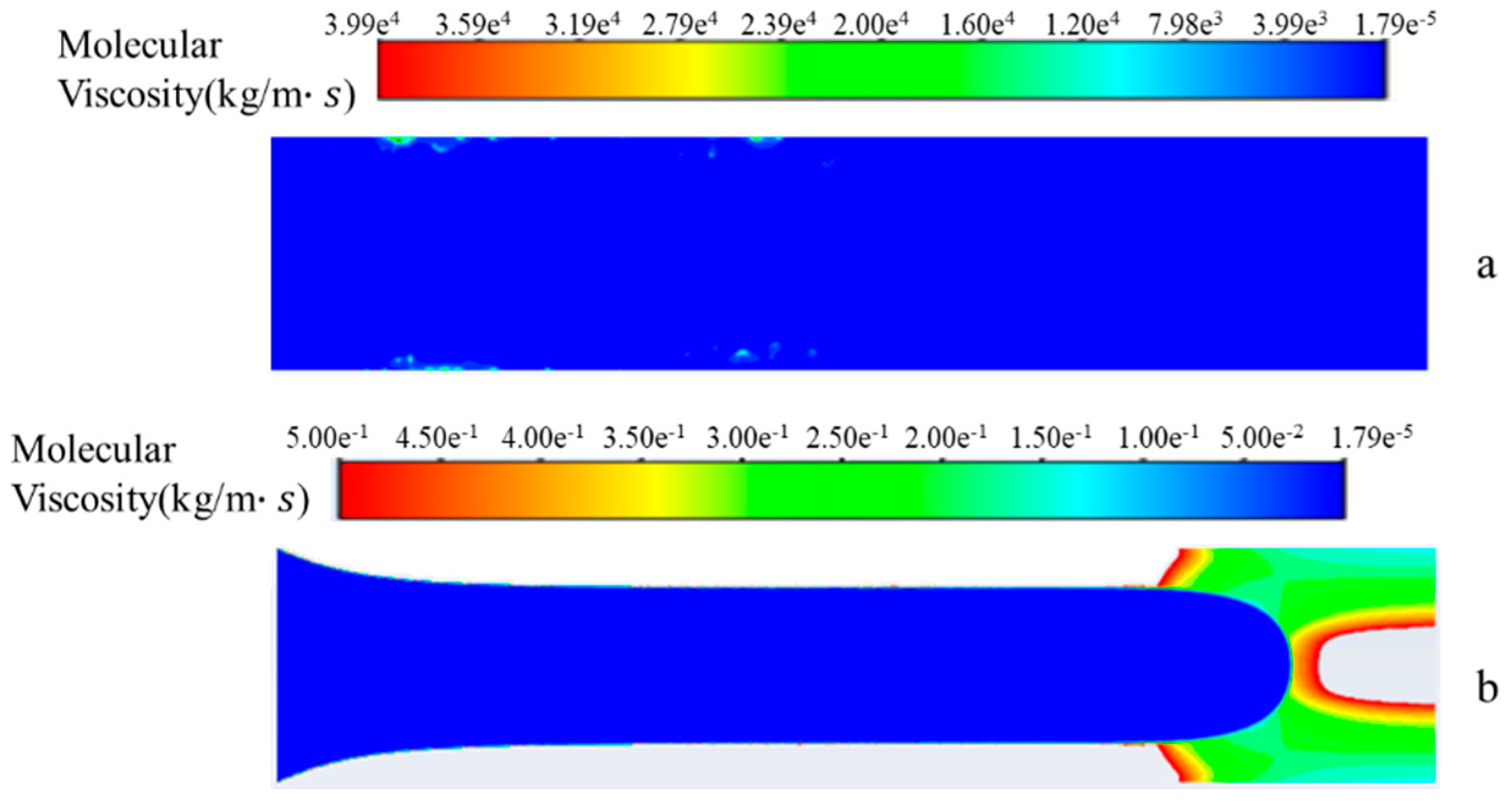
2. Numerical Method
3. Viscous Fingering under Different Depth Gradients at n = 0.4
4. Viscous Fingering of Different Power-Law Exponents with Depth Gradients
5. Influence of the Shape of the Upper Wall on the Viscous Fingering
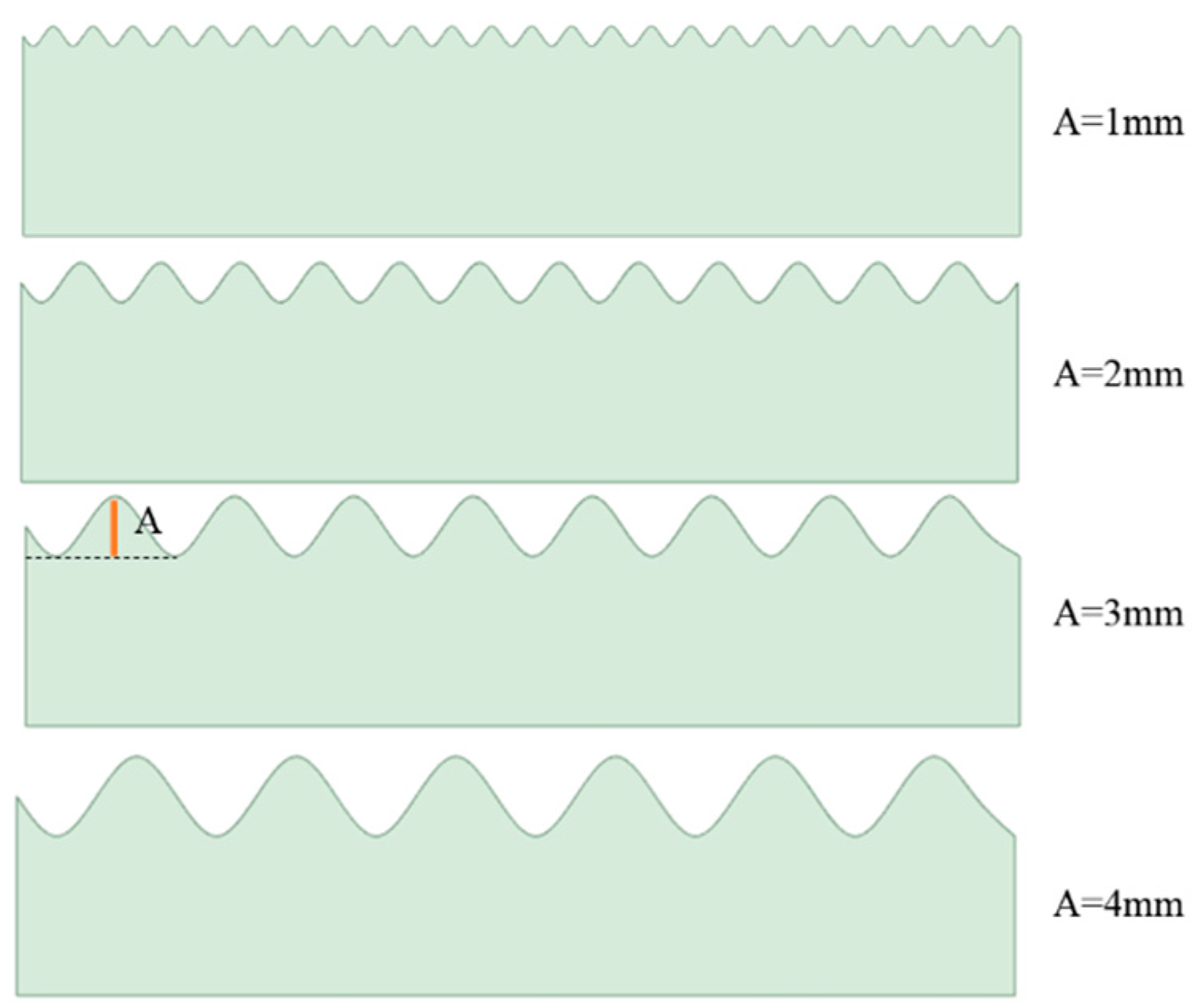
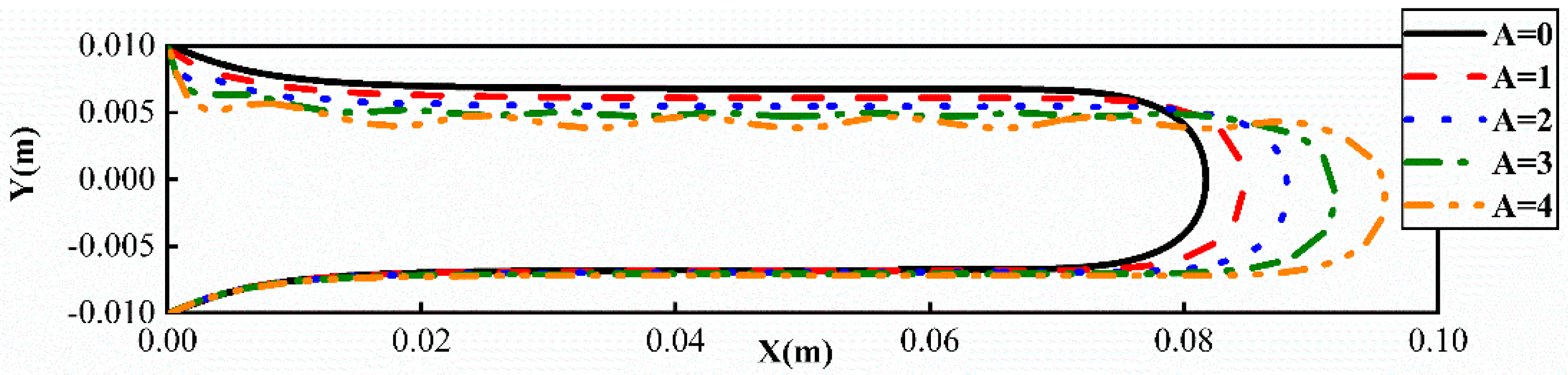
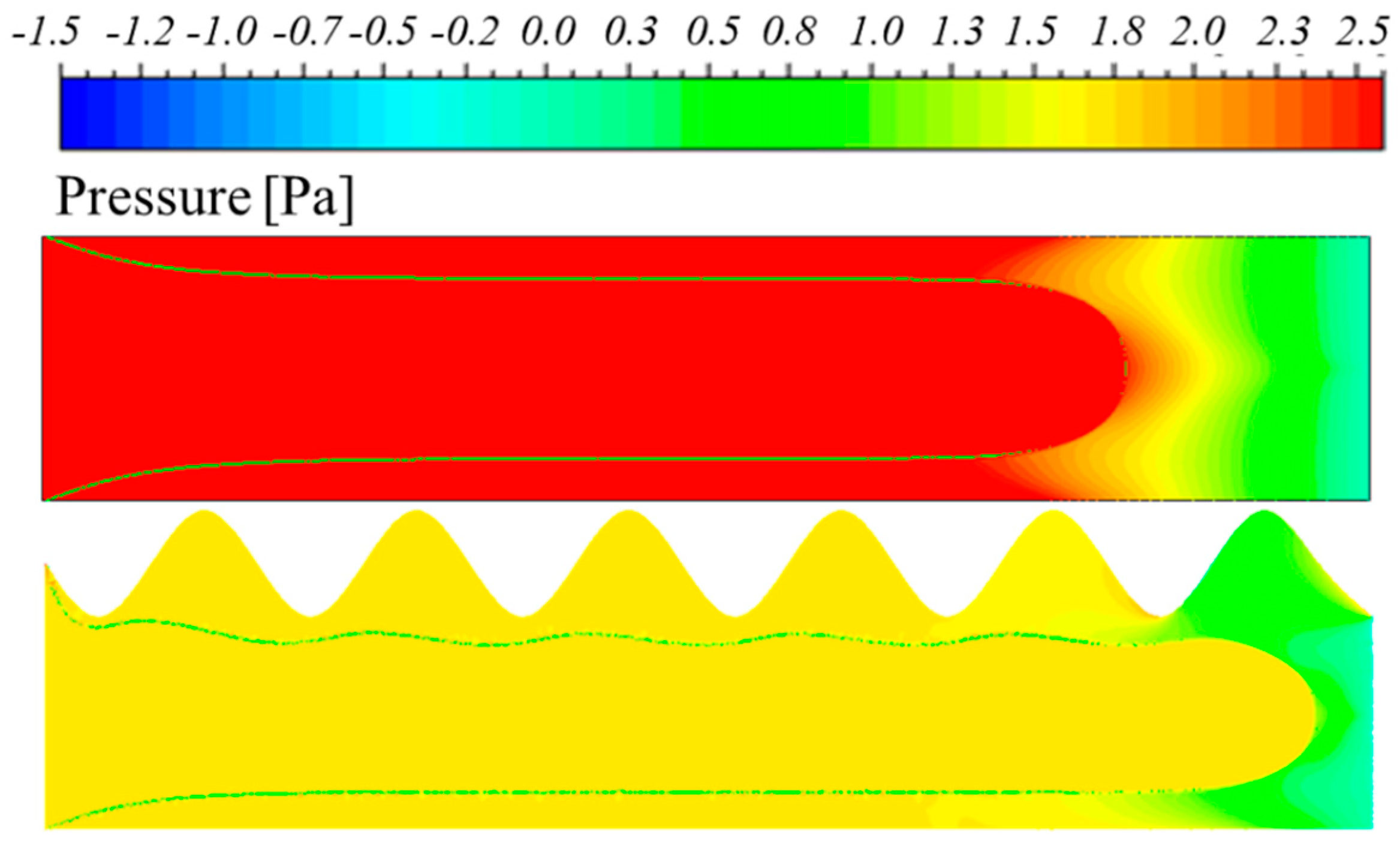
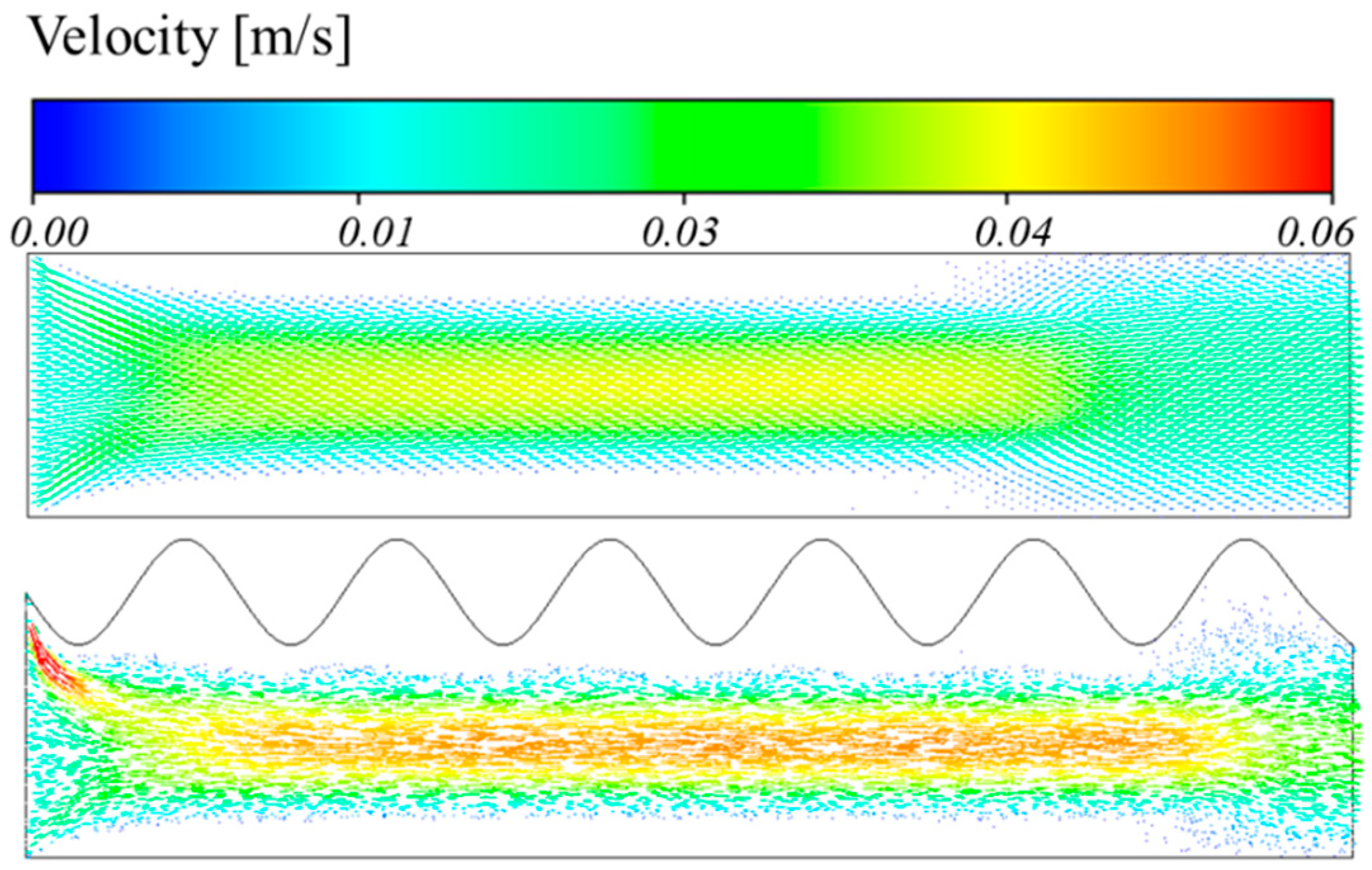
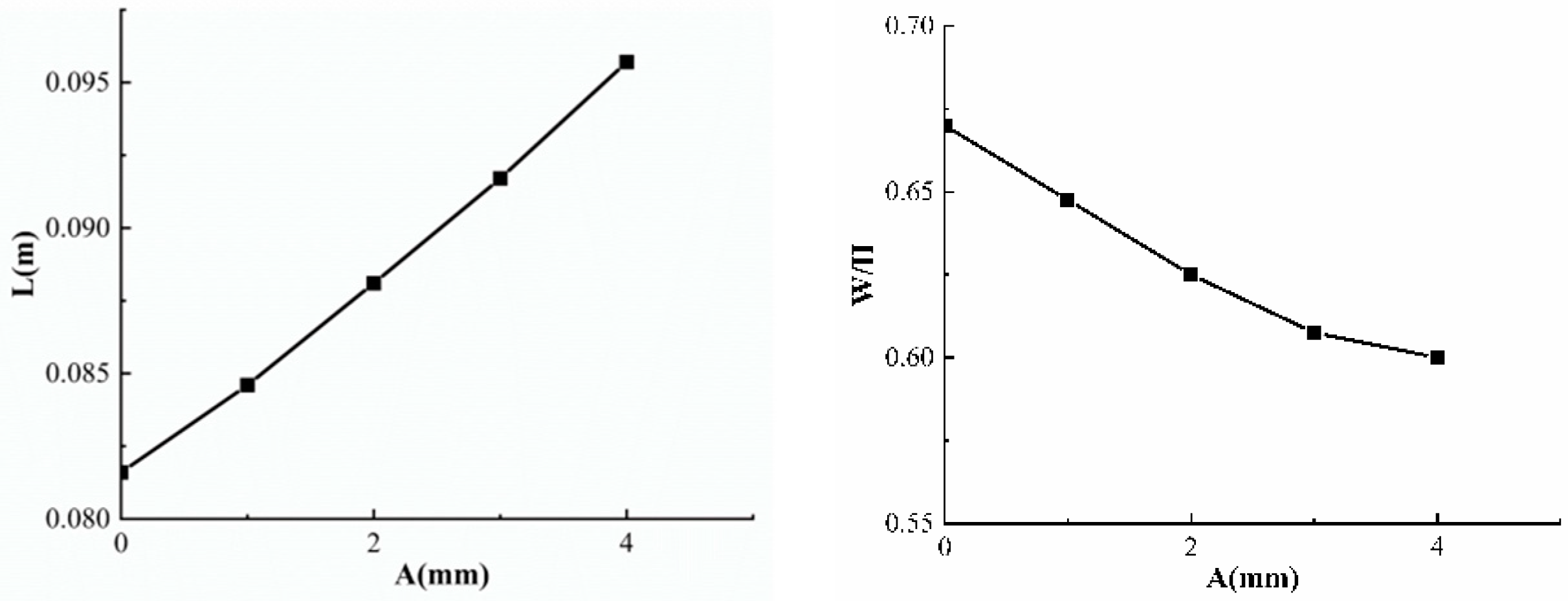
6. Effect of Different Amplitudes on Viscous Fingering
7. Conclusions
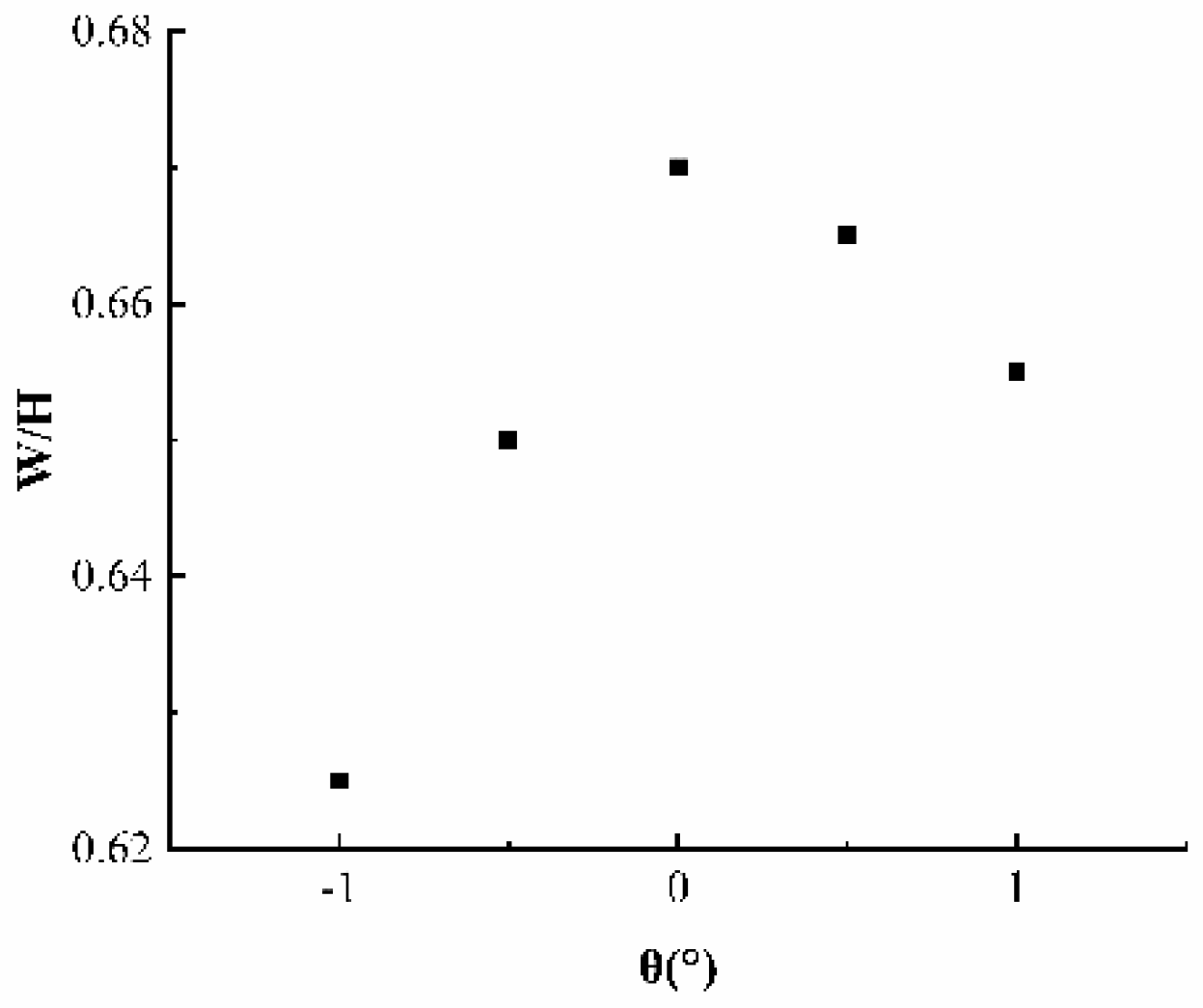
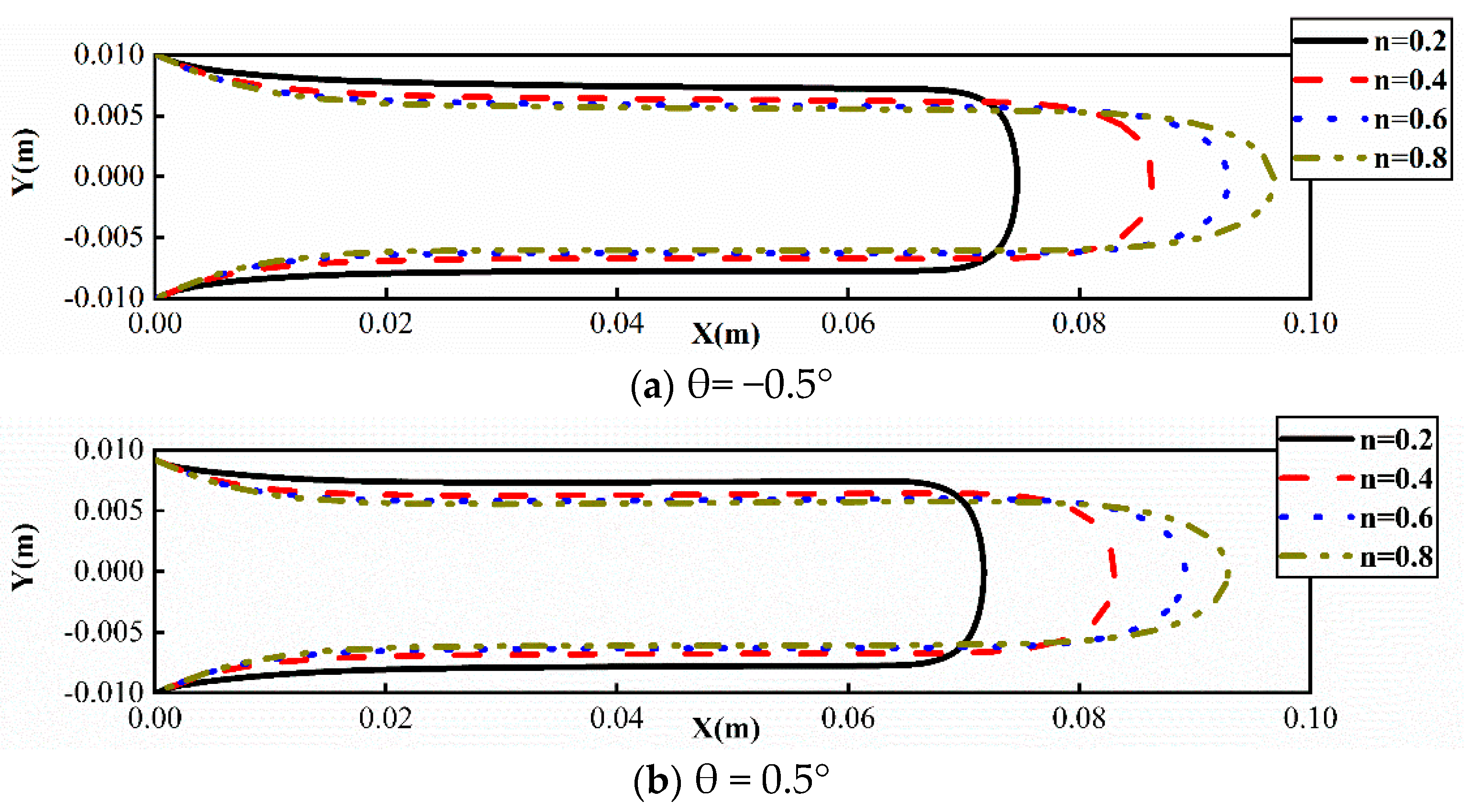
- When air is used to replace shear-thinning fluids in a classical HSC, a continuous tiny depth gradient is introduced, and this causes the viscous fingering length to be directly linked to the depth gradient. The gradient shifts from a negative to a positive value, which causes a progressive decrease in viscous fingering length. When there is a depth gradient, viscous fingering’s relative breadth shrinks but at , its relative breadth is at its greatest. Additionally, a positive depth gradient results in a wider relative breadth than a negative depth gradient. The smallest relative width is observed when the depth gradient is θ = −1°.
- The width of viscous fingering narrows and the distance travelled lengthens as the value of n rises, regardless of whether the depth gradient is positive or negative. This is due to the fact that when n decreases, the viscosity non-uniformity increases, and as the viscosity ratio rises, resistance also rises. The front end of the two-phase contact line moves more slowly as a result, and the flow distance is reduced. These qualities may be used in petroleum engineering to create high-quality and effective oil displacement through the introduction of innovative power-law fluids.
- It has been found that altering the form and amplitude of the top plate has no effect on viscous fingering when the control amplitude is maintained constant. Additionally, for various amplitudes (A), there is a linear connection between amplitude and length. This implies that the viscous finger moves farther as the amplitude rises. On the hand, a power-exponential function may be seen in the connection between the relative width and amplitude, where the relative width shrinks as the amplitude increases. Engineers may easily calculate the displacement efficiency in engineering applications by using these concepts to forecast the relative width and length of viscous fingering under various top plate amplitudes and geometries.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hosmy, G.M. Viscous Fingering in Porous Media. Annu. Rev. Fluid Mech. 1987, 19, 271–311. [Google Scholar]
- Wooding, R.A.; Morel-Seytoux, H.J. Multiphase Fluid Flow Through Porous Media. Annu. Rev. Fluid Mech. 1976, 8, 233–274. [Google Scholar] [CrossRef]
- Bensimon, D.; Kadanoff, L.P.; Liang, S.; Shraiman, B.I.; Tang, C. Viscous flows in two dimensions. Rev. Mod. Phys. 1986, 58, 977. [Google Scholar] [CrossRef]
- Chen, X.L.; Li, Y.Q.; Liao, G.Z.; Zhang, C.M.; Xu, S.Z.; Qi, H.; Tang, X. Experimental investigation on stable displacement mechanism and oil recovery enhancement of oxygen-reduced air assisted and oil recovery enhancement of oxygen-reduced air assisted gravity drainage. Pet. Explor. Dev. 2020, 47, 836–845. [Google Scholar] [CrossRef]
- Tsai, M.C.; Wei, S.Y.; Fang, L.; Chen, Y.C. Viscous fingering as a rapid 3D pattering Technique for Engineering cell-Laden Vascular-Like Constructs. Adv. Healthc. Mater. 2022, 11, 2101392. [Google Scholar] [CrossRef] [PubMed]
- Grotberg, J.B.; Jensen, O.E. Biofluid Mechanics in Flexible Tubes. Annu. Rev. Fluids Mech. 2004, 36, 121–147. [Google Scholar] [CrossRef]
- Huh, D.; Fujioka, H.; Tung, Y.C.; Futai, N.; Paine, R.; Grotberg, J.B.; Takayma, S. Acoustically detectable cellular-level lung injury induced by fluid mechanical stresses in microfluidic airway systems. Proc. Natl. Acad. Sci. USA 2007, 104, 18886–18891. [Google Scholar] [CrossRef]
- Nadirah, L.; Abdurahman, H.N.; Rizauddin, D. Rheological study of Petroleum fluid and oil-in water emulsion. Int. J. Eng. Sci. Res. Technol. 2014, 3, 2277–9655. [Google Scholar]
- Gorell, S.B.; Homsy, G.M. A theory of the optimal policy of oil recovery by secondary displacement processes. SlAM J. Appl. Math. 1983, 43, 79–98. [Google Scholar] [CrossRef]
- Orr, F.M., Jr.; Taber, J.J. Use of carbon dioxide in enhanced oil recovery. Science 1984, 224, 563–569. [Google Scholar] [CrossRef] [PubMed]
- Saffman, P.G.; Taylor, G.I. The Penetration of a fluid into a porous medium or Hele-Shaw cell containing a more viscous liquid. Proceeding of the Royal Society of London. Ser. A Math. Phys. Sci. 1958, 245, 312–329. [Google Scholar]
- Chuoke, R.L.; Van der Meurs, P.; Van der pole, C. The instability of slow, immiscible, viscous liquid-liquid displacement in permeable media. Trans. AIME 1959, 216, 188–194. [Google Scholar] [CrossRef]
- He, A.; Belmonte, A. Inertial effects on viscous fingering in the complex plane. J. Fluid Mech. 2011, 668, 436–445. [Google Scholar] [CrossRef]
- Al-Housseiny, T.T.; Tsai, P.A.; Stone, H.A. Control of interracial instabilities using flow geometry. Nat. Phys. 2012, 8, 747–750. [Google Scholar] [CrossRef]
- Eriksen, F.K.; Moura, M.; Jankov, M.; Turquet, A.L. Transition from viscous fingers to compact displacement during unstable drainage in porous media. Phys. Rev. Fluids 2022, 7, 013901. [Google Scholar] [CrossRef]
- Singh, A.; Pandey, K.M.; Singh, Y. CFD analysis of viscous fingering in Hele-Shaw cell for air-glycerine system. Mater. Today Proc. 2021, 45, 6381–6385. [Google Scholar] [CrossRef]
- Redapangu, P.R.; Vanka, S.P.; Sahu, K.C. Multiphase lattice Bolt zmann simulations of buoyancy-induced flow of two immiscible fluids with different viscosities. Eur. J. Mech. 2012, 34, 105–114. [Google Scholar] [CrossRef]
- Bakharev, F.; Campoli, L.; Enin, A.; Matveenko, S.; Petrova, Y.; Tikhomirov, S.; Yakovlev, A. Numerical investigation of viscous fingering phenomenon for raw field data. Transp. Porous Media 2020, 132, 443–464. [Google Scholar] [CrossRef]
- Guo, Y.A.; Cai, S.; Li, D. Effect and mechanism analysis of porous media heterogeneity on water-oil immiscible displacement. Sci. China Technol. Sci. 2022, 52, 807–818. [Google Scholar]
- Jha, B.; Cueto-Felgueroso, L.; Juanes, R. Fluid mixing from viscous fingering. Phys. Rev. Lett. 2011, 106, 194502. [Google Scholar] [CrossRef]
- Logvinov, O.A. Viscous fingering in poorly miscible power-law fluids. Phys. Fluids 2022, 34, 063105. [Google Scholar] [CrossRef]
- Qin, Z.; Wu, Y.T.; Ma, C.; Lyu, S.K. Effect of power law on viscous fingering behaviour of shear-thinning fluid in a lifted hele-shaw cell. J. Mech. Sci. Technol. 2023, 37, 3555–3562. [Google Scholar]
- Leung, L.T.Y.; Kowal, K.N. Lubricated viscous gravity currents of power-law fluids. Part 1. Self-similar flow regimes. J. Fluids Mech. 2022, 940, A26. [Google Scholar] [CrossRef]
- Lindner, A.; Bonn, D.; Poire, E.C.; Amar, M.B.; Meunier, J. Viscous fingering in non-newtonian fluids. J. Fluids Mech. 2002, 469, 237–256. [Google Scholar] [CrossRef]
- Pouplard, A.; Tsai, P.A. Viscous Fingering Instability of Complex Fluids in a Tapered Geometry. arXiv 2022, arXiv:2205.08019. [Google Scholar]
- Gallaway, J.W.; Desai, D.; Gaikwad, A.; Corredor, C.; Banerjee, S.; Steingart, D. A lateral microfluidic cell for imaging electro deposited zinc near the shorting condition. J. Electrochem. Soc. 2010, 157, A1279. [Google Scholar] [CrossRef]
- Taylor, G.I. Cavitation of a viscous fluid in narrow passages. J. Fluids Mech. 1963, 16, 595–619. [Google Scholar] [CrossRef]
- Shi, Y.; Tang, G.H. Simulation of Newtonian and non-Newtonian rheology behavior of viscous fingering in channels by the lattice Boltzmann method. Comput. Math. Appl. 2014, 68, 1279–1291. [Google Scholar] [CrossRef]








| Ca |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mafi, M.; Qin, Z.; Wu, Y.; Lyu, S.-K.; Ma, C. Research on the Interfacial Instability of Non-Newtonian Fluid Displacement Using Flow Geometry. Coatings 2023, 13, 1848. https://doi.org/10.3390/coatings13111848
Mafi M, Qin Z, Wu Y, Lyu S-K, Ma C. Research on the Interfacial Instability of Non-Newtonian Fluid Displacement Using Flow Geometry. Coatings. 2023; 13(11):1848. https://doi.org/10.3390/coatings13111848
Chicago/Turabian StyleMafi, MD, Zhen Qin, Yuting Wu, Sung-Ki Lyu, and Chicheng Ma. 2023. "Research on the Interfacial Instability of Non-Newtonian Fluid Displacement Using Flow Geometry" Coatings 13, no. 11: 1848. https://doi.org/10.3390/coatings13111848
APA StyleMafi, M., Qin, Z., Wu, Y., Lyu, S.-K., & Ma, C. (2023). Research on the Interfacial Instability of Non-Newtonian Fluid Displacement Using Flow Geometry. Coatings, 13(11), 1848. https://doi.org/10.3390/coatings13111848







