Thermal–Fluid–Solid Coupling Analysis on the Effect of Cooling Gas Temperature on the Fatigue Life of Turbine Blades with TBCs
Abstract
:1. Introduction
2. Geometric Models and Numerical Methods
2.1. Geometric Model
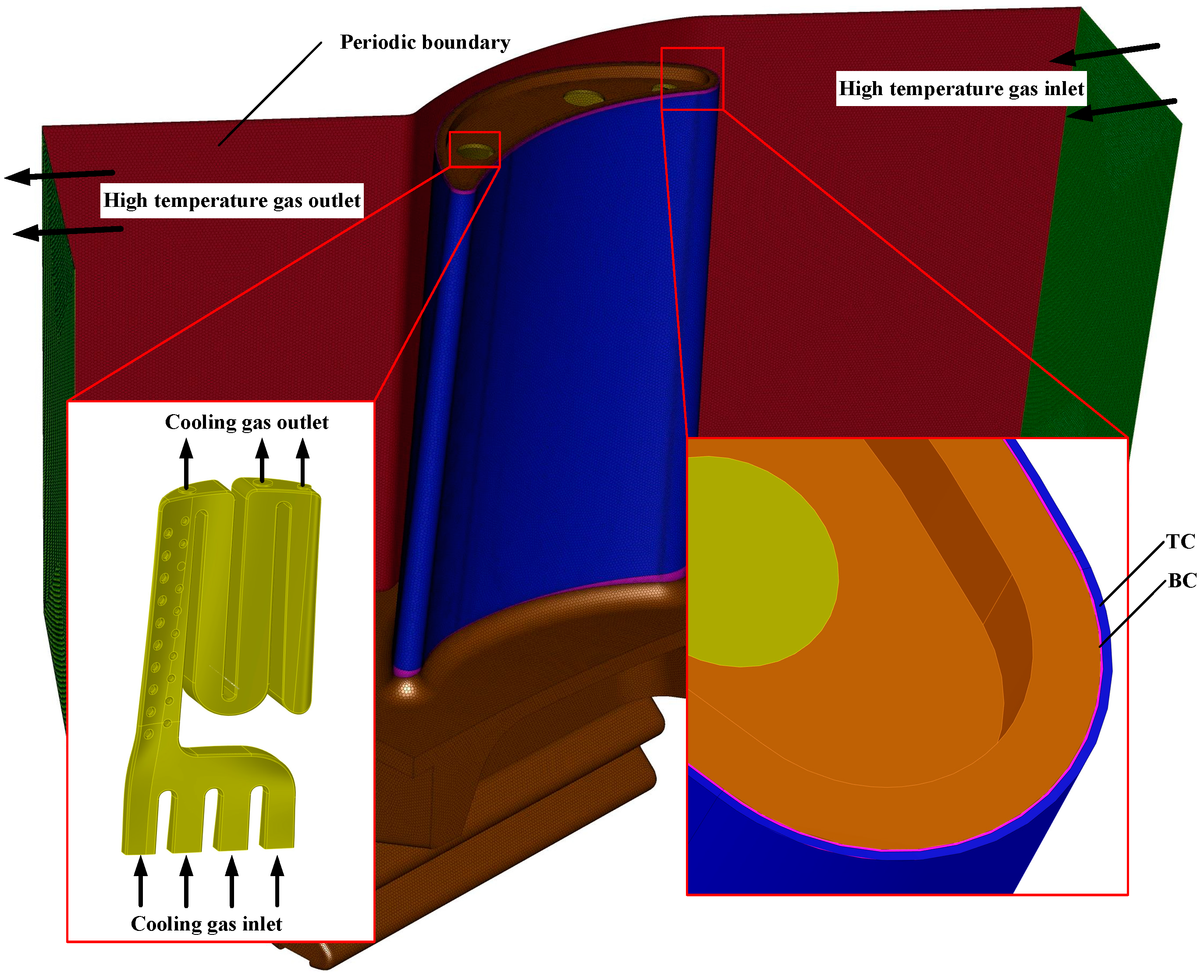
2.2. Boundary Conditions
2.3. Thermal–Fluid–Solid Coupling Numerical Method
2.4. Fatigue Life Analysis Method
3. Results and Discussion
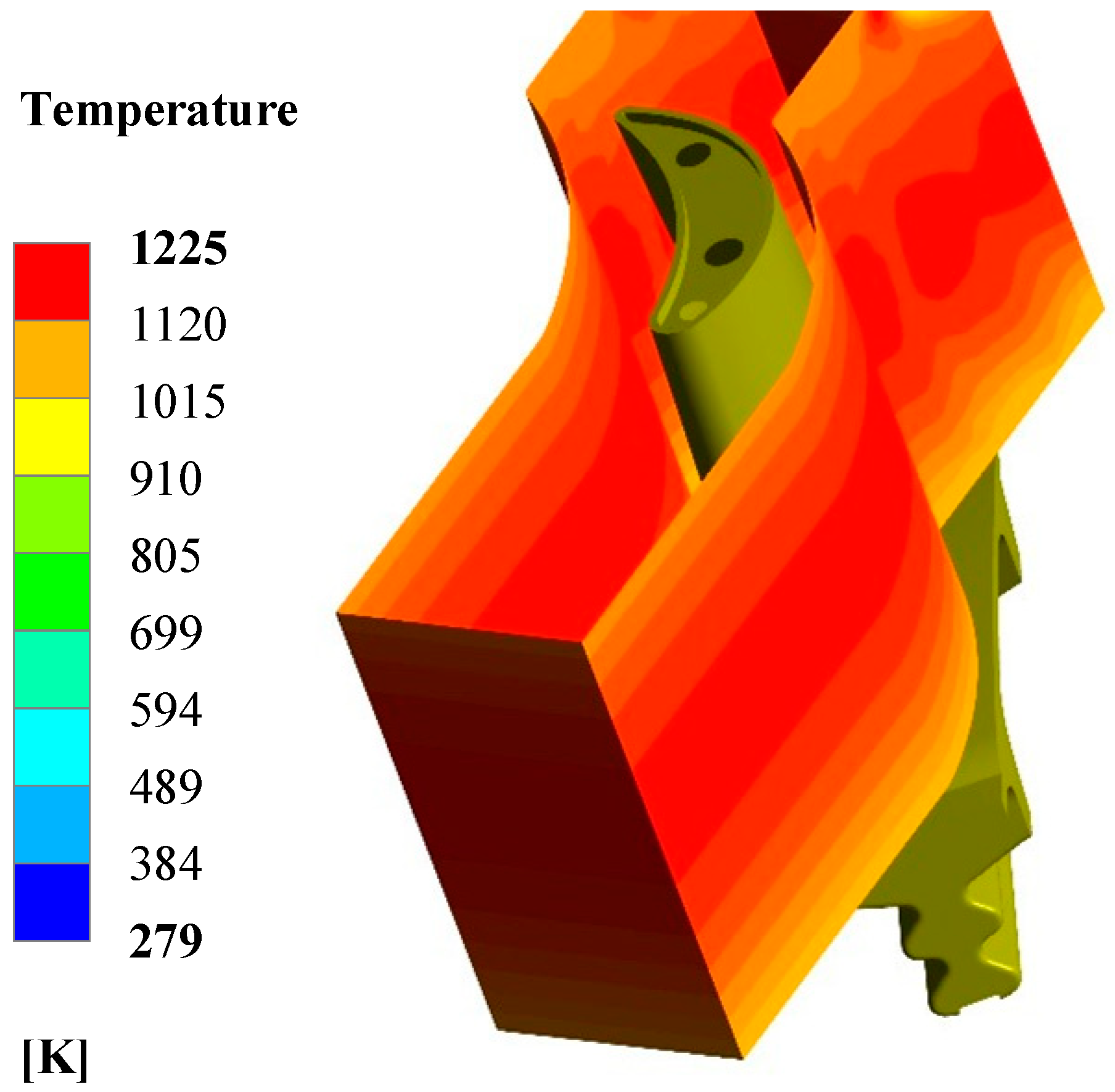
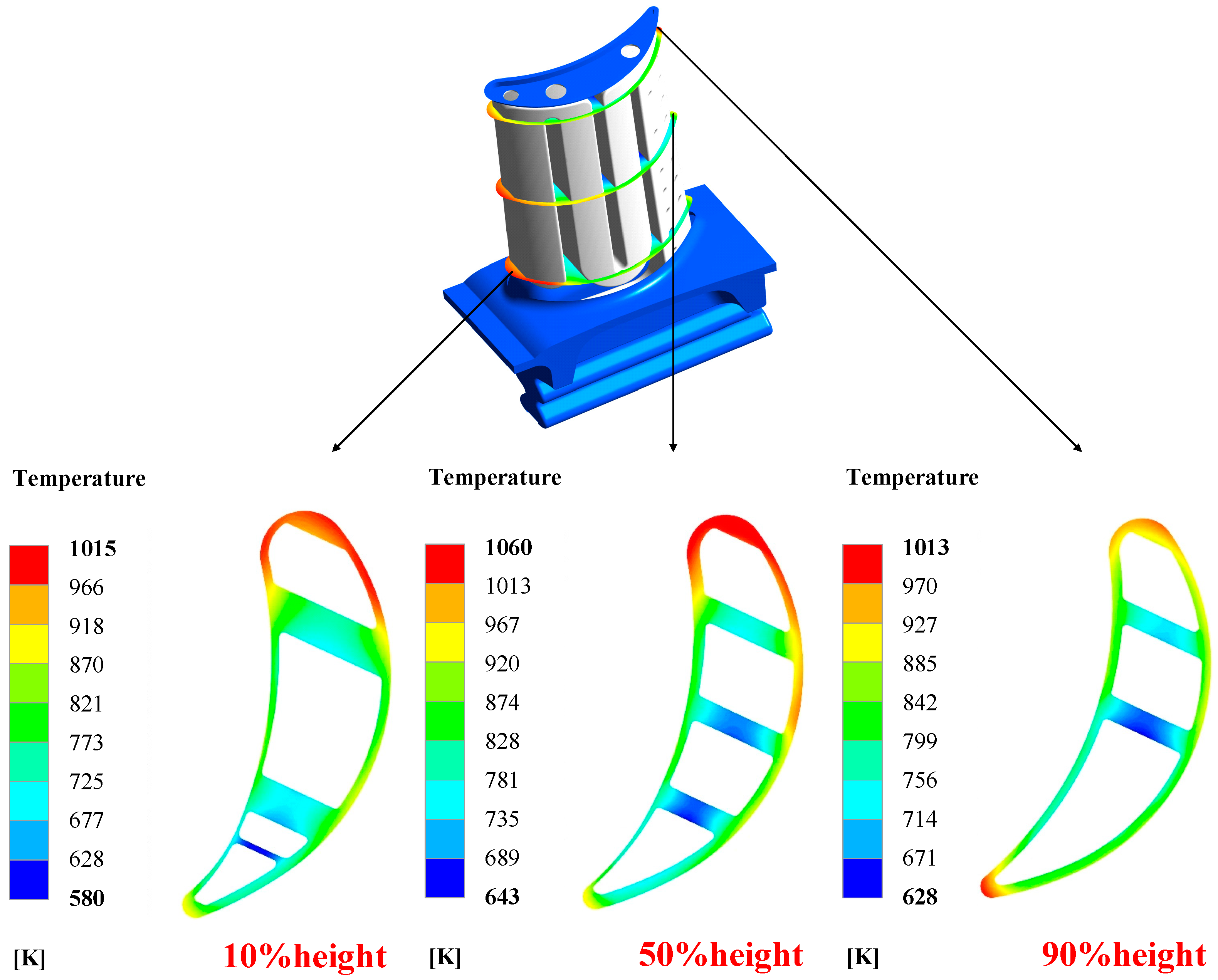
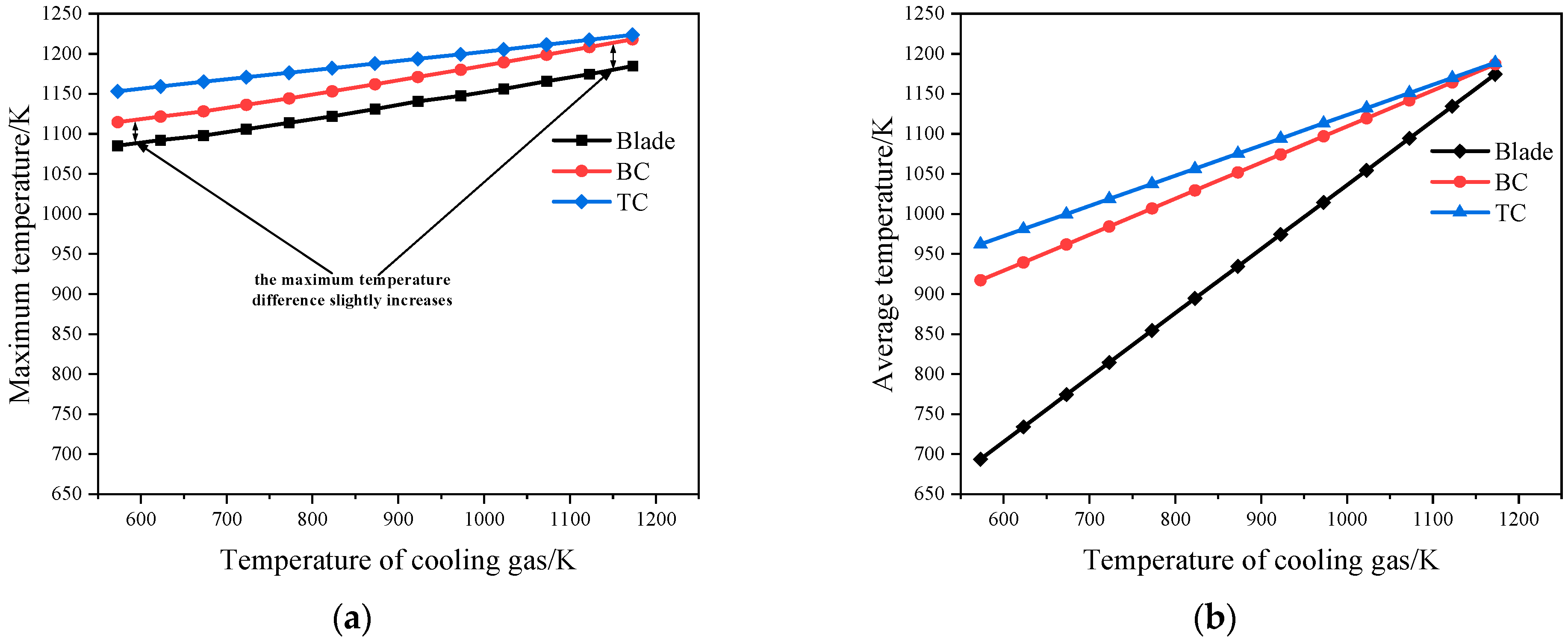
3.1. TBCs and Blade Heat Transfer Analysis
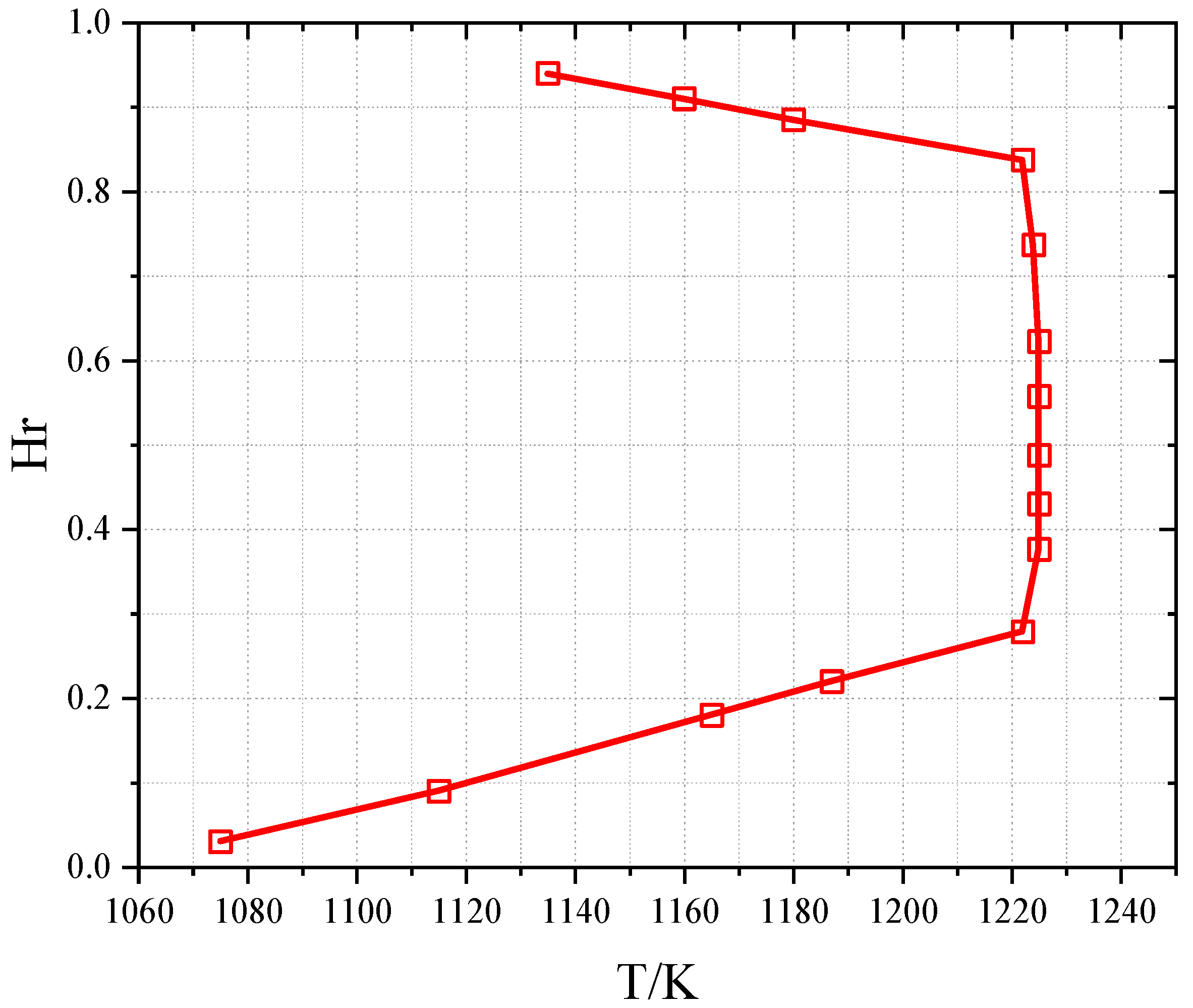
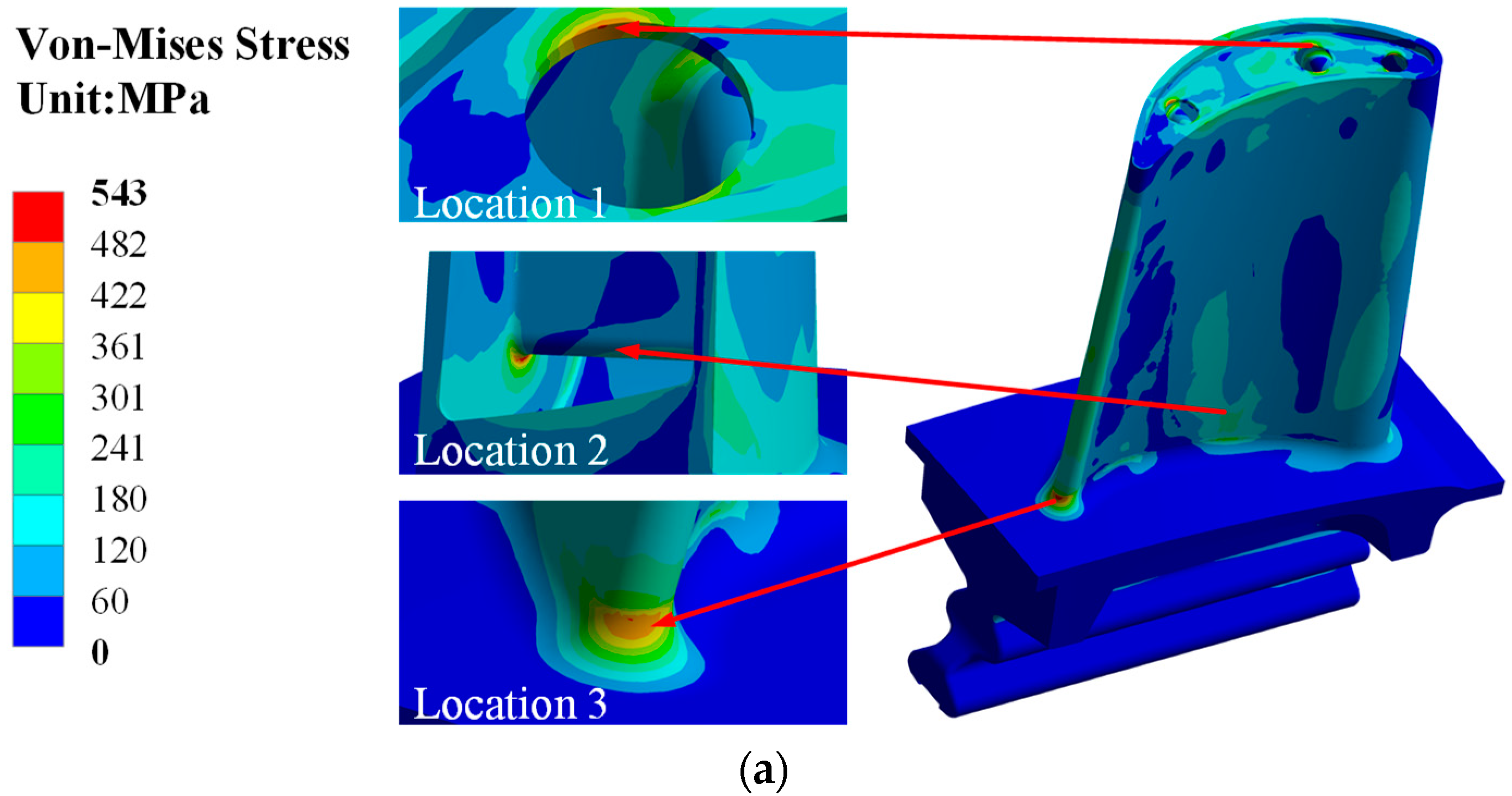
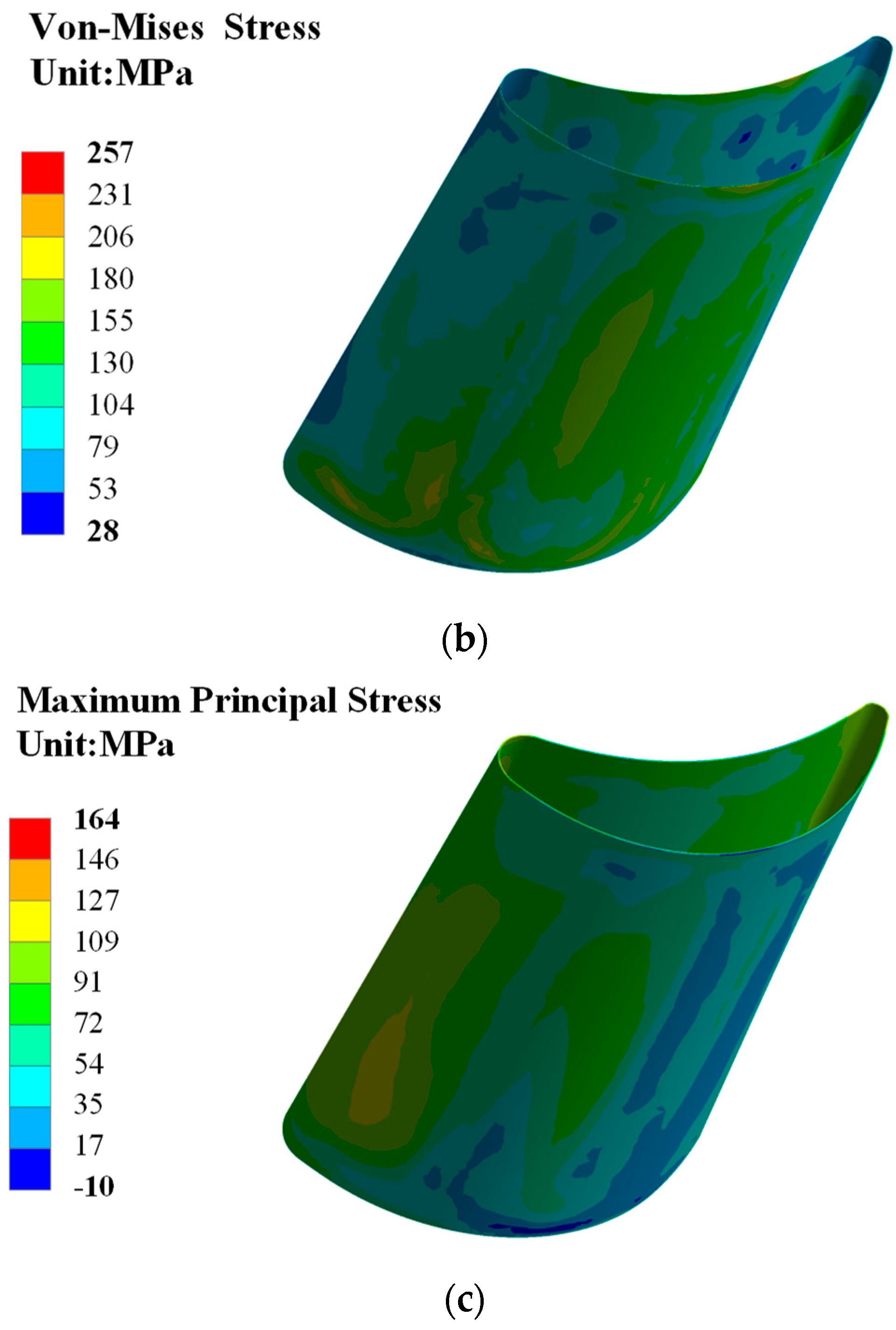
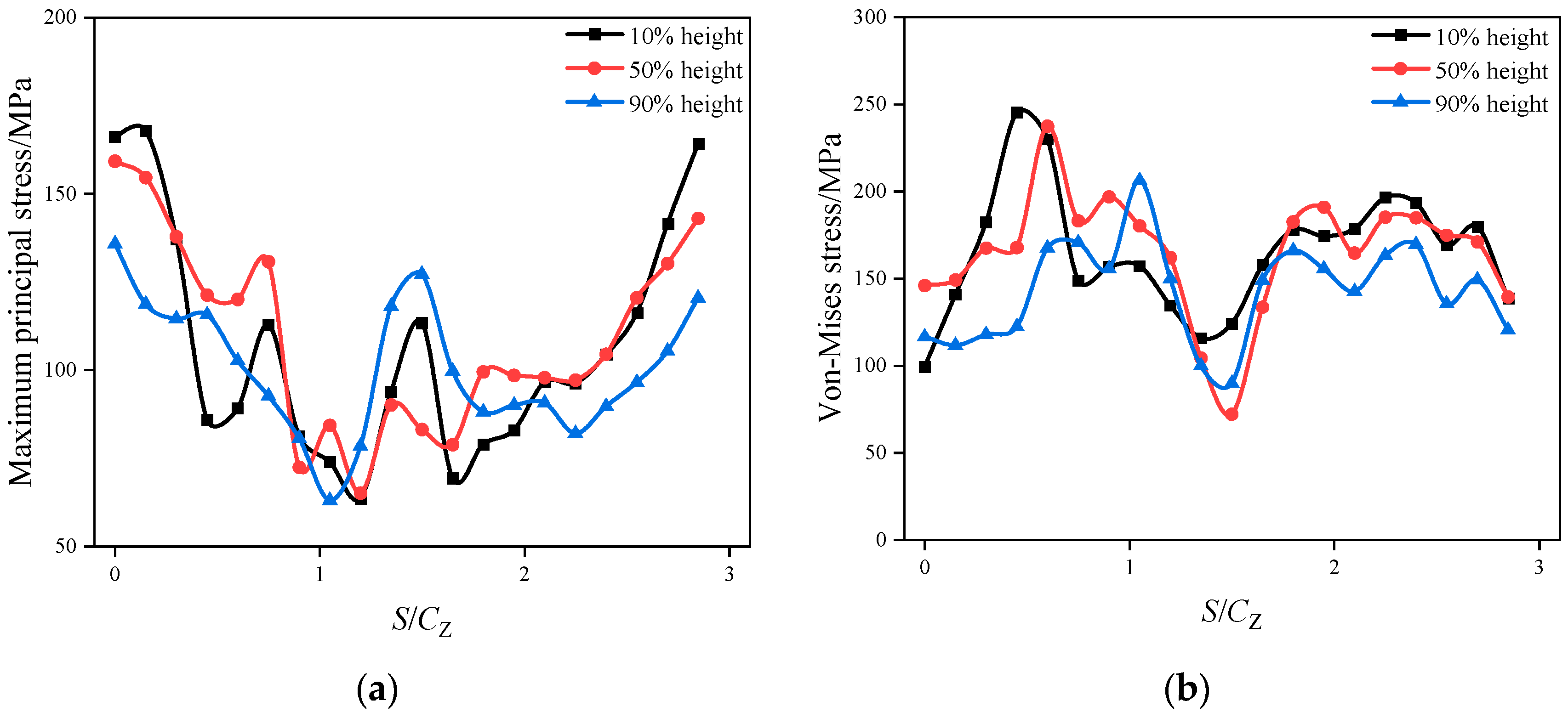
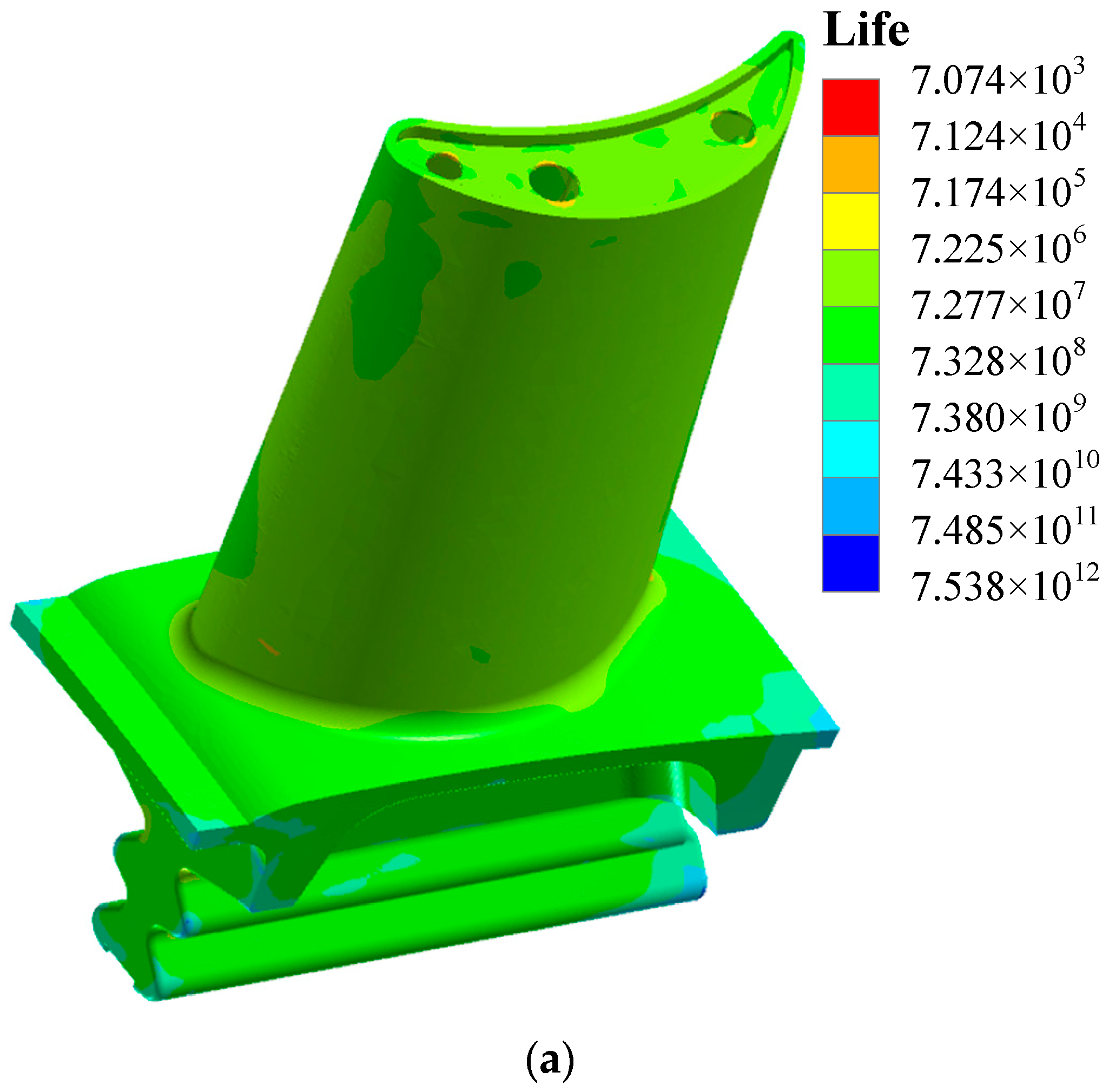
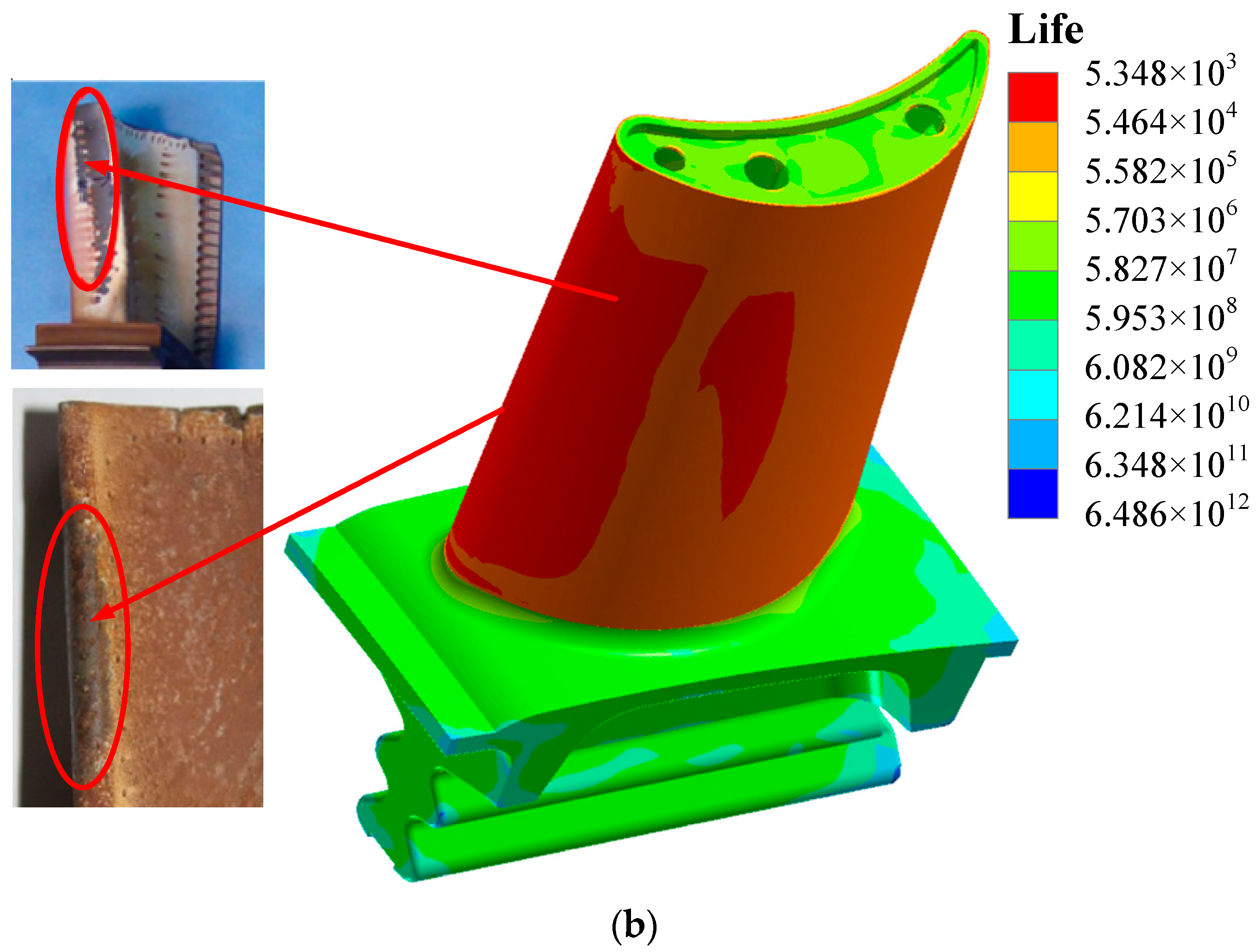
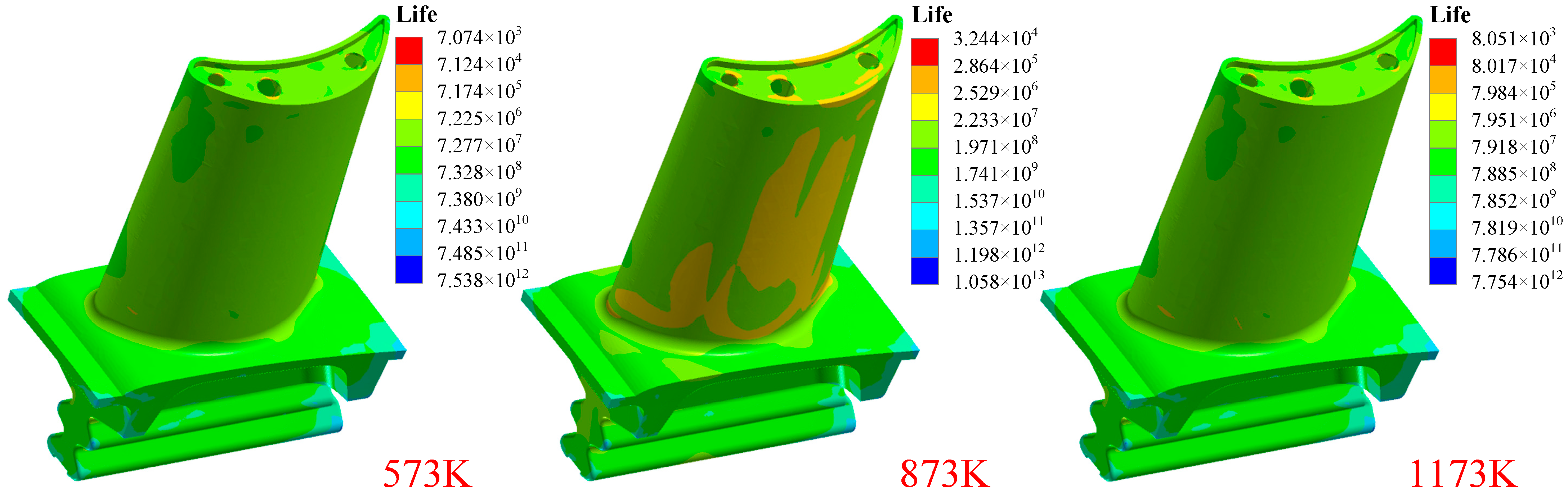
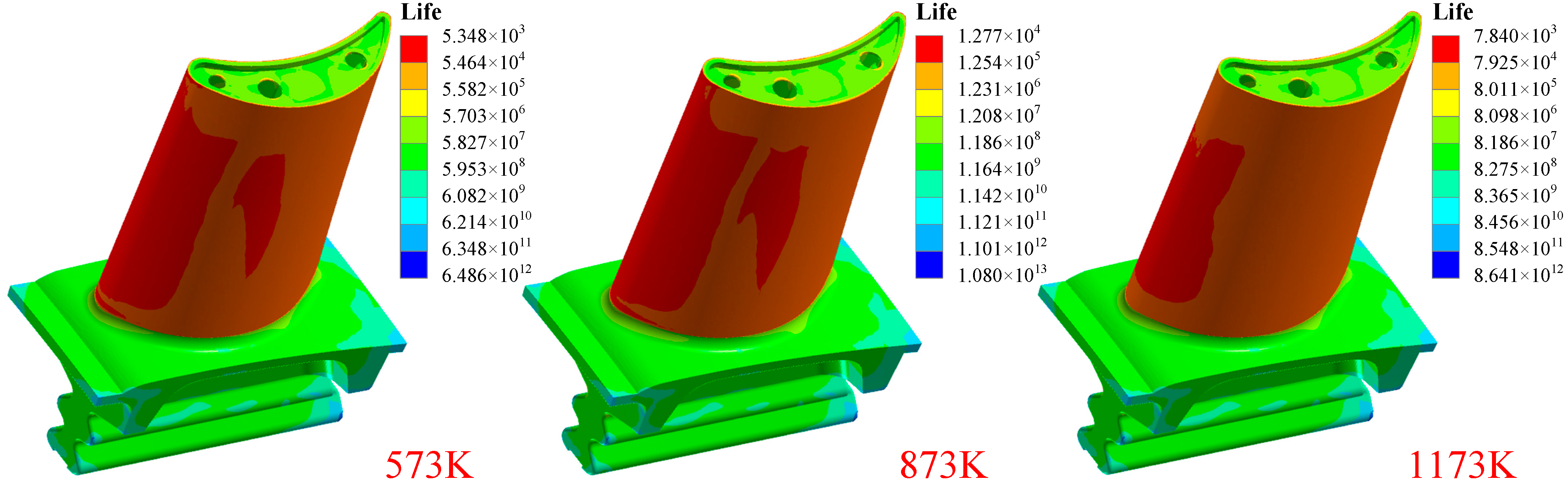
3.2. Fatigue Life Analysis
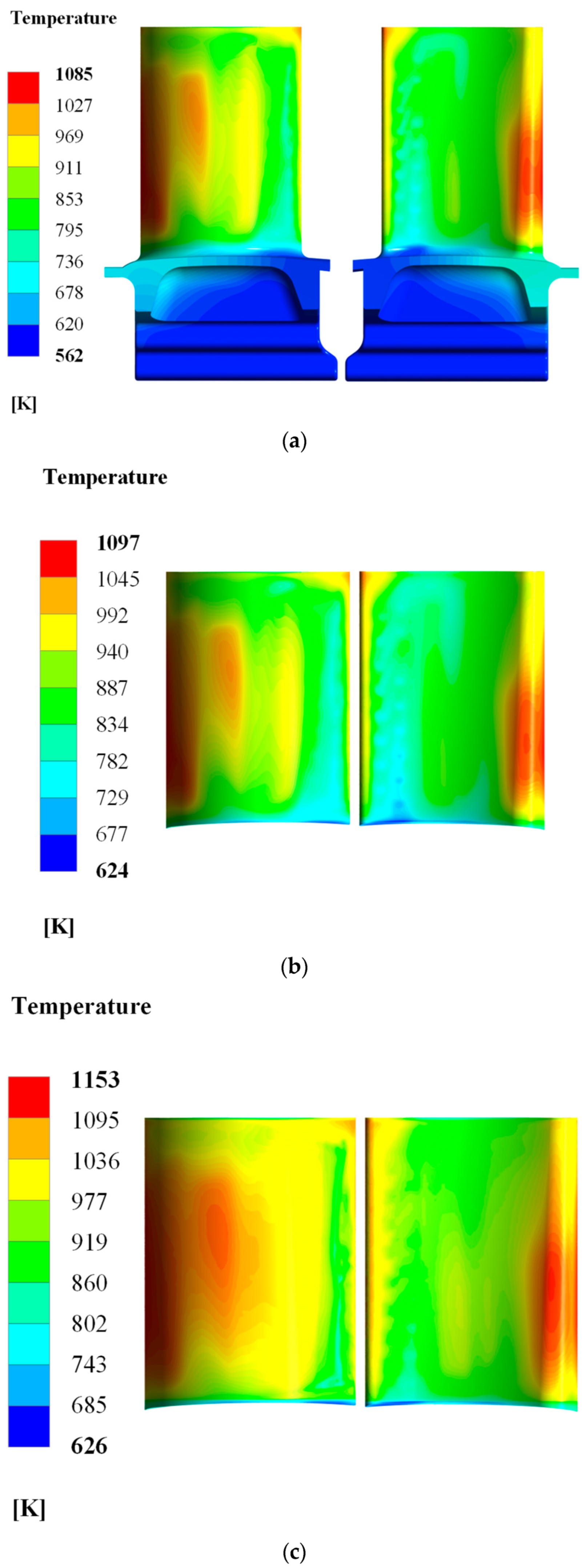
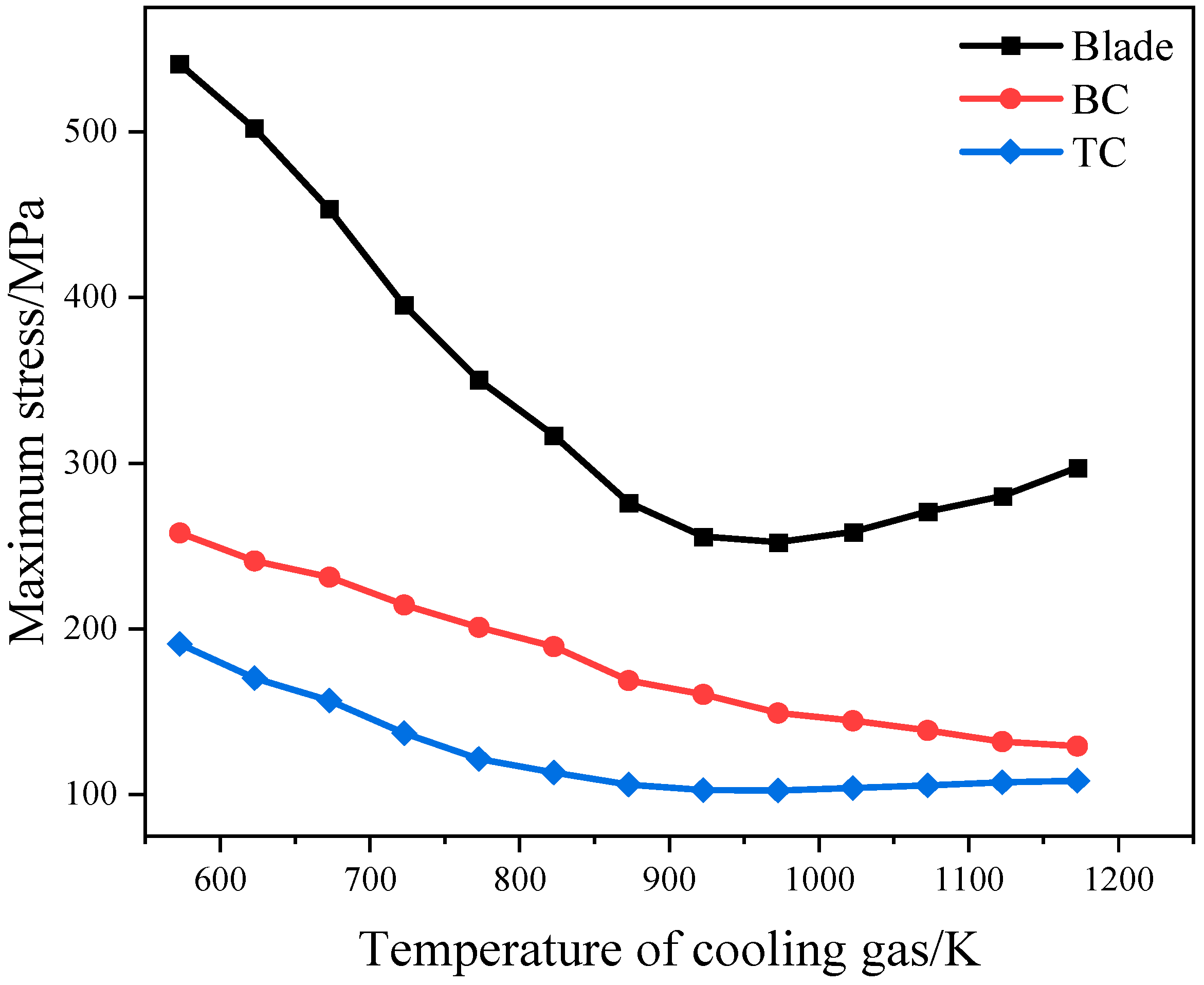
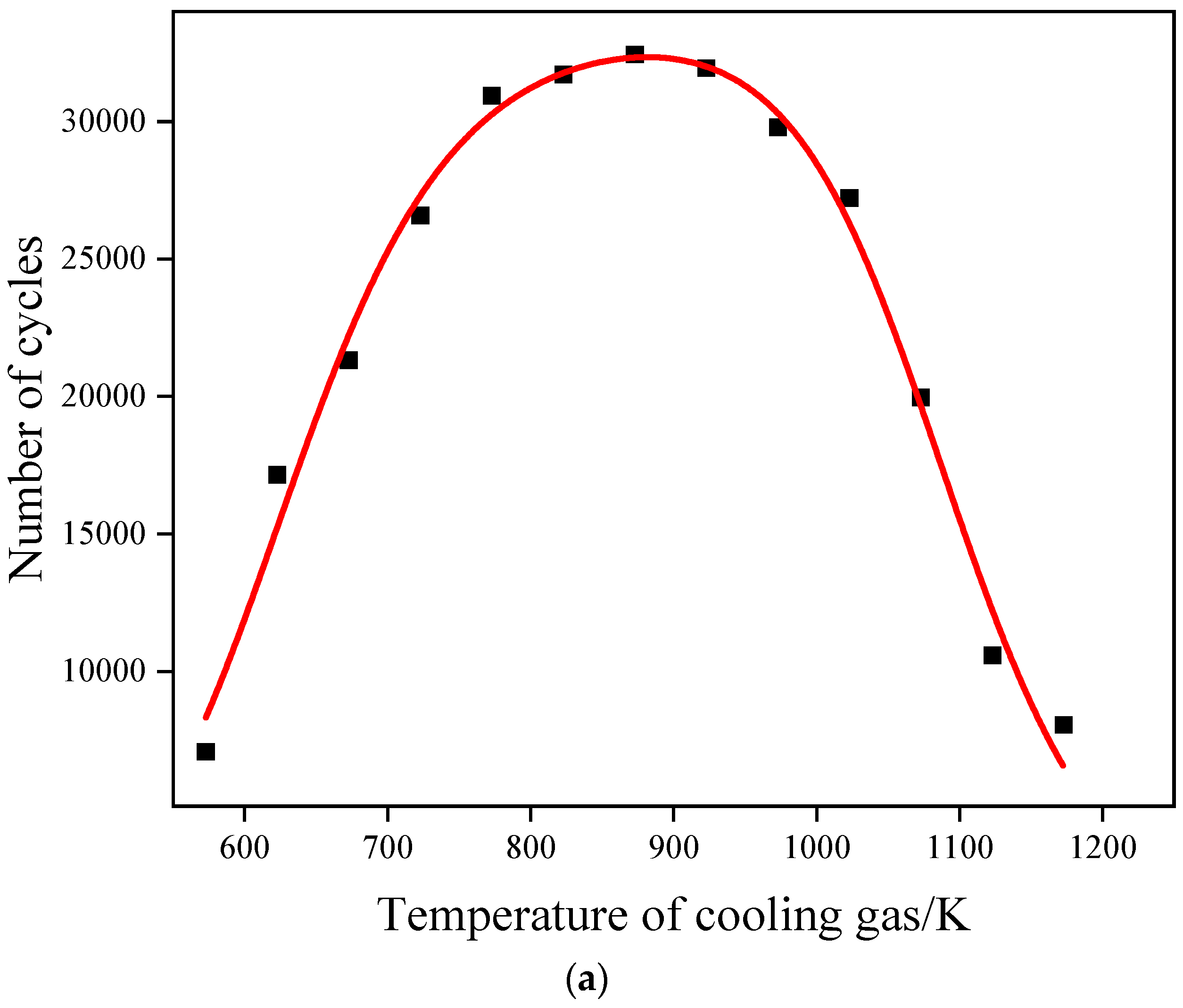
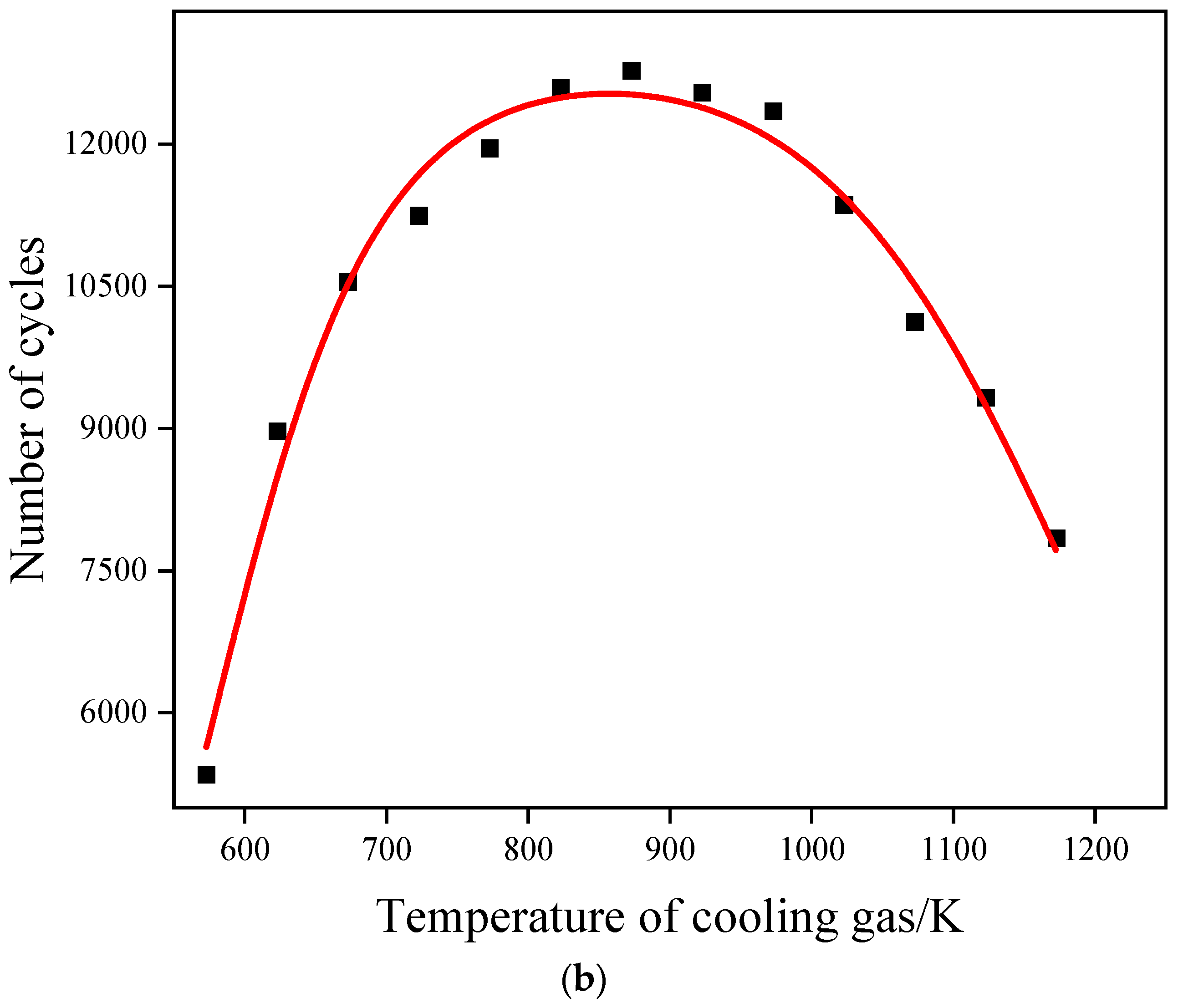
3.3. Influence of Cooling Gas Temperature on the Fatigue Life of Blade with TBCs
4. Conclusions and Future Work
- (1)
- The thermal insulation effect of the TBCs is different in different positions of the blade, and it shows a better heat insulation effect in the leading-edge region of the suction side of the blade, with a maximum temperature difference of 135.19 K. In the region close to the trailing edge of the blade, the TBCs show a poorer thermal insulation effect, with a minimum temperature difference of 14.13 K. This provides data support for the future design of thermal insulation for blades with TBCs.
- (2)
- The localized high thermal stresses in the blade and TBCs are related to the geometry of the blade in addition to the temperature gradient, and the fatigue life shows a significant negative correlation with the stresses. The fatigue life of the TBCs is lower than that of the blade, and the low-life region of the TBCs is located at the leading edge of the blade, which is consistent with the TBCs shedding region of the real blade and verifies the accuracy of the life prediction method in this paper. Providing new ideas for life prediction of blades with TBCs.
- (3)
- The life of the blade and TBCs increases and then decreases with increasing cooling gas temperature, with the opposite trend for stress. When the cooling gas temperature was increased from 573 K to 973 K, the maximum stress in the blade decreased by 53.4%, and the maximum stress in the TBCs decreased by 48.3%. When the cooling gas temperature is increased from 573 K to 873 K, the minimum life of the blade increases by 358.5%, and the minimum life of TBCs increases by 138.7%. Appropriately increasing the cooling gas temperature can effectively reduce the localized high stress levels in the blades and TBCs while increasing the service life of the blades and TBCs. This finding can provide guidance for the design of long-life blades with TBCs.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xiang, F.; He, W.; Dong, Y. Design of automatic interpretation and monitoring system for aeroengine parameters. Mech. Elect. Eng. Technol. 2022, 51, 149–152. [Google Scholar]
- Liu, C.G.; Wang, W. Research on manufacturing and remanufacturing technology of aero engine blade. Sci. Technol. Innov. Appl. 2022, 12, 145–148. [Google Scholar]
- Guo, H.B.; Gong, S.K.; Xu, H.B. Research progress of advanced aero-engine thermal barrier coating technology. J. Aeronaut. 2009, 28, 18–26. [Google Scholar]
- Caron, P.; Khan, T. Improvement of creep strength in a nickel-base single-crystal superalloy by heat treatment. Mater. Sci. Eng. 1983, 61, 173–184. [Google Scholar] [CrossRef]
- Miller, R.A. Thermal barrier coatings for aircraft engines: History and directions. J. Therm. Spray Technol. 1997, 6, 35–42. [Google Scholar] [CrossRef]
- Padture, N.P.; Gell, M.; Jordan, E.H. Thermal barrier coatings for gas-turbine engine applications. Science 2002, 296, 280–284. [Google Scholar] [CrossRef]
- Sahith, M.S.; Gridhara, G.; Kumar, R.S. Development and analysis of thermal barrier coatings on gas turbine blades—A Review. Mater. Today Proc. 2018, 5, 2746–2751. [Google Scholar] [CrossRef]
- Peng, Z.Y.; Lu, W.L. A comparative test approach for thermal fatigue life of high-heat blade under thermal shock. J. Aeronaut. 1997, 1, 34–37. [Google Scholar]
- Rezazadeh, R.M.; Alizadeh, M.; Fathi, A.; Khaledi, H. Turbine blade temperature calculation and life estimation-A Sensitivity Analysis. Propuls. Power. Res. 2013, 2, 148–161. [Google Scholar] [CrossRef]
- Khalesi, J.; Modaresahmadi, S.; Atefi, G. SEM gamma prime observation in a thermal and stress analysis of a first-stage rene’80H gas turbine blade: Numerical and experimental investigation. J. Sci. Technol. Trans. Mech. Eng. 2019, 43, 613–626. [Google Scholar] [CrossRef]
- Cai, L.X.; He, Y.; Wang, S.S.; Li, Y.; Li, F. Thermal-fluid-solid coupling analysis on the temperature and thermal stress field of a nickel-base superalloy turbine blade. Materials 2021, 14, 3315. [Google Scholar] [CrossRef] [PubMed]
- Guan, P.; Ai, Y.T.; Wang, Z.; Wang, T.F. Numerical simulation of nozzle guide vane subjected to thermal shock load. J. Propul. Technol. 2016, 37, 1938–1945. [Google Scholar]
- Yin, Y.Y.; Wang, H.W. Effect of non-uniform inlet temperature profiles on thermal stress in turbine blade. Energy Res. Inf. 2014, 30, 113–117. [Google Scholar]
- Qian, H.H.; Li, H.; Cheng, T. Thermal fatigue analysis to nozzle guide vanes. J. Aeronaut. 2003, 18, 186–190. [Google Scholar]
- Krishnamurthy, R.; Srolovitz, D.J. Sintering and microstructure evolution in columnar thermal barrier coatings. Acta Mater. 2009, 57, 1035–1048. [Google Scholar] [CrossRef]
- Tsipas, S.A. Effect of dopants on the phase stability of zirconia-based plasma sprayed thermal barrier coatings. J. Eur. Ceram. Soc. 2010, 30, 61–72. [Google Scholar] [CrossRef]
- Wang, L.; Li, D.C.; Yang, J.S. Modeling of thermal properties and failure of thermal barrier coatings with the use of finite element methods: A review. J. Eur. Ceram. Soc. 2016, 36, 1313–1331. [Google Scholar] [CrossRef]
- Liu, Q.M.; Huang, S.Z.; He, A.J. Composite ceramics thermal barrier coatings of yttria stabilized zirconia for aero-engines. J. Mater. Sci. Technol. 2019, 35, 2814–2823. [Google Scholar] [CrossRef]
- Sohn, Y.H.; Lee, E.Y.; Nagaraj, B.A. Microstructural characterization of thermal barrier coatings on high pressure turbine blades. Surf. Coat. Technol. 2001, 146, 132–139. [Google Scholar] [CrossRef]
- Yang, L.; Liu, Q.X.; Zhou, Y.C.; Mao, W.G.; Lu, C. Finite element simulation on thermal fatigue of a turbine blade with thermal barrier coatings. J. Mater. Sci. Technol. 2014, 30, 371–380. [Google Scholar] [CrossRef]
- Wei, H.L.; Yang, X.G.; Qi, H.Y. Study on thermal fatigue life prediction for plasma sprayed thermal barrier coatings on the surface of turbine vane. J. Aerosp. Power. 2008, 23, 1–8. [Google Scholar]
- Hao, Y.Y.; Liang, L.H.; Qiu, T. Residual stress and high-temperature mechanical behavior of thermal barrier coated turbine blades. J. Theor. App. Mech. 2023, 55, 1319–1328. [Google Scholar]
- Shi, L.; Sun, Z.Y.; Lu, T.F. Numerical study on the effects of thermal barrier coating and cooling flow rate on cooling performance of coated turbine blades. J. Therm. Sci. Technol. 2022, 21, 227–234. [Google Scholar]
- Takahashi, T.; Watanabe, K.; Fujii, T.; Fujioka, T. Numerical analysis of temperature distribution of a film-cooled and TBC coated blade. In Proceedings of the ASME Turbo Expo 2008: Power for Land, Sea and Air, Berlin, Germany, 9–13 June 2008. [Google Scholar]
- Sadowski, T.; Golewski, P. Cracks path growth in turbine blades with TBC under thermo-mechanical cyclic loadings. Frat. Integrita Strutt. 2016, 35, 492–499. [Google Scholar] [CrossRef]
- Hou, N.X.; Wen, Z.X.; Yu, Q.M.; Yue, Z.F. Application of a combined high and low cycle fatigue life model on life prediction of SC blade. Int. J. Fatigue 2009, 31, 616–619. [Google Scholar] [CrossRef]
- Menter, F.R. Review of the shear-stress transport model experience from an industrial perspective. Int. J. Comput. Fluid D 2009, 23, 305–316. [Google Scholar] [CrossRef]
- Ho, K.; Liu, J.; Elliott, T.; Aguilar, B. Conjugate heat transfer analysis for gas turbine film-cooled blade. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, Republic of Korea, 13–17 June 2016. [Google Scholar]
- Yang, A.F. Cumulative fatigue damage and life prediction theories: A survey of the state of the art for homogeneous materials. Int. J. Fatigue 1998, 20, 9–34. [Google Scholar]
- Nie, H. The energy basis of Miner formula and Manson Coffin formula. J. Aeronaut. 1993, 14, 3. [Google Scholar]
- Ziaei-Asl, A.; Ramezanlou, M. Thermo-mechanical behavior of gas turbine blade equipped with cooling ducts and protective coating with different thicknesses. Int. J. Mech. Sci. 2019, 150, 656–664. [Google Scholar] [CrossRef]
- Darolia, R. Thermal barrier coatings technology: A critical review, progress update, remaining challenges and prospects. Int. Mater. Rev. 2013, 58, 315–348. [Google Scholar] [CrossRef]
- Hu, X.A.; Shi, D.Q.; Pang, X.J.; Yang, X.G. Thermal barrier coating life prediction method for a turbine blade. J. Aerosp. Power 2018, 33, 48–53. [Google Scholar]



| Temperature Range (°C) | Young’s Modulus (GPa) | Poisson’s Ratio | Thermal Expansion Coefficient (10−6/°C) | Yield Strength (MPa) | Thermal Conductivity (W/(m·K)) | Specific Heat (J/(kg·K)) | Density (kg/m3) | |
|---|---|---|---|---|---|---|---|---|
| Bond coat | 20–1600 | 200–110 | 0.30–0.33 | 13.6–17.6 | 426–114 | 5.8–17.0 | 450 | 7380 |
| Top coat | 20–1600 | 48–22 | 0.10–0.12 | 9.0–12.2 | - | 2.0–1.7 | 505 | 3610 |
| Ta K | λa W/m·K | Cpa J/kg·K | E GPa | μa - | Cte K−1 |
|---|---|---|---|---|---|
| 298.15 | 8.45 | 469 | 129.9 | 0.3 | - |
| 373.15 | 10 | 474 | 128 | 0.3 | 1.19 × 10−5 |
| 473.15 | 11.95 | 482 | 126 | 0.3 | 1.24 × 10−5 |
| 573.15 | 13.8 | 491 | 123 | 0.3 | 1.26 × 10−5 |
| 673.15 | 15.5 | 501 | 118 | 0.3 | 1.29 × 10−5 |
| 773.15 | 17.1 | 511 | 114 | 0.3 | 1.32 × 10−5 |
| 873.15 | 18.55 | 522 | 110 | 0.3 | 1.36 × 10−5 |
| 973.15 | 19.85 | 534 | 106 | 0.3 | 1.40 × 10−5 |
| 1073.15 | 21 | 547 | 101 | 0.3 | 1.45 × 10−5 |
| 1173.15 | 22 | 561 | 95 | 0.3 | 1.50 × 10−5 |
| 1273.15 | 22.8 | 575 | 86 | 0.3 | 1.56 × 10−5 |
| Parameters | Value | Units |
|---|---|---|
| Total temperature at the inlet of the main gas flow domain | Tin | K |
| Total pressure at the inlet of the main gas flow domain | 1.5 | MPa |
| Molar mass of gas | 28.29 | kg/kmol |
| Specific heat capacity of gas | Cpgas | J/kg·K |
| Kinematical viscosity coefficient of gas | 1.831 × 10−5 | kg/m·s |
| Thermal conductivity coefficients of gas | 2.61 × 10−5 | W/m·K |
| Mass flow at the outlet of the main gas flow domain | 6.5632 | kg/s |
| Mass flow at the inlet of cooling airflow | 0.3321 | kg/s |
| Total temperature at the inlet of cooling airflow | 573.15 | K |
| Rotation speed | 36,000 | rpm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Zhang, Z.; Ai, Y.; Guan, P.; Yao, Y.; Liu, H. Thermal–Fluid–Solid Coupling Analysis on the Effect of Cooling Gas Temperature on the Fatigue Life of Turbine Blades with TBCs. Coatings 2023, 13, 1795. https://doi.org/10.3390/coatings13101795
Chen Y, Zhang Z, Ai Y, Guan P, Yao Y, Liu H. Thermal–Fluid–Solid Coupling Analysis on the Effect of Cooling Gas Temperature on the Fatigue Life of Turbine Blades with TBCs. Coatings. 2023; 13(10):1795. https://doi.org/10.3390/coatings13101795
Chicago/Turabian StyleChen, Yingtao, Ziliang Zhang, Yanting Ai, Peng Guan, Yudong Yao, and Hongwei Liu. 2023. "Thermal–Fluid–Solid Coupling Analysis on the Effect of Cooling Gas Temperature on the Fatigue Life of Turbine Blades with TBCs" Coatings 13, no. 10: 1795. https://doi.org/10.3390/coatings13101795
APA StyleChen, Y., Zhang, Z., Ai, Y., Guan, P., Yao, Y., & Liu, H. (2023). Thermal–Fluid–Solid Coupling Analysis on the Effect of Cooling Gas Temperature on the Fatigue Life of Turbine Blades with TBCs. Coatings, 13(10), 1795. https://doi.org/10.3390/coatings13101795






