Simulation Evaluation of a Novel Ice-Melting Sprinkling Technique for Blade
Abstract
:1. Introduction
2. Modeling and Grid-Independent Verification
2.1. Materials and Models
2.2. Regulating Equations
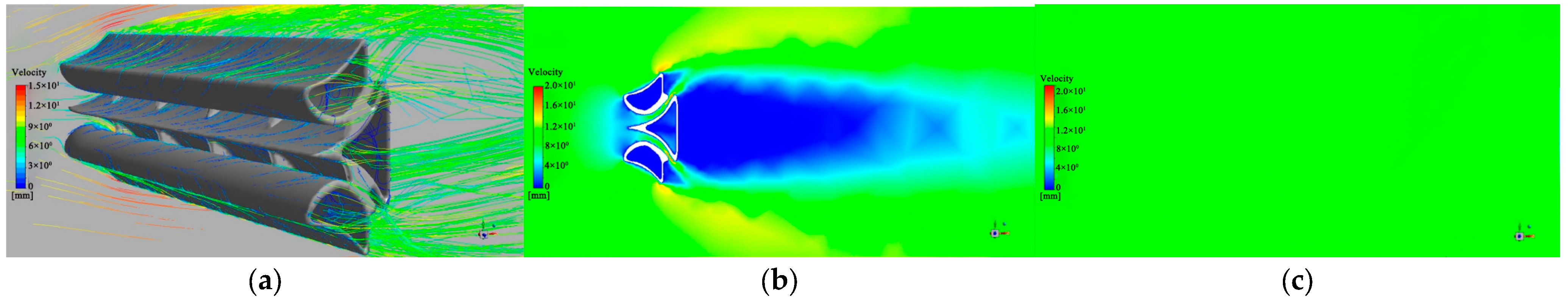
2.3. Choice of Boundary Conditions
2.4. Grid-Independent Verification
3. Result and Discussion
3.1. Influence of a Single Variable on Liquid Film Thickness and Coverage
3.1.1. Single-Factor Effect Parameter Configuration
3.1.2. Influence of Blade Strike Angle Variation
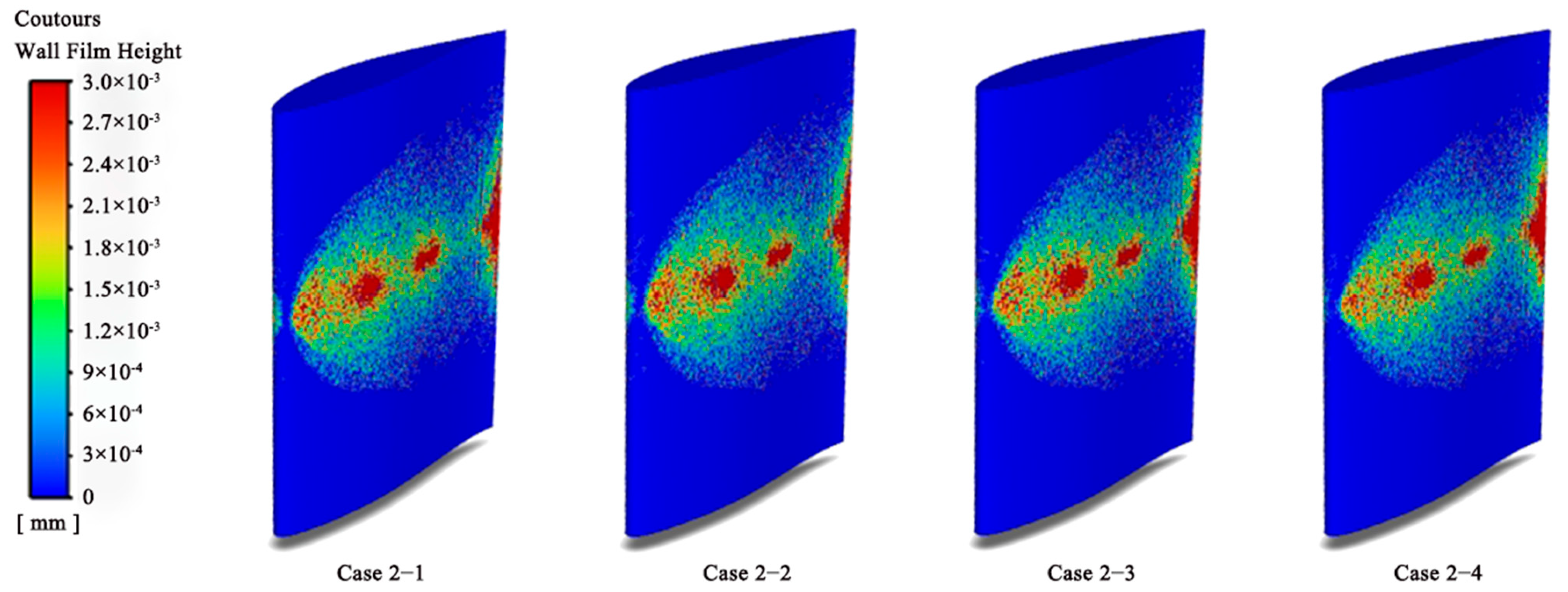
3.1.3. Influence of Variable Inlet Air Velocity
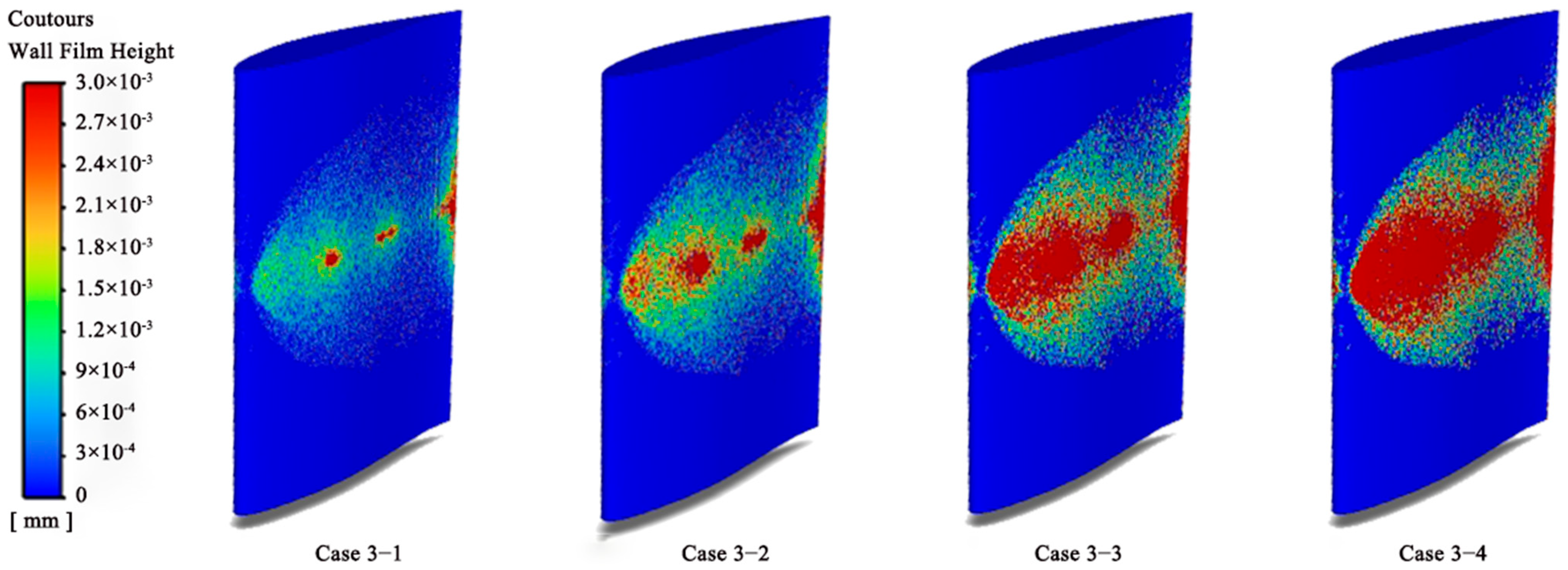
3.1.4. Influence of Changes in Nozzle Mass Flow Rate
3.2. Effects of Multi-Factor Changes on Liquid Film Thickness and Coverage
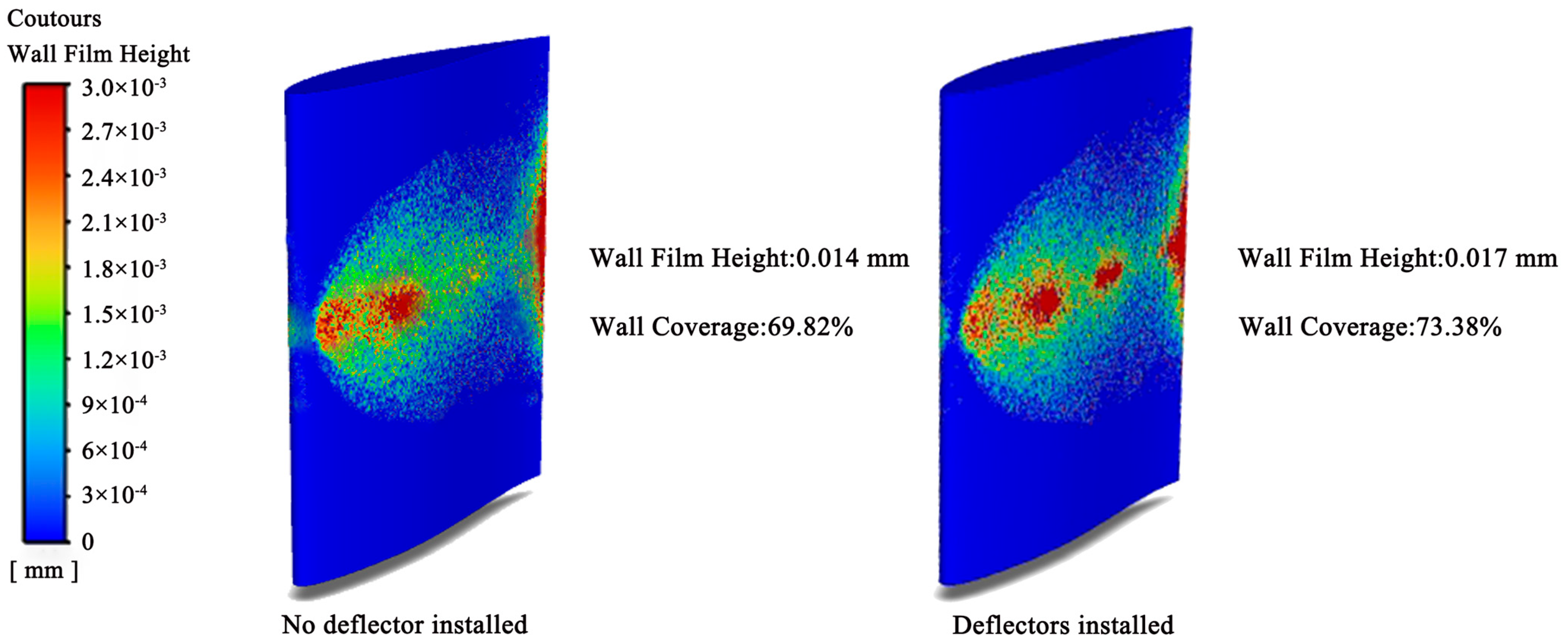
3.3. Verification of Deflector Effect
4. Conclusions
- (1)
- Upon studying the effects of the blade angle of attack separately, the inlet incoming wind speed, and the nozzle mass flow rate on the liquid film thickness and liquid film coverage, the following laws were derived: a 30° angle of attack and a 60° angle of attack produce the highest liquid film thickness and coverage, respectively. In contrast, a 90° angle of attack produces the lowest liquid film thickness and coverage. In terms of the inlet incoming air velocity, lower air velocities result in a greater film thickness and coverage, with the film thickness and coverage diminishing as the air velocity increases. In terms of the nozzle mass flow rate, using a nozzle with a higher mass flow rate can effectively improve the liquid film thickness and coverage, but as the mass flow rate increases, the magnitude of the improvement diminishes.
- (2)
- Using the orthogonal experimental method to determine the level of influence of the factors on the liquid film thickness and coverage, as well as the optimal combination of working conditions, the results demonstrate the following: In descending order, the influences on the liquid film thickness and coverage are the nozzle mass flow rate, blade angle of attack, and inlet inflow wind speed. The liquid film thickness was maximized at 0.037 mm for the optimal combination of conditions of α = 30°, u0 = 6 m/s, and Q = 0.003 kg/s, and the liquid film coverage was maximized at 99.81% for the optimal combination of conditions of α = 60°, u0 = 6 m/s, and Q = 0.003 kg/s.
- (3)
- Using CFD simulation, the flow-guiding effect of the deflector plate was validated. The deflector plate can cause the discharged liquid to form a benign cycle, effectively enhance the liquid film thickness and coverage, and increase the average liquid film thickness by 21.43% and the average liquid film coverage by 3.56%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| cp | Specific heat capacity | ST | Viscous dissipation term |
| I | Turbulence intensity | x, y, z | Cartesian space coordinates |
| g | Gravity | u, v, w | The velocity components in the x, y, and z directions, respectively |
| HL | Wall film height of leading edge of blade | u0 | Inlet incoming air velocity |
| HM | Average wall film height of blade | α | Blade angle of attack |
| HT | Wall film height of trailing edge of blade | ρ | Density |
| k | Heat transfer coefficient of fluid | Φ | General variables, which can represent u, v, and w |
| p0 | Outlet gauge pressure | Γ | Diffusion coefficient |
| Q | Mass flow | T | Temperature |
| SΦ | Source item | U | Velocity vector |
References
- O’Neill, B.C.; Dalton, M.; Fuchs, R.; Jiang, L.; Pachauri, S.; Zigova, K. Global demographic trends and future carbon emissions. Proc. Natl. Acad. Sci. USA 2010, 107, 17521–17526. [Google Scholar] [CrossRef] [PubMed]
- Manieniyan, V.; Thambidurai, M.; Selvakumar, R. Study on energy crisis and the future of fossil fuels. Proc. SHEE 2009, 10, 2234–3689. [Google Scholar] [CrossRef]
- Dincer, I. Renewable energy and sustainable development: A crucial review. Renew. Sust. Energ. Rev. 2000, 4, 157–175. [Google Scholar] [CrossRef]
- Leung, D.Y.; Yang, Y. Wind energy development and its environmental impact: A review. Renew. Sust. Energ. Rev. 2012, 16, 1031–1039. [Google Scholar] [CrossRef]
- Sesto, E.; Casale, C. Exploitation of wind as an energy source to meet the world’s electricity demand. J. Wind. Eng. Ind. Aerodyn. 1998, 74, 375–387. [Google Scholar] [CrossRef]
- Veers, P.; Dykes, K.; Lantz, E.; Barth, S.; Bottasso, C.L.; Carlson, O.; Wiser, R. Grand challenges in the science of wind energy. Science 2019, 366, eaau2027. [Google Scholar] [CrossRef]
- Fortin, G.; Perron, J. Wind turbine icing and de-icing. In Proceedings of the47th AIAA Aerospace Sciences Meeting including The New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 5–8 January 2009; p. 274. [Google Scholar] [CrossRef]
- Martini, F.; Contreras Montoya, L.T.; Ilinca, A. Review of wind turbine icing modelling approaches. Energies 2021, 14, 5207. [Google Scholar] [CrossRef]
- Etemaddar, M.; Hansen MO, L.; Moan, T. Wind turbine aerodynamic response under atmospheric icing conditions. Wind. Energy 2014, 17, 241–265. [Google Scholar] [CrossRef]
- Bose, N. Icing on a small horizontal-axis wind turbine—Part, 1: Glaze ice profiles. J. Wind. Eng. Ind. Aerodyn. 1992, 45, 75–85. [Google Scholar] [CrossRef]
- Parent, O.; Ilinca, A. Anti-icing and de-icing techniques for wind turbines: Critical review. Cold Reg. Sci. Technol. 2011, 65, 88–96. [Google Scholar] [CrossRef]
- Hochart, C.; Fortin, G.; Perron, J.; Ilinca, A. Wind turbine performance under icing conditions. Wind. Energy 2008, 11, 319–333. [Google Scholar] [CrossRef]
- Wei, K.; Yang, Y.; Zuo, H.; Zhong, D. A review on ice detection technology and ice elimination technology for wind turbine. Wind. Energy 2020, 23, 433–457. Available online: https://publons.com/publon/10.1002/we.2427 (accessed on 12 October 2022). [CrossRef]
- Gao, L.; Tao, T.; Liu, Y.; Hu, H. A field study of ice accretion and its effects on the power production of utility-scale wind turbines. Renew. Energy 2021, 167, 917–928. [Google Scholar] [CrossRef]
- Lewis, J.P. De-Icing Effectiveness of External Electric Heaters for Propeller Blades; (No. NACA-TN-1520); NASA: Washington, DC, USA, 1948. [Google Scholar]
- VonGlahn, U.; Blatz, R.E. Investigation of Power Requirements for Ice Prevention and Cyclical De-Icing of Inlet Guide Vanes with Internal Electric Heaters; (No. NACA-RM-E50H29); NASA: Washington, DC, USA, 1950. [Google Scholar]
- Yugulis, K.; Chase, D.; McCrink, M. Ice Accretion Analysis for the Development of the HeatCoat Electrothermal Ice Protection System. In Proceedings of the Aiaa Aviation 2020 Forum, Virtual, 15–19 June 2020; p. 2834. [Google Scholar] [CrossRef]
- Mayer, C.; Ilinca, A.; Fortin, G.; Perron, J. Wind tunnel study of electro-thermal de-icing of wind turbine blades. Int. J. Offshore Polar Eng. 2007, 17. [Google Scholar]
- Dalili, N.; Edrisy, A.; Carriveau, R. A review of surface engineering issues critical to wind turbine performance. Renew. Sust. Energy Rev. 2009, 13, 428–438. [Google Scholar] [CrossRef]
- Schulz, M.; Sinapius, M. Evaluation of Different Ice Adhesion Tests for Mechanical Deicing Systems; (No. 2015-01-2135); SAE International: Warrendale, PA, USA, 2015. [Google Scholar] [CrossRef]
- Laforte, J.L.; Allaire, M.A.; Laflamme, J. State-of-the-art on power line de-icing. Atmos. Res. 1998, 46, 143–158. [Google Scholar] [CrossRef]
- Frohboese, P.; Anders, A. Effects of icing on wind turbine fatigue loads. J. Phys. Conf. Ser. 2007, 75, 012061. [Google Scholar] [CrossRef]
- Laakso, T.; Holttinen, H.; Ronsten, G.; Tallhaug, L.; Horbaty, R.; Baring-Gould, I.; Tammelin, B. State-of-the-art of wind energy in cold climates. IEA Annex XIX 2003, 24, 53. [Google Scholar]
- Jiaqiang, E.; Jin, Y.; Deng, Y.; Zuo, W.; Zhao, X.; Han, D.; Zhang, Z. Wetting models and working mechanisms of typical surfaces existing in nature and their application on superhydrophobic surfaces: A review. Adv. Mater. Interfaces 2018, 5, 1701052. [Google Scholar] [CrossRef]
- Peng, C.; Xing, S.; Yuan, Z.; Xiao, J.; Wang, C.; Zeng, J. Preparation and anti-icing of superhydrophobic PVDF coating on a wind turbine blade. Appl. Surf. Sci. 2012, 259, 764–768. [Google Scholar] [CrossRef]
- Wang, Z. Recent progress on ultrasonic de-icing technique used for wind power generation, high-voltage transmission line and aircraft. Energy Build. 2017, 140, 42–49. [Google Scholar] [CrossRef]
- Wu, X.; Fu, Q.; Kumar, D.; Ho JW, C.; Kanhere, P.; Zhou, H.; Chen, Z. Mechanically robust superhydrophobic and superoleophobic coatings derived by sol–gel method. Mater. Design. 2016, 89, 1302–1309. [Google Scholar] [CrossRef]
- Faiçal, B.S.; Freitas, H.; Gomes, P.H.; Mano, L.Y.; Pessin, G.; de Carvalho, A.C.; Ueyama, J. An adaptive approach for UAV-based pesticide spraying in dynamic environments. Comput. Electron. Agr. 2017, 138, 210–223. [Google Scholar] [CrossRef]
- Cervantes, A.; Garcia, P.; Herrera, C.; Morales, E.; Tarriba, F.; Tena, E.; Ponce, H. A conceptual design of a firefighter drone. In Proceedings of the 2018 15th International Conference on Electrical Engineering, Computing Science and Automatic Control (CCE), Mexico City, Mexico, 5–7 September 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Villeneuve, E.; Karmouch, E.; Boulerice, X. Development of a small and transportable de-icing/anti-icing drone-mounted system. Part 1: System design. Drone Syst. Appl. 2022, 10, 155–177. [Google Scholar] [CrossRef]
- Aerones. Aerones DRONE Solutions. 2022. Available online: https://www.aerones.com/ (accessed on 12 October 2022).
- Gidinceanu, C.P. De-Icing and Maintenance of Wind Turbines with Drones. Master’s Thesis, Aalborg University, Aalborg, Denmark, 2019. [Google Scholar]
- Ernez, S.; Morency, F. Eulerian–Lagrangian CFD model for prediction of heat transfer between aircraft deicing liquid sprays and a surface. Int. J. Numer. Method H 2019, 29, 2450–2475. [Google Scholar] [CrossRef]
- Ernez, S.; Morency, F. A multi-region CFD model for aircraft ground deicing by dispersed liquid spray. Energies 2022, 15, 6220. [Google Scholar] [CrossRef]
- Amini, Y.; Nasr Esfahany, M. CFD simulation of the structured packings: A review. Sep. Sci. Technol. 2019, 54, 2536–2554. [Google Scholar] [CrossRef]
- Kim, K.; Yoon, J.Y.; Kwon, H.J.; Han, J.H.; Son, J.E.; Nam, S.W.; Lee, I.B. 3-D CFD analysis of relative humidity distribution in greenhouse with a fog cooling system and refrigerative dehumidifiers. Biosyst. Eng. 2008, 100, 245–255. [Google Scholar] [CrossRef]
- Lew, A.J.; Buscaglia, G.C.; Carrica, P.M. A note on the numerical treatment of the k-epsilon turbulence model. Int. J. Comput. Fluid D 2001, 14, 201–209. [Google Scholar] [CrossRef]
- Zahari, N.M.; Zawawi, M.H.; Sidek, L.M.; Mohamad, D.; Itam, Z.; Ramli, M.Z.; Rashid, M. Introduction of discrete phase model (DPM) in fluid flow: A review. In AIP Conference Proceedings; AIP Publishing: Woodbury, NY, USA, 2018; Volume 2030. [Google Scholar] [CrossRef]


| Model | Boundary Conditions | ||
|---|---|---|---|
| Model | Mode | Parameter | Value |
| Viscous | Realize k−ε Mode | u | 6, 9, 12, 15 (m/s) |
| Discrete Phase | Break Up Stochastic Cossion Coalescence | P0 | 0 (Pa) |
| I | 5% | ||
| turbulent viscosity ratio | 10 | ||
| g | −9.81 (m/s2) | ||
| Case | Number of Cells | Wall Film Height (mm) | Coverage Rate | ||
|---|---|---|---|---|---|
| HL | HT | HM | |||
| 1 | 26,577,481 | 0.007 | 0.036 | 0.011 | 67.28% |
| 2 | 37,345,291 | 0.009 | 0.070 | 0.017 | 73.38% |
| 3 | 42,417,247 | 0.009 | 0.072 | 0.016 | 72.89% |
| α | u0 | Q | |||
|---|---|---|---|---|---|
| Working Conditions | Value (°) | Working Conditions | Value (m/s) | Working Conditions | Value (kg/s) |
| Case 1-1 | 0 | Case 2-1 | 6 | Case 3-1 | 0.0005 |
| Case 1-2 | 30 | Case 2-2 | 9 | Case 3-2 | 0.001 |
| Case 1-3 | 60 | Case 2-3 | 12 | Case 3-3 | 0.002 |
| Case 1-4 | 90 | Case 2-4 | 15 | Case 3-4 | 0.003 |
| Case | HM (mm) | Mean Coverage Rate |
|---|---|---|
| Case 1-1 | 0.015 | 76.86% |
| Case 1-2 | 0.017 | 73.38% |
| Case 1-3 | 0.016 | 87.42% |
| Case 1-4 | 0.011 | 72.15% |
| Case | HM (mm) | Average Coverage Rate |
|---|---|---|
| Case 2-1 | 0.008 | 54.81% |
| Case 2-2 | 0.017 | 73.38% |
| Case 2-3 | 0.028 | 88.87% |
| Case 2-4 | 0.033 | 98.04% |
| Case | HM (mm) | Average Coverage Rate |
|---|---|---|
| Case 3-1 | 0.008 | 54.81% |
| Case 3-2 | 0.017 | 73.38% |
| Case 3-3 | 0.028 | 88.87% |
| Case 3-4 | 0.033 | 98.04% |
| No. | A | B | C |
|---|---|---|---|
| α | u0 | Q | |
| (°) | (m/s) | (kg/s) | |
| 1 | 0 | 6 | 0.0005 |
| 2 | 30 | 9 | 0.001 |
| 3 | 60 | 12 | 0.002 |
| 4 | 90 | 15 | 0.003 |
| Test No. | A | B | C | HM | Average Coverage Rate |
|---|---|---|---|---|---|
| 1 | 1 | 1 | 1 | 0.008 | 58.77% |
| 2 | 1 | 2 | 2 | 0.016 | 76.86% |
| 3 | 1 | 3 | 3 | 0.022 | 89.59% |
| 4 | 1 | 4 | 4 | 0.028 | 96.82% |
| 5 | 2 | 1 | 2 | 0.017 | 75.56% |
| 6 | 2 | 2 | 1 | 0.008 | 54.81% |
| 7 | 2 | 3 | 4 | 0.034 | 98.65% |
| 8 | 2 | 4 | 3 | 0.022 | 94.10% |
| 9 | 3 | 1 | 3 | 0.027 | 96.71% |
| 10 | 3 | 2 | 4 | 0.031 | 98.79% |
| 11 | 3 | 3 | 1 | 0.006 | 60.08% |
| 12 | 3 | 4 | 2 | 0.015 | 80.42% |
| 13 | 4 | 1 | 4 | 0.026 | 91.72% |
| 14 | 4 | 2 | 3 | 0.020 | 82.43% |
| 15 | 4 | 3 | 2 | 0.012 | 63.43% |
| 16 | 4 | 4 | 1 | 0.005 | 38.39% |
| Parameters | A | B | C |
|---|---|---|---|
| K1 | 0.074 | 0.078 | 0.027 |
| K2 | 0.081 | 0.075 | 0.060 |
| K3 | 0.079 | 0.074 | 0.091 |
| K4 | 0.063 | 0.070 | 0.119 |
| k1 | 0.010571 | 0.011143 | 0.003857 |
| k2 | 0.011571 | 0.010714 | 0.008571 |
| k3 | 0.011286 | 0.010571 | 0.013 |
| k4 | 0.009 | 0.010 | 0.017 |
| R | 0.002571 | 0.001143 | 0.013143 |
| Order | C > A > B | ||
| Optimal combination | A2B1C4 | ||
| Parameters | A | B | C |
|---|---|---|---|
| K1 | 3.2204 | 3.2276 | 2.1205 |
| K2 | 3.2312 | 3.1289 | 2.9627 |
| K3 | 3.36 | 3.1175 | 3.6283 |
| K4 | 2.7597 | 3.0973 | 3.8598 |
| k1 | 0.8051 | 0.8069 | 0.530125 |
| k2 | 0.8078 | 0.782225 | 0.740675 |
| k3 | 0.84 | 0.779375 | 0.907075 |
| k4 | 0.689925 | 0.774325 | 0.96495 |
| R | 0.150075 | 0.032575 | 0.434825 |
| Order | C > A > B | ||
| Optimal combination | A3B1C4 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lei, G.; Li, G.; Feng, F. Simulation Evaluation of a Novel Ice-Melting Sprinkling Technique for Blade. Coatings 2023, 13, 1752. https://doi.org/10.3390/coatings13101752
Lei G, Li G, Feng F. Simulation Evaluation of a Novel Ice-Melting Sprinkling Technique for Blade. Coatings. 2023; 13(10):1752. https://doi.org/10.3390/coatings13101752
Chicago/Turabian StyleLei, Gang, Guohao Li, and Fang Feng. 2023. "Simulation Evaluation of a Novel Ice-Melting Sprinkling Technique for Blade" Coatings 13, no. 10: 1752. https://doi.org/10.3390/coatings13101752
APA StyleLei, G., Li, G., & Feng, F. (2023). Simulation Evaluation of a Novel Ice-Melting Sprinkling Technique for Blade. Coatings, 13(10), 1752. https://doi.org/10.3390/coatings13101752








