Dynamics of Colloidal Mixture of Cu-Al2O3/Water in an Inclined Porous Channel Due to Mixed Convection: Significance of Entropy Generation
Abstract
:1. Introduction
- Is it a thermal advantage to use a Cu-Al2O3/water hybrid nanofluid instead of using a regular Al2O3/water nanofluid in a porous inclined channel?
- Is the obtained analytical solution the most appropriate solution to calculate the entropy generation rate in the channel?
- Does the addition of the Cu nanoparticle volume fraction in the Al2O3/water nanofluid enhance the heat transfer in all the cases of the inclination angle of the channel?
- Is it significant to change the influence of free convection over forced convection into the channel to improve the thermal performance of the system?
- Is it relevant to change the main parameters, such as the nanoparticle volume fraction, the mixed-convection parameter and the inclination angle of the channel from horizontal, to obtain the maximum thermal advantage at a minimum of the entropy generation rate in the system?
2. Mathematical Model
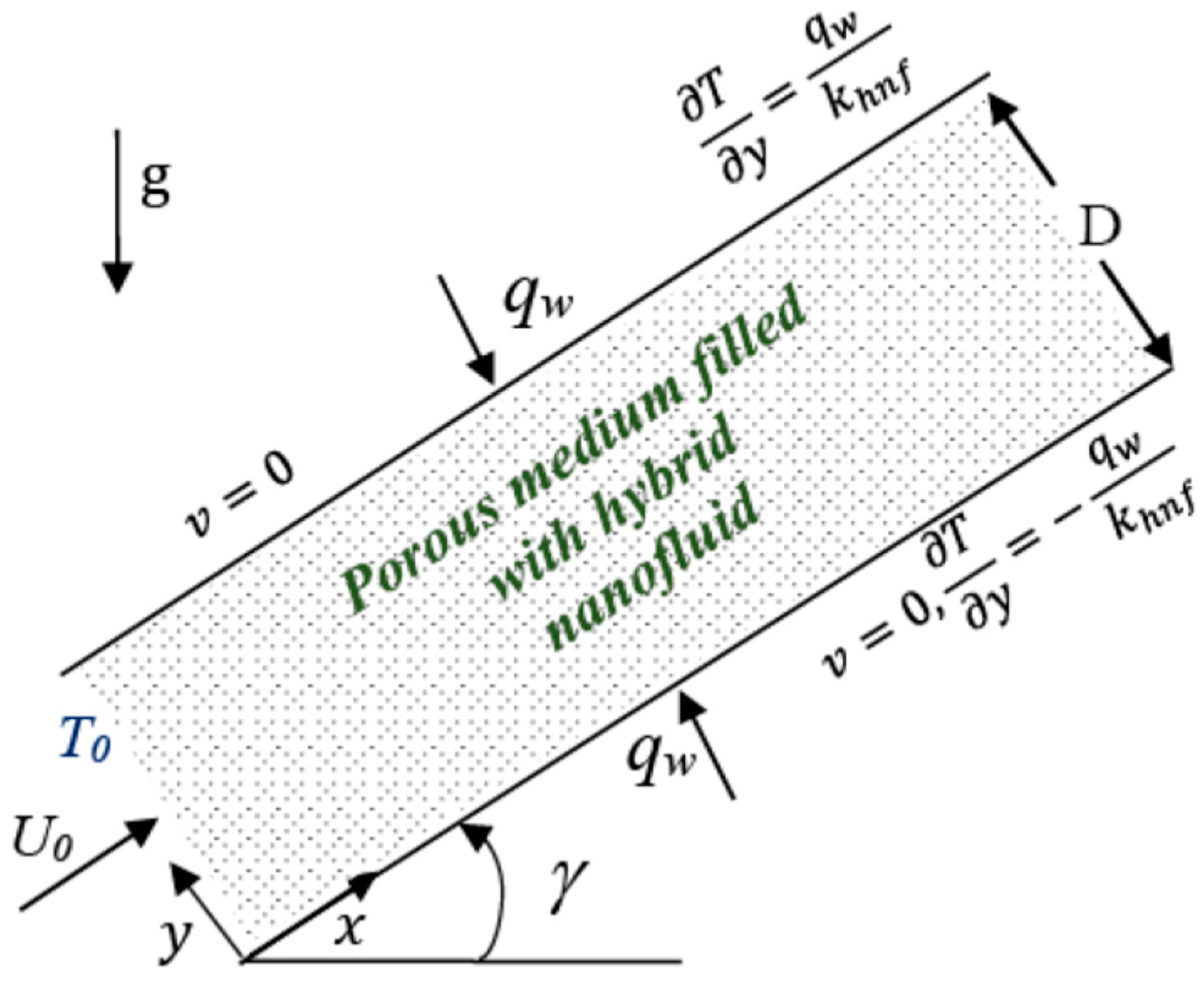
2.1. Basic Equations and Boundary Conditions
2.2. Thermophysical Models of Hybrid Nanofluids
- -
- Hybrid nanofluid density
- -
- Hybrid nanofluid buoyancy coefficient
- -
- Hybrid nanofluid heat capacitance
- -
- Hybrid nanofluid thermal conductivity
- -
- Hybrid nanofluid viscosity
- -
- Hybrid nanofluid density
- -
- Hybrid nanofluid buoyancy coefficient
- -
- Hybrid nanofluid heat capacitance
- -
- Hybrid nanofluid thermal conductivity
- -
- Hybrid nanofluid viscosity
2.3. Nondimensionalization Method
3. Analytical Solution
3.1. The Solution for the General Case of
3.2. The Solution for
3.2.1. The Solution for a Horizontal Channel ()
3.2.2. The Solution for the Forced Convection Limit (
4. Entropy Generation
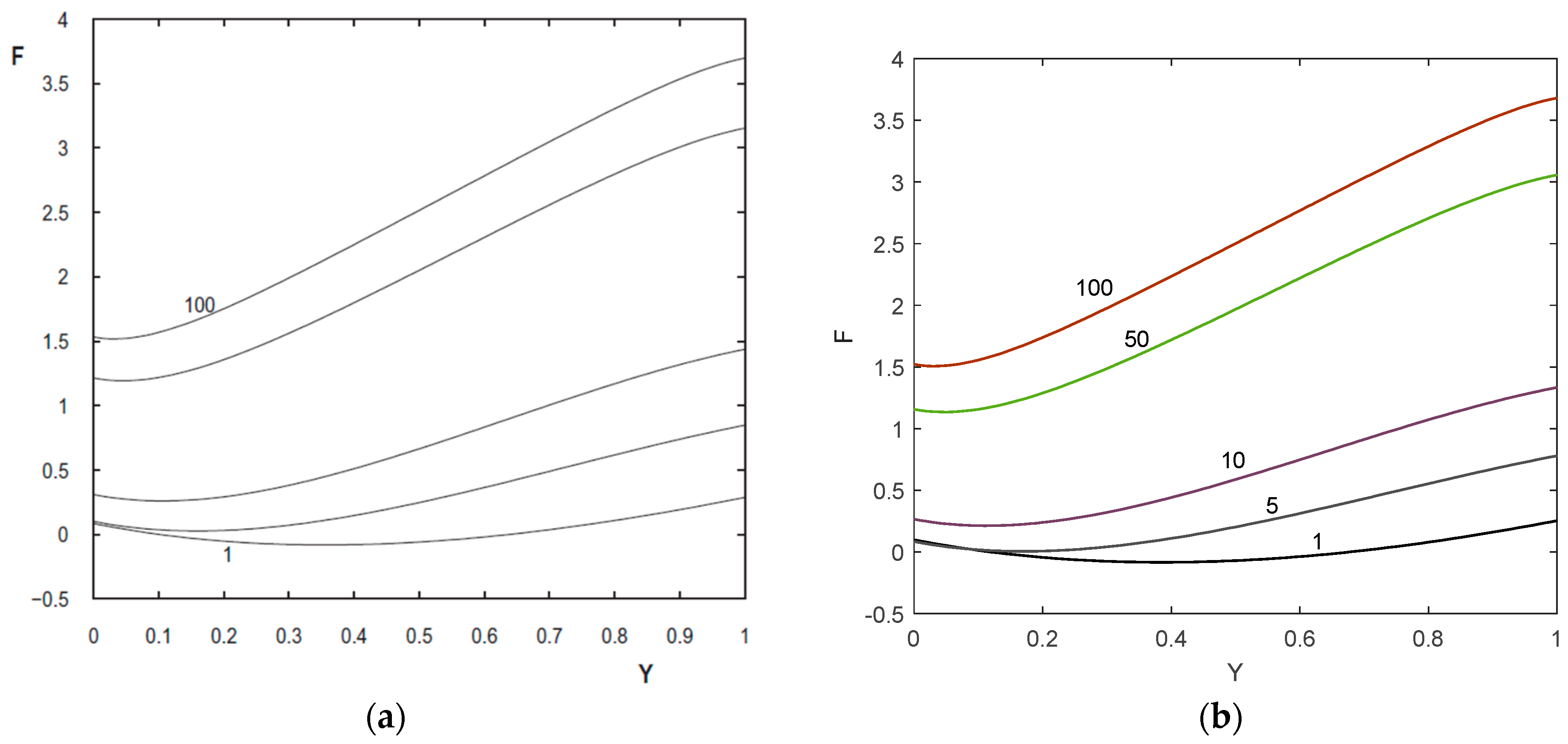
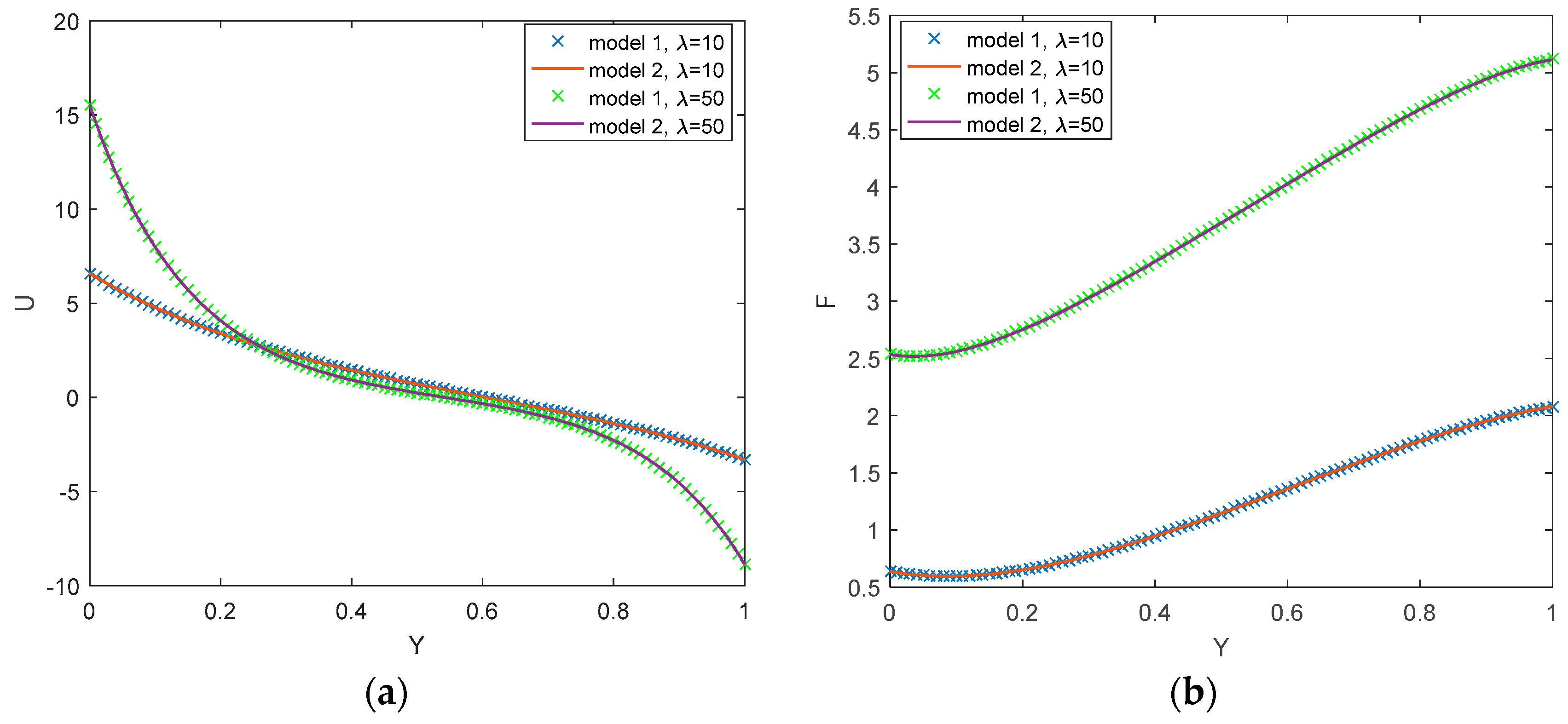
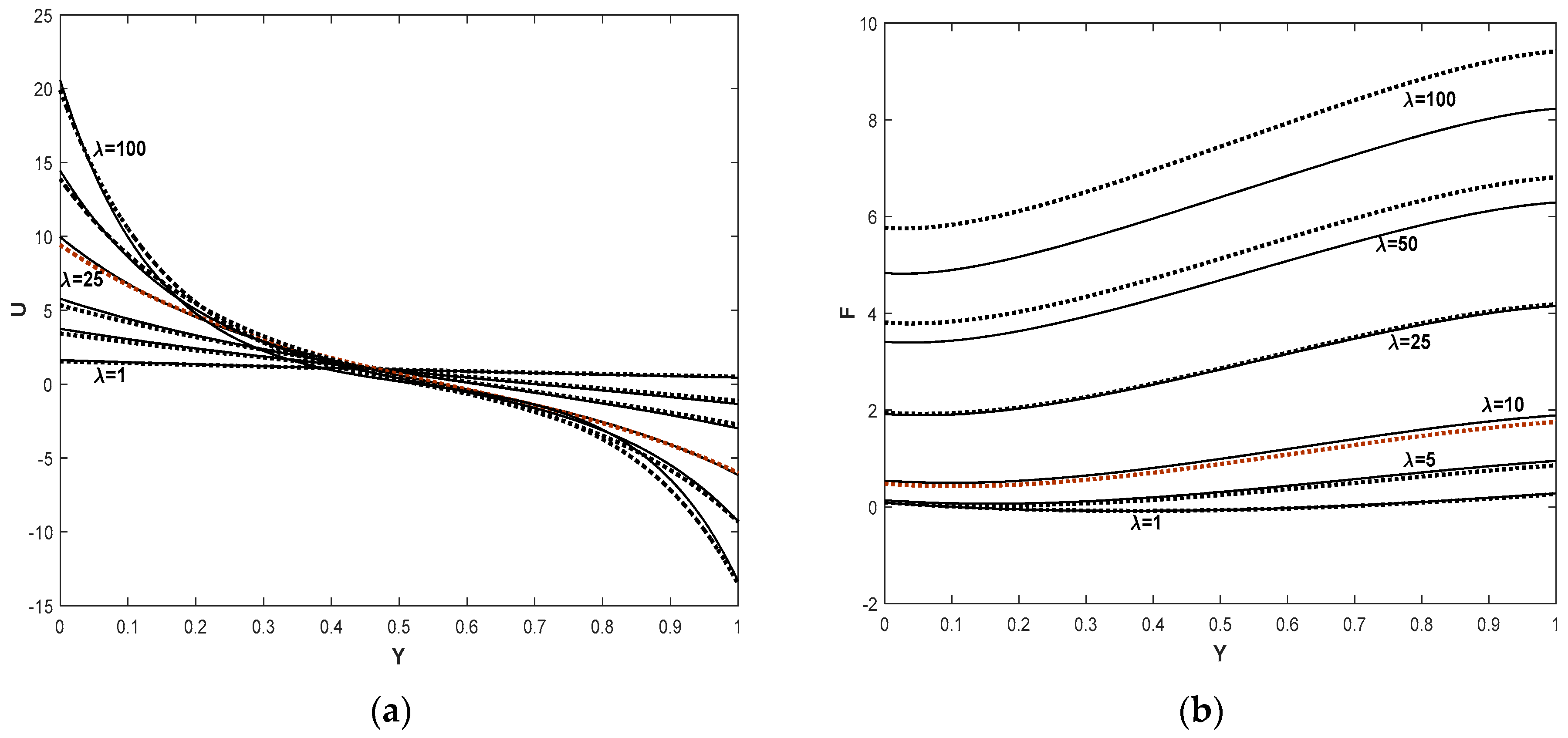
5. Results and Discussion
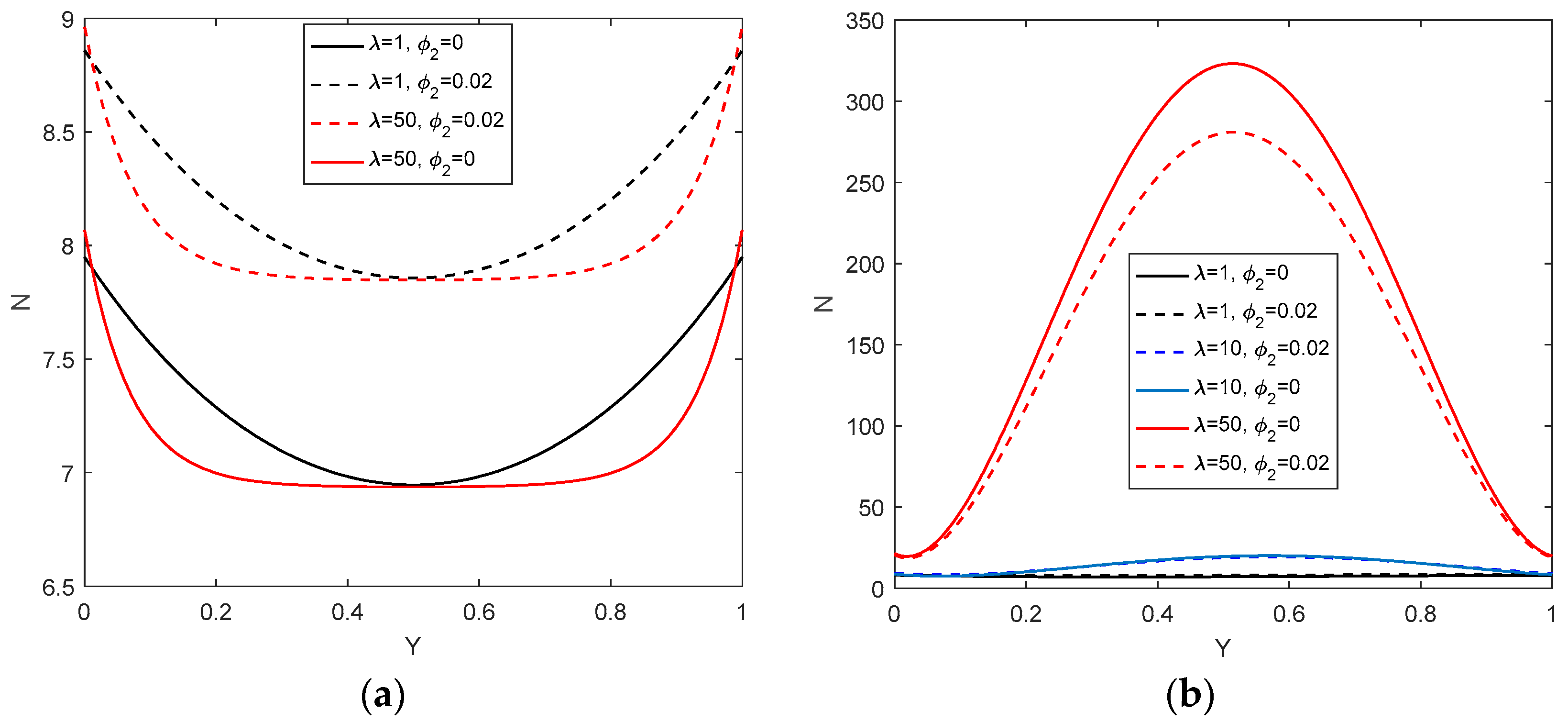
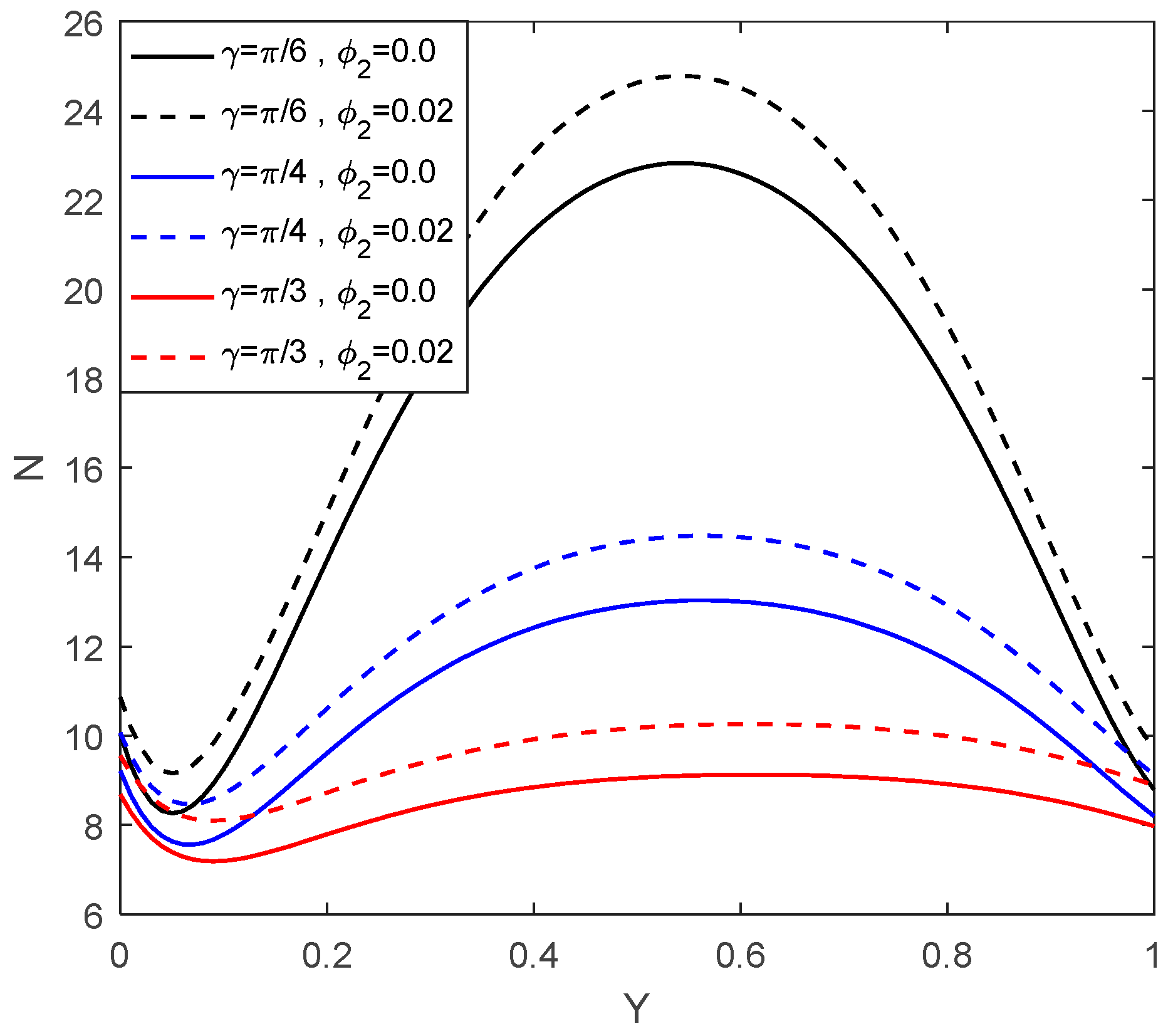
Entropy Generation for the Hybrid Nanofluid Flow
6. Conclusions
- The obtained analytical solution of the problem includes for the first time, all the cases: the inclined, the horizontal and the vertical channel, respectively. This new solution is the most appropriate for an accurate calculating of entropy generation since it is an analytical (not an approximate) solution. Moreover, this exact solution was used to observe the thermal advantage of the hybrid nanofluid for mixed-convective flow in a porous channel.
- The thermal properties of the fluid were enhanced considerably by adding small concentrations of the Cu nanoparticle volume fraction in the regular nanofluid Al2O3/water, but the velocity was not significantly affected by this change. This behavior was only relevant for values of the mixed-convection parameter λ > 25.
- The use of a Cu-Al2O3/water hybrid nanofluid instead of a regular Al2O3/water nanofluid in the porous inclined channel was not always a thermal advantage. For smaller values of the mixed-convection parameter (λ < 25), a simple nanofluid model has increased thermal properties at a minimum entropy generation in the system. This result could be useful to improve the systems dedicated to solar power collectors.
- The inclination angle of the channel from horizontal has an important role on the behavior of the hybrid nanofluid flow inside the channel. Reversed flow was reported for balanced conditions of the heat transfer by fluid motion over the heat transfer by thermal conductivity, Peclet number Pe = 1, for all the values of the inclination angle of the channel. In addition, the temperature increased with a decrease in the inclination angle of the channel. The cumulated results could be used in thermal transmission applications such as heat pipes, etc.
- In the case of the horizontal channel, the hybrid nanofluid flow decreased the thermal performance of the system compared to a regular nanofluid and the entropy generation had minimum values for a higher mixed-convection parameter (λ < 25). This case could be suitable for cooling energetic systems, for example, electronic equipment.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| D | channel width (m) |
| g | acceleration due to gravity (m s−2) |
| K | specific permeability (m2) |
| k | thermal conductivity (W·m−1·K−1) |
| Pe | Péclet number |
| Ra | Rayleigh number |
| qw | heat flux (W·m−2) |
| c | specific heat capacity (kJ·kg−1·K−1) |
| p | pressure (Pa) |
| T | hybrid nanofluid temperature (K) |
| F | dimensionless temperature |
| U | dimensionless velocity |
| coordinate along the channel (m) | |
| coordinate normal to the wall (m) | |
| U0 | velocity at the channel entrance (m·s−1) |
| velocity component along x-axis (m·s−1) | |
| velocity component along y-axis (m·s−1) | |
| uniform fluid temperature at the inflow (K) | |
| Greek symbols | |
| α | thermal diffusivity (m2·s−1) |
| thermal expansion coefficient (K−1) | |
| γ | inclination angle of the channel (°) |
| τ | dimensionless temperature |
| φ | nanoparticles volume fraction |
| λ | mixed-convection parameter |
| ρ | density (kg·m−3) |
| μ | dynamic viscosity (kg·m−1·s−1) |
| Subscripts | |
| f | base fluid |
| hnf | hybrid nanofluid |
| nf | nanofluid |
| p | nanoparticle |
References
- Cheng, P. Natural convection in a porous medium: External flows. In Natural Convection: Fundamentals and Applications; Kakaç, S., Aung, W., Viskanta, W.R., Eds.; Hemisphere: Washington, DC, USA, 1985. [Google Scholar]
- Bejan, A. Convective heat transfer in porous media. In Handbook of Single-Phase Convective Heat Transfer; Kakaç, S., Shah, R.K., Aung, W., Eds.; Wiley: New York, NY, USA, 1987; Chapter 16. [Google Scholar]
- Nield, D.A.; Bejan, A. Convection in Porous Media, 4th ed.; Springer: New York, NY, USA, 2013. [Google Scholar]
- Ingham, D.B.; Pop, I. (Eds.) Transport Phenomena in Porous Media; Pergamon: Oxford, UK, 1998. [Google Scholar]
- Ingham, D.B.; Pop, I. (Eds.) Transport Phenomena in Porous Media; Pergamon: Oxford, UK, 2002; Volume II. [Google Scholar]
- Ingham, D.B.; Pop, I. (Eds.) Transport Phenomena in Porous Media; Elsevier: Oxford, UK, 2005; Volume III. [Google Scholar]
- Pop, I.; Ingham, D.B. Convective Heat Transfer: Mathematical and Computational Modelling of Viscous Fluids and Porous Media; Pergamon: Oxford, UK, 2001. [Google Scholar]
- Vafai, K. Porous Media: Applications in Biological Systems and Biotechnology; CRC Press: Tokyo, Japan, 2010. [Google Scholar]
- Bear, J. Modeling Phenomena of Flow and Transport in Porous Media; Springer: New York, NY, USA, 2018. [Google Scholar]
- Choi, S.U.S. Enhancing thermal conductivity of fluids with nanoparticles. ASME Fluids Eng. Div. 1995, 231, 99105. [Google Scholar]
- Das, S.K.; Yu, W.; Pradeep, T.; Choi, S.U.S. Nanofluids: Science and Technology; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Buongiorno, J. Convective transport in nanofluids. ASME J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Kang, H.U.; Kim, S.H.; Oh, J.M. Estimation of thermal conductivity of nanofluid using experimental effective particle volume. Exp. Heat Transf. 2006, 19, 181–191. [Google Scholar] [CrossRef]
- Abu-Nada, E. Application of nanofluids for heat transfer enhancement of separated flows encountered in a backward facing step. Int. J. Heat Fluid Flow 2008, 29, 242–249. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Abu-Nada, E.; Oztop, H.F. Effects of inclination angle on natural convection in enclosures filled with Cu–Water nanofluid. Int. J. Heat Fluid Flow 2009, 30, 669–678. [Google Scholar] [CrossRef]
- Uddin, M.J.; Rahman, M.M. Finite element computational procedure for convective flow of nanofluids in an annulus. Therm. Sci. Eng. Prog. 2018, 6, 251–267. [Google Scholar] [CrossRef]
- Dogonchi, A.; Sheremet, M.; Pop, I.; Ganji, D. MHD natural convection of Cu/H2O nanofluid in a horizontal semi-cylinder with a local triangular heater. Int. J. Numer. Methods Heat Fluid Flow 2018, 28, 2979–2996. [Google Scholar] [CrossRef]
- Gowda, R.J.P.; Kumar, R.N.; Jyothi, A.M.; Prasannakumara, B.C.; Sarris, I.E. Impact of Binary Chemical Reaction and Activation Energy on Heat and Mass Transfer of Marangoni Driven Boundary Layer Flow of a Non-Newtonian Nanofluid. Processes 2021, 9, 702. [Google Scholar] [CrossRef]
- Groşan, T.; Revnic, C.; Pop, I.; Ingham, D.B. Free convection heat transfer in a square cavity filled with a porous medium saturated by a nanofluid. Int. J. Heat Mass Transf. 2015, 87, 36–41. [Google Scholar] [CrossRef]
- Karvelas, E.; Liosis, C.; Benos, L.; Karakasidis, T.; Sarris, I. Micromixing Efficiency of Particles in Heavy Metal Removal Processes under Various Inlet Conditions. Water 2019, 11, 1135. [Google Scholar] [CrossRef] [Green Version]
- Rosca, A.V.; Rosca, N.C.; Grosan, T.; Pop, I. Non-Darcy mixed convection from a horizontal plate embedded in a nanofluid saturated porous media. Int. Comm. Heat Mass Transf. 2012, 39, 1080–1085. [Google Scholar] [CrossRef]
- Bakar, S.A.; Arifin, N.M.; Ali, F.M.; Bachok, N.; Nazar, R.; Pop, I. A Stability Analysis on Mixed Convection Boundary Layer Flow along a Permeable Vertical Cylinder in a Porous Medium Filled with a Nanofluid and Thermal Radiation. Appl. Sci. 2018, 8, 483. [Google Scholar] [CrossRef]
- Salleh, S.N.A.; Bachok, N.; Arifin, N.M.; Ali, F.M.; Pop, I. Stability Analysis of Mixed Convection Flow towards a Moving Thin Needle in Nanofluid. Appl. Sci. 2018, 8, 842. [Google Scholar] [CrossRef]
- Huminic, G.; Huminic, A. Hybrid nanofluids for heat transfer applications A state-of-the-art review. Int. J. Heat Mass Transf. 2018, 125, 82–103. [Google Scholar] [CrossRef]
- Sarkarn, J.; Ghosh, P.; Adil, A. A review on hybrid nanofluids: Recent research, development and applications. Renew. Sustain. Energy Rev. 2015, 43, 164–177. [Google Scholar] [CrossRef]
- Sidik, N.A.C.; Adamu, I.M.; Jamil, M.M.; Kefayati, G.H.R.; Mamat, R.; Najafi, G. Recent progress on hybrid nanofluids in heat transfer applications: A comprehensive review. Int. Comm. Heat Mass Transf. 2016, 78, 68–79. [Google Scholar] [CrossRef]
- Babu, J.R.; Kumar, K.K.; Rao, S.S. State-of-art review on hybrid nanofluids. Renew. Sust. Energy Rev. 2017, 77, 551–565. [Google Scholar] [CrossRef]
- Kumar, R.N.; Gowda, R.J.P.; Gireesha, B.J.; Prasannakumara, B.C. Non-Newtonian hybrid nanofluid flow over vertically upward/downward moving rotating disk in a Darcy–Forchheimer porous medium. Eur. Phys. J. Spec. Top. 2021, 230, 1227–1237. [Google Scholar] [CrossRef]
- Aly, E.; Pop, I. MHD flow and heat transfer over a permeable stretching/shrinking sheet in a hybrid nanofluid with a convective boundary condition. Int. J. Num. Methods Heat Fluid Flow 2019, 29, 3012–3038. [Google Scholar] [CrossRef]
- Jana, S.; Saheli-Khojin, A.; Zhong, W.H. Enhancement of fluid thermal conductivity by the addition of single and hybrid nano-additives. Thermochim. Acta 2007, 462, 45–55. [Google Scholar] [CrossRef]
- Roşca, A.V.; Roşca, N.C.; Pop, I. Mixed convection stagnation point flow of a hybrid nanofluid past a vertical flat plate with a second order velocity model. Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 75–91. [Google Scholar] [CrossRef]
- Wainia, I.; Ishak, A.; Pop, I. Hiemenz flow over a shrinking sheet in a hybrid nanofluid. Results Phys. 2020, 19, 103351. [Google Scholar] [CrossRef]
- Reza, A.H.; Alireza, S. MHD natural convection of hybrid nanofluid in an open wavy cavity. Results Phys. 2018, 9, 440–455. [Google Scholar] [CrossRef]
- Tlili, I.; Khan, W.A.; Khan, I. Multiple slips effects on MHD SA-Al2O3 and SA-Cu non-Newtonian nanofluids flow over a stretching cylinder in porous medium with radiation and chemical reaction. Results Phys. 2018, 9, 213–222. [Google Scholar] [CrossRef]
- Mehryan, S.A.M.; Farshad, M.; Ghalambaz, M.; Chamkha, A.J. Free convection of hybrid Al2O3-Cu water nanofluid in a differentially heated porous cavity. Adv. Powder Technol. 2017, 28, 2295–2305. [Google Scholar] [CrossRef]
- Izadi, M.; Mohebbi, R.; Delouei, A.A.; Sajjadi, H. Natural convection of a magnetizable hybrid nanofluid inside a porous enclosure subjected to two variable magnetic fields. Int. J. Mech. Sci. 2019, 151, 154–169. [Google Scholar] [CrossRef]
- Mansour, M.A.; Siddiqa, S.; Gorla, R.S.R.; Rashad, A.M. Effects of heat source and sink on entropy generation and MHD natural convection of Al2O3-Cu/water hybrid nanofluid filled with square porous cavity. Therm. Sci. Eng. Prog. 2018, 6, 57–71. [Google Scholar] [CrossRef]
- Zehba, A.S.R. Natural Convection of Dusty Hybrid Nanofluids in an Enclosure Including Two Oriented Heated Fins. Appl. Sci. 2019, 9, 2673. [Google Scholar] [CrossRef]
- Dalkılıç, A.S.; Türk, O.A.; Mercan, H.; Nakkaew, S.; Wongwises, S. An experimental investigation on heat transfer characteristics of graphite-SiO2/water hybrid nanofluid flow in horizontal tube with various quad-channel twisted tape inserts. Int. Comm. Heat Mass Transf. 2009, 107, 1–13. [Google Scholar] [CrossRef]
- Saba, F.; Ahmed, N.; Khan, U.; Mohyud-Din, S.T. A novel coupling of (CNT−Fe3O4/H2O) hybrid nanofluid for improvements in heat transfer for flow in an asymmetric channel with dilating/squeezing walls. Int. J. Heat Mass Transf. 2019, 136, 186–195. [Google Scholar] [CrossRef]
- Moghadassi, A.; Ghomi, E.; Parvizian, F. A numerical study of water based Al2O3 and Al2O3-Cu hybrid nanofluid effect on forced convective heat transfer. Int. J. Therm. Sci. 2015, 92, 50–57. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.; Selvakumar, P.; Chandrasekar, M. Synthesis of Al2O3–Cu/water hybrid nanofluids using two step method and its thermo physical properties. Colloids Surf. A. 2011, 388, 41–48. [Google Scholar] [CrossRef]
- Tayebi, T.; Chamkha, A.J. Free convection enhancement in an annulus between horizontal confocal elliptical cylinders using hybrid nanofluids. Numer. Heat Transf. Part A Appl. 2016, 70, 1141–1156. [Google Scholar] [CrossRef]
- Devi, S.U.S.; Devi, S.P.A. Heat transfer enhancement of Cu-Al2O3/water hybrid nanofluid flow over a stretching sheet. J. Niger. Math. Soc. 2017, 36, 419–433. [Google Scholar]
- Bejan, A. Entropy Generation Minimization; CRC Press: Boca Raton, FL, USA, 1996. [Google Scholar]
- Narusawa, U. The second-law analysis of mixed convection in rectangular ducts. Heat Mass Transf. 2001, 37, 197–203. [Google Scholar] [CrossRef]
- Yusuf, T.A.; Kumar, R.N.; Gowda, R.J.P.; Akpan, U.D. Entropy generation on flow and heat transfer of a reactive MHD Sisko fluid through inclined walls with porous medium. Int. J. Ambient Energy 2022. [Google Scholar] [CrossRef]
- Hamzah, H.K.; Ali, F.H.; Hatami, M. MHD mixed convection and entropy generation of CNT-water nanofluid in a wavy lid-driven porous enclosure at different boundary conditions. Sci. Rep. 2022, 12, 2881. [Google Scholar] [CrossRef]
- Atashafrooz, M. The effects of buoyancy force on mixed convection heat transfer of MHD nanofluid flow and entropy generation in an inclined duct with separation considering Brownian motion effects. J. Therm. Anal. Calorim. 2019, 138, 3109–3126. [Google Scholar] [CrossRef]
- Khan, W.U.; Awais, M.; Parveen, N.; Ali, A.; Awan, S.E.; Malik, M.Y.; He, Y. Analytical Assessment of (Al2O3–Ag/H2O) Hybrid Nanofluid Influenced by Induced Magnetic Field for Second Law Analysis with Mixed Convection, Viscous Dissipation and Heat Generation. Coatings 2021, 11, 498. [Google Scholar] [CrossRef]
- Cimpean, D.S.; Pop, I. Fully developed mixed convection flow of a nanofluid through an inclined channel filled with a porous medium. Int. J. Heat Mass Transf. 2012, 55, 907–914. [Google Scholar] [CrossRef]
- Ghalambaz, M.; Sheremet, M.A.; Mehryan, S.A.M.; Kashkooli, F.M.; Pop, I. Local thermal non-equilibrium analysis of conjugate free convection within a porous enclosure occupied with Ag–MgO hybrid nanofluid. J. Therm. Anal. Calorim. 2019, 135, 1381–1398. [Google Scholar] [CrossRef]
- Bagheri, H.; Behrang, M.; Assareh, E.; Izadi, M.; Sheremet, M.A. Free Convection of Hybrid Nanofluids in a C-ShapedChamber under Variable Heat Flux and Magnetic Field: Simulation, Sensitivity Analysis, and Artificial Neural Networks. Energies 2019, 12, 2807. [Google Scholar] [CrossRef]
- Manjunatha, S.; Kuttan, B.A.; Jayanthi, S.; Chamkha, A.; Gireesha, B.J. Heat transfer enhancement in the boundary layer flow of hybrid nanofluids due to variable viscosity and natural convection. Heliyon 2019, 5, e01469. [Google Scholar] [CrossRef]
- Baytas, A.C. Entropy generation for natural convection in an inclined porous cavity. Int. J. Heat Mass Transf. 2000, 43, 2089–2099. [Google Scholar] [CrossRef]
- Bejan, A. Entropy Generation through Heat and Fluid Flow; John Wiley and Sons: Hoboken, NJ, USA, 1994. [Google Scholar]
- Yazdi, M.H.; Abdullah, S.; Hashim, I.; Zaharim, A.; Sopian, K. Entropy Generation Analysis of the MHD Flow over Nonlinear Permeable Stretching Sheet with Partial Slip. In Proceedings of the 6th IASME/WSEAS International Conference on Energy & Environment, Cambridge, UK, 23–25 February 2011; pp. 292–297. [Google Scholar]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cimpean, D.S. Dynamics of Colloidal Mixture of Cu-Al2O3/Water in an Inclined Porous Channel Due to Mixed Convection: Significance of Entropy Generation. Coatings 2022, 12, 1347. https://doi.org/10.3390/coatings12091347
Cimpean DS. Dynamics of Colloidal Mixture of Cu-Al2O3/Water in an Inclined Porous Channel Due to Mixed Convection: Significance of Entropy Generation. Coatings. 2022; 12(9):1347. https://doi.org/10.3390/coatings12091347
Chicago/Turabian StyleCimpean, Dalia Sabina. 2022. "Dynamics of Colloidal Mixture of Cu-Al2O3/Water in an Inclined Porous Channel Due to Mixed Convection: Significance of Entropy Generation" Coatings 12, no. 9: 1347. https://doi.org/10.3390/coatings12091347
APA StyleCimpean, D. S. (2022). Dynamics of Colloidal Mixture of Cu-Al2O3/Water in an Inclined Porous Channel Due to Mixed Convection: Significance of Entropy Generation. Coatings, 12(9), 1347. https://doi.org/10.3390/coatings12091347







