Entropy Analysis of an MHD Synthetic Cilia Assisted Transport in a Microchannel Enclosure with Velocity and Thermal Slippage Effects
Abstract
:1. Introduction
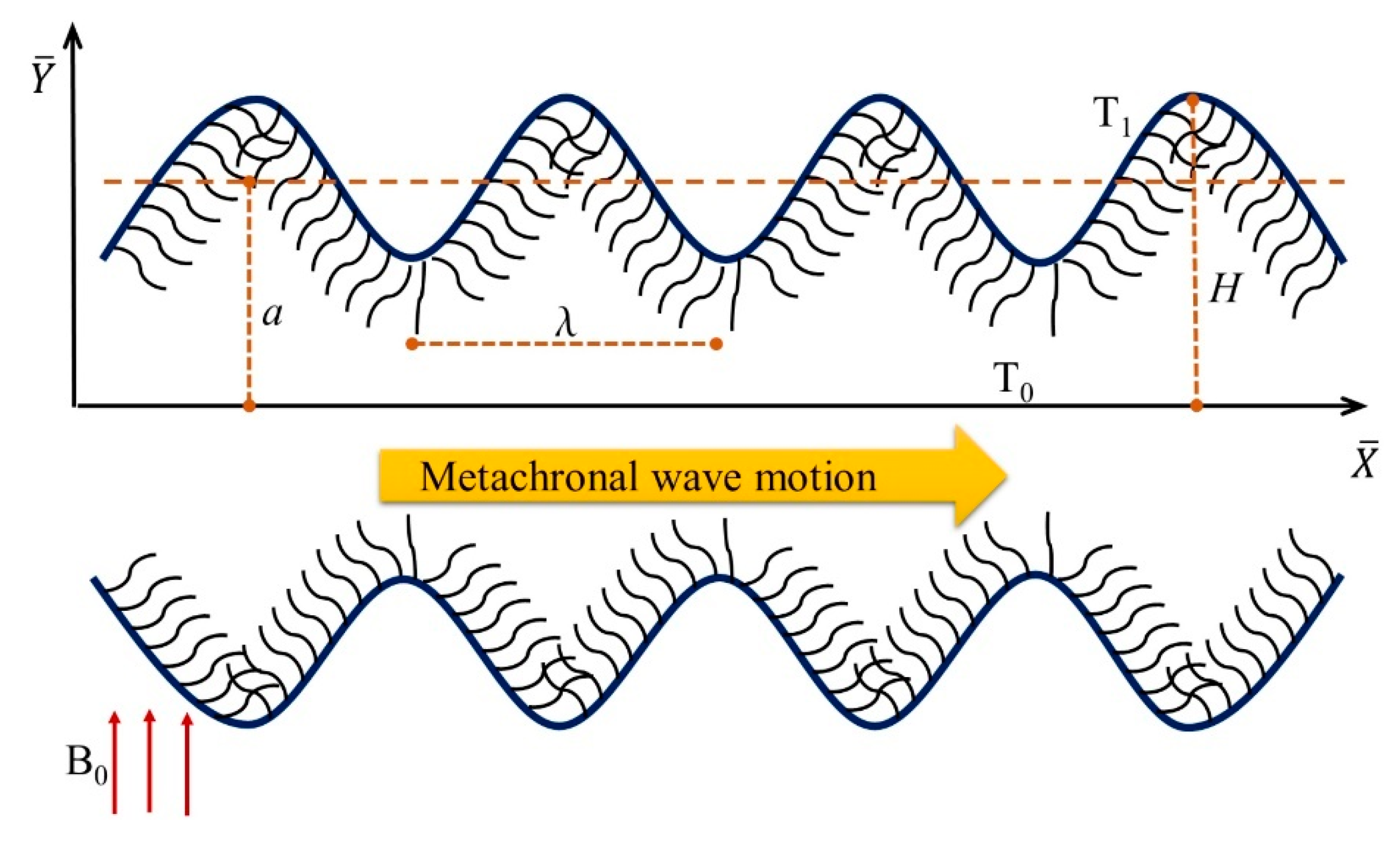
2. Mathematical Formulation
3. Perturbation Solution
3.1. Zeroth Order System
3.2. First Order System
4. Entropy Analysis
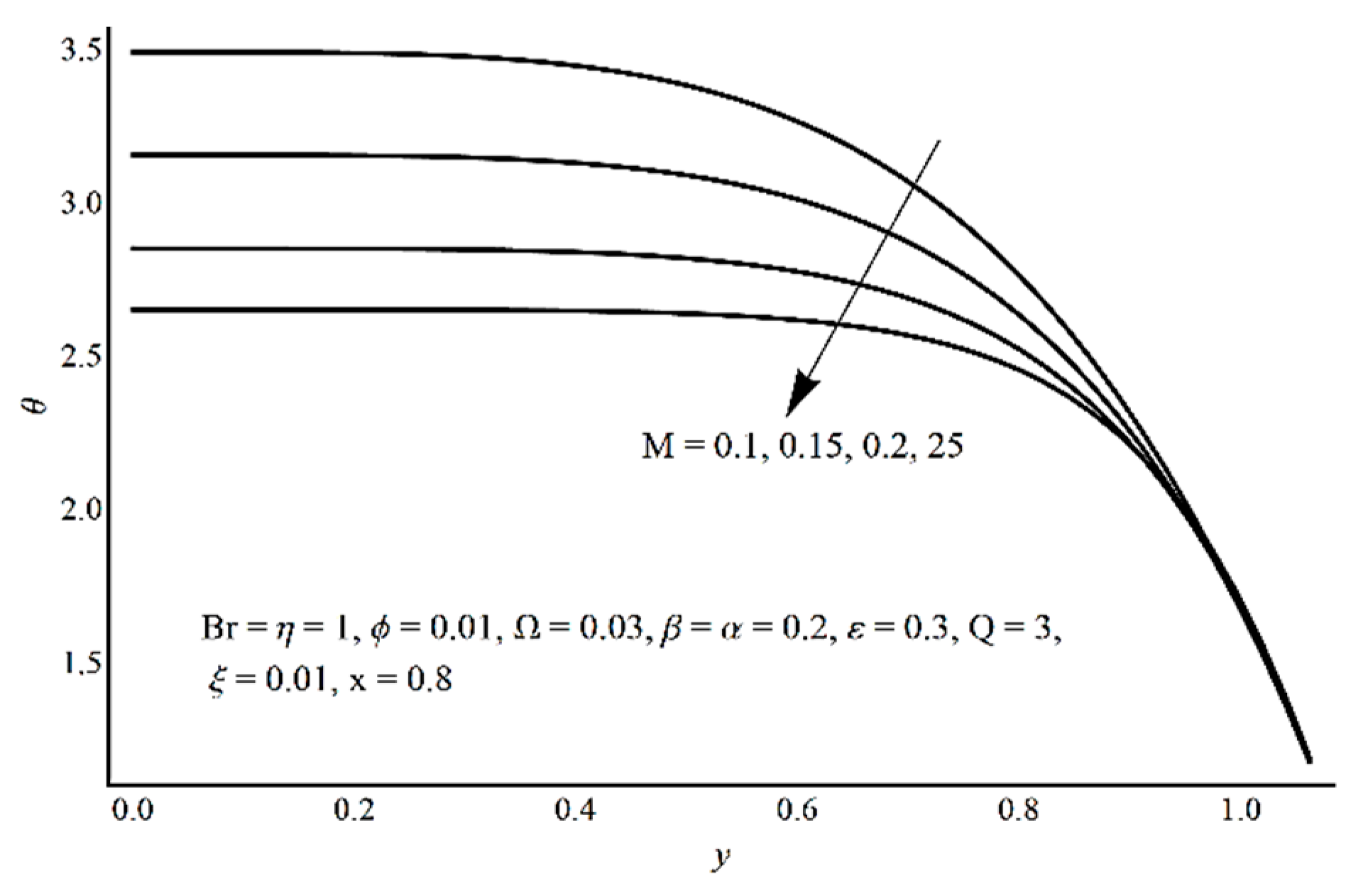
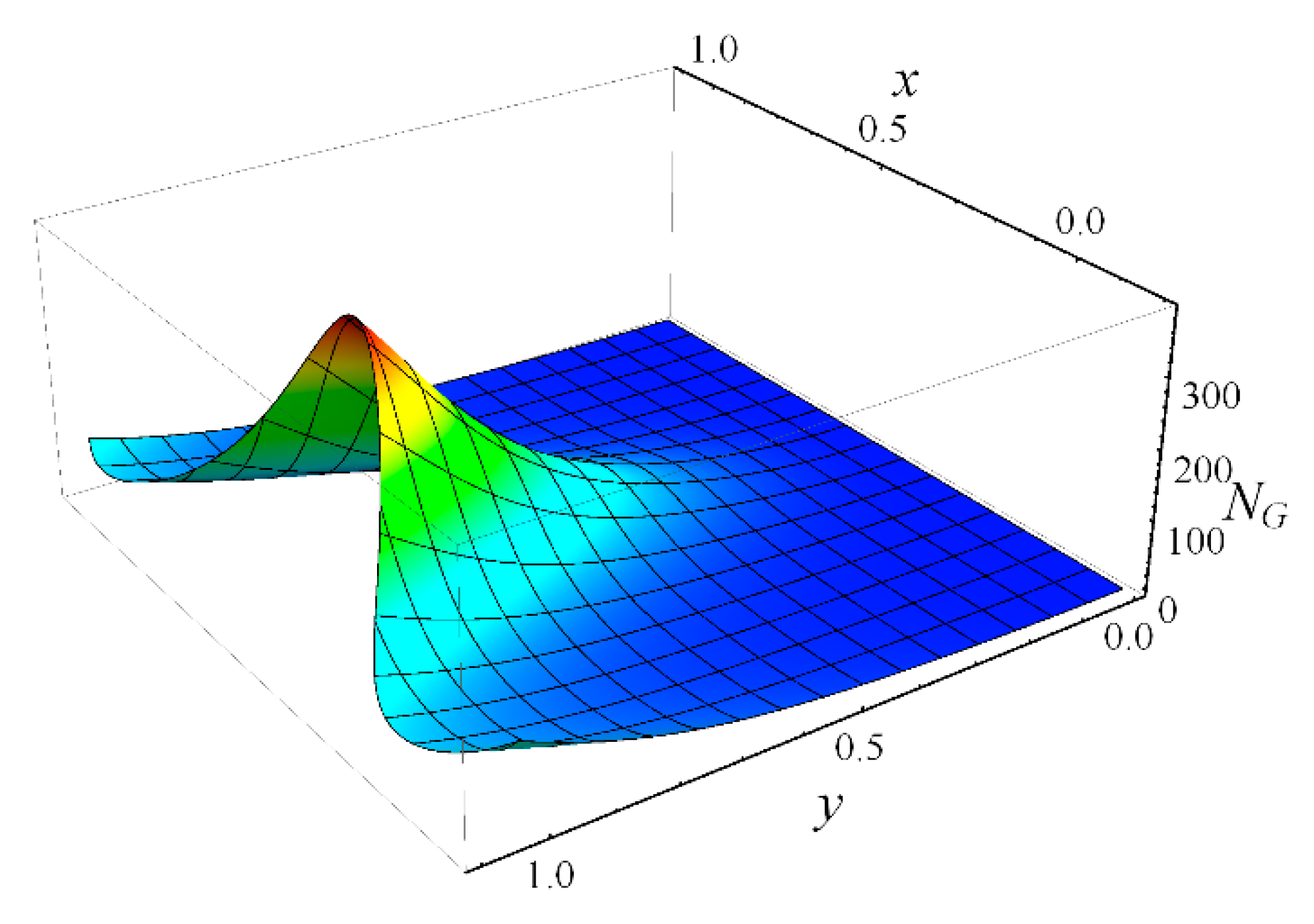
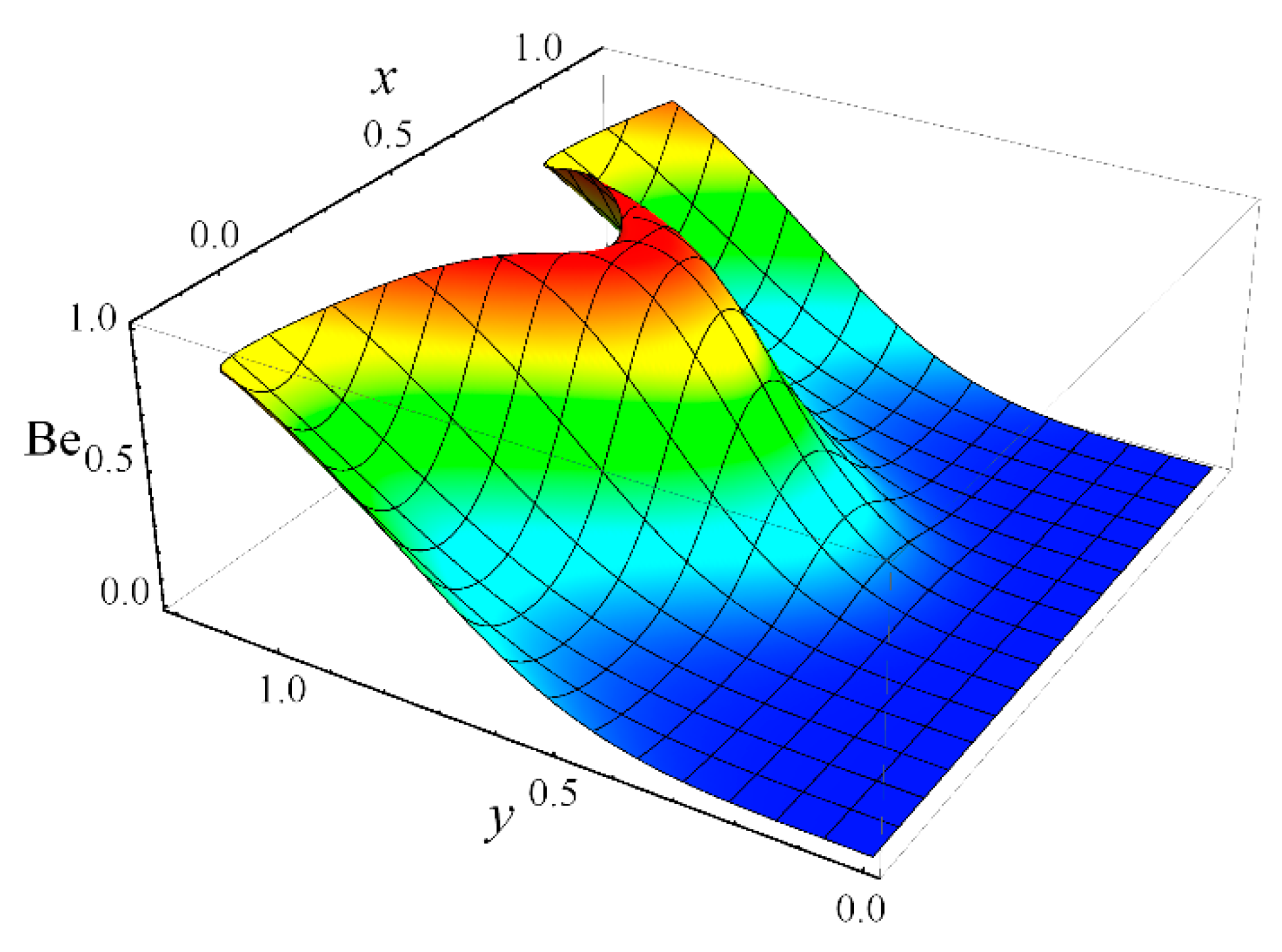
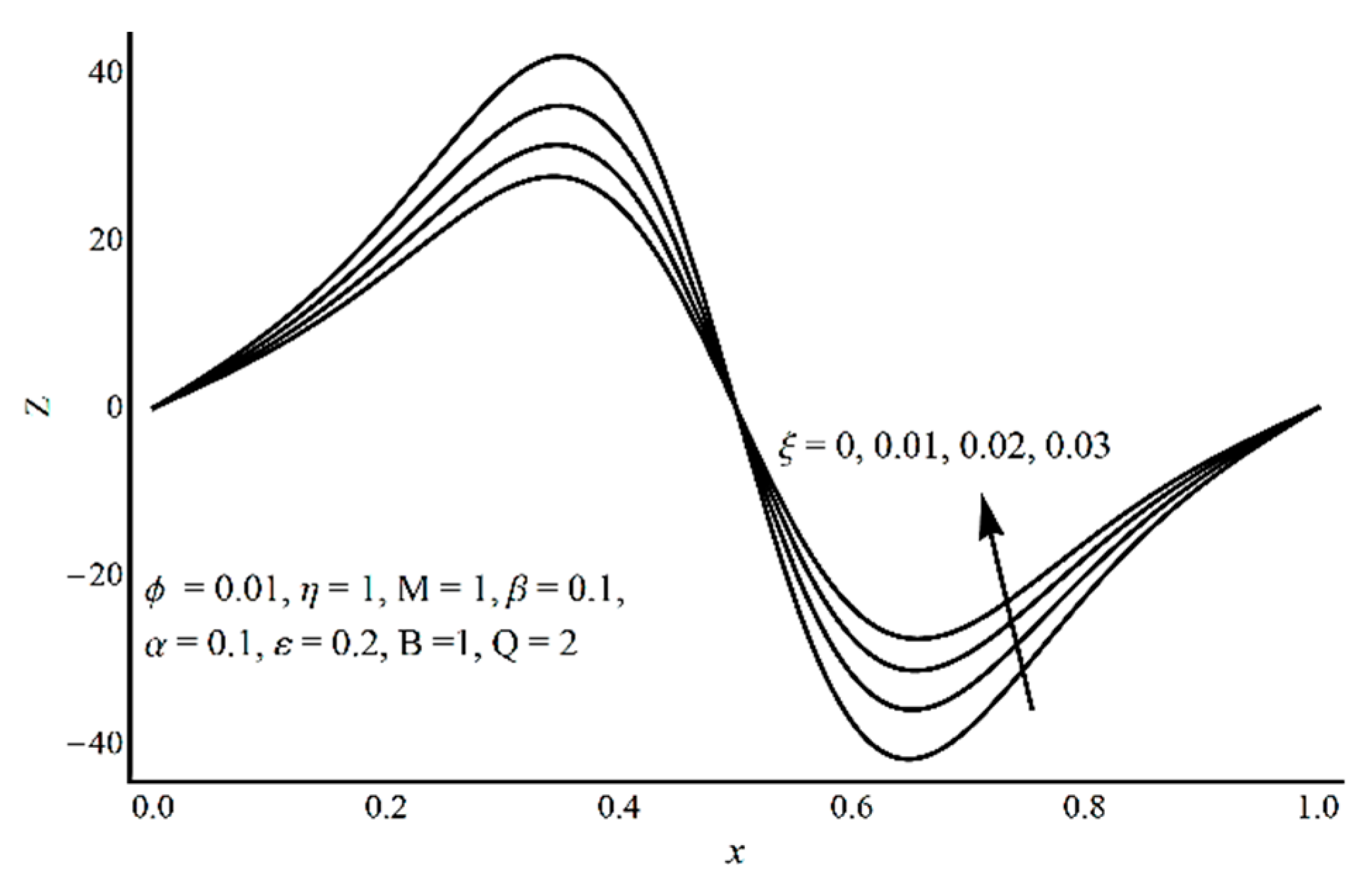
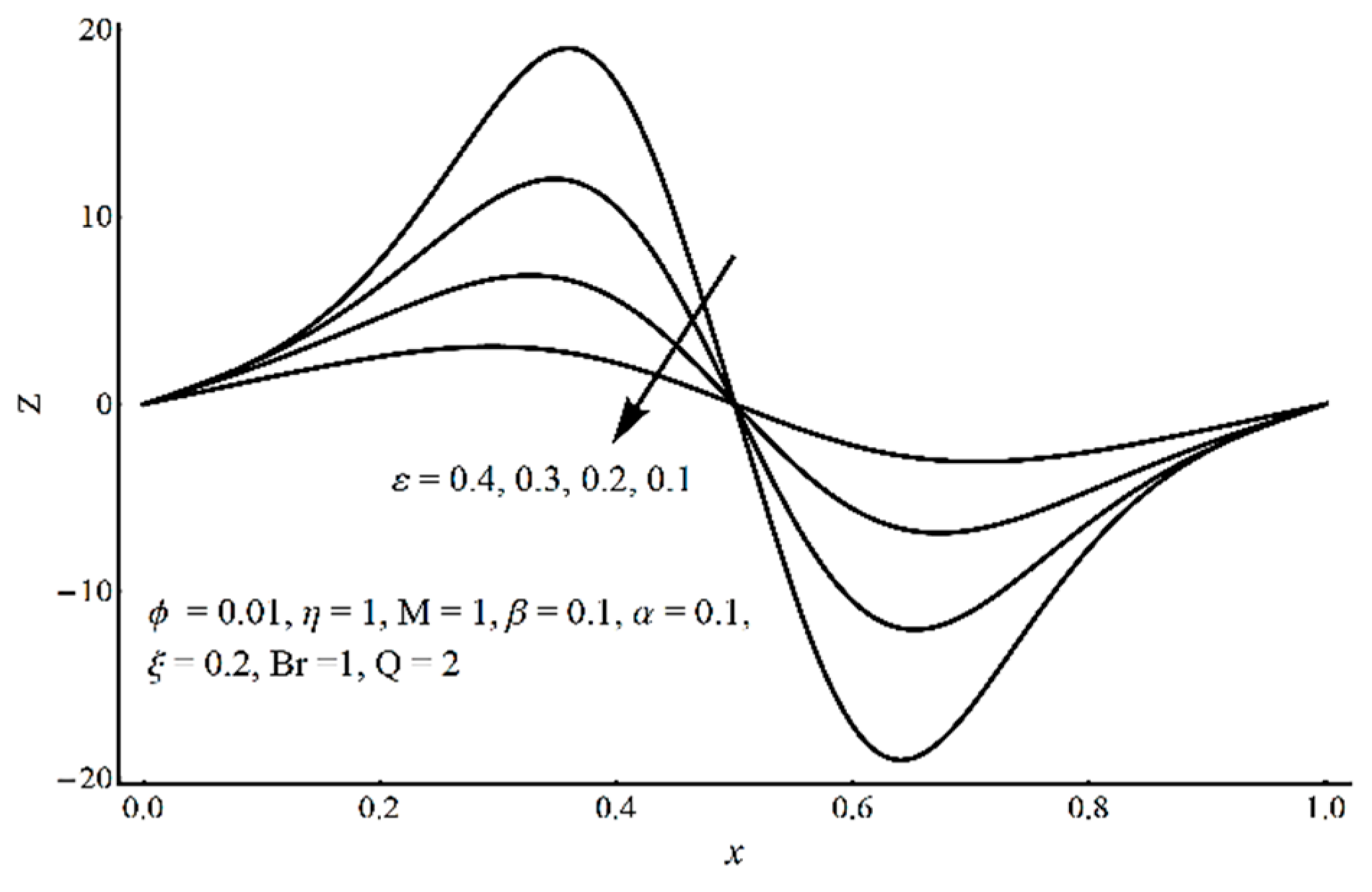
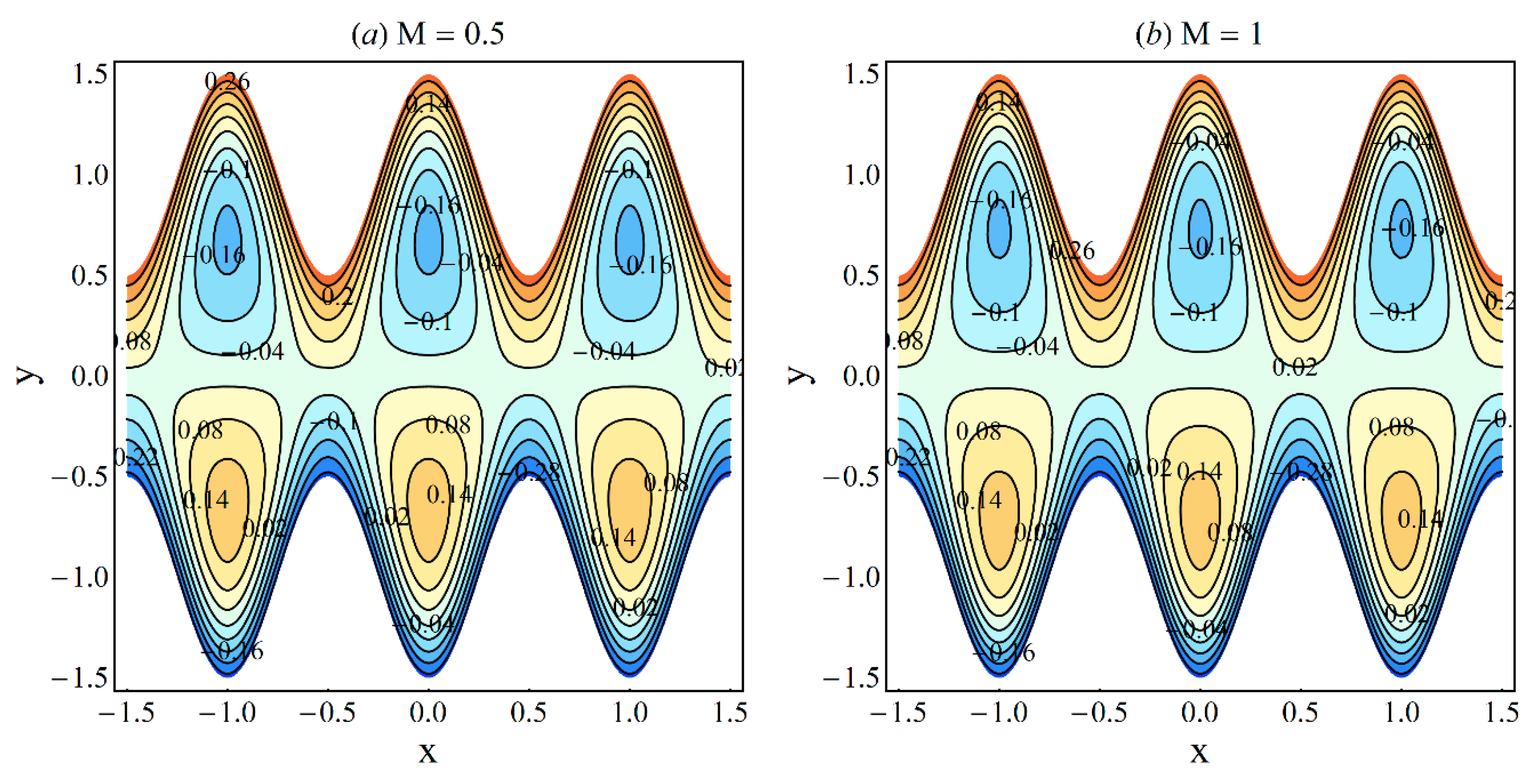
5. Results and Discussion
6. Conclusions
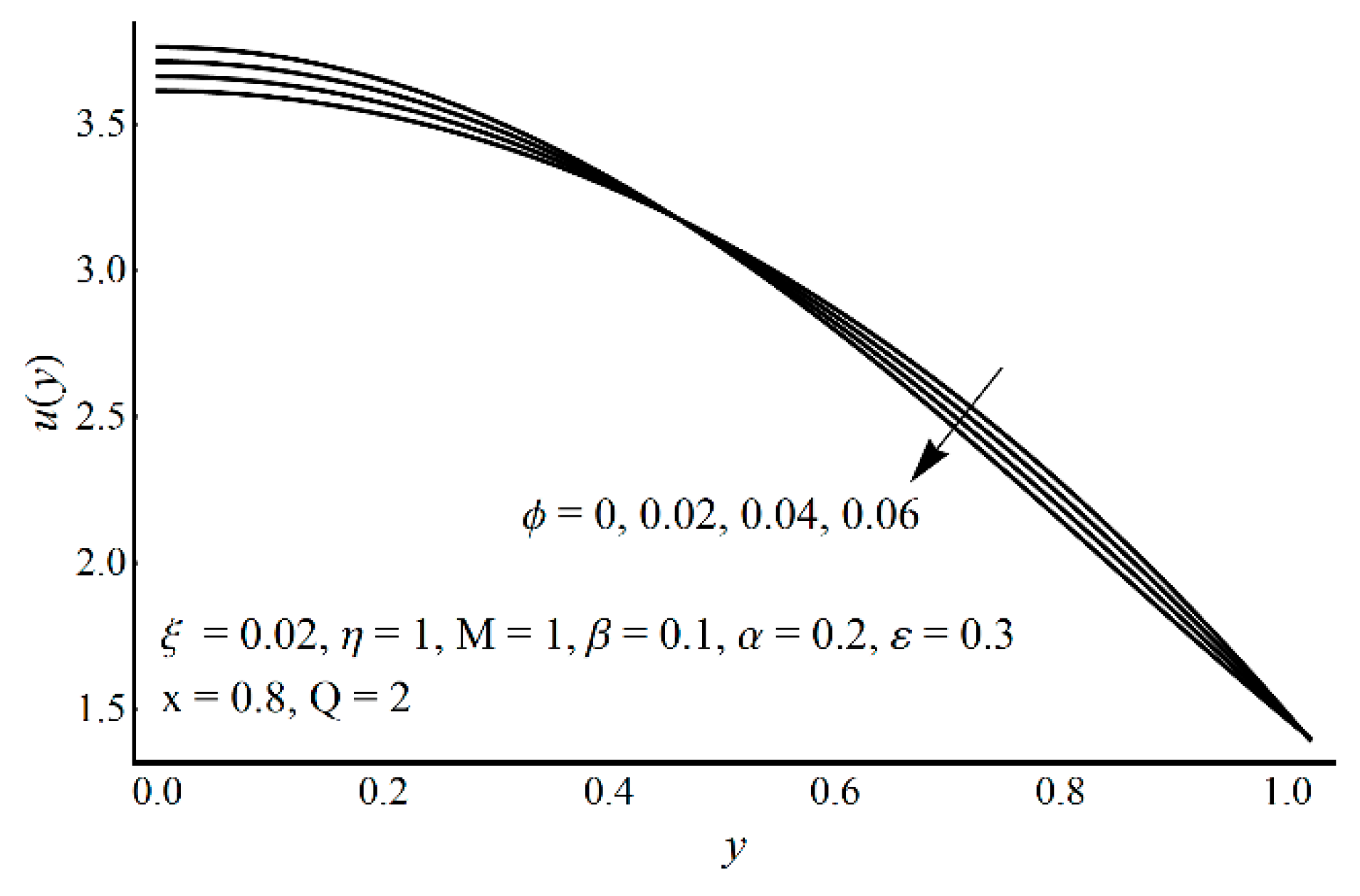
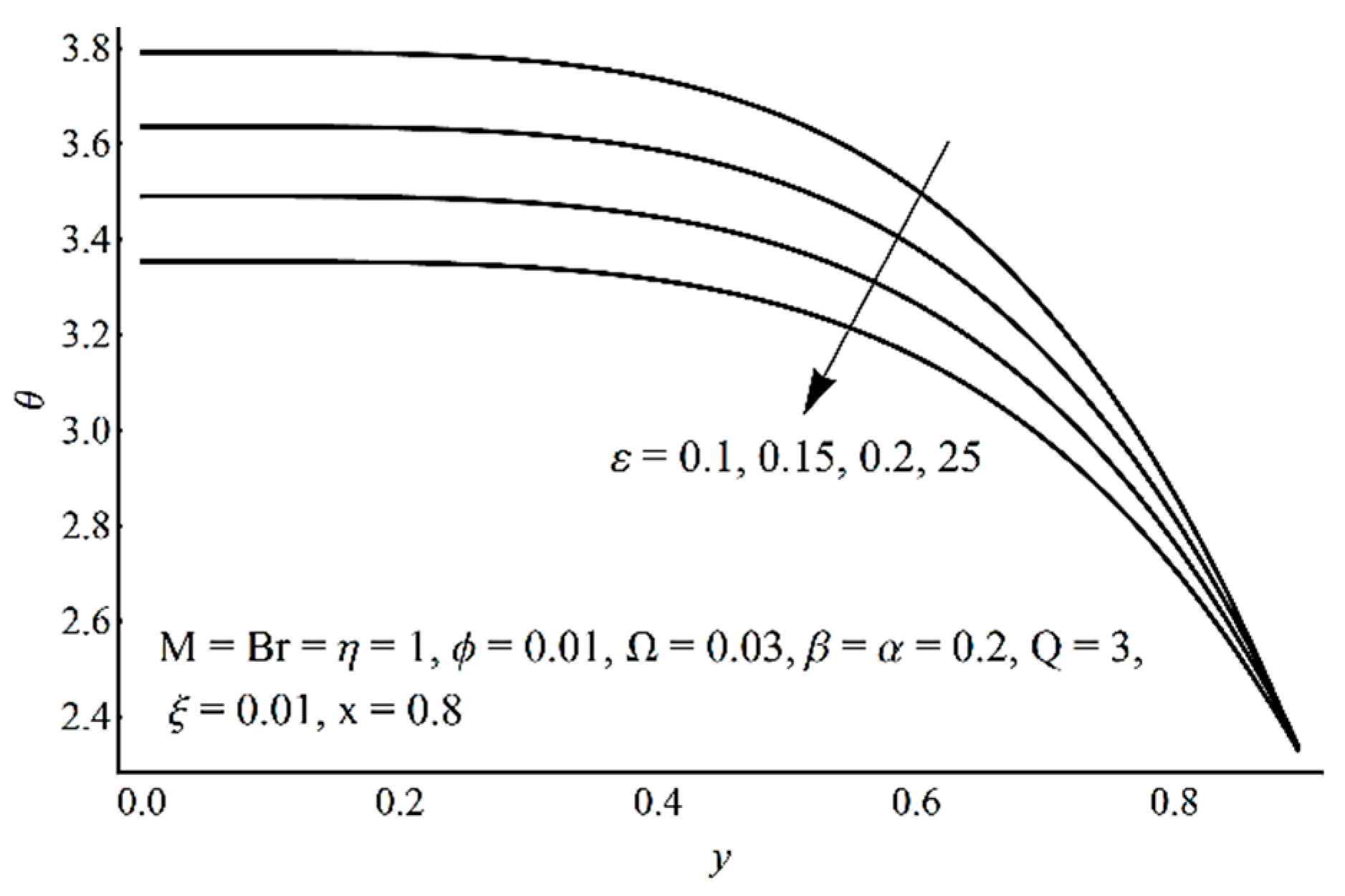
- As the cilia length increases, the temperature profile and the entropy production decrease, while the velocity profile shows the opposite effect near the boundary and demonstrates decreasing effects near the center;
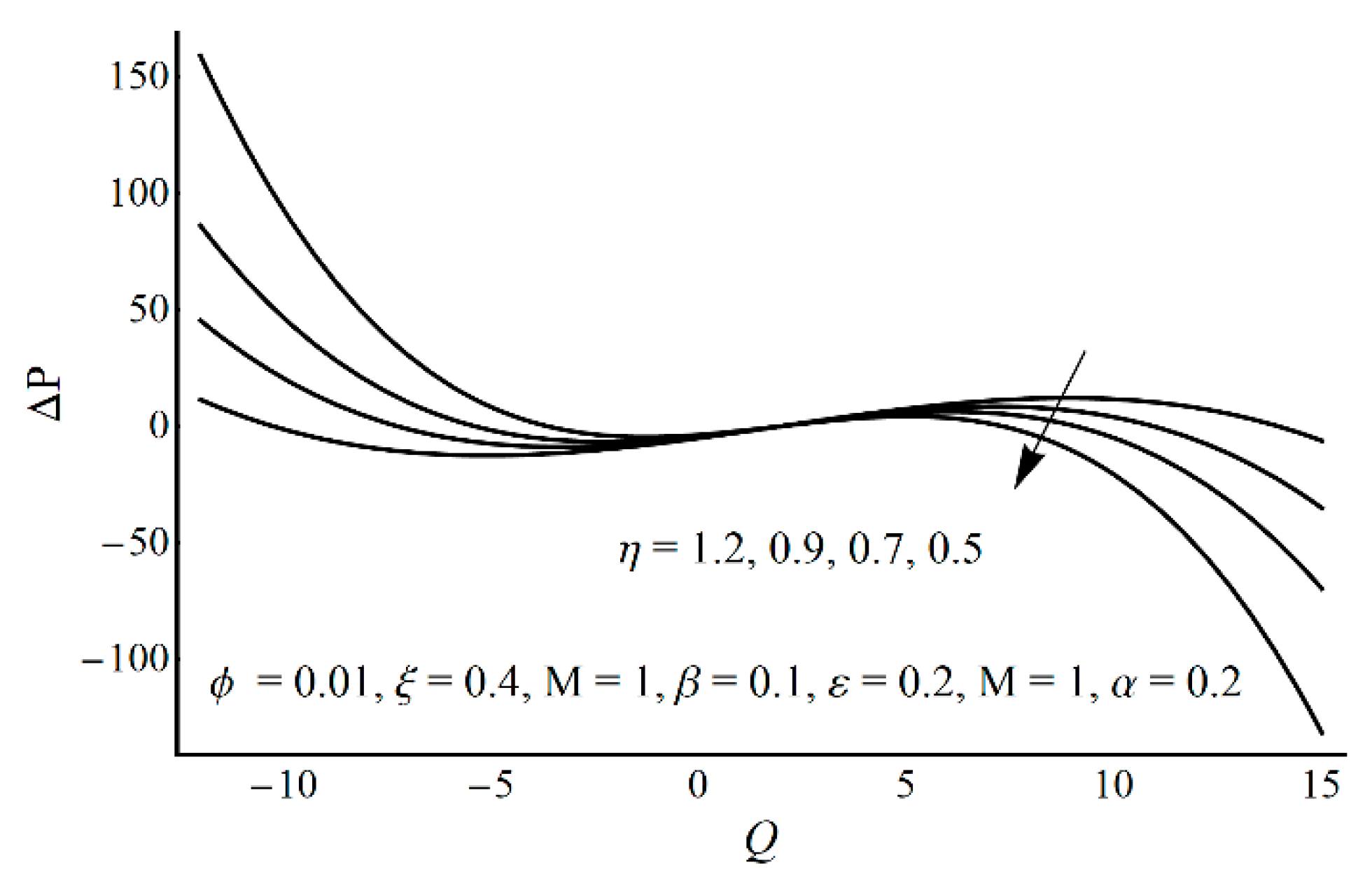
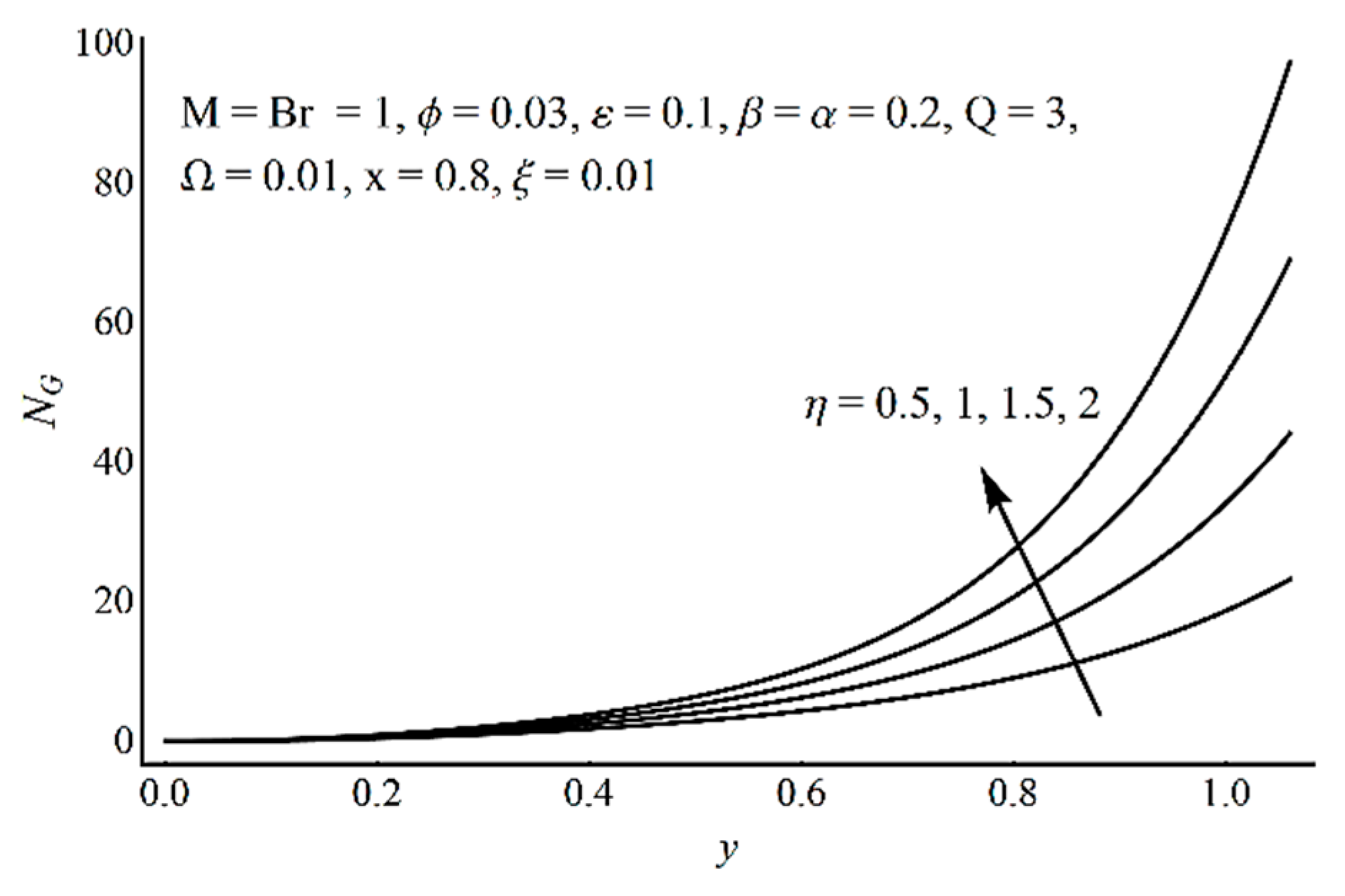
- The Prandtl fluid material parameter η has increasing effects on the velocity and entropy production near the boundary;
- Due to wall slip, the velocity increases, while temperature and the entropy production decrease;
- The temperature jump brought an increasing change in the temperature profile;
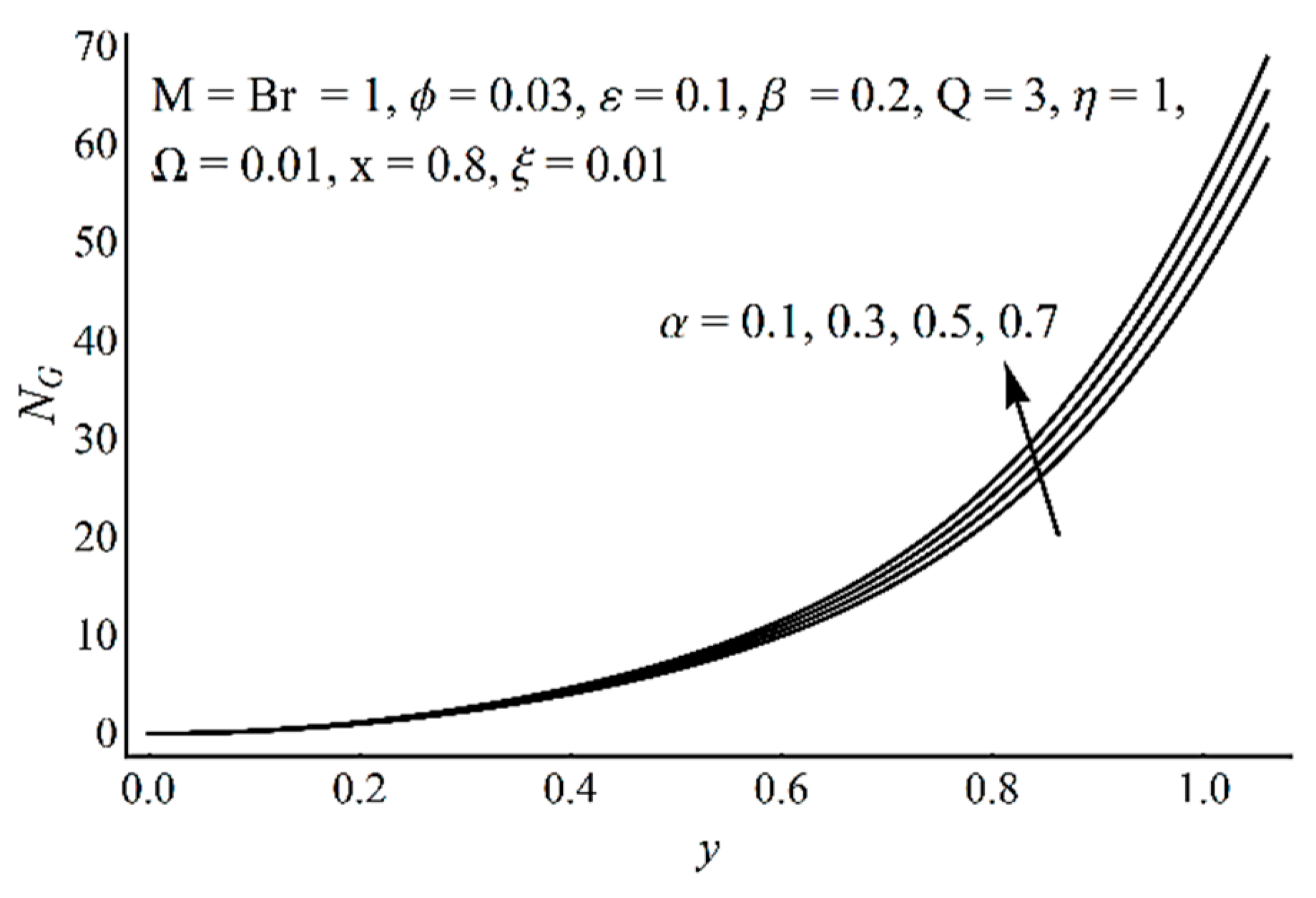
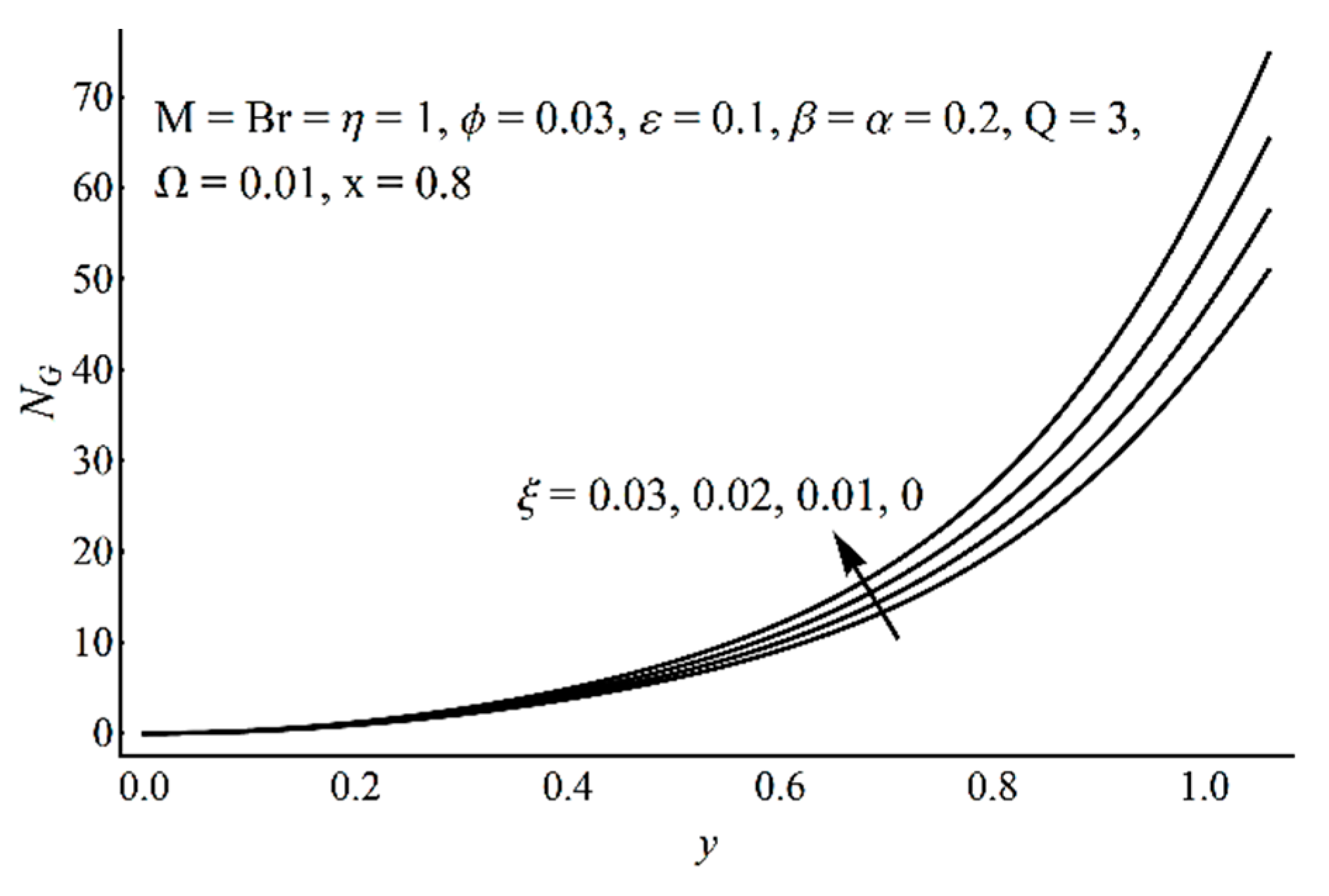
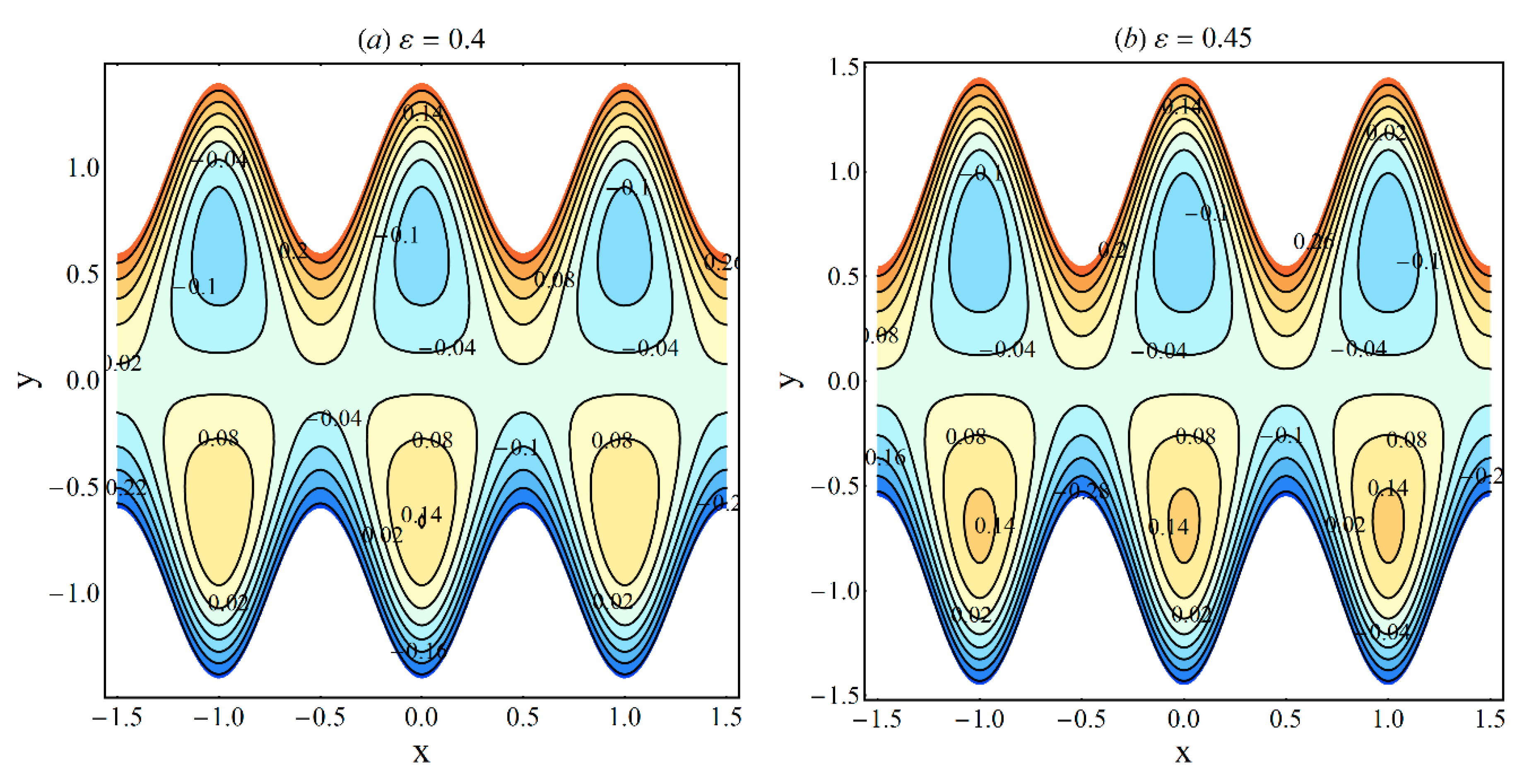
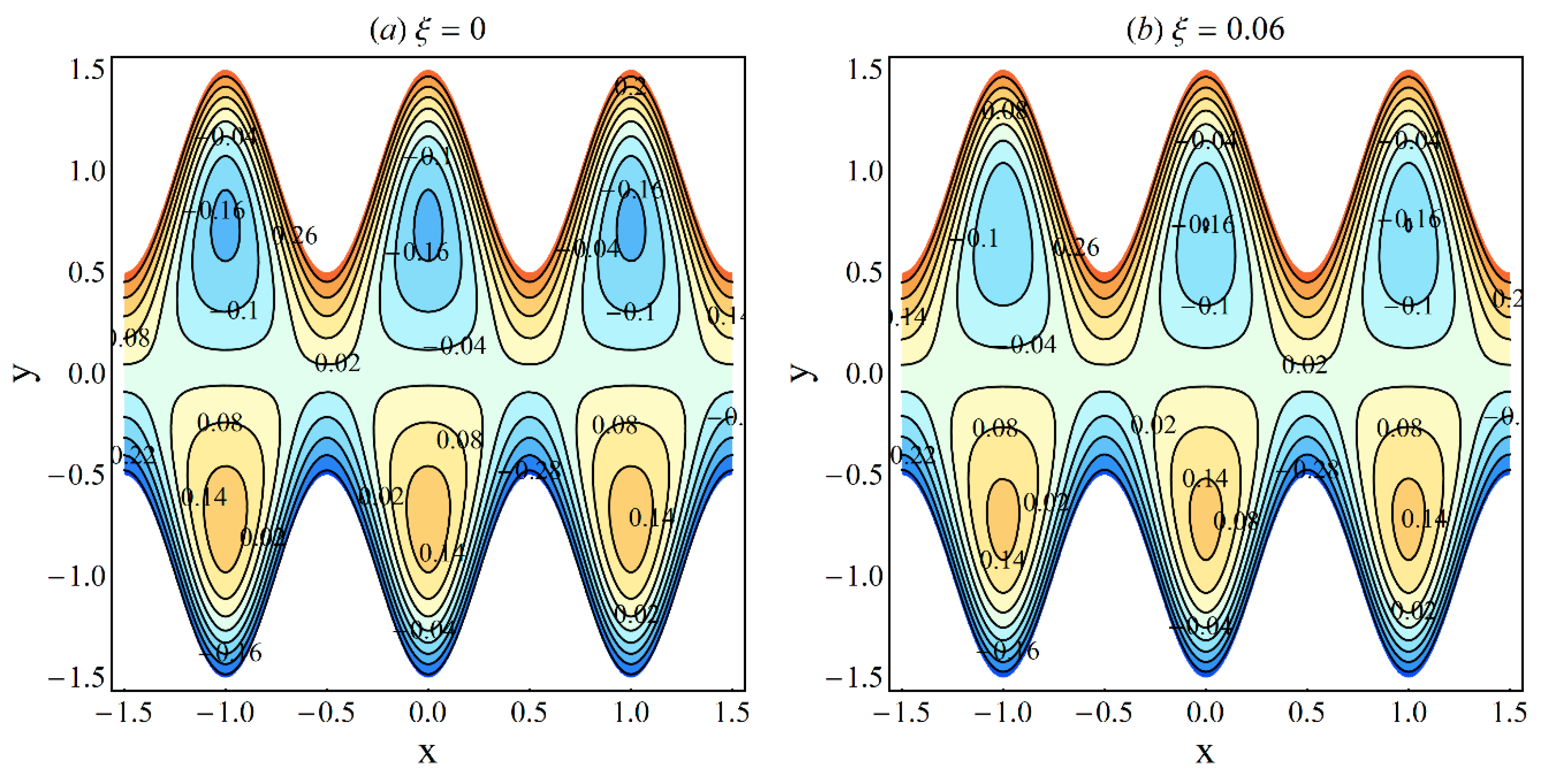
- Near the channel center, fluid friction irreversibility is dominant, whereas near the heated ciliated wall, the effect of heat transfer irreversibility is noticeable;
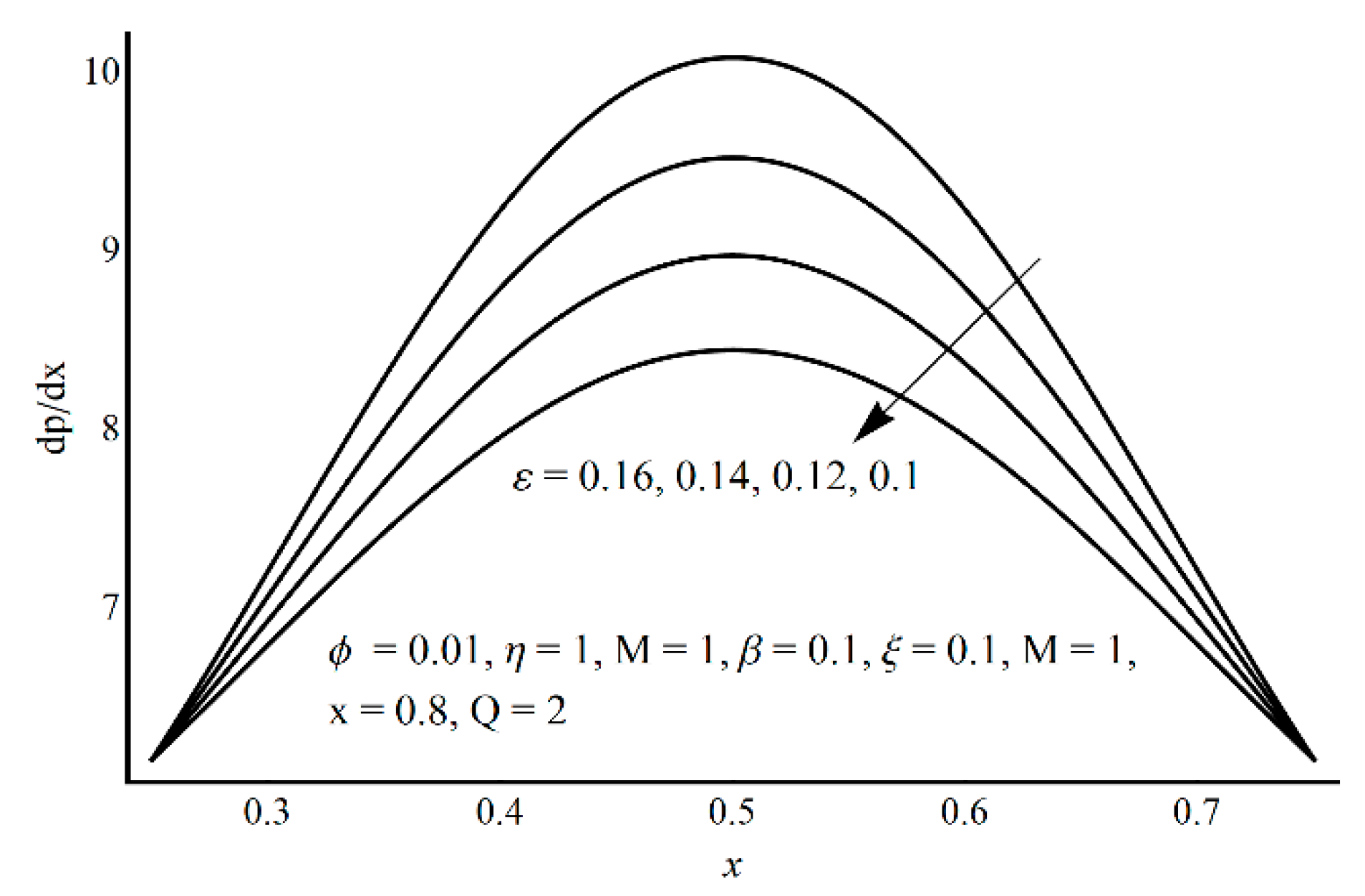
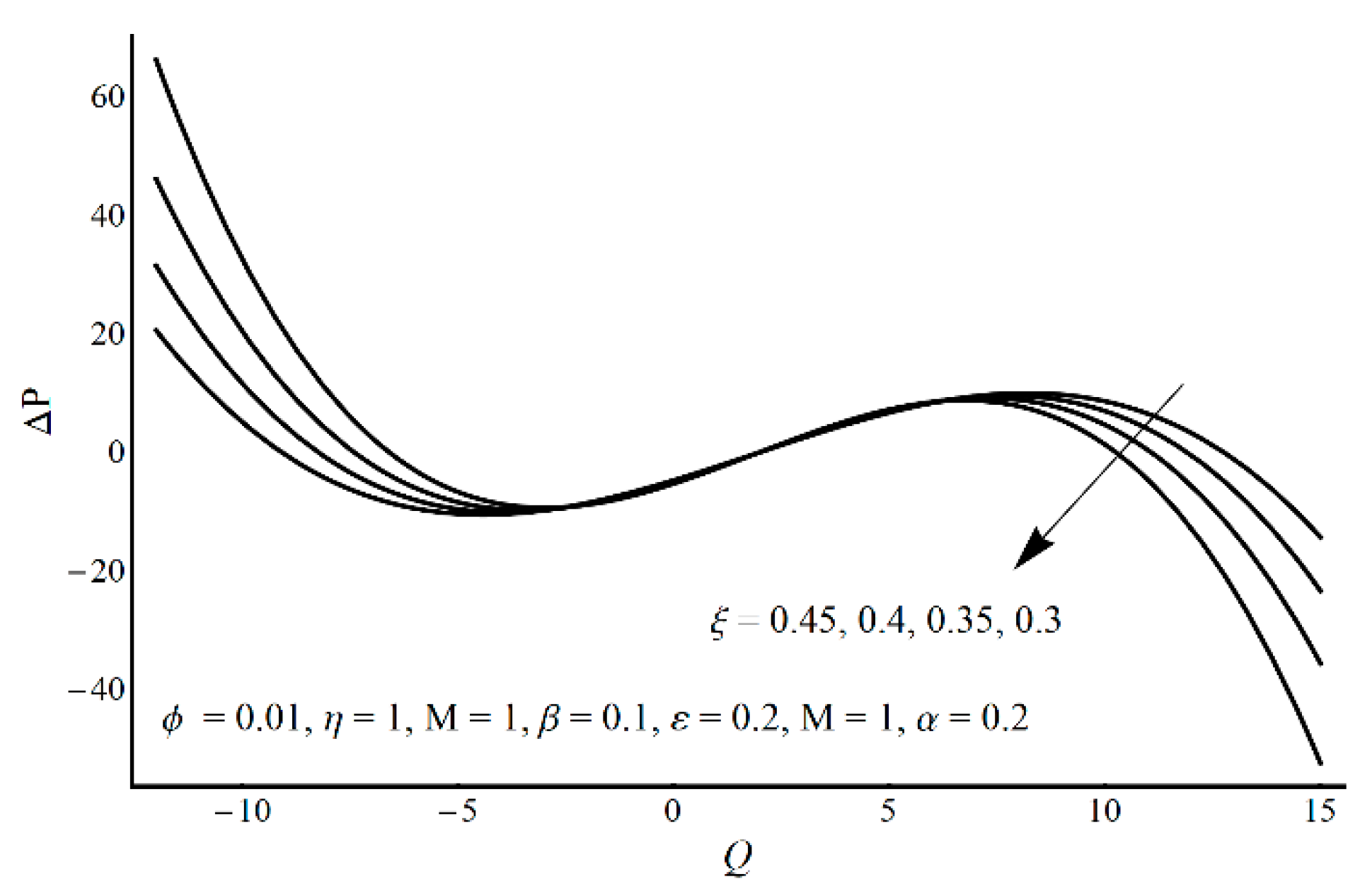
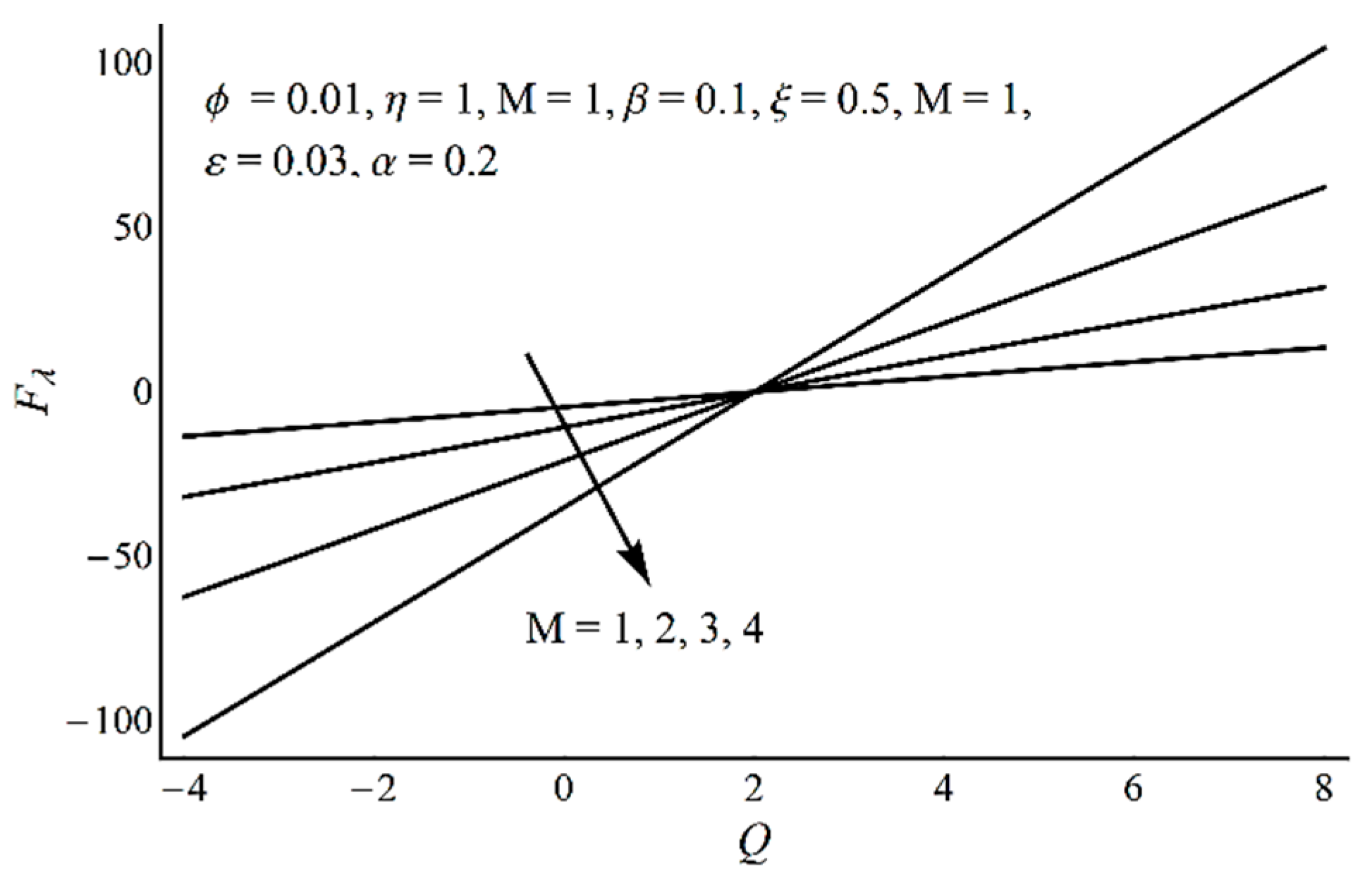
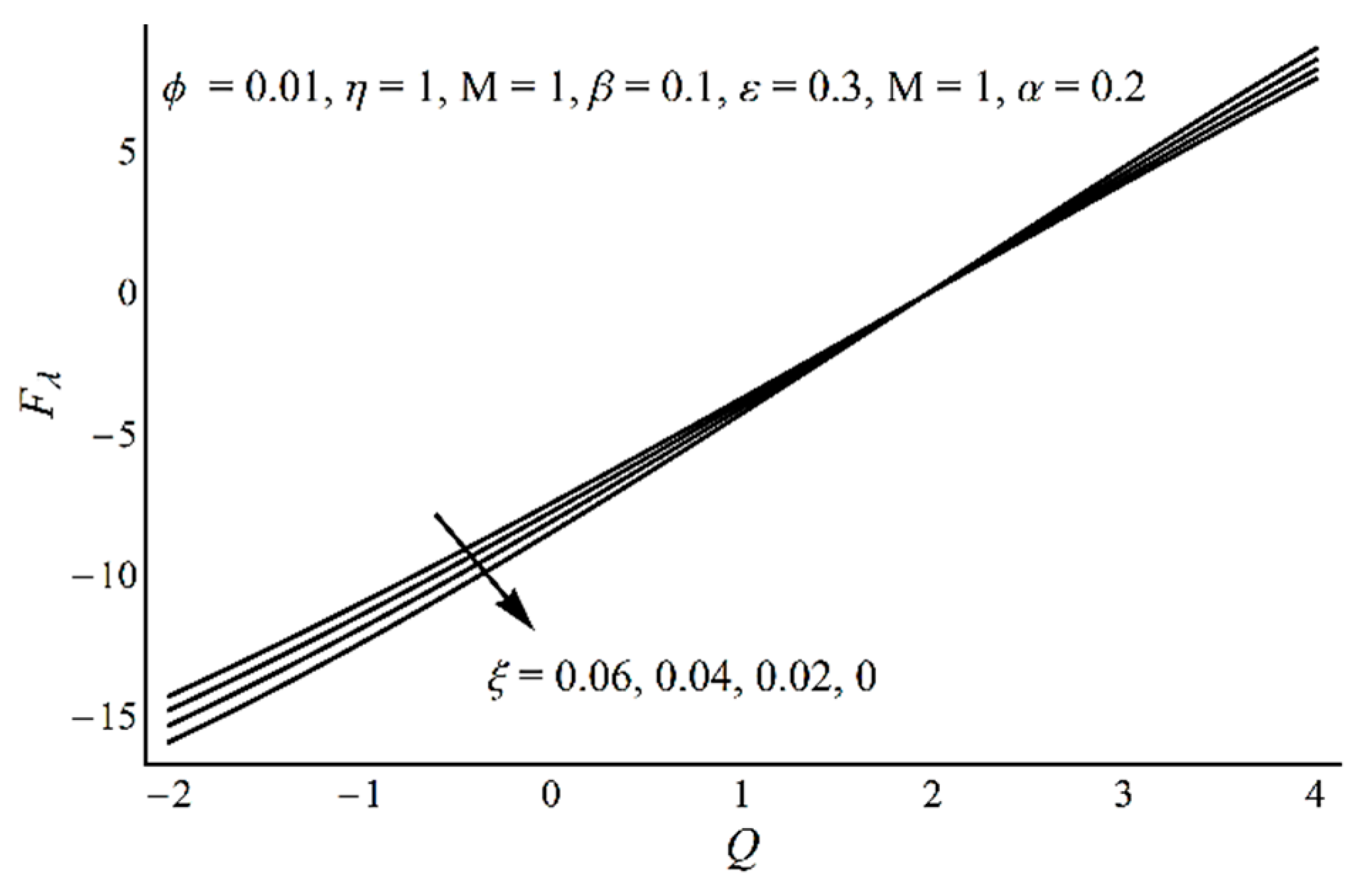
- In the contracted part, a significant elevation in pressure gradient is achieved for small values of ξ and high values of ε and η;
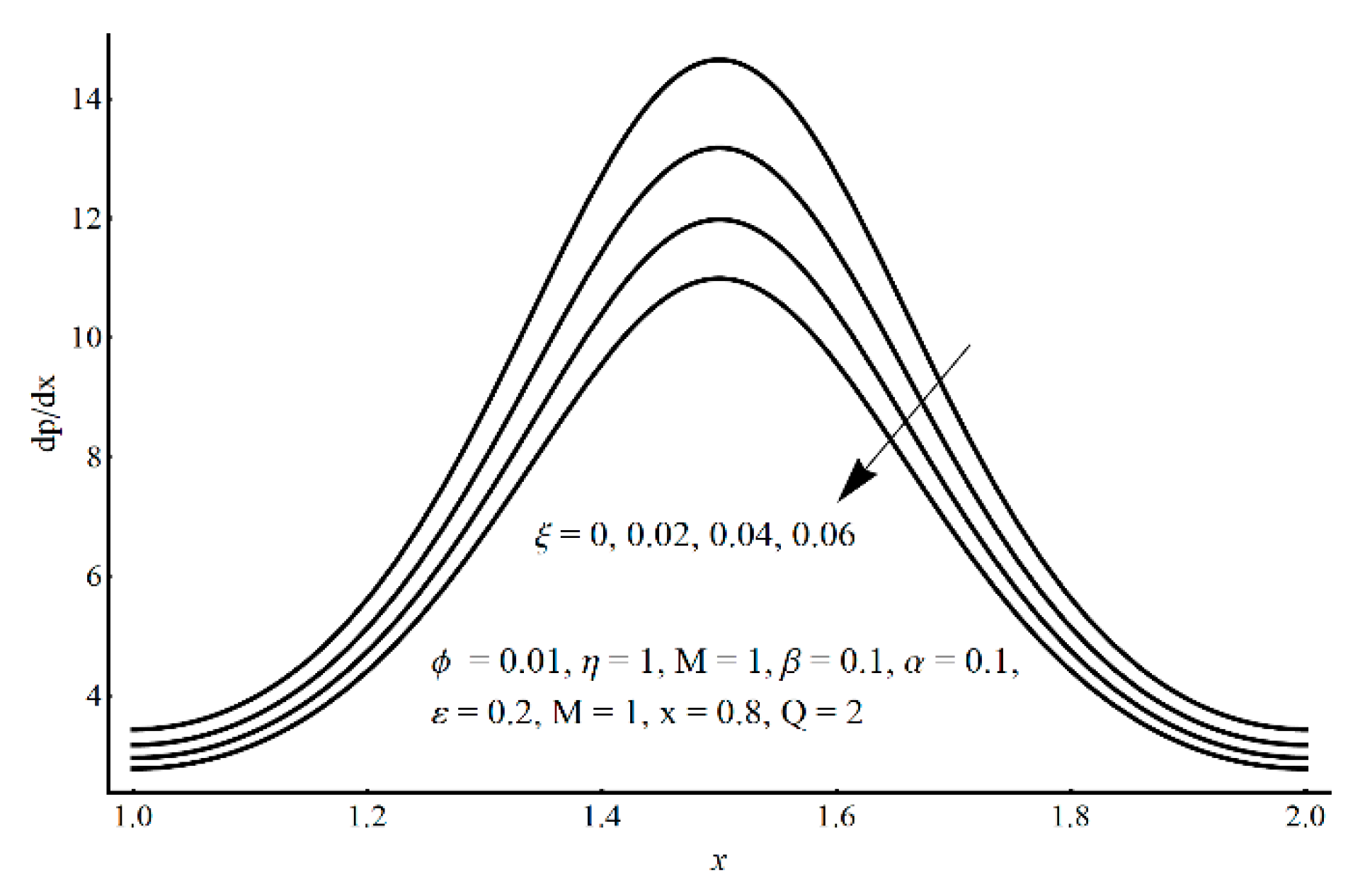
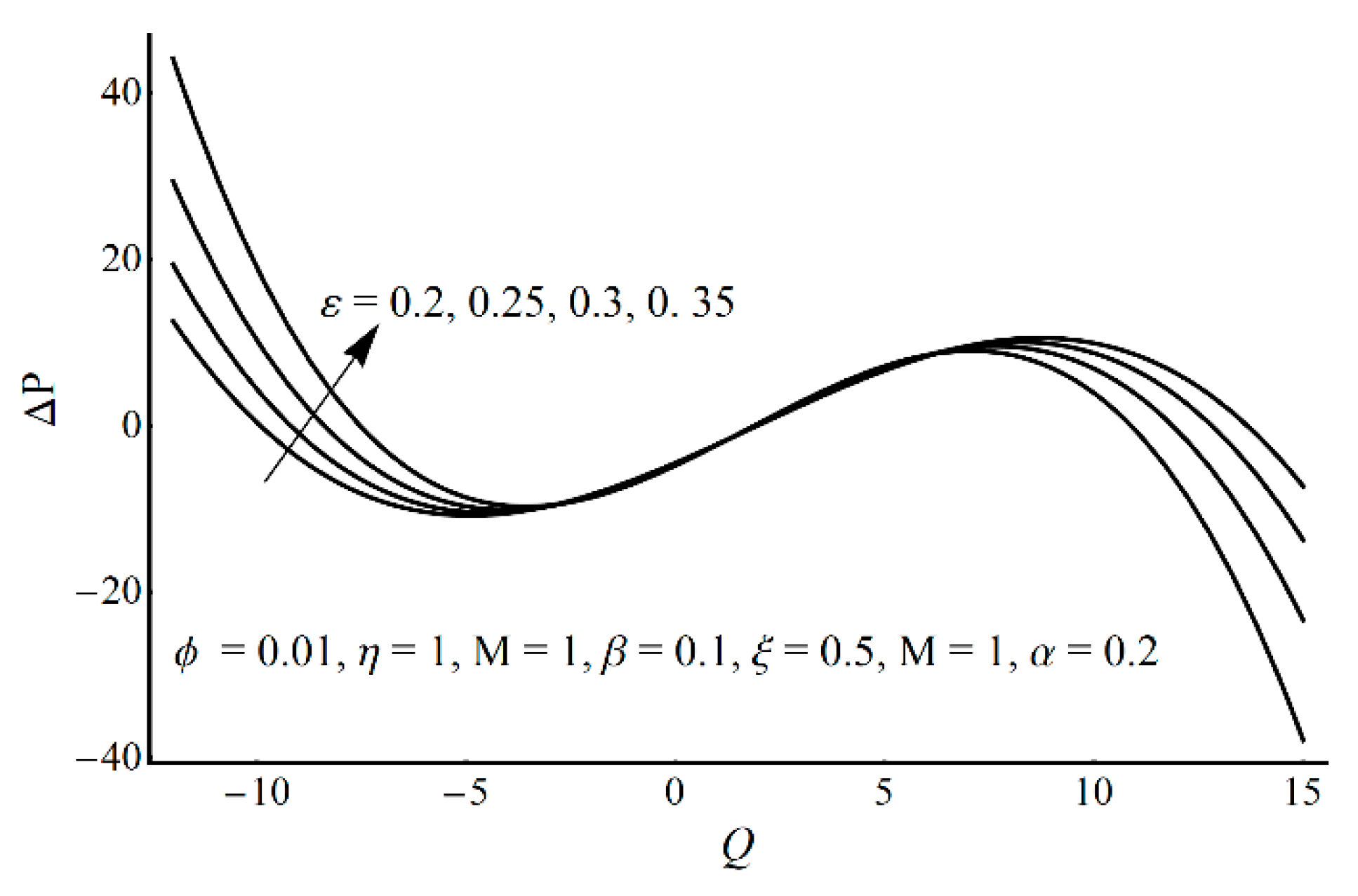
- Pressure-rise per wavelength increases as ε increases and shows a decreasing trend as ξ and η increase in the pumping part and completely reverse conduct in the augmented pumping part;
- Frictional force at the wavy wall explains a direct proportionality with mean flow rate.
Author Contributions
Funding
Conflicts of Interest
References
- Bustamante-Marin, X.M.; Ostrowski, L.E. Cilia and mucociliary clearance. Cold Spring Harb Perspect Biol. 2017, 4, a028241. [Google Scholar] [CrossRef]
- Knowles, M.R.; Boucher, R.C. Mucus clearance as a primary innate defense mechanism for mammalian airways. J. Clin. Investig. 2002, 109, 571–577. [Google Scholar] [CrossRef]
- Pablo, J.L.; DeCaen, P.G.; Clapham, D.E. Progress in ciliary ion channel physiology. J. Gen. Physiol. 2016, 1, 37–41. [Google Scholar] [CrossRef] [Green Version]
- Ghazal, S.; Makarov, J.K.; de Jonge, C.J. Egg transport and fertilization. Glob. Libr. Women Med. 2014, 2014. [Google Scholar] [CrossRef]
- Eddy, C.A.; Pauerstein, C.J. Anatomy and physiology of the fallopian tube. Clin. Obstet. Gynecol. 1980, 4, 1177–1193. [Google Scholar] [CrossRef]
- Lehti, M.S.; Sironen, A. Formation and function of sperm tail structures in association with sperm motility defects. Biol. Reprod. 2017, 97, 522–536. [Google Scholar] [CrossRef]
- Mills, Z.; Aziz, B.; Alexeev, A. Beating synthetic cilia enhance heat transport in microfluidic channels. Soft Matter 2012, 45, 11508–11513. [Google Scholar] [CrossRef]
- Drummond, I.A. Cilia functions in development. Curr. Opin. Cell Biol. 2012, 1, 24–30. [Google Scholar] [CrossRef] [Green Version]
- Agrawal, H.L. Anawaruddin. Cilia transport of bio-fluid wih variable viscosity. Indian J. Pure Appl. Math. 1984, 15, 1128–1139. [Google Scholar]
- Qiu, T.; Lee, T.; Mark, A.G.; Morozov, K.I.; Münster, R.; Mierka, O.; Turek, S.; Leshansky, A.M.; Fischer, P. Swimming by reciprocal motion at low Reynolds number. Nat. Commun. 2014, 5, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Brennen, C. Oscillating-boundary layer theory for ciliary propulsion. J. Fluid Mech. 1974, 65, 799–824. [Google Scholar] [CrossRef] [Green Version]
- Wu, A.; Abbas, S.Z.; Asghar, Z.; Sun, H.; Waqas, M.; Khan, W.A. A shear-rate-dependent flow generated via magnetically controlled metachronal motion of artificial cilia. Biomech. Model. Mechanobiol. 2020. [Google Scholar] [CrossRef]
- Farooq, A.A.; Siddiqui, A.M. Mathematical model for the ciliary-induced transport of seminal liquids through the ductuli efferentes. Int. J. Biomath. 2017, 10, 1750031. [Google Scholar] [CrossRef]
- Farooq, A.A.; Tripathi, D.; Elnaqeeb, T. On the propulsion of micropolar fluid inside a channel due to ciliary induced metachronal wave. Appl. Math. Comput. 2019, 347, 225–235. [Google Scholar] [CrossRef]
- Stud, V.K.; Sephon, G.S.; Mishra, R.K. Pumping action on blood flow by a magnetic field. Bull. Math. Biol. 1977, 39, 385–390. [Google Scholar]
- Mekheimer, K.S. Peristaltic transport of a couple stress fluid in a uniform and non-uniform channels. Biorheology 2002, 39, 755–765. [Google Scholar]
- Hatzikonstantinou, P.M.; Vafeas, P. A general theoretical model for the magnetohydrodynamic flow of micropolar magnetic fluids. Application to Stokes flow. Math. Methods Appl. Sci. 2010, 33, 233–248. [Google Scholar] [CrossRef]
- Tripathi, D.; Jhorar, R.; Beg, O.A. A KadirElectro-magneto-hydrodynamic peristaltic pumping of couple stress biofluids through a complex wavy micro-channel. J. Mol. Liq. 2017, 236, 358–367. [Google Scholar] [CrossRef]
- Akram, S.; Afzal, F.; Imran, M. Influence of metachronal wave on hyperbolic tangent fluid model with inclined magnetic field. Int. J. Geom. Methods. Mod. Phys. 2019, 16, 1950139. [Google Scholar] [CrossRef]
- Mekheimer, K.S.; Mohamed, M.S. Interaction of pulsatile flow on the peristaltic motion of a magneto-micropolar fluid through porous medium in a flexible channel: Blood flow model. Int. J. Pure Appl. Math. 2014, 94, 323–339. [Google Scholar] [CrossRef]
- Saleem, N.; Hayat, T.; Alsaedi, A. A hydromagnetic mathematical model for blood flow of Carreau fluid. Int. J. Biomath. 2014, 7, 1–14. [Google Scholar] [CrossRef]
- Papadopoulos, P.K.; Vafeas, P.; Hatzikonstantinou, P.M. Ferrofluid pipe flow under the influence of the magnetic field of a cylindrical coil. Phys. Fluids 2012, 24, 1–13. [Google Scholar] [CrossRef]
- Taherali, F.; Varum, F.; Basit, A.W. A slippery slope: On the origin, role and physiology of mucus. Adv. Drug Deliv. Rev. 2018, 124, 16–33. [Google Scholar] [CrossRef]
- Tripathi, D.; Beg, O.A.; Curiel-Sosa, J.L. Homotopy semi-numerical simulation of peristaltic flow of generalised Oldroyd-B fluids with slip effects. Comput. Method. Biomec. 2014, 17, 433–442. [Google Scholar] [CrossRef]
- Makinde, O.D.; Reddy, M.G.; Reddy, K.V. Effects of thermal radiation on MHD peristaltic motion of walters-B fluid with heat source and slip conditions. J. Appl. Fluid Mech. 2017, 10, 1105–1112. [Google Scholar] [CrossRef]
- Akram, S. Effects of slip and heat transfer on a peristaltic flow of a Carreau fluid in a vertical asymmetric channel. Comp. Math. Math. Phys. 2014, 54, 1886–1902. [Google Scholar] [CrossRef]
- Akbar, N.; Nadeem, S. Thermal and velocity slip effects on the peristaltic flow of a six constant Jeffrey’s fluid model. Int. J. Heat Mass Tran. 2012, 55, 3964–3970. [Google Scholar] [CrossRef]
- Makinde, O.D. Entropy analysis for MHD boundary layer flow and heat transfer over a flat plate with a convective surface boundary condition. Int. J. Exergy 2012, 10, 142–154. [Google Scholar] [CrossRef]
- Adesanya, S.O.; Makinde, O.D. Thermodynamic analysis for a third-grade fluid through a vertical channel with internal heat generation. J. Hydrodyn. 2015, 27, 264–272. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Selimefendigil, F. MHD free convection and entropy generation in a corrugated cavity filled with a porous medium saturated with nanofluids. Entropy 2018, 20, 846. [Google Scholar] [CrossRef] [Green Version]
- Butt, A.S.; Ali, A.; Munawar, S. Slip effects on entropy generation in MHD flow over a stretching surface in the presence of thermal radiation. Int. J. Exergy. 2013, 13, 1–20. [Google Scholar] [CrossRef]
- Bejan, A. A study of entropy generation in fundamental convective heat transfer. J. Heat Transf. 1979, 101, 718–725. [Google Scholar] [CrossRef]
- Bejan, A. Second-law analysis in heat transfer and thermal design. Adv. Heat Transf. 1982, 15, 1–58. [Google Scholar]
- Souidi, F.; Ayachi, K.; Benyahia, N. Entropy generation rate for a peristaltic pump. J. Non-Equilib. Thermodyn. 2009, 34, 171–194. [Google Scholar] [CrossRef]
- Akbar, N.S. Entropy generation and energy conversion rate for the peristaltic flow in a tube with magnetic field. Energy 2015, 82, 23–30. [Google Scholar] [CrossRef]
- Saleem, N. Entropy production in peristaltic flow of a space dependent viscosity fluid in asymmetric channel. Therm. Sci. 2018, 22, 2909–2918. [Google Scholar] [CrossRef]
- Munawar, S.; Saleem, N.; Aboura, K. Second law analysis in the peristaltic flow of variable viscosity fluid. Int. J. Exergy 2016, 20, 170–185. [Google Scholar]
- Saleem, N.; Munawar, S. Entropy analysis in cilia driven pumping flow of hyperbolic tangent fluid with magnetic field effects. Fluid Dyn. Res. 2020, 52, 2. [Google Scholar] [CrossRef]
- Siddiqui, A.M.; Farooq, A.A.; Rana, M.A. Hydromagnetic flow of Newtonian fluid due to ciliary motion in a channel. Magnetohydrodynamics 2014, 50, 109–122. [Google Scholar]
- Ramesh, K.; Tripathi, D.; Beg, O.A. Cilia-assisted hydromagnetic pumping of biorheological couple stress fluids. J. Propul. Power 2019, 8, 221–233. [Google Scholar] [CrossRef]
- Alsaedi, A.; Batool, N.; Yasmin, H.; Hayat, T. Convective heat transfer analysis on prandtl fluid model with peristalsis. Appl. Bionic. Biomech. 2013, 10, 197–208. [Google Scholar] [CrossRef]
- Patel, M.; Timol, M.G. The stress strain relationship for viscous-inelastic non-Newtonian fluids. Int. J. Appl. Math. Mech. 2010, 6, 79–93. [Google Scholar]
- Hayat, T.; Saleem, N.; Ali, N. Peristaltic flow of a Carreau fluid in a channel with different wave forms. Numer. Meth. Part. Differ. Equ. 2010, 26, 519–534. [Google Scholar] [CrossRef]
- Tripathi, D. A mathematical model for the peristaltic flow of chyme movement in small intestine. Math. Biosci. 2011, 233, 90–97. [Google Scholar] [CrossRef]
- Mehmood, O.U.; Mustapha, N.; Shafie, S. Heat transfer on peristaltic flow of fourth grade fluid in inclined asymmetric channel with partial slip. Appl. Math. Mech. 2012, 33, 1313–1328. [Google Scholar] [CrossRef] [Green Version]
- Jothi, S.; Prasad, A.R.; Reddy, M.V.S. Peristaltic flow of a Prandtl fluid in a symmetric channel under the effect of a magnetic field. Adv. Appl. Sci. Res. 2012, 3, 2108–2119. [Google Scholar]
- Bejan, A. Second law analysis in heat transfer. Energy 1980, 5, 720–732. [Google Scholar] [CrossRef]
- Munawar, S.; Saleem, N. Second law analysis of ciliary pumping transport in an inclined channel coated with carreau fluid under a magnetic field. Coatings 2020, 10, 240. [Google Scholar] [CrossRef] [Green Version]











© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Munawar, S.; Saleem, N. Entropy Analysis of an MHD Synthetic Cilia Assisted Transport in a Microchannel Enclosure with Velocity and Thermal Slippage Effects. Coatings 2020, 10, 414. https://doi.org/10.3390/coatings10040414
Munawar S, Saleem N. Entropy Analysis of an MHD Synthetic Cilia Assisted Transport in a Microchannel Enclosure with Velocity and Thermal Slippage Effects. Coatings. 2020; 10(4):414. https://doi.org/10.3390/coatings10040414
Chicago/Turabian StyleMunawar, Sufian, and Najma Saleem. 2020. "Entropy Analysis of an MHD Synthetic Cilia Assisted Transport in a Microchannel Enclosure with Velocity and Thermal Slippage Effects" Coatings 10, no. 4: 414. https://doi.org/10.3390/coatings10040414
APA StyleMunawar, S., & Saleem, N. (2020). Entropy Analysis of an MHD Synthetic Cilia Assisted Transport in a Microchannel Enclosure with Velocity and Thermal Slippage Effects. Coatings, 10(4), 414. https://doi.org/10.3390/coatings10040414





