Heat Transfer Effect on Viscoelastic Fluid Used as a Coating Material for Wire with Variable Viscosity

Abstract

This article examines a wire coating technique using a viscoelastic Eyring–Powell fluid in which magnetohydrodynamic (MHD) flow, thermal transfer, and Joule heating effects are studied. Temperature-dependent, variable-viscosity models are used. Flexible-viscosity models which are temperature dependent are also considered. The interface of the thermal boundary layer which describe the flux and thermal convection phenomena, are evaluated by using a dominant numerical technique known as the fourth-order Runge–Kutta method. In particular, this article takes into account the impact of a permeable matrix which behaves like a dielectric in order to avoid heat dissipation. The effect of thermal generation is also explained, since it controls power. The novel effects for the numerous parameters which affect the velocity and temperature profiles on the wire coating process are investigated through graphs explained in detail. These include non-Newtonian, hydromagnetic, permeability, and heat source/sink effects. For validation purposes, the numerical scheme is also compared with a semi-numerical technique HAM and BVPh2 software, and found a closed agreement with the numerical results.

1. Introduction

Wire coating is an extrusion process that is usually used in the polymer manufacturing industries for the insulation of wires and cables. There are five units in a typical wire coating apparatus: a pay-off device, a wire preheater, an extruder equipped with a crosshead die, a cooling trough, and a take-up device. There are two classes of cross-sectional dies that are normally used in wire coating analysis—tubing-type die and pressure-type die. The pressure-type die closely resembles an annulus. Consequently, flow through this type of die has a similarity with the flow through the annular region formed by two coaxial cylinders, where the inner cylinder is moving in the axial direction while the outer cylinder is fixed. Different types of fluids are used for wire coating, depending on the geometry of the die, the fluid viscosity, and the temperature of the wire and molten polymer. Significant attention has been given to studying heat transfer analysis in Newtonian fluids. Numerous fluids such as air, water, and some oils are considered as non-Newtonian fluids in science and engineering technologies. In many circumstances, Newtonian fluid behavior may be complex. Therefore, a perturbed non-Newtonian model must be considered. Non-Newtonian behavior exists in liquid materials such as glue, paint, ketchup, custard, and blood. Because it has vast and significant applications in fluid mechanics and industries such as petroleum and chemical engineering, Non-Newtonian behavior has therefore gained the attention of researchers [1,2,3,4,5,6,7,8,9].

Ellahi et al. [10] discussed the non-Newtonian micro-polar liquid in blood movement through a composite stenosis. An Eyring–Powell fluid is a non-Newtonian fluid which was first presented in 1944 by Eyring and Powell. Many important features of an Eyring–Powell fluid are discussed by researchers [11,12,13,14,15]. Wire coating technique is very important in order to avoid injuries and decrease the losses that could be generated by machine oscillation. In manufacturing industries, various liquid polymers are used in the wire coating process. Melt polymer is poured over a wire and then the wire is pulled through the die that is covered by viscoelastic material. Three methods of wire coating are electro-static deposition, coaxial processing, and dipping. Compared to the first two, the dipping method of wire coating provides a stronger bond in the field, but is very slow. It contains a payoff device, a die and extruder device, a cooling device, a preheater, a straightener, a tester, a capstan, and a take-up reel. In this process, a bare wire is rolled over the payoff device and then goes through the straightener. Heat is then applied to the wire by a preheater and crosshead die, which has a conical die holding the liquid polymer where the wire is coated. The temperature of the hot, coated wire is reduced with a cooling device, after which it passes through a capstan and then a tester. Finally, the coated wire is puffed at the take-up reel. Many other researchers [16,17,18,19,20,21,22,23,24] have used various non-Newtonian materials for the wire coating process. In the magnetohydrodynamic (MHD) process, a magnetic field induces a current, which has a major effect on the motion of the fluid material. In recent years, the MHD process has been an attractive area for researchers because of its extensive use in industries such as magnetic-material and glass manufacturing. Many researchers [25,26,27,28,29,30,31,32,33] describe the MHD process as a current conducting liquid in the presence of an applied magnetic field.

Fluid flux through a permeable medium has a countless significance for scientists because of its broad scope in engineering technology. Some renowned permeable media are wood, carbonate rocks, and metal foams. Many researchers [34,35,36,37,38] have paid attention to permeable media. Currently, a thin permeable layer has domestic and industrial applications such as filters, batteries, fuel cells, and printing papers. The study of heat convection in non-Newtonian liquids has gained interest with time because of its application to different industries. Rehman and Nadeem [39] investigated heat convective analysis for multi-directional stagnation flow movement. Zeeshan, along with other researchers [40,41,42,43,44,45,46], studied the effect of thermal convection and hydromagnetic fluid flow. By studying all of the above articles, we found that a wire coating technique with an MHD process with a viscoelastic Eyring–Powell liquid as a coating substance has yet not been discussed. This paper discusses the procedure of a wire coating process with the impact of thermal generation and permeable media along with temperature-dependent flexible viscosity using Vogel’s and Reynolds’ models.

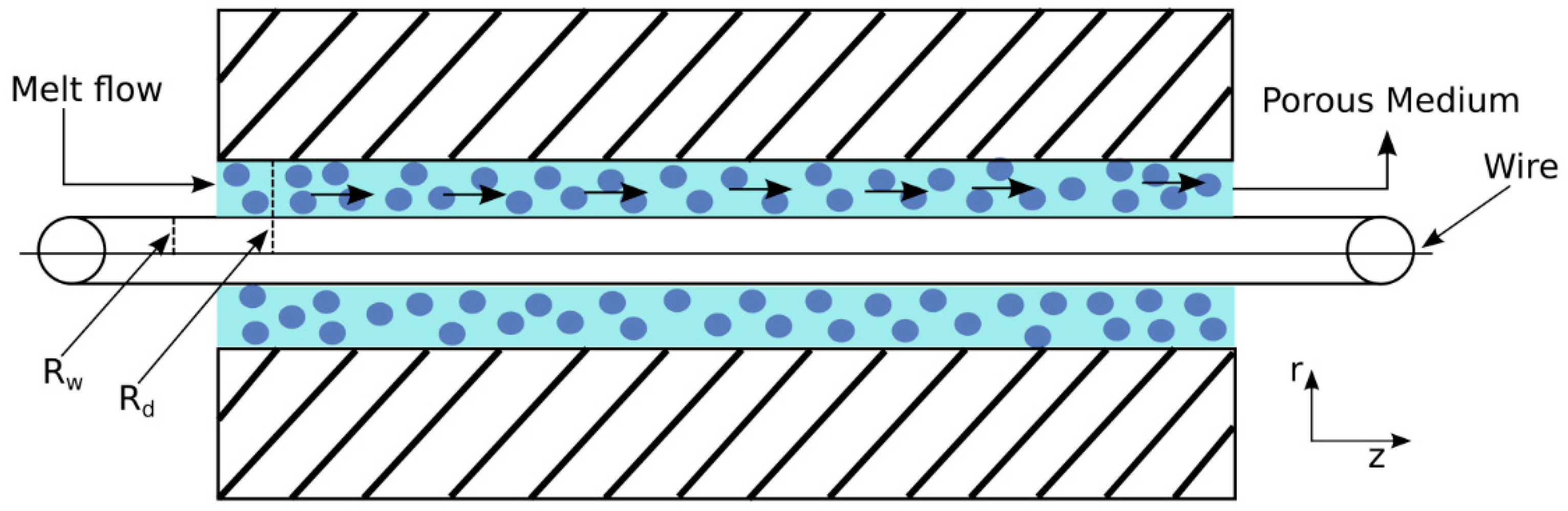
2. Modeling of Wire Coating

In Figure 1 the geometry of our problem is described. The parameters include the length of the pressure die $L$, radius $R_d$, and the saturated temperature ${\theta }_d$ due to incompressible viscoelastic Eyring–Powell material. As the temperature of the wire reaches ${\theta }_W,$ the radius equals $R_W$ and the velocity becomes $U_W$ in the permeable medium. The wire is then pulled through the center length of the die in the static-pressure die. The out-flux fluid is acted upon simultaneously by uniform pressure gradient $\frac{dp}{dz}$ along the axis of the object and by a magnetic field of strength $B_0$. The magnetic field is perpendicular to the direction of the Eyring–Powell incompressible fluid movement. To reduce or neglect the perturbation in the magnetic field, we used the Reynolds number in our problem. The wire and die have a common axis of symmetry, which was taken as a reference for the coordinated system. The appropriate expressions for the fluid velocity $\overrightarrow{q}$, the stress tensor $S$, and the field temperature for this problem can be taken as:

$$\overrightarrow{\mathit{q}}=oi+0j+w\left(r\right)k$$

(1)

$$S=S\left(r\right)$$

(2)

$$\theta =\theta \left(r\right)$$

(3)

The Cauchy stress tensor for the Eyring–Powell viscoelastic fluid is as

$$S=\mu \nabla \mathrm{v}+\frac{1}{\beta }{\sin }^{-1}\left(\frac{1}{C}\nabla \mathrm{v}\right)$$

(4)

where $\mu$ denotes the viscosity, $S$ denotes the Cauchy stress tensor, $V$ denotes the velocity, and $C$ denotes the material constant. Equation (4) can be expressed as

$${\sin }^{-1}\left(\frac{1}{C}\nabla \mathrm{v}\right)=\frac{1}{C}\nabla \mathrm{v}-\frac{1}{6}{\left(\frac{1}{C}\nabla \mathrm{v}\right)}^3,|\frac{1}{C}\nabla \mathrm{v}|\ll 1$$

(5)

The boundary conditions for the proposed model take the form of

$$w\left(R_w\right)=U_w,\theta \left(R_w\right)={\theta }_w,w\left(R_d\right)=0,\theta \left(R_d\right)={\theta }_d$$

(6)

Governing equations are given by

$$\nabla \overrightarrow{\mathit{q}}=0$$

(7)

$$\rho \left(\frac{D\overrightarrow{\mathit{q}}}{Dt}\right)=\overrightarrow{F}-\nabla p+\frac{\mu \overrightarrow{\mathit{q}}}{K_p^{*}}$$

(8)

$$\rho C_p\left(\frac{D\overrightarrow{\mathit{q}}}{Dt}\right)=k{\nabla }^2+\phi +Q_0\left(\theta -{\theta }_w\right)+J_d$$

(9)

where $\overrightarrow{\mathit{q}}$ is the velocity vector, $\rho$ represents the density, $\frac{D}{Dt}$ represents the temporal derivative, $Q_0$ represents the rate of volumetric heat generation, and $J_d$ is the Joule dissipation term.

Applying Equations (1)–(3), the continuity of Equation (7) is identically satisfied and we get nonvanishing components of extra stress tensor $S$ as

$$S_{zr}=\left(\mu +\frac{1}{\beta C}\right)\frac{dw}{dr}-\frac{1}{6\beta C^3}{\left(\frac{dw}{dr}\right)}^3$$

(10)

Putting the velocity field and Equations (9)–(10) into Equation (8), we get

$$\frac{\partial p}{\partial r}=0$$

(11)

$$\frac{\partial p}{\partial \theta }=0$$

(12)

$$\frac{\partial p}{\partial z}=\frac{1}{r}\frac{d}{dr}\left[r\left\{\left(\mu +\frac{1}{\beta C}\right)\frac{dw}{dr}-\frac{1}{6\beta C^3}{\left(\frac{dw}{dr}\right)}^3\right\}\right]-\frac{\mu w}{K_p^{*}}$$

(13)

However, Equation (13) shows the flow due to the pressure gradient. When we leave the die, only dragging of the wire occurs. Therefore, the pressure gradient contributes nothing in the axial direction. So, Equation (14) takes the form

$$\frac{1}{r}\frac{d}{dr}\left[r\left\{\left(\mu +\frac{1}{\beta C}\right)\frac{dw}{dr}-\frac{1}{6\beta C^3}{\left(\frac{dw}{dr}\right)}^3\right\}\right]-\frac{\mu w}{K_p^{*}}=0$$

(14)

and the energy Equation (9) becomes

$$K\left(\frac{d^2\theta }{d{r}^2}+\frac{1}{r}\frac{d\theta }{dr}\right)+\left(\left(\mu +\frac{1}{\beta C}\right)\frac{dw}{dr}-\frac{1}{6\beta C^3}{\left(\frac{dw}{dr}\right)}^3\right).\frac{dw}{dr}+Q_0\left(\theta -{\theta }_w\right)=0$$

(15)

The dimensionless parameters are defined as:

$$\begin{array}{l}{r}^{*}=\frac{r}{R_w},w^{*}=\frac{w}{U_w},K_p=\frac{R_w^2}{K_p^{*}},w=\frac{v_0}{U_w},N=\frac{1}{\mu \beta C},{\theta }^{*}=\frac{\left(\theta -{\theta }_w\right)}{\left({\theta }_d-{\theta }_w\right)}\\ Q=\frac{Q_0{R}_w^2}{K},Br=\frac{\mu U_w^2}{K\left({\theta }_d-{\theta }_w\right)},R_w=\frac{\beta v_0}{\mu },\epsilon =\frac{\mu }{6w^2{\left(\beta C\right)}^3}\end{array}$$

(16)

Using these new variables in Equations (13) and (14) with Equation (6) and after removing asterisks, we get the following:

$$\left(1+N\right)\left(r\frac{d^{2}w}{d{r}^2}+\frac{dw}{dr}\right)-\epsilon \left({\left(\frac{dw}{dr}\right)}^3+3r{\left(\frac{dw}{dr}\right)}^2\frac{d^{2}w}{d{r}^2}\right)-K_{p}wr=0$$

(17)

$$w\left(1\right)=1andw\left(\delta \right)=0$$

(18)

$$\frac{d^2\theta }{d{r}^2}+\frac{1}{r}\frac{d\theta }{dr}+B_r\left(1+N\right){\left(\frac{dw}{dr}\right)}^2+\epsilon B_r{\left(\frac{dw}{dr}\right)}^4+Q\theta =0$$

(19)

$$\theta \left(1\right)=0and\theta \left(\delta \right)=1$$

(20)

3. Temperature-Dependent Viscosity

Two basic models are used for temperature-dependent viscosity: Reynolds’ model and Vogel’s model, whose details are given below.

3.1. Reynolds’ Model

Here we use Reynolds’ model to explain temperature-dependent viscosity. The temperature-dependent viscosity for the Reynolds model can be expressed by the following relation:

$$\mu =1-{\beta }_{0}m\theta$$

(21)

This is applied for the variation of temperature-dependent viscosity, while $m$ is used for the viscosity parameter. Using nondimensional parameters,

$$\begin{array}{l}{r}^{*}=\frac{r}{R_w},w^{*}=\frac{w}{U_w},K_p=\frac{R_w^2}{K_p^{*}},w=\frac{v_0}{U_w},N=\frac{1}{{\mu }_0\beta C},{\mu }^{*}=\frac{\mu }{{\mu }_0}\\ {\theta }^{*}=\frac{\left(\theta -{\theta }_w\right)}{\left({\theta }_d-{\theta }_w\right)},Q=\frac{Q_0{R}_w^2}{K},Br=\frac{{\mu }_0{U}_w^2}{K\left({\theta }_d-{\theta }_w\right)},R_w=\frac{\beta v_0}{{\mu }_0},\epsilon =\frac{{\mu }_0}{6w^2{\left(\beta C\right)}^3}\end{array}$$

(22)

After removing asterisks, we obtain nondimensional forms of momentum and energy equations along boundary conditions:

$$\begin{array}{l}\frac{d^{2}w}{d{r}^2}\left(r\left(1-{\beta }_{0}m\theta \right)+rN-3r\epsilon {\left(\frac{dw}{dr}\right)}^2\right)+\frac{dw}{dr}\left(1-{\beta }_{0}m\theta +N-{\beta }_{0}mr\frac{d\theta }{dr}\right)\\ -\epsilon {\left(\frac{dw}{dr}\right)}^3-K_{p}wr=0\end{array}$$

(23)

$$w\left(1\right)=1andw\left(\delta \right)=0$$

(24)

$$\frac{d^2\theta }{d{r}^2}+\frac{1}{r}\frac{d\theta }{dr}+\left(1-{\beta }_{0}m\theta \right)B_r{\left(\frac{dw}{dr}\right)}^2+B_r{\left(\frac{dw}{dr}\right)}^2\left(N+\epsilon \right)+Q\theta =0$$

(25)

$$\theta \left(1\right)=0and\theta \left(\delta \right)=1$$

(26)

3.2. Vogel’s Model

In this case, we take temperature-dependent viscosity as

$$\mu ={\mu }_0\exp \left(\frac{D}{B^{\prime }+\theta }-{\theta }_w\right)$$

(27)

Applying expansions, we get

$$\mu =\mathsf{\Omega }\left(1-\frac{D\theta }{{\left(B^{\prime }\right)}^2}\right)$$

(28)

where $D,B$ are parameters of viscosity and

$$\mathsf{\Omega }={\mu }_0\exp \left(\frac{D}{{\left(B^{\prime }\right)}^2}-{\theta }_w\right)$$

(29)

We obtain nondimensional equations of momentum and energy along boundary conditions after removing asterisks

$$\begin{array}{l}\frac{d^{2}w}{d{r}^2}\left(r\mathsf{\Omega }\left(1-\frac{D\theta }{{\left(B^{\prime }\right)}^2}\right)+rN-3r\epsilon {\left(\frac{dw}{dr}\right)}^2\right)+\frac{dw}{dr}\left(\mathsf{\Omega }\left(1-\frac{D\theta }{{\left(B^{\prime }\right)}^2}\right)+N-\mathsf{\Omega }\frac{D}{{\left(B^{\prime }\right)}^2}r\frac{d\theta }{dr}\right)\\ -\epsilon {\left(\frac{dw}{dr}\right)}^3-K_{p}wr=0\end{array}$$

(30)

$$w\left(1\right)=1andw\left(\delta \right)=0$$

(31)

$$\frac{d^2\theta }{d{r}^2}+\frac{1}{r}\frac{d\theta }{dr}+\mathsf{\Omega }\left(1-\frac{D\theta }{{\left(B^{\prime }\right)}^2}\right)B_r{\left(\frac{dw}{dr}\right)}^2+B_r{\left(\frac{dw}{dr}\right)}^2\left(N+\epsilon \right)+Q\theta =0$$

(32)

$$\theta \left(1\right)=0and\theta \left(\delta \right)=1$$

(33)

4. Solution Procedure via Fourth-Order Runge–Kutta Method

Equations (23)–(26) in the case of Reynolds’ model, and Equations (30)–(33) for Vogel’s model are solved by using the fourth-order Runge–Kutta method along with shooting techniques. First, the above governing higher-order differential equations are converted into first-order ordinary differential equations.

Equations (23) and (25) can be written as

$$\frac{d^{2}w}{d{r}^2}=\frac{\epsilon {\left(\frac{dw}{dr}\right)}^3-\left(1+N\right)\frac{dw}{dr}+K_{p}wr}{\left(1+N\right)r+3r\epsilon {\left(\frac{dw}{dr}\right)}^2}.$$

(34)

$$\frac{d^2\theta }{d{r}^2}=-\left[\frac{1}{r}\frac{d\theta }{dr}+B_r\left(1+N\right){\left(\frac{dw}{dr}\right)}^2+\epsilon B_r{\left(\frac{dw}{dr}\right)}^4+Q\theta \right]$$

(35)

New variables are defined to convert higher-order ordinary differential equations into first order as

$$w=z_1,w^{\prime }=z_2,w^{″}=z_2^{\prime }and\theta =z_3,{\theta }^{\prime }=z_4,{\theta }^{″}=z_4^{\prime }$$

(36)

$$z_2^{\prime }=\frac{\epsilon {\left(z_2\right)}^3-\left(1+N\right)z_2+K_p{z}_{1}r}{\left(1+N\right)r+3r\epsilon {\left(z_2\right)}^2}$$

(37)

$$z_4^{\prime }=-\left[\frac{1}{r}{z}_4+B_r\left(1+N\right){\left(z_2\right)}^2+\epsilon B_r{\left(z_2\right)}^4+Q{z}_3\right]$$

(38)

The boundary conditions given in Equations (23) and (25) are changed into initial conditions as

$$z_1\left(1\right)=1and{\mathrm{z}}_1\left(\delta \right)=0$$

(39)

$$z_3\left(1\right)=0and{\mathrm{z}}_3\left(\delta \right)=1$$

(40)

4.1. Reynolds’ Model

Equations (30) and (32) may be written after using Equation (36) as

$$z_2^{\prime }=\frac{\epsilon {\left(z_2\right)}^3+K_p{z}_{1}r-z_2\left(1-{\beta }_{0}m{z}_3+N-{\beta }_{0}mr{z}_4\right)}{\left(r\left(1-{\beta }_{0}m{z}_3\right)+rN-3r\epsilon {\left(z_2\right)}^2\right)}$$

(41)

$$z_4^{\prime }=-\frac{1}{r}\left[z_4+\left(1-{\beta }_{0}m{z}_3\right)B_r{\left(z_2\right)}^2+B_r{\left(z_2\right)}^2\left(N+\epsilon \right)+Q{z}_3\right]$$

(42)

The boundary conditions given in Equations (31) and (33) are changed into initial conditions as

$$z_1\left(1\right)=1and{\mathrm{z}}_1\left(\delta \right)=0$$

(43)

$$z_3\left(1\right)=0and{\mathrm{z}}_3\left(\delta \right)=1$$

(44)

4.2. Vogel’s Model

Using Equation (36) in Equations (30) and (32) we get the desired first-order differential equations as

$$z_2^{\prime }=\frac{\epsilon {\left(z_2\right)}^3+K_p{z}_{1}r-z_2\left(\mathsf{\Omega }\left(1-\frac{D{z}_3}{{\left(B^{\prime }\right)}^2}\right)+N-\mathsf{\Omega }\frac{D}{{\left(B^{\prime }\right)}^2}r{z}_4\right)}{r\mathsf{\Omega }\left(1-\frac{D{z}_3}{{\left(B^{\prime }\right)}^2}\right)+rN-3r\epsilon {\left(z_2\right)}^2}$$

(45)

$$z_4^{\prime }=-\left[\frac{1}{r}{z}_4+\mathsf{\Omega }\left(1-\frac{D{z}_3}{{\left(B^{\prime }\right)}^2}\right)B_r{\left(z_2\right)}^2+B_r{\left(z_2\right)}^2\left(N+\epsilon \right)+Q{z}_3\right]$$

(46)

along with transformed boundary conditions as

$$z\left(1\right)=1and{\mathrm{z}}_1\left(\delta \right)=0$$

(47)

$$z_3\left(1\right)=0and{\mathrm{z}}_3\left(\delta \right)=1$$

(48)

5. Validation of the Method

To validate our numerical solution, we made the following comparison, which proves the thoroughness of the proposed method. Our comparison is illustrated in

Table 1. Comparison of numerical, HAM, and BVPh2 methods for velocity profile.

$\mathit{w}(\mathit{r})$	Numerical Solution	HAM Solution	BVPh2
1.0	$1.0000000000$	$1.0000000000$	$1.0000000000$
1.2	$0.6369559249$	$0.6369559343$	$0.6369559239$
1.4	$0.4274349292$	$0.4274349325$	$0.4274349352$
1.6	$0.3071882095$	$0.3071882321$	$0.3071882632$
1.8	$0.1968612792$	$0.1968612624$	$0.1968612547$
2.0	0.00000000000	0.00000000000	0.00000000000

and

Table 2. Comparison of numerical, HAM, and BVPh2 methods for temperature profile.

$\mathit{\theta }(\mathit{r})$	Numerical Solution	HAM Solution	BVPh2
1.0	0.00000000000	0.00000000000	0.00000000000
1.2	$0.9500451152$	$0.9500451271$	$0.9500451321$
1.4	$1.9209191828$	$1.9209191651$	$1.9209191658$
1.6	$2.7196458893$	$2.7196458689$	$2.7196458567$
1.8	$2.7456308985$	$2.7456308634$	$2.7456308695$
2.0	1.00000000000	1.00000000000	1.00000000000

. An excellent agreement is noted between the fourth-order Runge–Kutta method, the semi-numerical method HAM, and BVPh2.

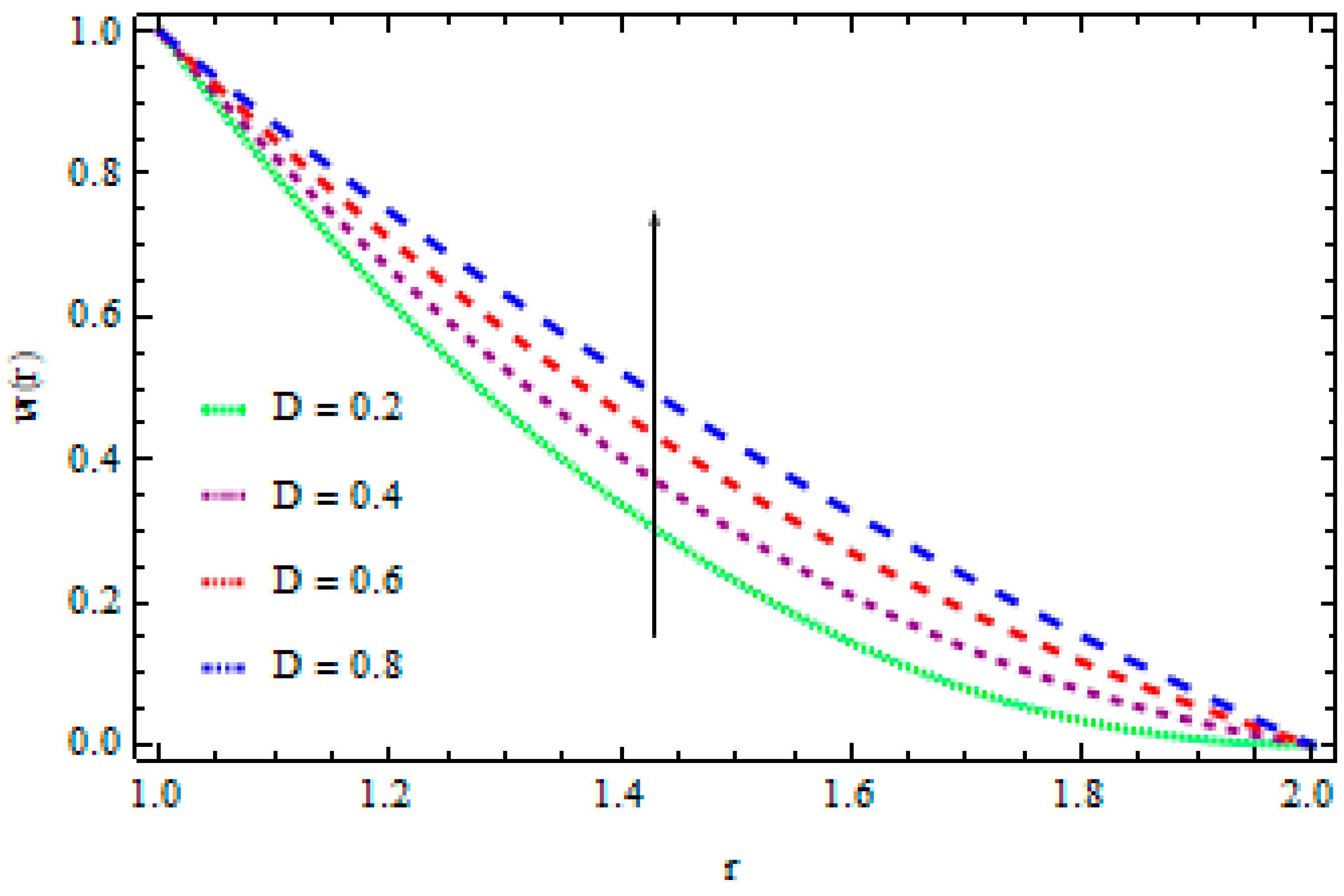
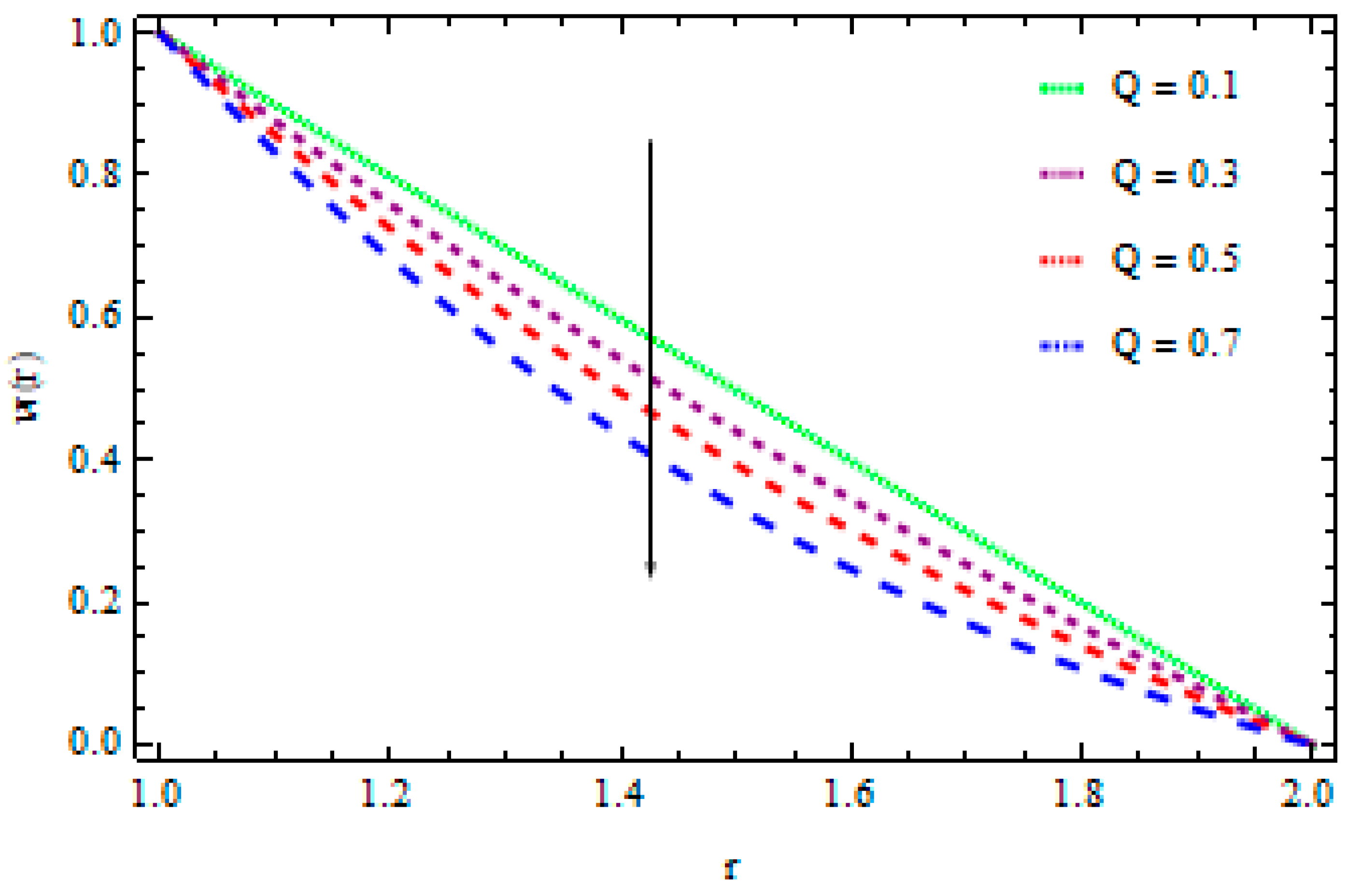
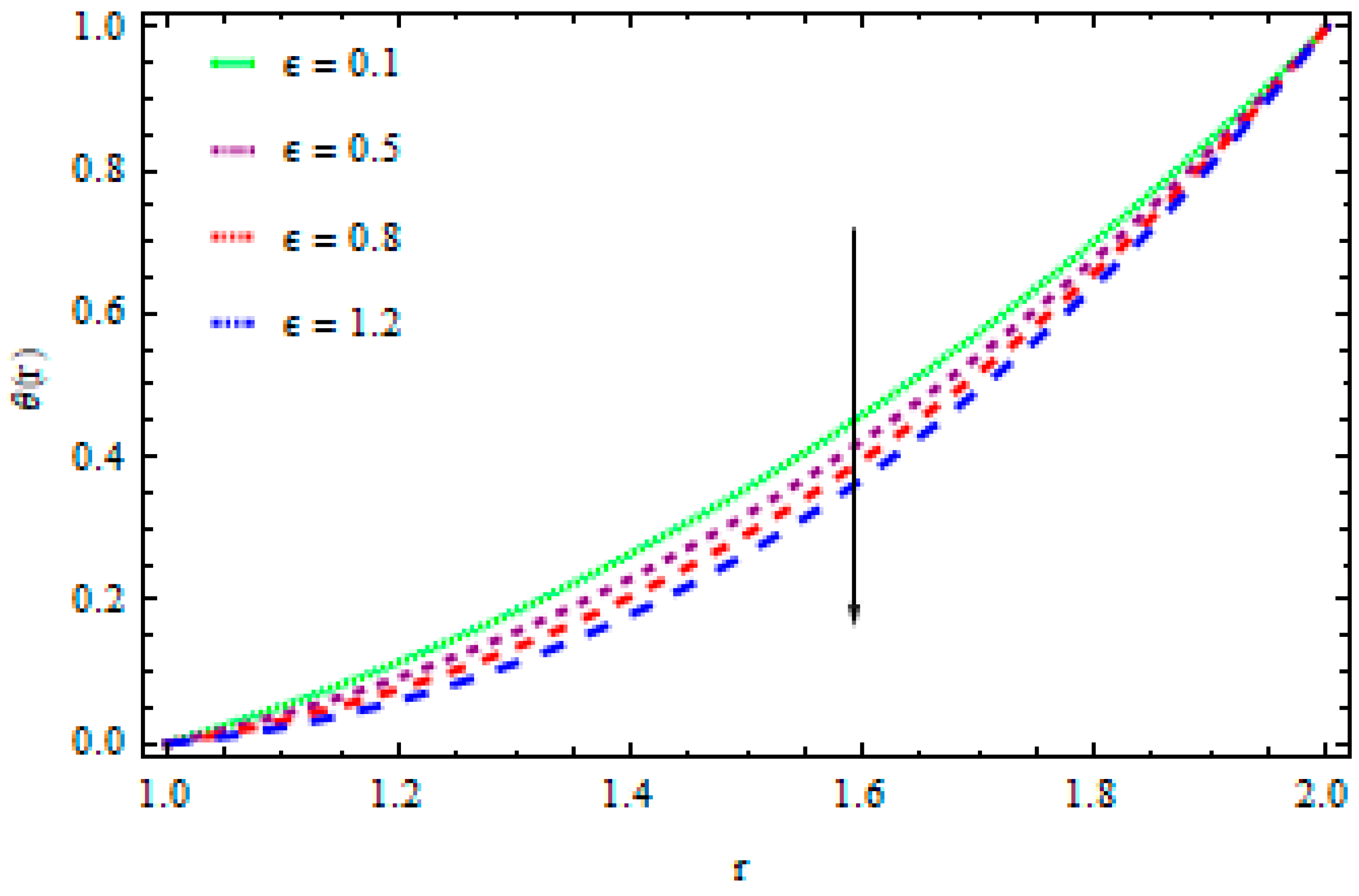
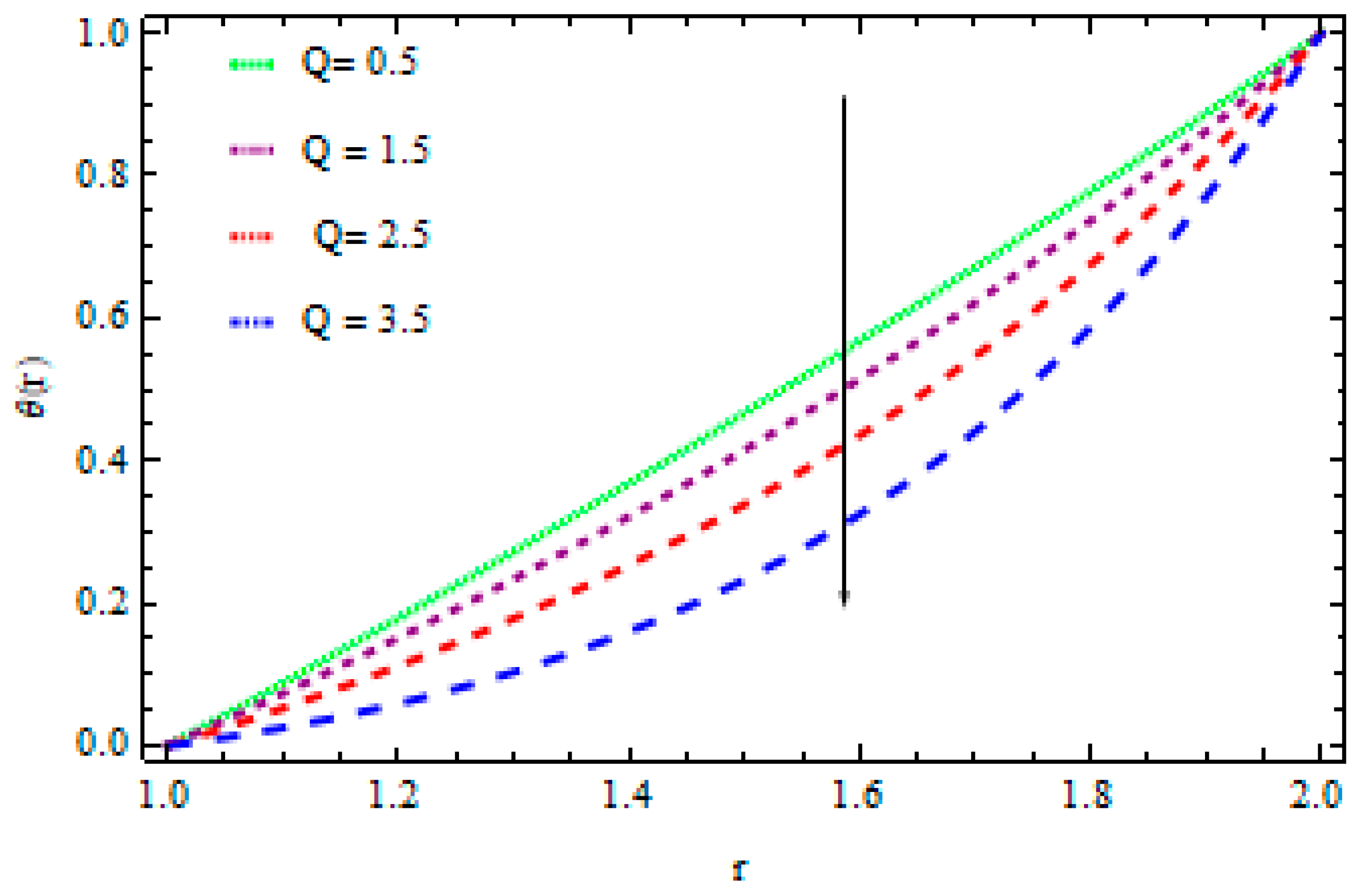
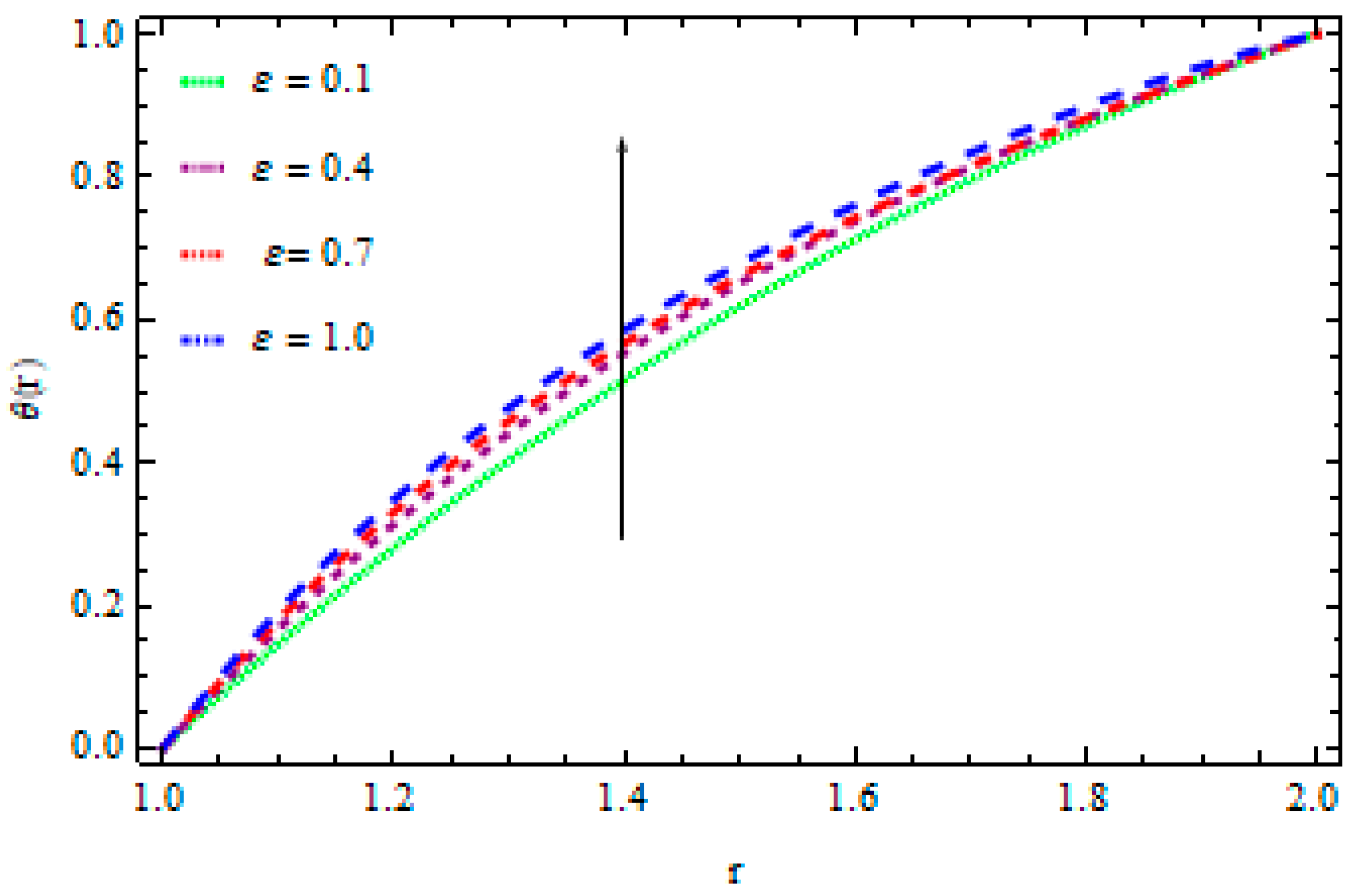
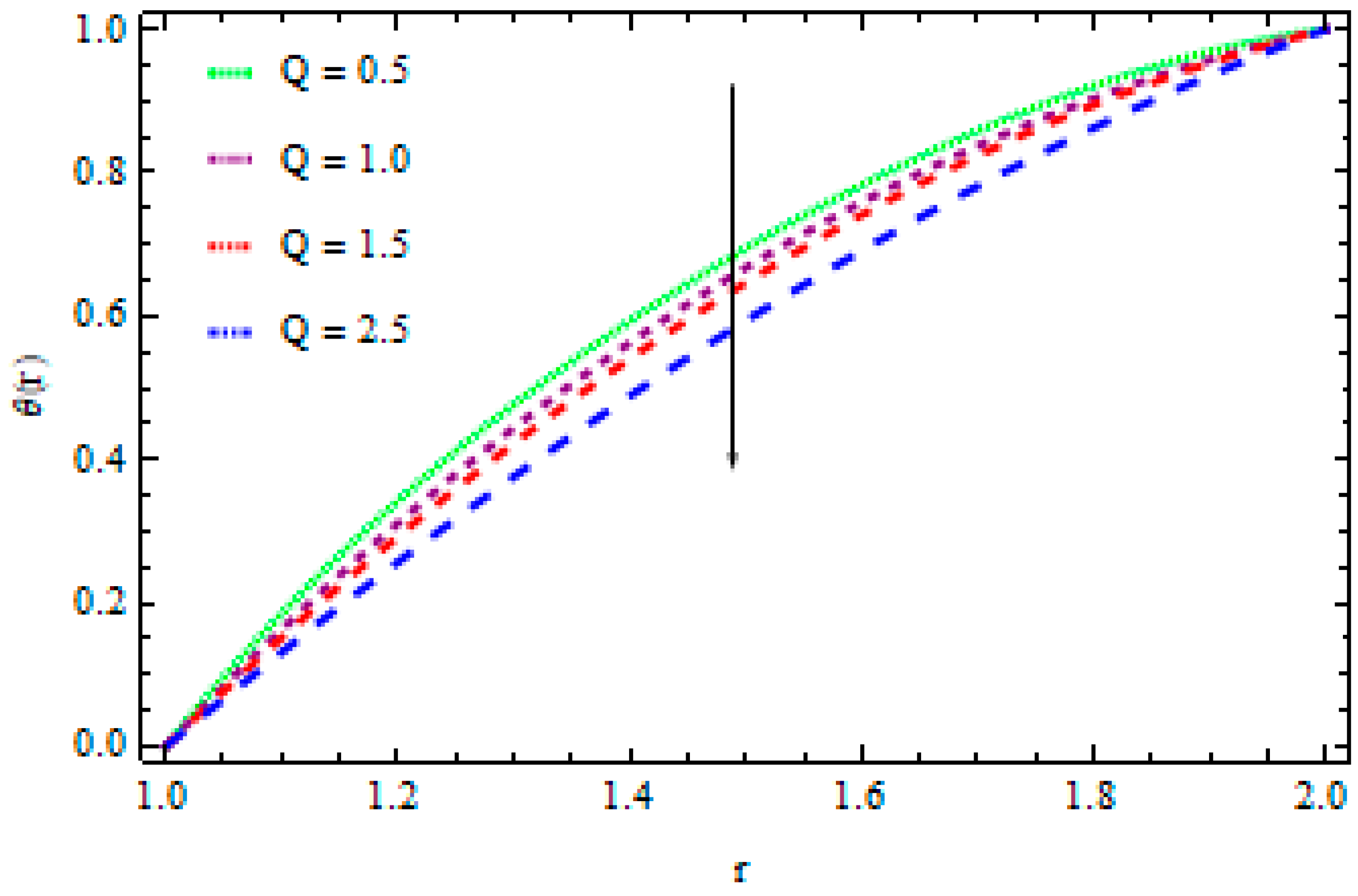
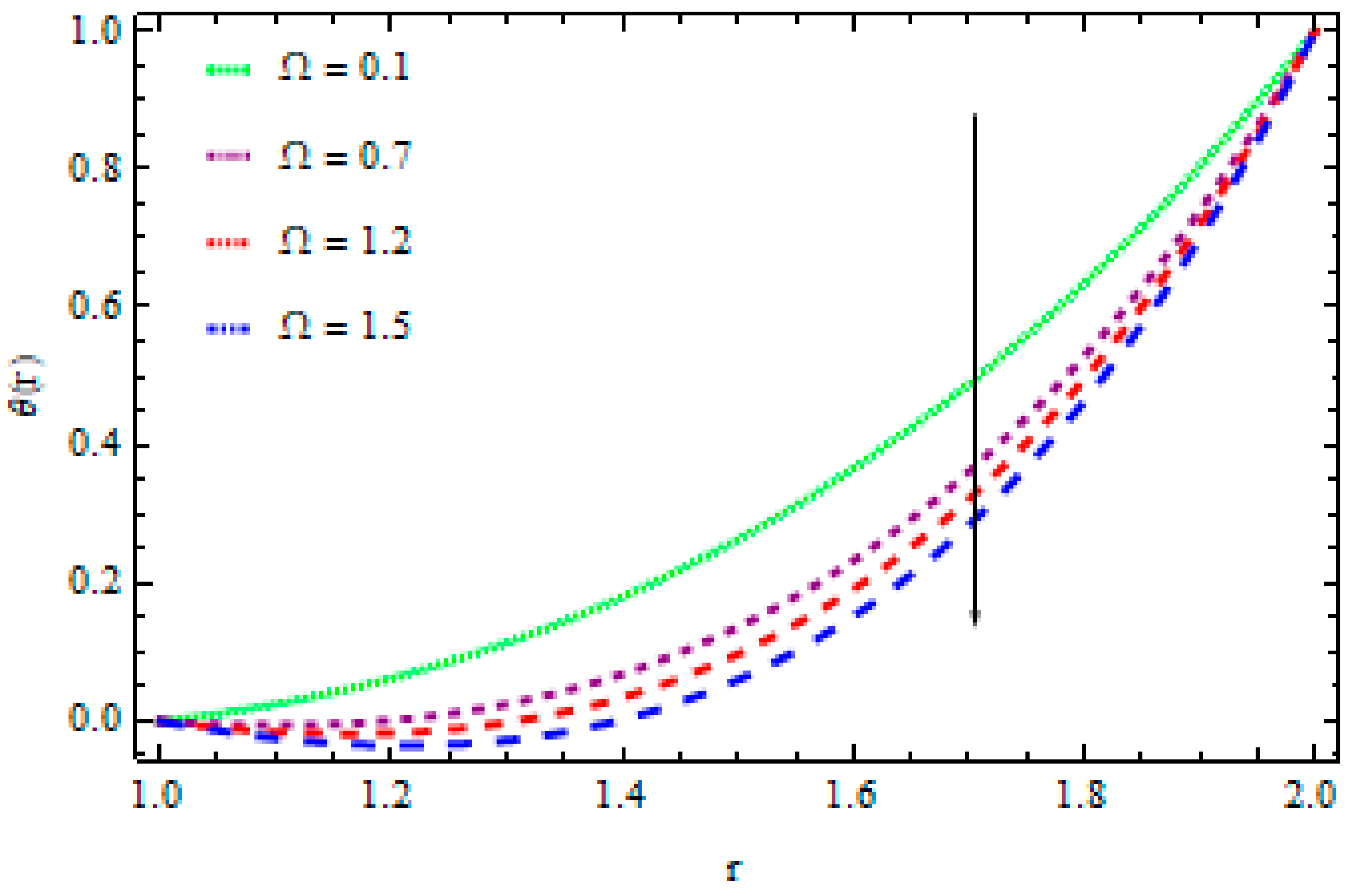
6. Results and Discussion

This paper on wire coating considers the Eyring–Powell fluid. The method of coating a wire takes place in a die with a constant magnetic field and thermal generation effects in permeable media. Different measurable emerging parameters on velocity and temperature profiles are explained through graphs, including the non-Newtonian parameter ${\beta }_0,$ viscosity parameter $m,$ thermal generation parameters $Q$ and $\mathsf{\Omega }$ for Reynolds’ and Vogel’s models respectively, permeable medium parameter $K_p$, Brinkman number $B_r$ and the other parameter $D$. In Figure 2, the geometry of the problem is explained. Figure 2 displays the Brinkman number $B_r$ over velocity distribution for the Reynolds’ model, and the velocity profile shows an increasing behavior as $B_r$ increases. In Figure 3, the results of the permeable medium parameter $K_p$ on the velocity distribution for Reynolds’ model were investigated when $Q=0.1,{\beta }_0=0.1,m=0.3\mathrm{and}{B}_r=0.1.$ From this, the velocity profile decreases with increasing values of $K_p$. By increasing the value of $N$, the velocity curve shows a decreasing behavior, as predicted in Figure 4 for Reynolds’ model. In Figure 5, it is observed that by increasing the Brinkman number $B_r$ for Vogel’s model, the velocity profile increases while keeping some parameters fixed. Figure 6 represents the velocity profile for different values of $D$. The velocity profiles increase with increasing $D$. In Figure 7, it is shown that taking $D=0.2,B_r=0.2$, the velocity profile rises with increasing $Q$ for Vogel’s model. Figure 8 describes the inequality in temperature profile as a result of ε for uniform viscosity while keeping other parameters fixed. The velocity profile decreases as the value of $\epsilon$ increases. Figure 9 illustrates the output of B_r on temperature distribution for uniform viscosity. When the value of $B_r$ increases, the velocity profile decreases. In Figure 10, the impact of $Q$ on the temperature profile was observed, while keeping the viscosity and other parameters constant. Increasing the value of $Q$ causes an increase in the velocity profile. In Figure 11 it is observed that the temperature curve increases by increasing $\epsilon$ for Reynolds’ model. A clear decline in the behavior of the velocity profile curve can be seen in Figure 12 as $Q$ increases. In Figure 13, a decreasing behavior in temperature profile is observed by increasing Ω for Vogel’s model, keeping $N=0.2,B^{\prime }=1.3,K_p=0.1\mathrm{and}D=0.3.$

7. Concluding Remarks

In this article we investigated the effect of pertinent parameters such as hydromagnetic stream movement and heat transmission in a wire coating process using liquid polymer in a permeable medium along with a Joule heating effect and changeable viscosity. The wire is layered into a pressure-type die in order to interact with an Eyring–Powell liquid. A permeable matrix is used as a dielectric in order to enhance the heating/cooling process and to reduce the Joule heating effect during the flow and heat conduction process. The result is derived from the fourth-order Runge–Kutta method and sketched onto velocity and temperature profiles. The consequences are also verified by using the semi-numerical method techniques HAM and BVPh2. From these methods, a good agreement is found. The significant outcomes of the analysis presented in this research work are given below:

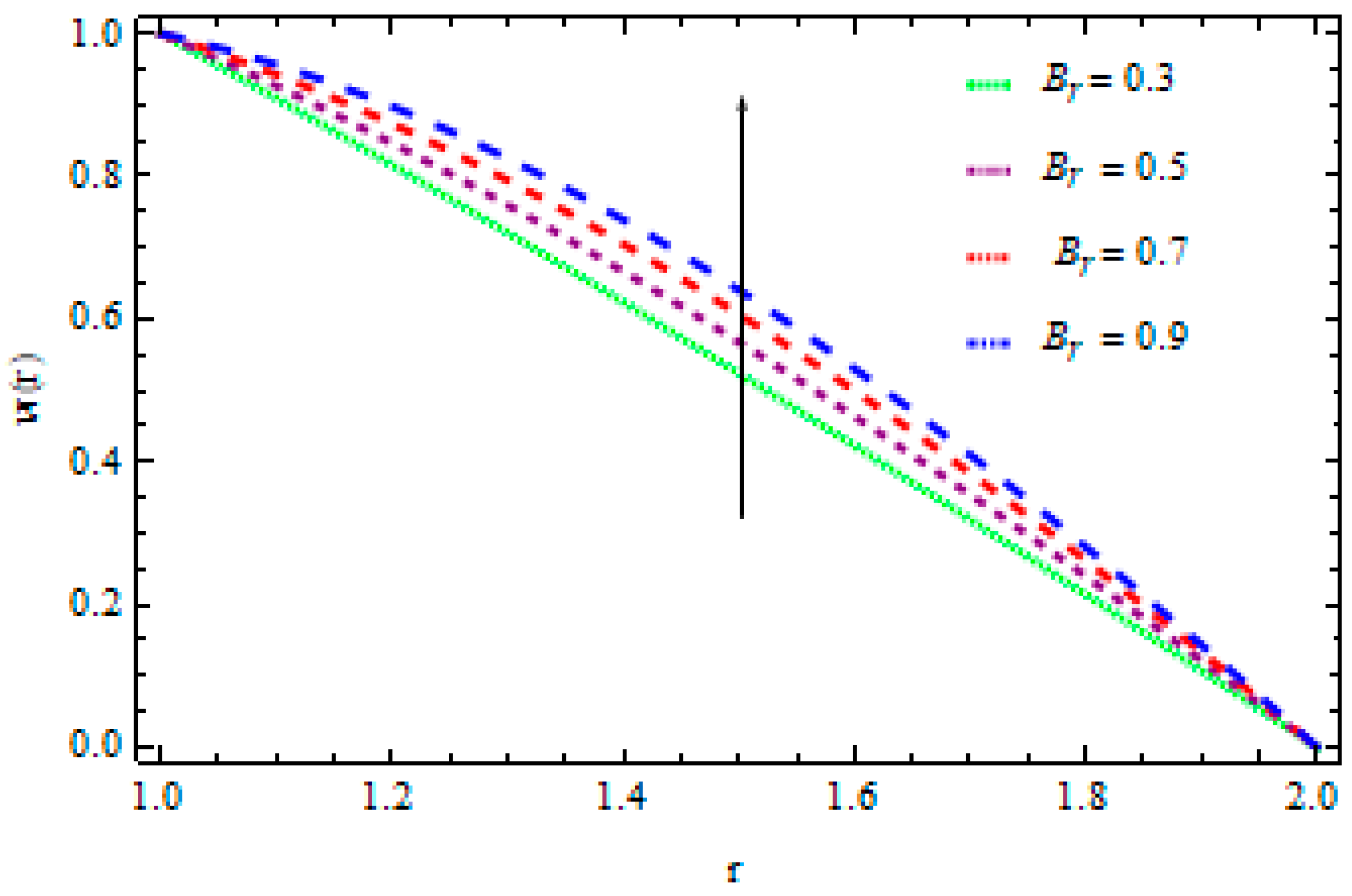
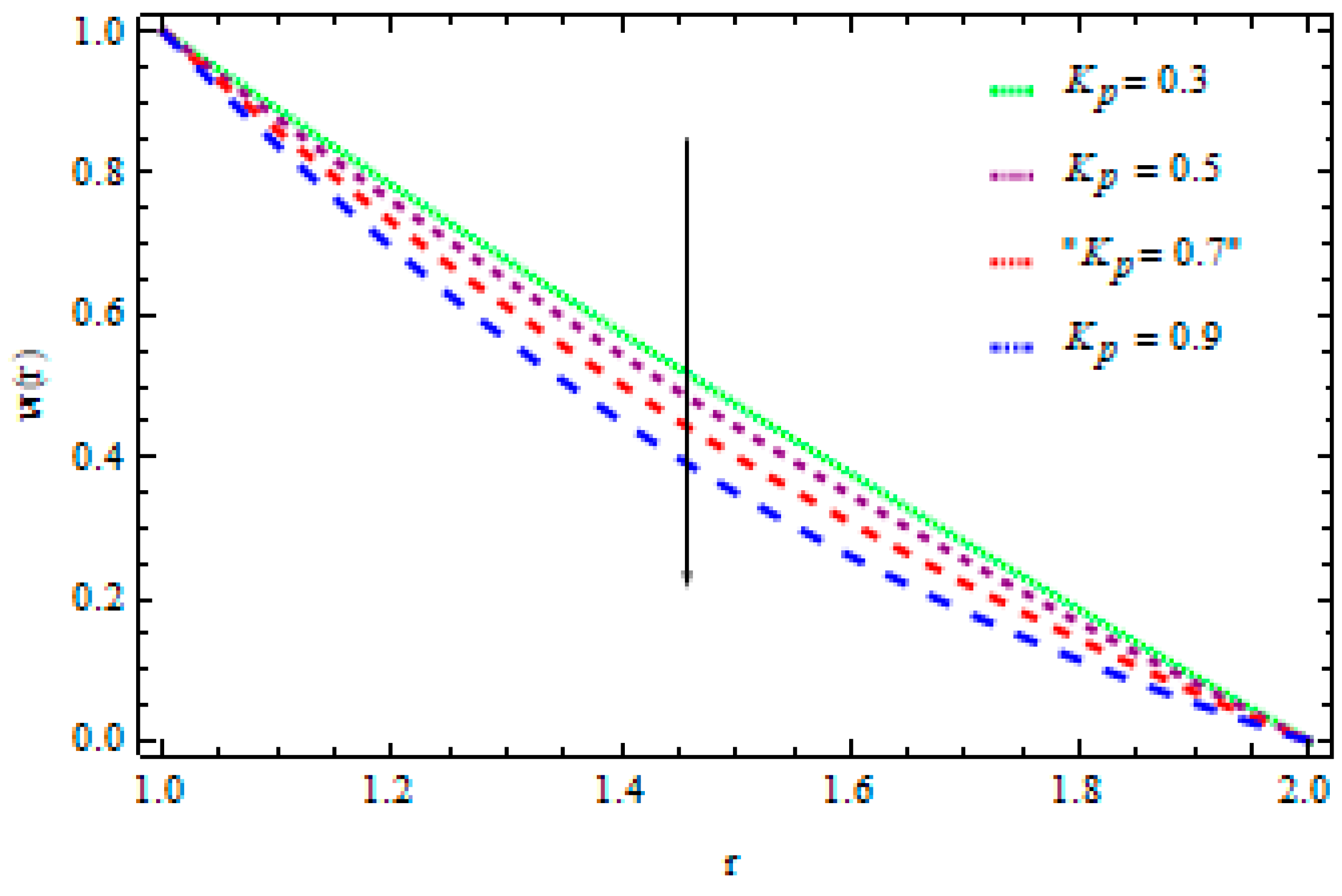
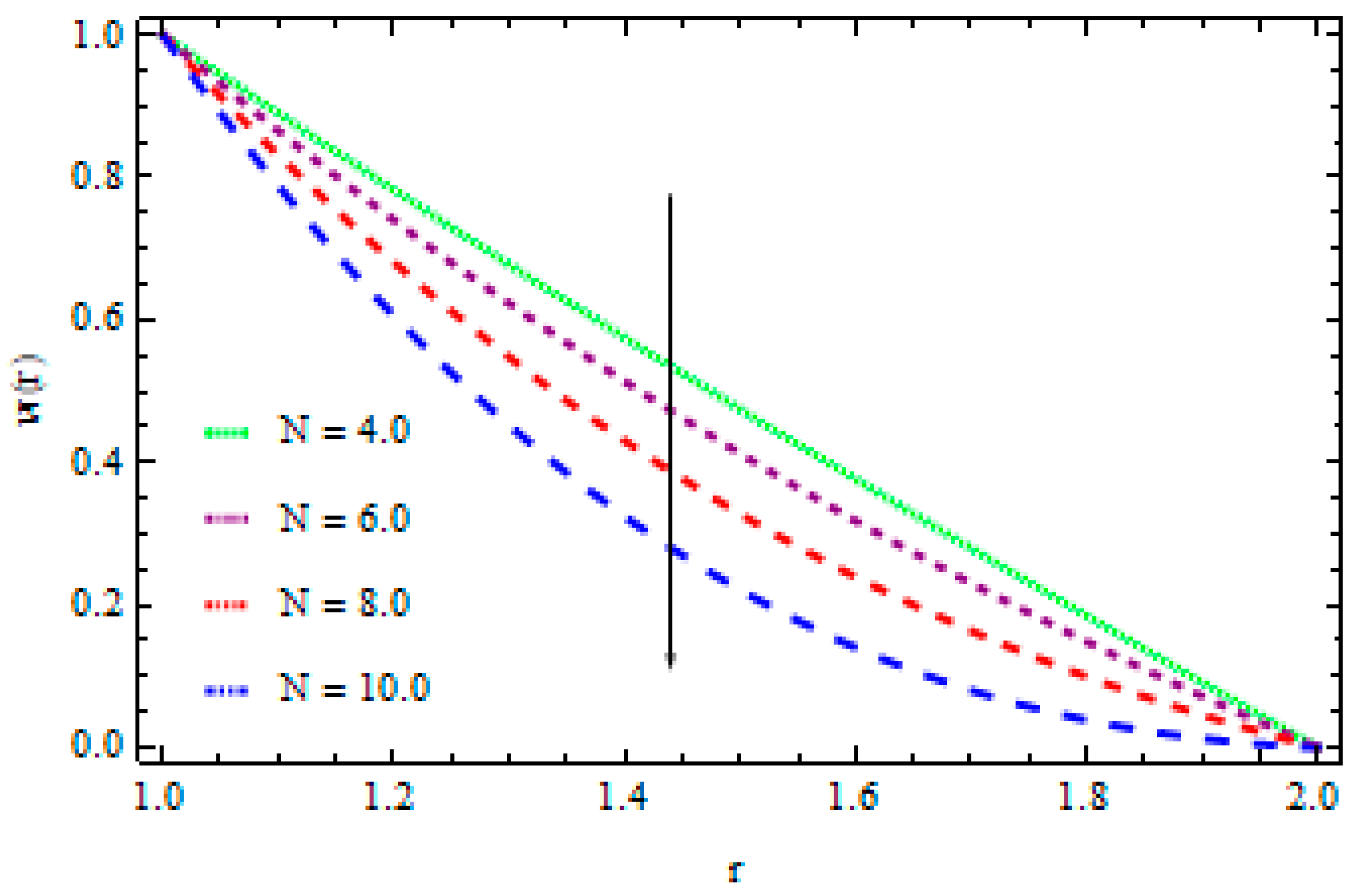
• An increase in fluid velocity behavior occurs as the values of ${\mathrm{B}}_r,M,N,D,\mathrm{and}\epsilon$ increase, and a decrease in fluid velocity behavior occurs as the values of $Q,\epsilon \mathrm{and}{K}_p$ increase.
• The temperature distribution displays an increasing effect as the values of $M\mathrm{and}\epsilon$ increase, while it shows a decreasing effect when the values of $Q,\epsilon \mathrm{and}{B}_r$ increase.