Analysing the Initial Bacterial Adhesion to Evaluate the Performance of Antifouling Surfaces
Abstract
1. Introduction
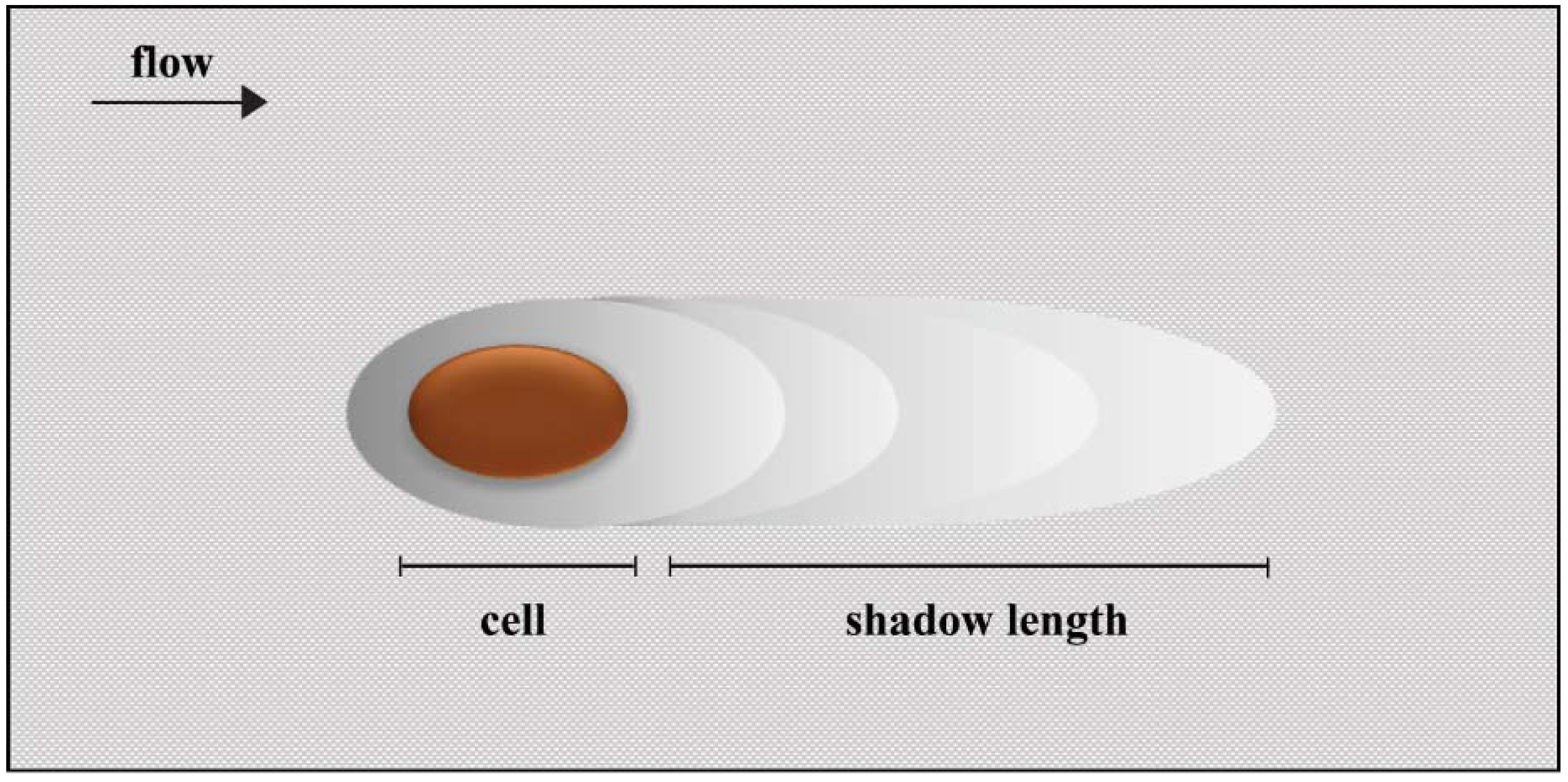
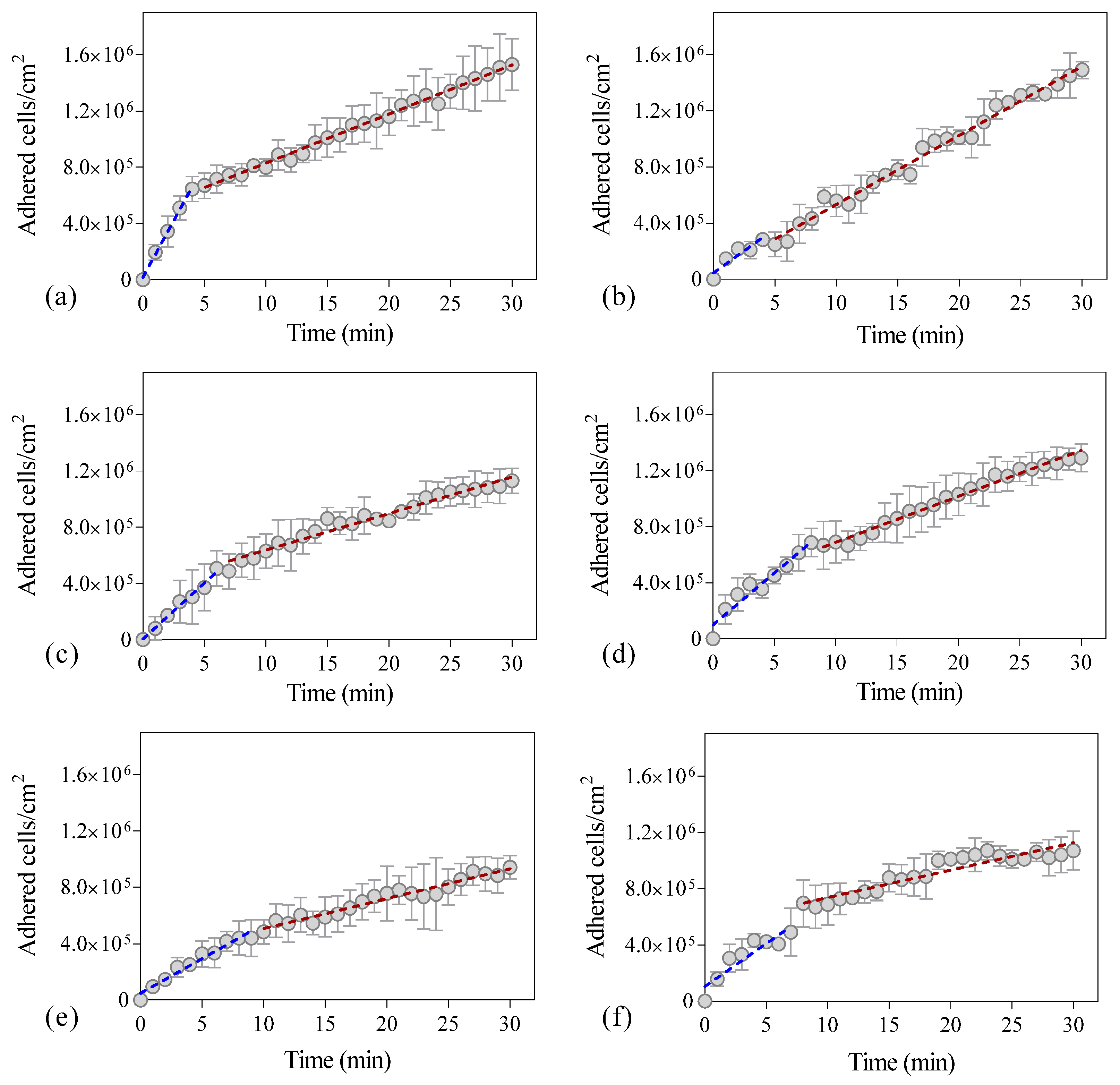
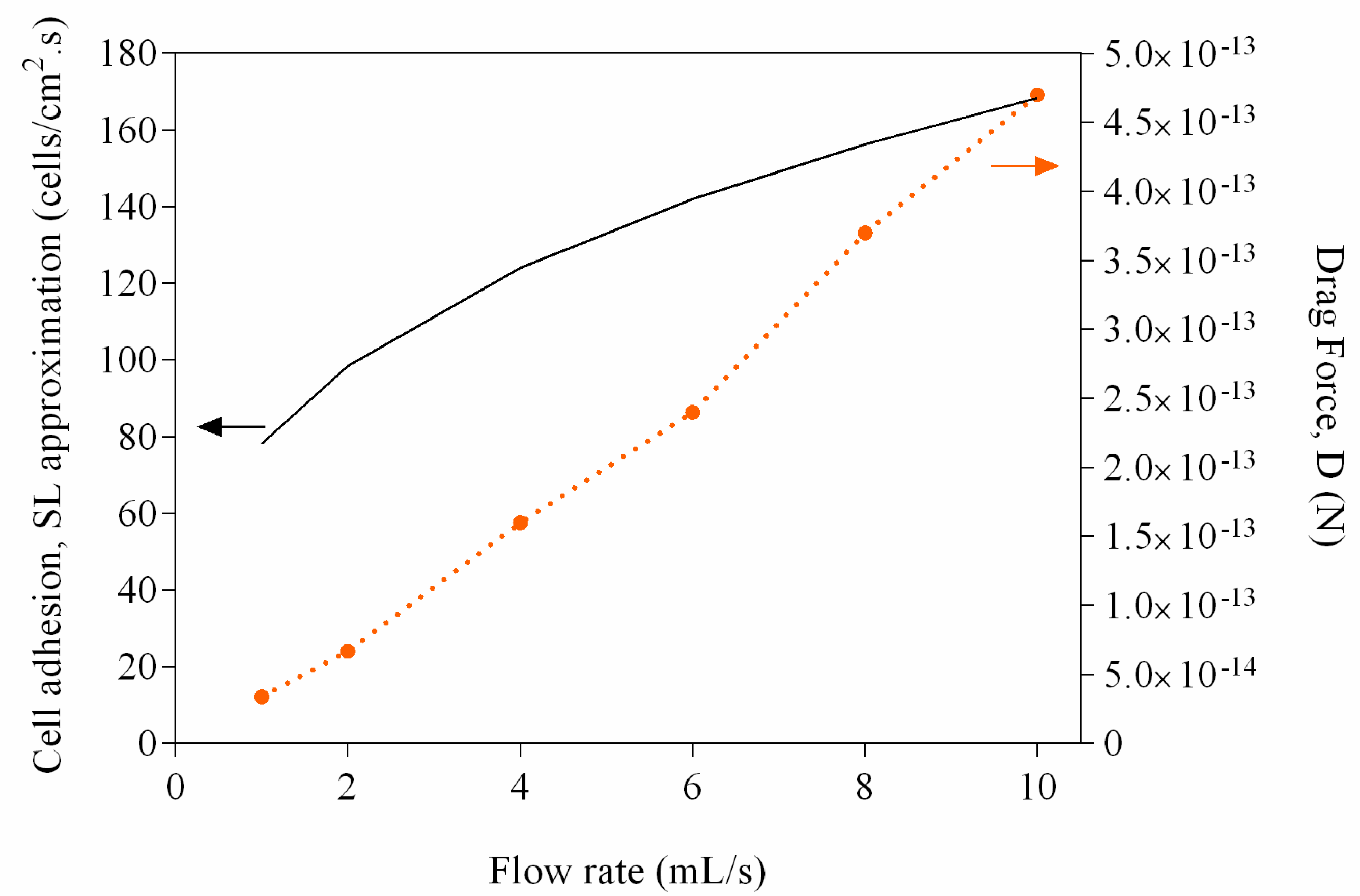
2. Results and Discussion
3. Materials and Methods
3.1. Bacteria and Culture Conditions
3.2. Surface Preparation and Experimental Setup
3.3. Adhesion Experiments
3.4. Blocking Analysis
3.5. Mass Transfer and Drag Force
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Gusnaniar, N.; van der Mei, H.C.; Qu, W.; Nuryastuti, T.; Hooymans, J.M.M.; Sjollema, J.; Busscher, H.J. Physico-chemistry of bacterial transmission versus adhesion. Adv. Colloid Interface Sci. 2017, 250, 15–24. [Google Scholar] [CrossRef] [PubMed]
- Costa, A.M.; Mergulhão, F.J.; Briandet, R.; Azevedo, N.F. It is all about location: How to pinpoint microorganisms and their functions in multispecies biofilms. Future Microbiol. 2017, 12, 987–999. [Google Scholar] [CrossRef] [PubMed]
- Brooks, J.D.; Flint, S.H. Biofilms in the food industry: Problems and potential solutions. Int. J. Food Sci. Technol. 2008, 43, 2163–2176. [Google Scholar] [CrossRef]
- Miquel, S.; Lagrafeuille, R.; Souweine, B.; Forestier, C. Anti-biofilm activity as a health issue. Front. Microbiol. 2016, 7, 592. [Google Scholar] [CrossRef] [PubMed]
- Soares, A.; Azevedo, A.; Gomes, L.C.; Mergulhão, F.J. Recombinant protein expression in biofilms. AIMS Microbiol. 2019, 5, 232–250. [Google Scholar] [CrossRef]
- Soares, A.; Gomes, L.C.; Mergulhão, F.J. Comparing the Recombinant Protein Production Potential of Planktonic and Biofilm Cells. Microorganisms 2018, 6, 48. [Google Scholar] [CrossRef]
- Moreira, J.; Gomes, L.; Whitehead, K.; Lynch, S.; Tetlow, L.; Mergulhão, F. Effect of surface conditioning with cellular extracts on Escherichia coli adhesion and initial biofilm formation. Food Bioprod. Process. 2017, 104, 1–12. [Google Scholar] [CrossRef]
- Busscher, H.J.; van der Mei, H.C. Microbial adhesion in flow displacement systems. Clin. Microbiol. Rev. 2006, 19, 127–141. [Google Scholar] [CrossRef] [PubMed]
- Ramstedt, M.; Ribeiro, I.A.C.; Bujdakova, H.; Mergulhão, F.J.M.; Jordao, L.; Thomsen, P.; Alm, M.; Burmølle, M.; Vladkova, T.; Can, F.; et al. Evaluating efficacy of antimicrobial and antifouling materials for urinary tract medical devices: Challenges and recommendations. Macromol. Biosci. 2019, 19, e1800384. [Google Scholar] [CrossRef] [PubMed]
- Lopez-Mila, B.; Alves, P.; Riedel, T.; Dittrich, B.; Mergulhão, F.; Rodriguez-Emmenegger, C. Effect of shear stress on the reduction of bacterial adhesion to antifouling polymers. Bioinspir. Biomim. 2018, 13, 065001. [Google Scholar] [CrossRef] [PubMed]
- Azeredo, J.; Azevedo, N.F.; Briandet, R.; Cerca, N.; Coenye, T.; Costa, A.R.; Desvaux, M.; Di Bonaventura, G.; Hébraud, M.; Jaglic, Z. Critical review on biofilm methods. Crit. Rev. Microbiol. 2017, 43, 313–351. [Google Scholar] [CrossRef]
- van Loenhout, M.T.; Kooij, E.S.; Wormeester, H.; Poelsema, B. Hydrodynamic flow induced anisotropy in colloid adsorption. Colloids Surf. A Physicochem. Eng. Asp. 2009, 342, 46–52. [Google Scholar] [CrossRef]
- Meinders, J.; Noordmans, J.; Busscher, H. Simultaneous monitoring of the adsorption and desorption of colloidal particles during deposition in a parallel plate flow chamber. J. Colloid Interface Sci. 1992, 152, 265–280. [Google Scholar] [CrossRef][Green Version]
- Dabroś, T.; Van de Ven, T. Kinetics of coating by colloidal particles. J. Colloid Interface Sci. 1982, 89, 232–244. [Google Scholar] [CrossRef]
- Adamczyk, Z. Modeling adsorption of colloids and proteins. Curr. Opin. Colloid Interface Sci. 2012, 17, 173–186. [Google Scholar] [CrossRef]
- Adamczyk, Z.; Warszyński, P.; Szyk-Warszyńska, L.; Weroński, P. Role of convection in particle deposition at solid surfaces. Colloids Surf. A Physicochem. Eng. Asp. 2000, 165, 157–187. [Google Scholar] [CrossRef]
- Adamczyk, Z. Particle adsorption and deposition: Role of electrostatic interactions. Adv. Colloid Interface Sci. 2003, 100, 267–347. [Google Scholar] [CrossRef]
- Alves, P.; Gomes, L.C.; Vorobii, M.; Rodriguez-Emmenegger, C.; Mergulhão, F.J. The potential advantages of using a poly(HPMA) brush in urinary catheters: Effects on biofilm cells and architecture. Colloids Surf. B Biointerfaces 2020, 191, 110976. [Google Scholar] [CrossRef] [PubMed]
- Carlsen, P.N. Polydimethylsiloxane: Structure and Applications; Nova Science Publishers, Inc.: New York, NY, USA, 2020. [Google Scholar]
- Moreira, J.; Araújo, J.; Miranda, J.M.; Simões, M.; Melo, L.; Mergulhão, F. The effects of surface properties on Escherichia coli adhesion are modulated by shear stress. Colloids Surf. B Biointerfaces 2014, 123, 1–7. [Google Scholar] [CrossRef]
- Gomes, L.; Moreira, J.; Teodósio, J.; Araújo, J.; Miranda, J.; Simões, M.; Melo, L.; Mergulhão, F. 96-well microtiter plates for biofouling simulation in biomedical settings. Biofouling 2014, 30, 535–546. [Google Scholar] [CrossRef]
- Van der Mei, W.N.; Krom, B.; Sjollema, J. Analysis of the contribution of sedimentation to bacterial mass transport in a parallel plate flow chamber Part II: Use of fluorescence imaging. Colloids Surf. B Biointerfaces 2011, 87, 427–432. [Google Scholar] [CrossRef]
- Sjollema, J.; Busscher, H. Deposition of polystyrene particles in a parallel plate flow cell. 2. Pair distribution functions between deposited particles. Colloids Surf. 1990, 47, 337–352. [Google Scholar] [CrossRef]
- Dabros, T. Interparticle hydrodynamic interactions in deposition processes. Colloids Surf. 1989, 39, 127–141. [Google Scholar] [CrossRef]
- Lecuyer, S.; Rusconi, R.; Shen, Y.; Forsyth, A.; Vlamakis, H.; Kolter, R.; Stone, H.A. Shear stress increases the residence time of adhesion of Pseudomonas aeruginosa. Biophys. J. 2011, 100, 341–350. [Google Scholar] [CrossRef]
- Dickinson, R.B.; Cooper, S.L. Analysis of shear-dependent bacterial adhesion kinetics to biomaterial surfaces. AIChE J. 1995, 41, 2160–2174. [Google Scholar] [CrossRef]
- Nejadnik, M.R.; van der Mei, H.C.; Busscher, H.J.; Norde, W. Determination of the shear force at the balance between bacterial attachment and detachment in weak-adherence systems, using a flow displacement chamber. Appl. Environ. Microbiol. 2008, 74, 916. [Google Scholar] [CrossRef]
- Marshall, B.T.; Sarangapani, K.K.; Lou, J.; McEver, R.P.; Zhu, C. Force history dependence of receptor-ligand dissociation. Biophys. J. 2005, 88, 1458–1466. [Google Scholar] [CrossRef]
- Xu, L.C.; Vadillo-Rodriguez, V.; Logan, B.E. Residence time, loading force, pH, and ionic strength affect adhesion forces between colloids and biopolymer-coated surfaces. Langmuir 2005, 21, 7491–7500. [Google Scholar] [CrossRef]
- Garrett, T.R.; Bhakoo, M.; Zhang, Z. Bacterial adhesion and biofilms on surfaces. Prog. Nat. Sci. 2008, 18, 1049–1056. [Google Scholar] [CrossRef]
- Lichter, J.A.; Thompson, M.T.; Delgadillo, M.; Nishikawa, T.; Rubner, M.F.; Van Vliet, K.J. Substrata mechanical stiffness can regulate adhesion of viable bacteria. Biomacromolecules 2008, 9, 1571–1578. [Google Scholar] [CrossRef]
- Alves, P.; Gomes, L.C.; Rodríguez-Emmenegger, C.; Mergulhão, F.J. Efficacy of a poly(MeOEGMA) brush on the prevention of Escherichia coli biofilm formation and susceptibility. Antibiotics 2020, 9, 216. [Google Scholar] [CrossRef] [PubMed]
- Alves, P.; Nir, S.; Reches, M.; Mergulhão, F. The effects of fluid composition and shear conditions on bacterial adhesion to an antifouling peptide-coated surface. MRS Commun. 2018, 8, 938–946. [Google Scholar] [CrossRef]
- Teodósio, J.; Simões, M.; Melo, L.; Mergulhão, F. Flow cell hydrodynamics and their effects on E. coli biofilm formation under different nutrient conditions and turbulent flow. Biofouling 2011, 27, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Simões, M.; Simões, L.C.; Cleto, S.; Pereira, M.O.; Vieira, M.J. The effects of a biocide and a surfactant on the detachment of Pseudomonas fluorescens from glass surfaces. Int. J. Food Microbiol. 2008, 121, 335–341. [Google Scholar] [CrossRef][Green Version]
- Schneider, C.A.; Rasband, W.S.; Eliceiri, K.W. NIH Image to ImageJ: 25 years of image analysis. Nat. Methods 2012, 9, 671. [Google Scholar] [CrossRef]
- Xia, Z.; Woo, L.; van de Ven, T.G. Microrheological aspects of adhesion of Escherichia coli on glass. Biorheology 1989, 26, 359–375. [Google Scholar] [CrossRef] [PubMed]
- Boulbene, B.; Morchain, J.; Bonin, M.M.; Janel, S.; Lafont, F.; Schmitz, P. A combined computational fluid dynamics (CFD) and experimental approach to quantify the adhesion force of bacterial cells attached to a plane surface. AIChE J. 2012, 58, 3614–3624. [Google Scholar] [CrossRef]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alves, P.; Moreira, J.M.; Miranda, J.M.; Mergulhão, F.J. Analysing the Initial Bacterial Adhesion to Evaluate the Performance of Antifouling Surfaces. Antibiotics 2020, 9, 421. https://doi.org/10.3390/antibiotics9070421
Alves P, Moreira JM, Miranda JM, Mergulhão FJ. Analysing the Initial Bacterial Adhesion to Evaluate the Performance of Antifouling Surfaces. Antibiotics. 2020; 9(7):421. https://doi.org/10.3390/antibiotics9070421
Chicago/Turabian StyleAlves, Patrícia, Joana Maria Moreira, João Mário Miranda, and Filipe José Mergulhão. 2020. "Analysing the Initial Bacterial Adhesion to Evaluate the Performance of Antifouling Surfaces" Antibiotics 9, no. 7: 421. https://doi.org/10.3390/antibiotics9070421
APA StyleAlves, P., Moreira, J. M., Miranda, J. M., & Mergulhão, F. J. (2020). Analysing the Initial Bacterial Adhesion to Evaluate the Performance of Antifouling Surfaces. Antibiotics, 9(7), 421. https://doi.org/10.3390/antibiotics9070421





