Evidence of Self-Association and Conformational Change in Nisin Antimicrobial Polypeptide Solutions: A Combined Raman and Ultrasonic Relaxation Spectroscopic and Theoretical Study
Abstract
1. Introduction
2. Results and Discussion
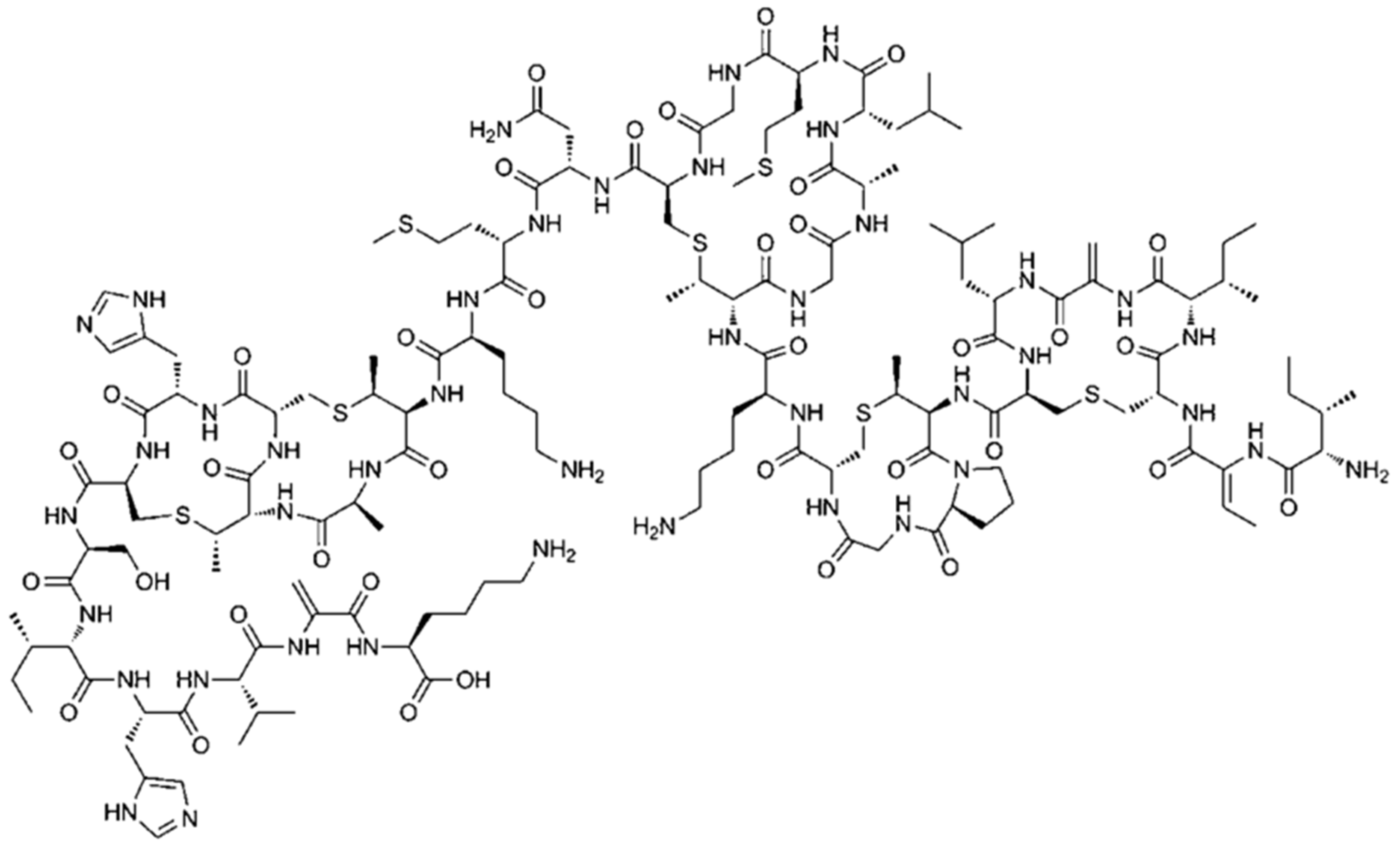
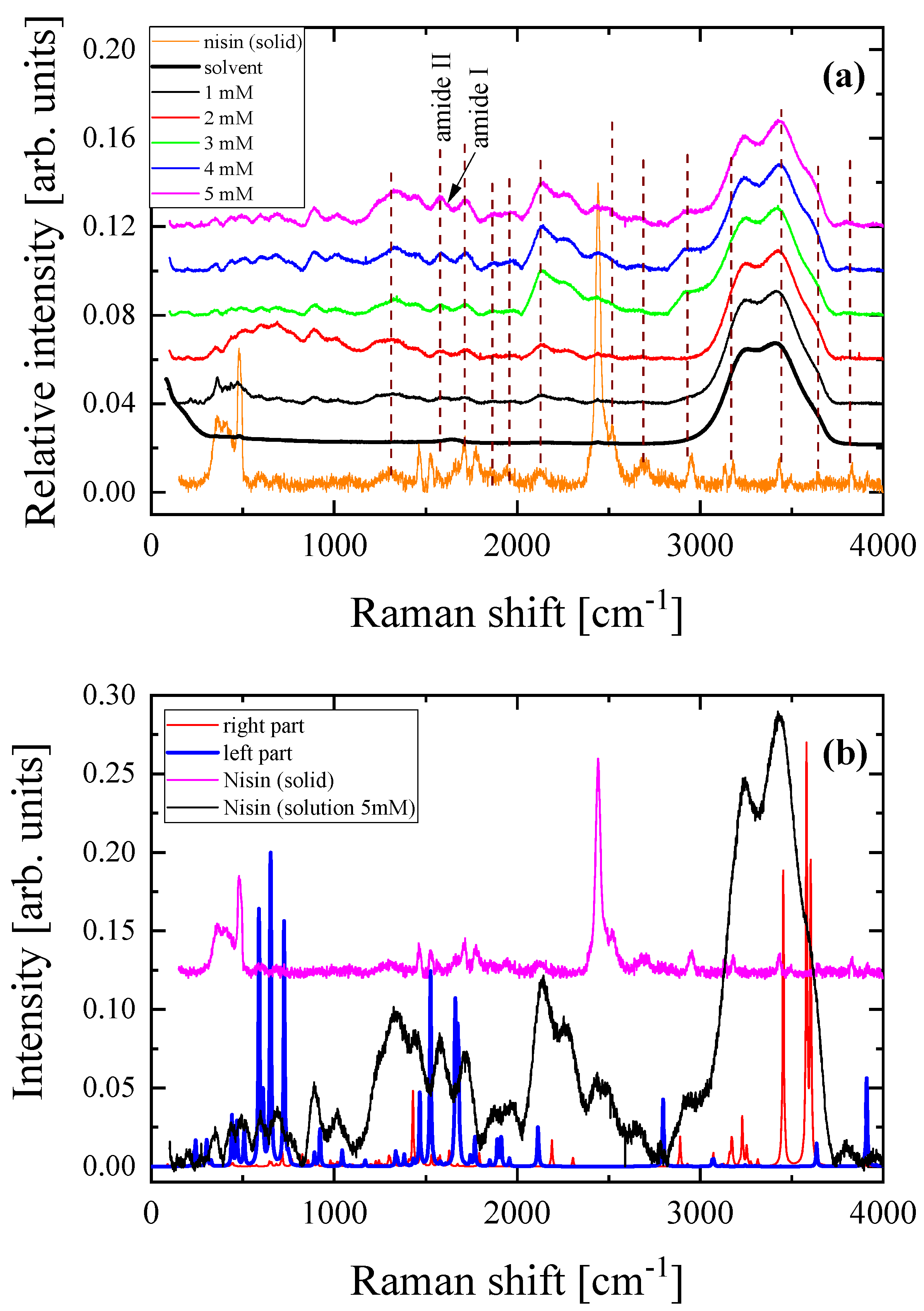
2.1. Dynamic and Structural Processes in Aqueous Nisin Solutions
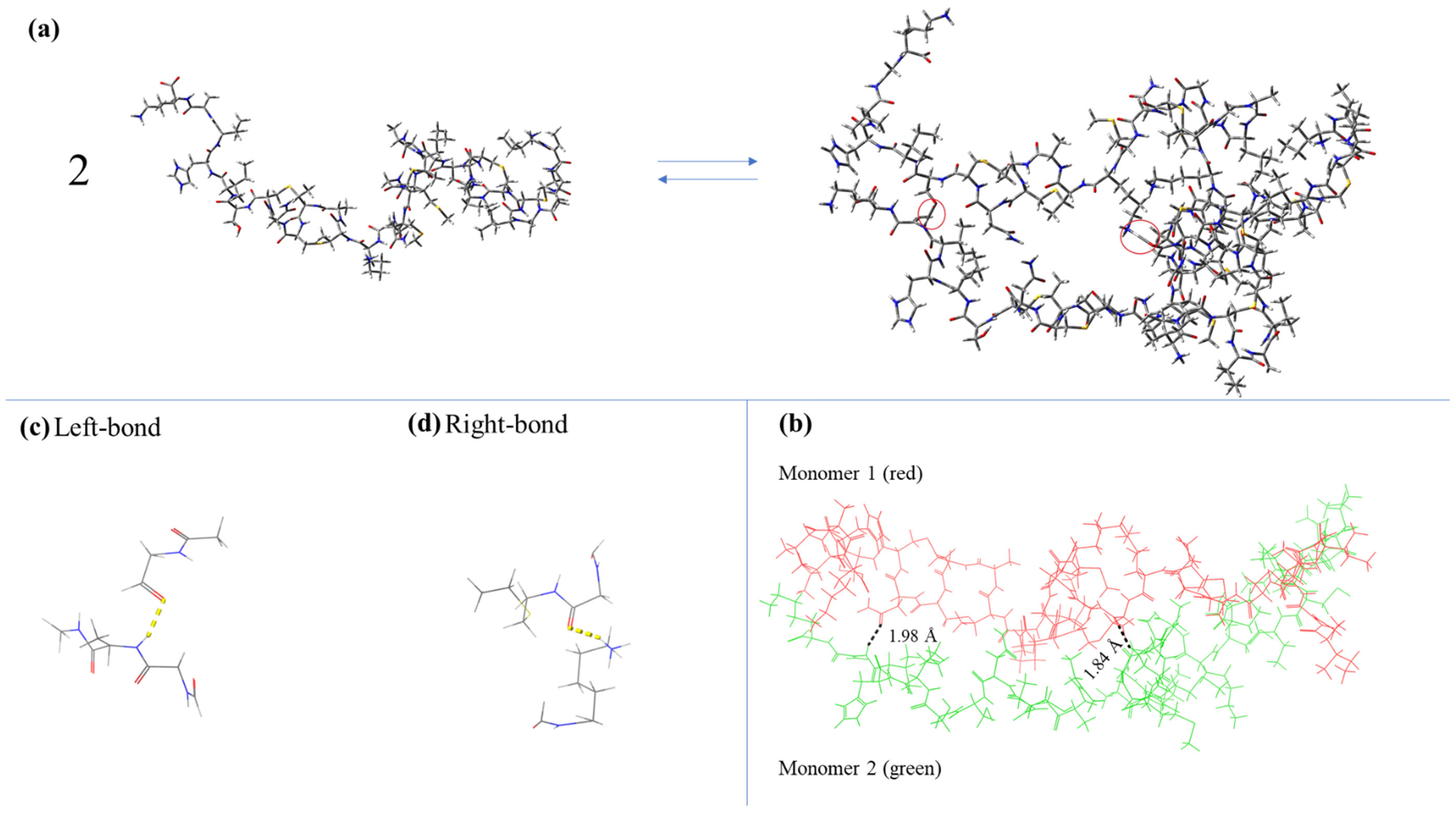
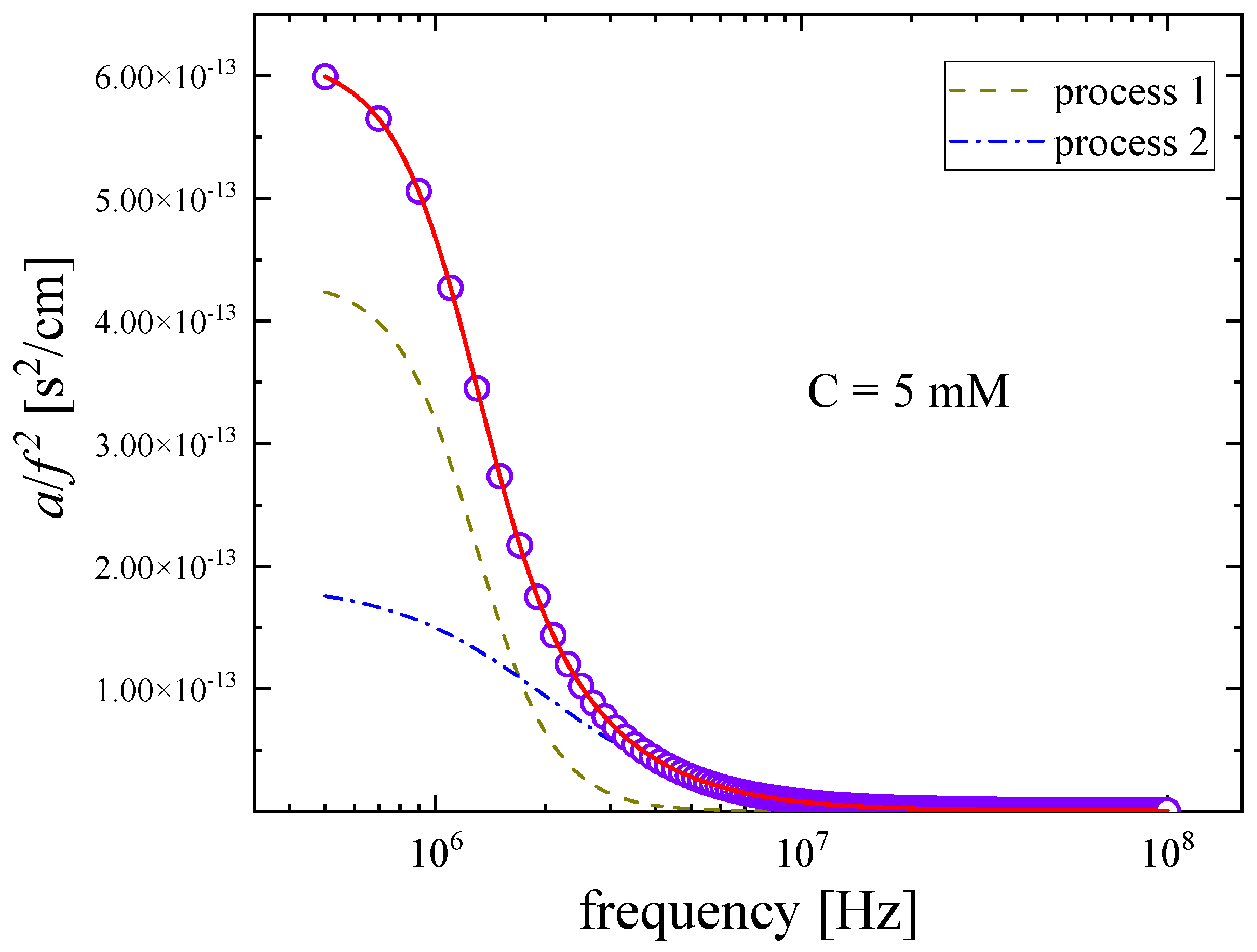
2.2. Elucidation and Assignment of the Observed Structural Mechanisms
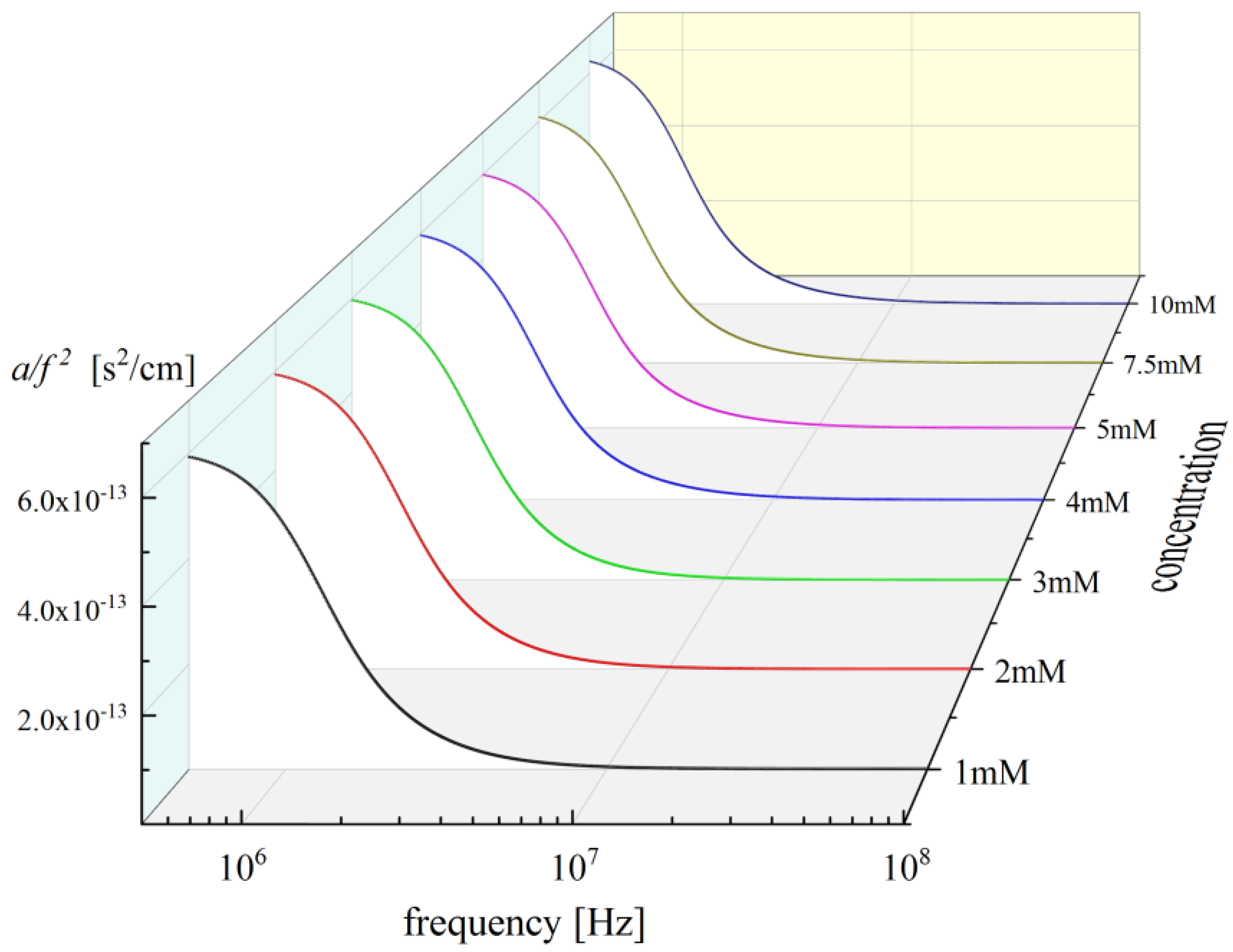
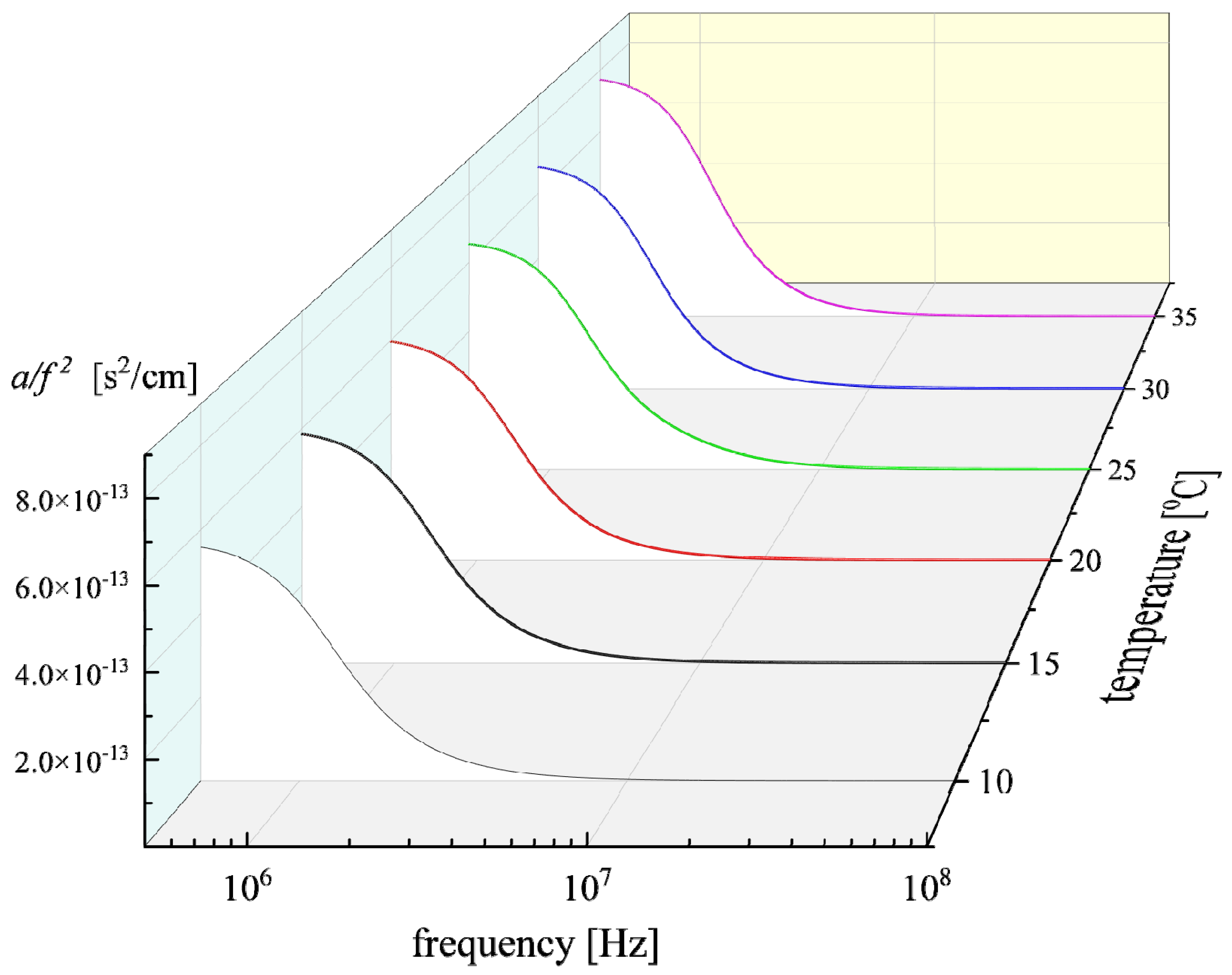
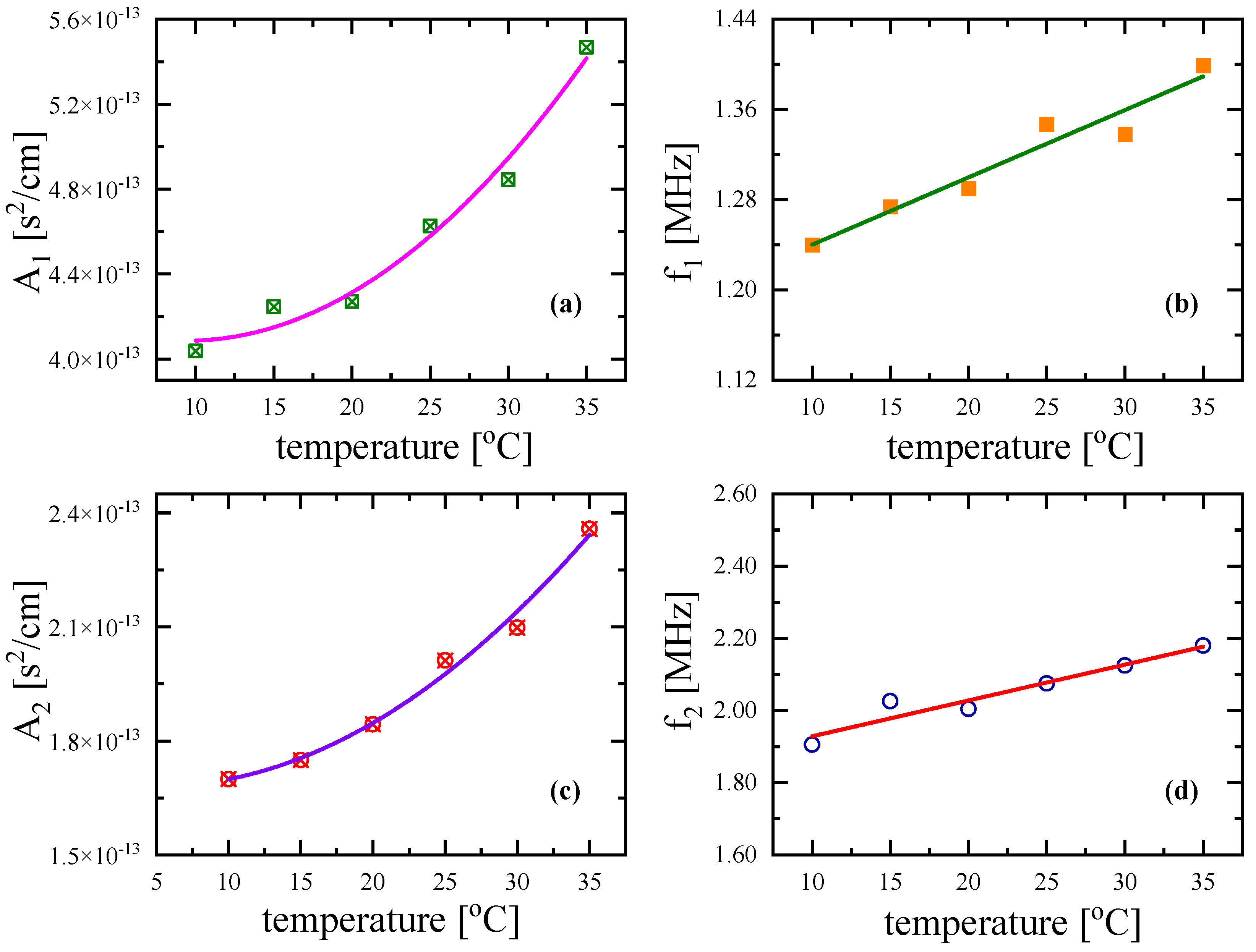
2.3. Effects of Concentration and Temperature on the Relaxation Behaviour
2.4. Kinetic Study of the Self-Aggregation Reaction of Nisin
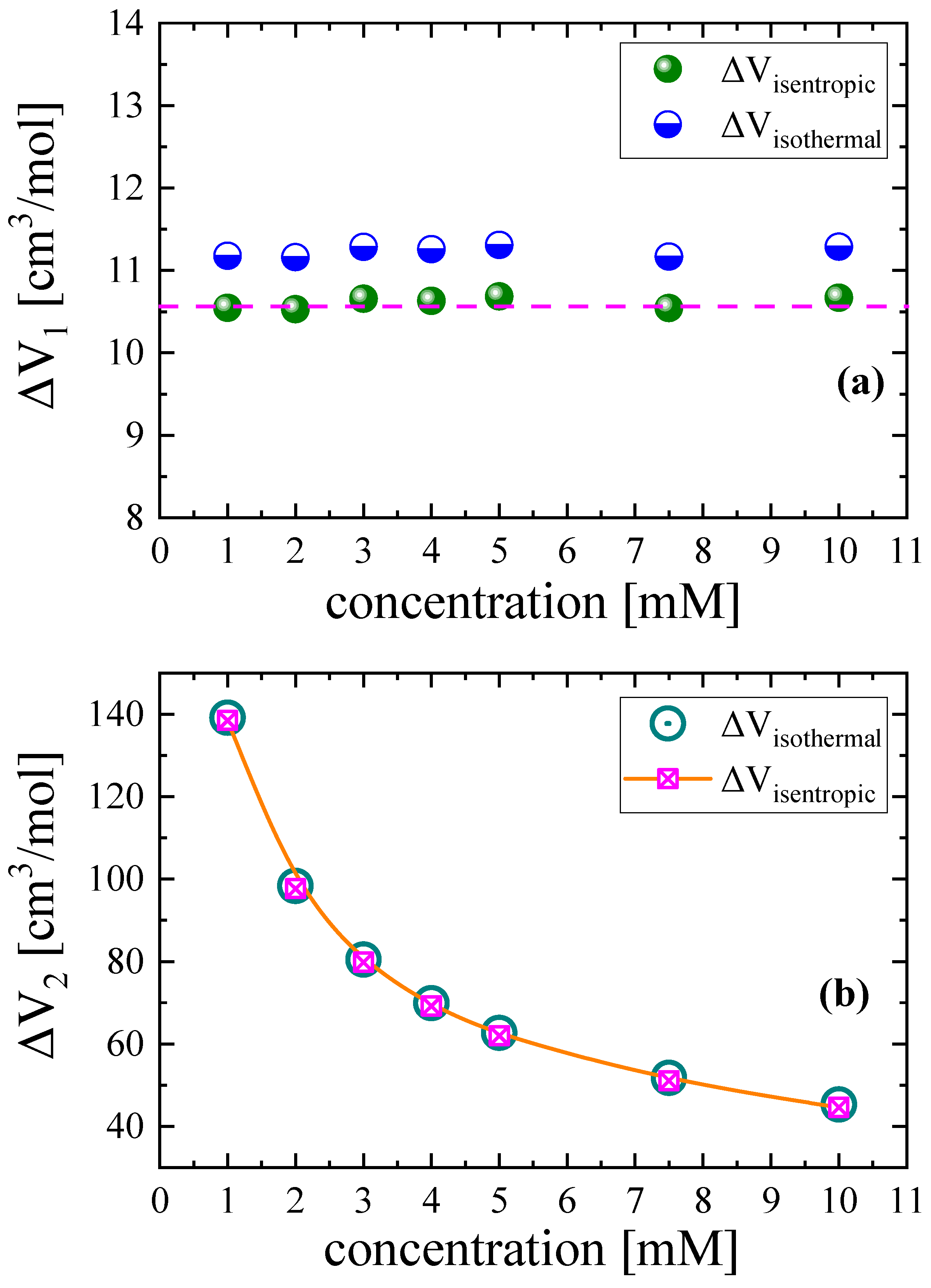
2.5. Volume Changes Accompanying the Relaxation Processes
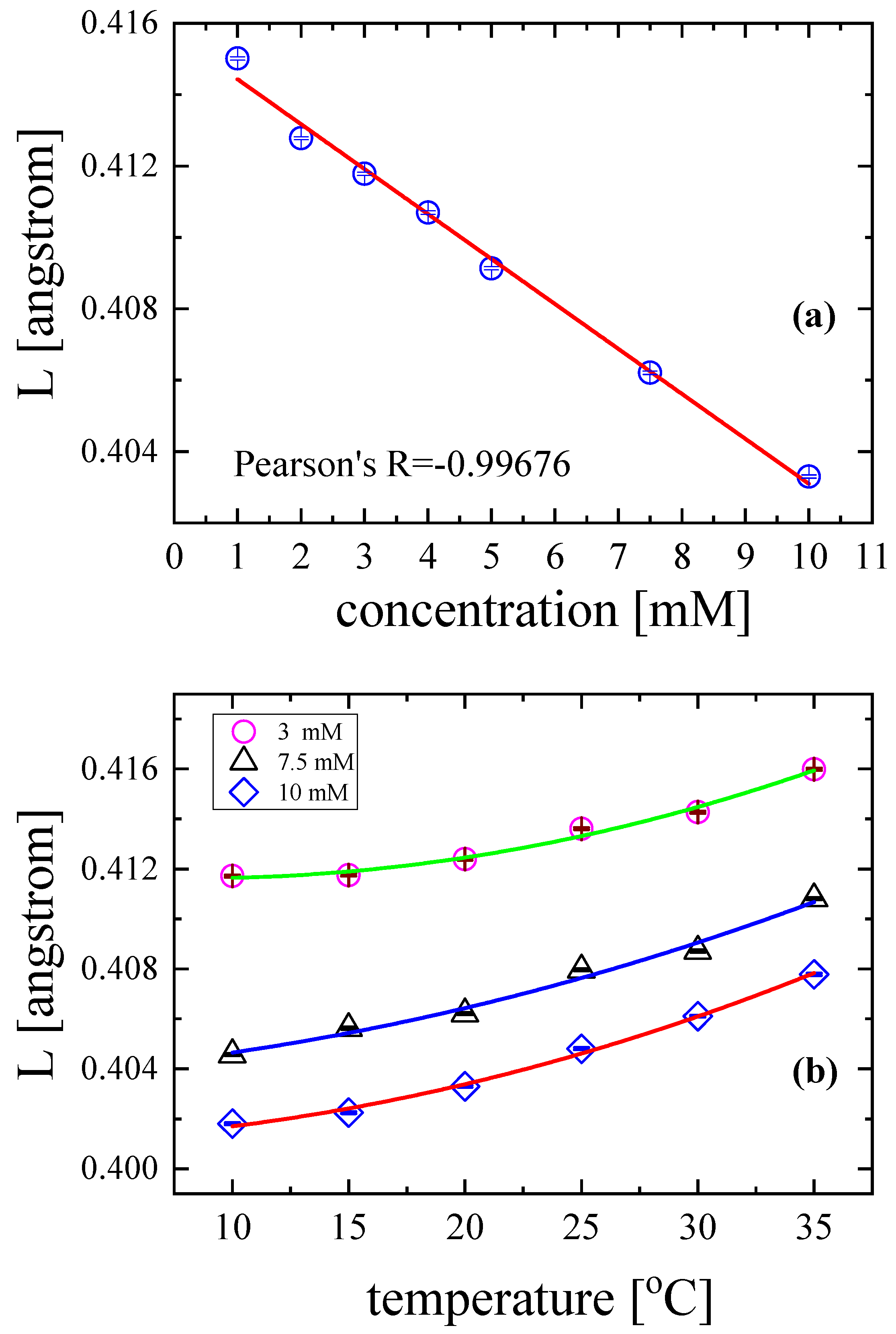
2.6. Intermolecular Free Length of Nisin Solutions
3. Materials and Methods
3.1. Materials
3.2. Raman Spectroscopy
3.3. Ultrasonic Relaxation Spectroscopy
3.4. Computational Details
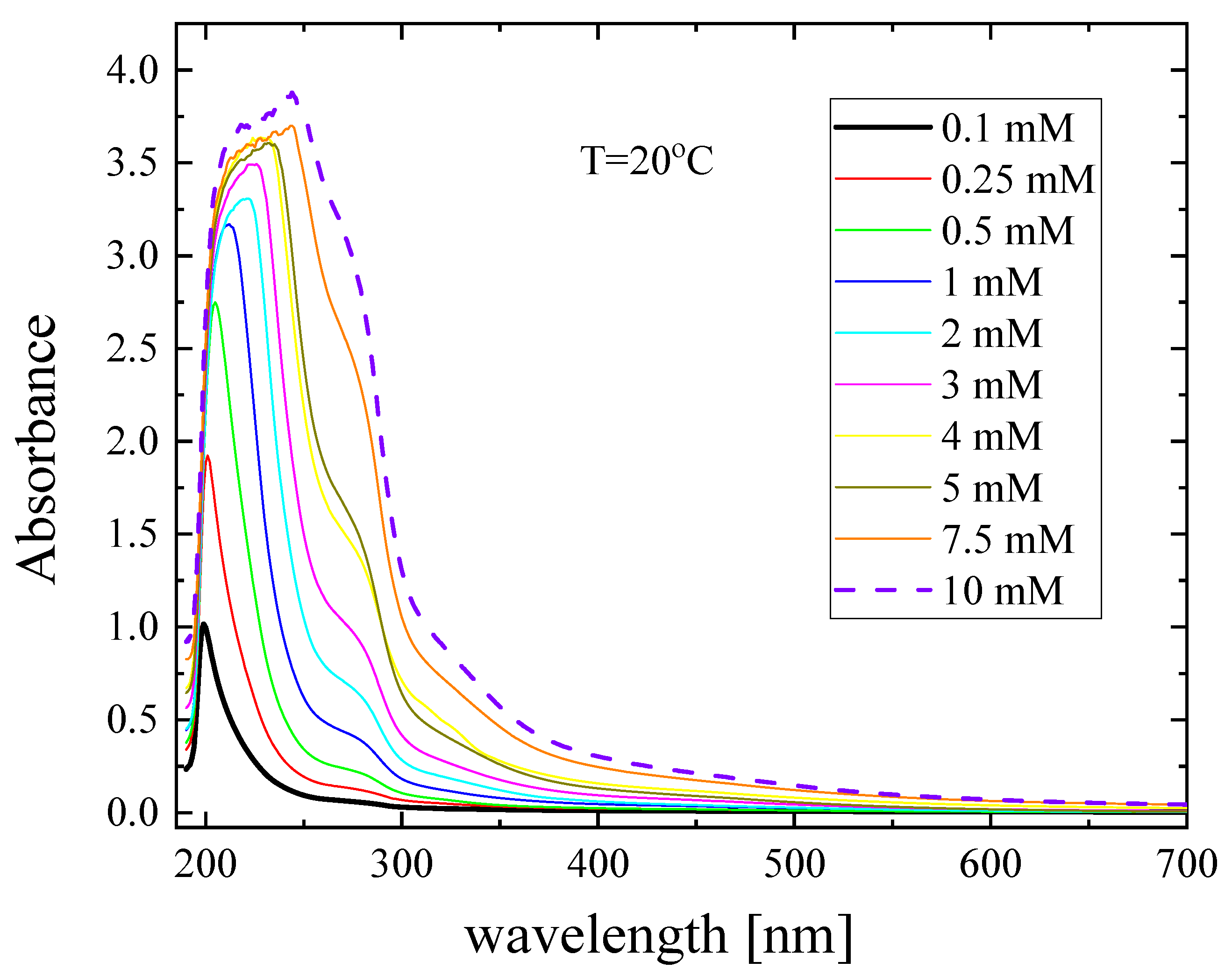
3.5. Additional Measurements
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Avorn, J. Antibiotic Resistance: Synthesis of Recommendations by Expert Policy Groups; World Health Organization: Geneva, Switzerland, 2001. [Google Scholar]
- Cotter, P.D.; Ross, R.P.; Hill, C. Bacteriocins—A viable alternative to antibiotics? Nat. Rev. Microbiol. 2013, 11, 95. [Google Scholar] [CrossRef]
- Buchman, G.W.; Banerjee, S.; Hansen, J.N. Structure, expression, and evolution of a gene encoding the precursor of nisin, a small protein antibiotic. J. Biol. Chem. 1988, 263, 16260. [Google Scholar] [CrossRef]
- Chandrasekar, V.; Knabel, S.J.; Anantheswaran, R.C. Effects of Talinum triangulare leaf flavonoid extract on streptozotocin-induced hyperglycemia and associated complications in rats. Food Sci. Nutr. 2018, 3, 394. [Google Scholar] [CrossRef]
- Chandrasekar, V.; Coupland, J.N.; Anantheswaran, R.C. Functionalization of Crosslinked Sodium Alginate/Gelatin Wet-Spun Porous Fibers with Nisin Z for the Inhibition of Staphylococcus aureus-Induced Infections. Food Eng. Mater. Sci. 2016, 81, E2503. [Google Scholar]
- Kouderis, C.; Siafarika, P.; Kalampounias, A.G. Molecular relaxation dynamics and self-association of dexamethasone sodium phosphate solutions. Chem. Pap. 2021, 75, 6115. [Google Scholar] [CrossRef] [PubMed]
- Siafarika, P.; Kouderis, C.; Kalampounias, A.G. Non-Debye segmental relaxation of poly-N-vinyl-carbazole in dilute solution. Mol. Phys. 2020, 119, e1802075. [Google Scholar] [CrossRef]
- Tsigoias, S.; Kouderis, C.; Mylona-Kosmas, A.; Boghosian, S.; Kalampounias, A.G. Proton-transfer in 1,1,3,3 tetramethyl guanidine by means of ultrasonic relaxation and Raman spectroscopies and molecular orbital calculations. Spectrochim. Acta A 2020, 229, 117958. [Google Scholar] [CrossRef]
- Williams, G.C.; Delves-Broughton, J. Nisin. In Encyclopedia of Food Sciences and Nutrition, 2nd ed.; Caballero, B., Trugo, L., Finglas, P.M., Eds.; Academic Press: London, UK, 2003. [Google Scholar]
- Antosiewicz, J.N.; Shuger, D. UV–Vis spectroscopy of tyrosine side-groups in studies of protein structure. Part 2: Selected applications. Biophys. Rev. 2016, 8, 163. [Google Scholar] [CrossRef]
- Herzfeld, K.F.; Litovitz, T.A. Absorption and Dispersion of Ultrasonic Waves; Academic Press: New York, NY, USA, 1959. [Google Scholar]
- Ensminger, D.; Bond, L.J. Ultrasonics: Fundamentals, Technologies, and Applications, 3rd ed.; CRC Press: New York, NY, USA, 2011. [Google Scholar]
- Blandamer, M.J. Introduction to Chemical Ultrasonics; Academic Press: New York, NY, USA, 1973. [Google Scholar]
- Davidson, D.W.; Cole, R.H. Dielectric Relaxation in Glycerine. J. Chem. Phys. 1950, 18, 1417. [Google Scholar] [CrossRef]
- Davidson, D.W.; Cole, R.H. Dielectric Relaxation in Glycerol, Propylene Glycol, and n-Propanol. J. Chem. Phys. 1951, 19, 1484. [Google Scholar] [CrossRef]
- Slutsky, L.J. Ultrasonic Chemical Relaxation Spectroscopy. In Methods of Experimental Physics; Edmonds, P.D., Ed.; Academic Press Inc.: New York, NY, USA, 1981; pp. 179–235. [Google Scholar]
- Kalampounias, A.G. Establishing the role of shear viscosity on the rate constants of conformational fluctuations in unsaturated aldehydes. Chem. Phys. 2022, 561, 111618. [Google Scholar] [CrossRef]
- Kaseman, D.C.; Retsinas, A.; Kalampounias, A.G.; Papatheodorou, G.N.; Sen, S. Cooperative or Anticooperative: How Noncovalent Interactions Influence Each Other. J. Phys. Chem. B 2015, 119, 8440. [Google Scholar] [CrossRef] [PubMed]
- Wyn-Jones, E. (Ed.) Chemical and Biological Applications of Relaxation Spectrometry; NATO Advanced Study Institute; D. Reidel Publishing Company: Dordrecht, The Netherlands, 1974. [Google Scholar]
- Lamb, J. Thermal Relaxation in Liquids. In Physical Acoustics; Academic Press Inc.: Cambridge, MA, USA, 1965; Volume 2. [Google Scholar]
- Nishikawa, S.; Haraguchi, H.; Fukuyama, Y. Sulfur Nitride in Organic Chemistry. Part 19. Selective Formation of Benzo- and Benzobis[1,2,5]thiadiazole Skeleton in the Reaction of Tetrasulfur Tetranitride with Naphthalenols and Related Compounds. Bull. Chem. Soc. Jpn. 1991, 64, 1274. [Google Scholar] [CrossRef]
- Nishikawa, S.; Kamimura, E. β-Phase Formation of Poly(9,9-dioctylfluorene) Induced by Liposome Phospholipid Bilaye. J. Phys. Chem. A 2011, 115, 535. [Google Scholar] [CrossRef] [PubMed]
- Pethrick, R.A. Properties of crosslinked polyolefin-based materials. Prog. Polym. Sci. 1983, 9, 197. [Google Scholar] [CrossRef]
- Kaatze, U.; Hushcha, T.O.; Eggers, F. FTIR Spectroscopy of Ion Solvation of LiClO4 and LiSCN in Acetonitrile, Benzonitrile, and Propylene Carbonate. J. Solution Chem. 2000, 29, 299. [Google Scholar] [CrossRef]
- Jacobson, B. Ultrasonic Velocity in Liquids and Liquid Mixtures. J. Chem. Phys. 1952, 20, 927. [Google Scholar] [CrossRef]
- Jacobson, B. A Method for Condensations of Esters with Diethyl Oxalate. Acta Chem. Scand. 1952, 5, 485. [Google Scholar]
- Pandey, J.D.; Jain, R.P.; Pandey, H.C. Circumferential Stress Waves Around a Circular Cavity in a Polymeric Plate. Acustica 1975, 34, 123. [Google Scholar]
- Pandey, J.D.; Dubey, P.; Kumar, V. Acta Acustica united with Acustica. Acustica 1979, 42, 342. [Google Scholar]
- Liu, W.; Hansen, J.N. Some chemical and physical properties of nisin, a small-protein antibiotic produced by Lactococcus lactis. Appl. Environ. Microbiol. 1990, 56, 2551. [Google Scholar] [CrossRef] [PubMed]
- Knudsen, C.B.; Kalampounias, A.G.; Fehrmann, R.; Boghosian, S. On the Molecular Mechanism of Water Reorientation. J. Phys. Chem. B 2008, 112, 11996. [Google Scholar] [CrossRef] [PubMed]
- Kalampounias, A.G.; Kirillov, S.A.; Steffen, W.; Yannopoulos, S.N. Peculiarities of benzophenone structure in heterogeneous systems with Fe- and Cu-doped TiO2 particles. J. Mol. Struct. 2003, 651–653, 475. [Google Scholar] [CrossRef]
- Kouderis, C.; Siafarika, P.; Kalampounias, A.G. Disentangling proton-transfer and segmental motion relaxations in poly-vinyl-alcohol aqueous solutions by means of ultrasonic relaxation spectroscopy. Polymer 2021, 217, 123479. [Google Scholar] [CrossRef]
- Kalampounias, A.G. Exploring conformational change profile of n-propyl ester of formic acid by combining ultrasonic relaxation spectroscopy and molecular orbital calculations. J. Mol. Struct. 2020, 1212, 128146. [Google Scholar] [CrossRef]
- Stogiannidis, G.; Tsigoias, S.; Kalampounias, A.G. Conformational energy barriers in methyl acetate – Ethanol solutions: A temperature-dependent ultrasonic relaxation study and molecular orbital calculations. J. Mol. Liq. 2020, 302, 112519. [Google Scholar] [CrossRef]
- Ricci, C.G.; Netz, P.A. Docking Studies on DNA-Ligand Interactions: Building and Application of a Protocol To Identify the Binding Mode. J. Chem. Inf. Model. 2009, 49, 1925. [Google Scholar] [CrossRef] [PubMed]
- Gasteiger, J.; Marsili, M. A new model for calculating atomic charges in molecules. Tetrahedron Lett. 1978, 19, 3181. [Google Scholar] [CrossRef]
- Gasteiger, J.; Marsili, M. Iterative partial equalization of orbital electronegativity—A rapid access to atomic charges. Tetrahedron 1980, 36, 3219. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, M.W.; Baldridge, K.K.; Boatz, J.A.; Elbert, S.T.; Gordon, M.S.; Jensen, J.H.; Koseki, S.; Matsunaga, N.; Nguyen, K.A.; Su, S.; et al. General atomic and molecular electronic structure system. Comput. Chem. 1993, 14, 1347. [Google Scholar] [CrossRef]


| T (°C) | u (m/s) | ρ (g/cm3) | A1 (×10−13 s2/cm) | B1 (×10−16 s2/cm) | fr1 (MHz) | A2 (×10−13 s2/cm) | B2 (×10−16 s2/cm) | fr2 (MHz) | μmax1 | μmax2 | r1 | r2 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 10 | 1462.0 | 1.0069 | 4.04 | 1.46 | 1.24 | 1.70 | 3.80 | 1.91 | 0.0366 | 0.0237 | 0.0232 | 0.0150 |
| 15 | 1479.3 | 1.0062 | 4.25 | 1.46 | 1.27 | 1.75 | 4.42 | 2.03 | 0.0400 | 0.0262 | 0.0253 | 0.0166 |
| 20 | 1494.8 | 1.0051 | 4.27 | 1.46 | 1.29 | 1.84 | 3.26 | 2.00 | 0.0412 | 0.0276 | 0.0260 | 0.0175 |
| 25 | 1508.3 | 1.0037 | 4.63 | 1.46 | 1.35 | 2.01 | 4.37 | 2.08 | 0.0470 | 0.0315 | 0.0297 | 0.0199 |
| 30 | 1521.3 | 1.0024 | 4.84 | 1.46 | 1.34 | 2.10 | 3.68 | 2.12 | 0.0493 | 0.0339 | 0.0311 | 0.0215 |
| 35 | 1531.4 | 0.9999 | 5.47 | 1.46 | 1.40 | 2.36 | 3.28 | 2.18 | 0.0586 | 0.0394 | 0.0369 | 0.0249 |
| C (mM) | u (m/s) | ρ (g/cm3) | A1 (×10−13 s2/cm) | B1 (×10−16 s2/cm) | fr1 (MHz) | A2 (×10−13 s2/cm) | B2 (×10−16 s2/cm) | fr2 (MHz) | μmax1 | μmax2 | r1 | r2 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1488.6 | 1.0007 | 4.24 | 1.46 | 1.26 | 1.84 | 2.55 | 2.000 | 0.0398 | 0.0274 | 0.0252 | 0.0174 |
| 2 | 1494.8 | 1.0032 | 4.26 | 1.45 | 1.26 | 1.84 | 2.48 | 2.002 | 0.0401 | 0.0275 | 0.0254 | 0.0174 |
| 3 | 1497.0 | 1.0051 | 4.27 | 1.46 | 1.29 | 1.84 | 2.26 | 2.005 | 0.0412 | 0.0276 | 0.0261 | 0.018 |
| 4 | 1499.2 | 1.0074 | 4.30 | 1.47 | 1.28 | 1.85 | 2.54 | 2.010 | 0.0413 | 0.0279 | 0.0261 | 0.0177 |
| 5 | 1503.5 | 1.0093 | 4.33 | 1.46 | 1.29 | 1.86 | 2.26 | 2.015 | 0.0420 | 0.0282 | 0.0266 | 0.0179 |
| 7.5 | 1510.6 | 1.0144 | 4.43 | 1.46 | 1.24 | 1.89 | 2.46 | 2.034 | 0.0415 | 0.0290 | 0.0262 | 0.0184 |
| 10 | 1517.4 | 1.0198 | 4.58 | 1.45 | 1.24 | 1.94 | 2.46 | 2.060 | 0.0431 | 0.0303 | 0.0272 | 0.0192 |
| Concentration in mM of Νisin Solutions | Mass in g of Solid Nisin from Lactococcus lactis 2.5% (Balance Sodium Chloride) |
|---|---|
| 1 | 0.0168 |
| 2 | 0.0335 |
| 3 | 0.0503 |
| 4 | 0.0671 |
| 5 | 0.0839 |
| 7.5 | 0.1258 |
| 10 | 0.1677 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tryfon, A.; Siafarika, P.; Kouderis, C.; Kaziannis, S.; Boghosian, S.; Kalampounias, A.G. Evidence of Self-Association and Conformational Change in Nisin Antimicrobial Polypeptide Solutions: A Combined Raman and Ultrasonic Relaxation Spectroscopic and Theoretical Study. Antibiotics 2023, 12, 221. https://doi.org/10.3390/antibiotics12020221
Tryfon A, Siafarika P, Kouderis C, Kaziannis S, Boghosian S, Kalampounias AG. Evidence of Self-Association and Conformational Change in Nisin Antimicrobial Polypeptide Solutions: A Combined Raman and Ultrasonic Relaxation Spectroscopic and Theoretical Study. Antibiotics. 2023; 12(2):221. https://doi.org/10.3390/antibiotics12020221
Chicago/Turabian StyleTryfon, Afrodite, Panagiota Siafarika, Constantine Kouderis, Spyridon Kaziannis, Soghomon Boghosian, and Angelos G. Kalampounias. 2023. "Evidence of Self-Association and Conformational Change in Nisin Antimicrobial Polypeptide Solutions: A Combined Raman and Ultrasonic Relaxation Spectroscopic and Theoretical Study" Antibiotics 12, no. 2: 221. https://doi.org/10.3390/antibiotics12020221
APA StyleTryfon, A., Siafarika, P., Kouderis, C., Kaziannis, S., Boghosian, S., & Kalampounias, A. G. (2023). Evidence of Self-Association and Conformational Change in Nisin Antimicrobial Polypeptide Solutions: A Combined Raman and Ultrasonic Relaxation Spectroscopic and Theoretical Study. Antibiotics, 12(2), 221. https://doi.org/10.3390/antibiotics12020221







