Biosensing by Polymer-Coated Etched Long-Period Fiber Gratings Working near Mode Transition and Turn-around Point
Abstract
1. Introduction
- (a)
- (b)
- (c)
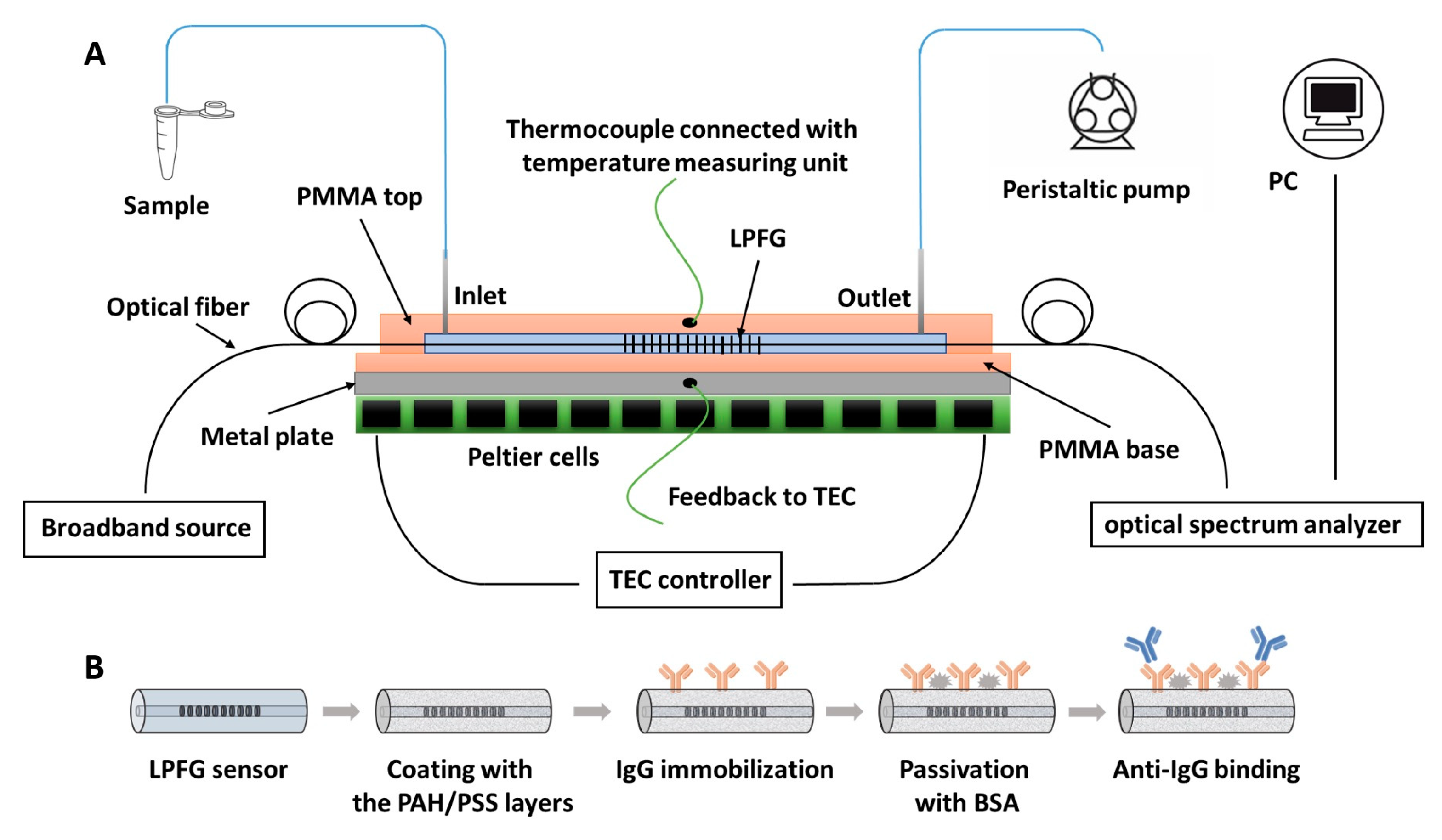
2. Materials and Methods
2.1. Materials
2.2. Inscription of LPFG Sensor
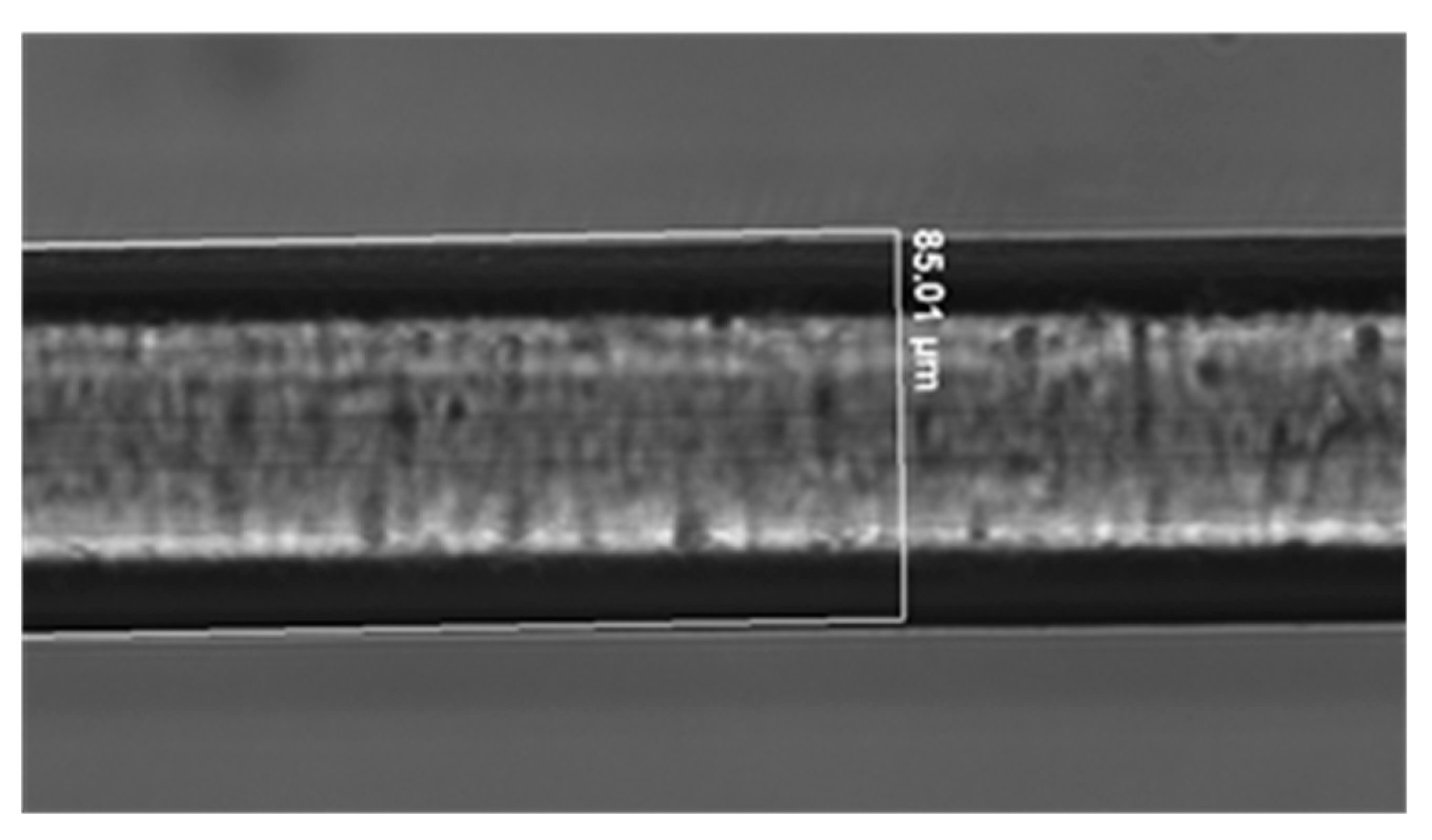
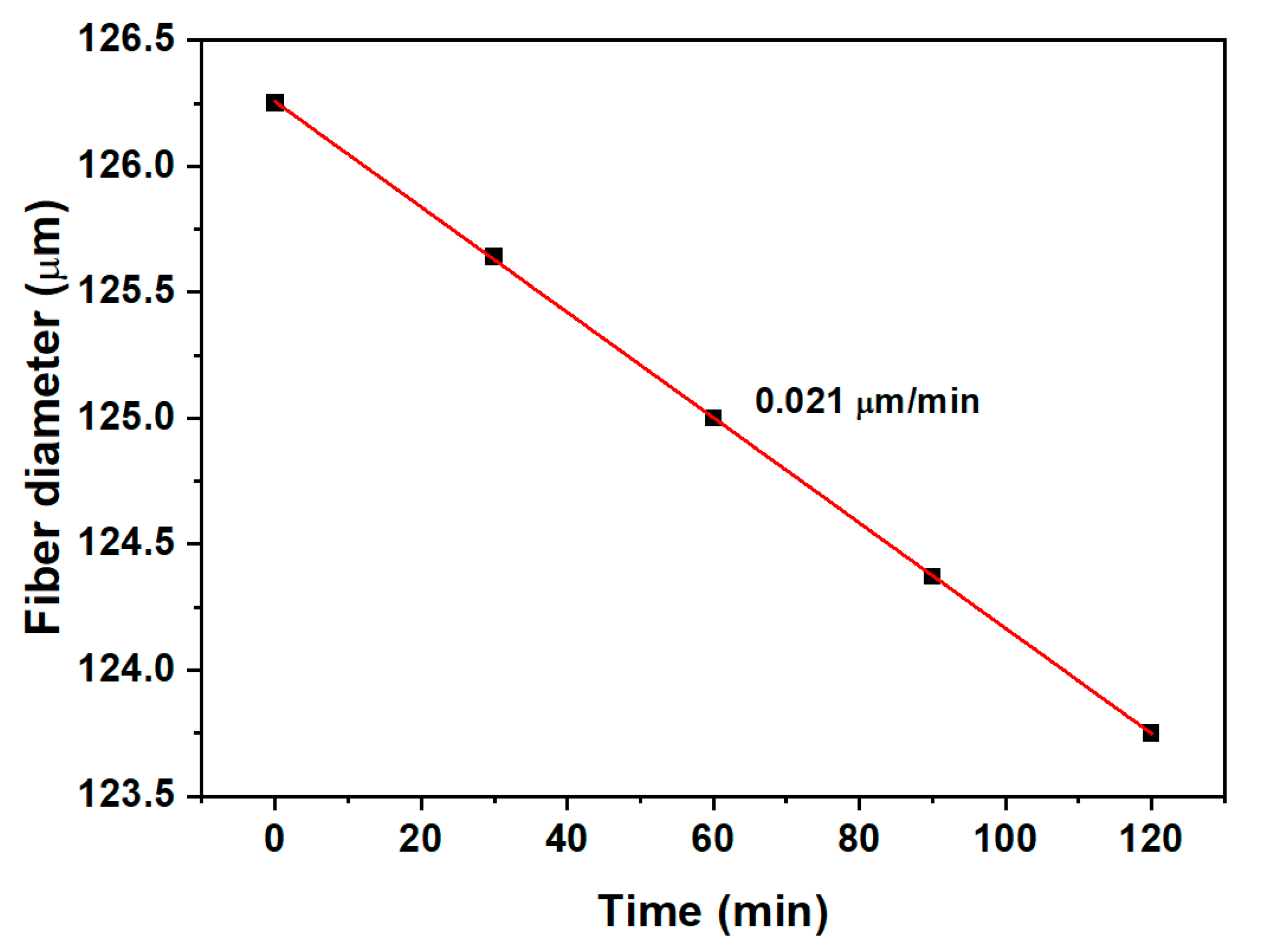
2.3. Etching Process
2.4. Polymer-Coating Process
2.5. Immunoassay Protocol
- Fast injection at 45 μL/min for 3 min (fast filling of the flow cell).
- Slow flow at 6.75 μL/min for ~20 min (binding phase at slow flow rate).
- PBS washing after each step at a flow rate of 45 μL/min for 5 min.
2.6. Data Acquisition and Analysis
3. Results and Discussion
3.1. Theory and Simulation
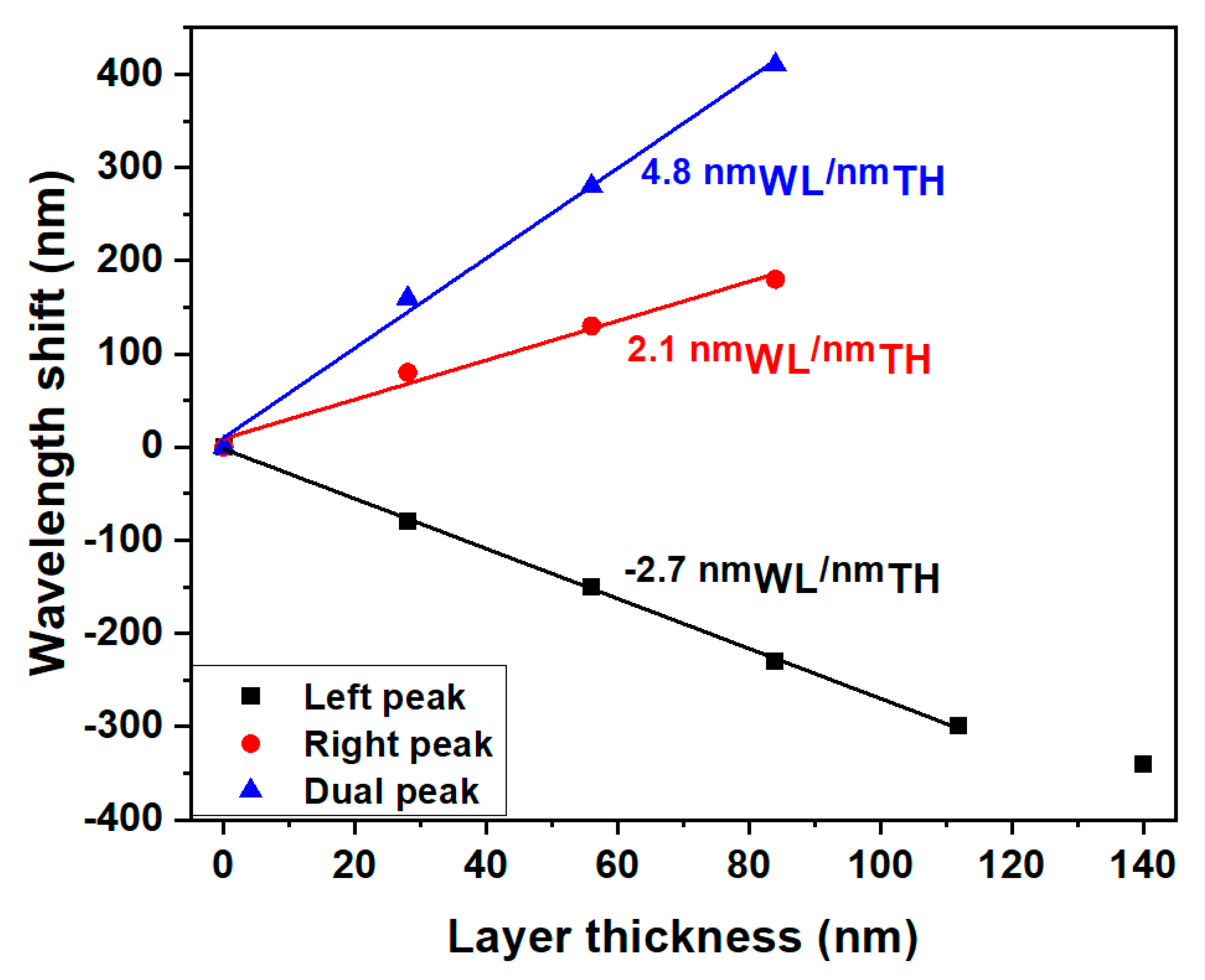
3.2. Fabrication of the LPFG Sensor
3.3. Immunoassay to Evaluate the Sensor Performance
4. Conclusions
- -
- The mode transition of a cladding mode by the deposition of an overlay with an RI greater than the fiber core RI;
- -
- The turn-around point of a cladding mode;
- -
- The enhancement of the evanescent field by reducing the fiber cladding diameter through chemical etching.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Soares, M.S.; Vidal, M.; Santos, N.F.; Costa, F.M.; Marques, C.; Pereira, S.O.; Leitão, C. Immunosensing Based on Optical Fiber Technology: Recent Advances. Biosensors 2021, 11, 305. [Google Scholar] [CrossRef]
- Janczuk-Richter, M.; Dominik, M.; Roźniecka, E.; Koba, M.; Mikulic, P.; Bock, W.J.; Łoś, M.; Śmietana, M.; Niedziółka-Jönsson, J. Long-period fiber grating sensor for detection of viruses. Sens. Actuators B Chem. 2017, 250, 32–38. [Google Scholar]
- Du, C.; Wang, Q.; Zhao, S.; Deng, X. Biological sensors based on long period fiber grating. Opt. Laser Technol. 2023, 158, 108936. [Google Scholar] [CrossRef]
- Jiang, Y.; Yin, S.; Dong, J.; Kaynak, O. A Review on Soft Sensors for Monitoring, Control, and Optimization of Industrial Processes. IEEE Sens. J. 2021, 21, 12868–12881. [Google Scholar] [CrossRef]
- Yang, F.; Chang, T.; Liu, T.; Wu, D.; Du, H.; Liang, J.; Tian, F. Label-free detection of Staphylococcus aureus bacteria using long-period fiber gratings with functional polyelectrolyte coatings. Biosens. Bioelectron. 2019, 133, 147–153. [Google Scholar] [CrossRef]
- Esposito, F.; Sansone, L.; Taddei, C.; Campopiano, S.; Giordano, M.; Iadicicco, A. Ultrasensitive biosensor based on long period grating coated with polycarbonate-graphene oxide multilayer. Sens. Actuators B Chem. 2018, 274, 517–526. [Google Scholar] [CrossRef]
- Janczuk-Richter, M.; Piestrzynska, M.; Burnat, D.; Sezemsky, P.; Stranak, V.; Bock, W.J.; Bogdanowicz, R.; Niedziolka-Jonsson, J.; Smietana, M. Optical investigations of electrochemical processes using a long-period fiber grating functionalized by indium tin oxide. Sens. Actuators B Chem. 2019, 279, 223–229. [Google Scholar] [CrossRef]
- Del Villar, I.; Matias, I.R.; Arregui, F.J.; Lalanne, P. Optimization of sensitivity in long period fiber gratings with overlay deposition. Opt. Express 2005, 13, 56–69. [Google Scholar] [CrossRef]
- Pilla, P.; Foglia Manzillo, P.; Malachovska, V.; Buosciolo, A.; Campopiano, S.; Cutolo, A.; Ambrosio, L.; Giordano, M.; Cusano, A. Long period grating working in transition mode as promising technological platform for label free biosensing. Opt. Exp. 2009, 17, 20039–20050. [Google Scholar] [CrossRef]
- Pilla, P.; Malachovska, V.; Borriello, A.; Buosciolo, A.; Giordano, M.; Ambrosio, L.; Cutolo, A.; Cusano, A. Transition mode long period grating biosensor with functional multilayer coatings. Opt. Exp. 2011, 19, 512–526. [Google Scholar] [CrossRef] [PubMed]
- Shu, X.; Zhang, L.; Bennion, I. Sensitivity characteristics of long-period fiber grating. J. Light. Technol. 2022, 20, 255–266. [Google Scholar]
- Biswas, P.; Basumallick, N.; Bandyopadhyay, S.; Dasgupta, K.; Ghosh, A.; Bandyopadhyay, S. Sensitivity enhancement of turn-around-point long period grating by tuning initial coupling condition. IEEE Sens. J. 2015, 15, 1240–1245. [Google Scholar] [CrossRef]
- Chiang, K.S.; Liu, Y.; Ng, M.N.; Dong, X. Analysis of etched long-period fiber grating and its response to external refractive index. Electron. Lett. 2000, 36, 966–967. [Google Scholar] [CrossRef]
- Śmietana, M.; Koba, M.; Mikulic, P.; Bock, W.J. Measurements of reactive ion etching process effect using long-period fiber gratings. Opt. Exp. 2014, 22, 5986–5994. [Google Scholar] [CrossRef]
- Zou, F.; Liu, Y.; Mou, C.; Zhu, S. Optimization of refractive index sensitivity in nano-film coated long-period fiber grating near dispersion turning point. J. Light. Technol. 2020, 38, 889–897. [Google Scholar] [CrossRef]
- Del Villar, I.; Cruz, J.L.; Socorro, A.B.; Corres, J.M.; Matias, I.R. Sensitivity optimization with cladding-etched long period fiber gratings at dispersion turning point. Opt. Exp. 2016, 24, 17680–17685. [Google Scholar] [CrossRef]
- Piestrzyńska, M.; Dominik, M.; Kosiel, K.; Janczuk-Richter, M.; Szot-Karpińska, K.; Brzozowska, E.; Shao, L.; Niedziółka-Jonsson, J.; Bock, W.J.; Śmietana, M. Ultrasensitive tantalum oxide nano-coated long-period grating for detection of various biological targets. Biosens. Bioelectron. 2019, 133, 8–15. [Google Scholar] [CrossRef]
- Śmietana, M.; Koba, M.; Mikulic, P.; Bock, W.J. Towards refractive index sensitivity of long period gratings at level of tens of µm per refractive index unit: Fiber cladding etching and nano-coating deposition. Opt. Exp. 2016, 24, 11897–11904. [Google Scholar] [CrossRef] [PubMed]
- Śmietana, M.; Koba, M.; Mikulic, P.; Bock, W.J. Combined plasma-based fiber etching and diamond-like carbon nanooverlay deposition for enhancing sensitivity of long-period grating. J. Light. Technol. 2016, 34, 4615–4619. [Google Scholar] [CrossRef]
- Del Villar, I. Ultrahigh-sensitivity sensors based on thin-film coated long period grating with reduced diameter, in transition mode and near the dispersion turning point. Opt. Exp. 2015, 23, 8389–8398. [Google Scholar] [CrossRef]
- Bandyopadhyay, S.; Del Villar, I.; Basumallick, N.; Biswas, P.; Dey, T.K.; Bandyopadhyay, S. Long period fiber grating for biosensing: An improved design methodology to enhance add-layer sensitivity. J. Light. Technol. 2018, 36, 1178–1184. [Google Scholar] [CrossRef]
- Dey, T.K.; Biswas, P.; Basumallick, N.; Bandyopadhyay, S. Long Period Fiber Grating Near Turn Around Point: Suitable Design for Bio-Sensing. IEEE Sens. J. 2021, 21, 18800–18805. [Google Scholar] [CrossRef]
- Dey, T.K.; Roy, A.; Biswas, P.; Basumallick, N.; Bandyopadhyay, S. Investigations on the add-layer sensitivity near mode transition of a stretched mode long period fiber grating. Opt. Fiber Technol. 2022, 72, 102969. [Google Scholar] [CrossRef]
- Elżbieciak-Wodka, M.; Kolasińska-Sojka, M.; Nowak, P.; Warszyński, P. Comparison of permeability of poly(allylamine hydrochloride)/and poly(diallyldimethylammonium chloride)/poly(4-styrenesulfonate) multilayer films: Linear vs. exponential growth. J. Electroanal. Chem. 2015, 738, 195–202. [Google Scholar] [CrossRef]
- Feldo, Z.; Varga, I.; Blomberg, E. Influence of Salt and Rinsing Protocol on the Structure of PAH/PSS Polyelectrolyte Multilayers. Langmuir. 2010, 26, 17048–17057. [Google Scholar] [CrossRef] [PubMed]
- Dey, T.K.; Bandyopadhyay, S.; Biswas, P.; Basumallick, N.; Sinha, P.K.; Dasgupta, K.; Bandyopadhyay, S. A technique of fine tuning self assembled layer thickness on long period grating near mode transition. In Proceedings of the 12th International Conference on Fiber Optics and Photonics, Virtual, 13–16 December 2014; paper M4A.8. [Google Scholar]
- Dey, T.K.; Tombelli, S.; Biswas, P.; Giannetti, A.; Basumallick, N.; Baldini, F.; Bandyopadhyay, S.; Trono, C. Analysis of the lowest order cladding mode of long period fiber gratings near turn around point. J. Light. Technol. 2021, 39, 4006–4012. [Google Scholar] [CrossRef]
- Erdogan, T. Cladding-mode resonances in short- and long-period fiber grating filters. J. Opt. Soc. Am. 1997, 14, 1760–1773. [Google Scholar] [CrossRef]
- DeLisa, M.P.; Zhang, Z.; Shiloach, M.; Pilevar, S.; Davis, C.C.; Sirkis, J.S.; Bentley, W.E. Evanescent wave long-period fiber bragg grating as an immobilized antibody biosensor. Anal. Chem. 2000, 72, 2895–2900. [Google Scholar] [CrossRef]
- Kim, D.W.; Zhang, Y.; Cooper, K.L.; Wang, A. Fibre-optic interferometric immuno-sensor using long period grating. Electron. Lett. 2006, 42, 324–325. [Google Scholar] [CrossRef]
- Pilla, P.; Sandomenico, A.; Malachovská, V.; Borriello, A.; Giordano, M.; Cutolo, A.; Ruvo, M.; Cusano, A. A protein-based biointerfacing route toward label-free immunoassays with long period gratings in transition mode. Biosens. Bioelectron. 2012, 31, 486–491. [Google Scholar] [CrossRef]
- Chiavaioli, F.; Biswas, P.; Trono, C.; Bandyopadhyay, S.; Basumallick, N.; Giannetti, A.; Tombelli, S.; Dasgupta, K.; Baldini, F. Towards sensitive label-free immunosensing by means of turn-around point long period fiber gratings. Biosens. Bioelectron. 2014, 60, 305–310. [Google Scholar] [CrossRef]
- Liu, C.; Cai, Q.; Xu, B.; Zhu, W.; Zhang, L.; Zhao, J.; Chen, X. Graphene oxide functionalized long period grating for ultrasensitive label-free immunosensing. Biosens. Bioelectron. 2017, 94, 200–206. [Google Scholar] [CrossRef]
- Ascorbe, J.; Corres, J.M.; Arregui, F.; Matias, I.R. Optical Fiber Immunosensor Based on Long Period Gratings Built by Periodic Laser Ablation. In Proceedings of the IEEE Sensors, New Delhi, India, 29–31 October 2018; pp. 1–4. [Google Scholar]
- Dong, J.; Sang, M.; Wang, S.; Xu, T.; Yu, X.; Liu, T. Ultrasensitive label-free biosensor based on the graphene-oxide-coated-u-bent long-period fiber grating inscribed in a two-mode fiber. J. Light. Technol. 2021, 39, 4013–4019. [Google Scholar] [CrossRef]
- Dey, T.K.; Tombelli, S.; Biswas, P.; Giannetti, A.; Basumallick, N.; Baldini, F.; Bandyopadhyay, S.; Trono, C. Label-free immunosensing by long period fiber gratings at the lowest order cladding mode and near turn around point. Opt. Laser Technol. 2021, 142, 107194. [Google Scholar] [CrossRef]
- Wu, P.; Liu, L.; Morgan, S.P.; Correia, R.; Korposh, S. Label-Free Detection of Antibodies Using Functionalised Long Period Grating Optical Fibre Sensors. Results Opt. 2021, 5, 100172. [Google Scholar] [CrossRef]
- He, C.; Tarr, A.; Tighe, P.; Korposh, S.; Morgan, S. Peptide Functionalized Long Period Grating Optical Fibre Sensor for Antibody Measurement with Microfluidic System. In Proceedings of the 13th International Symposium on Communication Systems, Networks and Digital Signal Processing (CSNDSP), Porto, Portugal, 20–22 July 2022; pp. 649–652. [Google Scholar]
- O’Connell, M.A.; Belanger, B.A.; Haaland, P.D. Calibration and assay development using the four-parameter logistic model. Chemom. Intell. Lab. Syst. 1993, 20, 97–114. [Google Scholar] [CrossRef]
- Inczedy, J.; Lengyel, T.; Ure, A.M. Compendium of analytical nomenclature. In The IUPAC ‘Orange Book’, 3rd ed.; Blackwell Science Ltd.: Oxford, UK, 1998. [Google Scholar]







| Parameters | Values Used for Simulation |
|---|---|
| Core RI | 1.44985 |
| Cladding RI | 1.44400 |
| Core diameter | 7.3 µm |
| Fiber diameter | 124.6 µm |
| Index modulation (Δn) | 1.6 × 10−4 |
| Grating period (Λ) | 220 µm |
| No. of grating planes | 140 |
| Grating length | 30.58 mm |
| Value | Error | |
|---|---|---|
| A1 | −0.14 | 0.25 |
| A2 | 296 | 2790 |
| x0 | 6.81 × 1010 | 4.27 × 1012 |
| p | 0.166 | 0.038 |
| Adjusted R-Square | 0.996 |
| Kind of Sensor | Immobilized Receptor (Concentration) | Antigen | Measurement Setup | LOD | Ref. |
|---|---|---|---|---|---|
| LPFG | Anti-IgG (0.5 mg/mL) | IgG | Flow cell | Not given | [29] |
| Reflection mode LPFG | IgG (0.050 mg/mL) | Anti-IgG | Dip coating | Not given | [30] |
| LPFG in MT + overlay (polystyrene) | IgG (0.1 mg/mL) | Anti-IgG | Dip coating | Not given | [31] |
| LPFG at TAP | IgG (1 mg/mL) | Anti-IgG | Flow cell | 7 × 10−2 μg/mL | [32] |
| Graphene oxide nanosheets functionalized dual-peak LPFG | IgG (1 mg/mL) | Anti-IgG | Dip coating | 7 × 10−3 µg/mL | [33] |
| LPFG by laser ablation + overlay (tin dioxide) | IgG (2.4 mg/mL) | Anti-IgG | Dip coating | Not given Sensitivity: 1.1 nm/(mg/L) | [34] |
| Graphene-oxide-coated U-bent LPFG in a two-mode fiber | Anti-IgG | IgG | Dip coating | 2 × 10−2 µg/mL | [35] |
| LPFG at the lowest-order CM and near TAP | IgG (1 mg/mL) | Anti-IgG | Flow cell | 2 × 10−4 µg/mL | [36] |
| LPFG coated with PAH, SiO2 nanoparticles and gold nanoparticles | Anti-IgG (1 mg/mL) | IgG | Dip coating | Minimum detected concentration 10 µg/ml | [37] |
| LPFG coated with PAH, SiO2 nanoparticles and gold nanoparticles | Peptide | IgG | Flow cell | 16.8 pg/mm2 (lowest conc. tested 1 × 10−4 µg/mL | [38] |
| LPFG near MT and near TAP + overlay (poly-cation and the poly-anion multiple layers) | IgG (1 mg/mL) | Anti-IgG | Flow cell | 5 × 10−4 µg/mL 4.5 × 10−6 µg/mL | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dey, T.K.; Trono, C.; Biswas, P.; Giannetti, A.; Basumallick, N.; Baldini, F.; Bandyopadhyay, S.; Tombelli, S. Biosensing by Polymer-Coated Etched Long-Period Fiber Gratings Working near Mode Transition and Turn-around Point. Biosensors 2023, 13, 731. https://doi.org/10.3390/bios13070731
Dey TK, Trono C, Biswas P, Giannetti A, Basumallick N, Baldini F, Bandyopadhyay S, Tombelli S. Biosensing by Polymer-Coated Etched Long-Period Fiber Gratings Working near Mode Transition and Turn-around Point. Biosensors. 2023; 13(7):731. https://doi.org/10.3390/bios13070731
Chicago/Turabian StyleDey, Tanoy Kumar, Cosimo Trono, Palas Biswas, Ambra Giannetti, Nandini Basumallick, Francesco Baldini, Somnath Bandyopadhyay, and Sara Tombelli. 2023. "Biosensing by Polymer-Coated Etched Long-Period Fiber Gratings Working near Mode Transition and Turn-around Point" Biosensors 13, no. 7: 731. https://doi.org/10.3390/bios13070731
APA StyleDey, T. K., Trono, C., Biswas, P., Giannetti, A., Basumallick, N., Baldini, F., Bandyopadhyay, S., & Tombelli, S. (2023). Biosensing by Polymer-Coated Etched Long-Period Fiber Gratings Working near Mode Transition and Turn-around Point. Biosensors, 13(7), 731. https://doi.org/10.3390/bios13070731









