Oxygen Vacancy in Magnéli Phases and Its Effect on Thermoelectric Performances
Abstract
1. Introduction
2. Experimental Section
2.1. Preparation
2.2. Characterization
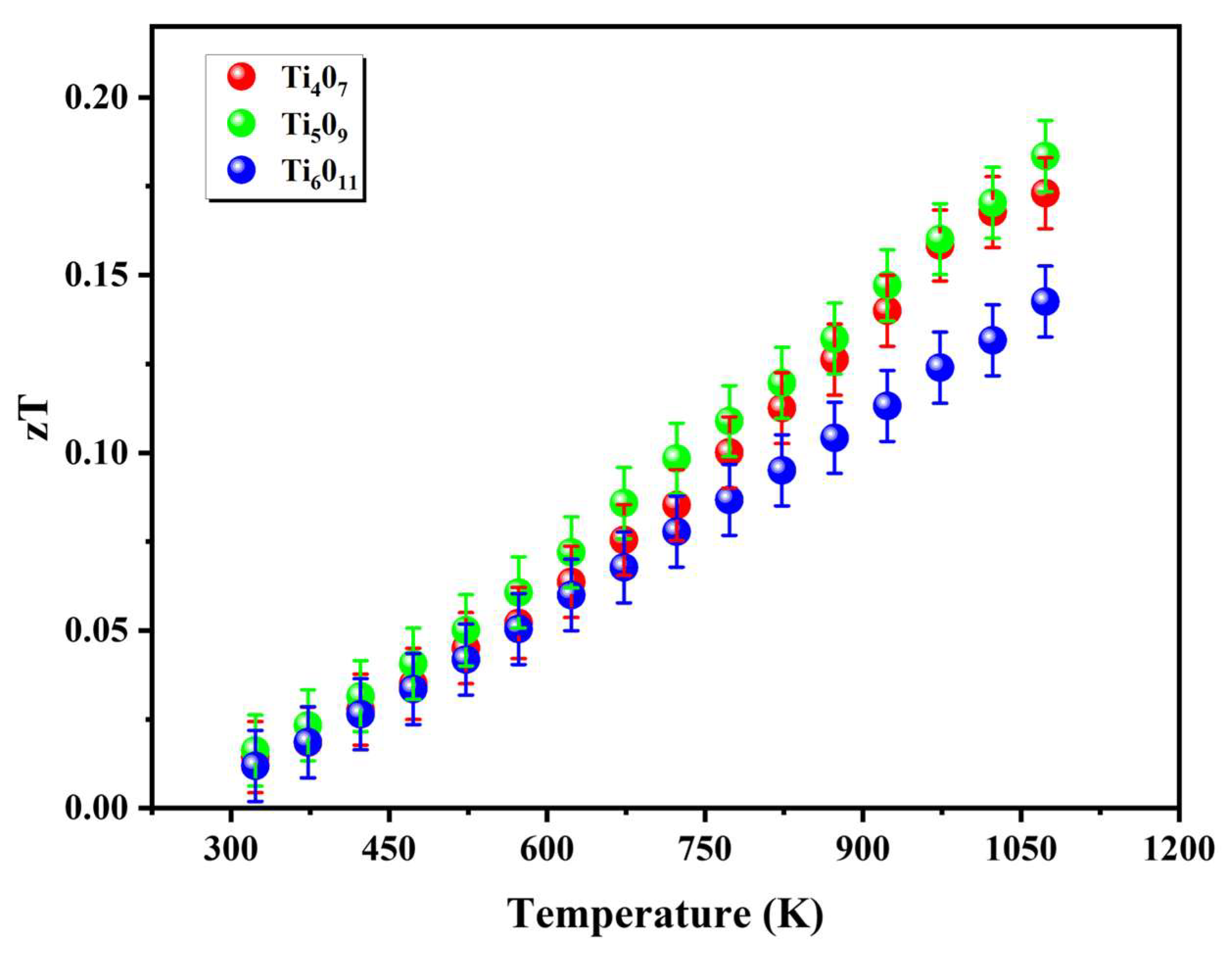
3. Results and Discussion
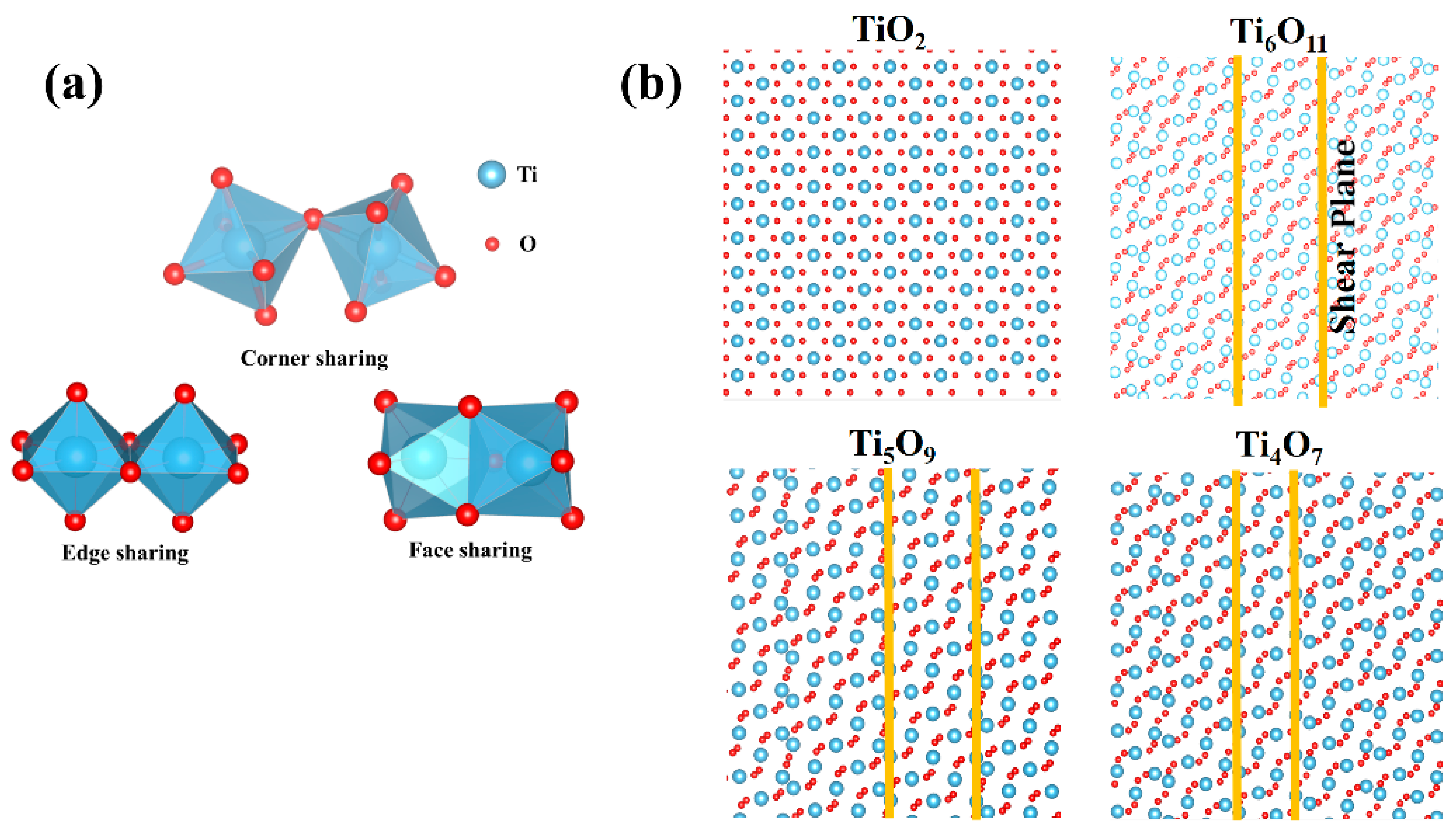
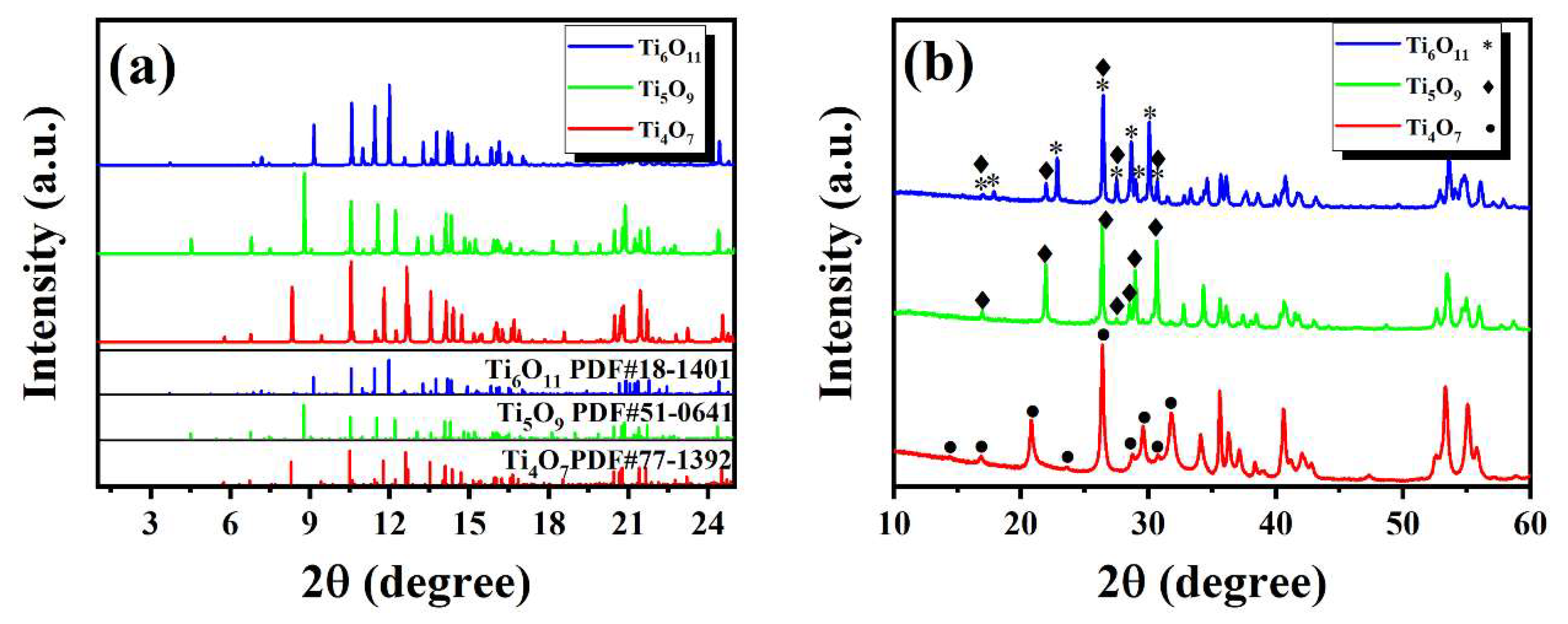
3.1. Characterization of Structure
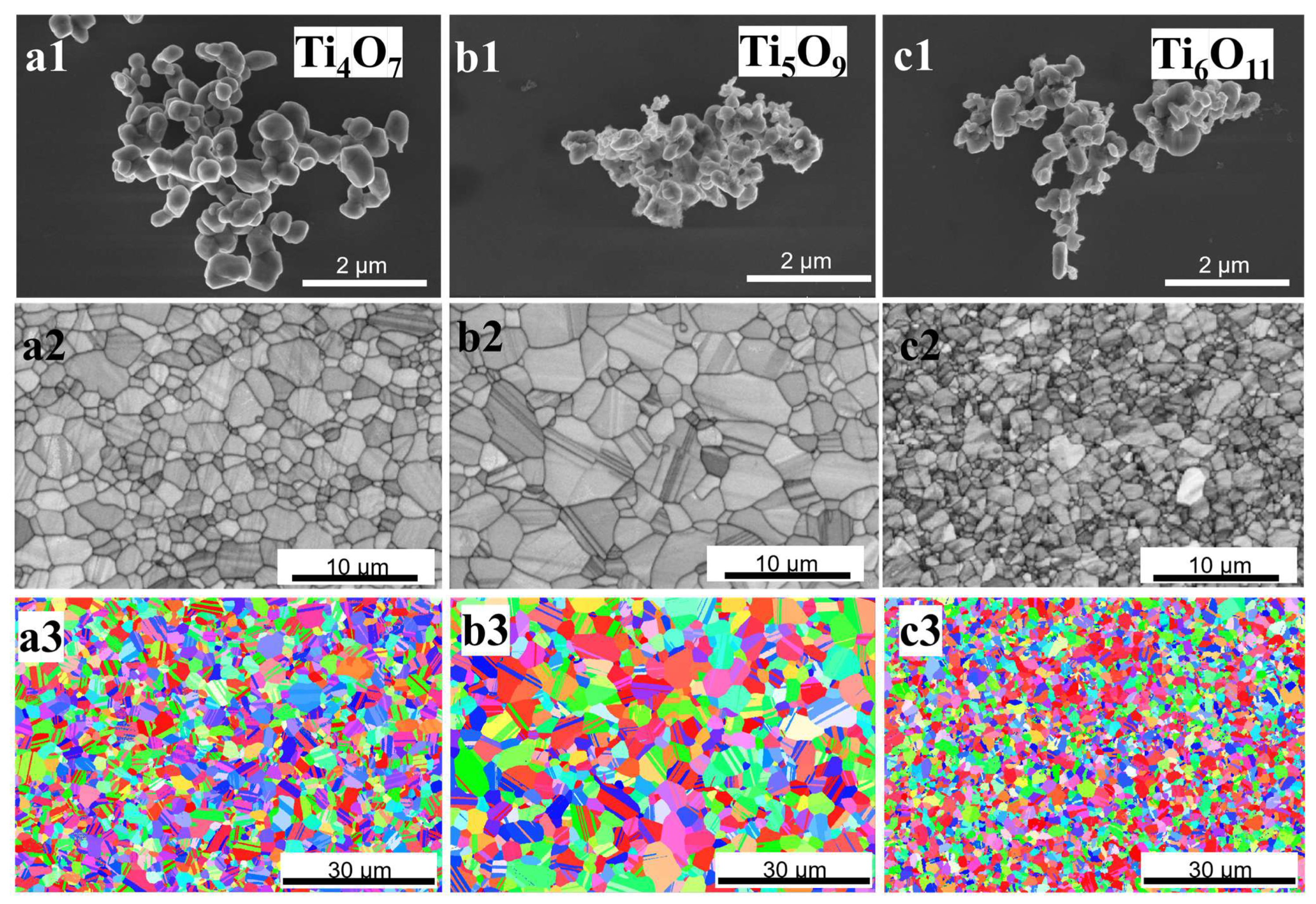
3.2. Microstructure
3.3. Electrical Properties
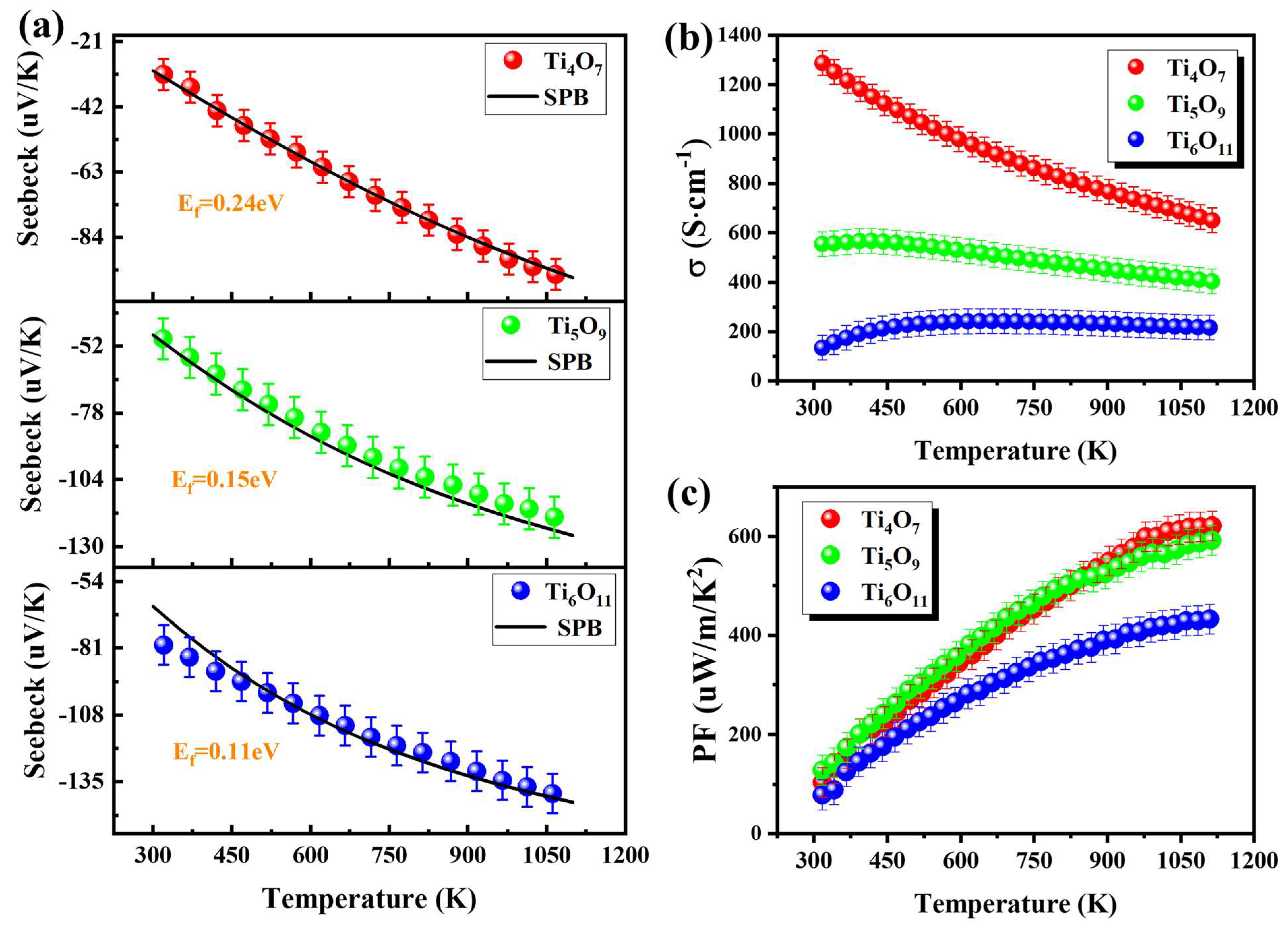
3.4. Thermal Conductivity

4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chen, Z.G.; Shi, X.; Zhao, L.D.; Zou, J. High-Performance SnSe Thermoelectric Materials: Progress and Future Challenge. Prog. Mater. Sci. 2018, 97, 283–346. [Google Scholar] [CrossRef]
- Cho, H.; Yun, J.H.; Back, S.Y.; Lee, J.S.; Kang, N.; Jang, Y.I.; Lim, J.; Son, J.H.; Park, J.Y.; Kim, J.; et al. Superior Thermoelectric Cooling Performance by Suppressing Bipolar Diffusion Effect and Enhancing Anisotropic Texture in P-/n-Type Bi2Te3 Based Compounds. J. Alloys Compd. 2021, 888, 161572. [Google Scholar] [CrossRef]
- Hsieh, H.C.; Wang, C.H.; Lan, T.W.; Lee, T.H.; Chen, Y.Y.; Chu, H.S.; Wu, A.T. Joint Properties Enhancement for PbTe Thermoelectric Materials by Addition of Diffusion Barrier. Mater. Chem. Phys. 2020, 246, 122848. [Google Scholar] [CrossRef]
- Basu, R.; Singh, A. High Temperature Si–Ge Alloy towards Thermoelectric Applications: A Comprehensive Review. Mater. Today Phys. 2021, 21, 100468. [Google Scholar] [CrossRef]
- Ghafoor, F.; Ghafoor, B.; Kim, D.k.; Khan, M.F.; Anis-ur-Rehman, M. Enhancement in Figure of Merit in N-Type Bi(R)-Te Thermoelectric Nanomaterials. J. Mater. Res. Technol. 2023, 23, 3617–3625. [Google Scholar] [CrossRef]
- Chen, W.Y.; Shi, X.L.; Yang, Q.; Li, M.; Lyu, W.; Liu, T.; Cao, T.; Hu, B.; Liu, W.; Sun, S.; et al. Solvothermally Silver Doping Boosting the Thermoelectric Performance of Polycrystalline Bi2Te3. Chem. Eng. J. 2023, 475, 146428. [Google Scholar] [CrossRef]
- Zhang, L.; Cao, Z.; Fu, Q.Q.; Li, C.Y.; Du, Y.Q.; Li, Y.N.; La, T.; Zhang, D.B.; Wang, J. Thermoelectric Properties of Yb-La-Nb-Doped SrTiO3. J. Eur. Ceram. Soc. 2024, 44, 1647–1653. [Google Scholar] [CrossRef]
- Wang, K.X.; Wang, J.; Li, Y.; Zou, T.; Wang, X.H.; Li, J.B.; Cao, Z.; Shi, W.J.; Yaer, X. Enhancement of Thermoelectric Properties of SrTiO3/LaNb-SrTiO3 Composite by Different Doping Levels. Chin. Phys. B 2018, 27, 048401. [Google Scholar] [CrossRef]
- Liu, H.J.; Luo, M.Q.; Yang, L.X.; Zeng, C.L.; Fu, C. A High Strength and Conductivity Bulk Magnéli Phase Ti4O7 with Superior Electrochemical Performance. Ceram. Int. 2022, 48, 25538–25546. [Google Scholar] [CrossRef]
- He, Q.; Hao, Q.; Chen, G.; Poudel, B.; Wang, X.; Wang, D.; Ren, Z. Thermoelectric Property Studies on Bulk TiOx with x from 1 to 2. Appl. Phys. Lett. 2007, 91, 052505. [Google Scholar] [CrossRef]
- Thébaud, S.; Adessi, C.; Bouzerar, G. Investigating the Higherature Thermoelectric Properties of N-Type Rutile TiO2. Phys. Rev. B 2019, 100, 195202. [Google Scholar] [CrossRef]
- Tao, J.; Luttrell, T.; Batzill, M. A Two-Dimensional Phase of TiO2 with a Reduced Bandgap. Nat. Chem. 2011, 3, 296–300. [Google Scholar] [CrossRef]
- Eddy, D.R.; Permana, M.D.; Sakti, L.K.; Sheha, G.A.N.; Solihudin, G.A.N.; Hidayat, S.; Takei, T.; Kumada, N.; Rahayu, I. Heterophase Polymorph of TiO2 (Anatase, Rutile, Brookite, TiO2 (B)) for Efficient Photocatalyst: Fabrication and Activity. Nanomaterials 2023, 13, 704. [Google Scholar] [CrossRef]
- Luttrell, T.; Halpegamage, S.; Tao, J.; Kramer, A.; Sutter, E.; Batzill, M. Why Is Anatase a Better Photocatalyst than Rutile?—Model Studies on Epitaxial TiO2 Films. Sci. Rep. 2015, 4, 4043. [Google Scholar] [CrossRef]
- Liu, X.; Yu, J.; Wang, B.; Maji, K.; Alvarez-Ruiz, D.T.; Guilmeau, E.; Freer, R. Enhancing the Thermoelectric Properties of Nb-Doped TiO2-Based Ceramics through in-Situ Synthesis of β-Sn Inclusions at Grain Boundaries. J. Eur. Ceram. Soc. 2023, 43, 2523–2533. [Google Scholar] [CrossRef]
- Alim, M.A.; Bak, T.; Atanacio, A.; Plessis, J.D.; Zhou, M.; Davis, J.; Nowotny, J. Electrical Conductivity and Defect Disorder of Tantalum-Doped TiO2. J. Am. Ceram. Soc. 2017, 100, 4088–4100. [Google Scholar] [CrossRef]
- Liu, H.; Ma, H.; Wang, F.; Liu, B.; Liu, B.; Chen, J.; Jia, X. Further Insights into Thermoelectric Properties of Nonstoichiometric Titanium Oxide Fabricated by High Pressure and High Temperature. Ceram. Int. 2018, 44, 8043–8047. [Google Scholar] [CrossRef]
- Jaćimović, J.; Gaál, R.; Magrez, A.; Piatek, J.; Forró, L.; Nakao, S.; Hirose, Y.; Hasegawa, T. Low Temperature Resistivity, Thermoelectricity, and Power Factor of Nb Doped Anatase TiO2. Appl. Phys. Lett. 2013, 102, 10–13. [Google Scholar] [CrossRef]
- Portehault, D.; Maneeratana, V.; Candolfi, C.; Oeschler, N.; Veremchuk, I.; Grin, Y.; Sanchez, C.; Antonietti, M. Facile General Route toward Tunable Magnéli Nanostructures and Their Use as Thermoelectric Metal Oxide/Carbon Nanocomposites. ACS Nano 2011, 5, 9052–9061. [Google Scholar] [CrossRef]
- Pandey, S.J.; Joshi, G.; Wang, S.; Curtarolo, S.; Gaume, R.M. Modeling the Thermoelectric Properties of Ti5O9 Magnéli Phase Ceramics. J. Electron. Mater. 2016, 45, 5526–5532. [Google Scholar] [CrossRef]
- Fan, Y.; Feng, X.; Zhou, W.; Murakami, S.; Kikuchi, K.; Nomura, N.; Wang, L.; Jiang, W.; Kawasaki, A. Preparation of Monophasic Titanium Sub-Oxides of Magnéli Phase with Enhanced Thermoelectric Performance. J. Eur. Ceram. Soc. 2018, 38, 507–513. [Google Scholar] [CrossRef]
- Wang, L.; Li, Z.; Hao, L.; Ohira, S.; Itoi, T.; Yoshida, H.; Lu, Y. Thermoelectric Performance Enhancement of Magnéli Phase TinO2n−1 Compacts by In Situ Reduction of TiO2 with Charcoal Powder via Spark Plasma Sintering. J. Electron. Mater. 2022, 51, 7078–7084. [Google Scholar] [CrossRef]
- Walsh, F.C.; Wills, R.G.A. The Continuing Development of Magnéli Phase Titanium Sub-Oxides and Ebonex® Electrodes. Electrochim. Acta 2010, 55, 6342–6351. [Google Scholar] [CrossRef]
- Malik, H.; Sarkar, S.; Mohanty, S.; Carlson, K. Modelling and Synthesis of Magnéli Phases in Ordered Titanium Oxide Nanotubes with Preserved Morphology. Sci. Rep. 2020, 10, 8050. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, J.; Liu, Y.; Zheng, J.; Lee, W.; Shi, J.; Horlyck, J.; Xie, J.; Tay, Y.Y.; Tan, T.T.; et al. Manipulation of Planar Oxygen Defect Arrangements in Multifunctional Magnèli Titanium Oxide Hybrid Systems: From Energy Conversion to Water Treatment. Energy Environ. Sci. 2020, 13, 5080–5096. [Google Scholar] [CrossRef]
- Harada, S.; Tanaka, K.; Inui, H. Thermoelectric Properties and Crystallographic Shear Structures in Titanium Oxides of the Magǹli Phases. J. Appl. Phys. 2010, 108, 6–11. [Google Scholar] [CrossRef]
- Acha, C.; Monteverde, M.; Núñez-Regueiro, M.; Kuhn, A.; Alario Franco, M.A. Electrical Resistivity of the Ti4O7 Magneli Phase under High Pressure. Eur. Phys. J. B 2003, 34, 421–428. [Google Scholar] [CrossRef]
- Wei, W.; Yuan, T.; Ye, J. Recent Progress in Electrochemical Application of Magnéli Phase Ti4O7-Based Materials: A Review. J. Mater. Sci. 2023, 58, 14911–14944. [Google Scholar] [CrossRef]
- Ying, H.; Tian, H.; Meng, Z.; Han, W. TinO2n-1 Series Compounds-Properties, Preparation Methods and Applications. Prog. Chem. 2015, 27, 361–372. [Google Scholar] [CrossRef]
- Zhu, J.; Zhang, X.; Guo, M.; Li, J.; Hu, J.; Cai, S.; Cai, W.; Zhang, Y.; Sui, J. Restructured Single Parabolic Band Model for Quick Analysis in Thermoelectricity. npj Comput. Mater. 2021, 7, 116. [Google Scholar] [CrossRef]
- Li, X.; Zhu, A.L.; Qu, W.; Wang, H.; Hui, R.; Zhang, L.; Zhang, J. Magneli Phase Ti4O7 Electrode for Oxygen Reduction Reaction and Its Implication for Zinc-Air Rechargeable Batteries. Electrochim. Acta 2010, 55, 5891–5898. [Google Scholar] [CrossRef]
- Watanabe, M. Raman Spectroscopy of Charge-Ordered States in Magnéli Titanium Oxides. Phys. Status Solidi C Curr. Top. Solid State Phys. 2009, 6, 260–263. [Google Scholar] [CrossRef]
- Wang, G.; Liu, Y.; Ye, J. Thermoelectric Properties of Non-Stoichiometric Magnéli Phase TinO2n−1 (n = 4, 5, 8, 9) Monoliths. Sci. Adv. Mater. 2018, 10, 39–45. [Google Scholar] [CrossRef]
- Hao, L.; Kikuchi, Y.; Yoshida, H.; Jin, Y.; Lu, Y. Magnèli Phase TinO2n-1 Bulks Prepared by SPS Followed by Carbon Reduction and Their Thermoelectric Performance. J. Alloys Compd. 2017, 722, 846–851. [Google Scholar] [CrossRef]
- Torres, P.; Rurali, R. Thermal Conductivity of Rutile and Anatase TiO2 from First-Principles. J. Phys. Chem. C 2019, 123, 30851–30855. [Google Scholar] [CrossRef]


| Sample | Ti4O7 | Ti5O9 | Ti6O11 |
|---|---|---|---|
| grain size, μm | 2.4 (1) | 3.8 (1) | 2.0 (1) |
| Density, g/cm3 (theory) | 4.238 | 4.2829 | 4.295 |
| Density, g/cm3 (measured) | 4.231 | 4.228 | 4.184 |
| Relative density, % | 99.8 | 98.7 | 97.4 |
| Conductivity, S cm−1 (measured) | 1287 | 554 | 137 |
| Conductivity, S cm−1 (literature) | 992 [21] 1252 [28] | 631 [29] | 63 [29] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, Z.; Feng, C.; Song, H.; Yang, L.; Wang, X.; Liu, H.; Zhang, J.; Wei, F.; Yuan, X.; Yang, H.; et al. Oxygen Vacancy in Magnéli Phases and Its Effect on Thermoelectric Performances. Nanomaterials 2025, 15, 684. https://doi.org/10.3390/nano15090684
Guan Z, Feng C, Song H, Yang L, Wang X, Liu H, Zhang J, Wei F, Yuan X, Yang H, et al. Oxygen Vacancy in Magnéli Phases and Its Effect on Thermoelectric Performances. Nanomaterials. 2025; 15(9):684. https://doi.org/10.3390/nano15090684
Chicago/Turabian StyleGuan, Zhou, Chuangshi Feng, Hongquan Song, Lingxu Yang, Xin Wang, Huijun Liu, Jiawei Zhang, Fanqian Wei, Xin Yuan, Hengyong Yang, and et al. 2025. "Oxygen Vacancy in Magnéli Phases and Its Effect on Thermoelectric Performances" Nanomaterials 15, no. 9: 684. https://doi.org/10.3390/nano15090684
APA StyleGuan, Z., Feng, C., Song, H., Yang, L., Wang, X., Liu, H., Zhang, J., Wei, F., Yuan, X., Yang, H., Tang, Y., & Zhang, F. (2025). Oxygen Vacancy in Magnéli Phases and Its Effect on Thermoelectric Performances. Nanomaterials, 15(9), 684. https://doi.org/10.3390/nano15090684







