Unlocking the Potential of Mg-Doped Rare Earth Manganites: Machine Learning-Guided Synthesis and Insights into Structural and Optical Properties
Abstract
1. Introduction
2. Exploring Materials: Advanced Research Techniques
3. Strategic Insights into Prepared Samples Selection
4. Results and Discussion
4.1. Preparation
4.2. Morphology and Elemental Composition
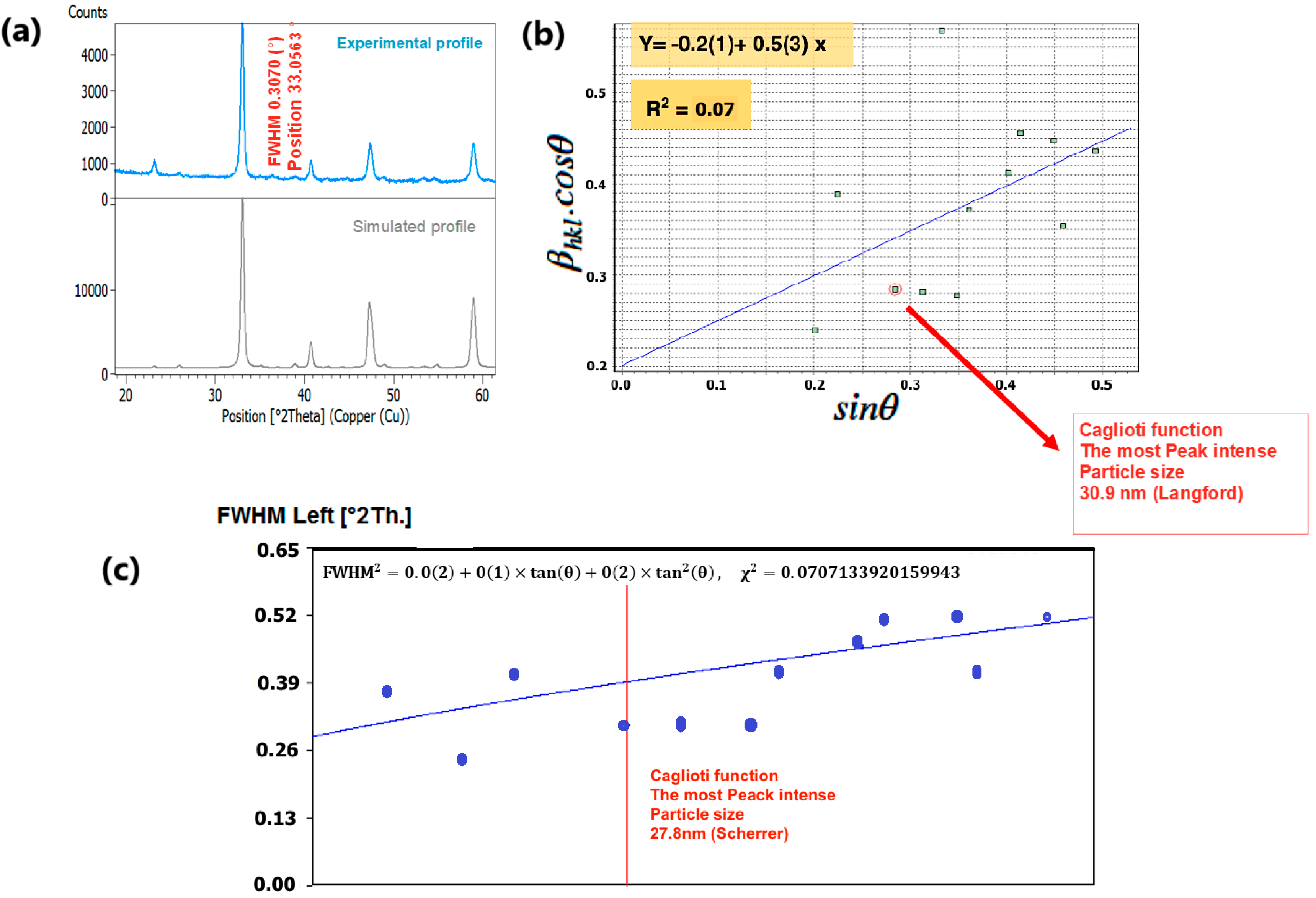
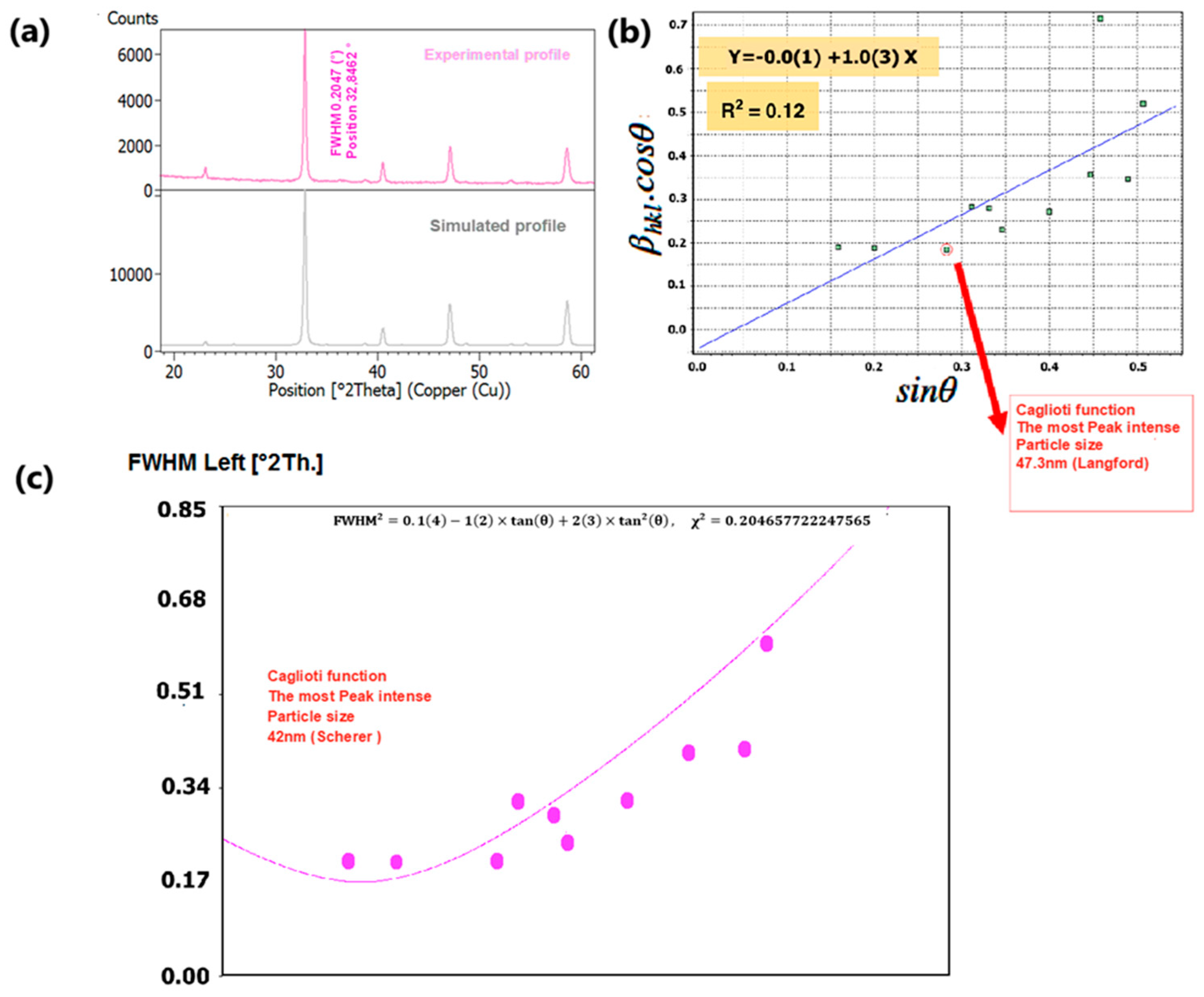
4.3. XRD Characterization
4.4. Exploring Optical Characteristics
4.4.1. Fourier Transform Infrared Spectroscopy
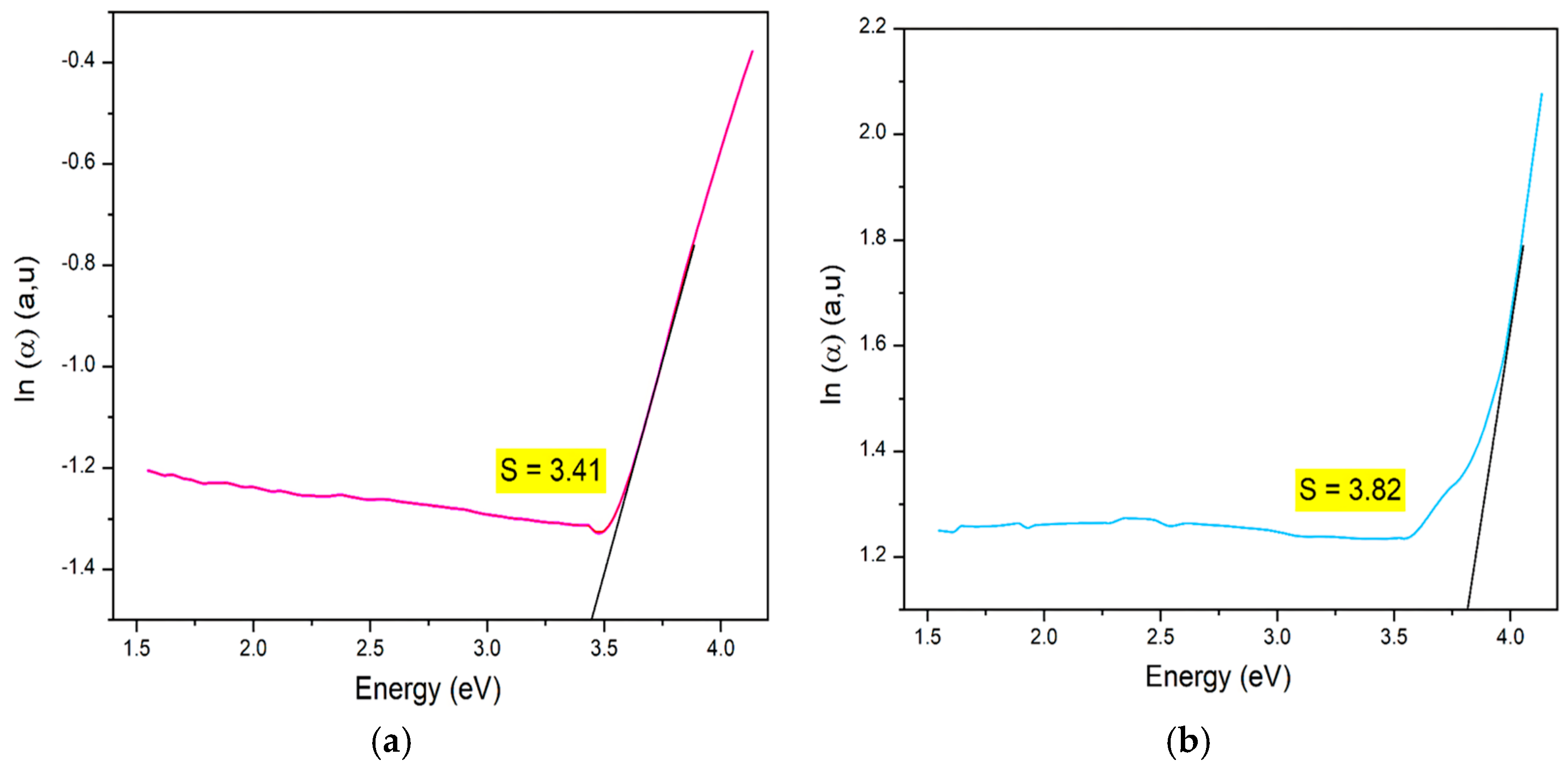
4.4.2. UV–Vis Absorbance Spectroscopy

5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ben Kamri, A.L.; Fadla, M.A.; Lefkaier, I.K.; Messaoud, C.L.B.; Kanoun, M.B.; Goumri-Said, S. AI-driven ensemble learning for accurate Seebeck coefficient prediction in half-Heusler compounds based on chemical formulas. Comput. Condens. Matter 2024, 40, e00923. [Google Scholar] [CrossRef]
- Touati, S.; Benghia, A.; Hebboul, Z.; Lefkaier, I.K.; Kanoun, M.B.; Goumri-Said, S. Predictive machine learning approaches for perovskites properties using their chemical formula: Towards the discovery of stable solar cells materials. Neural Comput. Appl. 2024, 36, 16319–16329. [Google Scholar] [CrossRef]
- Benghia, A.; Mechraoui, B.K.; Ferchane, S.; Lefkaier, I.K.; Fadla, M.A.; Hebboul, Z.; Arar, R.; Kanoun, M.B.; Goumri-Said, S. Data driven enhancement of mid-infrared non-linear optical properties of quaternary and ternary chalcogenides. Optik 2023, 293, 171432. [Google Scholar] [CrossRef]
- Touati, S.; Benghia, A.; Hebboul, Z.; Lefkaier, I.K.; Kanoun, M.B.; Goumri-Said, S. Machine Learning Models for Efficient Property Prediction of ABX3 Materials: A High-Throughput Approach. ACS Omega 2024, 9, 47519–47531. [Google Scholar] [CrossRef]
- Tao, Q.; Xu, P.; Li, M.; Lu, W. Machine learning for perovskite materials design and discovery. Npj Comput. Mater. 2021, 7, 23. [Google Scholar] [CrossRef]
- Zeng, Z.; Xu, Y.; Zhang, Z.; Gao, Z.; Luo, M.; Yin, Z.; Zhang, C.; Xu, J.; Huang, B.; Luo, F.; et al. Rare-earth-containing perovskite nanomaterials: Design, synthesis, properties and applications. Chem. Soc. Rev. 2020, 49, 1109–1143. [Google Scholar] [CrossRef]
- Jonker, G.H.; Van Santen, J.H. Ferromagnetic compounds of manganese with perovskite structure. Physica 1950, 16, 337–349. [Google Scholar] [CrossRef]
- Tian, H.; Zheng, W.; Zhao, Z.; Ding, T.; Yu, S.; Zheng, B.; Li, X.; Meng, F.; Jiang, Q. Magnetic properties and electron spin resonance of charge ordering manganite Y0.5Ca0.5MnO3. Chem. Phys. Lett. 2005, 401, 585–589. [Google Scholar] [CrossRef]
- Von Helmolt, R.; Wecker, J.; Holzapfel, B.; Schultz, L.; Samwer, K. Giant negative magnetoresistance in perovskitelike La 2/3 Ba 1/3 MnO x ferromagnetic films. Phys. Rev. Lett. 1993, 71, 2331–2333. [Google Scholar] [CrossRef]
- Salamon, M.B.; Jaime, M. The physics of manganites: Structure and transport. Rev. Mod. Phys. 2001, 73, 583–628. [Google Scholar] [CrossRef]
- Zener, C. Interaction Between the d Shells in the Transition Metals. Phys. Rev. 1951, 81, 440–444. [Google Scholar] [CrossRef]
- Shankar, U.; Singh, A.K. Origin of Suppression of Charge Ordering Transition in Nanocrystalline Ln 0.5 Ca 0.5 MnO 3 (Ln = La, Nd, Pr) Ceramics. J. Phys. Chem. C 2015, 119, 28620–28630. [Google Scholar] [CrossRef]
- Koriba, I.; Lagoun, B.; Guibadj, A.; Belhadj, S.; Ameur, A.; Cheriet, A. Structural, electronic, magnetic and mechanical properties of three LaMnO3 phases: Theoretical investigations. Comput. Condens. Matter 2021, 29, e00592. [Google Scholar] [CrossRef]
- De Gennes, P.-G. Effects of Double Exchange in Magnetic Crystals. Phys. Rev. 1960, 118, 141–154. [Google Scholar] [CrossRef]
- Zener, C. Interaction between the d -Shells in the Transition Metals. II. Ferromagnetic Compounds of Manganese with Perovskite Structure. Phys. Rev. 1951, 82, 403–405. [Google Scholar] [CrossRef]
- Millis, A.J.; Littlewood, P.B.; Shraiman, B.I. Double Exchange Alone Does Not Explain the Resistivity of La1-xSrxMnO3. Phys. Rev. Lett. 1995, 74, 5144–5147. [Google Scholar] [CrossRef]
- Millis, A.J.; Mueller, R.; Shraiman, B.I. Fermi-liquid-to-polaron crossover. II. Double exchange and the physics of colossal magnetoresistance. Phys. Rev. B 1996, 54, 5405–5417. [Google Scholar] [CrossRef]
- Cheng, S.; Xu, C.; Deng, S.; Han, M.-G.; Bao, S.; Ma, J.; Nan, C.; Duan, W.; Bellaiche, L.; Zhu, Y.; et al. Interface reconstruction with emerging charge ordering in hexagonal manganite. Sci. Adv. 2018, 4, eaar4298. [Google Scholar] [CrossRef]
- Jaiswar, S.; Mandal, K.D. Evidence of Enhanced Oxygen Vacancy Defects Inducing Ferromagnetism in Multiferroic CaMn 7 O 12 Manganite with Sintering Time. J. Phys. Chem. C 2017, 121, 19586–19601. [Google Scholar] [CrossRef]
- Morelli, D.T.; Mance, A.M.; Mantese, J.V.; Micheli, A.L. Magnetocaloric properties of doped lanthanum manganite films. J. Appl. Phys. 1996, 79, 373–375. [Google Scholar]
- Gschneidner, K.A.; Pecharsky, V.K.; Tsokol, A.O. Recent developments in magnetocaloric materials. Rep. Prog. Phys. 2005, 68, 1479–1539. [Google Scholar] [CrossRef]
- Phan, M.-H.; Yu, S.-C. Review of the magnetocaloric effect in manganite materials. J. Magn. Magn. Mater. 2007, 308, 325–340. [Google Scholar]
- Abuova, A.U.; Mastrikov, Y.A.; Kotomin, E.A.; Piskunov, S.N.; Inerbaev, T.M.; Akilbekov, A.T. First-Principles Modeling of Oxygen Adsorption on Ag-Doped LaMnO3 (001) Surface. J. Elec Mater. 2020, 49, 1421–1434. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, X. Predicting AsxSe1-x Glass Transition Onset Temperature. Int. J. Thermo phys. 2020, 41, 149. [Google Scholar] [CrossRef]
- Ito, D.; Fujimura, N.; Yoshimura, T.; Ito, T. Ferroelectric properties of YMnO3 epitaxial films for ferroelectric-gate field-effect transistors. J. Appl. Phys. 2003, 93, 5563–5567. [Google Scholar] [CrossRef]
- Bowen, M.; Maurice, J.L.; Barthélémy, A.; Bibes, M.; Imhoff, D.; Bellini, V.; Bertacco, R.; Wortmann, D.; Seneor, P.; Jacquet, E. Using half-metallic manganite interfaces to reveal insights into spintronicsnics. J. Phys. Condens. Matter 2007, 19, 315208. [Google Scholar] [CrossRef]
- Han, H.; Song, S.; Lee, J.H.; Kim, K.J.; Kim, G.W.; Park, T.; Jang, H.M. Switchable Photovoltaic Effects in Hexagonal Manganite Thin Films Having Narrow Band Gaps. Chem. Mater. 2015, 27, 7425–7432. [Google Scholar] [CrossRef]
- Lonkai, T.; Hohlwein, D.; Ihringer, J.; Prandl, W. The magnetic structures of YMnO3-δ and HoMnO3. Appl. Phys. A 2002, 74, s843–s845. [Google Scholar] [CrossRef]
- Chadli, A.; Halit, M.; Lagoun, B.; Mohamedi, F.; Maabed, S.; Cheriet, A.; Hlil, E.; Farh, H. Structural and Anisotropic Elastic Properties of Hexagonal YMnO3 in Low Symmetry Determined by First-Principles Calculations. Solid State Phenom. 2019, 297, 120–130. [Google Scholar] [CrossRef]
- Schiffer, P.; Ramirez, A.P.; Bao, W.; Cheong, S.-W. Low Temperature Magnetoresistance and the Magnetic Phase Diagram of La1-xCaxMnO3. Phys. Rev. Lett. 1995, 75, 3336–3339. [Google Scholar] [CrossRef]
- Mahesh, R.; Mahendiran, R.; Raychaudhuri, A.K.; Rao, C.N.R. Effect of particle size on the giant magnetoresistance of La0.7Ca0.3MnO3. Appl. Phys. Lett. 1996, 68, 2291–2293. [Google Scholar] [CrossRef]
- Siwach, P.K.; Goutam, U.K.; Srivastava, P.; Singh, H.K.; Tiwari, R.S.; Srivastava, O.N. Colossal magnetoresistance study in nanophasic La0.7Ca0.3MnO3 manganite. J. Phys. D Appl. Phys. 2005, 39, 14. [Google Scholar] [CrossRef][Green Version]
- Dey, P.; Nath, T.K. Effect of grain size modulation on the magneto- and electronic-transport properties of La0.7Ca0.3MnO3 nanoparticles: The role of spin-polarized tunneling at the enhanced grain surface. Phys. Rev. B 2006, 73, 214425. [Google Scholar] [CrossRef]
- López-Quintela, M.A.; Hueso, L.E.; Rivas, J.; Rivadulla, F. Intergranular magnetoresistance in nanomanganites. Nanotechnology 2003, 14, 212–219. [Google Scholar] [CrossRef]
- Venkataiah, G.; Krishna, D.C.; Vithal, M.; Rao, S.S.; Bhat, S.V.; Prasad, V.; Subramanyam, S.V.; Reddy, P.V. Effect of sintering temperature on electrical transport properties of La0.67Ca0.33MnO3. Phys. B Condens. Matter 2005, 357, 370–379. [Google Scholar] [CrossRef]
- Shankar, K.S.; Kar, S.; Subbanna, G.N.; Raychaudhuri, A.K. Enhanced ferromagnetic transition temperature in nanocrystalline lanthanum calcium manganese oxide (La0.67Ca0.33MnO3). Solid State Commun. 2004, 129, 479–483. [Google Scholar] [CrossRef]
- Ferreira, M.C.; Pimentel, B.; Andrade, V.; Zverev, V.; Gimaev, R.R.; Pomorov, A.S.; Pyatakov, A.; Alekhina, Y.; Komlev, A.; Makarova, L.; et al. Understanding the Dependence of Nanoparticles Magnetothermal Properties on Their Size for Hyperthermia Applications: A Case Study for La-Sr Manganites. Nanomaterials 2021, 11, 1826. [Google Scholar] [CrossRef]
- Pashchenko, A.V.; Liedienov, N.A.; Fesych, I.V.; Li, Q.; Pitsyuga, V.G.; Turchenko, V.A.; Pogrebnyak, V.G.; Liu, B.; Levchenko, G.G. Smart magnetic nanopowder based on the manganite perovskite for local hyperthermia. RSC Adv. 2020, 10, 30907–30916. [Google Scholar] [CrossRef]
- Ahmad, A.; Akbar, H.; Zada, I.; Anjum, F.; Afzal, A.M.; Javed, S.; Muneeb, M.; Ali, A.; Choi, J.R. Improvement of the Self-Controlled Hyperthermia Applications by Varying Gadolinium Doping in Lanthanum Strontium Manganite Nanoparticles. Molecules 2023, 28, 7860. [Google Scholar] [CrossRef]
- Sánchez, R.D.; Rivas, J.; Vázquez-Vázquez, C.; López-Quintela, A.; Causa, M.T.; Tovar, M.; Oseroff, S. Giant magnetoresistance in fine particle of La0.67Ca0.33MnO3 synthesized at low temperatures. Appl. Phys. Lett. 1996, 68, 134–136. [Google Scholar] [CrossRef]
- Siwach, P.K.; Singh, H.K.; Srivastava, O.N. Low field magnetotransport in manganites. J. Phys. Condens. Matter 2008, 20, 273201. [Google Scholar] [CrossRef]
- Yang, H.; Zhu, Y.H.; Xian, T.; Jiang, J.L. Synthesis and magnetocaloric properties of La0.7Ca0.3MnO3 nanoparticles with different sizes. J. Alloys Compd. 2013, 555, 150–155. [Google Scholar] [CrossRef]
- Hueso, L.E.; Sande, P.; Miguéns, D.R.; Rivas, J.; Rivadulla, F.; López-Quintela, M.A. Tuning of the magnetocaloric effect in δ nanoparticles synthesized by sol–gel techniques. J. Appl. Phys. 2002, 91, 9943–9947. [Google Scholar] [CrossRef]
- Lampen, P.; Puri, A.; Phan, M.-H.; Srikanth, H. Structure, magnetic, and magnetocaloric properties of amorphous and crystalline La0.4Ca0.6MnO3+δ nanoparticles. J. Alloys Compd. 2012, 512, 94–99. [Google Scholar] [CrossRef]
- Siwach, P.K.; Prasad, R.; Gaur, A.; Singh, H.K.; Varma, G.D.; Srivastava, O.N. Microstructure-magnetotransport correlation in La0.7Ca0.3MnO3. J. Alloys Compd. 2007, 443, 26–31. [Google Scholar] [CrossRef]
- Kumar, S.; Dwivedi, G.D.; Kumar, S.; Mathur, R.B.; Saxena, U.; Ghosh, A.K.; Joshi, A.G.; Yang, H.D.; Chatterjee, S. Structural, transport and optical properties of (La0.6Pr0.4)0.65Ca0.35MnO3 nanocrystals: A wide band-gap magnetic semiconductor. Dalton Trans. 2015, 44, 3109–3117. [Google Scholar] [CrossRef]
- Karikar, A.; Ahmed, A.; Das, K.; Das, I. Magnetic and magnetocaloric properties of polycrystalline Pr1− xCaxMnO3 (x= 0.85, 0.90, 0.95) compounds: Emergence of large inverse and conventional magnetocaloric effects. J. Magn. Magn. Mater. 2023, 587, 171334. [Google Scholar] [CrossRef]
- Jammalamadaka, S.N.; Rao, S.S.; Bhat, S.V.; Vanacken, J.; Moshchalkov, V.V. Magnetocaloric effect and nature of magnetic transition in nanoscale Pr0.5Ca0.5MnO3. J. Appl. Phys. 2012, 112, 083917. [Google Scholar] [CrossRef]
- Rao, S.S.; Bhat, S.V. Probing the existing magnetic phases in Pr0.5Ca0.5MnO3 (PCMO) nanowires and nanoparticles: Magnetization and magneto-transport investigations. J. Phys. Condens. Matter 2010, 22, 116004. Available online: https://www.osti.gov/etdeweb/biblio/21375830 (accessed on 3 June 2024).
- Li, Q.; Wang, H.S.; Hu, Y.F.; Wertz, E. Anomalous anisotropic magnetoresistance in Pr0.67Sr0.33MnO3 thin films. J. Appl. Phys. 2000, 87, 5573–5575. [Google Scholar] [CrossRef]
- Liu, J.-M.; Yuan, G.L.; Sang, H.; Wu, Z.C.; Chen, X.Y.; Liu, Z.G.; Du, Y.W.; Huang, Q.; Ong, C.K. Low-field magnetoresistance in nanosized La 0.7 Sr 0.3 MnO 3/Pr 0.5 Sr 0.5 MnO 3 composites. Appl. Phys. Lett. 2001, 78, 1110–1112. [Google Scholar] [CrossRef]
- Zhou, X.Z.; Kunkel, H.P.; Zhao, J.H.; Stampe, P.A.; Williams, G. Evidence for an enhanced magnetoresistance accompanying a continuous phase transition in semiconducting La 0.67 Mg 0.33 MnO 3. Phys. Rev. B 1997, 56, R12714–R12717. [Google Scholar] [CrossRef]
- Li, Y.; Duan, X.F.; Zhang, J.H.; Wang, H.R.; Qian, Y.T.; Huang, Z.; Zhou, J.; Yuan, S.L.; Liu, W.; Zhu, C.F. Giant magnetoresistance in bulk La0.6Mg0.4MnO3. J. Mater. Res. 1997, 12, 2648–2650. Available online: https://www.osti.gov/biblio/554347 (accessed on 3 June 2024). [CrossRef]
- Selmi, R.; Cherif, W.; Sarabando, A.R.; Ferreira, N.M.; Ktari, L. Enhanced relative cooling power of lanthanum-deficiency manganites La0.77−xMg0.23MnO3 (0 ≤ x ≤ 0.2): Structural, magnetic and magnetocaloric properties. J. Mater. Sci. Mater. Electron. 2022, 33, 1703–1723. [Google Scholar] [CrossRef]
- Liu, L.; Xia, Z.C.; Yuan, S.L. Effect of Mg doping on the transport properties and magnetoresistance of La2/3Ca1/3MnO3 prepared in low temperature. Mater. Sci. Eng. B 2006, 128, 50–52. [Google Scholar] [CrossRef]
- Supelano, G.I.; Barón-González, A.J.; Santos, A.S.; Ortíz, C.; Gómez, J.A.M.; Vargas, C.A.P. Effect of Mg addition on LaMnO3 ceramic system. J. Mater. Res. Technol. 2018, 7, 77–81. [Google Scholar] [CrossRef]
- Tian, C.-S.; Chen, X.-L.; Ni, J.; Liu, J.-M.; Zhang, D.-K.; Huang, Q.; Zhao, Y.; Zhang, X.-D. Transparent conductive Mg and Ga co-doped ZnO thin films for solar cells grown by magnetron sputtering: H2 induced changes. Sol. Energy Mater. Sol. Cells 2014, 125, 59–65. [Google Scholar] [CrossRef]
- Kang, H.; Lu, Z.; Zhong, Z.; Gu, J. Structural, optical and electrical characterization of Ga-Mg co-doped ZnO transparent conductive films. Mater. Lett. 2018, 215, 102–105. [Google Scholar] [CrossRef]
- Panda, B.; Routray, K.L.; Behera, D. Studies on conduction mechanism and dielectric properties of the nano-sized La0.7Ca0.3MnO3 (LCMO) grains in the paramagnetic state. Phys. B Condens. Matter 2020, 583, 411967. [Google Scholar] [CrossRef]
- Wang, Y.X.; Du, Y.; Qin, R.W.; Han, B.; Du, J.; Lin, J.H. Phase equilibrium of the La–Ca–Mn–O system. J. Solid State Chem. 2001, 156, 237–241. [Google Scholar]
- Jirák, Z.; Krupička, S.; Šimša, Z.; Dlouhá, M.; Vratislav, S. Neutron diffraction study of Pr1- xCaxMnO3 perovskites. J. Magn. Magn. Mater. 1985, 53, 153–166. [Google Scholar] [CrossRef]
- Jirak, Z.; Krupička, S.; Nekvasil, V.; Pollert, E.; Villeneuve, G.; Zounova, F. Structural and magnetization study of Pr1- xCaxMnO3. J. Magn. Magn. Mater. 1980, 15, 519–520. [Google Scholar]
- Kaduk, J.A. A Rietveld tutorial—Mullite. Powder Diffr. 2009, 24, 351–361. [Google Scholar] [CrossRef]
- Canchanya-Huaman, Y.; Mayta-Armas, A.F.; Pomalaya-Velasco, J.; Bendezú-Roca, Y.; Guerra, J.A.; Ramos-Guivar, J.A. Strain and Grain Size Determination of CeO2 and TiO2 Nanoparticles: Comparing Integral Breadth Methods versus Rietveld, ?-Raman, and TEM. Nanomaterials 2021, 11, 2311. [Google Scholar] [CrossRef]
- Last, J.T. Infrared-Absorption Studies on Barium Titanate and Related Materials. Phys. Rev. 1957, 105, 1740–1750. [Google Scholar] [CrossRef]
- Perry, C.H. Far infrared reflectance spectra and dielectric dispersion of a variety of materials having the perovskite and related structures. Jpn. J. Appl. Phys. 1964, 3, 564. [Google Scholar] [CrossRef]
- Hunt, G.R.; Perry, C.H.; Ferguson, J. Far-Infrared Reflectance and Transmittance of Potassium Magnesium Fluoride and Magnesium Fluoride. Phys. Rev. 1964, 134, A688–A691. [Google Scholar] [CrossRef]
- Gao, F.; Lewis, R.A.; Wang, X.L.; Dou, S.X. Far-infrared reflection and transmission of La1- xCaxMnO3. J. Alloys Compd. 2002, 347, 314–318. [Google Scholar] [CrossRef]
- Lewis, R.A. Phonon modes in CMR manganites at elevated temperatures. J. Supercond. Inc. Nov. Magn. 2001, 14, 143–148. [Google Scholar] [CrossRef]
- Williams, Q.; Jeanloz, R. Melting relations in the iron-sulfur system at ultra-high pressures: Implications for the thermal state of the Earth. J. Geophys. Res. 1990, 95, 19299–19310. [Google Scholar] [CrossRef]
- Kim, K.H.; Gu, J.Y.; Choi, H.S.; Park, G.W.; Noh, T.W. Frequency Shifts of the Internal Phonon Modes in La 0.7 Ca 0.3 Mn O 3. Phys. Rev. Lett. 1996, 77, 1877–1880. [Google Scholar] [CrossRef]
- Kusuma, A.K.; Kumar, K.V.; Raghavender, A.T. Optical and Magnetic Properties of Chromium Doped Pr0.5Sr0.5MnO3 System. Int. J. Mech. Eng. 2022, 13, 290–299. [Google Scholar]
- Kusuma, A.K. Tunable Optical Bandgap of Chromium Doped Nd0. 5sr0. 5mno3 System Ashok Kumar Kusuma, Katrapally Vijaya Kumar* and At Raghavender. Stochastic Modeling. Available online: https://www.researchgate.net/profile/Jhansi-Rani-M-R-2/publication/382643050_Overconfidence_Bias_Finance_Risk_Taking_Attitude_and_Mutual_Fund_Investment_Decision/links/66a786c9de060e4c7e671254/Overconfidence-Bias-Finance-Risk-Taking-Attitude-and-Mutual-Fund-Investment-Decision.pdf#page=124 (accessed on 7 December 2024).
- Mishra, S.K.; Kumari, D.; Gupta, B.D. Surface plasmon resonance-based fiber optic ammonia gas sensor using ITO and polyaniline. Sens. Actuators B Chem. 2012, 171–172, 976–983. [Google Scholar] [CrossRef]
- Wu, X. High-efficiency polycrystalline CdTe thin-film solar cells. Sol. Energy 2004, 77, 803–814. [Google Scholar] [CrossRef]
- Mishra, B.; Nanda, J.; Munisha, B.; Parida, C. Transition metal ion doped Mg-Zn manganites for optoelectronic device applications. Mater. Lett. 2024, 369, 136741. [Google Scholar] [CrossRef]
- H-e, M.M.S.; Alsobhi, B.O. Consequences of Tuning Rare-Earth RE3+-Site and Exchange–Correlation Energy U on the Optoelectronic, Mechanical, and Thermoelectronic Properties of Cubic Manganite Perovskites REMnO3 for Spintronics and Optoelectronics Applications. ACS Omega 2022, 7, 27903–27917. [Google Scholar] [CrossRef]
- Yoo, J.; Lee, J.; Kim, S.; Yoon, K.; Park, I.J.; Dhungel, S.; Karunagaran, B.; Mangalaraj, D.; Yi, J. High transmittance and low resistive ZnO: Al films for thin film solar cells. Thin Solid Film. 2005, 480, 213–217. [Google Scholar] [CrossRef]
- Arshad, M.; Abushad, M.; Husain, S.; Khan, W. Investigation of Structural, Optical and Electrical Transport Properties of Yttrium Doped La0.7Ca0.3MnO3 Perovskites. Electron. Mater. Lett. 2020, 16, 321–331. [Google Scholar] [CrossRef]
- Kharrat, A.B.J.; Khirouni, K.; Boujelben, W. Electrical and optical analysis of Pr0.5−xGdxSr0.5MnO3 (x = 0, 0.05, and 0.1) manganite compounds prepared via solid-state process. Ionics 2024, 30, 1209–1222. [Google Scholar] [CrossRef]
- Hassanien, A.S.; Akl, A.A. Effect of Se addition on optical and electrical properties of chalcogenide CdSSe thin films. Superlattices Microstruct. 2016, 89, 153–169. [Google Scholar] [CrossRef]
- Kesavulu, C.; Kim, H.; Lee, S.; Kaewkhao, J.; Wantana, N.; Kaewnuam, E.; Kothan, S.; Kaewjaeng, S. Spectroscopic investigations of Nd3+ doped gadolinium calcium silica borate glasses for the NIR emission at 1059 nm. J. Alloys Compd. 2017, 695, 590–598. [Google Scholar] [CrossRef]
- Raddaoui, Z.; El Kossi, S.; Smiri, B.; Al-Shahrani, T.; Dhahri, J.; Belmabrouk, H. Raman scattering and red emission of Mn4+ in La0.7Sr0.25Na0.05Mn0.7Ti0.3O3 manganite phosphor for LED applications. RSC Adv. 2020, 10, 23615–23623. [Google Scholar] [CrossRef]


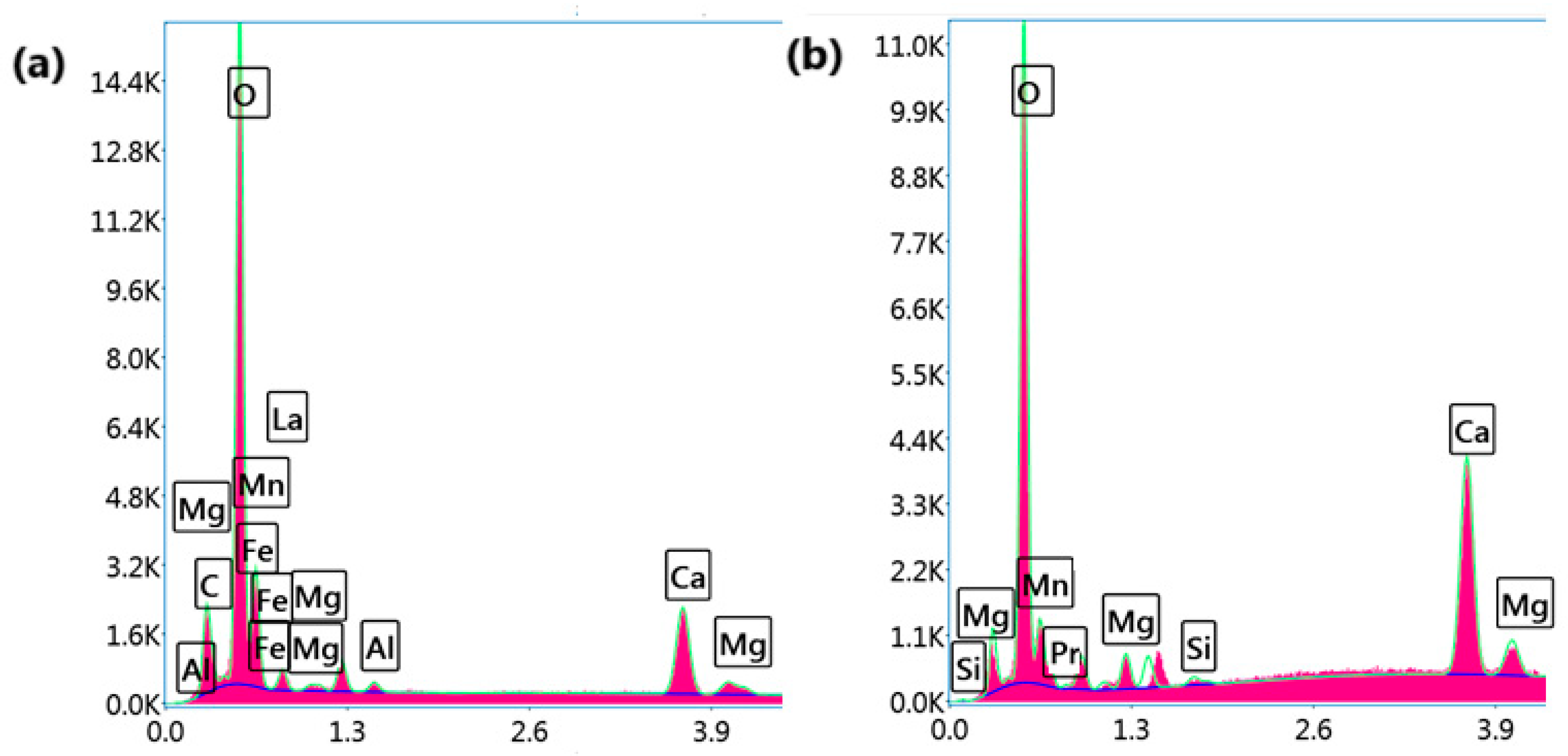

| At. % | La | Pr | Ca | Mg | Mn |
|---|---|---|---|---|---|
| LCMMO | 4.44 | / | 2.75 | 2.10 | 7.31 |
| PCMMO | / | 7.38 | 4.36 | 3.83 | 13.69 |
| PCMMO | Pos. [°2Th.] | 32.843 | 40.4961 | 47.095 | 58.585 |
| FWHM (β) [°2Th.] | 0.2047 | 0.2558 | 0.2578 | 0.3581 | |
| D (nm) | 42.00 | 33.09 | 33.87 | 25.43 | |
| Dmoy (nm) | 32.59 | ||||
| LCMMO | Pos. [°2Th.] | 33.056 | 40.762 | 47.419 | 58.993 |
| FWHM (β) [°2Th.] | 0.30708 | 0.3581 | 0.5628 | 0.6140 | |
| D (nm) | 27.8 | 23.66 | 15.41 | 14.86 | |
| Dmoy (nm) | 20.43 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ben Messaoud, C.L.; Hebboul, Z.; Lefkaier, I.K.; Draoui, A.; Ben Kamri, A.L.; Goumri-Said, S.; Kanoun, M.B.; Silva, R.S., Jr.; Alonso, J.A.; Laurent, S. Unlocking the Potential of Mg-Doped Rare Earth Manganites: Machine Learning-Guided Synthesis and Insights into Structural and Optical Properties. Nanomaterials 2025, 15, 561. https://doi.org/10.3390/nano15070561
Ben Messaoud CL, Hebboul Z, Lefkaier IK, Draoui A, Ben Kamri AL, Goumri-Said S, Kanoun MB, Silva RS Jr., Alonso JA, Laurent S. Unlocking the Potential of Mg-Doped Rare Earth Manganites: Machine Learning-Guided Synthesis and Insights into Structural and Optical Properties. Nanomaterials. 2025; 15(7):561. https://doi.org/10.3390/nano15070561
Chicago/Turabian StyleBen Messaoud, Chikh Lakhdar, Zoulikha Hebboul, Ibn Khaldoun Lefkaier, Ahmed Draoui, Ahmed Lamine Ben Kamri, Souraya Goumri-Said, Mohammed Benali Kanoun, Romualdo S. Silva, Jr., José A. Alonso, and Sophie Laurent. 2025. "Unlocking the Potential of Mg-Doped Rare Earth Manganites: Machine Learning-Guided Synthesis and Insights into Structural and Optical Properties" Nanomaterials 15, no. 7: 561. https://doi.org/10.3390/nano15070561
APA StyleBen Messaoud, C. L., Hebboul, Z., Lefkaier, I. K., Draoui, A., Ben Kamri, A. L., Goumri-Said, S., Kanoun, M. B., Silva, R. S., Jr., Alonso, J. A., & Laurent, S. (2025). Unlocking the Potential of Mg-Doped Rare Earth Manganites: Machine Learning-Guided Synthesis and Insights into Structural and Optical Properties. Nanomaterials, 15(7), 561. https://doi.org/10.3390/nano15070561









