Pushing the Limits of Thermal Resistance in Nanocomposites: A Comparative Study of Carbon Black and Nanotube Modifications
Abstract
1. Introduction
2. Materials
3. Methods
3.1. Material Properties
3.2. Thermal Resistance
4. Results and Discussion
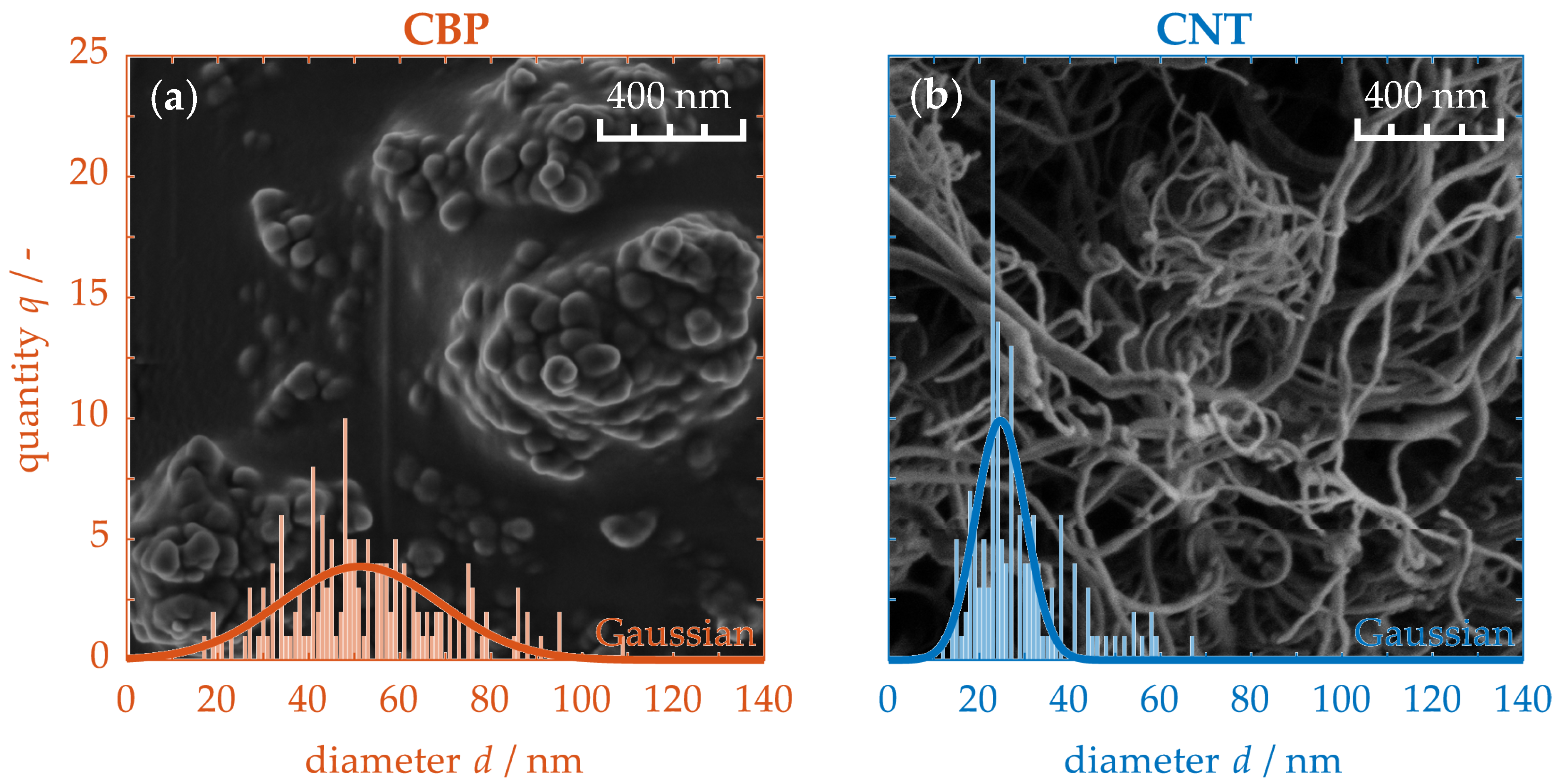
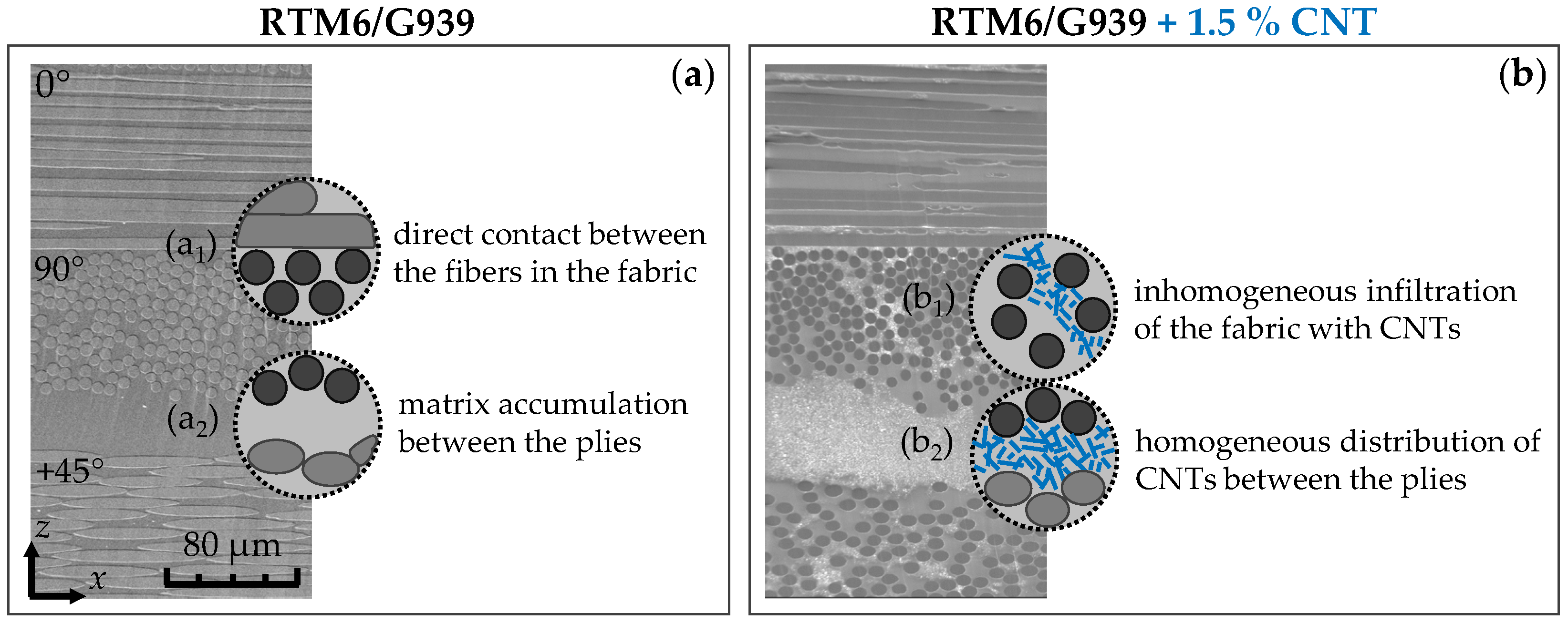
4.1. Morphology of the Nanoparticles
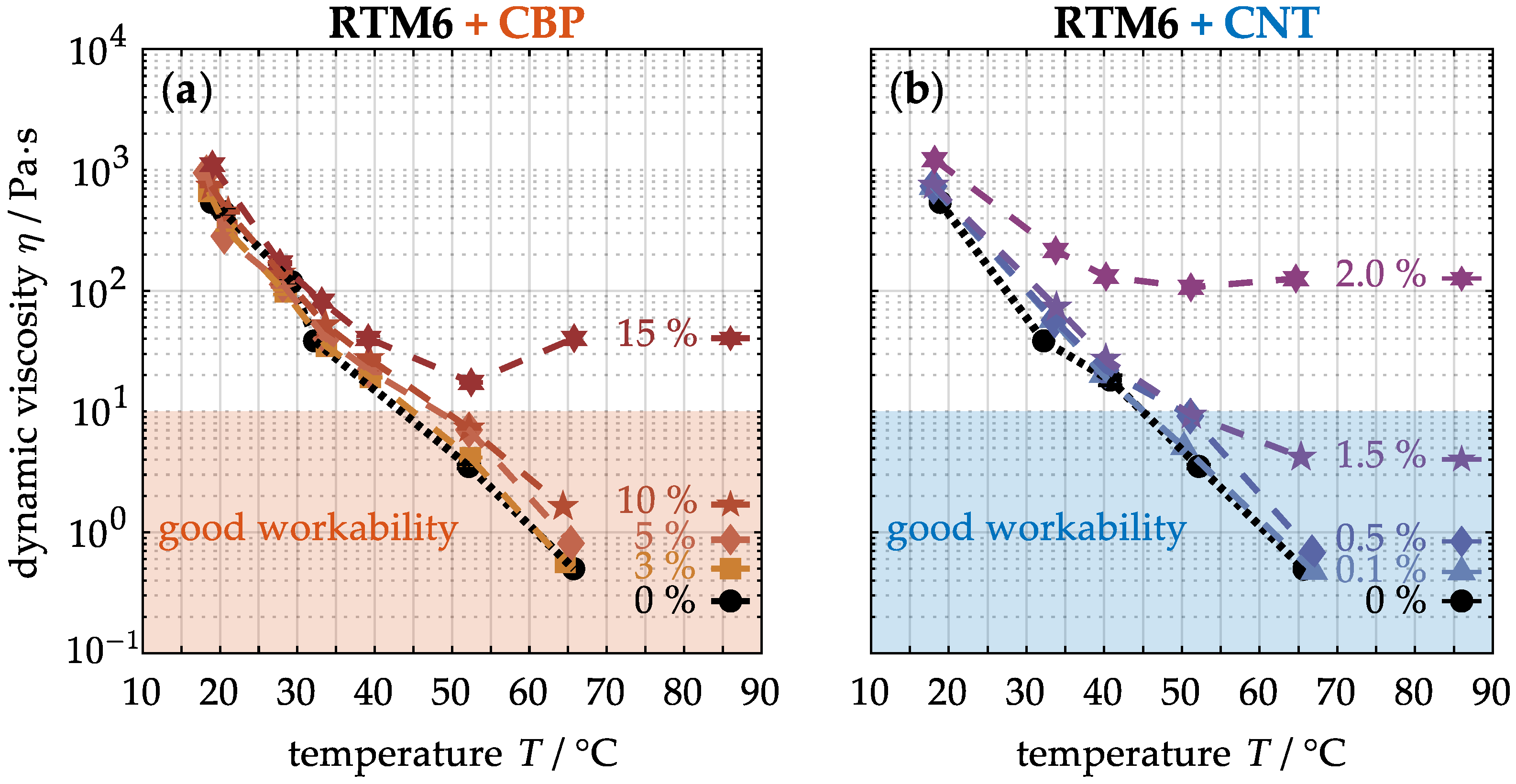
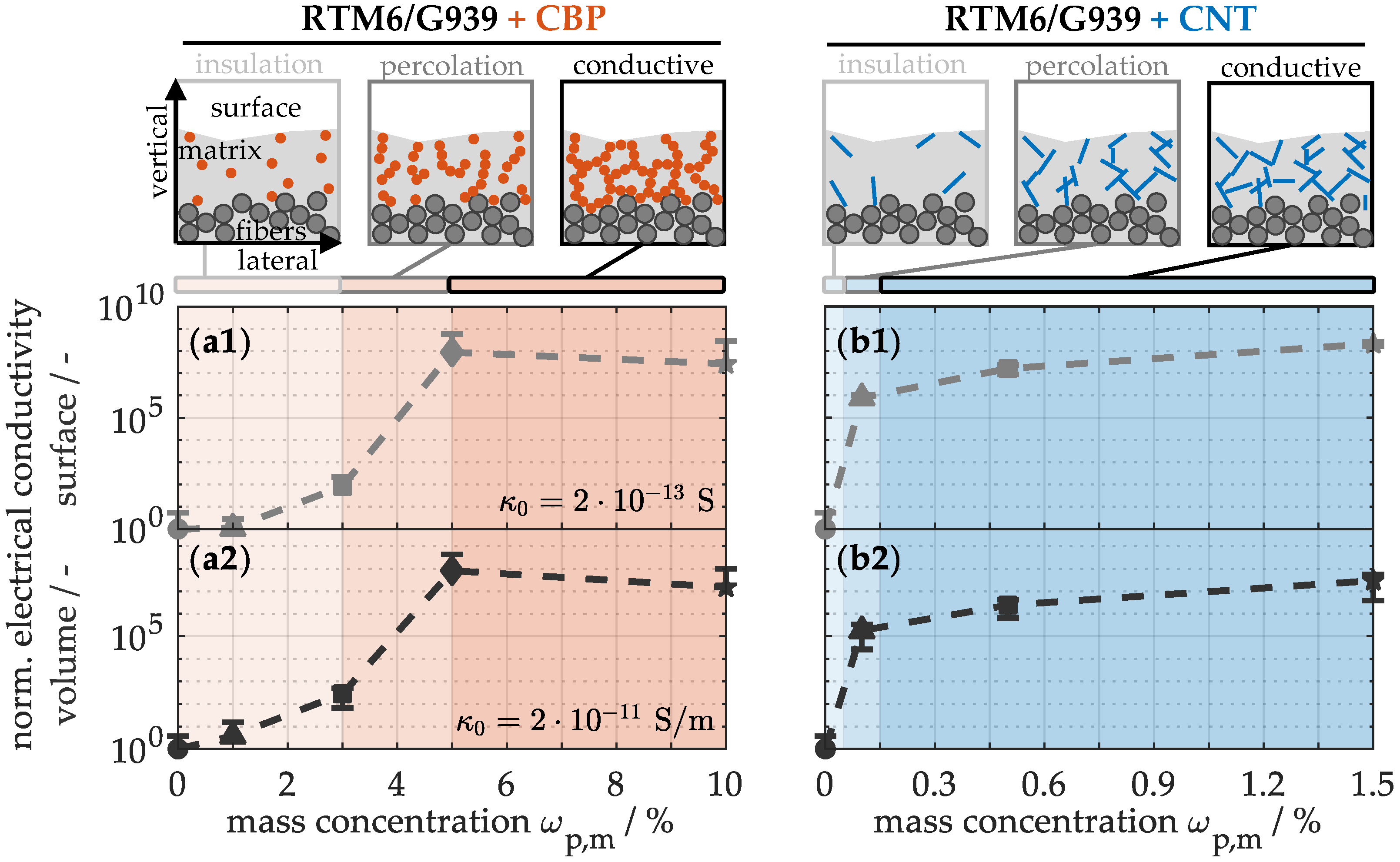
4.2. Characterization of the Nanocomposites
4.2.1. Transport and Energy Properties
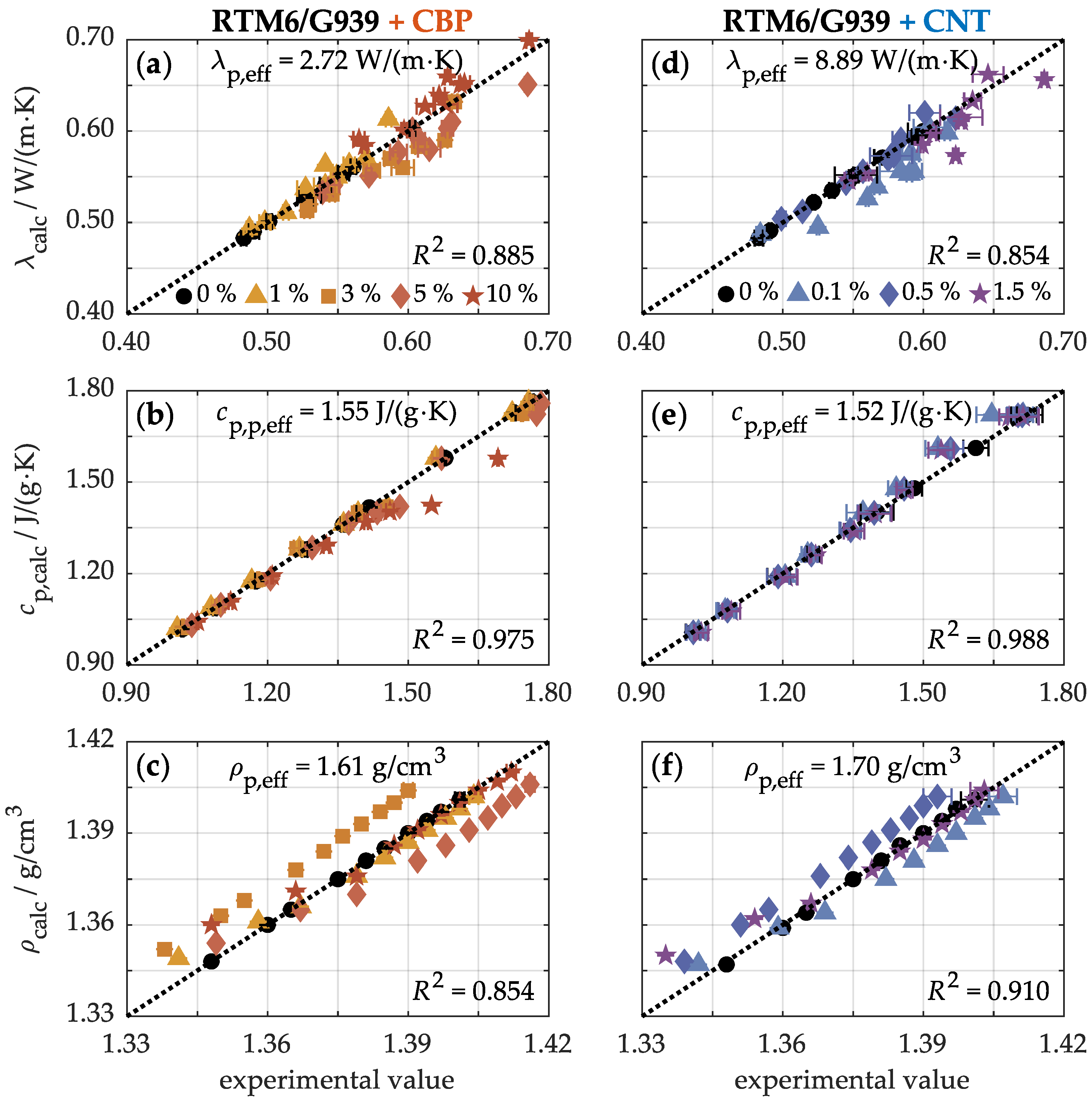
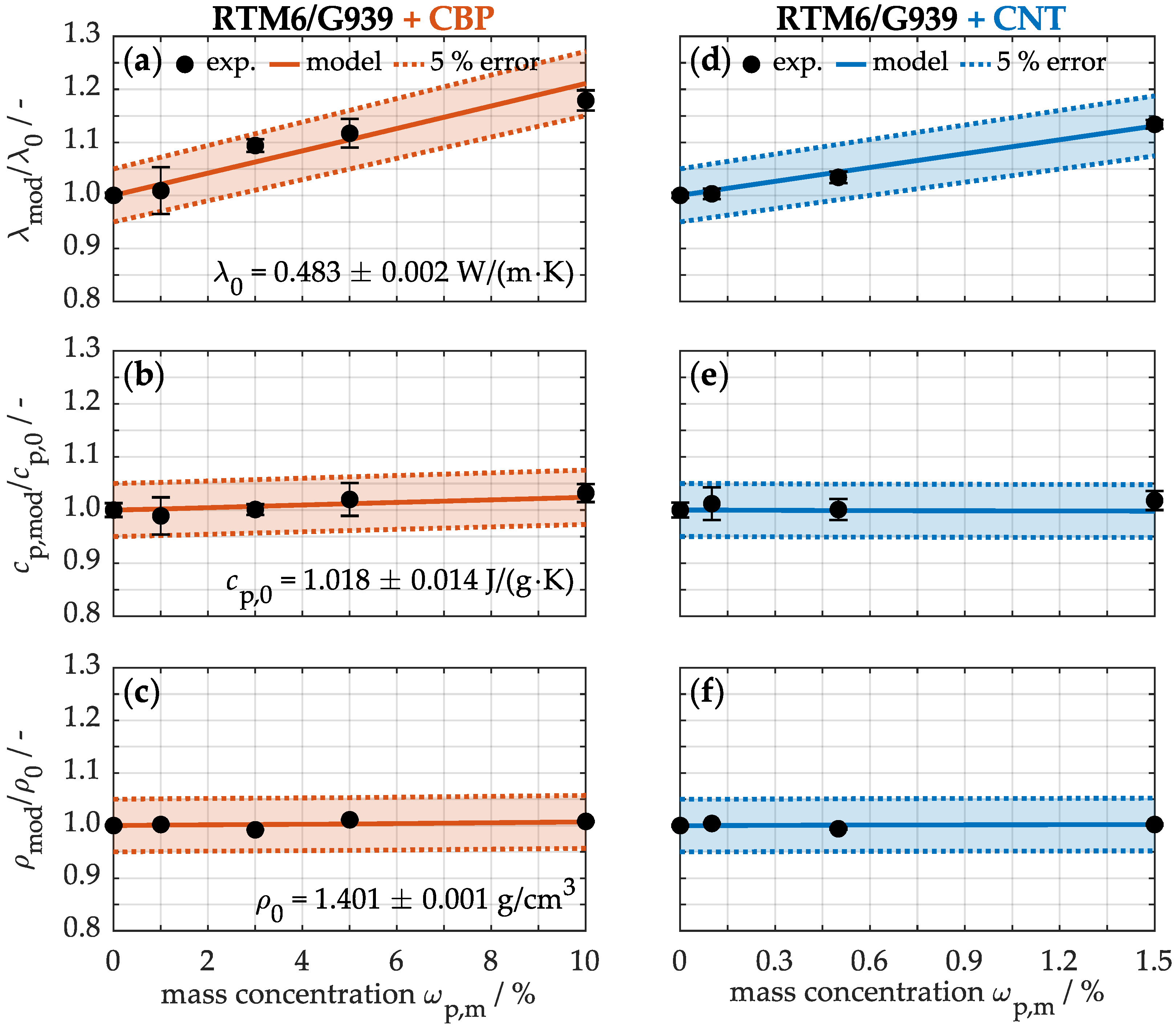
4.2.2. Modeling of Thermal Properties
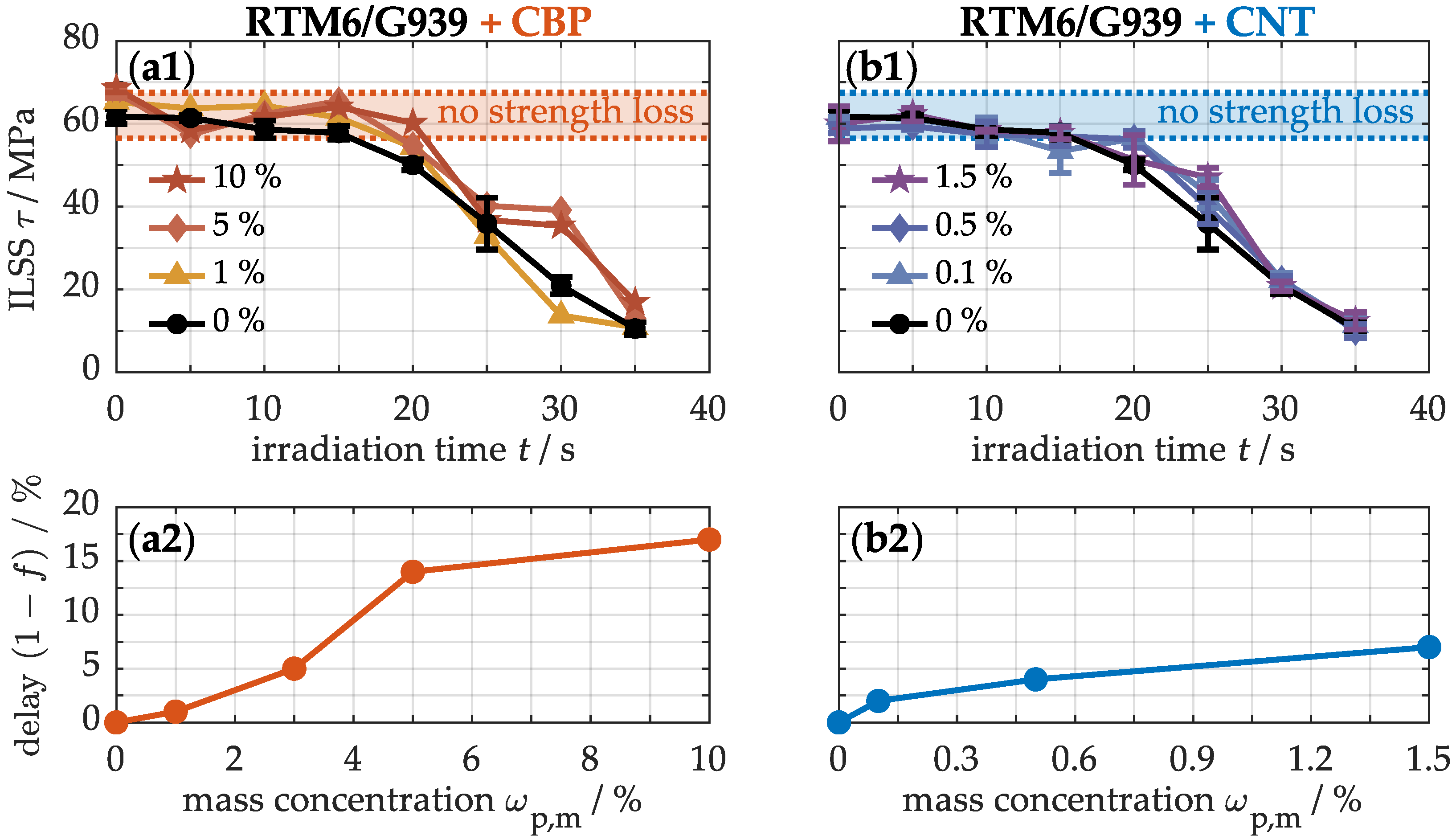
4.2.3. Mechanical Properties
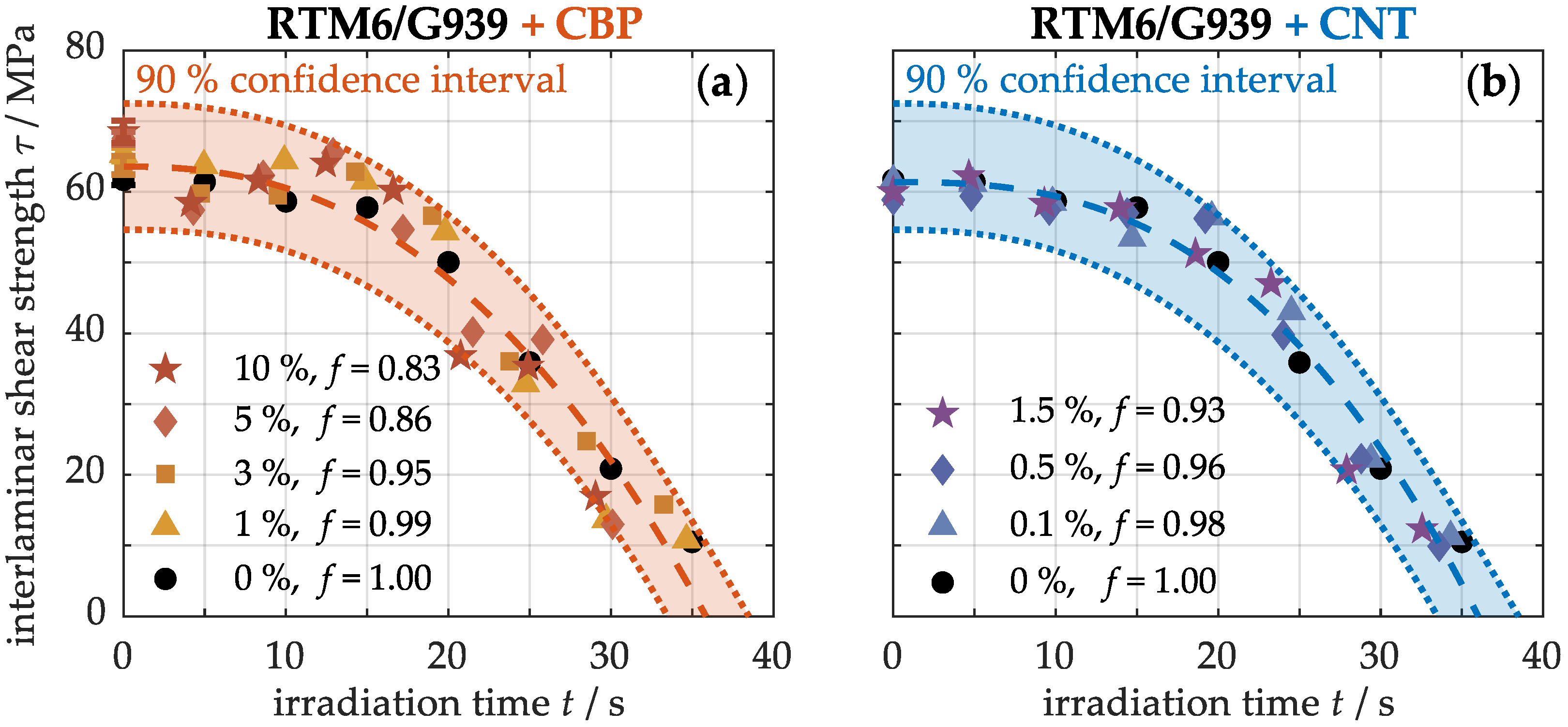
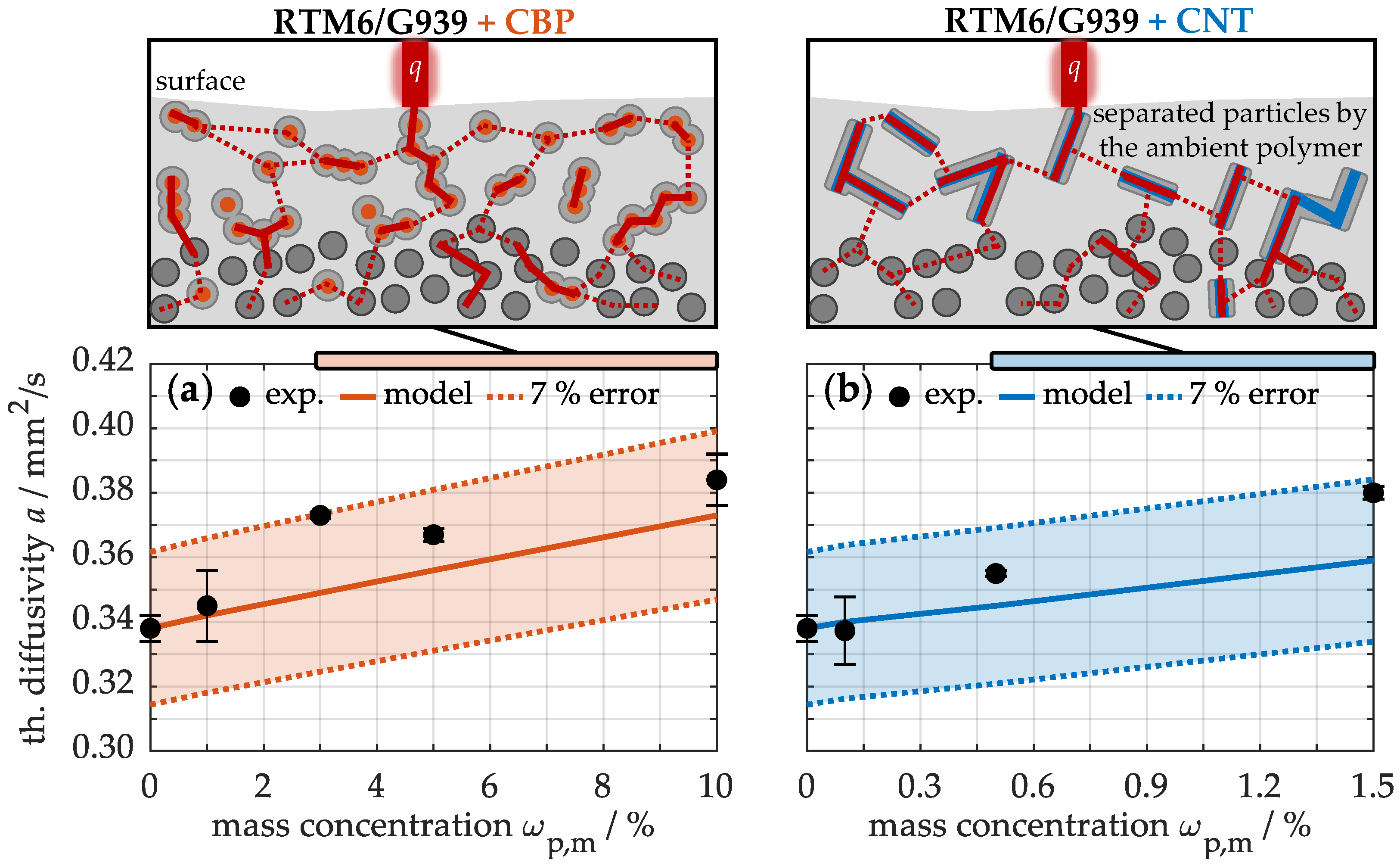
4.3. Thermal Resistance of the Modified Materials
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CBP | Carbon black particle |
| CFRP | Carbon fiber-reinforced polymer |
| CNT | Carbon nanotube |
| ILSS | Interlaminar shear strength |
| MBDA | 4,4′-Methylenebis(2,6-diethylaniline) |
| MBIMA | 4,4′-Methylenebis(2-isopropyl-6-methylaniline) |
| SEM | Scanning electron microscope |
| TGDDM | Tetraglycidyl-4,4′-diaminodiphenylmethane |
Appendix A
References
- Lengsfeld, H.; Wolff-Fabris, F.; Krämer, J.; Lacalle, J.; Altstädt, V. Faserverbundwerkstoffe: Prepregs und Ihre Verarbeitung, 1st ed.; Carl Hanser Verlag GmbH & Co. KG: Munich, Germany, 2016; ISBN 9783446433007. [Google Scholar]
- Schürmann, H. Konstruieren mit Faser-Kunststoff-Verbunden, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2007; ISBN 9783540721895. [Google Scholar] [CrossRef]
- Mallick, P.K. Fiber-Reinforced Composites: Materials, Manufacturing, and Design, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2007; ISBN 9780849342059. [Google Scholar] [CrossRef]
- AVK—Industrievereinigung Verstärkte Kunststoffe e.V. Handbuch Faserverbundkunststoffe/Composites: Grundlagen, Verarbeitung, Anwendungen, 4th ed.; Springer Vieweg: Wiesbaden, Germany, 2013; ISBN 9783658027544. [Google Scholar] [CrossRef]
- Støren, S.; Moe, P.T. Extrusion. In Handbook of Aluminum: Physical Metallurgy and Processes, 1st ed.; Totten, G.E., MacKenzie, D.S., Eds.; Marcel Dekker: New York, NY, USA, 2003; pp. 385–480. [Google Scholar] [CrossRef]
- Ostermann, F. Anwendungstechnologie Aluminium, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2014; ISBN 9783662438060. [Google Scholar] [CrossRef]
- Ehrenstein, G.W. Faserverbund-Kunststoffe: Werkstoffe—Verarbeitung—Eigenschaften, 2nd ed.; Carl Hanser Verlag GmbH & Co. KG: Munich, Germany, 2006; ISBN 9783446227163. [Google Scholar] [CrossRef]
- Gandhi, U.; Goris, S.; Osswald, T.A. Discontinuous Fiber-Reinforced Composites: Fundamentals and Applications, 1st ed.; Carl Hanser Verlag GmbH & Co. KG: Munich, Germany, 2020; ISBN 9781569906941. [Google Scholar]
- Eurofighter Jagdflugzeug GmbH. Eurofighter Typhoon: Technical Guide. 2013. Available online: https://www.eurofighter.com (accessed on 27 April 2020).
- Zhang, J.; Lin, G.; Vaidya, U.; Wang, H. Past, present and future prospective of global carbon fibre composite developments and applications. Compos. Part B Eng. 2023, 250, 110463. [Google Scholar] [CrossRef]
- Sreejith, M.; Rajeev, R.S. Fiber reinforced composites for aerospace and sports applications. In Fiber Reinforced Composites, 1st ed.; Kuruvilla, J., Kristiina, O., Gejo, G., Runcy, W., Saritha, A., Eds.; Woodhead Publishing: Sawston, UK, 2021; pp. 821–859. [Google Scholar] [CrossRef]
- Kim, I.Y. Battlefield Flame/Thermal Threats or Hazards and Thermal Performance Criteria. Report, U.S. Army Soldier and Biological Chemical Command. 2000. Available online: https://discover.dtic.mil (accessed on 2 April 2022).
- Maurente, A.; Alves, C.G. Radiation heat transfer in a gas slab with properties characteristics of a jet engine combustor. Int. J. Heat Mass Transf. 2019, 145, 118734. [Google Scholar] [CrossRef]
- Gong, T.; Xie, Q.; Huang, X. Fire behaviors of flame-retardant cables part I: Decomposition, swelling and spontaneous ignition. Fire Saf. J. 2018, 95, 113–121. [Google Scholar] [CrossRef]
- Spinner, N.S.; Field, C.R.; Hammond, M.H.; Williams, B.A.; Myers, K.M.; Lubrano, A.L.; Rose-Pehrsson, S.L.; Tuttle, S.G. Physical and chemical analysis of lithium-ion battery cell-to-cell failure events inside custom fire chamber. J. Power Sources 2015, 279, 713–721. [Google Scholar] [CrossRef]
- McAllister, J.L.; Carpenter, D.J.; Roby, R.J.; Purser, D. The Importance of Autopsy and Injury Data in the Investigation of Fires. Fire Technol. 2013, 50, 1357–1377. [Google Scholar] [CrossRef]
- Schartel, B.; Hull, T.R. Development of fire-retarded materials—Interpretation of cone calorimeter data. Fire Mater. 2007, 31, 327–354. [Google Scholar] [CrossRef]
- McLean, A.D. Burns and military clothing. J. R. Army Med. Corps 2001, 147, 97–106. [Google Scholar] [CrossRef]
- Wang, Y.; Zhupanska, O.I. Lightning strike thermal damage model for glass fiber reinforced polymer matrix composites and its application to wind turbine blades. Compos. Struct. 2015, 132, 1182–1191. [Google Scholar] [CrossRef]
- Dorofeev, S.B.; Sidorov, V.P.; Efimenko, A.A.; Kochurko, A.S.; Kuznetsov, M.S.; Chaivanov, B.B.; Matsukov, D.I.; Pereverzev, A.K.; Avenyan, V.A. Fireballs from deflagration and detonation of heterogeneous fuel-rich clouds. Fire Saf. J. 1995, 25, 323–336. [Google Scholar] [CrossRef]
- Rajic, D.; Kamberovic, Z.; Karkalic, R.; Ivankovic, N.; Senic, Z. Thermal resistance testing of standard and protective filtering military garment on the burning napalm mixture. Hem. Ind. 2013, 67, 941–950. [Google Scholar] [CrossRef]
- Eibl, S. Damage by Improvised Incendiary Devices on Carbon Fiber-Reinforced Polymer Matrix Composites. J. Compos. Sci. 2021, 5, 72. [Google Scholar] [CrossRef]
- Nestor, O.H. Heat Intensity and Current Density Distributions at the Anode of High Current, Inert Gas Arcs. J. Appl. Phys. 1962, 33, 1638–1648. [Google Scholar] [CrossRef]
- Satheesh Chandran, M.; Sunitha, K.; Uday Bhaskar, N.; Santhosh Kumar, K.S.; Sreenivas, N.; Mathew, D. Low Density Syntactic Foam Composites as Ablative TPS Material for High Heat Flux Conditions for Reentry Missions. Trans. Indian Natl. Acad. Eng. 2021, 6, 153–158. [Google Scholar] [CrossRef]
- Tanaka, M.; Terasaki, H.; Ushio, M. Effect of Anode Heat Transfer on Melted Penetration in Welding Process by Free-burning Argon Arc. ISIJ Int. 2002, 42, 1005–1009. [Google Scholar] [CrossRef]
- Larrimbe, L.; Pettinà, M.; Nikbin, K.; Jones, E.L.; Katz, A.P.; Hawkins, C.J.; DeCerbo, J.; Brown, P.; Vandeperre, L.J.; White, M.A. High Heat Flux Laser Testing of HfB2 Cylinders. J. Am. Ceram. Soc. 2016, 100, 293–303. [Google Scholar] [CrossRef]
- Wolfrum, J.; Eibl, S.; Oeltjen, E.; Osterholz, J.; Wickert, M. High-energy laser effects on carbon fiber reinforced polymer composites with a focus on perforation time. J. Compos. Mater. 2021, 55, 2249–2262. [Google Scholar] [CrossRef]
- Baker, A.; Dutton, S.; Kelly, D. Composite Materials for Aircraft Structures, 2nd ed.; American Institute of Aeronautics & Astronautics: Reston, VA, USA, 2004; ISBN 9781563475405. [Google Scholar]
- Hopmann, C.; Greif, H.; Wolters, L. Technologie der Kunststoffe: Lern- und Arbeitsbuch für die Aus- und Weiterbildung, 5th ed.; Carl Hanser Verlag GmbH & Co. KG: Munich, Germany, 2021; ISBN 9783446467521. [Google Scholar] [CrossRef]
- Sorina, T.; Gunyaev, G. Structural carbon-fibre-reinforced plastics and their properties. In Polymer Matrix Composites, 1st ed.; Shalin, R.E., Ed.; Springer: Dordrecht, The Netherlands, 1995; ISBN 9789401042291. [Google Scholar] [CrossRef]
- Bibinger, J.; Eibl, S.; Gudladt, H.J. Influence of Low and Extreme Heat Fluxes on Thermal Degradation of Carbon Fibre-reinforced Polymers. Appl. Compos. Mater. 2022, 29, 1817–1840. [Google Scholar] [CrossRef]
- Hexcel Corporation. HexFlow® RTM 6 180 °C Mono-Component Epoxy System for Resin Transfer Moulding and Infusion Technologies: Product Data. 2016. Available online: https://www.hexcel.com (accessed on 3 November 2022).
- Bibinger, J.; Eibl, S.; Gudladt, H.J. Ply-Resolved Quantification of Thermal Degradation in Carbon Fibre-reinforced Polymers. J. Compos. Mater. 2023, 57, 1057–1072. [Google Scholar] [CrossRef]
- Vetter, T.M.; Bibinger, J.; Zimmer, F.; Eibl, S.; Gudladt, H.J. Characterization of one-sided thermal damage of carbon fiber reinforced polymers by means of depth profiles. J. Compos. Mater. 2020, 54, 3699–3713. [Google Scholar] [CrossRef]
- Schuster, T.J.; Eibl, S.; Gudladt, H.J. Influence of carbon nanotubes on thermal response and reaction to fire properties of carbon fibre-reinforced plastic material. J. Compos. Mater. 2017, 52, 567–579. [Google Scholar] [CrossRef]
- Weil, E.D.; Levchik, S.V. Flame Retardants for Plastics and Textiles: Practical Applications, 2nd ed.; Carl Hanser Verlag GmbH & Co. KG: Munich, Germany, 2015; ISBN 9781569905791. [Google Scholar] [CrossRef]
- Greiner, L.; Kukla, P.; Eibl, S.; Doring, M. Phosphorus Containing Polyacrylamides as Flame Retardants for Epoxy-Based Composites in Aviation. Polymers 2019, 11, 284. [Google Scholar] [CrossRef] [PubMed]
- Jianjun, L.; Yuxiang, O. Theory of Flame Retardation of Polymeric Materials, 1st ed.; De Gruyter: Berlin, Germany, 2019; ISBN 9783110349351. [Google Scholar] [CrossRef]
- Eyerer, P.; Schüle, H. Polymer Engineering 1: Einführung, Synthese, Eigenschaften, 2nd ed.; Springer Vieweg: Berlin/Heidelberg, Germany, 2020; ISBN 9783662598375. [Google Scholar] [CrossRef]
- Kandola, B.K.; Kandare, E. Composites having improved fire resistance. In Advances in Fire Retardant Materials; Horrocks, A., Price, D., Eds.; Woodhead Publishing: Sawston, UK, 2008; pp. 398–442. [Google Scholar] [CrossRef]
- Tabaka, W.; Meinel, D.; Schartel, B. Sacrifice Few to Save Many: Fire Protective Interlayers in Carbon-Fiber-Reinforced Laminates. ACS Omega 2024, 9, 23703–23712. [Google Scholar] [CrossRef] [PubMed]
- Tabaka, W.; Timme, S.; Lauterbach, T.; Medina, L.; Berglund, L.A.; Carosio, F.; Duquesne, S.; Schartel, B. Bench-scale fire stability testing—Assessment of protective systems on carbon fibre reinforced polymer composites. Polym. Test. 2021, 102, 107340. [Google Scholar] [CrossRef]
- Eibl, S. Reaction-to-fire properties of polymer matrix composites with integrated intumescent barriers. Fire Mater. 2014, 39, 658–674. [Google Scholar] [CrossRef]
- Eibl, S. Quantification of a thermal pre-load on polymer matrix composites by the degradation and colour change of polyurethane top coats. Mater. Werkst. 2016, 47, 388–399. [Google Scholar] [CrossRef]
- Eibl, S.; Wolfrum, J. Prospects to separately estimate temperature and duration of a thermal pre-load on a polymer matrix composite. J. Compos. Mater. 2012, 47, 3011–3025. [Google Scholar] [CrossRef]
- Hamdi, K.; Aboura, Z.; Harizi, W.; Khellil, K. Improvement of the electrical conductivity of carbon fiber reinforced polymer by incorporation of nanofillers and the resulting thermal and mechanical behavior. J. Compos. Mater. 2017, 52, 1495–1503. [Google Scholar] [CrossRef]
- Eibl, S. Observing Inhomogeneity of Plastic Components in Carbon Fiber Reinforced Polymer Materials by ATR-FTIR Spectroscopy in the Micrometer Scale. J. Compos. Mater. 2008, 42, 1231–1246. [Google Scholar] [CrossRef]
- Orion Engineered Carbons. Printex® L6: Safety Data Sheet. 2022. Available online: https://www.orioncarbons.com (accessed on 27 January 2023).
- Camacho, M.d.C.; Galao, O.; Baeza, F.; Zornoza, E.; Garcés, P. Mechanical Properties and Durability of CNT Cement Composites. Materials 2014, 7, 1640–1651. [Google Scholar] [CrossRef]
- DIN 53019-1; Viskosimetrie—Messung von Viskositäten und Fließkurven mit Rotationsviskosimetern—Teil 1: Grundlagen und Messgeometrie. Beuth Verlag GmbH: Berlin, Germany, 2008.
- Echlin, P. Handbook of Sample Preparation for Scanning Electron Microscopy and X-Ray Microanalysis, 1st ed.; Springer: New York, NY, USA, 2009; ISBN 9780387857305. [Google Scholar] [CrossRef]
- Ul-Hamid, A. A Beginners’ Guide to Scanning Electron Microscopy, 1st ed.; Springer Nature: Cham, Switzerland, 2018; ISBN 9783319984810. [Google Scholar] [CrossRef]
- DIN EN 61340-2-3; Elektrostatik—Prüfverfahren zur Bestimmung des Widerstandes und des Spezifischen Widerstandes von Festen Werkstoffen, die zur Vermeidung Elektrostatischer Aufladung Verwendet Werden. Beuth Verlag GmbH: Berlin, Germany, 2017. (In German)
- DIN EN ISO 22007-4; Kunststoffe—Bestimmung der Wärmeleitfähigkeit und der Temperaturleitfähigkeit—Teil 4: Laserblitzverfahren. German version; Beuth Verlag GmbH: Berlin, Germany, 2017.
- DIN EN ISO 1183-1; Kunststoffe—Verfahren zur Bestimmung der Dichte von Nicht Verschäumten Kunststoffen—Teil 1: Eintauchverfahren, Verfahren mit Flüssigkeitspyknometer und Titrationsverfahren. Beuth Verlag GmbH: Berlin, Germany, 2019. (In German)
- DIN EN 2563; Kohlenstofffaserverstärkte Kunststoffe—Unidirektionale Laminate—Bestimmung der Scheinbaren Interlaminaren Scherfestigkeit. Beuth Verlag GmbH: Berlin, Germany, 1997. (In German)
- DIN EN ISO 14126; Faserverstärkte Kunststoffe—Bestimmung der Druckeigenschaften in der Laminatebene. Beuth Verlag GmbH: Berlin, Germany, 2000. (In German)
- DIN EN ISO 527-4; Kunststoffe—Bestimmung der Zugeigenschaften—Teil 4: Prüfbedingungen für Isotrope und Orthotrope Faserverstärkte Kunststoffverbundwerkstoffe. Beuth Verlag GmbH: Berlin, Germany, 1997. (In German)
- Brown, S.C.; Robert, C.; Koutsos, V.; Ray, D. Methods of modifying through-thickness electrical conductivity of CFRP for use in structural health monitoring, and its effect on mechanical properties— A review. Compos. Part A Appl. Sci. Manuf. 2020, 133, 105885. [Google Scholar] [CrossRef]
- Han, Z.; Fina, A. Thermal conductivity of carbon nanotubes and their polymer nanocomposites: A review. Prog. Polym. Sci. 2011, 36, 914–944. [Google Scholar] [CrossRef]
- Bigg, D.M. Thermal conductivity of heterophase polymer compositions. In Thermal and Electrical Conductivity of Polymer Materials. Advances in Polymer Science; Springer: Berlin/Heidelberg, Germany, 1995; Volume 119, pp. 1–30. ISBN 9783540490159. [Google Scholar] [CrossRef]
- Rahaman, M.; Khastgir, D.; Aldalbahi, A.K. Carbon-Containing Polymer Composites; Springer: Singapore, 2019; ISBN 9789811326875. [Google Scholar] [CrossRef]
- Fourier, J. The Analytical Theory of Heat, 1st ed.; Cambridge University Press: Cambridge, UK, 2009. Original work published 1878; ISBN 9781108001786. [Google Scholar]
- Riande, E.; Diaz-Calleja, R.; Prolongo, M.; Masegosa, R.; Salom, C. Polymer Viscoelasticity: Stress and Strain in Practice, 1st ed.; CRC Press Inc.: Boca Raton, FL, USA, 1999; ISBN 9780429175534. [Google Scholar] [CrossRef]
- Mouritz, A.P.; Gibson, A.G. Fire Properties of Polymer Composite Materials, 1st ed.; Springer: Dordrecht, The Netherlands, 2006; ISBN 9781402053566. [Google Scholar] [CrossRef]
- Dao, B.; Hodgkin, J.; Krstina, J.; Mardel, J.; Tian, W. Accelerated aging versus realistic aging in aerospace composite materials. II. Chemistry of thermal aging in a structural composite. J. Appl. Polym. Sci. 2006, 102, 3221–3232. [Google Scholar] [CrossRef]
- Levchik, S.V.; Weil, E.D. Thermal decomposition, combustion and flame-retardancy of epoxy resins—A review of the recent literature. Polym. Int. 2004, 53, 1901–1929. [Google Scholar] [CrossRef]

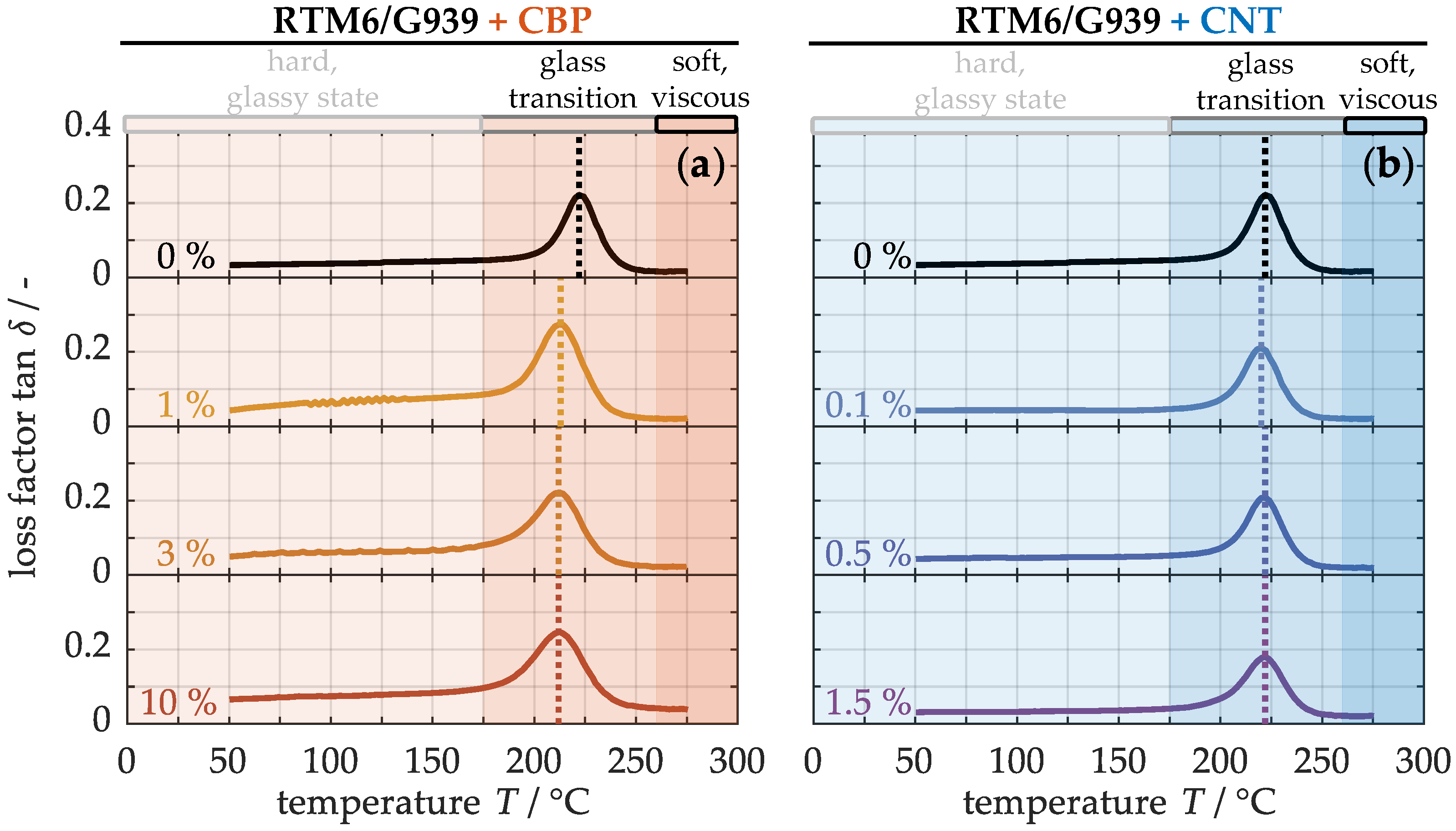
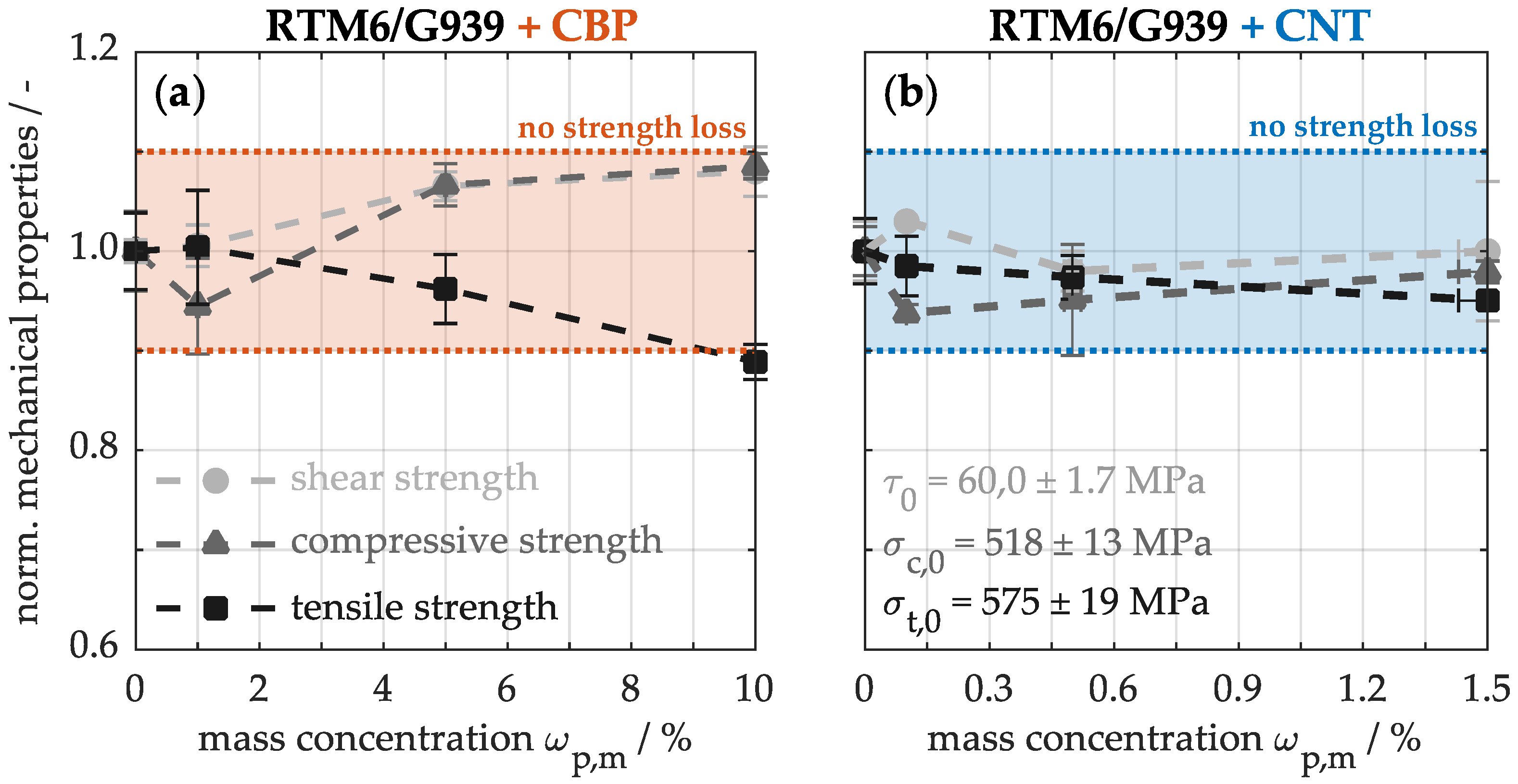

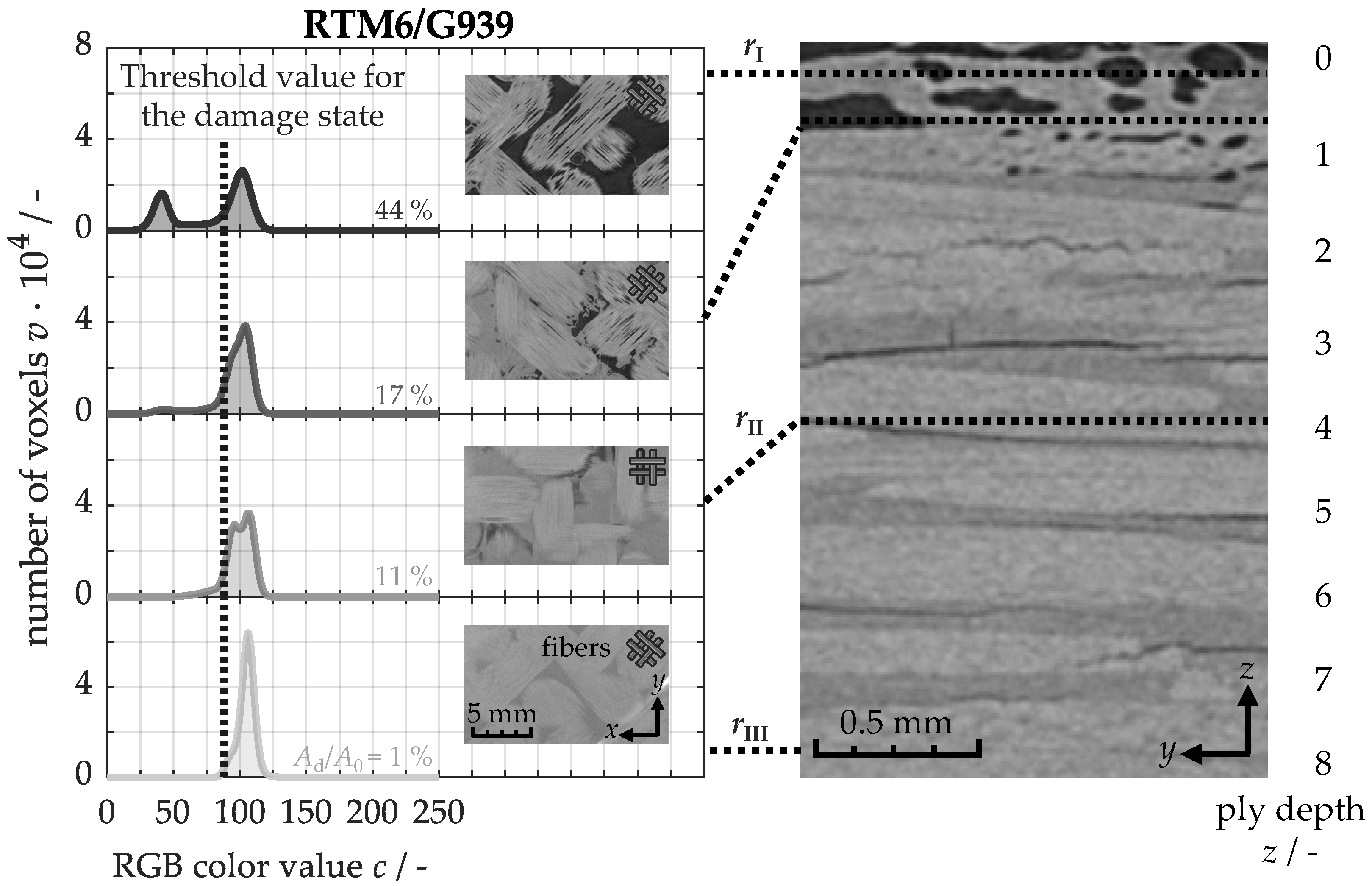


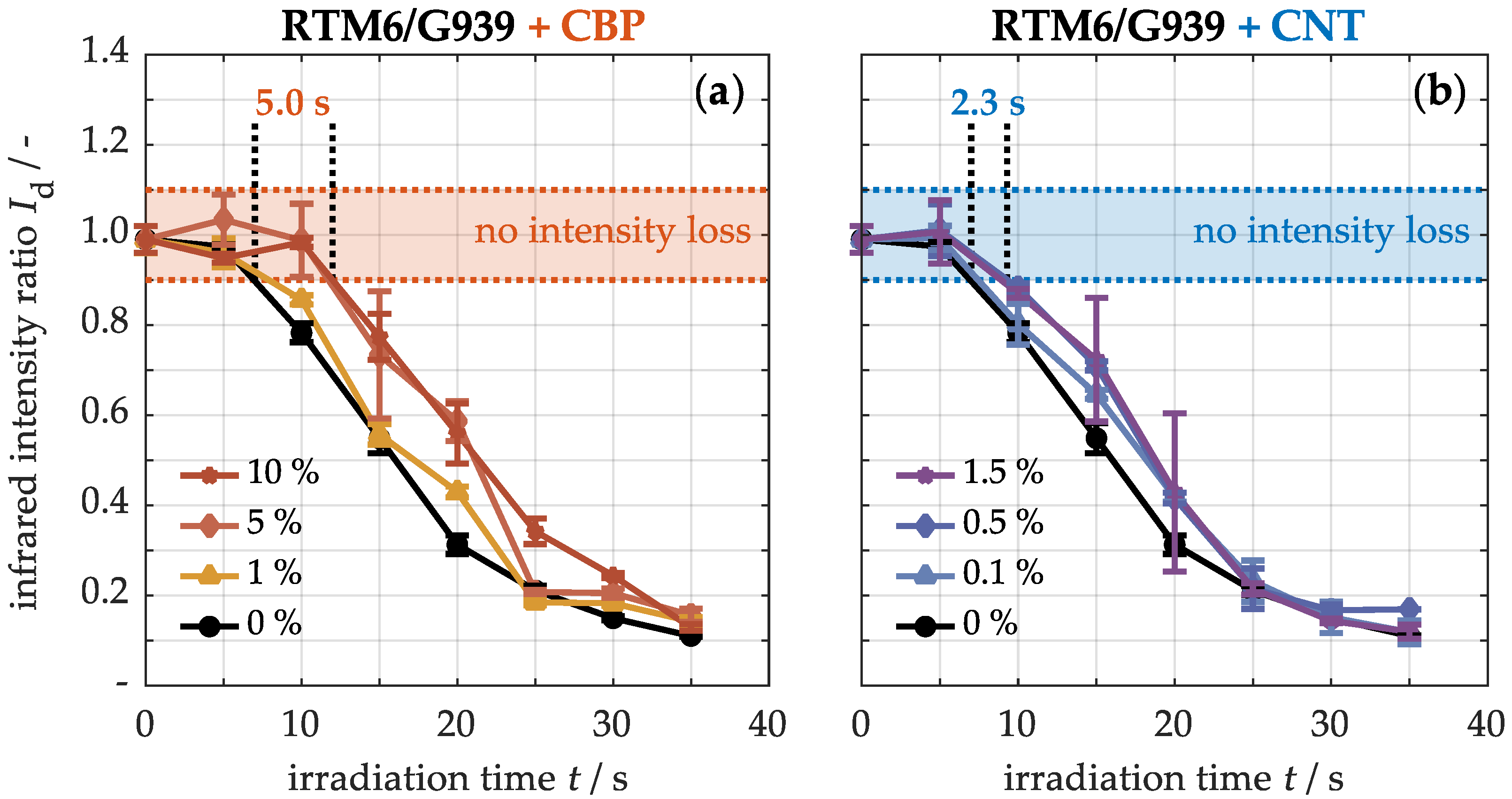
| Effective Thermal Properties | CFRP | CBP | CNT |
|---|---|---|---|
| Thermal Conductivity/W/(m·K) | 0.483 ± 0.002 | 2.72 ± 0.14 | 8.89 ± 0.44 |
| Specific Heat Capacity/J/(g·K) | 1.018 ± 0.014 | 1.55 ± 0.08 | 1.52 ± 0.08 |
| Density/g/cm3 | 1.401 ± 0.001 | 1.61 ± 0.08 | 1.70 ± 0.09 |
| Thermal Diffusivity/mm2/s | 0.338 ± 0.004 | 1.09 ± 0.05 | 3.44 ± 0.17 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bibinger, J.; Eibl, S.; Gudladt, H.-J.; Schartel, B.; Höfer, P. Pushing the Limits of Thermal Resistance in Nanocomposites: A Comparative Study of Carbon Black and Nanotube Modifications. Nanomaterials 2025, 15, 546. https://doi.org/10.3390/nano15070546
Bibinger J, Eibl S, Gudladt H-J, Schartel B, Höfer P. Pushing the Limits of Thermal Resistance in Nanocomposites: A Comparative Study of Carbon Black and Nanotube Modifications. Nanomaterials. 2025; 15(7):546. https://doi.org/10.3390/nano15070546
Chicago/Turabian StyleBibinger, Johannes, Sebastian Eibl, Hans-Joachim Gudladt, Bernhard Schartel, and Philipp Höfer. 2025. "Pushing the Limits of Thermal Resistance in Nanocomposites: A Comparative Study of Carbon Black and Nanotube Modifications" Nanomaterials 15, no. 7: 546. https://doi.org/10.3390/nano15070546
APA StyleBibinger, J., Eibl, S., Gudladt, H.-J., Schartel, B., & Höfer, P. (2025). Pushing the Limits of Thermal Resistance in Nanocomposites: A Comparative Study of Carbon Black and Nanotube Modifications. Nanomaterials, 15(7), 546. https://doi.org/10.3390/nano15070546






