Grain Boundary Guided Folding of Graphene for Twisted Bilayer Graphene
Abstract
1. Introduction
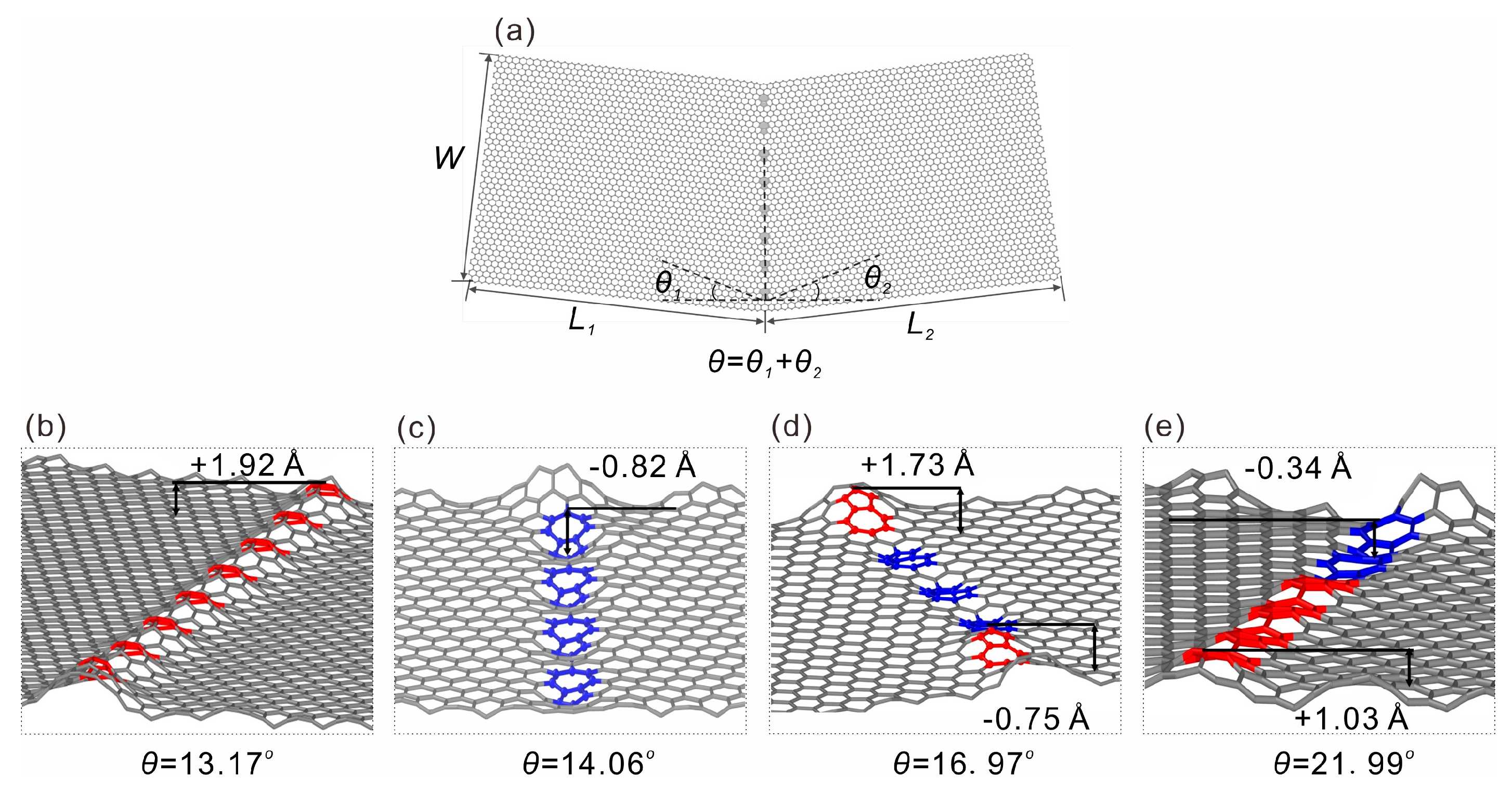
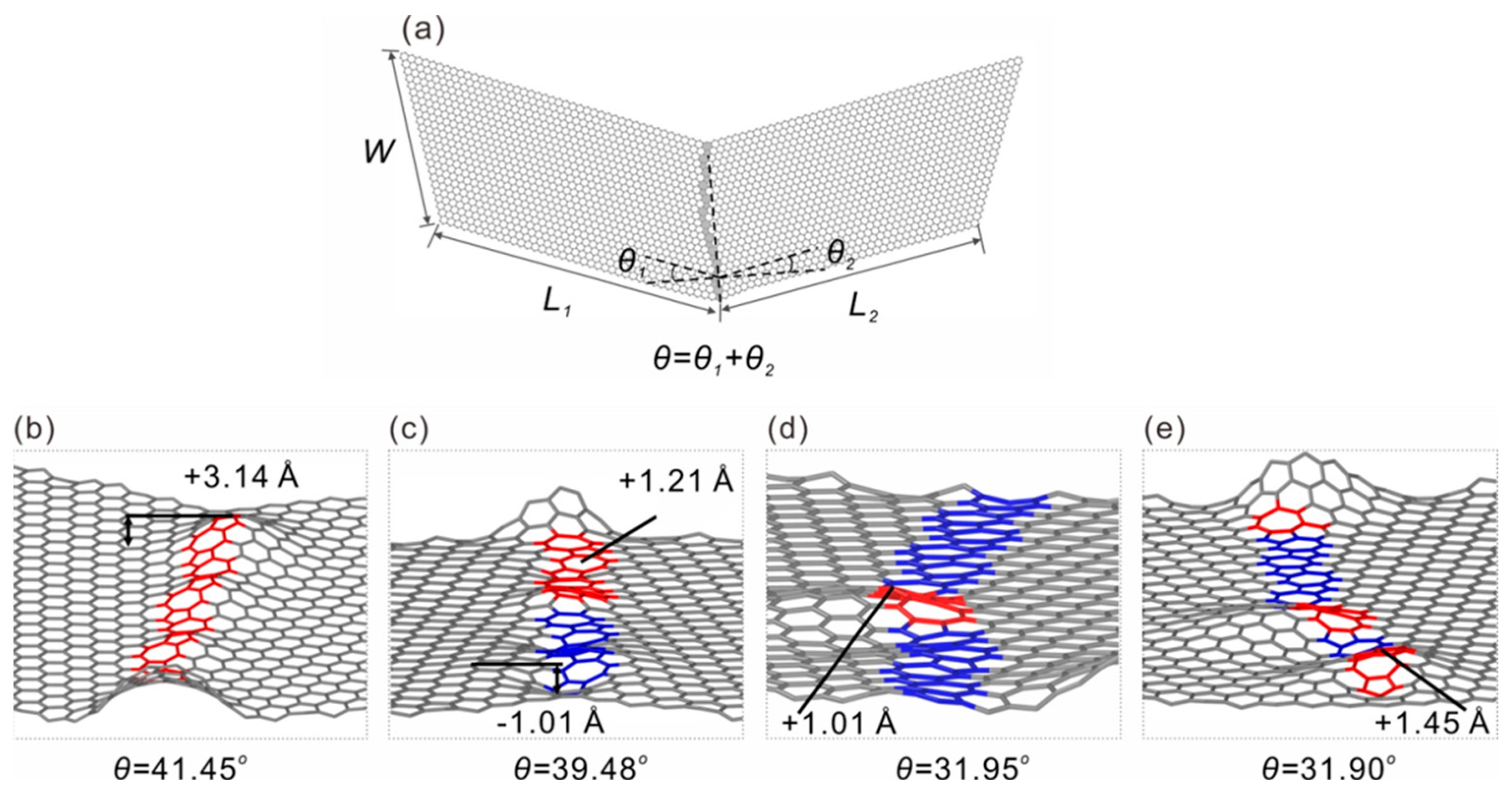
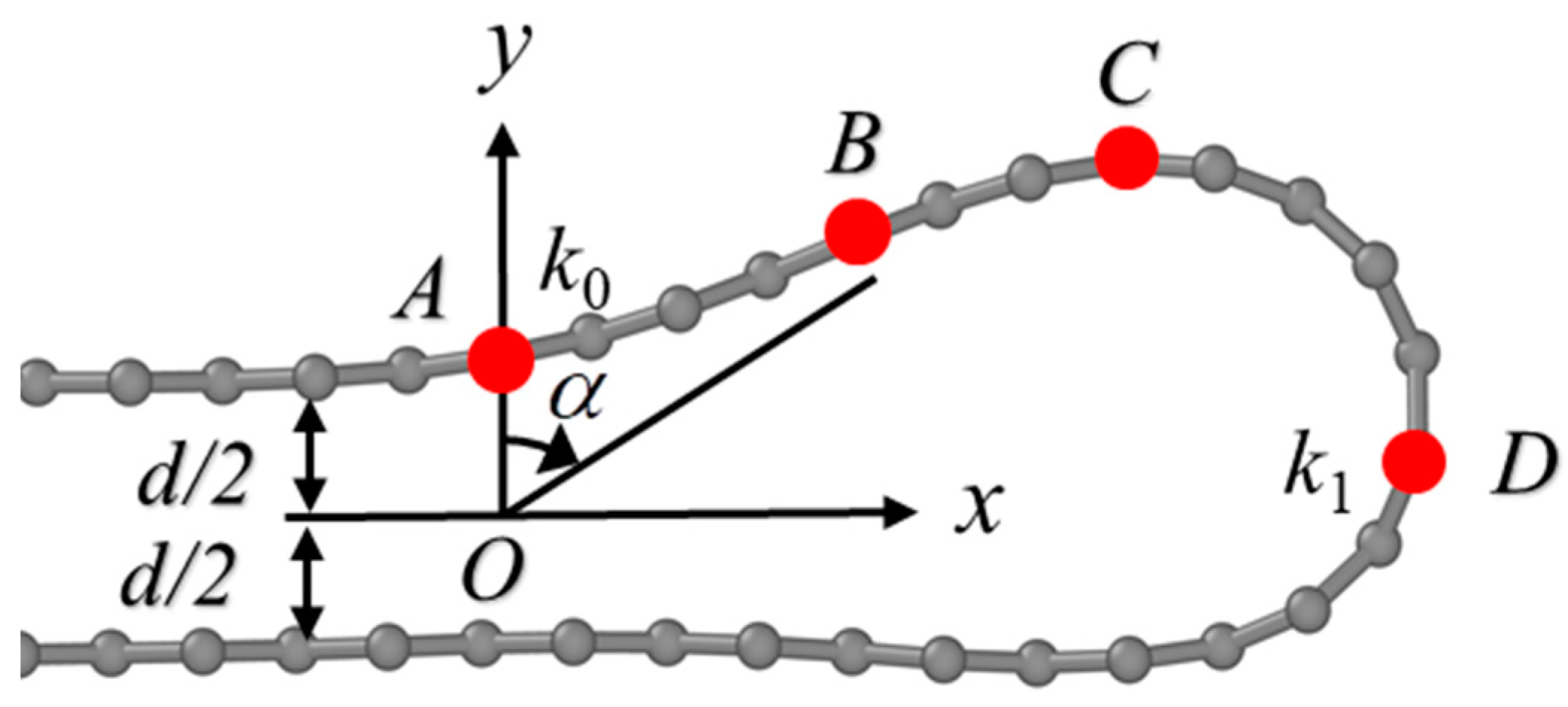
2. Materials and Methods
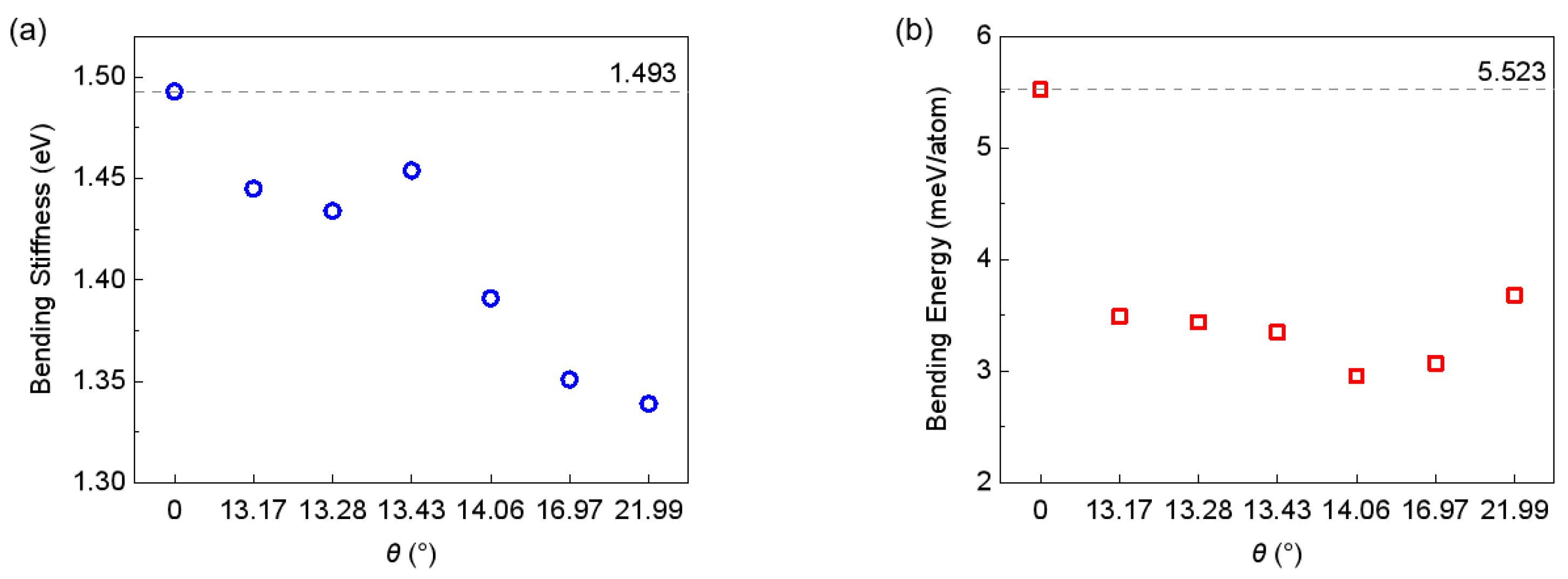
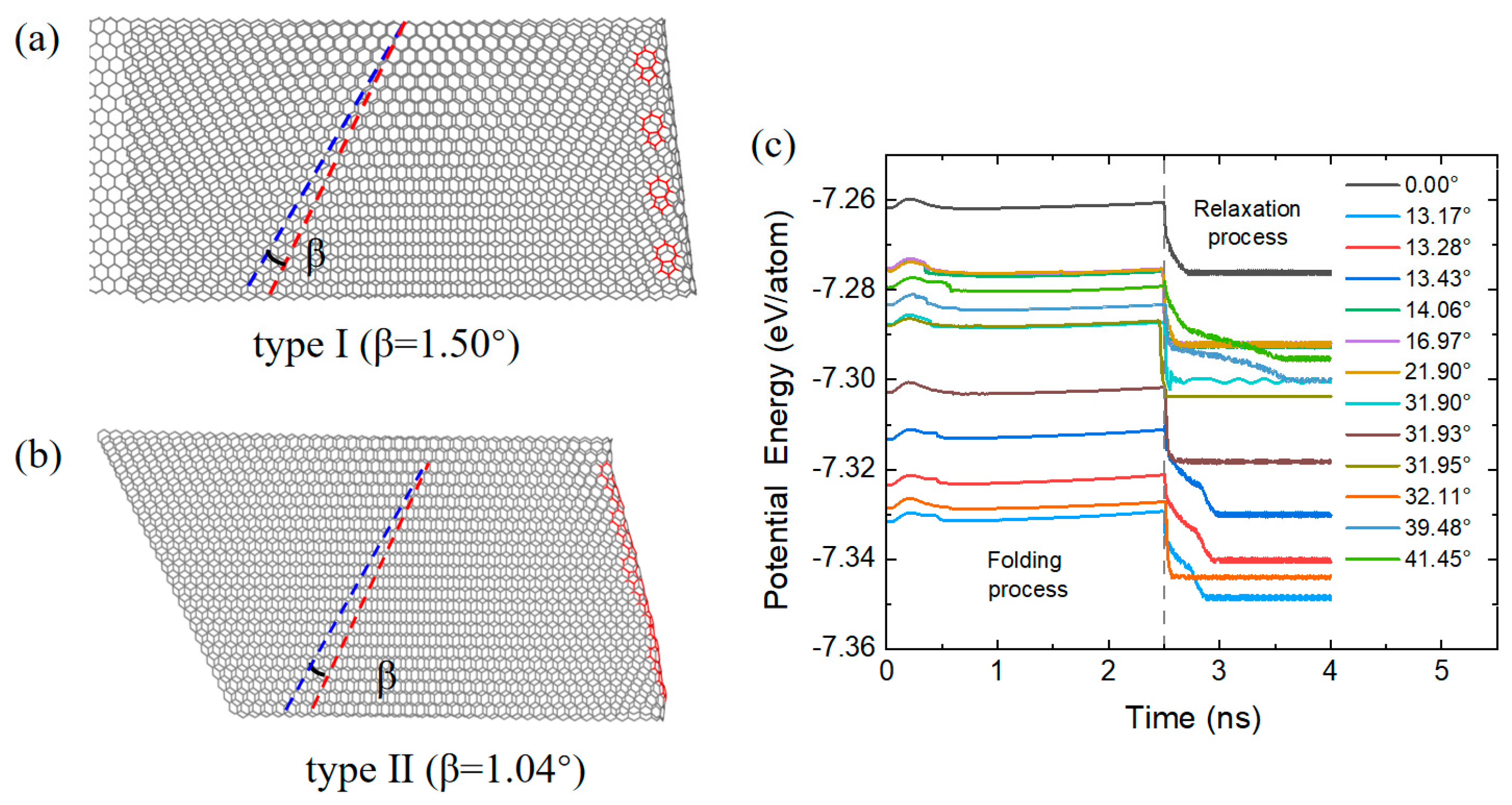
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric Field Effect in Atomically Thin Carbon Films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Schwierz, F. Graphene transistors. Nat. Nanotechnol. 2010, 5, 487–496. [Google Scholar]
- Tan, Y.B.; Lee, J.-M. Graphene for supercapacitor applications. J. Mater. Chem. A 2013, 1, 14814. [Google Scholar] [CrossRef]
- Wu, P.; Li, X.; Zhang, C.; Chen, X.; Lin, S.; Sun, H.; Lin, C.-T.; Zhu, H.; Luo, J. Self-Assembled Graphene Film as Low Friction Solid Lubricant in Macroscale Contact. Acs Appl. Mater. Interfaces 2017, 9, 21554–21562. [Google Scholar] [CrossRef] [PubMed]
- Hod, O.; Meyer, E.; Zheng, Q.; Urbakh, M. Structural superlubricity and ultralow friction across the length scales. Nature 2018, 563, 485–492. [Google Scholar] [CrossRef]
- Zhao, J.; Ji, P.; Li, Y.; Li, R.; Zhang, K.; Tian, H.; Yu, K.; Bian, B.; Hao, L.; Xiao, X.; et al. Ultrahigh-mobility semiconducting epitaxial graphene on silicon carbide. Nature 2024, 625, 60–65. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Zou, Y.-C.; Woods, C.R.; Shi, Y.; Yin, J.; Xu, S.; Ozdemir, S.; Taniguchi, T.; Watanabe, K.; Geim, A.K.; et al. Stacking Order in Graphite Films Controlled by van der Waals Technology. Nano Lett. 2019, 19, 8526–8532. [Google Scholar]
- Zhou, K.; Wang, L.; Wang, R.; Wang, C.; Tang, C. One Dimensional Twisted Van der Waals Structures Constructed by Self-Assembling Graphene Nanoribbons on Carbon Nanotubes. Materials 2022, 15, 8220. [Google Scholar] [CrossRef]
- Cao, Y.; Fatemi, V.; Fang, S.; Watanabe, K.; Taniguchi, T.; Kaxiras, E.; Jarillo-Herrero, P. Unconventional superconductivity in magic-angle graphene superlattices. Nature 2018, 556, 43–50. [Google Scholar] [CrossRef]
- Cao, Y.; Park, J.M.; Watanabe, K.; Taniguchi, T.; Jarillo-Herrero, P. Pauli-limit violation and re-entrant superconductivity in moiré graphene. Nature 2021, 595, 526–531. [Google Scholar] [CrossRef]
- Zhang, E.; Lin, F.; Lei, Z.; Qi, S.; Ban, S.; Vinu, A.; Yi, J.; Liu, Y. Twist the doorknob to open the electronic properties of graphene-based van der Waals structure. Matter 2021, 4, 3444–3482. [Google Scholar]
- Park, J.M.; Cao, Y.; Watanabe, K.; Taniguchi, T.; Jarillo-Herrero, P. Tunable strongly coupled superconductivity in magic-angle twisted trilayer graphene. Nature 2021, 590, 249–255. [Google Scholar] [PubMed]
- Nimbalkar, A.; Kim, H. Opportunities and Challenges in Twisted Bilayer Graphene: A Review. Nano-Micro Lett. 2020, 12, 126. [Google Scholar]
- Saumya, K.; Naskar, S.; Mukhopadhyay, T. ‘Magic’ of twisted multi-layered graphene and 2D nano-heterostructures. Nano Futures 2023, 7, 032005. [Google Scholar]
- Sun, Y.; Zhou, K.; Wang, R.; Zhang, Z.; Tang, C.; Guo, W. Formation of One-Dimensional van der Waals Heterostructures via Self-Assembly of Blue Phosphorene Nanoribbons to Carbon Nanotubes. Acta Mech. Solida Sin. 2022, 35, 913–921. [Google Scholar]
- Georgakilas, V.; Perman, J.A.; Tucek, J.; Zboril, R. Broad Family of Carbon Nanoallotropes: Classification, Chemistry, and Applications of Fullerenes, Carbon Dots, Nanotubes, Graphene, Nanodiamonds, and Combined Superstructures. Chem. Rev. 2015, 115, 4744–4822. [Google Scholar]
- Cao, K.; Feng, S.; Han, Y.; Gao, L.; Hue Ly, T.; Xu, Z.; Lu, Y. Elastic straining of free-standing monolayer graphene. Nat. Commun. 2020, 11, 284. [Google Scholar]
- Wei, Y.; Wang, B.; Wu, J.; Yang, R.; Dunn, M.L. Bending Rigidity and Gaussian Bending Stiffness of Single-Layered Graphene. Nano Lett. 2013, 13, 26–30. [Google Scholar]
- Wang, B.; Huang, M.; Kim, N.Y.; Cunning, B.V.; Huang, Y.; Qu, D.; Chen, X.; Jin, S.; Biswal, M.; Zhang, X.; et al. Controlled Folding of Single Crystal Graphene. Nano Lett. 2017, 17, 1467–1473. [Google Scholar]
- Liu, L.; Chen, Y.; Chen, L.; Xie, B.; Li, G.; Kong, L.; Tao, Q.; Li, Z.; Yang, X.; Lu, Z.; et al. Ultrashort vertical-channel MoS2 transistor using a self-aligned contact. Nat. Commun. 2024, 15, 165. [Google Scholar]
- Tang, C.; Oppenheim, T.; Tung, V.C.; Martini, A. Structure–stability relationships for graphene-wrapped fullerene-coated carbon nanotubes. Carbon 2013, 61, 458–466. [Google Scholar]
- Liu, T.-H.; Gajewski, G.; Pao, C.-W.; Chang, C.-C. Structure, energy, and structural transformations of graphene grain boundaries from atomistic simulations. Carbon 2011, 49, 2306–2317. [Google Scholar]
- Zhang, J.; Zhao, J.; Lu, J. Intrinsic Strength and Failure Behaviors of Graphene Grain Boundaries. ACS Nano 2012, 6, 2704–2711. [Google Scholar]
- Wu, J.; Wei, Y. Grain misorientation and grain-boundary rotation dependent mechanical properties in polycrystalline graphene. J. Mech. Phys. Solids 2013, 61, 1421–1432. [Google Scholar]
- Zhang, Z.; Yang, Y.; Xu, F.; Wang, L.; Yakobson, B.I. Unraveling the Sinuous Grain Boundaries in Graphene. Adv. Funct. Mater. 2015, 25, 367–373. [Google Scholar]
- Huang, P.Y.; Ruiz-Vargas, C.S.; Van Der Zande, A.M.; Whitney, W.S.; Levendorf, M.P.; Kevek, J.W.; Garg, S.; Alden, J.S.; Hustedt, C.J.; Zhu, Y.; et al. Grains and grain boundaries in single-layer graphene atomic patchwork quilts. Nature 2011, 469, 389–392. [Google Scholar]
- Chen, X.; Zhang, L.; Zhao, Y.; Wang, X.; Ke, C. Graphene folding on flat substrates. J. Appl. Phys. 2014, 116, 164301. [Google Scholar]
- González-Herrero, H.; Gómez-Rodríguez, J.M.; Mallet, P.; Moaied, M.; Palacios, J.J.; Salgado, C.; Ugeda, M.M.; Veuillen, J.-Y.; Yndurain, F.; Brihuega, I. Atomic-scale control of graphene magnetism by using hydrogen atoms. Science 2016, 352, 437–441. [Google Scholar]
- Shi, L.-J.; Yang, L.-Z.; Deng, J.-Q.; Tong, L.-H.; Wu, Q.; Zhang, L.; Zhang, L.; Yin, L.-J.; Qin, Z. Constructing graphene nanostructures with zigzag edge terminations by controllable STM tearing and folding. Carbon 2020, 165, 169–174. [Google Scholar]
- Grantab, R.; Shenoy, V.B.; Ruoff, R.S. Anomalous Strength Characteristics of Tilt Grain Boundaries in Graphene. Science 2010, 330, 946–948. [Google Scholar]
- Zhang, H.; Lee, G.; Gong, C.; Colombo, L.; Cho, K. Grain Boundary Effect on Electrical Transport Properties of Graphene. J. Phys. Chem. C 2014, 118, 2338–2343. [Google Scholar]
- Malola, S.; Häkkinen, H.; Koskinen, P. Structural, chemical, and dynamical trends in graphene grain boundaries. Phys. Rev. B 2010, 81, 165447. [Google Scholar] [CrossRef]
- Tsen, A.W.; Brown, L.; Levendorf, M.P.; Ghahari, F.; Huang, P.Y.; Havener, R.W.; Ruiz-Vargas, C.S.; Muller, D.A.; Kim, P.; Park, J. Tailoring Electrical Transport Across Grain Boundaries in Polycrystalline Graphene. Science 2012, 336, 1143–1146. [Google Scholar] [PubMed]
- Tuckerman, M.E.; Martyna, G.J. Understanding Modern Molecular Dynamics: Techniques and Applications. J. Phys. Chem. B 2000, 104, 159–178. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar]
- Wei, Y.; Wu, J.; Yin, H.; Shi, X.; Yang, R.; Dresselhaus, M. The nature of strength enhancement and weakening by pentagon–heptagon defects in graphene. Nat. Mater. 2012, 11, 759–763. [Google Scholar] [CrossRef]
- Xu, Z. Defects in two-dimensional materials: Topological and geometrical effects. Chin. Sci. Bull. 2016, 61, 501–510. [Google Scholar] [CrossRef]
- Stuart, S.J.; Tutein, A.B.; Harrison, J.A. A reactive potential for hydrocarbons with intermolecular interactions. J. Chem. Phys. 2000, 112, 6472–6486. [Google Scholar]
- Brenner, D.W.; Shenderova, O.A.; Harrison, J.A.; Stuart, S.J.; Ni, B.; Sinnott, S.B. A second-generation reactive empirical bond order (REBO) potential energy expression for hydrocarbons. J. Phys. Condens. Matter 2002, 14, 783–802. [Google Scholar] [CrossRef]
- Fan, L.; Yao, W. Effects of vacancy defects on the mechanical properties of graphene/hexagonal BN superlattice nanoribbons. New Carbon Mater. 2020, 35, 165–175. [Google Scholar] [CrossRef]
- Stillinger, F.H.; Weber, T.A. Computer simulation of local order in condensed phases of silicon. Phys. Rev. B 1985, 31, 5262–5271. [Google Scholar]
- Nosé, S. A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 1984, 81, 511–519. [Google Scholar]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2010, 18, 015012. [Google Scholar] [CrossRef]
- Zhang, J.; Xiao, J.; Meng, X.; Monroe, C.; Huang, Y.; Zuo, J.-M. Free Folding of Suspended Graphene Sheets by Random Mechanical Stimulation. Phys. Rev. Lett. 2010, 104, 166805. [Google Scholar]
- Zhong, X.; Pandey, R.; Karna, S.P. Stacking dependent electronic structure and transport in bilayer graphene nanoribbons. Carbon 2012, 50, 784–790. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, F.; Zhou, K.; Zhang, K.; Wang, L.; Wang, R.; Xia, J.; Tang, C. Grain Boundary Guided Folding of Graphene for Twisted Bilayer Graphene. Nanomaterials 2025, 15, 482. https://doi.org/10.3390/nano15070482
Feng F, Zhou K, Zhang K, Wang L, Wang R, Xia J, Tang C. Grain Boundary Guided Folding of Graphene for Twisted Bilayer Graphene. Nanomaterials. 2025; 15(7):482. https://doi.org/10.3390/nano15070482
Chicago/Turabian StyleFeng, Feiru, Kun Zhou, Kang Zhang, Liya Wang, Ruijie Wang, Jun Xia, and Chun Tang. 2025. "Grain Boundary Guided Folding of Graphene for Twisted Bilayer Graphene" Nanomaterials 15, no. 7: 482. https://doi.org/10.3390/nano15070482
APA StyleFeng, F., Zhou, K., Zhang, K., Wang, L., Wang, R., Xia, J., & Tang, C. (2025). Grain Boundary Guided Folding of Graphene for Twisted Bilayer Graphene. Nanomaterials, 15(7), 482. https://doi.org/10.3390/nano15070482





