Instability of Oldroyd-B Liquid Films with Odd Viscosity on Porous Inclined Substrates
Abstract
1. Introduction
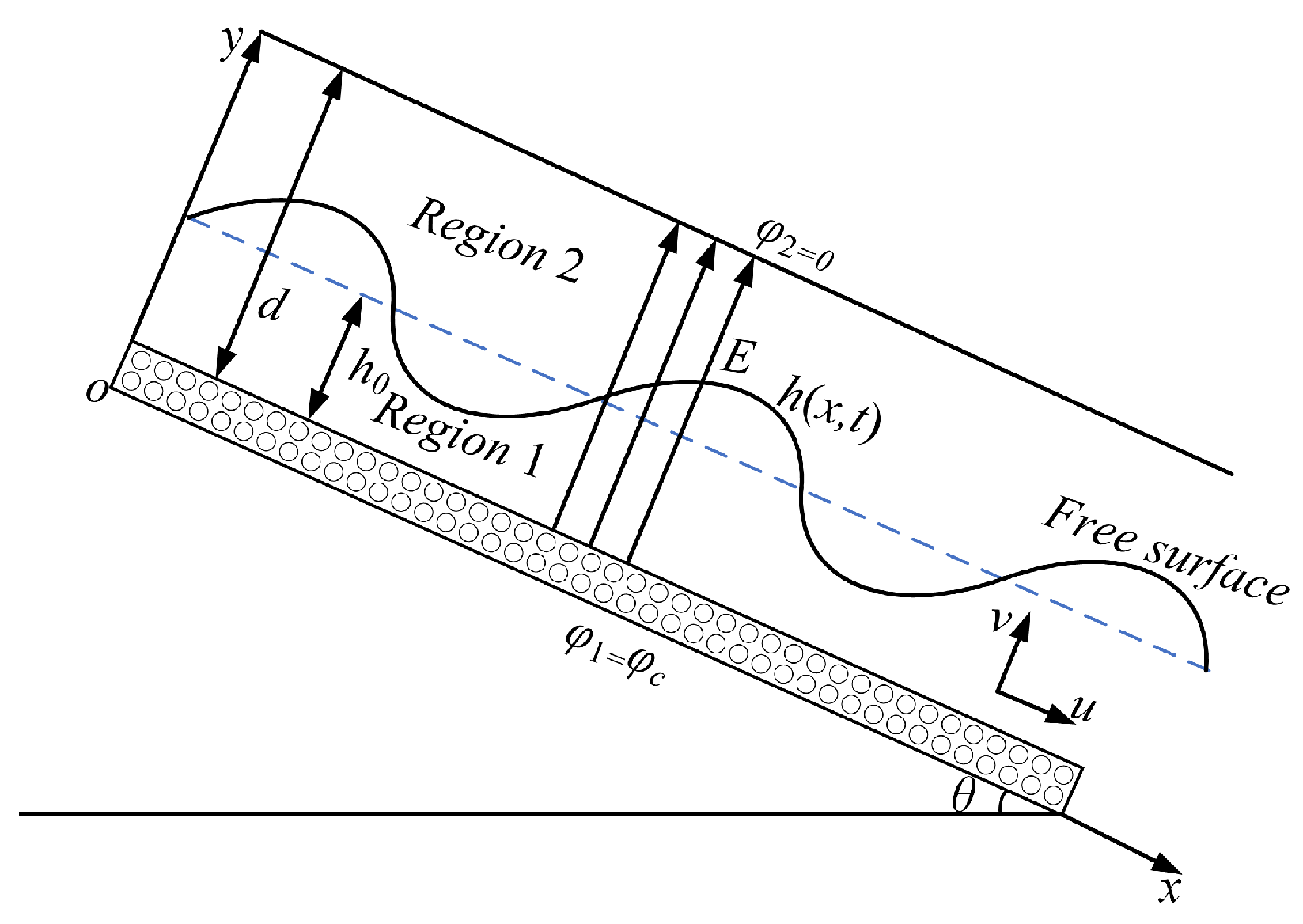
2. Formulation of the Problem
2.1. Governing Equations
2.2. Boundary Conditions
2.3. Dimensionless Analysis
3. Nonlinear Evolution Equation
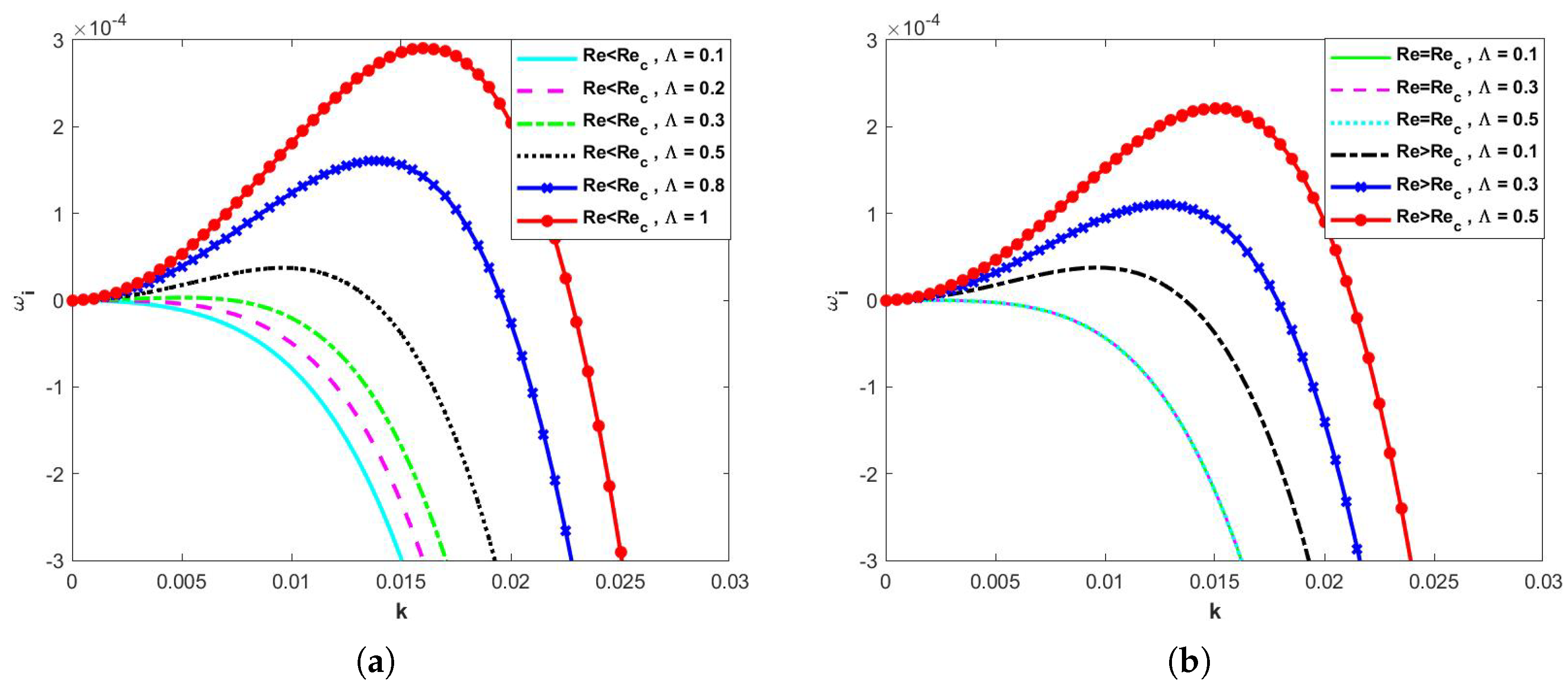
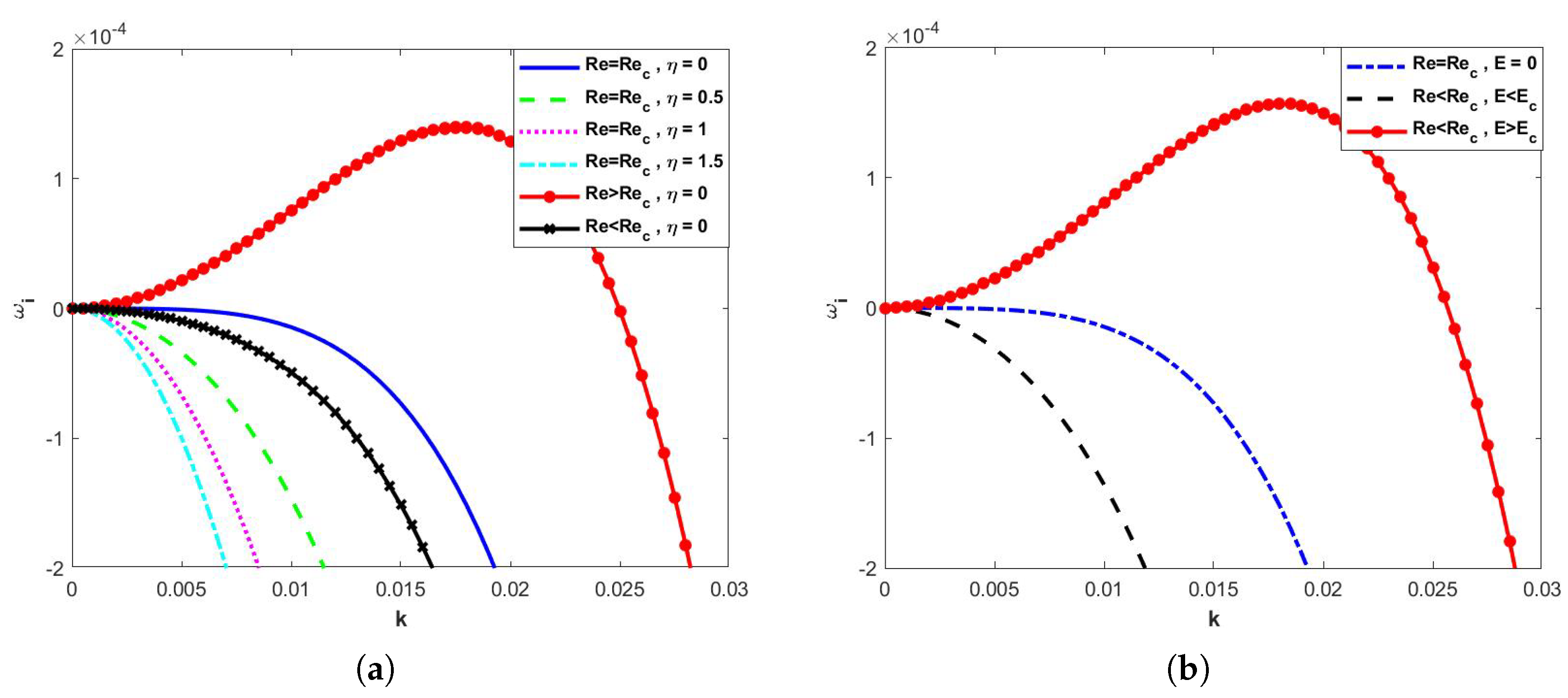
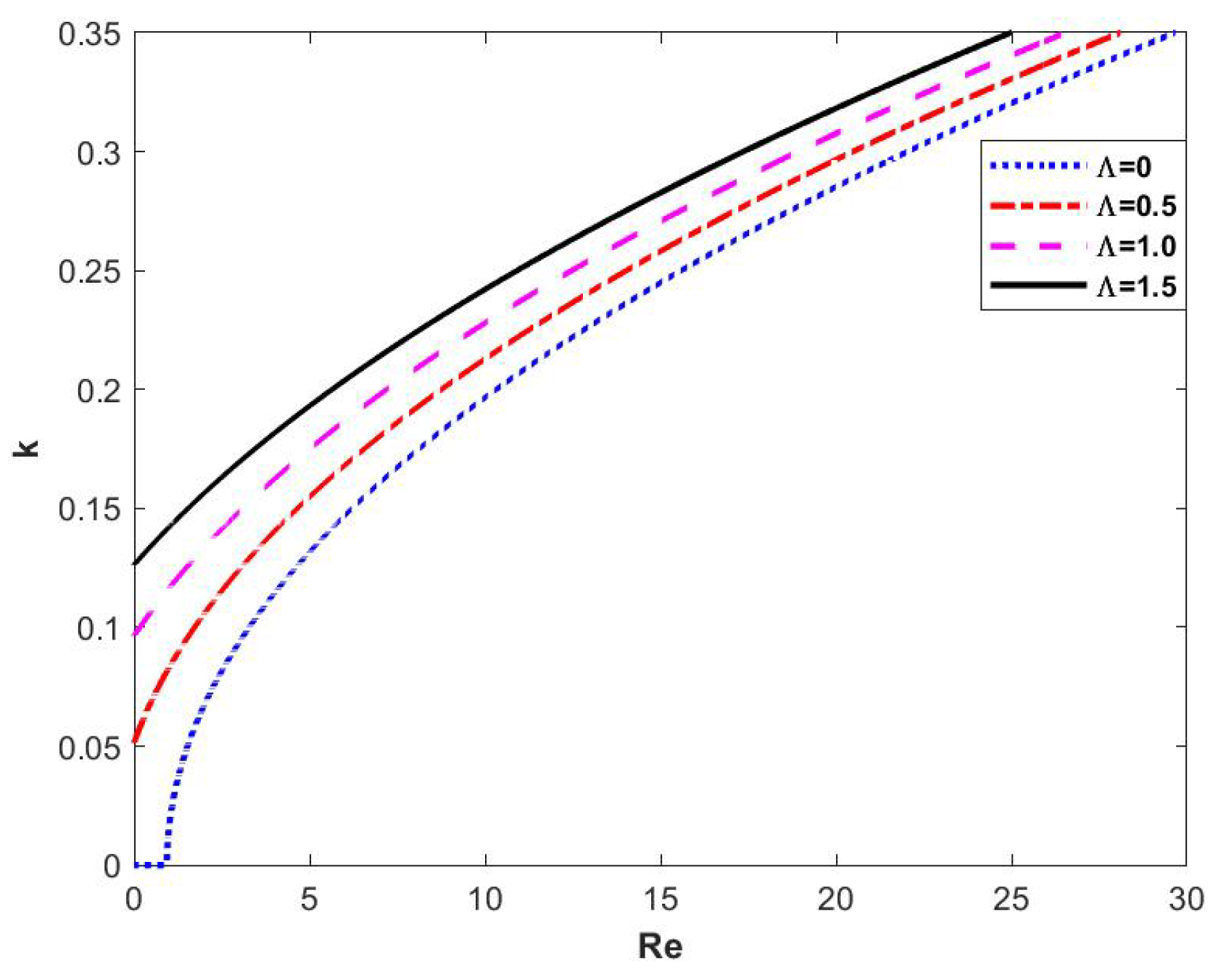
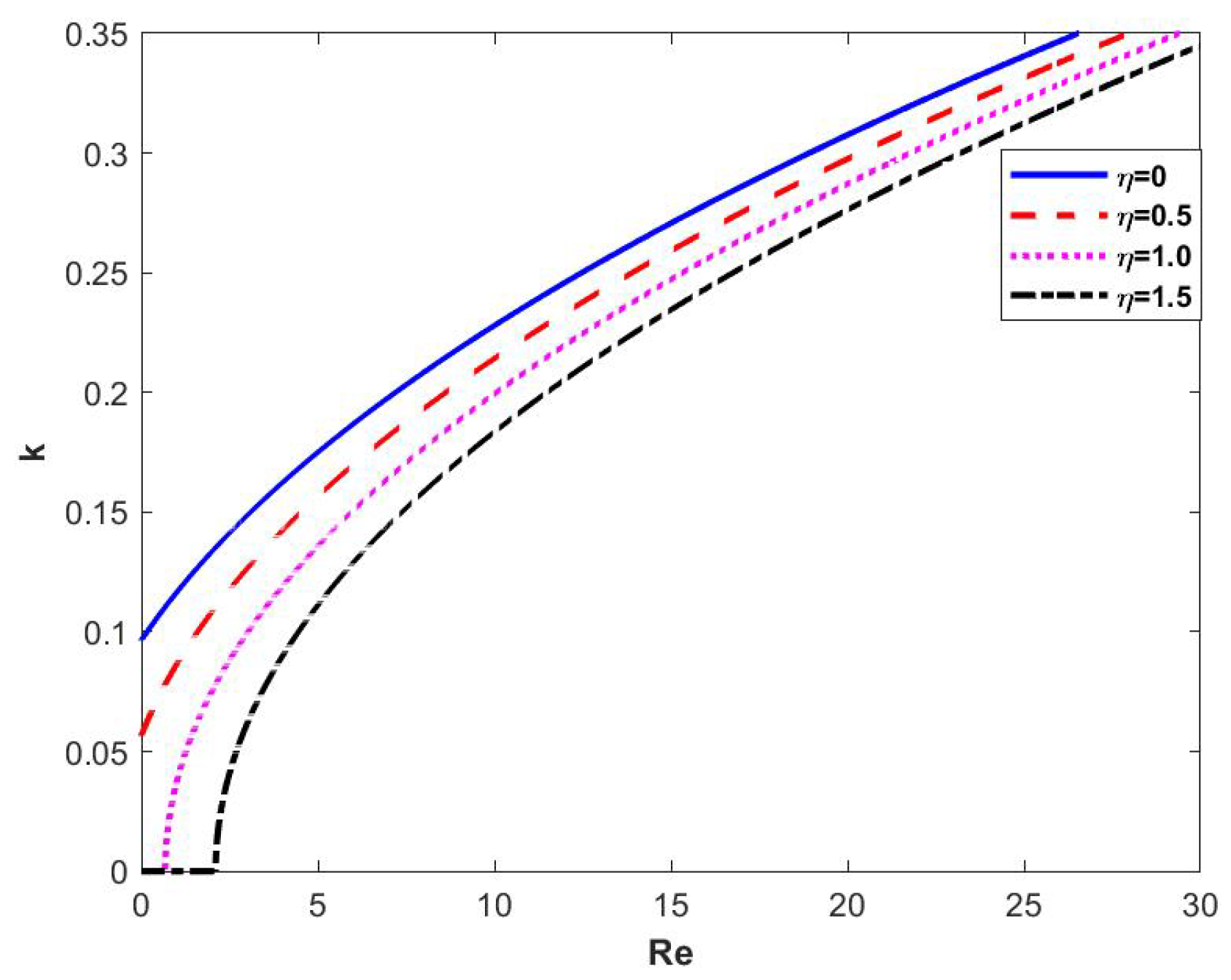
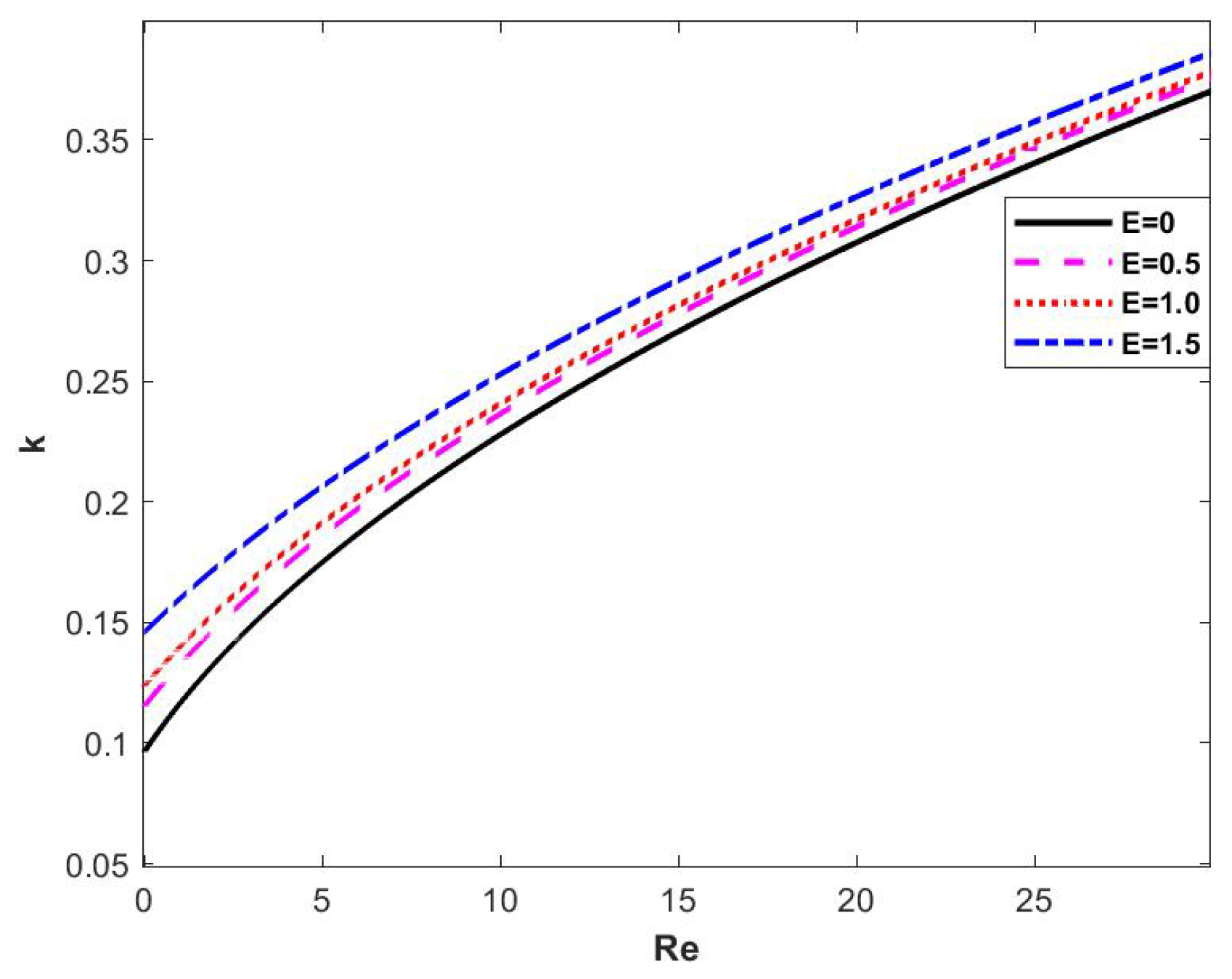
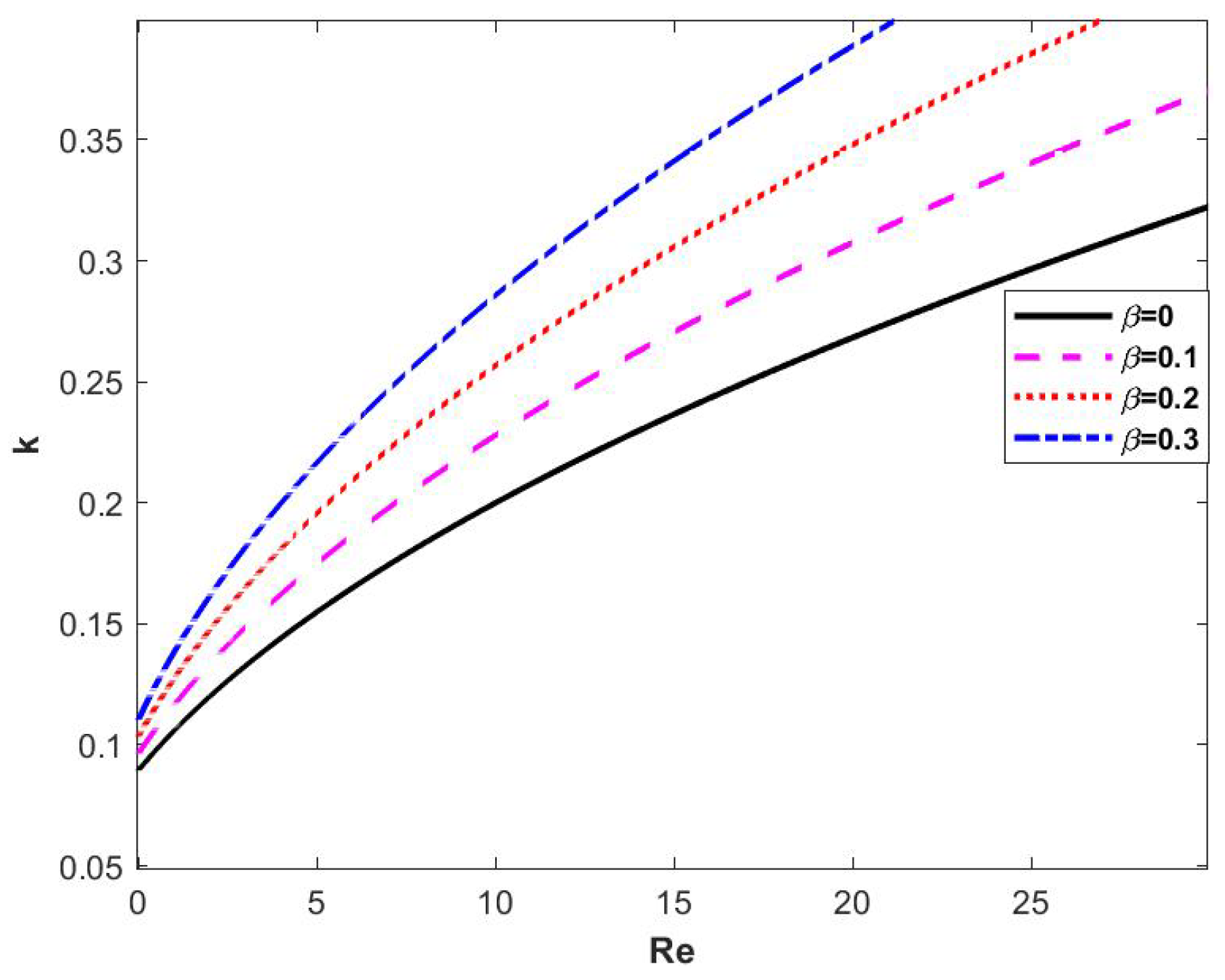
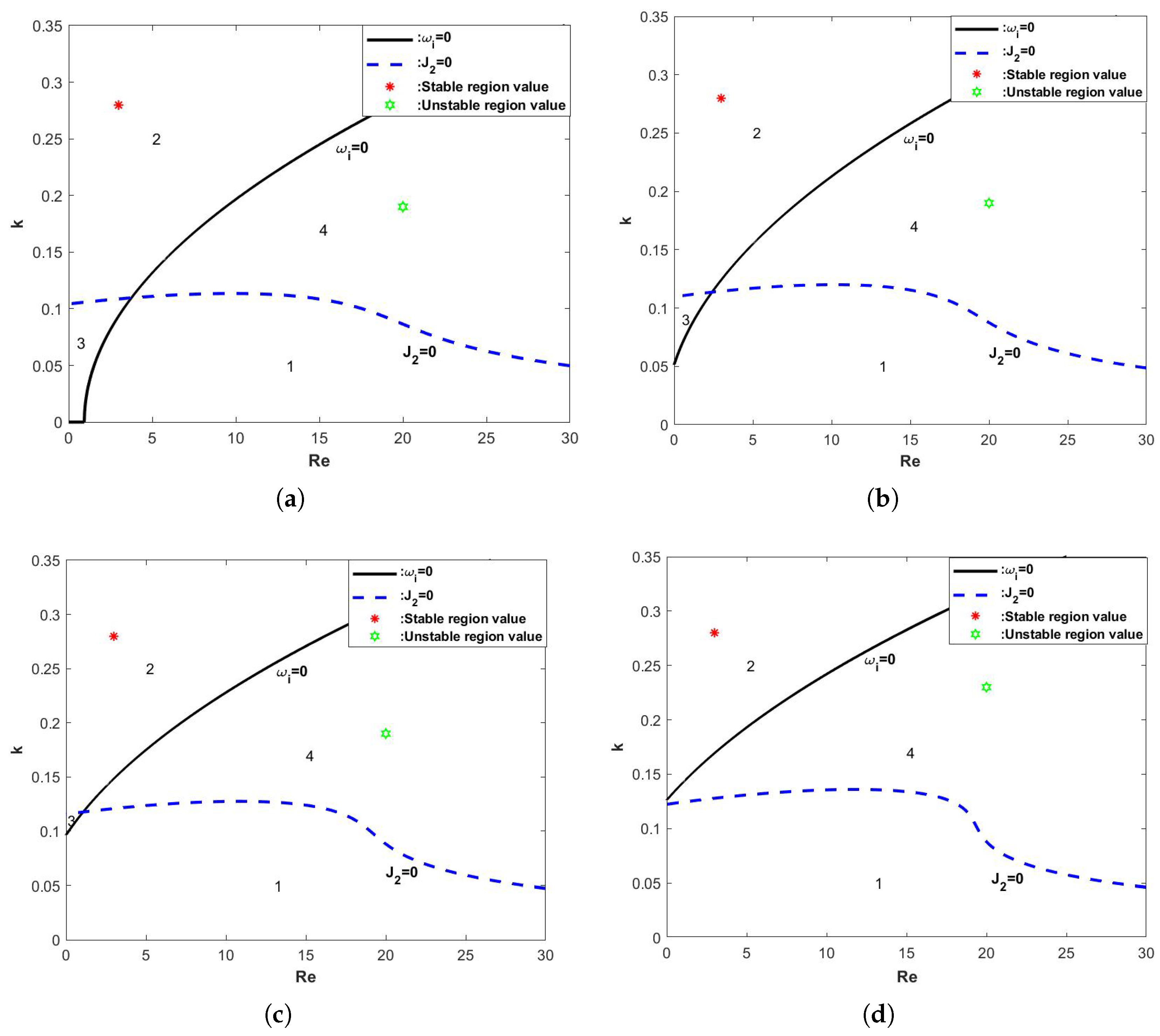
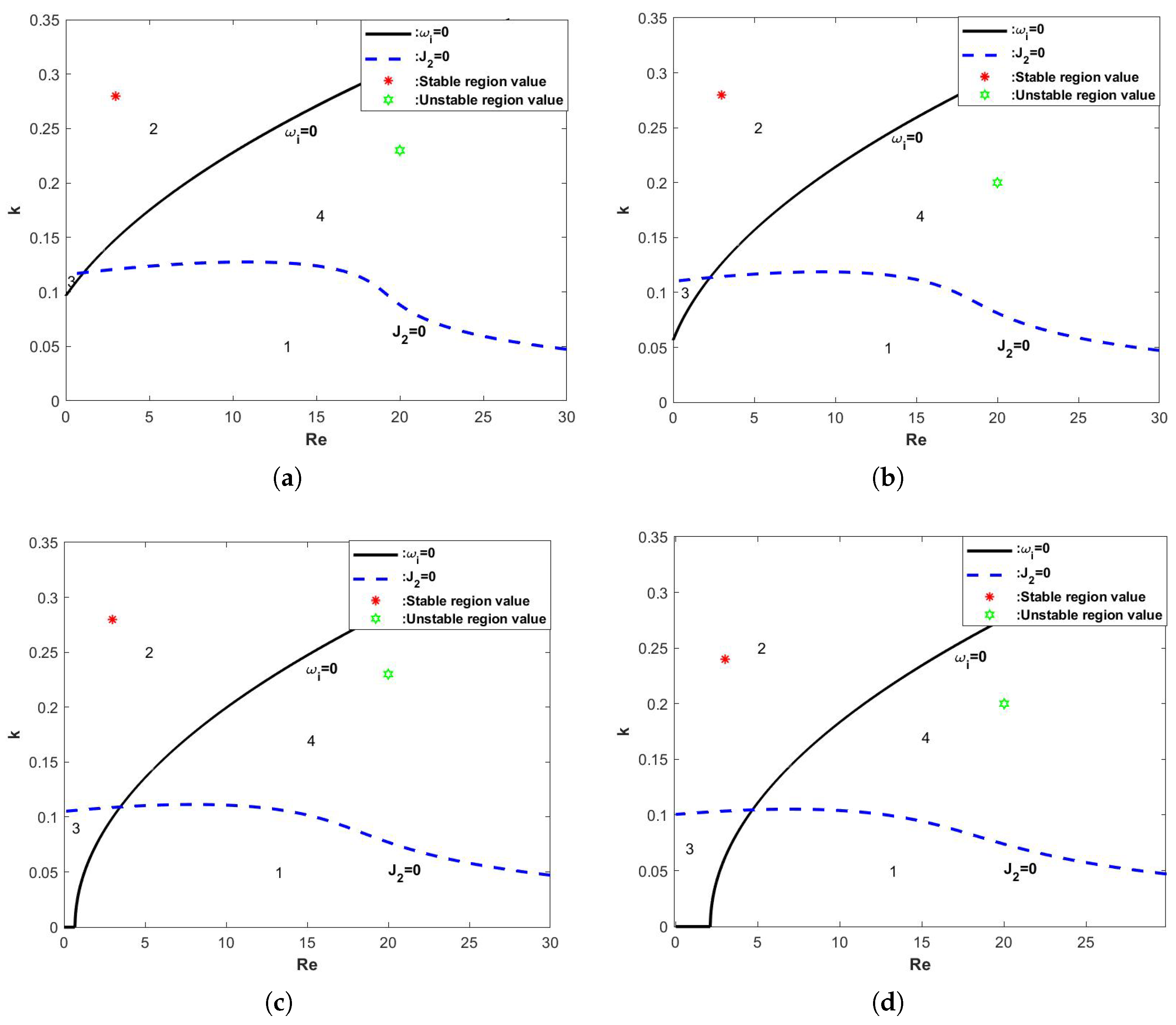
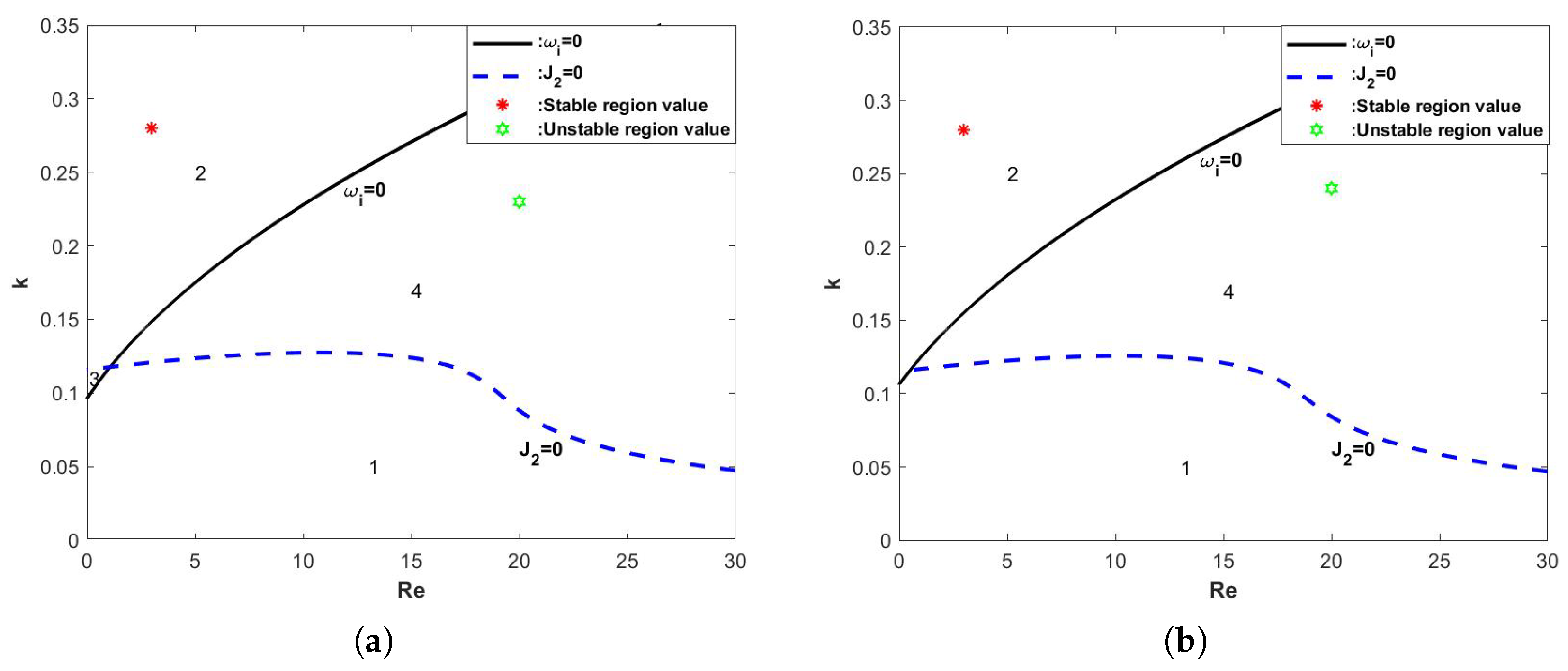
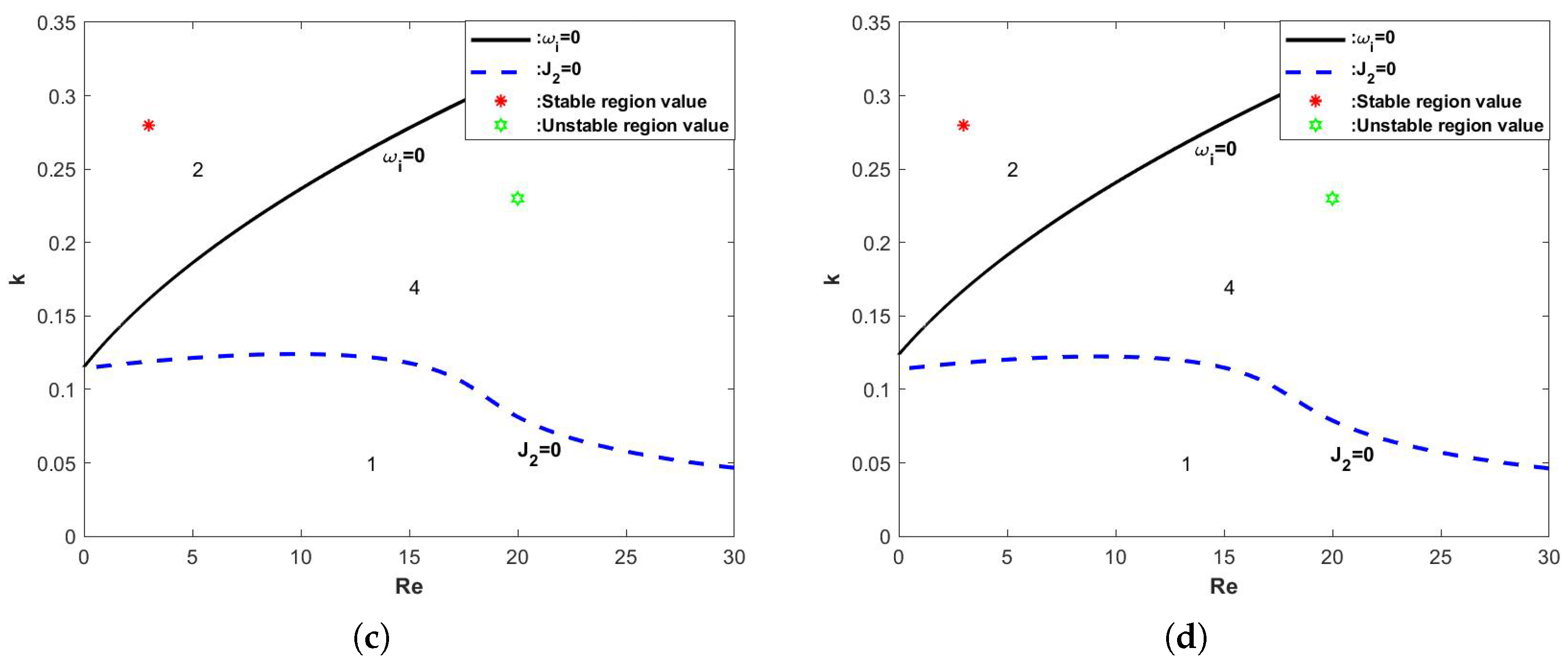
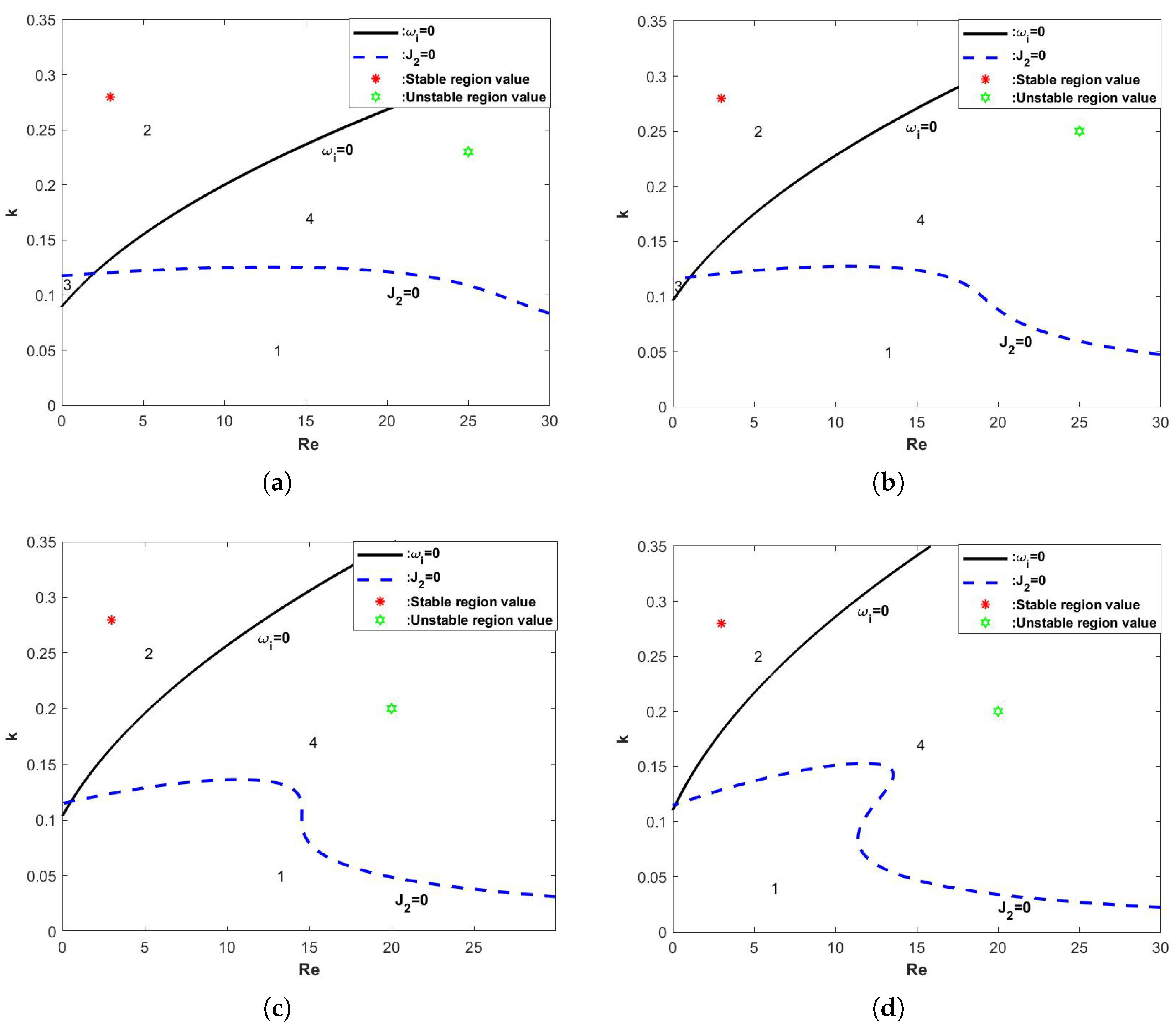
4. Linear Stability Analysis
5. Ginzburg–Landau Equation
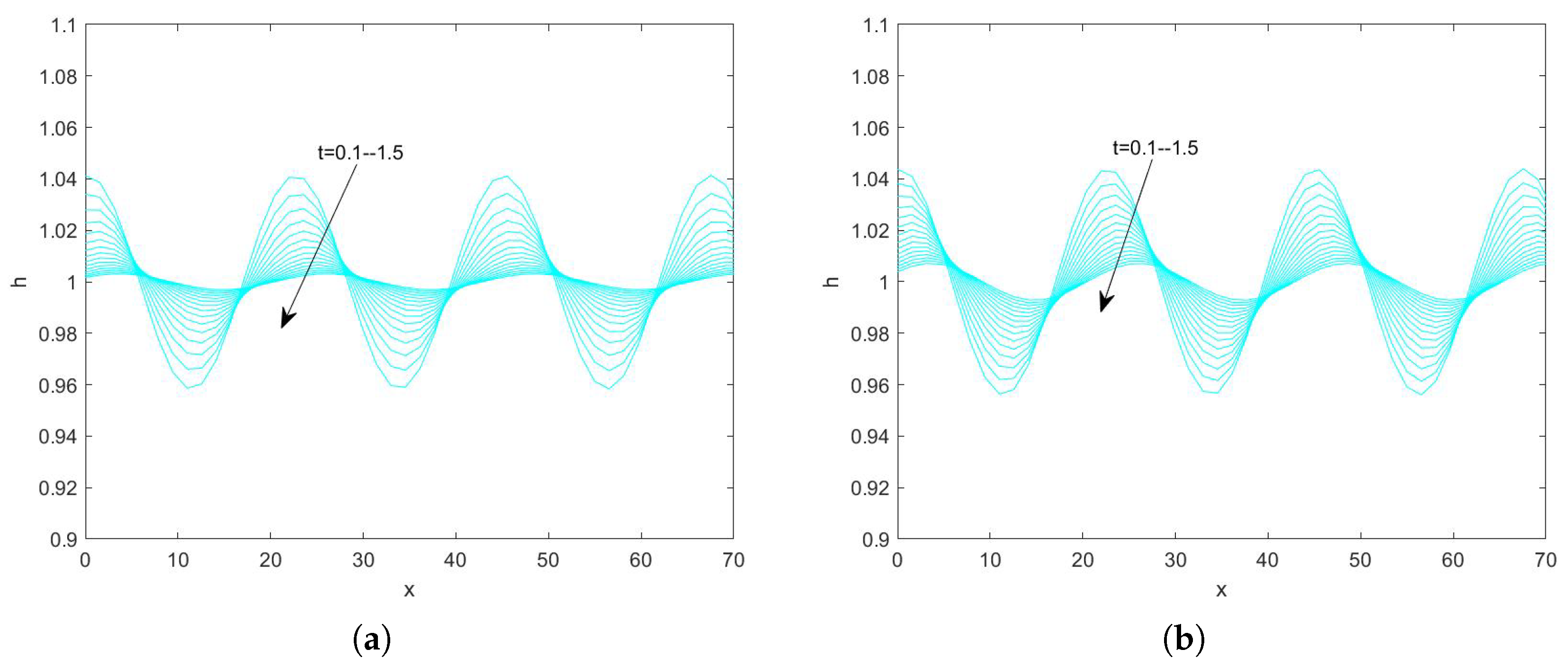
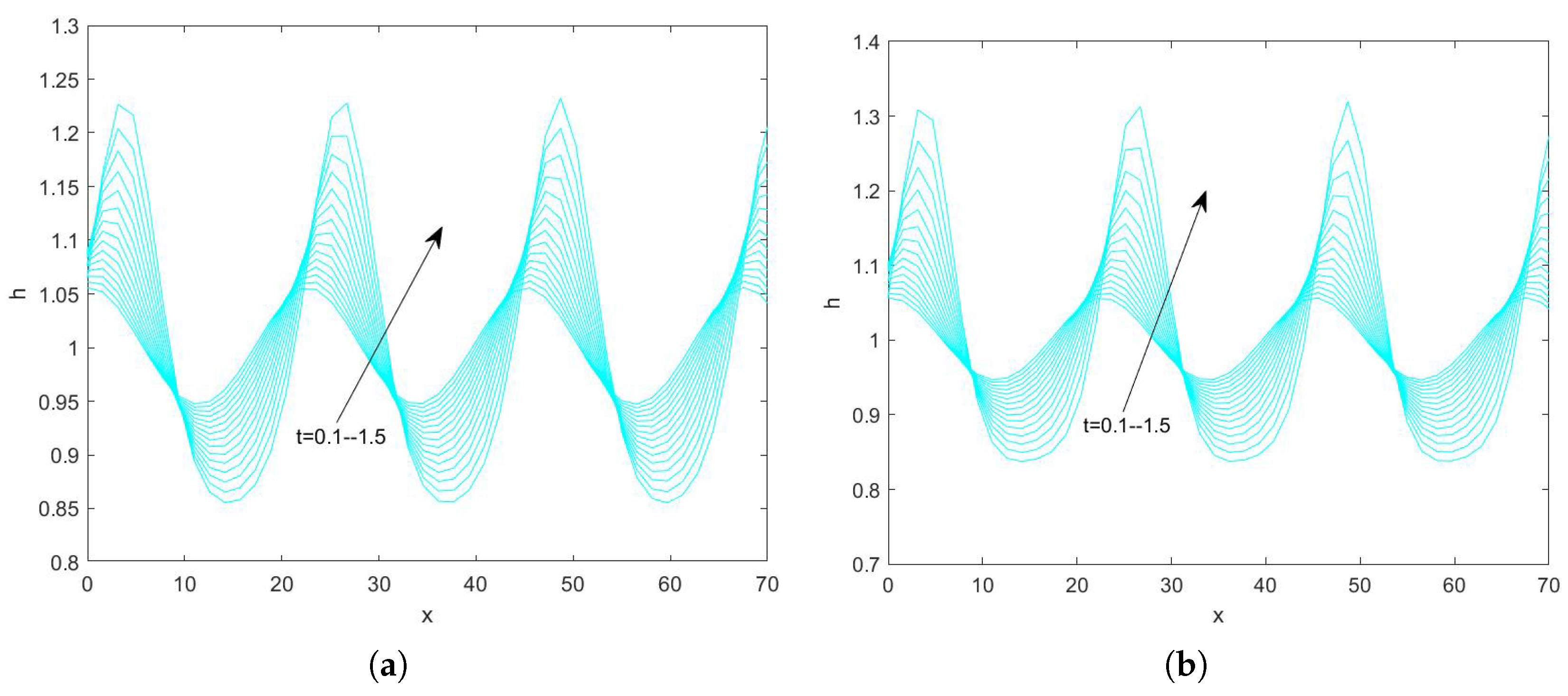
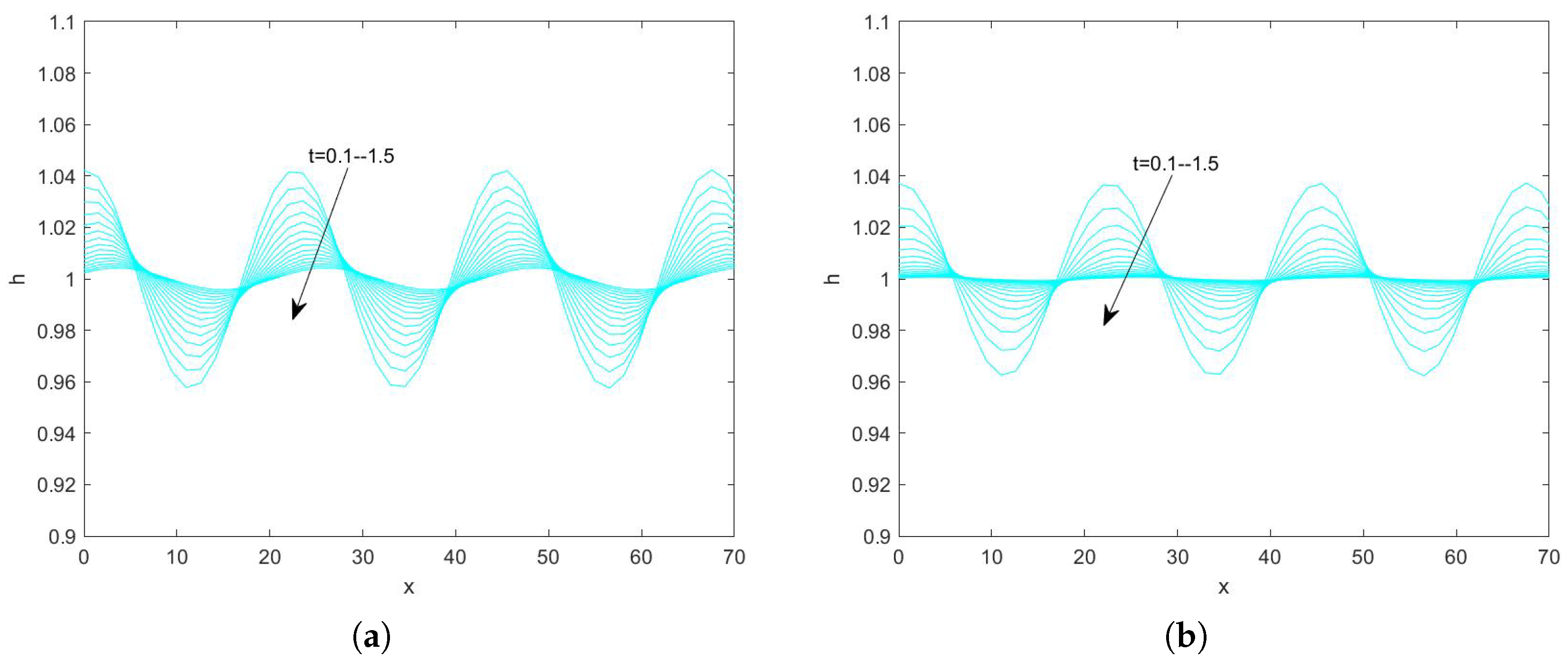
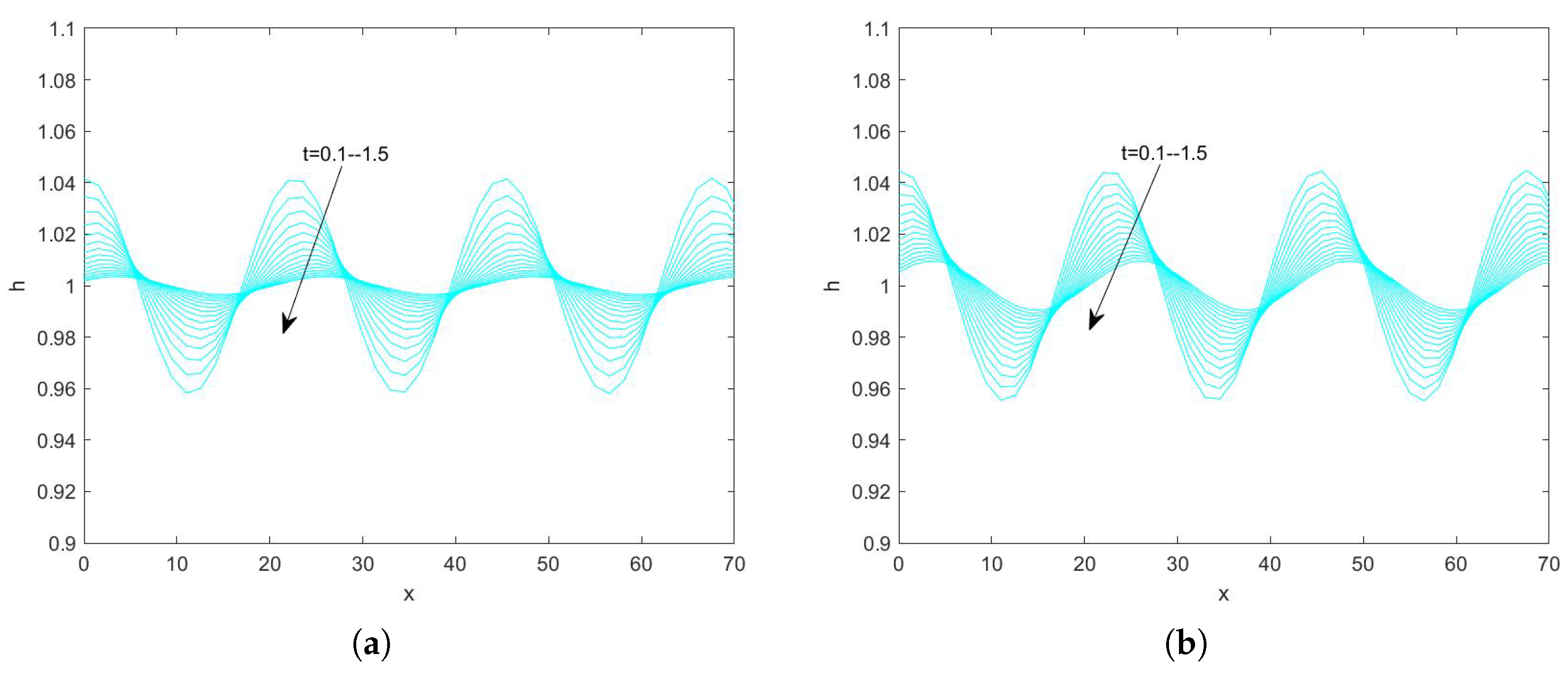
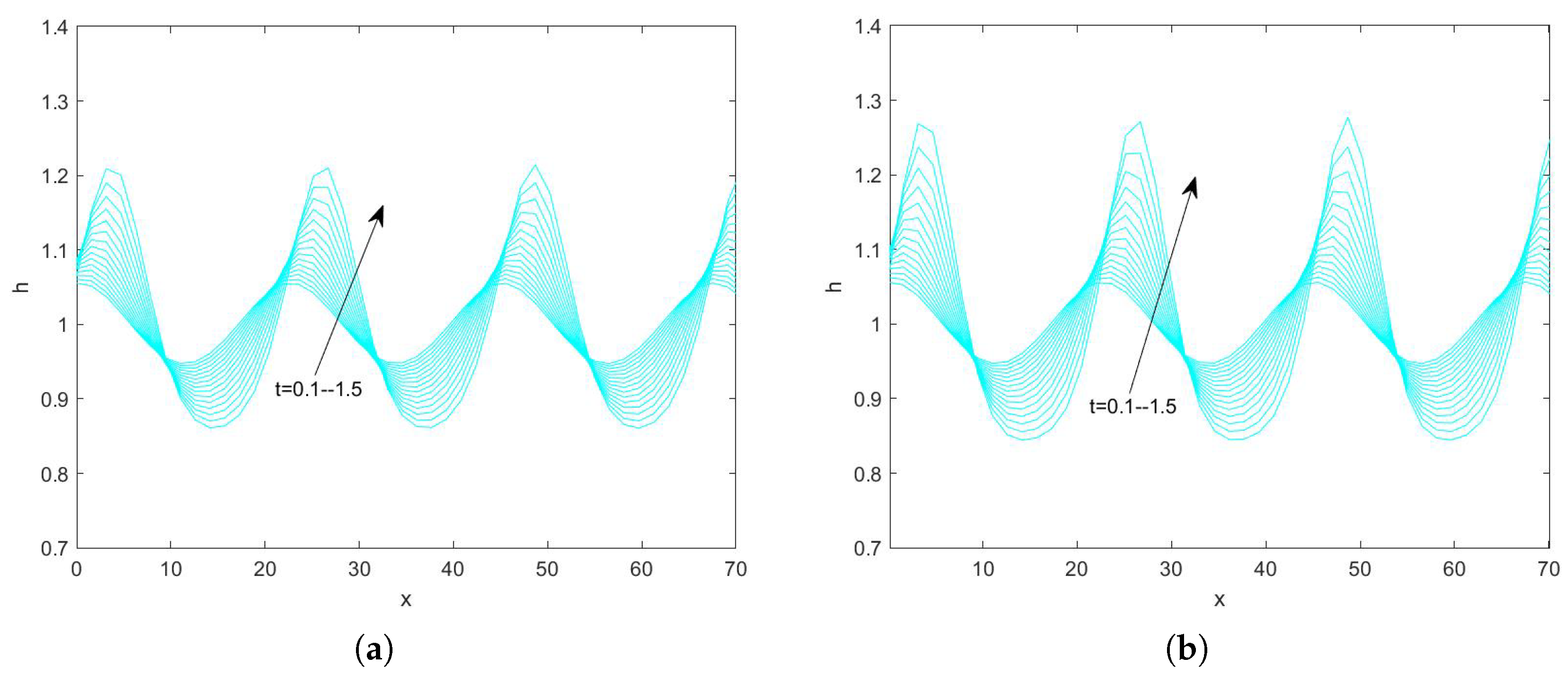
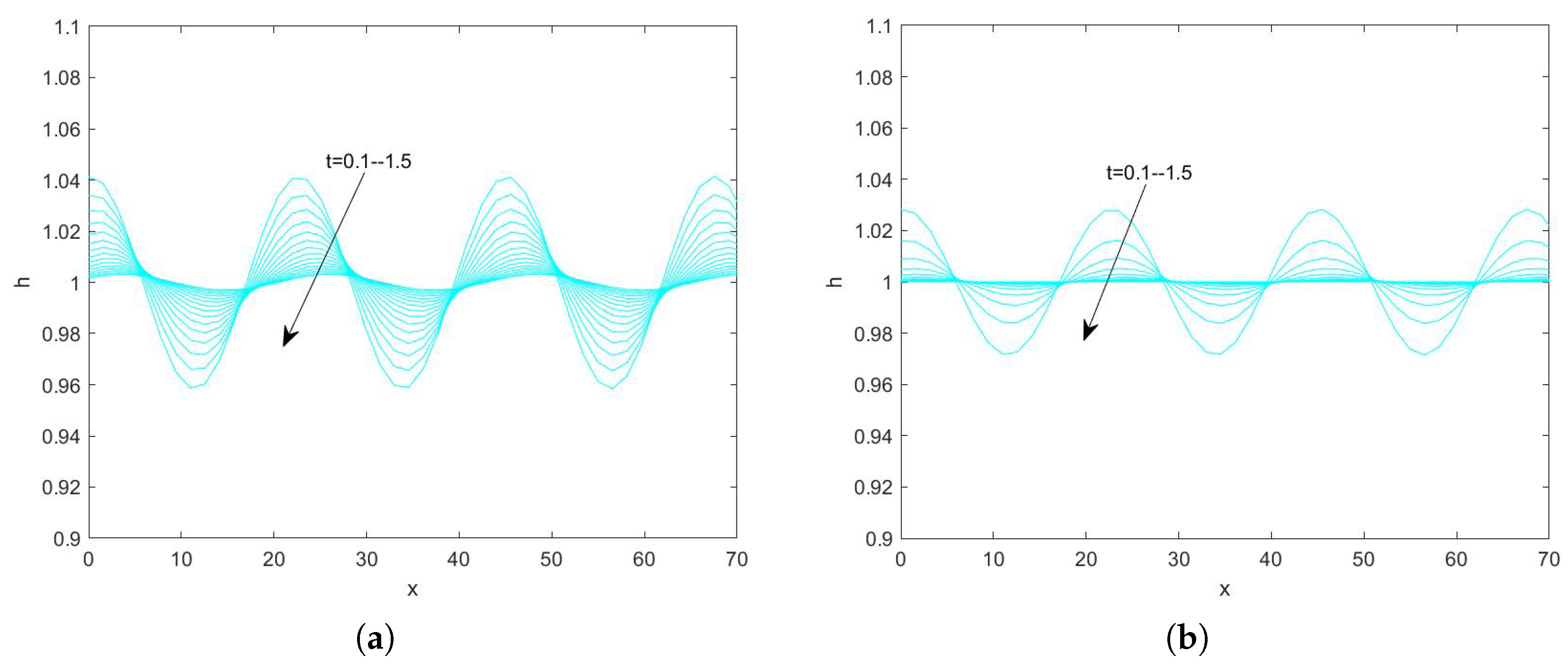
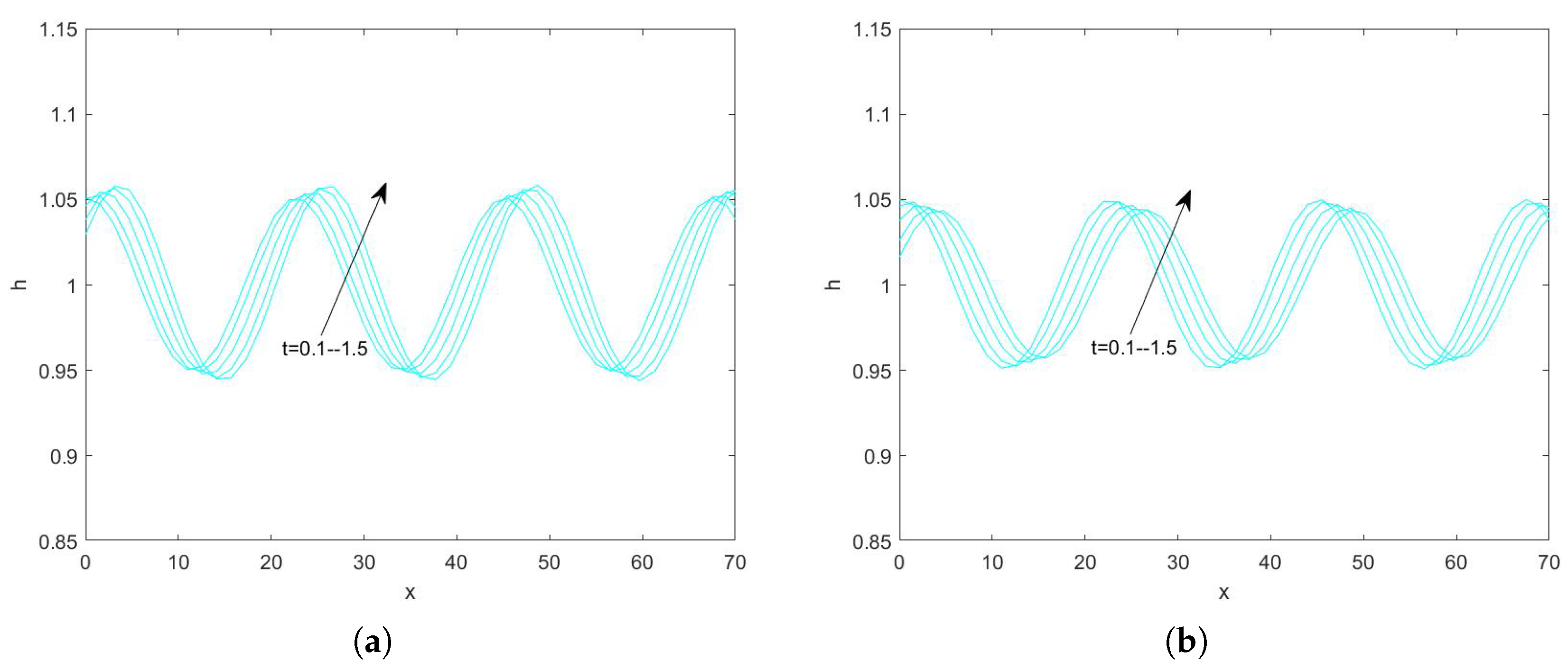
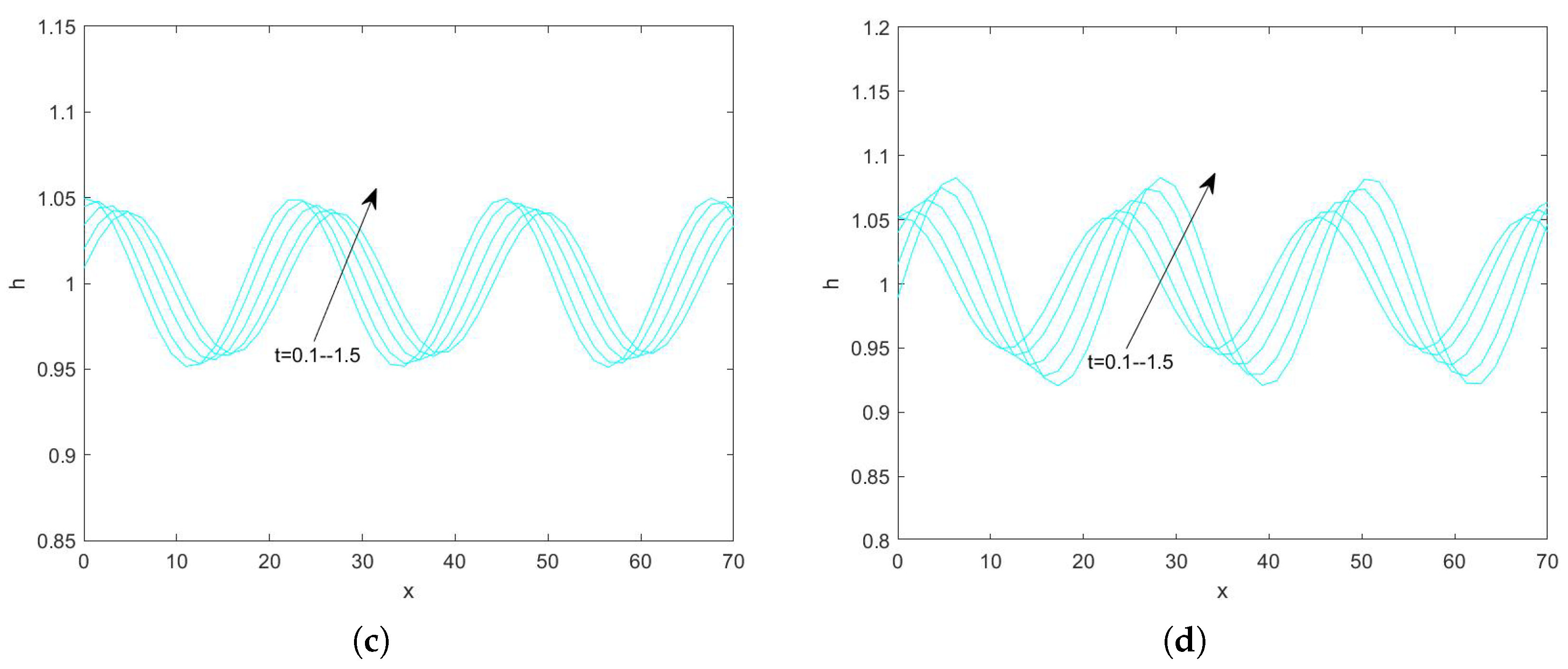
6. Numerical Analysis of Nonlinear Evolution Equations
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Mukhopadhyay, A.; Gaonkar, A.K. Effects of the variation of viscosity on the stability of thin liquid film flows along a uniformly heated substrate under heat flux boundary condition. Phys. Fluids 2023, 35, 052109. [Google Scholar] [CrossRef]
- Xue, D.; Zhang, R.; Liu, Q.; Ding, Z. Instability of liquid film with Odd Viscosity over a Non-Uniformly heated and corrugated substrate. Nanomaterials 2023, 13, 2660. [Google Scholar] [CrossRef] [PubMed]
- Issokolo, R.J.; Tchouobiap, S.E.M.; Nzoupe, F.N.; Dikande, A.M. Modulational instability in thin liquid film flowing down an inclined uniformly heated plate. AIP Adv. 2021, 11, 065017. [Google Scholar] [CrossRef]
- Xu, A.; Zhou, Z.; Hu, G. Molecular dynamics of dewetting of ultra-thin water films on solid substrate. Appl. Math. Mech.-Engl. Ed. 2007, 28, 1555–1559. [Google Scholar] [CrossRef]
- Datt, C.; Kansal, M.; Snoeijer, J.H. A thin-film equation for a viscoelastic fluid, and its application to the Landau-Levich problem. J. Nonnewton. Fluid. Mech. 2022, 305, 104816. [Google Scholar] [CrossRef]
- Chattopadhyay, S.; Mukhopadhyay, A. Weakly viscoelastic film flowing down a rotating inclined plane. Phys. Fluids 2022, 34, 012115. [Google Scholar] [CrossRef]
- Sekhar, S.; Shankar, V. Instability of ultrathin viscoelastic freestanding films. Phys. Fluids 2021, 33, 032115. [Google Scholar] [CrossRef]
- Sekhar, S.; Sharma, A.; Shankar, V. Instability and rupture of ultrathin freestanding viscoelastic solid films. Phys. Rev. E 2022, 106, 024803. [Google Scholar] [CrossRef]
- Dholy, S. Instabilities of a thin viscoelastic liquid film flowing down an inclined plane in the presence of a uniform electromagnetic field. Rheol. Acta 2017, 56, 325–340. [Google Scholar] [CrossRef]
- Oldroyd, J.G. Non-Newtonian effects in steady motion of some idealized elastico-viscous liquids. Proc. Math. Phys. Eng. Sci. 1958, 245, 278–297. [Google Scholar]
- Khan, M.; Hayat, T.; Asghar, S. Exact solution for MHD flow of a generalized Oldroyd-B fluid with modified Darcy’s law. Int. J. Eng. Sci. 2006, 44, 333–339. [Google Scholar] [CrossRef]
- Jia, B.; Xie, L.; Yang, L.; Fu, Q.; Cui, X. Linear instability of viscoelastic planar liquid sheets in the presence of gas velocity oscillations. J. Non-Newton. Fluid Mech. 2019, 273, 104169. [Google Scholar] [CrossRef]
- Moatimid, G.M.; Mostaphy, D.R. Nonlinear electrohydrodynamic instability through two jets of an Oldroydian viscoelastic fluids with a porous medium under the influence of electric field. AIP Adv. 2019, 5, 055302. [Google Scholar] [CrossRef]
- Moatimid, G.M.; El-Dib, Y. Nonlinear Kelvin-Helmholtz instability of Oldroydian viscoelastic fluid in porous media. Phys. A 2004, 333, 41–64. [Google Scholar] [CrossRef]
- Moatimid, G.M.; Alali, E.M.M.; Ali, H.S.M. Nonlinear instability of an Oldroyd elastico-viscous magnetic nanofluid saturated in a porous medium. Phys. Fluids 2014, 21, 092113. [Google Scholar] [CrossRef]
- Kang, F.; Chen, K. Nonlinear elastic instability of gravity-driven flow of a thin viscoelastic film down an inclined plane. J. Non-Newton. Fluid Mech. 1995, 60, 357–367. [Google Scholar] [CrossRef]
- Alkharashi, S.A.; Alrashidi, A. Dynamical behaviour of a porous liquid layer of an Oldroyd-B model flowing over an oscillatory heated substrate. Sādhanā 2019, 45, 7–23. [Google Scholar] [CrossRef]
- Song, Z.; Ding, Z. Elastic stability of viscoelastic liquid films flowing on a porous substrate. J. Non-Newton Fluid Mech. 2023, 322, 105147. [Google Scholar] [CrossRef]
- Hu, T.; Fu, Q.; Xing, Y.; Yang, L.; Xie, L. Stability of a thin viscoelastic film falling down an inclined plane. Phys. Rev. Fluids 2021, 6, 083902. [Google Scholar] [CrossRef]
- Avron, J.E. Odd viscosity. J. Stat. Phys. 1998, 92, 543–557. [Google Scholar] [CrossRef]
- Fruchart, M.; Scheibner, C.; Vitelli, V. Odd viscosity and odd elasticity. Annu. Rev. Condens. Matter. Phys. 2023, 14, 471–510. [Google Scholar] [CrossRef]
- Doak, A.; Baardink, G.; Milewski, M.A.; Souslov, A. Nonlinear shallow-water waves with vertical odd viscosity. Siam J. Appl. Math. 2023, 83, 938–965. [Google Scholar] [CrossRef]
- Yuan, H.; De La Cruz, M.O. Stokesian dynamics with odd viscosity. Phys. Rev. Fluids 2023, 8, 054101. [Google Scholar] [CrossRef]
- Kirkinis, E.; Andreev, A.V. Odd-viscosity-induced stabilization of viscous thin liquid films. J. Non-Newton Fluid Mech. 2019, 878, 169–189. [Google Scholar] [CrossRef]
- Chattopadhyay, S.; Desai, A.S.; Gaonkar, A.K.; Mukhopadhyay, A. Role of odd viscosity on falling films over compliant substrates. Phys. Rev. Fluids 2023, 8, 064003. [Google Scholar] [CrossRef]
- Bao, G.; Jian, Y. Odd-viscosity-induced instability of a falling thin film with an external electric field. Phys. Rev. E 2021, 103, 013104. [Google Scholar] [CrossRef] [PubMed]
- Chattopadhyay, S. Falling liquid film down a non-uniformly heated slippery inclined plane with odd viscosity effects. Int. J. Heat Mass Transf. 2024, 218, 124807. [Google Scholar] [CrossRef]
- Jia, B.; Jian, Y. The effect of odd-viscosity on Rayleigh-Taylor instability of a liquid film under a heated inclined substrate. Phys. Fluids 2022, 34, 044104. [Google Scholar] [CrossRef]
- Zhao, J.; Jian, Y. Effect of odd viscosity on the stability of thin viscoelastic liquid film flowing along an inclined plate. Phys. Scr. 2021, 96, 055214. [Google Scholar] [CrossRef]
- Whitaker, S. Flow in porous media I: A theoretical derivation of Darcy’s law. Transp. Porous Media 1986, 1, 3–25. [Google Scholar] [CrossRef]
- Sochi, T. Non-Newtonian flow in porous media. Polymer 2010, 51, 5007–5023. [Google Scholar] [CrossRef]
- Dimitrienko, Y.I.; Li, S. Mathematical simulation of local transfer for non-Newtonian fluid in porous fabrics. J. Phys. Conf. Ser. 2019, 1392, 012023. [Google Scholar] [CrossRef]
- Bowers, C.A.; Miller, C.T. Generalized Newtonian fluid flow in porous media. Phys. Rev. Fluids 2021, 6, 123302. [Google Scholar] [CrossRef] [PubMed]
- De Oliveira, H.B.; Paiva, A. Partial regularity of the solutions to a turbulent problem in porous media. Proc. Am. Math. Soc. 2019, 147, 3961–3981. [Google Scholar] [CrossRef]
- Khan, M.B.; Sasmal, C. Electro-elastic instability in electroosmotic flows of viscoelastic fluids through a model porous system. Eur. J. Mech. B Fluids 2023, 97, 173–186. [Google Scholar] [CrossRef]
- Kumawat, T.C.; Tiwari, N. Flow and stability of a gravity-driven thin film over a locally heated porous wall. Phys. Fluids 2020, 32, 092106. [Google Scholar] [CrossRef]
- Cherian, M.A.; Ghosh, S.; Mukhopadhyay, S. Hydrodynamic instability of flow through a rotating channel filled with isotropic porous media. Phys. Fluids 2022, 34, 094104. [Google Scholar] [CrossRef]
- Beavers, G.S.; Joseph, D.D. Boundary conditions at a naturally permeable wall. J. Fluid Mech. 1967, 30, 197–207. [Google Scholar] [CrossRef]
- Li, X.; Ding, Z. Instability of liquid film with odd viscosity and slip effect under the action of external electric field. Phys. Fluids 2023, 35, 092114. [Google Scholar] [CrossRef]
- Ashdhir, P.; Arya, J.; Rani, C.E. Exploring the fundamentals of fast Fourier transform technique and its elementary applications in physics. Eur. J. Phys. 2021, 42, 065805. [Google Scholar] [CrossRef]
- Dhawan, S.; Singh, B. Application of fast fourier transformation in numerical solution of differential equations. AIP Conf. Proc. 2024, 2986, 030092. [Google Scholar]
- Nguyen, T.K.; Monchiet, V.; Bonnet, G. A Fourier based numerical method for computing the dynamic permeability of periodic porous media. Eur. J. Mech. B Fluids 2013, 37, 90–98. [Google Scholar] [CrossRef]
- Nguyen, N.S.; Bignonnet, F. Numerical estimation of the permeability of granular soils using the DEM and LBM or FFT-based fluid computation method. Granul Matter 2023, 25, 53. [Google Scholar] [CrossRef]
- Chen, J. Spectrum features analysis of ocean wind and wave based on fast fourier transform algorithm. J. Coastal Res. 2018, 83, 418–422. [Google Scholar] [CrossRef]
- Khan, N.S.; Gul, T.; Khan, M.A.; Bonyah, E.; Islam, S. Mixed convection in gravity-driven thin film non-Newtonian nanofluids flow with gyrotactic microorganisms. Results Phys. 2017, 7, 4033–4049. [Google Scholar] [CrossRef]
- Jian, Y. Oscillatory squeeze flow through an Oldroyd-B fluid-saturated porous layer. Appl. Math. Mech.-Engl. Ed. 2024, 45, 2037–2054. [Google Scholar] [CrossRef]
- Agi, A.; Junin, R.; Gbonhinbor, J.; Onyekonwu, M. Natural polymer flow behaviour in porous media for enhanced oil recovery applications: A review. J. Pet Explor. Prod. Technol. 2018, 8, 1349–1362. [Google Scholar] [CrossRef]
- Wajihah, S.A.; Sankar, D.S. A review on non-Newtonian fluid models for multi-layered blood rheology in constricted arteries. Arch. Appl. Mech. 2023, 93, 1771–1796. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Q.; Liu, Q.; Zhang, R.; Ding, Z. Instability of Oldroyd-B Liquid Films with Odd Viscosity on Porous Inclined Substrates. Nanomaterials 2025, 15, 244. https://doi.org/10.3390/nano15030244
Zhou Q, Liu Q, Zhang R, Ding Z. Instability of Oldroyd-B Liquid Films with Odd Viscosity on Porous Inclined Substrates. Nanomaterials. 2025; 15(3):244. https://doi.org/10.3390/nano15030244
Chicago/Turabian StyleZhou, Qingqin, Quansheng Liu, Ruigang Zhang, and Zhaodong Ding. 2025. "Instability of Oldroyd-B Liquid Films with Odd Viscosity on Porous Inclined Substrates" Nanomaterials 15, no. 3: 244. https://doi.org/10.3390/nano15030244
APA StyleZhou, Q., Liu, Q., Zhang, R., & Ding, Z. (2025). Instability of Oldroyd-B Liquid Films with Odd Viscosity on Porous Inclined Substrates. Nanomaterials, 15(3), 244. https://doi.org/10.3390/nano15030244





