Temperature Dependence of Optical Properties of MoS2 and WS2 Heterostructures Assessed by Spectroscopic Ellipsometry
Abstract
1. Introduction
2. Materials and Methods
2.1. Sample Fabrication and Characterization
2.2. SE Temperature Dependence
3. Results and Discussion
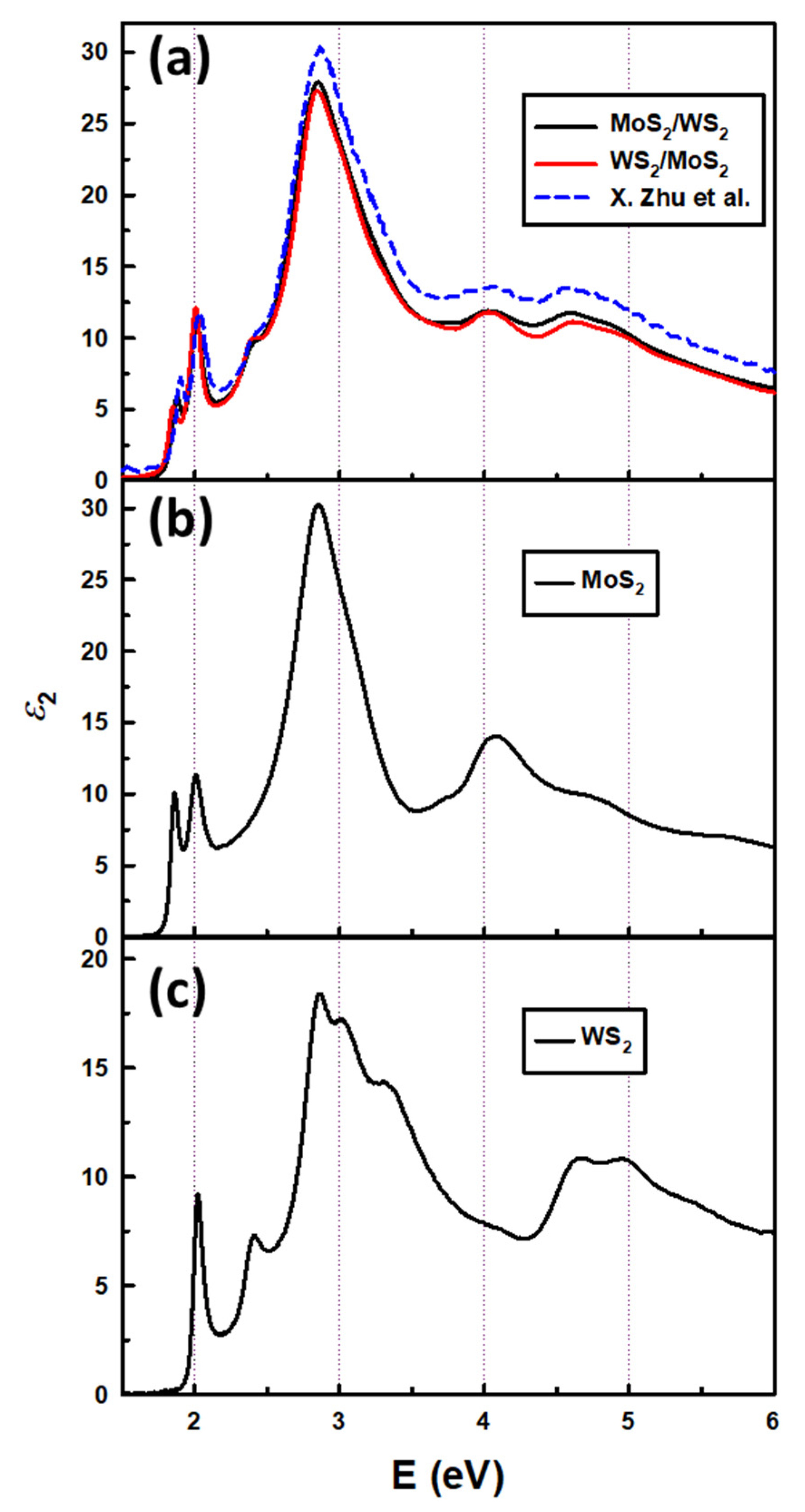
3.1. Analysis of <ε>
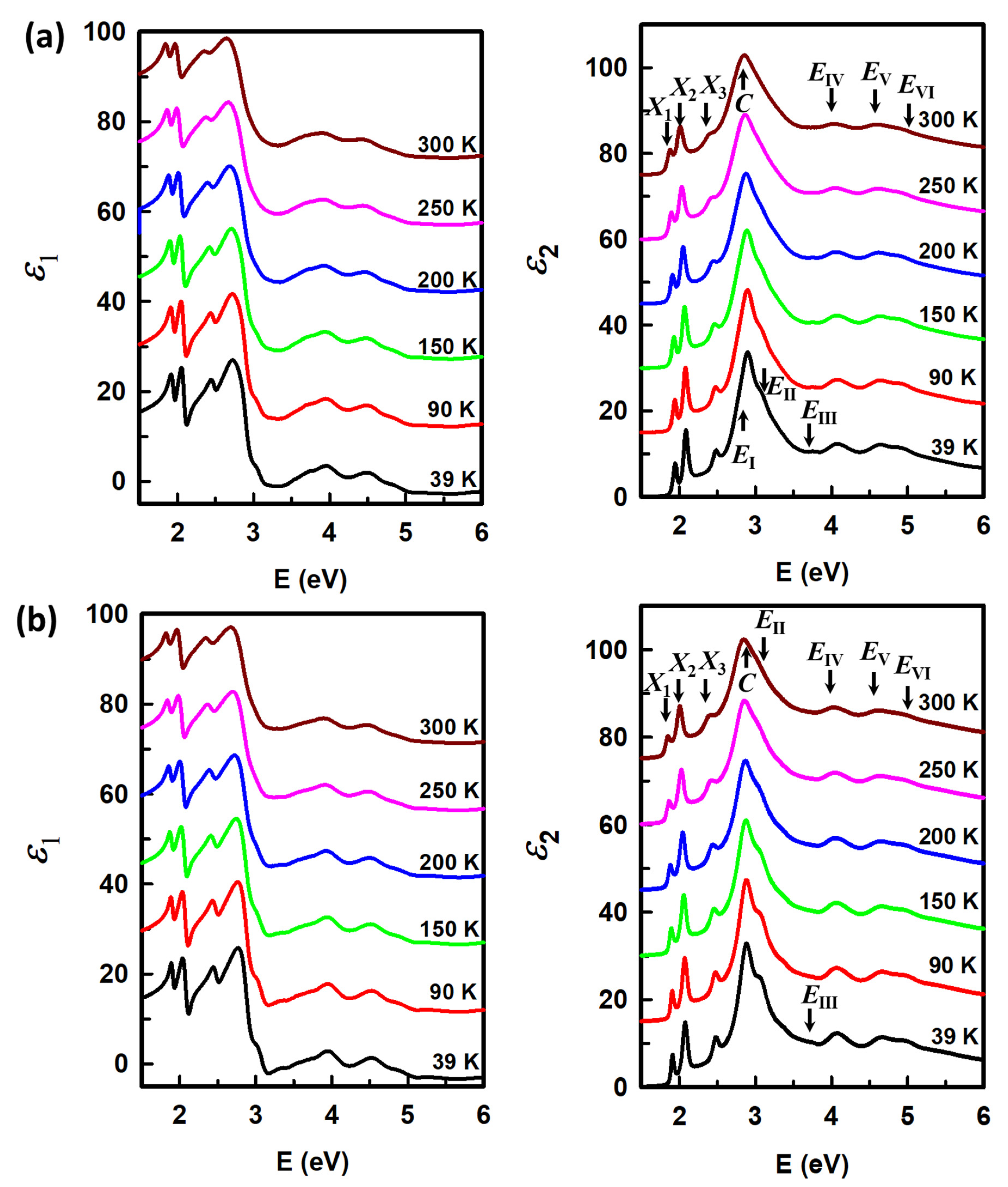
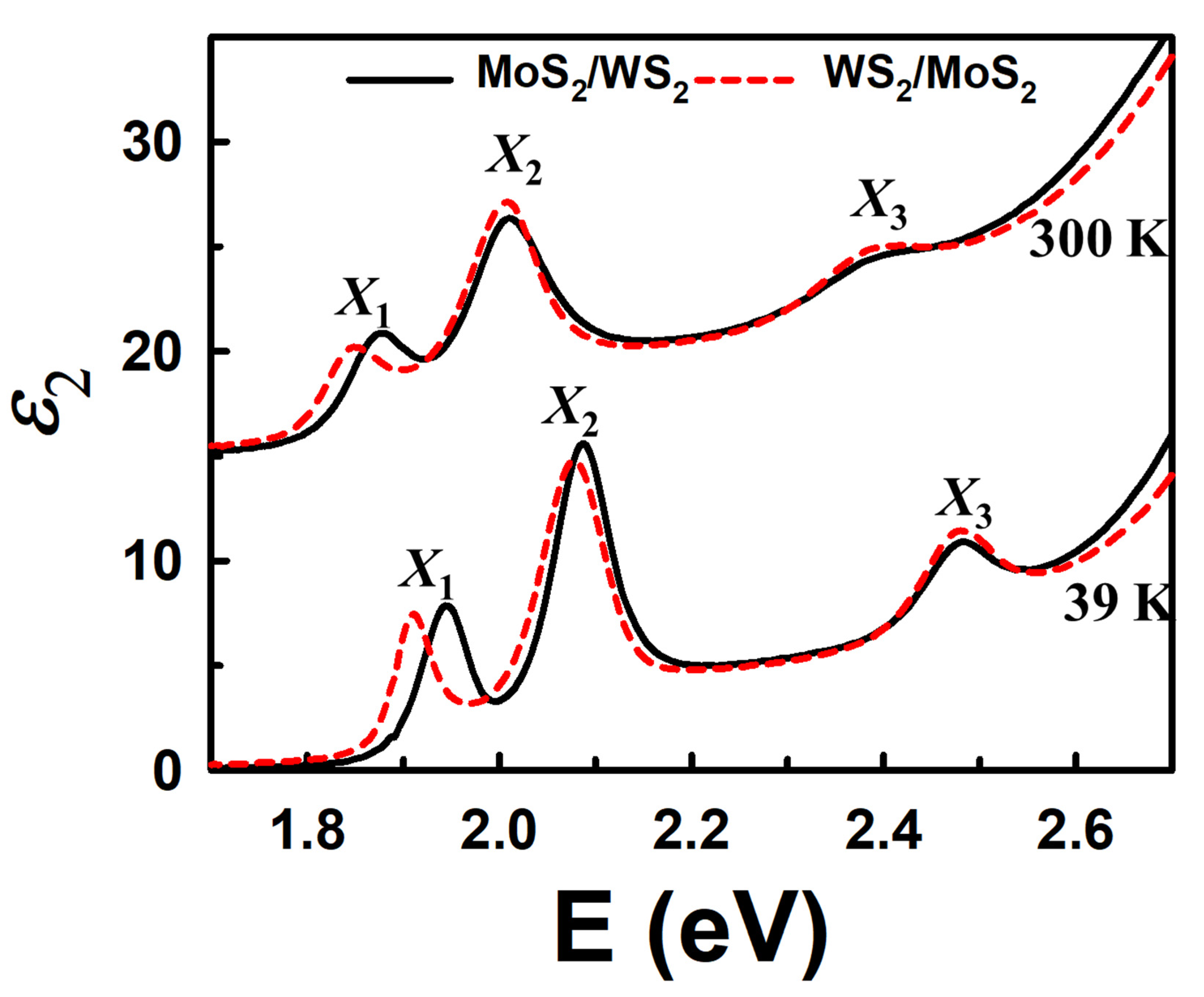
3.2. Critical-Point Analysis
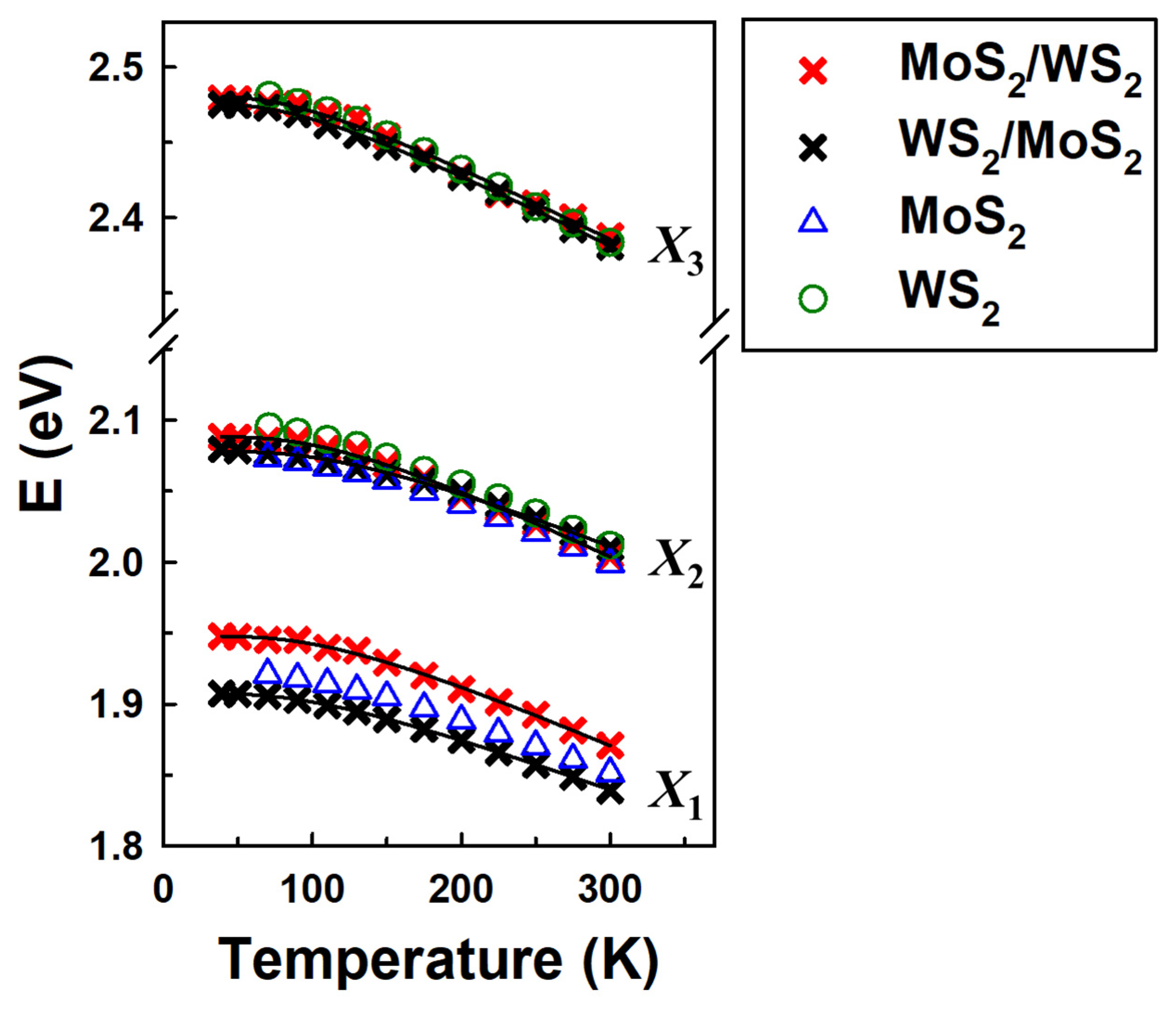
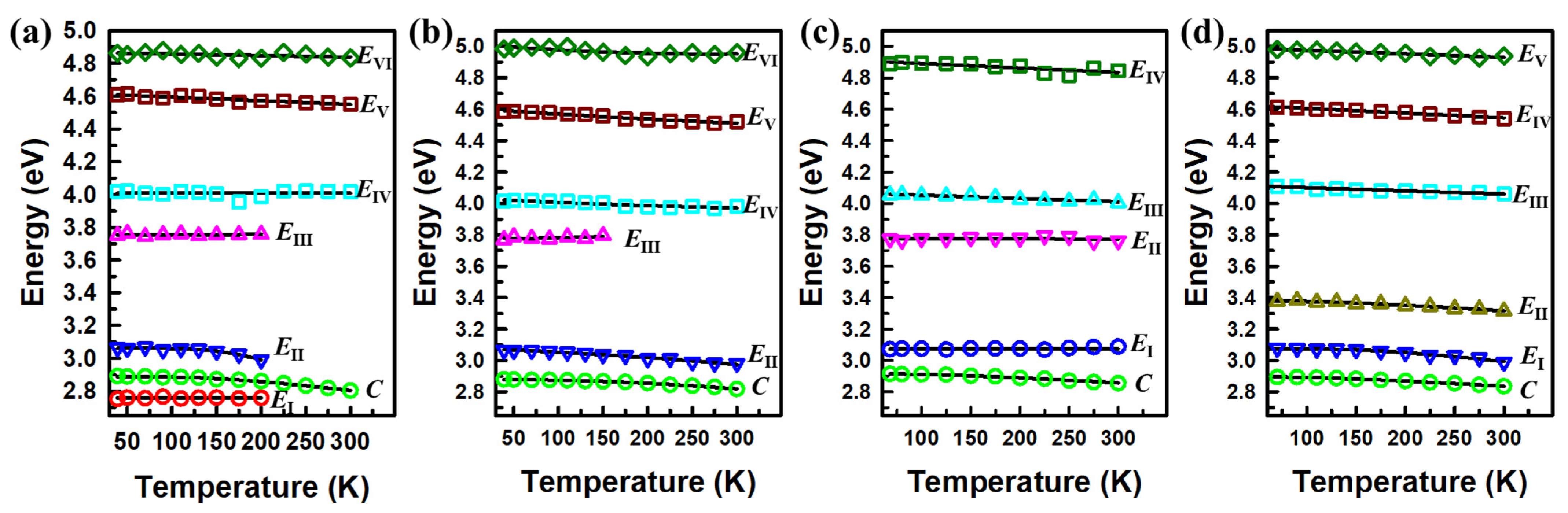
3.3. Temperature Dependence of Critical Point Energies
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pak, S.; Lee, J.; Lee, Y.-W.; Jang, A.-R.; Ahn, S.; Ma, K.Y.; Cho, Y.; Hong, J.; Lee, S.; Jeong, H.Y. Strain-mediated interlayer coupling effects on the excitonic behaviors in an epitaxially grown MoS2/WS2 van der Waals heterobilayer. Nano Lett. 2017, 17, 5634–5640. [Google Scholar] [CrossRef] [PubMed]
- Shi, Z.; Wang, X.; Sun, Y.; Li, Y.; Zhang, L. Interlayer coupling in two-dimensional semiconductor materials. Semicond. Sci. Technol. 2018, 33, 093001. [Google Scholar] [CrossRef]
- Yuan, L.; Chung, T.-F.; Kuc, A.; Wan, Y.; Xu, Y.; Chen, Y.P.; Heine, T.; Huang, L. Photocarrier generation from interlayer charge-transfer transitions in WS2-graphene heterostructures. Sci. Adv. 2018, 4, e1700324. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Hong, H.; Lian, C.; Ma, W.; Xu, X.; Zhou, X.; Fu, H.; Liu, K.; Meng, S. Interlayer-state-coupling dependent ultrafast charge transfer in MoS2/WS2 bilayers. Adv. Sci. 2017, 4, 1700086. [Google Scholar] [CrossRef]
- Wu, X.; Wang, X.; Li, H.; Zeng, Z.; Zheng, B.; Zhang, D.; Li, F.; Zhu, X.; Jiang, Y.; Pan, A. Vapor growth of WSe2/WS2 heterostructures with stacking dependent optical properties. Nano Res. 2019, 12, 3123–3128. [Google Scholar] [CrossRef]
- Kolobov, A.V.; Tominaga, J. TMDC Heterostructures. In Two-Dimensional Transition-Metal Dichalcogenides; Springer: Berlin/Heidelberg, Germany, 2016; pp. 447–471. [Google Scholar]
- Hong, X.; Kim, J.; Shi, S.-F.; Zhang, Y.; Jin, C.; Sun, Y.; Tongay, S.; Wu, J.; Zhang, Y.; Wang, F. Ultrafast charge transfer in atomically thin MoS2/WS2 heterostructures. Nat. Nanotechnol. 2014, 9, 682–686. [Google Scholar] [CrossRef]
- Li, Y.; Chernikov, A.; Zhang, X.; Rigosi, A.; Hill, H.M.; Van Der Zande, A.M.; Chenet, D.A.; Shih, E.-M.; Hone, J.; Heinz, T.F. Measurement of the optical dielectric function of monolayer transition-metal dichalcogenides: MoS2, MoSe2, WS2, and WSe2. Phys. Rev. B 2014, 90, 205422. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Nguyen, X.A.; Hoang, A.T.; Kim, T.J. Spectroscopic Ellipsometry Study of the Temperature Dependences of the Optical and Exciton Properties of MoS2 and WS2 Monolayers. Materials 2024, 17, 5455. [Google Scholar] [CrossRef]
- Park, H.G.; Kim, T.J.; Kim, H.S.; Yoo, C.H.; Barange, N.S.; Le, V.L.; Kim, H.U.; Senthilkumar, V.; Le, C.T.; Kim, Y.S. Temperature dependence of the critical points of monolayer MoS2 by ellipsometry. Appl. Spectrosc. Rev. 2016, 51, 621–635. [Google Scholar] [CrossRef]
- Gu, H.; Guo, Z.; Huang, L.; Fang, M.; Liu, S. Investigations of Optical Functions and Optical Transitions of 2D Semiconductors by Spectroscopic Ellipsometry and DFT. Nanomaterials 2023, 13, 196. [Google Scholar] [CrossRef]
- Ermolaev, G.A.; Yakubovsky, D.I.; Stebunov, Y.V.; Arsenin, A.V.; Volkov, V.S. Spectral ellipsometry of monolayer transition metal dichalcogenides: Analysis of excitonic peaks in dispersion. J. Vac. Sci. Technol. B 2020, 38, 014002. [Google Scholar] [CrossRef]
- Liu, H.-L.; Yang, T.; Chen, J.-H.; Chen, H.-W.; Guo, H.; Saito, R.; Li, M.-Y.; Li, L.-J. Temperature-dependent optical constants of monolayer MoS2, MoSe2, WS2, and WSe2: Spectroscopic ellipsometry and first-principles calculations. Sci. Rep. 2020, 10, 15282. [Google Scholar] [CrossRef]
- Zhu, X.; He, J.; Zhang, R.; Cong, C.; Zheng, Y.; Zhang, H.; Zhang, S.; Chen, L. Effects of dielectric screening on the excitonic and critical points properties of WS2/MoS2 heterostructures. Nanoscale 2020, 12, 23732–23739. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; Sun, M. Two-dimensional WS2/MoS2 heterostructures: Properties and applications. Nanoscale 2021, 13, 5594–5619. [Google Scholar] [CrossRef]
- Wurstbauer, U.; Miller, B.; Parzinger, E.; Holleitner, A.W. Light–matter interaction in transition metal dichalcogenides and their heterostructures. J. Phys. D Appl. Phys. 2017, 50, 173001. [Google Scholar] [CrossRef]
- Dell’Anna, L.; Merano, M. Optical response of a bilayer crystal. Phys. Rev. A 2019, 99, 013802. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Kim, T.J.; Park, H.G.; Le, V.L.; Nguyen, X.A.; Koo, D.; Lee, C.-H.; Cuong, D.D.; Hong, S.C.; Kim, Y.D. Temperature dependence of optical properties of monolayer WS2 by spectroscopic ellipsometry. Appl. Surf. Sci. 2020, 511, 145503. [Google Scholar] [CrossRef]
- Kim, T.J.; Hwang, S.Y.; Byun, J.S.; Diware, M.S.; Choi, J.; Park, H.G.; Kim, Y.D. Temperature dependence of the dielectric functions and the critical points of InSb by spectroscopic ellipsometry from 31 to 675 K. J. Appl. Phys. 2013, 114, 103501. [Google Scholar]
- Nguyen, X.A.; Le, L.V.; Kim, S.H.; Kim, Y.D.; Diware, M.S.; Kim, T.J.; Kim, Y.D. Temperature dependence of the dielectric function and critical points of monolayer WSe2. Sci. Rep. 2024, 14, 13486. [Google Scholar] [CrossRef]
- WS2-Full Area Monolayer on c-cut Sapphire. Available online: https://www.2dsemiconductors.com/full-area-coverage-monolayer-ws2-on-c-cut-sapphire/ (accessed on 12 December 2023).
- De Fazio, D.; Purdie, D.G.; Ott, A.K.; Braeuninger-Weimer, P.; Khodkov, T.; Goossens, S.; Taniguchi, T.; Watanabe, K.; Livreri, P.; Koppens, F.H. High-mobility, wet-transferred graphene grown by chemical vapor deposition. ACS Nano 2019, 13, 8926–8935. [Google Scholar] [CrossRef]
- Yu, Y.; Hu, S.; Su, L.; Huang, L.; Liu, Y.; Jin, Z.; Purezky, A.A.; Geohegan, D.B.; Kim, K.W.; Zhang, Y. Equally efficient interlayer exciton relaxation and improved absorption in epitaxial and nonepitaxial MoS2/WS2 heterostructures. Nano Lett. 2015, 15, 486–491. [Google Scholar] [CrossRef] [PubMed]
- Yang, W.; Kawai, H.; Bosman, M.; Tang, B.; Chai, J.; Le Tay, W.; Yang, J.; Seng, H.L.; Zhu, H.; Gong, H. Interlayer interactions in 2D WS2/MoS2 heterostructures monolithically grown by in situ physical vapor deposition. Nanoscale 2018, 10, 22927–22936. [Google Scholar] [CrossRef] [PubMed]
- Unuchek, D.; Ciarrocchi, A.; Avsar, A.; Sun, Z.; Watanabe, K.; Taniguchi, T.; Kis, A. Valley-polarized exciton currents in a van der Waals heterostructure. Nat. Nanotechnol. 2019, 14, 1104–1109. [Google Scholar] [CrossRef] [PubMed]
- Bai, Y.; Zhou, L.; Wang, J.; Wu, W.; McGilly, L.J.; Halbertal, D.; Lo, C.F.B.; Liu, F.; Ardelean, J.; Rivera, P. Excitons in strain-induced one-dimensional moiré potentials at transition metal dichalcogenide heterojunctions. Nat. Mater. 2020, 19, 1068–1073. [Google Scholar] [CrossRef]
- Nourbakhsh, A.; Zubair, A.; Sajjad, R.N.; Tavakkoli KG, A.; Chen, W.; Fang, S.; Ling, X.; Kong, J.; Dresselhaus, M.S.; Kaxiras, E. MoS2 field-effect transistor with sub-10 nm channel length. Nano Lett. 2016, 16, 7798–7806. [Google Scholar] [CrossRef]
- Najmaei, S.; Liu, Z.; Ajayan, P.; Lou, J. Thermal effects on the characteristic Raman spectrum of molybdenum disulfide (MoS2) of varying thicknesses. Appl. Phys. Lett. 2012, 100, 013106. [Google Scholar] [CrossRef]
- Savitzky, A.; Golay, M.J.E. Smoothing and differentiation of data by simplified least squares procedures. Anal. Chem. 1964, 36, 1627–1639. [Google Scholar] [CrossRef]
- Liu, F. Direct determination of momentum-resolved electron transfer in photoexcited MoS2/WS2 van der Waals heterobilayer. Phys. Rev. B 2020, 101, 201405. [Google Scholar] [CrossRef]
- Lautenschlager, P.; Garriga, M.; Cardona, M. Temperature dependence of the interband critical-point parameters of InP. Phys. Rev. B 1987, 36, 4813. [Google Scholar] [CrossRef]


| CP Energies (eV) | MoS2/WS2 | WS2/MoS2 | CP Energies (eV) | MoS2 | CP Energies (eV) | WS2 | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| 39 K | 300 K | 39 K | 300 K | 70 K | 300 K | 70 K | 300 K | |||
| X1 | 1.95 | 1.87 | 1.91 | 1.84 | A− | 1.92 | 1.85 | A− | - | - |
| X2 | 2.09 | 2.00 | 2.08 | 2.01 | A0 | - | - | A0 | 2.10 | 2.01 |
| B− | 2.08 | 2.00 | B− | - | - | |||||
| X3 | 2.48 | 2.39 | 2.48 | 2.38 | B0 | - | - | B0 | 2.48 | 2.38 |
| EI | 2.75 | - | - | - | - | - | - | E0 * | 2.79 * | 2.72 * |
| C | 2.89 | 2.80 | 2.88 | 2.82 | C | 2.91 | 2.86 | C | 2.90 | 2.84 |
| EII | 3.06 | - | 3.07 | 2.98 | EI | 3.07 | 3.09 | EI | 3.08 | 2.98 |
| EII | 3.38 | 3.25 | ||||||||
| EIII | 3.75 | - | 3.77 | - | EII | 3.77 | 3.76 | |||
| EIV | 4.02 | 4.02 | 4.01 | 4.01 | EIII | 4.05 | 4.00 | EIII | 4.11 | 4.06 |
| EV | 4.61 | 4.55 | 4.58 | 4.52 | EIV | 4.61 | 4.54 | |||
| EVI | 4.86 | 4.83 | 4.98 | 4.96 | EIV | 4.89 | 4.84 | EV | 4.98 | 4.94 |
| Exciton | EB (eV) | aB (meV) | Θ (K) | EL (eV) | λ (10−4 eVK−1) |
|---|---|---|---|---|---|
| X1 | 2.03 | 84 | 347 | - | - |
| X2 | 2.18 | 84 | 352 | - | - |
| X3 | 2.55 | 70 | 273 | - | - |
| EI | - | - | - | 2.76 | 0.06 |
| C | 3.11 | 218 | 545 | - | - |
| EII | 5.09 | 202 | 814 | - | - |
| EIII | - | - | - | 3.75 | 0.20 |
| EIV | - | - | - | 4.01 | 0.01 |
| EV | - | - | - | 4.62 | 2.23 |
| EVI | - | - | - | 4.87 | 0.95 |
| Exciton | EB (eV) | aB (meV) | Θ (K) | EL (eV) | λ (10−4 eVK−1) |
|---|---|---|---|---|---|
| X1 | 1.97 | 62 | 312 | - | - |
| X2 | 2.16 | 82 | 371 | - | - |
| X3 | 2.54 | 68 | 267 | - | - |
| C | 2.98 | 106 | 463 | - | - |
| EII | 3.11 | 51 | 225 | - | - |
| EIII | - | - | - | 3.77 | 1.09 |
| EIV | - | - | - | 4.03 | 1.99 |
| EV | - | - | - | 4.60 | 3.11 |
| EVI | - | - | - | 5.00 | 1.86 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, H.T.; Le, V.L.; Nguyen, T.M.; Bui, X.K.; Nguyen, T.G.; Nguyen, N.L.; Nguyen, X.A.; Kim, T.J. Temperature Dependence of Optical Properties of MoS2 and WS2 Heterostructures Assessed by Spectroscopic Ellipsometry. Nanomaterials 2025, 15, 76. https://doi.org/10.3390/nano15010076
Nguyen HT, Le VL, Nguyen TM, Bui XK, Nguyen TG, Nguyen NL, Nguyen XA, Kim TJ. Temperature Dependence of Optical Properties of MoS2 and WS2 Heterostructures Assessed by Spectroscopic Ellipsometry. Nanomaterials. 2025; 15(1):76. https://doi.org/10.3390/nano15010076
Chicago/Turabian StyleNguyen, Hoang Tung, Van Long Le, Thi Mai Nguyen, Xuan Khuyen Bui, Thi Giang Nguyen, Nhat Linh Nguyen, Xuan Au Nguyen, and Tae Jung Kim. 2025. "Temperature Dependence of Optical Properties of MoS2 and WS2 Heterostructures Assessed by Spectroscopic Ellipsometry" Nanomaterials 15, no. 1: 76. https://doi.org/10.3390/nano15010076
APA StyleNguyen, H. T., Le, V. L., Nguyen, T. M., Bui, X. K., Nguyen, T. G., Nguyen, N. L., Nguyen, X. A., & Kim, T. J. (2025). Temperature Dependence of Optical Properties of MoS2 and WS2 Heterostructures Assessed by Spectroscopic Ellipsometry. Nanomaterials, 15(1), 76. https://doi.org/10.3390/nano15010076









