Steering of Vortices by Magnetic Field Tilting in Open Superconductor Nanotubes
Abstract
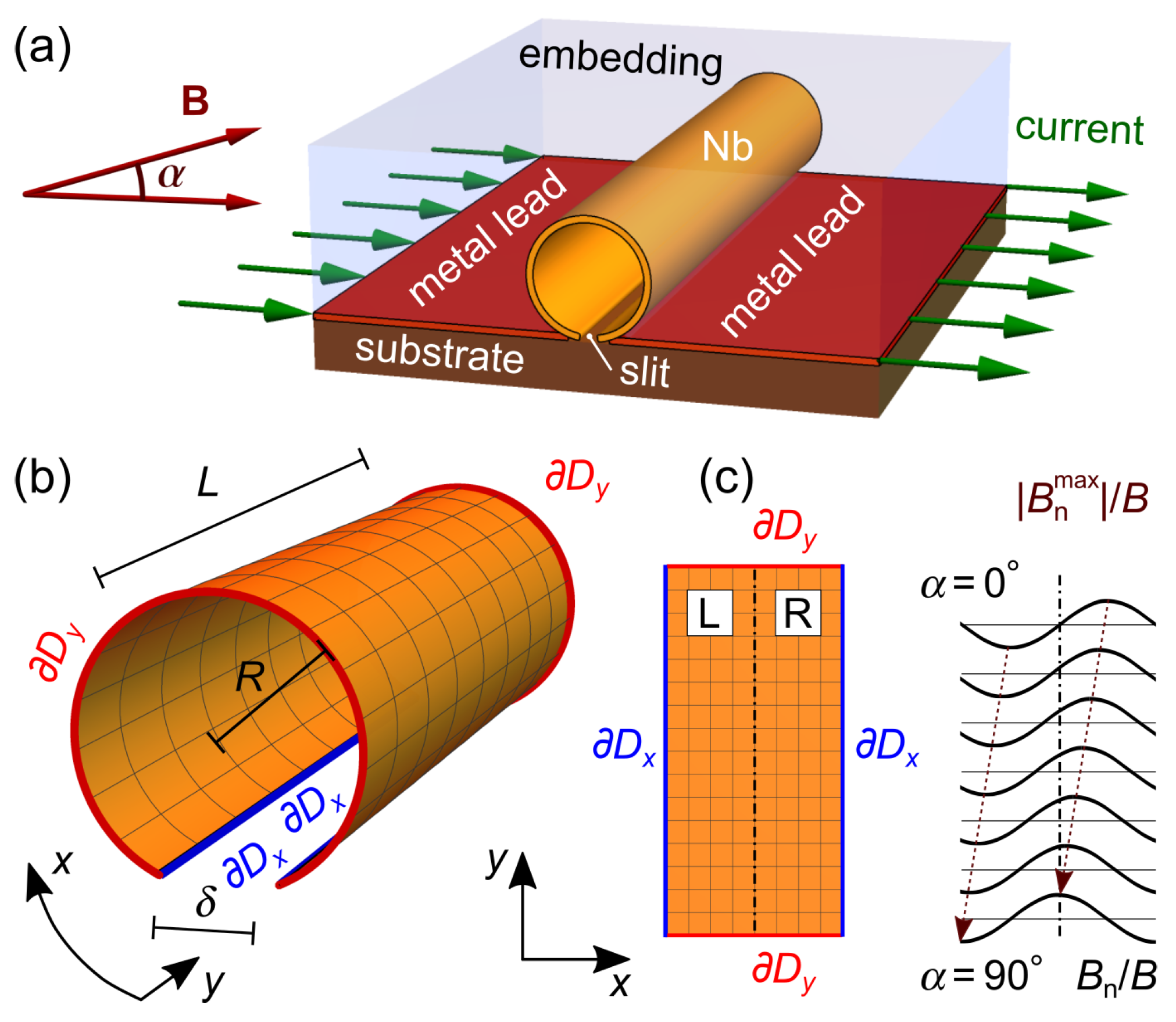
1. Introduction
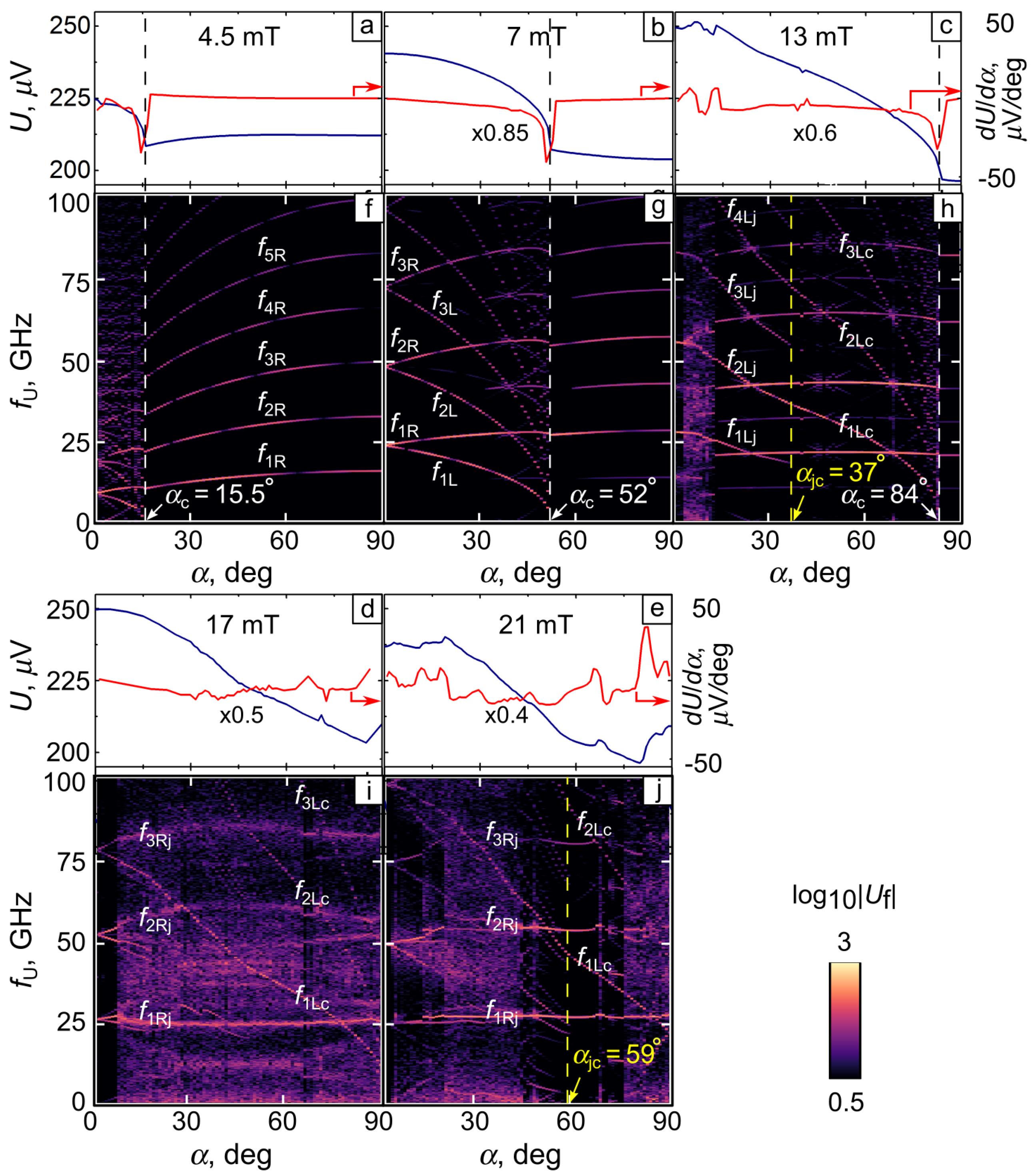
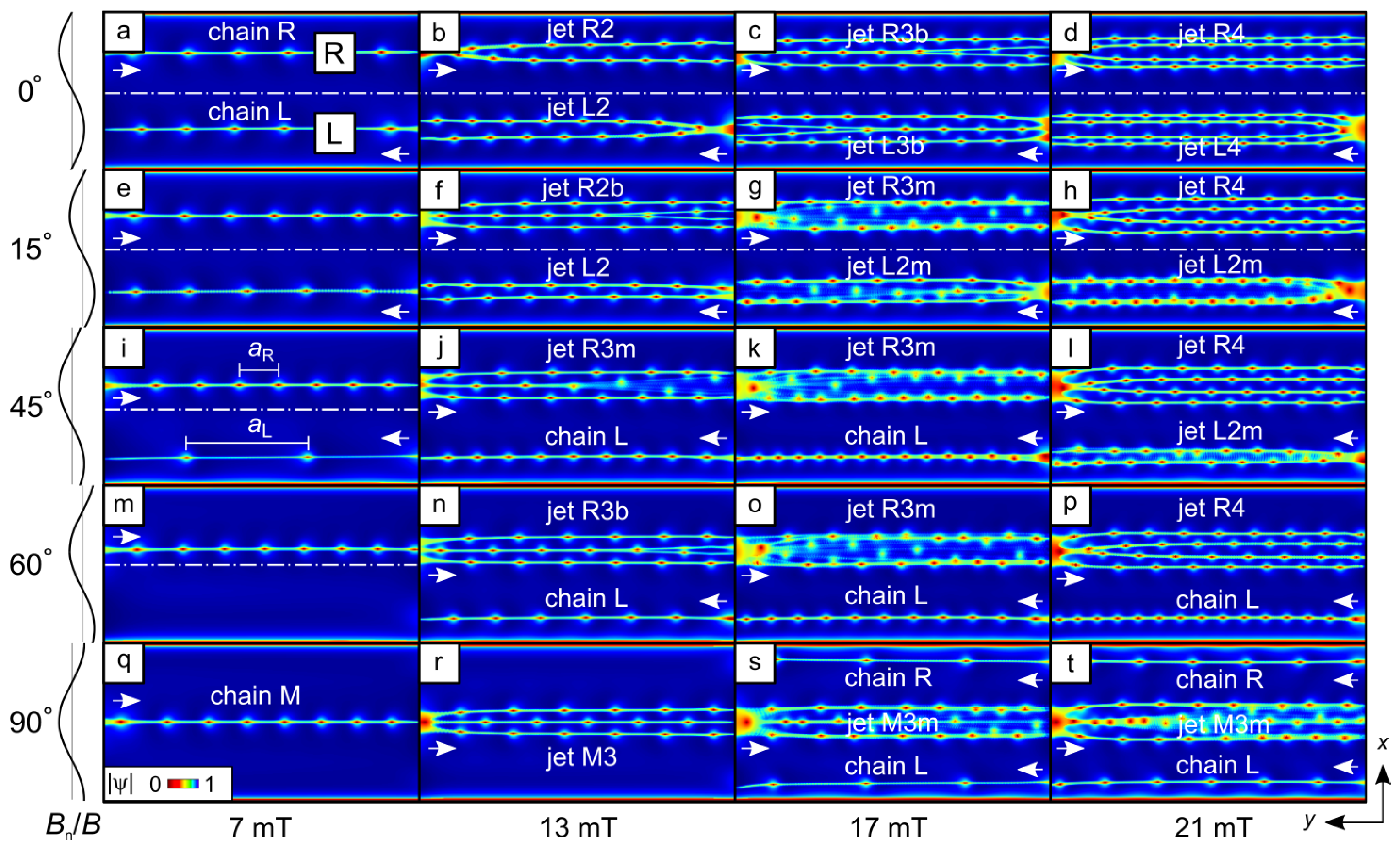
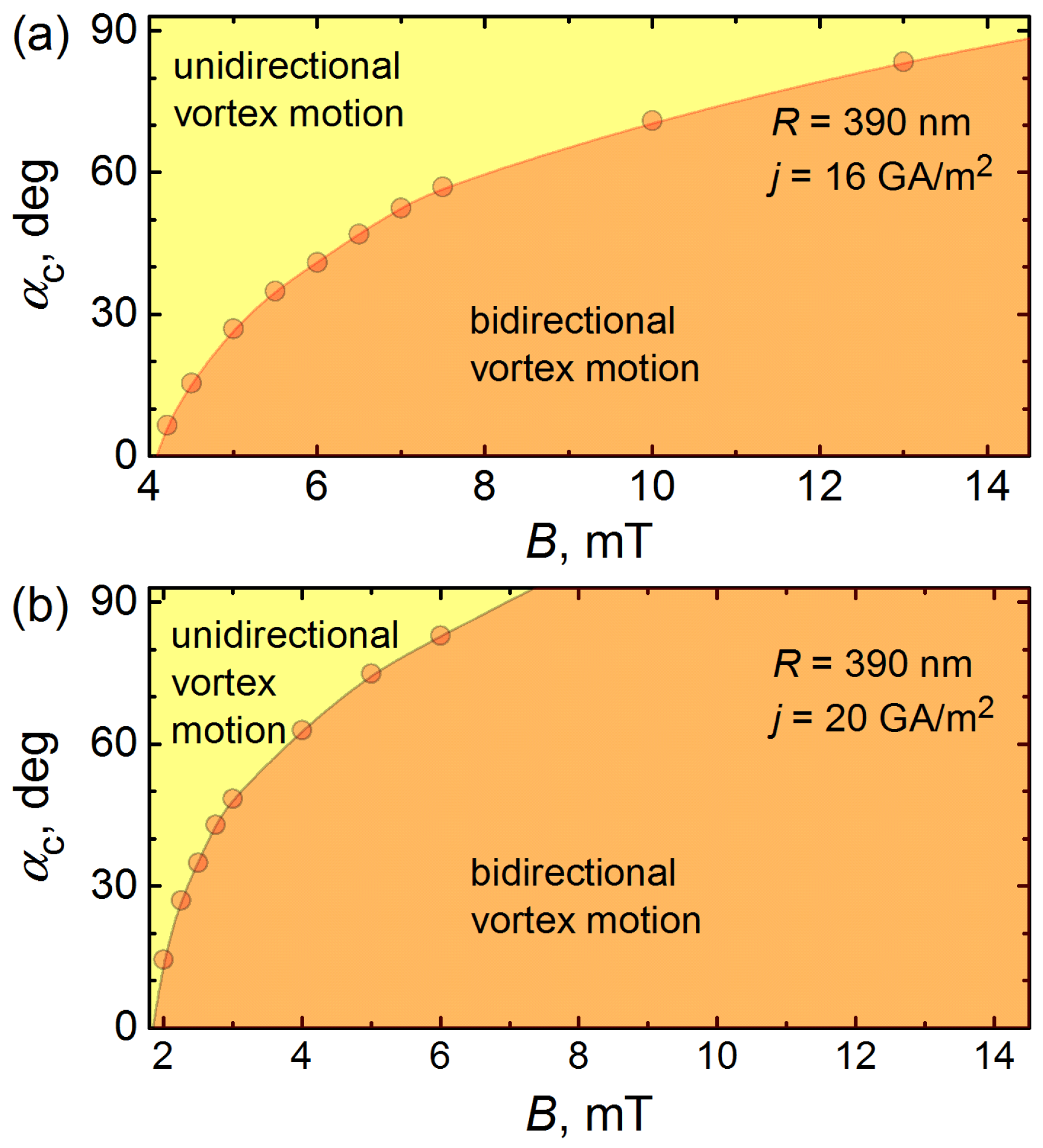
2. Results
3. Methods
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Brandt, E.H. The flux-line lattice in superconductors. Rep. Progr. Phys. 1995, 58, 1465. [Google Scholar] [CrossRef]
- Reichhardt, C.; Olson, C.J.; Nori, F. Dynamic Phases of Vortices in Superconductors with Periodic Pinning. Phys. Rev. Lett. 1997, 78, 2648–2651. [Google Scholar] [CrossRef]
- Guillamón, I.; Suderow, H.; Fernández-Pacheco, A.; Sesé, J.; Córdoba, R.; De Teresa, J.M.; Ibarra, M.R.; Vieira, S. Direct observation of melting in a two-dimensional superconducting vortex lattice. Nat. Phys. 2009, 5, 651–655. [Google Scholar] [CrossRef]
- Silhanek, A.V.; Milošević, M.V.; Kramer, R.B.G.; Berdiyorov, G.R.; Van de Vondel, J.; Luccas, R.F.; Puig, T.; Peeters, F.M.; Moshchalkov, V.V. Formation of Stripelike Flux Patterns Obtained by Freezing Kinematic Vortices in a Superconducting Pb Film. Phys. Rev. Lett. 2010, 104, 017001. [Google Scholar] [CrossRef] [PubMed]
- Cerbu, D.; Gladilin, V.N.; Cuppens, J.; Fritzsche, J.; Tempere, J.; Devreese, J.T.; Moshchalkov, V.V.; Silhanek, A.V.; Van de Vondel, J. Vortex ratchet induced by controlled edge roughness. New J. Phys. 2013, 15, 063022. [Google Scholar]
- Vodolazov, D.Y.; Ilin, K.; Merker, M.; Siegel, M. Defect-controlled vortex generation in current-carrying narrow superconducting strips. Supercond. Sci. Technol. 2015, 29, 025002. [Google Scholar] [CrossRef]
- Clem, J.R.; Berggren, K.K. Geometry-dependent critical currents in superconducting nanocircuits. Phys. Rev. B 2011, 84, 174510. [Google Scholar] [CrossRef]
- Adami, O.A.; Cerbu, D.; Cabosart, D.; Motta, M.; Cuppens, J.; Ortiz, W.A.; Moshchalkov, V.V.; Hackens, B.; Delamare, R.; Van de Vondel, J.; et al. Current crowding effects in superconducting corner-shaped Al microstrips. Appl. Phys. Lett. 2013, 102, 052603. [Google Scholar] [CrossRef]
- Embon, L.; Anahory, Y.; Jelic, Z.L.; Lachman, E.O.; Myasoedov, Y.; Huber, M.E.; Mikitik, G.P.; Silhanek, A.V.; Milosevic, M.V.; Gurevich, A.; et al. Imaging of super-fast dynamics and flow instabilities of superconducting vortices. Nat. Commun. 2017, 8, 85. [Google Scholar] [CrossRef]
- Friesen, M.; Gurevich, A. Nonlinear current flow in superconductors with restricted geometries. Phys. Rev. B 2001, 63, 064521. [Google Scholar] [CrossRef]
- Ustavschikov, S.S.; Levichev, M.Y.; Pashenkin, I.Y.; Gusev, N.S.; Gusev, S.A.; Vodolazov, D.Y. Negative differential resistance and Shapiro steps in superconducting MoN strip with slit. JETP Lett. 2022, 135, 226. [Google Scholar] [CrossRef]
- Aladyshkin, A.; Mel’nikov, A.S.; Shereshevsky, I.A.; Tokman, I.D. What is the best gate for vortex entry into type-II superconductor? Phys. C 2001, 361, 67. [Google Scholar] [CrossRef]
- Bezuglyj, A.I.; Shklovskij, V.A.; Budinská, B.; Aichner, B.; Bevz, V.M.; Mikhailov, M.Y.; Vodolazov, D.Y.; Lang, W.; Dobrovolskiy, O.V. Vortex jets generated by edge defects in current-carrying superconductor thin strips. Phys. Rev. B 2022, 105, 214507. [Google Scholar] [CrossRef]
- Meservey, R.; Meyers, L. Phase Transition of Thin-Film Superconducting Cylinders in a Magnetic Field. II. Angular Dependence. Phys. Rev. B 1972, 6, 2632–2642. [Google Scholar] [CrossRef]
- Yeo, J.; Moore, M.A. Noninteger flux quanta for a spherical superconductor. Phys. Rev. B 1998, 57, 10785–10789. [Google Scholar] [CrossRef]
- Du, Q.; Ju, L. Numerical simulations of the quantized vortices on a thin superconducting hollow sphere. J. Comput. Phys. 2004, 201, 511–530. [Google Scholar] [CrossRef]
- Hayashi, M.; Ebisawa, H.; Kuboki, K. Superconductivity on a Möbius strip: Numerical studies of order parameter and quasiparticles. Phys. Rev. B 2005, 72, 024505. [Google Scholar] [CrossRef]
- de Romaguera, A.R.C.; Doria, M.M.; Peeters, F.M. Transverse magnetization and torque in asymmetrical mesoscopic superconductors. Phys. Rev. B 2007, 76, 020505. [Google Scholar] [CrossRef]
- Xu, B.; Milošević, M.V.; Peeters, F.M. Magnetic properties of vortex states in spherical superconductors. Phys. Rev. B 2008, 77, 144509. [Google Scholar] [CrossRef]
- Tempere, J.; Gladilin, V.N.; Silvera, I.F.; Devreese, J.T.; Moshchalkov, V.V. Coexistence of the Meissner and vortex states on a nanoscale superconducting spherical shell. Phys. Rev. B 2009, 79, 134516. [Google Scholar] [CrossRef]
- Qiu, C.; Qian, T. Numerical study of the phase slips in ultrathin doubly connected superconducting cylinders. Phys. Rev. B 2009, 79, 054513. [Google Scholar] [CrossRef]
- Mawatari, Y. Field distributions in curved superconducting tapes conforming to a cylinder carrying transport currents. Phys. Rev. B 2009, 80, 184508. [Google Scholar] [CrossRef]
- Sabatino, P.; Carapella, G.; Costabile, G. Magneto-transport properties of curved mesoscopic superconducting strips. Supercond. Sci. Technol. 2011, 24, 125007. [Google Scholar] [CrossRef]
- Gladilin, V.N.; Tempere, J.; Devreese, J.T.; Moshchalkov, V.V. Aharonov-Bohm oscillations in the vortex dynamics in superconducting hollow cylinders. Phys. Rev. B 2012, 86, 104508. [Google Scholar] [CrossRef]
- Gladilin, V.; Tempere, J.; Devreese, J.; Moshchalkov, V. Negative-μ regime in the ac magnetic response of superconductor nanoshells. Sol. Stat. Commun. 2012, 152, 1781–1785. [Google Scholar] [CrossRef][Green Version]
- Qin, F.; Shi, W.; Ideue, T.; Yoshida, M.; Zak, A.; Tenne, R.; Kikitsu, T.; Inoue, D.; Hashizume, D.; Iwasa, Y. Superconductivity in a chiral nanotube. Nat. Commun. 2017, 8, 14465. [Google Scholar] [CrossRef] [PubMed]
- Kvorning, T.; Hansson, T.H.; Quelle, A.; Smith, C.M. Proposed Spontaneous Generation of Magnetic Fields by Curved Layers of a Chiral Superconductor. Phys. Rev. Lett. 2018, 120, 217002. [Google Scholar] [CrossRef]
- Bogusch, I.; Dobrovolskiy, O.V.; Fomin, V.M. Microwave generation and vortex jets in superconductor nanotubes. arXiv 2023, arXiv:2311.02946. [Google Scholar]
- Fomin, V.M.; Rezaev, R.O.; Dobrovolskiy, O.V. Topological transitions in ac/dc-driven superconductor nanotubes. Sci. Rep. 2022, 12, 10069. [Google Scholar] [CrossRef]
- Bogush, I.; Fomin, V.M. Topological defects in open superconducting nanotubes after gradual and abrupt switching of the transport current and magnetic field. Phys. Rev. B 2022, 105, 094511. [Google Scholar] [CrossRef]
- Thurmer, D.J.; Deneke, C.; Schmidt, O.G. In situ monitoring of the complex rolling behaviour of InGaAs/GaAs/Nb hybrid microtubes. J. Phys. D Appl. Phys. 2008, 41, 205419. [Google Scholar] [CrossRef]
- Thurmer, D.J.; Bufon, C.C.B.; Deneke, C.; Schmidt, O.G. Nanomembrane-Based Mesoscopic Superconducting Hybrid Junctions. Nano Lett. 2010, 10, 3704–3709. [Google Scholar] [CrossRef] [PubMed]
- Lösch, S.; Alfonsov, A.; Dobrovolskiy, O.V.; Keil, R.; Engemaier, V.; Baunack, S.; Li, G.; Schmidt, O.G.; Bürger, D. Microwave Radiation Detection with an Ultra-Thin Free-Standing Superconducting Niobium Nanohelix. ACS Nano 2019, 13, 2948. [Google Scholar] [CrossRef] [PubMed]
- Grimaldi, G.; Leo, A.; Avitabile, F.; Martucciello, N.; Galluzzi, A.; Polichetti, M.; Pace, S.; Nigro, A. Vortex lattice instability at the nanoscale in a parallel magnetic field. Nanotechnology 2019, 30, 424001. [Google Scholar] [CrossRef]
- Peroz, C.; Villard, C. Flux flow properties of niobium thin films in clean and dirty superconducting limits. Phys. Rev. B 2005, 72, 014515. [Google Scholar] [CrossRef]
- Ferrari, G.; Cuoghi, G. Schrödinger Equation for a Particle on a Curved Surface in an Electric and Magnetic Field. Phys. Rev. Lett. 2008, 100, 230403. [Google Scholar] [CrossRef]
- Thinkham, M. Introduction to Superconductivity; McGraw Hill: New York, NY, USA, 1996. [Google Scholar]
- Sadovskyy, I.A.; Koshelev, A.E.; Phillips, C.L.; Karpeyev, D.A.; Glatz, A. Stable large-scale solver for Ginzburg–Landau equations for superconductors. J. Comp. Phys. 2015, 294, 639. [Google Scholar] [CrossRef]
- Kato, R.; Enomoto, Y.; Maekawa, S. Effects of the surface boundary on the magnetization process in type-II superconductors. Phys. Rev. B 1993, 47, 8016. [Google Scholar] [CrossRef]
- Mayadas, A.F.; Laibowitz, R.B.; Cuomo, J.J. Electrical characteristics of rf-sputtered single-crystal niobium films. J. Appl. Phys. 1972, 43, 1287. [Google Scholar] [CrossRef]
- Olson, C.J.; Reichhardt, C.; Nori, F. Superconducting vortex avalanches, voltage bursts, and vortex plastic flow: Effect of the microscopic pinning landscape on the macroscopic properties. Phys. Rev. B 1997, 56, 6175–6194. [Google Scholar] [CrossRef]
- Glatz, A.; Vlasko-Vlasov, V.K.; Kwok, W.K.; Crabtree, G.W. Vortex cutting in superconductors. Phys. Rev. B 2016, 94, 064505. [Google Scholar] [CrossRef]
- Velez, M.; Martin, J.I.; Villegas, J.E.; Hoffmann, A.; Gonzalez, E.M.; Vicent, J.L.; Schuller, I.K. Superconducting vortex pinning with artificial magnetic nanostructures. J. Magn. Magnet. Mat. 2008, 320, 2547–2562. [Google Scholar] [CrossRef]
- Staas, F.A.; Niessen, A.K.; Druyvesteyn, W.F.; Suchtelen, J.V. Guided motion of vortices in type II superconductors. Phys. Lett. 1964, 13, 293–295. [Google Scholar] [CrossRef]
- Dobrovolskiy, O.V.; Huth, M.; Shklovskij, V.A. Anisotropic magnetoresistive response in thin Nb films decorated by an array of Co stripes. Supercond. Sci. Technol. 2010, 23, 125014. [Google Scholar] [CrossRef]
- Silhanek, A.V.; Van de Vondel, J.; Moshchalkov, V.V. Guided Vortex Motion and Vortex Ratchets in Nanostructured Superconductors. In Nanoscience and Engineering in Superconductivity; Springer: Berlin/Heidelberg, Germany, 2010; pp. 1–24. [Google Scholar]
- Shklovskij, V.A.; Sosedkin, V.V.; Dobrovolskiy, O.V. Vortex ratchet reversal in an asymmetric washboard pinning potential subject to combined dc and ac stimuli. J. Phys. Cond. Matt. 2014, 26, 025703. [Google Scholar] [CrossRef] [PubMed]
| Parameter | Unit | Value of Nb at |
|---|---|---|
| Time | 2.8 ps | |
| Length | 60 nm | |
| Magnetic field | 92 mT | |
| Current density | 60 GA | |
| Electric potential | 111 µV | |
| Conductivity | 31 (µ |
| Parameter | Denotation | Value for Nb |
|---|---|---|
| Electron mean free path | l | 6 nm |
| Fermi velocity | 600 km/s | |
| Diffusion coefficient | 12 cm2/s | |
| Normal conductivity [35,40] | 16 (µ | |
| Relative temperature | 0.952 | |
| Penetration depth | 278 nm | |
| Coherence length | 60 nm | |
| GL parameter | 4.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bogush, I.; Fomin, V.M.; Dobrovolskiy, O.V. Steering of Vortices by Magnetic Field Tilting in Open Superconductor Nanotubes. Nanomaterials 2024, 14, 420. https://doi.org/10.3390/nano14050420
Bogush I, Fomin VM, Dobrovolskiy OV. Steering of Vortices by Magnetic Field Tilting in Open Superconductor Nanotubes. Nanomaterials. 2024; 14(5):420. https://doi.org/10.3390/nano14050420
Chicago/Turabian StyleBogush, Igor, Vladimir M. Fomin, and Oleksandr V. Dobrovolskiy. 2024. "Steering of Vortices by Magnetic Field Tilting in Open Superconductor Nanotubes" Nanomaterials 14, no. 5: 420. https://doi.org/10.3390/nano14050420
APA StyleBogush, I., Fomin, V. M., & Dobrovolskiy, O. V. (2024). Steering of Vortices by Magnetic Field Tilting in Open Superconductor Nanotubes. Nanomaterials, 14(5), 420. https://doi.org/10.3390/nano14050420








