Magnetic Properties of Zig-Zag-Edged Hexagonal Nanohelicenes: A Quantum Chemical Study
Abstract
1. Introduction
2. Computational Details
2.1. Line Symmetry Groups
2.2. Modelling of the Metal-Insulator Transition
- q is even, , . Parameters q’ and r are coprime integers.
- q is odd, , . Parameters q and r’ are coprime integers.
2.3. Calculation Parameters
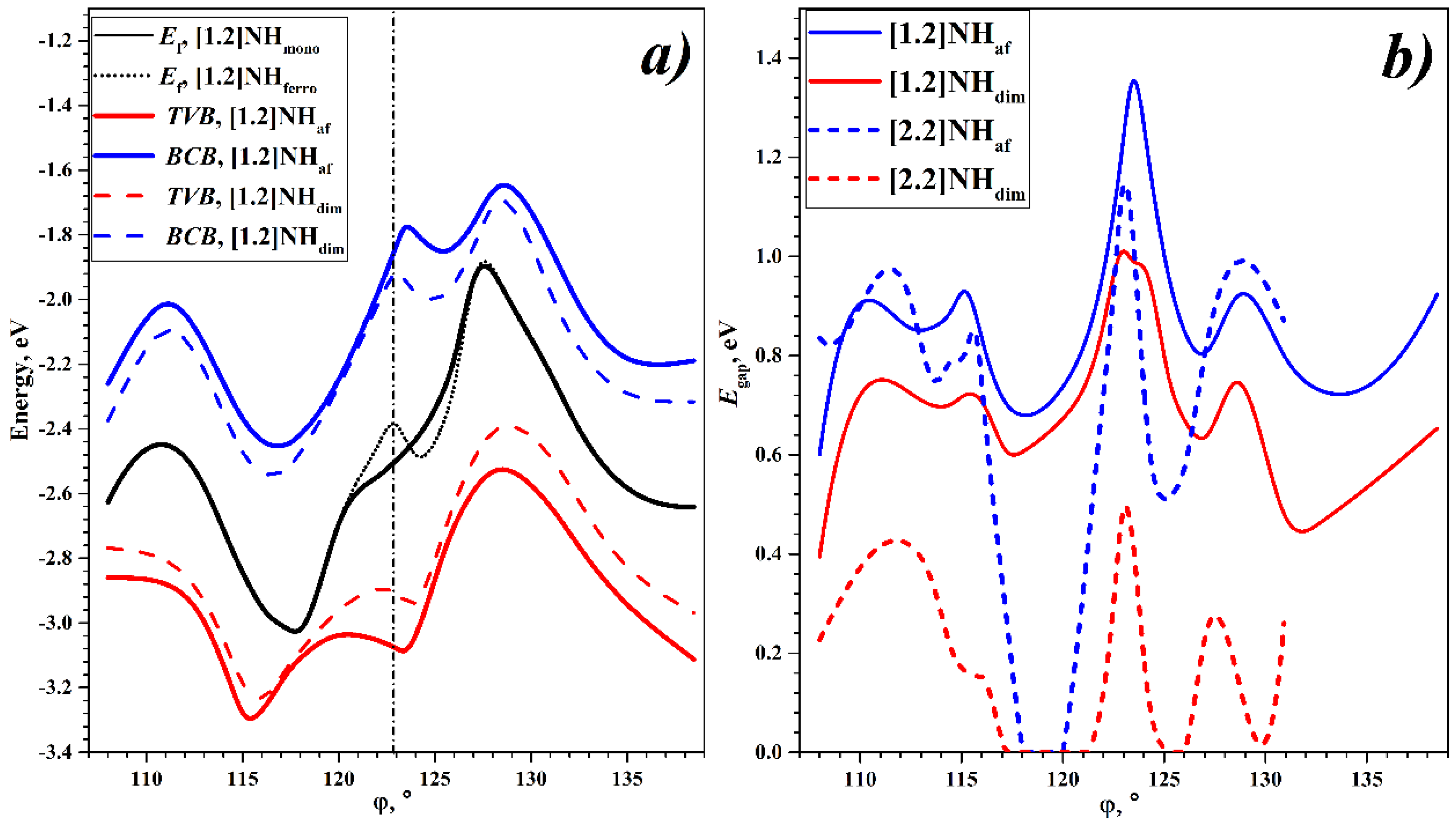
3. Results and Discussion
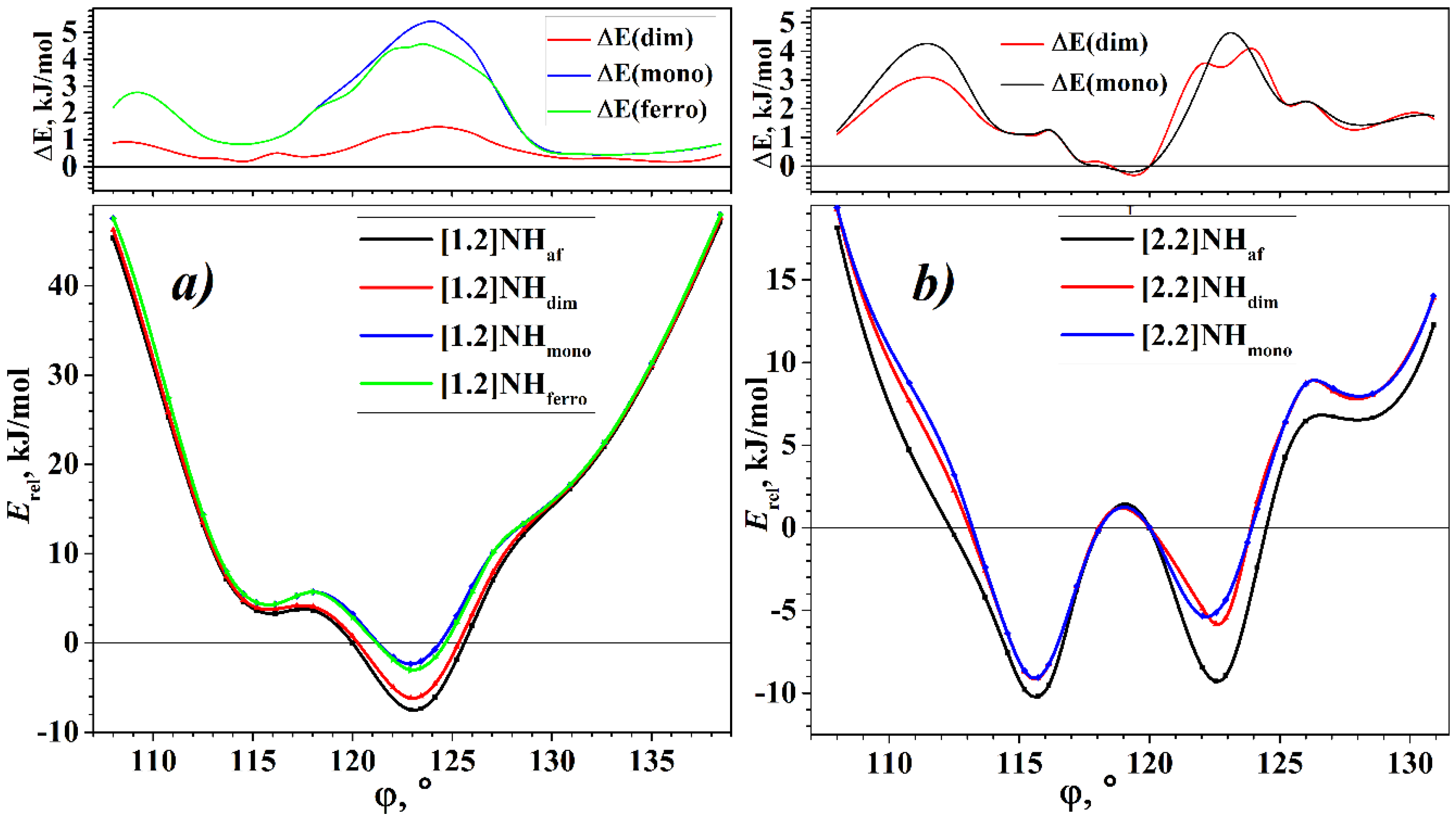
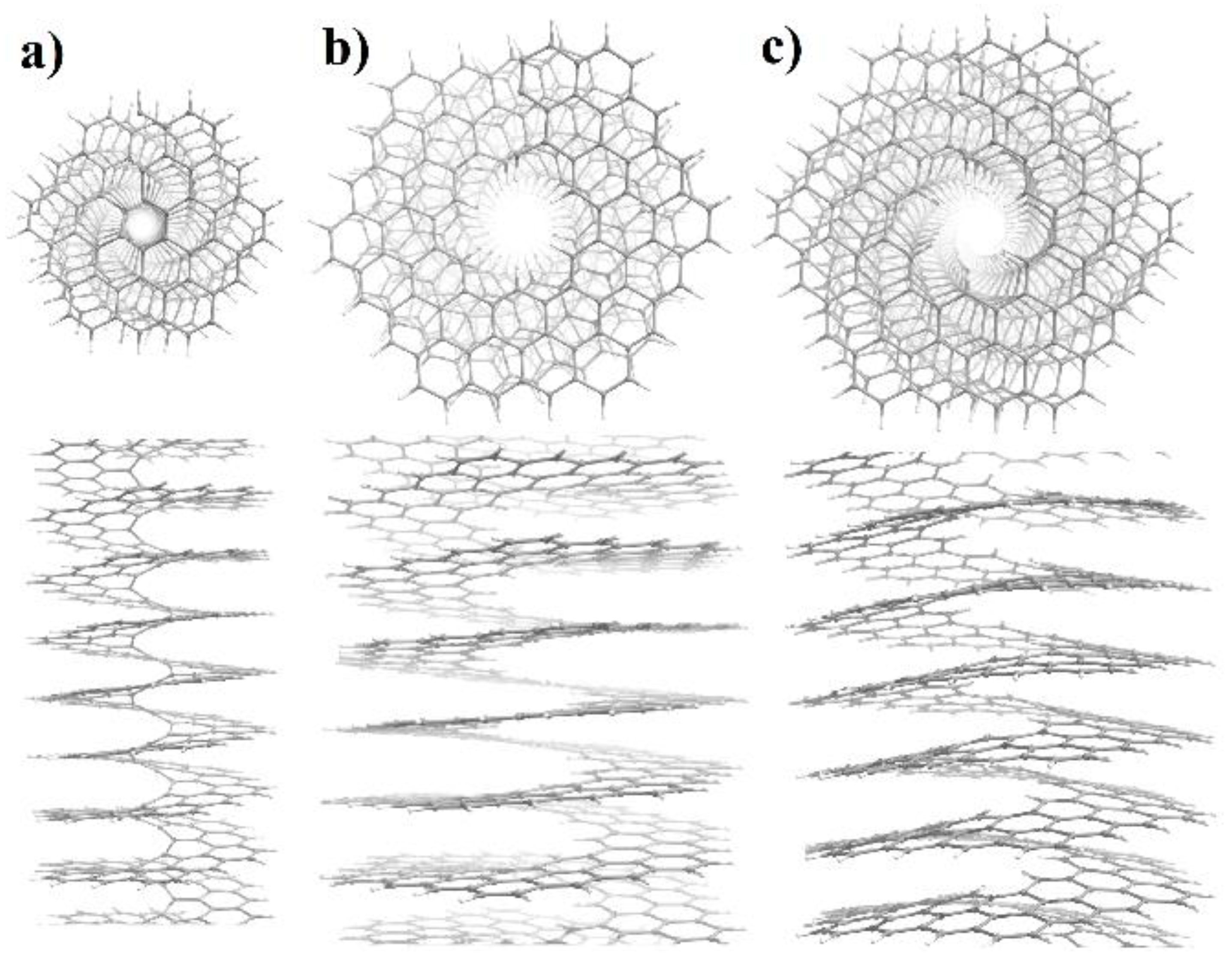
3.1. Energy Minima and Atomic Structure
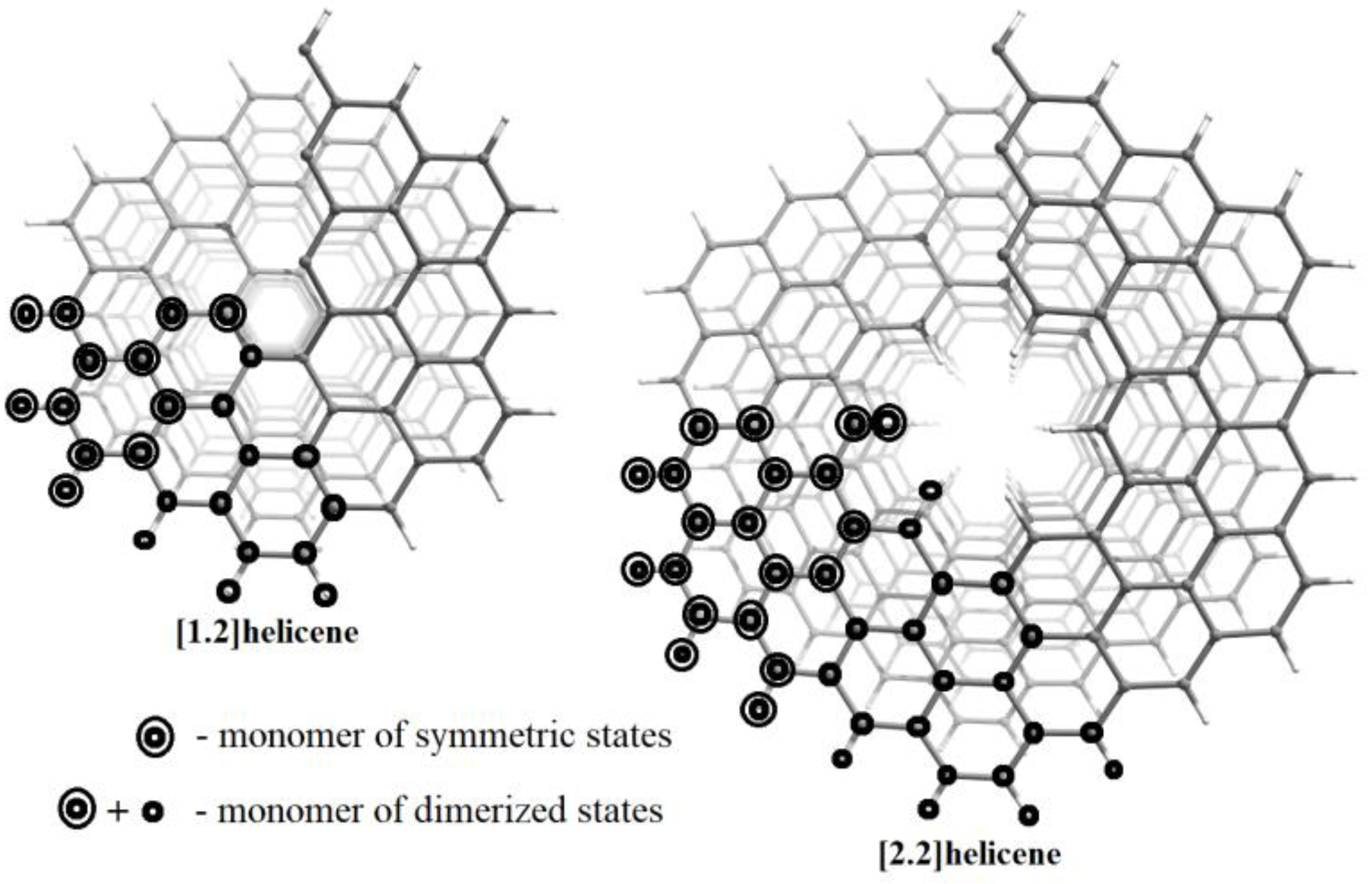
- [1.2]NHmono; and with monomer C9H3;
- [1.2]NHferro; and with monomer C9H3;
- [1.2]NHdim; and with monomer C18H6;
- [1.2]NHaf; and with monomer C18H6;
- [2.2]NHmono; and with monomer C15H5;
- [2.2]NHferro; and with monomer C15H5;
- [2.2]NHdim; and with monomer C30H10;
- [2.2]NHaf; and with monomer C30H10.
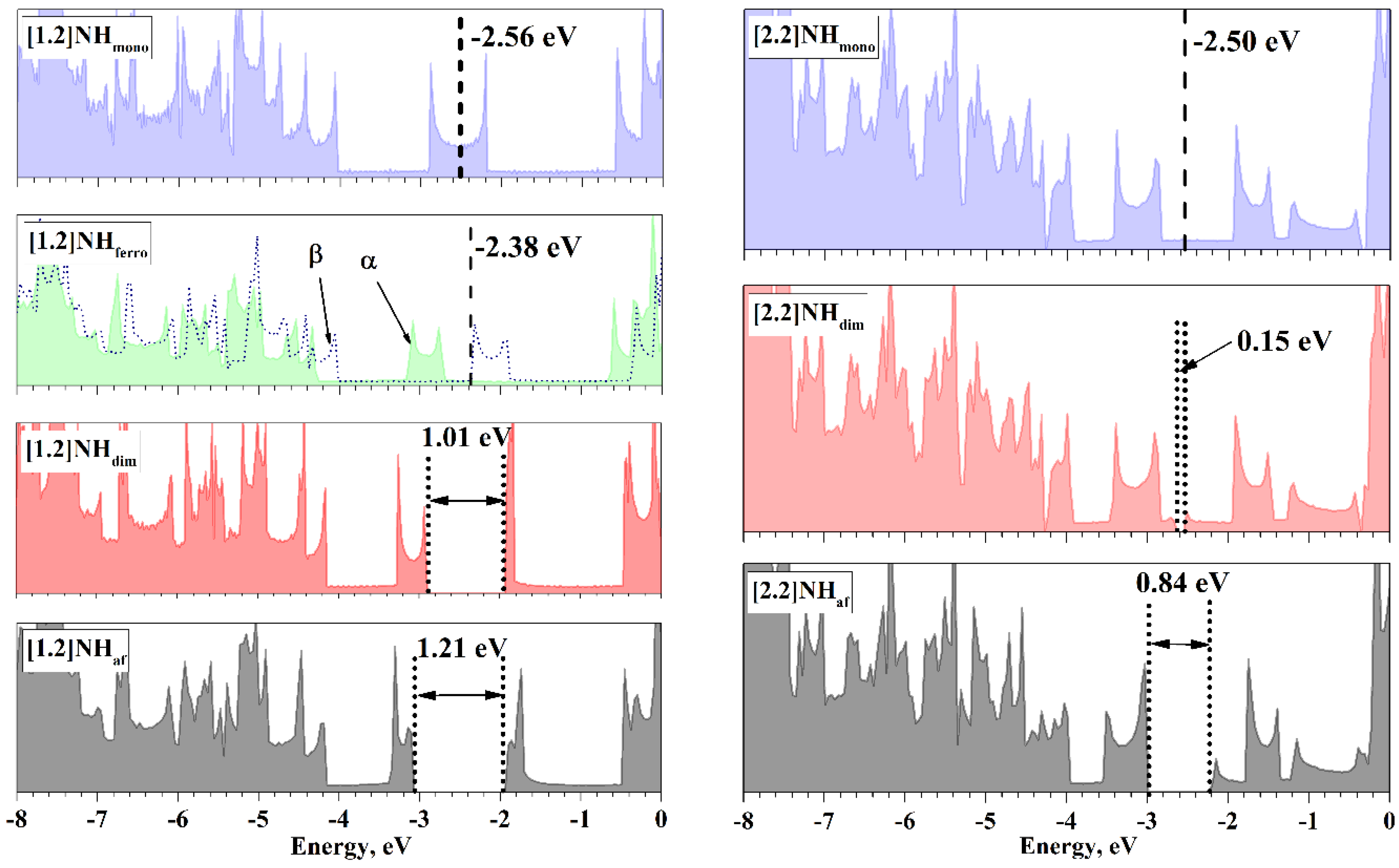
3.2. Electronic and Magnetic Properties
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, Y.; Xu, J.; Wang, Y.; Chen, H. Emerging chirality in nanoscience. Chem. Soc. Rev. 2013, 42, 2930–2962. [Google Scholar] [CrossRef] [PubMed]
- Meng, F.; Morin, S.A.; Forticaux, A.; Jin, S. Screw Dislocation Driven Growth of Nanomaterials. Acc. Chem. Res. 2013, 46, 1616–1626. [Google Scholar] [CrossRef] [PubMed]
- Yang, F.; Wang, M.; Zhang, D.; Yang, J.; Zheng, M.; Li, Y. Chirality Pure Carbon Nanotubes: Growth, Sorting, and Characterization. Chem. Rev. 2020, 120, 2693–2758. [Google Scholar] [CrossRef] [PubMed]
- Wang, P.; Yang, J.; Sun, G.; Zhang, X.; Zhang, H.; Zheng, Y.; Xu, S. Twist induced plasticity and failure mechanism of helical carbon nanotube fibers under different strain rates. Int. J. Plast. 2018, 110, 74–94. [Google Scholar] [CrossRef]
- Yashima, E.; Maeda, K.; Iida, H.; Furusho, Y.; Nagai, K. Helical Polymers: Synthesis, Structures, and Functions. Chem. Rev. 2009, 109, 6102–6211. [Google Scholar] [CrossRef]
- Percec, V.; Rudick, J.G.; Peterca, M.; Wagner, M.; Obata, M.; Mitchell, C.M.; Cho, W.-D.; Balagurusamy, V.S.K.; Heiney, P.A. Thermoreversible Cis-Cisoidal to Cis-Transoidal Isomerization of Helical Dendronized Polyphenylacetylenes. J. Am. Chem. Soc. 2005, 127, 15257–15264. [Google Scholar] [CrossRef]
- Zhang, S.; Liu, X.; Li, C.; Li, L.; Song, J.; Shi, J.; Morton, M.; Rajca, S.; Rajca, A.; Wang, H. Thiophene-Based Double Helices: Syntheses, X-ray Structures, and Chiroptical Properties. J. Am. Chem. Soc. 2016, 138, 10002–10010. [Google Scholar] [CrossRef]
- Varni, A.J.; Fortney, A.; Baker, M.A.; Worch, J.C.; Qiu, Y.; Yaron, D.; Bernhard, S.; Noonan, K.J.T.; Kowalewski, T. Photostable Helical Polyfurans. J. Am. Chem. Soc. 2019, 141, 8858–8867. [Google Scholar] [CrossRef]
- Ikai, T.; Yoshida, T.; Shinohara, K.; Taniguchi, T.; Wada, Y.; Swager, T.M. Triptycene-Based Ladder Polymers with One-Handed Helical Geometry. J. Am. Chem. Soc. 2019, 141, 4696–4703. [Google Scholar] [CrossRef]
- Rickhaus, M.; Mayor, M.; Juríček, M. Strain-induced helical chirality in polyaromatic systems. Chem. Soc. Rev. 2016, 45, 1542–1556. [Google Scholar] [CrossRef]
- Shen, Y.; Chen, C.-F. Helicenes: Synthesis and Applications. Chem. Rev. 2012, 112, 1463–1535. [Google Scholar] [CrossRef] [PubMed]
- Daigle, M.; Morin, J.-F. Helical conjugated ladder polymers: Tuning the conformation and properties through edge design. Macromolecules 2017, 50, 9257–9264. [Google Scholar] [CrossRef]
- Daigle, M.; Miao, D.; Lucotti, A.; Tommasini, M.; Morin, J.-F. Helically coiled graphene nanoribbons. Angew. Chem. Int. Ed. 2017, 56, 6213–6217. [Google Scholar] [CrossRef]
- Xiao, X.; Pedersen, S.K.; Aranda, D.; Yang, J.; Wiscons, R.A.; Pittelkow, M.; Steigerwald, M.L.; Santoro, F.; Schuster, N.J.; Nuckolls, C. Chirality Amplified: Long, Discrete Helicene Nanoribbons. J. Am. Chem. Soc. 2021, 143, 983–991. [Google Scholar] [CrossRef] [PubMed]
- Kiel, G.R.; Patel, S.C.; Smith, P.W.; Levine, D.S.; Tilley, T.D. Expanded helicenes: A general synthetic strategy and remarkable supramolecular and solid-state behavior. J. Am. Chem. Soc. 2017, 139, 18456–18459. [Google Scholar] [CrossRef] [PubMed]
- Zhan, H.; Zhang, Y.; Yang, C.; Zhang, G.; Gu, Y. Graphene helicoid as novel nanospring. Carbon 2017, 120, 258–264. [Google Scholar] [CrossRef]
- Zhan, H.; Zhang, G.; Yang, C.; Gu, Y. Breakdown of Hooke’s law at the nanoscale—2D material-based nanosprings. Nanoscale 2018, 10, 18961–18968. [Google Scholar] [CrossRef]
- Zhan, H.; Zhang, G.; Yang, C.; Gu, Y. Graphene Helicoid: Distinct Properties Promote Application of Graphene Related Materials in Thermal Management. J. Phys. Chem. C 2018, 122, 7605–7612. [Google Scholar] [CrossRef]
- Sharifian, A.; Naeini, V.F.; Baniassadi, M.; Wu, J.; Baghani, M. Role of Chemical Doping in Large Deformation Behavior of Spiral Carbon-Based Nanostructures: Unraveling Geometry-Dependent Chemical Doping Effects. J. Phys. Chem. C 2019, 123, 19208–19219. [Google Scholar] [CrossRef]
- Norouzi, S.; Fakhrabadi, M.M.S. Nanomechanical properties of single- and double-layer graphene spirals: A molecular dynamics simulation. Appl. Phys. A 2019, 125, 321. [Google Scholar] [CrossRef]
- Sharifian, A.; Moshfegh, A.; Javadzadegan, A.; Afrouzi, H.H.; Baghani, M.; Baniassadi, M. Hydrogenation-controlled mechanical properties in graphene helicoids: Exceptional distribution-dependent behavior. Phys. Chem. Chem. Phys. 2019, 21, 12423–12433. [Google Scholar] [CrossRef] [PubMed]
- Liu, R.; Zhao, J.; Wang, L.; Wei, N. Nonlinear vibrations of helical graphene resonators in the dynamic nano-indentation testing. Nanotechnology 2020, 31, 025709. [Google Scholar] [CrossRef]
- Norouzi, S.; Kianfar, A.; Fakhrabadi, M.M.S. Multiscale simulation study of anisotropic nanomechanical properties of graphene spirals and their polymer nanocomposites. Mech. Mater. 2020, 145, 103376. [Google Scholar] [CrossRef]
- Norouzi, S.; Fakhrabadi, M.M.S. Anisotropic nature of thermal conductivity in graphene spirals revealed by molecular dynamics simulations. J. Phys. Chem. Solids 2020, 137, 109228. [Google Scholar] [CrossRef]
- Zhu, C.; Ji, J.; Zhang, Z.; Dong, S.; Wei, N.; Zhao, J. Huge stretchability and reversibility of helical graphenes using molecular dynamics simulations and simplified theoretical models. Mech. Mater. 2021, 153, 103683. [Google Scholar] [CrossRef]
- Zhu, C.; Liu, M.; Wei, N.; Zhao, J. Molecular dynamics study on mechanical properties of helical graphenes/epoxy nanocomposites. Comput. Mater. Sci. 2022, 209, 111408. [Google Scholar] [CrossRef]
- Narjabadifam, A.; Abazadeh, B.; Fakhrabadi, M.M.S. Graphyne nano-spirals under tension: Effects of base structures on superelasticity and fracture mechanisms. Mech. Mater. 2022, 171, 104367. [Google Scholar] [CrossRef]
- Li, H.; Afrouzi, H.H.; Zahra, M.M.A.; Bashar, B.S.; Fathdal, F.; Hadrawi, S.K.; Alizadeh, A.; Hekmatifar, M.; Al-Majdi, K.; Alhani, I. A comprehensive investigation of thermal conductivity in of monolayer graphene, helical graphene with different percentages of hydrogen atom: A molecular dynamics approach. Colloids Surf. A 2023, 656, 130324. [Google Scholar] [CrossRef]
- Korhonen, T.; Koskinen, P. Electromechanics of graphene spirals. AIP Adv. 2014, 4, 127125. [Google Scholar] [CrossRef]
- Xu, F.; Yu, H.; Sadrzadeh, A.; Yakobson, B.I. Riemann surfaces of carbon as graphene nanosolenoids. Nano Lett. 2016, 16, 34–39. [Google Scholar] [CrossRef]
- Zhou, Z.; Yan, L.; Wang, X.-M.; Zhang, D.; Yan, J.-Y. The sensitive energy band structure and the spiral current in helical graphenes. Results Phys. 2022, 35, 105351. [Google Scholar] [CrossRef]
- Treboux, G.; Lapstun, P.; Wu, Z.; Silverbrook, K. Electronic conductance of helicenes. Chem. Phys. Lett. 1999, 301, 493–497. [Google Scholar] [CrossRef]
- Tian, Y.-H.; Park, G.; Kertesz, M. Electronic Structure of Helicenes, C2S Helicenes, and Thiaheterohelicenes. Chem. Mater. 2008, 20, 3266–3277. [Google Scholar] [CrossRef]
- Avdoshenko, S.M.; Koskinen, P.; Sevinçli, H.; Popov, A.A.; Rocha, C.G. Topological signatures in the electronic structure of graphene spirals. Sci. Rep. 2013, 3, 1632. [Google Scholar] [CrossRef]
- Zhang, X.M.; Zhao, M.W. Strain-induced phase transition and electron spin-polarization in graphene spirals. Sci. Rep. 2014, 4, 5699. [Google Scholar] [CrossRef]
- Šesták, P.; Wu, J.; He, J.; Pokluda, J.; Zhang, Z. Extraordinary deformation capacity of smallest carbohelicene springs. Phys. Chem. Chem. Phys. 2015, 17, 18684–18690. [Google Scholar] [CrossRef]
- Xu, X.; Liu, B.; Zhao, W.; Jiang, Y.; Liu, L.; Li, W.; Zhang, G.; Tian, W.Q. Mechanism of mechanically induced optoelectronic and spintronic phase transitions in 1D graphene spirals: Insight into the role of interlayer coupling. Nanoscale 2017, 9, 9693–9700. [Google Scholar] [CrossRef]
- Porsev, V.V.; Bandura, A.V.; Lukyanov, S.I.; Evarestov, R.A. Expanded hexagonal nanohelicenes of zigzag morphology under elastic strain: A quantum chemical study. Carbon 2019, 152, 755–765. [Google Scholar] [CrossRef]
- He, Y.-Y.; Chen, J.; Zheng, X.-L.; Xu, X.; Li, W.-Q.; Yang, L.; Tian, W.Q. Spiral Graphene Nanoribbons with Azulene Defects as Potential Nonlinear Optical Materials. ACS Appl. Nano Mater. 2019, 2, 1648–1654. [Google Scholar] [CrossRef]
- Liu, Z.-P.; Guo, Y.-D.; Yan, X.-H.; Zeng, H.-L.; Mou, X.-Y.; Wang, Z.-R.; Wang, J.-J. A metal-semiconductor transition in helical graphene nanoribbon. J. Appl. Phys. 2019, 126, 144303. [Google Scholar] [CrossRef]
- Liu, Z.-P.; Guo, Y.-D.; Zeng, H.-L.; Li, J.-F.; Jiang, Y.-Y.; Yan, X.-H. Electrical control of spin polarization of transmission in pure-carbon systems of helical graphene nanoribbons. J. Appl. Phys. 2020, 128, 154301. [Google Scholar] [CrossRef]
- Thakur, R.; Ahluwalia, P.K.; Kumar, A.; Sharma, R. Stability and electronic properties of bilayer graphene spirals. Phys. E 2021, 129, 114638. [Google Scholar] [CrossRef]
- Mori, K.; Murase, T.; Fujita, M. One-Step Synthesis of [16]Helicene. Angew. Chem. Int. Ed. 2015, 54, 6847–6851. [Google Scholar] [CrossRef] [PubMed]
- Porsev, V.V.; Bandura, A.V.; Evarestov, R.A. Ab initio modeling of helically periodic nanostructures using CRYSTAL17: A general algorithm first applied to nanohelicenes. Comput. Mater. Sci. 2022, 203, 111063. [Google Scholar] [CrossRef]
- Porsev, V.V.; Evarestov, R.A. Quantum-mechanical calculation of the electronic band structure of helically periodic systems: Nanotubes and nanohelicenes. Phys. Solid State 2022, 64, 1843. (In Russian) [Google Scholar]
- Alexander, E.Z. XVI. Systematik der eindimensionalen Baumgruppen. Z. Krist.-Cryst. Mater. 1929, 70, 367–382. [Google Scholar] [CrossRef]
- Shubnikov, A.V. Symmetry (The Laws of Symmetry and Their Application in Science, Technology, and Applied Art); Akademia Nauk SSSR: Moscow, Russia, 1940. (In Russian) [Google Scholar]
- Vujičić, M.; Božović, I.B.; Herbut, F. Construction of symmetry groups of polymer molecules. J. Phys. A 1977, 10, 1271–1279. [Google Scholar] [CrossRef]
- Milošević, I.; Živanovic, R.; Damnjanović, M. Symmetry classification of stereoregular polymers. Polymer 1997, 38, 4445–4453. [Google Scholar] [CrossRef]
- Damnjanović, M.; Milošević, I. Line Groups in Physics. Theory and Applications to Nanotubes and Polymers; Lecture Notes in Physics; Springer: Berlin/Heidelberg, Germany, 2010; Volume 801. [Google Scholar]
- Damnjanović, M.; Milošević, I. Full symmetry implementation in condensed matter and molecular physics—Modified group projector technique. Phys. Rep. 2015, 581, 1–43. [Google Scholar] [CrossRef]
- Domnin, A.V.; Porsev, V.V.; Evarestov, R.A. DFT modeling of electronic and mechanical properties of polytwistane using line symmetry group theory. Comput. Mater. Sci. 2022, 214, 111704. [Google Scholar] [CrossRef]
- Porsev, V.V.; Evarestov, R.A. Ab initio modeling of helical polyacetylenes: Peierls and Mott-Hubbard metal–insulator transitions. Comput. Mater. Sci. 2022, 213, 111642. [Google Scholar] [CrossRef]
- Lazić, N.; Vuković, N.; Volonakis, G.; Milošević, I.; Logothetes; Damnjanović, M. Natural torsion in chiral single-wall carbon nanotubes. J. Phys. Condens. Matter 2012, 24, 485302. [Google Scholar] [CrossRef] [PubMed]
- Peierls, R.E. Quantum Theory of Solids; Oxford University Press: New York, NY, USA, 1955. [Google Scholar]
- Mott, N.F. The Basis of the Electron Theory of Metals, with Special Reference to the Transition Metals. Proc. Phys. Soc. A 1949, 62, 416–422. [Google Scholar] [CrossRef]
- Damnjanović, M.; Vujičić, M. Magnetic line groups. Phys. Rev. B 1982, 25, 6987–6994. [Google Scholar] [CrossRef]
- Dovesi, R.; Erba, A.; Orlando, R.; Zicovich-Wilson, C.M.; Civalleri, B.; Maschio, L.; Rérat, M.; Casassa, S.; Baima, J.; Salustro, S.; et al. Quantum-mechanical condensed matter simulations with CRYSTAL. WIREs, Comput. Mol. Sci. 2018, 8, e1360. [Google Scholar] [CrossRef]
- Dovesi, R.; Pascale, F.; Civalleri, B.; Doll, K.; Harrison, N.M.; Bush, I.; D’Arco, P.; Noël, Y.; Rérat, M.; Carbonnière, P.; et al. The CRYSTAL code, 1976–2020 and beyond, a long story. J. Chem. Phys. 2020, 152, 204111. [Google Scholar] [CrossRef] [PubMed]
- Ferrari, A.M.; Civalleri, B.; Dovesi, R. Ab initio periodic study of the conformational behavior of glycine helical homopeptides. J. Comput. Chem. 2010, 31, 1777–1784. [Google Scholar] [PubMed]
- Perdew, J.P.; Ernzerhof, M.; Burke, K. Rationale for mixing exact exchange with density functional approximations. J. Chem. Phys. 1996, 105, 9982–9985. [Google Scholar] [CrossRef]
- Peintinger, M.F.; Oliveira, D.V.; Bredow, T. Consistent Gaussian basis sets of triple-zeta valence with polarization quality for solid-state calculations. J. Comput. Chem. 2013, 34, 451–459. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
| Monomeric States | Dimeric States | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| φ, ° | Q | q | r | p | φ’, ° | Q’ | q’ | r’ | p’ |
| 54.000 | 6.667 | 20 | 3 | 7 | 108.000 | 3.333 | 10 | 3 | 7 |
| 55.385 | 6.500 | 13 | 2 | 7 | 110.769 | 3.250 | 13 | 4 | 10 |
| 56.250 | 6.400 | 32 | 5 | 13 | 112.500 | 3.200 | 16 | 5 | 13 |
| 56.842 | 6.333 | 19 | 3 | 13 | 113.684 | 3.167 | 19 | 6 | 16 |
| 57.273 | 6.286 | 44 | 7 | 19 | 114.545 | 3.143 | 22 | 7 | 19 |
| 57.600 | 6.250 | 25 | 4 | 19 | 115.200 | 3.125 | 25 | 8 | 22 |
| 58.065 | 6.200 | 31 | 5 | 25 | 116.129 | 3.100 | 31 | 10 | 28 |
| 58.605 | 6.143 | 43 | 7 | 37 | 117.209 | 3.071 | 43 | 14 | 40 |
| 59.016 | 6.100 | 61 | 10 | 55 | 118.033 | 3.050 | 61 | 20 | 58 |
| 60.000 | 6.000 | 6 | 1 | 1 | 120.000 | 3.000 | 3 | 1 | 1 |
| 61.017 | 5.900 | 59 | 10 | 6 | 122.034 | 2.950 | 59 | 20 | 3 |
| 61.463 | 5.857 | 41 | 7 | 6 | 122.927 | 2.929 | 41 | 14 | 3 |
| 61.714 | 5.833 | 35 | 6 | 6 | 123.429 | 2.917 | 35 | 12 | 3 |
| 62.069 | 5.800 | 29 | 5 | 6 | 124.138 | 2.900 | 29 | 10 | 3 |
| 62.609 | 5.750 | 23 | 4 | 6 | 125.217 | 2.875 | 23 | 8 | 3 |
| 63.000 | 5.714 | 40 | 7 | 23 | 126.000 | 2.857 | 20 | 7 | 3 |
| 63.529 | 5.667 | 17 | 3 | 6 | 127.059 | 2.833 | 17 | 6 | 3 |
| 64.286 | 5.600 | 28 | 5 | 17 | 128.571 | 2.800 | 14 | 5 | 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Porsev, V.; Evarestov, R. Magnetic Properties of Zig-Zag-Edged Hexagonal Nanohelicenes: A Quantum Chemical Study. Nanomaterials 2023, 13, 415. https://doi.org/10.3390/nano13030415
Porsev V, Evarestov R. Magnetic Properties of Zig-Zag-Edged Hexagonal Nanohelicenes: A Quantum Chemical Study. Nanomaterials. 2023; 13(3):415. https://doi.org/10.3390/nano13030415
Chicago/Turabian StylePorsev, Vitaly, and Robert Evarestov. 2023. "Magnetic Properties of Zig-Zag-Edged Hexagonal Nanohelicenes: A Quantum Chemical Study" Nanomaterials 13, no. 3: 415. https://doi.org/10.3390/nano13030415
APA StylePorsev, V., & Evarestov, R. (2023). Magnetic Properties of Zig-Zag-Edged Hexagonal Nanohelicenes: A Quantum Chemical Study. Nanomaterials, 13(3), 415. https://doi.org/10.3390/nano13030415






