Optical Properties of Polyisocyanurate–Polyurethane Aerogels: Study of the Scattering Mechanisms
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Aerogels Production
2.3. Characterization Techniques
2.3.1. Density and Porosity
2.3.2. Scanning Electron Microscopy
2.3.3. Specific Surface Area
2.3.4. Particle and Pore Size
2.3.5. Transmittance Measurements
2.3.6. UV–Vis
3. Results and Discussion
3.1. Aerogels Structure
3.2. Optical Transmittance
Effect of Catalyst and Thickness
3.3. The Beer–Lambert Law
3.4. Effect of the Aerogel Structure on Transmittance
3.4.1. Refraction Index
3.4.2. Scattering Produced by Particles
3.5. Effect of the Light Wavelength
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Maleki, H.; Durães, L.; Portugal, A. An overview on silica aerogels synthesis and different mechanical reinforcing strategies. J. Non. Cryst. Solids 2014, 385, 55–74. [Google Scholar] [CrossRef] [Green Version]
- Reim, M.; Beck, A.; Körner, W.; Petricevic, R.; Glora, M.; Weth, M.; Schliermann, T.; Fricke, J.; Schmidt, C.; Pötter, F.J. Highly insulating aerogel glazing for solar energy usage. Sol. Energy 2002, 72, 21–29. [Google Scholar] [CrossRef]
- Buratti, C.; Belloni, E.; Merli, F.; Zinzi, M. Aerogel glazing systems for building applications: A review. Energy Build 2021, 231, 110587. [Google Scholar] [CrossRef]
- Duer, K.; Svendsen, S. Monolithic silica aerogel in superinsulating glazings. Sol. Energy 1998, 63, 259–267. [Google Scholar] [CrossRef]
- Svendsen, S. Solar collector with monolithic silica aerogel. J. Non-Cryst. Solids 1992, 145, 240–243. [Google Scholar] [CrossRef]
- Wang, J.; Petit, D.; Ren, S. Transparent thermal insulation silica aerogels. Nanoscale Adv. 2020, 2, 5504–5515. [Google Scholar] [CrossRef]
- Venkateswara Rao, A.; Haranath, D.; Pajonk, G.M.; Wagh, P.B. Optimisation of supercritical drying parameters for transparent silica aerogel window applications. Mater. Sci. Technol. 1998, 14, 1194–1199. [Google Scholar] [CrossRef]
- Tabata, M.; Adachi, I.; Ishii, Y.; Kawai, H.; Sumiyoshi, T.; Yokogawa, H. Development of transparent silica aerogel over a wide range of densities. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2010, 623, 339–341. [Google Scholar] [CrossRef] [Green Version]
- Bernardes, J.C.; Müller, D.; Pinheiro, K.; Rambo, C.R. Enhancing the optical transparency of TiO 2 aerogels with high surface area through water-based synthesis. Opt. Mater. 2020, 109, 110359. [Google Scholar] [CrossRef]
- Wang, H.; Du, A.; Ji, X.; Zhang, C.; Zhou, B.; Zhang, Z.; Shen, J. Enhanced Photothermal Conversion by Hot-Electron Effect in Ultrablack Carbon Aerogel for Solar Steam Generation. ACS Appl. Mater. Interfaces 2019, 11, 42057–42065. [Google Scholar] [CrossRef]
- Du, R.; Hu, Y.; Hübner, R.; Joswig, J.O.; Fan, X.; Schneider, K.; Eychmüller, A. Specific ion effects directed noble metal aerogels: Versatile manipulation for electrocatalysis and beyond. Sci. Adv. 2019, 5, 1–10. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1988; ISBN 9783527406647. [Google Scholar]
- Venkateswara Rao, A.; Pajonk, G.M.; Parvathy, N.N. Effect of solvents and catalysts on monolithicity and physical properties of silica aerogels. J. Mater. Sci. 1994, 29, 1807–1817. [Google Scholar] [CrossRef]
- Wolf, A.; Terheiden, B.; Brendel, R. Light scattering and diffuse light propagation in sintered porous silicon. J. Appl. Phys. 2008, 104, 033106. [Google Scholar] [CrossRef]
- Mandal, C.; Donthula, S.; Soni, R.; Bertino, M.; Leventis, N. Light scattering and haze in TMOS-co-APTES silica aerogels. J. Sol-Gel Sci. Technol. 2019, 90, 127–139. [Google Scholar] [CrossRef]
- Zhao, L.; Yang, S.; Bhatia, B.; Strobach, E.; Wang, E.N. Modeling silica aerogel optical performance by determining its radiative properties. AIP Adv. 2016, 6, 025123. [Google Scholar] [CrossRef] [Green Version]
- Emmerling, A.; Petricevic, R.; Beck, A.; Wang, P.; Scheller, H.; Fricke, J. Relationship between optical transparency and nanostructural features of silica aerogels. J. Non-Cryst. Solids 1995, 185, 240–248. [Google Scholar] [CrossRef]
- Twej, W.A.A.; Alattar, A.M.; Drexler, M.; Alamgir, F.M. Tuned optical transmittance in single-step-derived silica aerogels through pH-controlled microstructure. Int. Nano Lett. 2017, 7, 257–265. [Google Scholar] [CrossRef] [Green Version]
- Tan, C.; Fung, B.M.; Newman, J.K.; Vu, C. Organic aerogels with very high impact strength. Adv. Mater. 2001, 13, 644–646. [Google Scholar] [CrossRef]
- Pekala, R.W. Synthetic control of molecular structure in organic aerogels. MRS Online Proc. Libr. (OPL) 1989, 171, 285–291. [Google Scholar] [CrossRef]
- Nakanishi, Y.; Hara, Y.; Sakuma, W.; Saito, T.; Nakanishi, K.; Kanamori, K. Colorless Transparent Melamine-Formaldehyde Aerogels for Thermal Insulation. ACS Appl. Nano Mater. 2020, 3, 49–54. [Google Scholar] [CrossRef] [Green Version]
- Takeshita, S.; Yoda, S. Chitosan Aerogels: Transparent, Flexible Thermal Insulators. Chem. Mater. 2015, 27, 7569–7572. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Saito, T.; Isogai, A. Aerogels with 3D ordered nanofiber skeletons of liquid-crystalline nanocellulose derivatives as tough and transparent insulators. Angew. Chem. Int. Ed. Engl. 2014, 53, 10394–10397. [Google Scholar] [CrossRef] [PubMed]
- Vivod, S.L.; Meador, M.A.B.; Pugh, C.; Wilkosz, M.; Calomino, K.; McCorkle, L. Toward Improved Optical Transparency of Polyimide Aerogels. ACS Appl. Mater. Interfaces 2020, 12, 8622–8633. [Google Scholar] [CrossRef]
- Merillas, B.; Martín-de León, J.; Villafañe, F.; Rodríguez-Pérez, M.A. Transparent Polyisocyanurate-Polyurethane-Based Aerogels: Key Aspects on the Synthesis and Their Porous Structures. ACS Appl. Polym. Mater. 2021, 3, 4607–4615. [Google Scholar] [CrossRef]
- ASTM D1622-08; Standard Test Method for Apparent Density of Rigid Cellular Plastics. ASTM International: West Conshohocken, PA, USA, 2008.
- Juhász, L.; Moldován, K.; Gurikov, P.; Liebner, F.; Fábián, I.; Kalmár, J.; Cserháti, C. False morphology of aerogels caused by gold coating for sem imaging. Polymers 2021, 13, 588. [Google Scholar] [CrossRef] [PubMed]
- Barrett, E.P.; Joyner, L.G.; Halenda, P.P. The Determination of Pore Volume and Area Distributions in Porous Substances. I. Computations from Nitrogen Isotherms. J. Am. Chem. Soc. 1951, 73, 373–380. [Google Scholar] [CrossRef]
- Pinto, J.; Solórzano, E.; Rodriguez-Perez, M.A.; De Saja, J.A. Characterization of the cellular structure based on user-interactive image analysis procedures. J. Cell. Plast. 2013, 49, 555–575. [Google Scholar] [CrossRef]
- Bostain, D.A.; Brenizer, J.S.; Norris, P.M. Neutron radioscopic measurement of water adsorption coefficients in aerogels. Res. Nondestruct. Eval. 2002, 14, 47–57. [Google Scholar] [CrossRef]
- Thiagarajan, C.; Sinha, V.; Sriraman, R.; Pattanayak, A.; Kumar, M. Nano Mechanics of Engineered Foam for Super Structural, Thermal and Optical Properties; Report number 2006GRC536; GE Global Research: Niskayuna, NY, USA, 2006. [Google Scholar]
- Poelz, G.; Riethmüller, R. Preparation of silica aerogel for Cherenkov counters. Nucl. Instrum. Methods 1982, 195, 491–503. [Google Scholar] [CrossRef]
- Jackel, D.; Walter, B. Modeling and Rendering of the Atmosphere Using Mie-Scattering. Comput. Graph. Forum 1997, 16, 201–210. [Google Scholar] [CrossRef]
- Lockwood, D.J. (Ed.) Rayleigh and Mie Scattering; Springer: New York, NY, USA, 2016; ISBN 9781441980717. [Google Scholar]
- Martín-de León, J.; Pura, J.L.; Bernardo, V.; Rodríguez-Pérez, M.Á. Transparent nanocellular PMMA: Characterization and modeling of the optical properties. Polymer 2019, 170, 16–23. [Google Scholar] [CrossRef]
- Pisal, A.A.; Venkateswara Rao, A. Development of hydrophobic and optically transparent monolithic silica aerogels for window panel applications. J. Porous Mater. 2017, 24, 685–695. [Google Scholar] [CrossRef]
- Riffat, S.B.; Qiu, G. A review of state-of-the-art aerogel applications in buildings. Int. J. Low-Carbon Technol. 2013, 8, 1–6. [Google Scholar] [CrossRef]
- Soleimani Dorcheh, A.; Abbasi, M.H. Silica aerogel; synthesis, properties and characterization. J. Mater. Process. Technol. 2008, 199, 10–26. [Google Scholar] [CrossRef]
- Cao, W.; Hunt, A.J. Improving the visible transparency of silica aerogels. J. Non-Cryst. Solids 1994, 176, 18–25. [Google Scholar]
- Acharya, R. Interaction of waves with medium. In Satellite Signal Propagation, Impairments and Mitigation; Academic Press: Cambridge, MA, USA, 2017; pp. 57–86. ISBN 9780128097328. [Google Scholar]
- Pendleton, W.K.; Wriedt, T. (Eds.) The Mie Theory-Basics and Applications; Springer Series in Optical Sciences; Springer: Berlin/Heidelberg, Germany, 2012; Volume 169, ISBN 9783642287374. [Google Scholar]
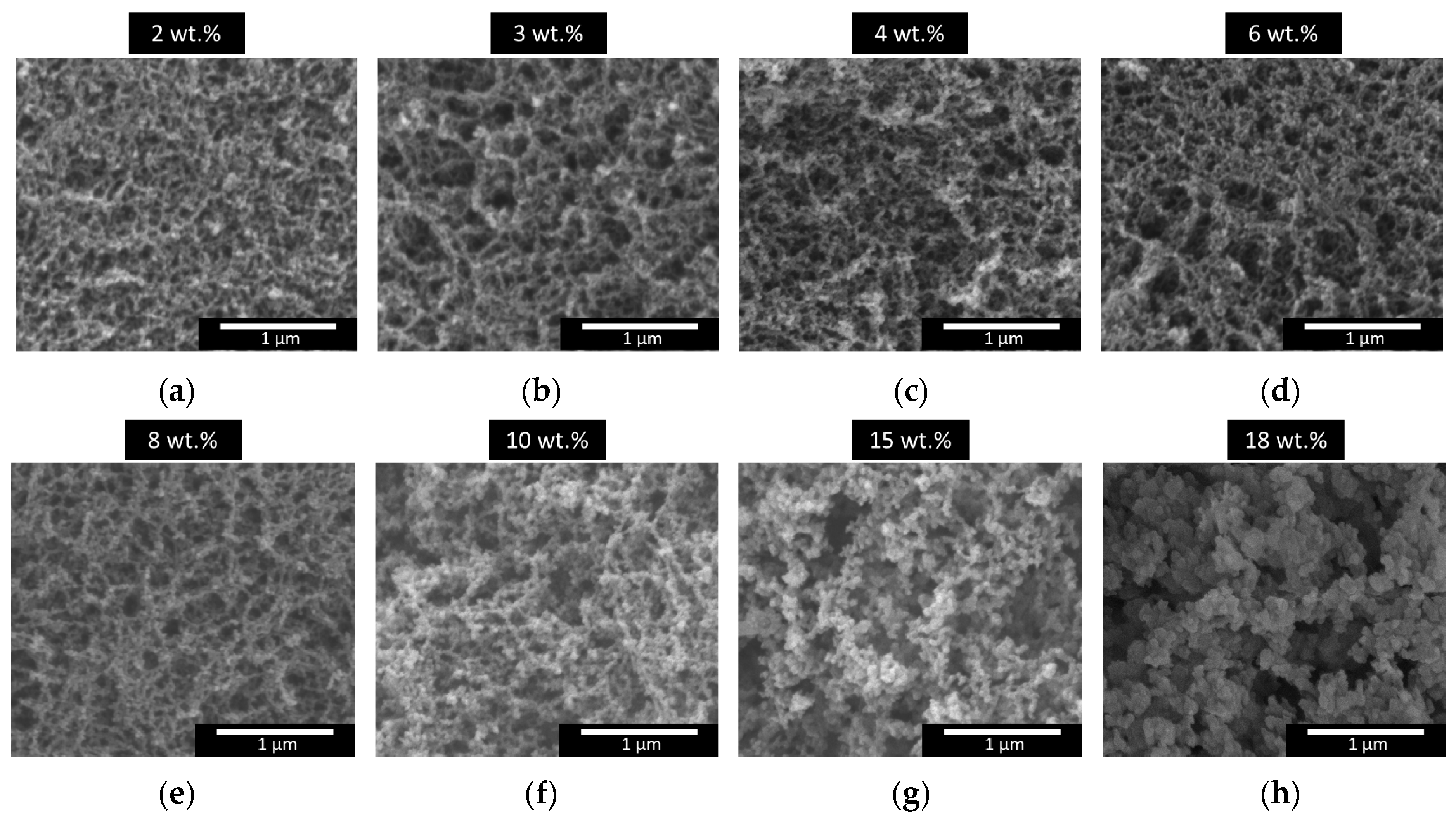

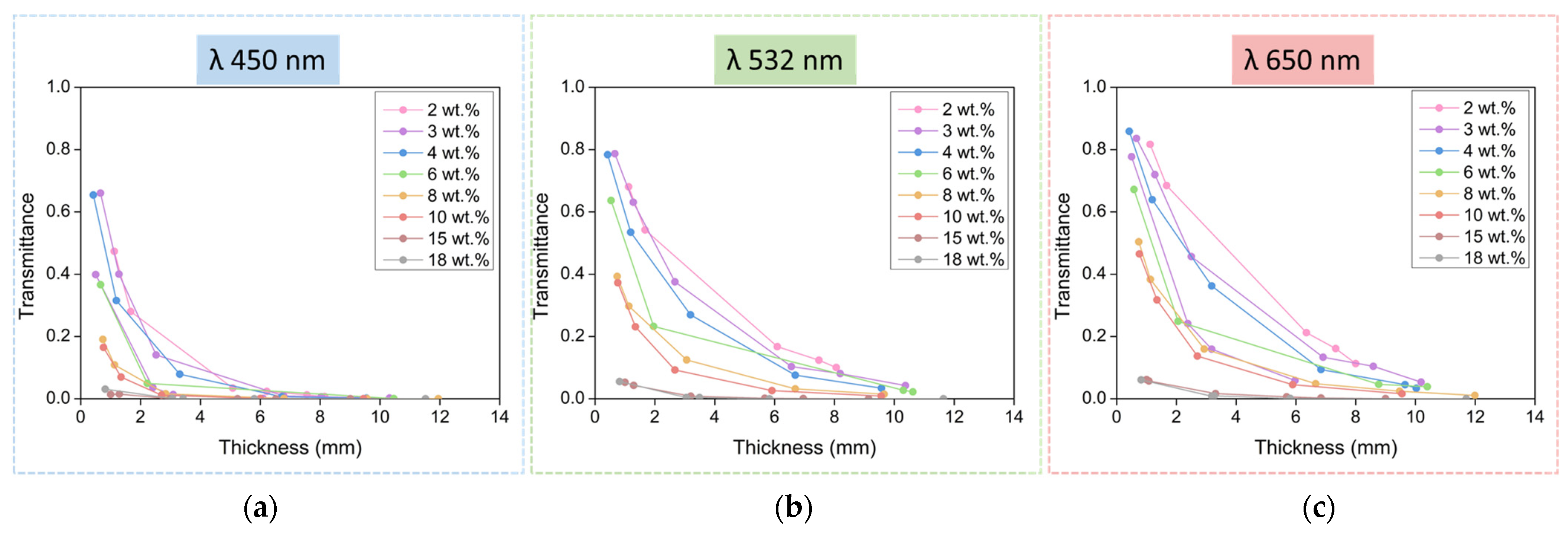


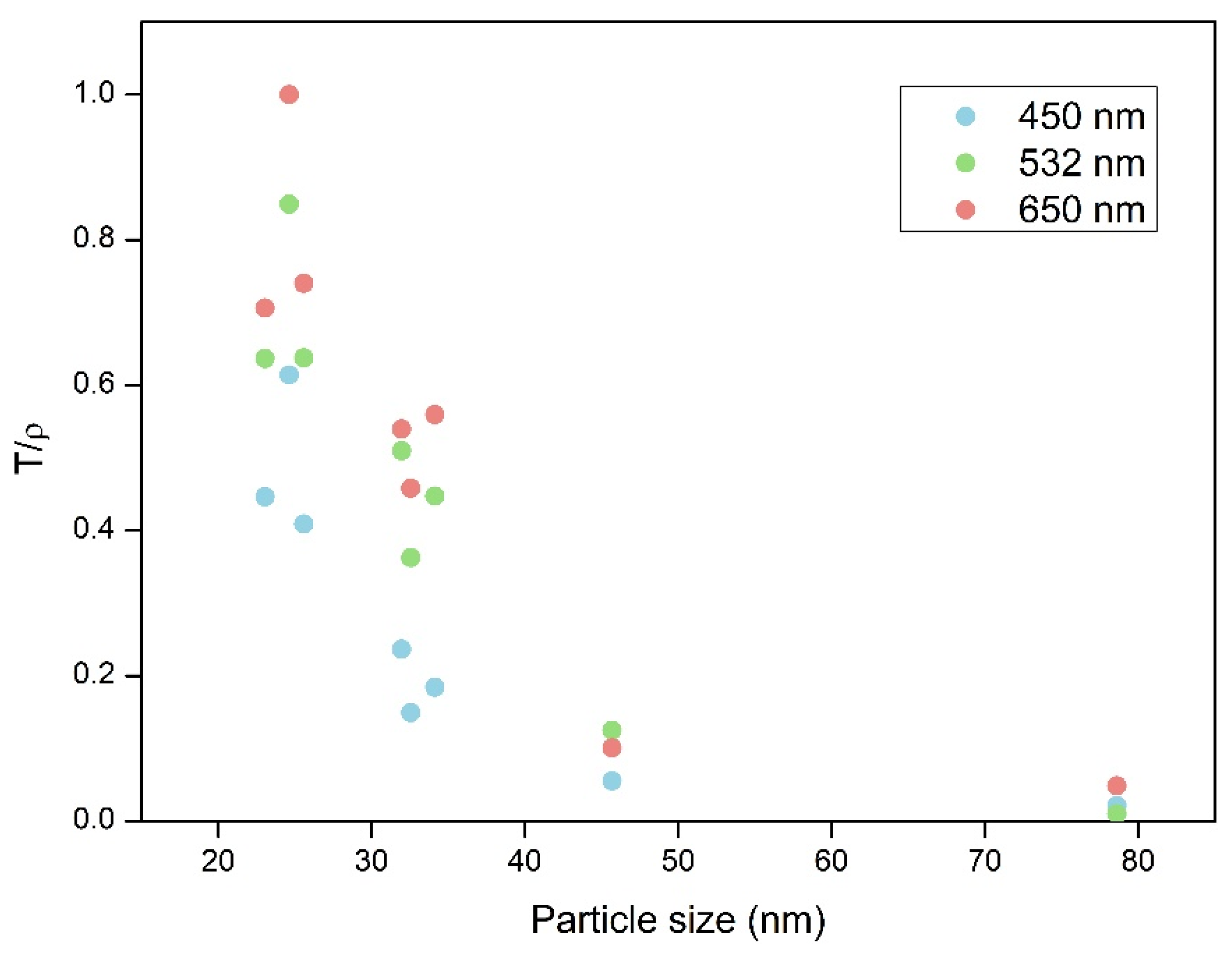
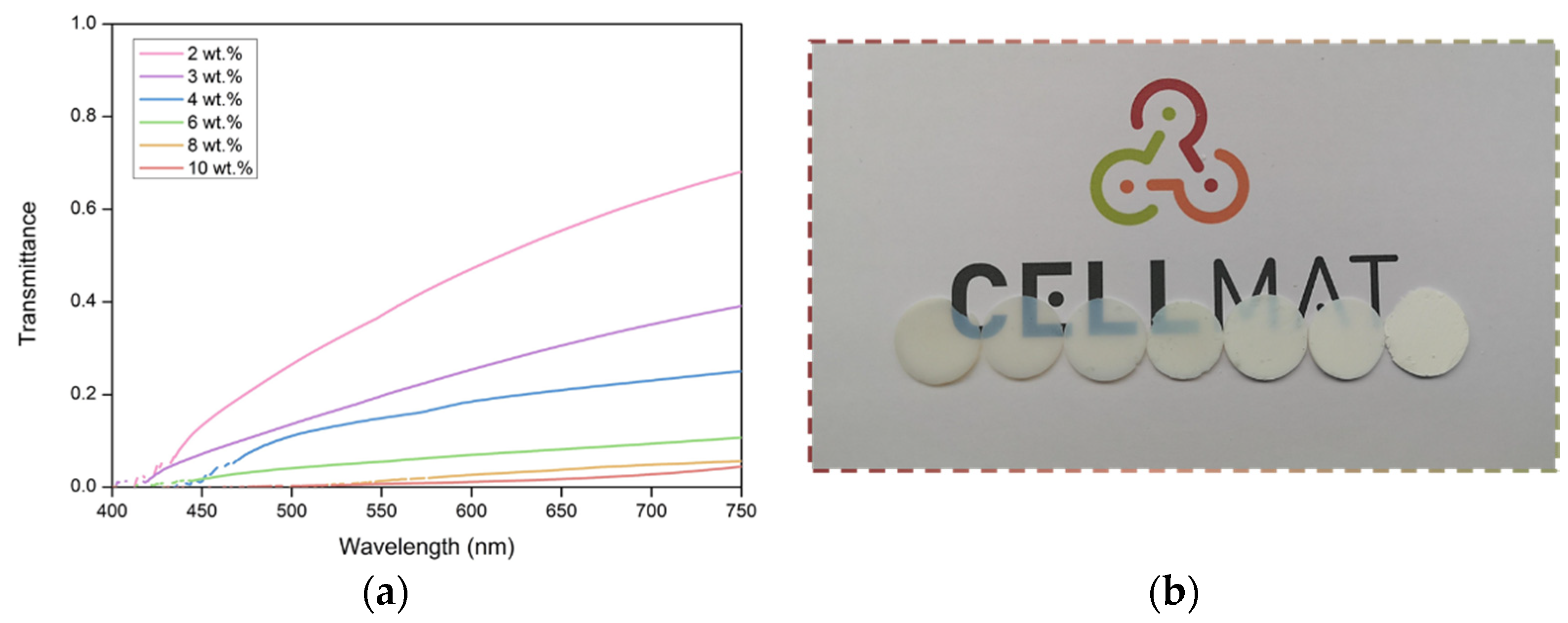
| Catalyst Amount (wt.%) | Density (g/cm3) | Porosity (%) | Particle Size (nm) | Average Pore Size (nm) |
|---|---|---|---|---|
| 2 | 0.097 ± 0.001 | 91.68 | 24.6 ± 5.4 | 85.0 |
| 3 | 0.128 ± 0.023 | 89.08 | 23.0 ± 6.0 | 72.1 |
| 4 | 0.108 ± 0.015 | 90.77 | 25.6 ± 6.4 | 93.1 |
| 6 | 0.109 ± 0.011 | 90.73 | 32.0 ± 6.9 | 91.1 |
| 8 | 0.089 ± 0.001 | 92.38 | 34.1 ± 7.8 | 137.9 |
| 10 | 0.109 ± 0.001 | 90.72 | 32.6 ± 6.9 | 140.6 |
| 15 | 0.081 ± 0.001 | 93.03 | 45.7 ± 12.3 | 249.1 |
| 18 | 0.079 ± 0.003 | 93.23 | 78.6 ± 16.0 | 722.0 |
| L/λ4 | L/λ | |
|---|---|---|
| wt.% | R2 | R2 |
| 2, 3, 4 | 0.994 | 0.962 |
| 6, 8 | 0.994 | 0.966 |
| 10 | 0.977 | 0.991 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Merillas, B.; Martín-de León, J.; Villafañe, F.; Rodríguez-Pérez, M.Á. Optical Properties of Polyisocyanurate–Polyurethane Aerogels: Study of the Scattering Mechanisms. Nanomaterials 2022, 12, 1522. https://doi.org/10.3390/nano12091522
Merillas B, Martín-de León J, Villafañe F, Rodríguez-Pérez MÁ. Optical Properties of Polyisocyanurate–Polyurethane Aerogels: Study of the Scattering Mechanisms. Nanomaterials. 2022; 12(9):1522. https://doi.org/10.3390/nano12091522
Chicago/Turabian StyleMerillas, Beatriz, Judith Martín-de León, Fernando Villafañe, and Miguel Ángel Rodríguez-Pérez. 2022. "Optical Properties of Polyisocyanurate–Polyurethane Aerogels: Study of the Scattering Mechanisms" Nanomaterials 12, no. 9: 1522. https://doi.org/10.3390/nano12091522
APA StyleMerillas, B., Martín-de León, J., Villafañe, F., & Rodríguez-Pérez, M. Á. (2022). Optical Properties of Polyisocyanurate–Polyurethane Aerogels: Study of the Scattering Mechanisms. Nanomaterials, 12(9), 1522. https://doi.org/10.3390/nano12091522










