Structural and Superconducting Proximity Effect of SnPb Bimetallic Nanoalloys
Abstract
1. Introduction
2. Methods
3. Results
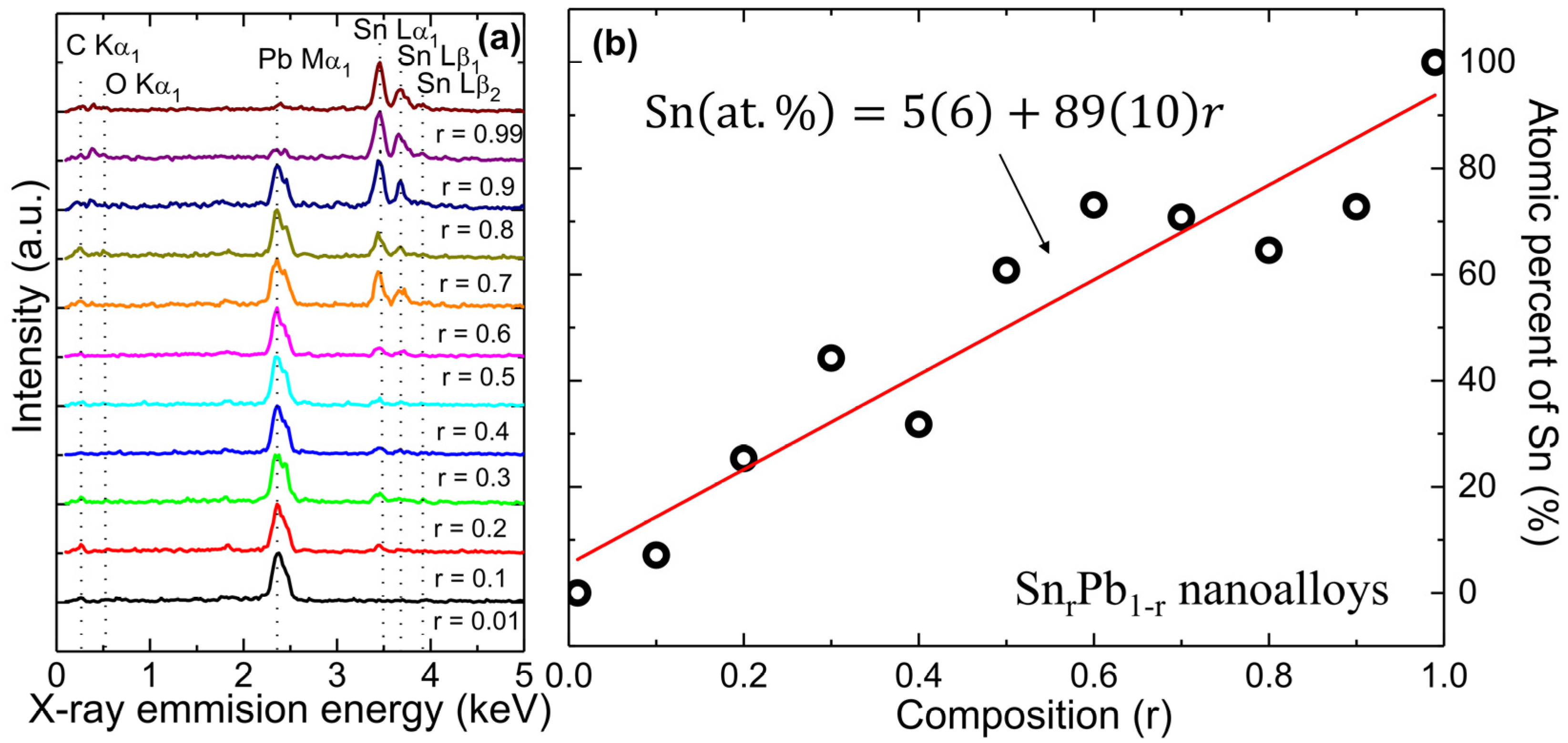
3.1. Elemental Analysis and EDS Mapping of SnPb
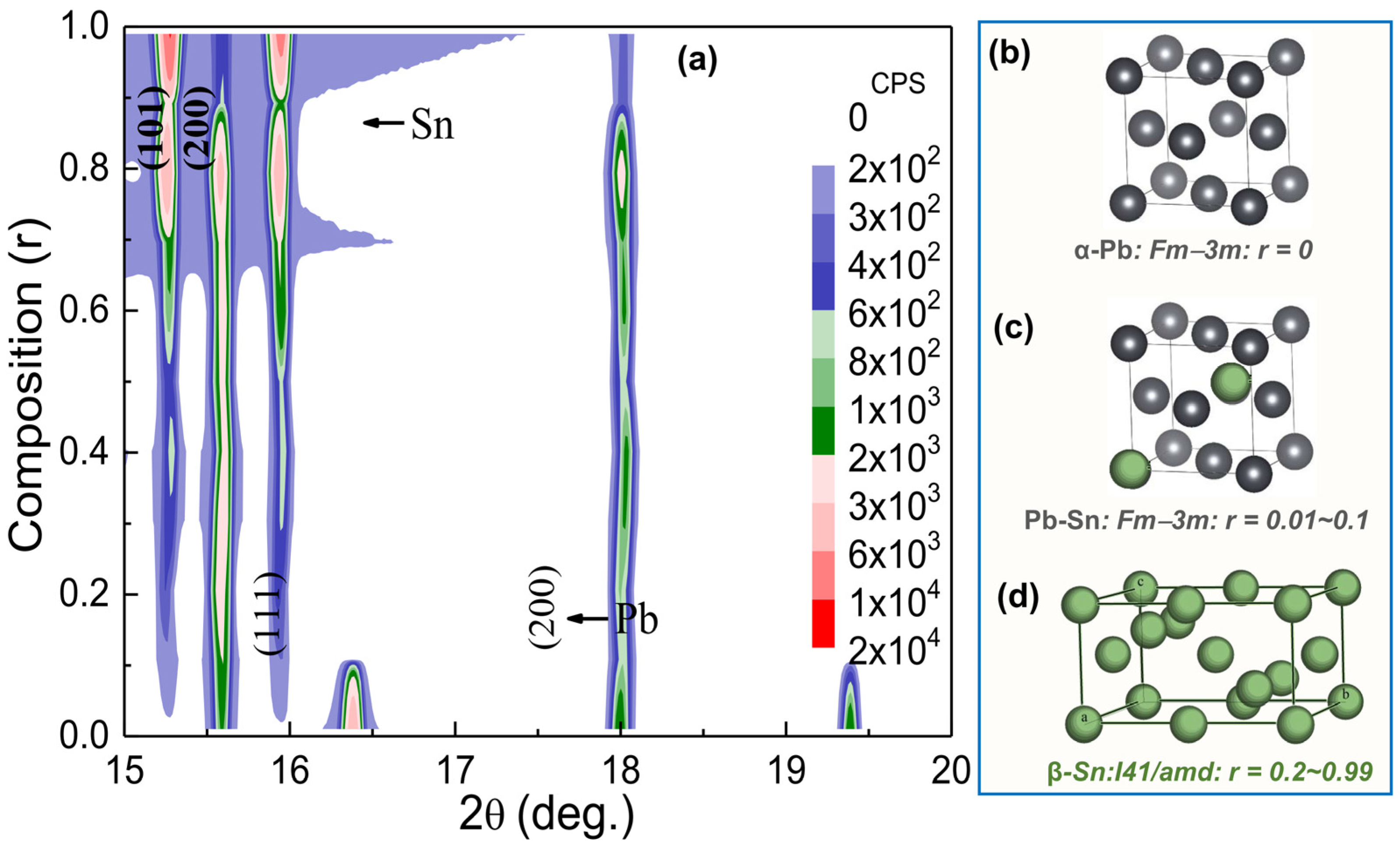
3.2. Crystal Structural and X-ray Refinement Analysis of SnPb
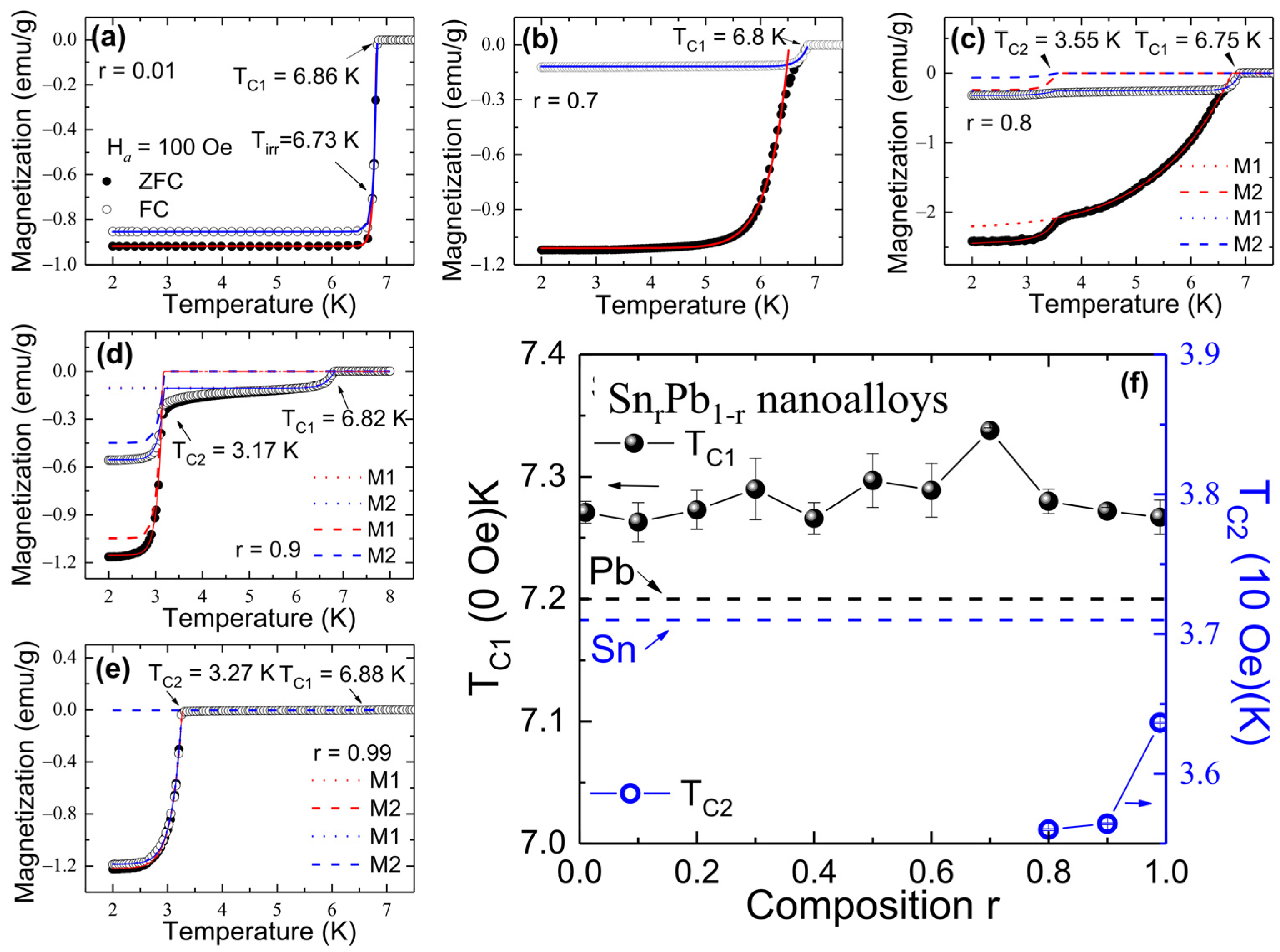
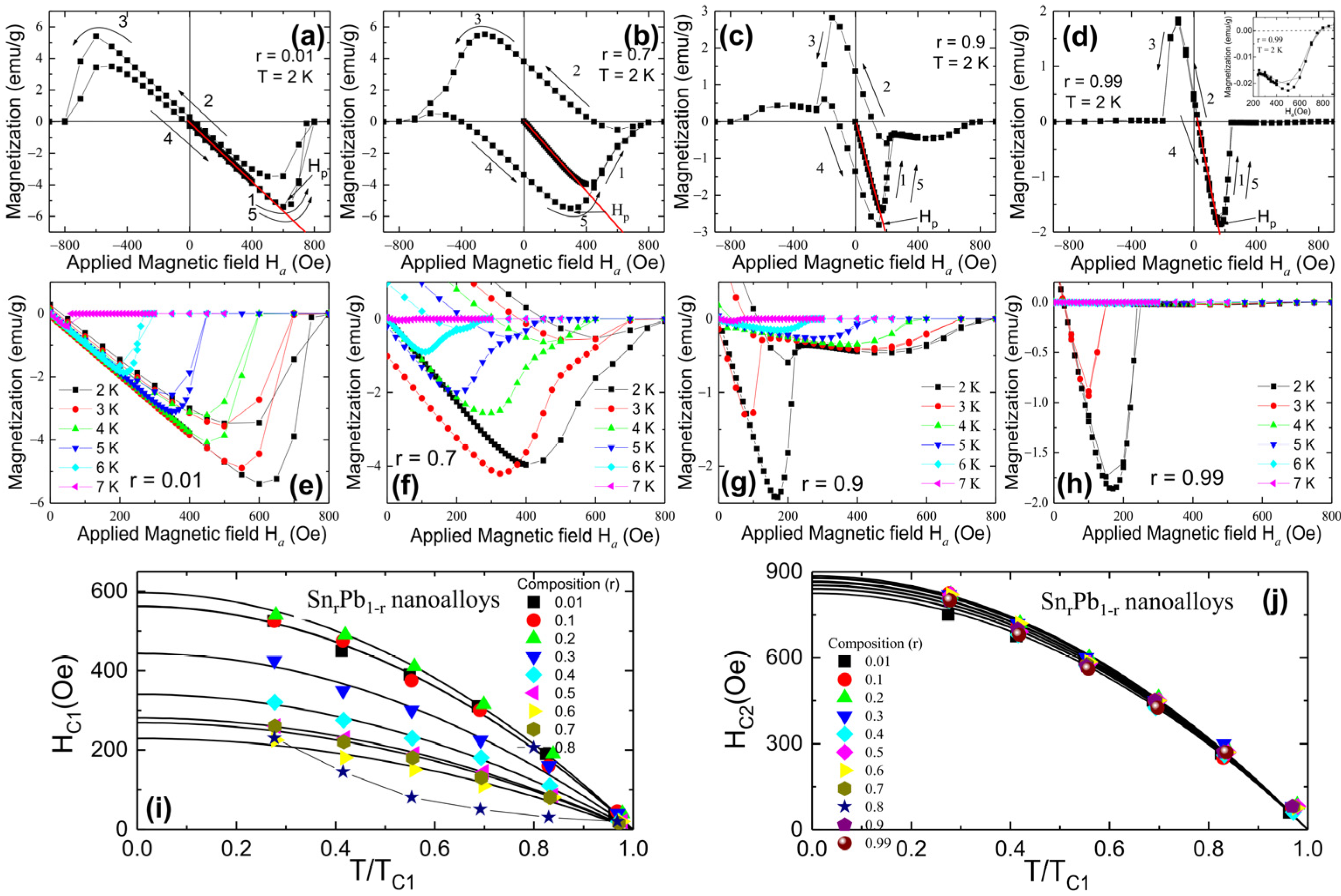
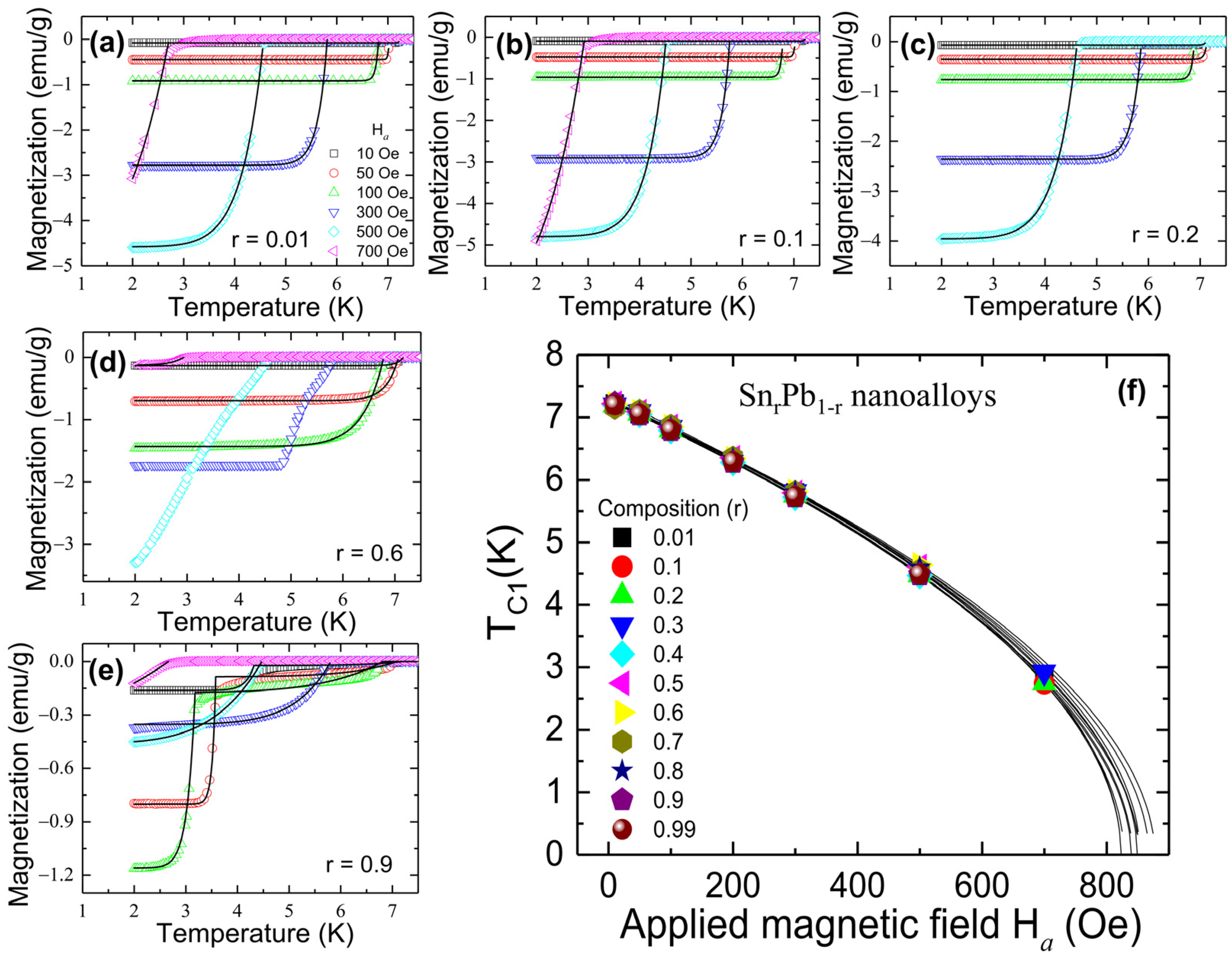
3.3. Temperature Dependence of Magnetization
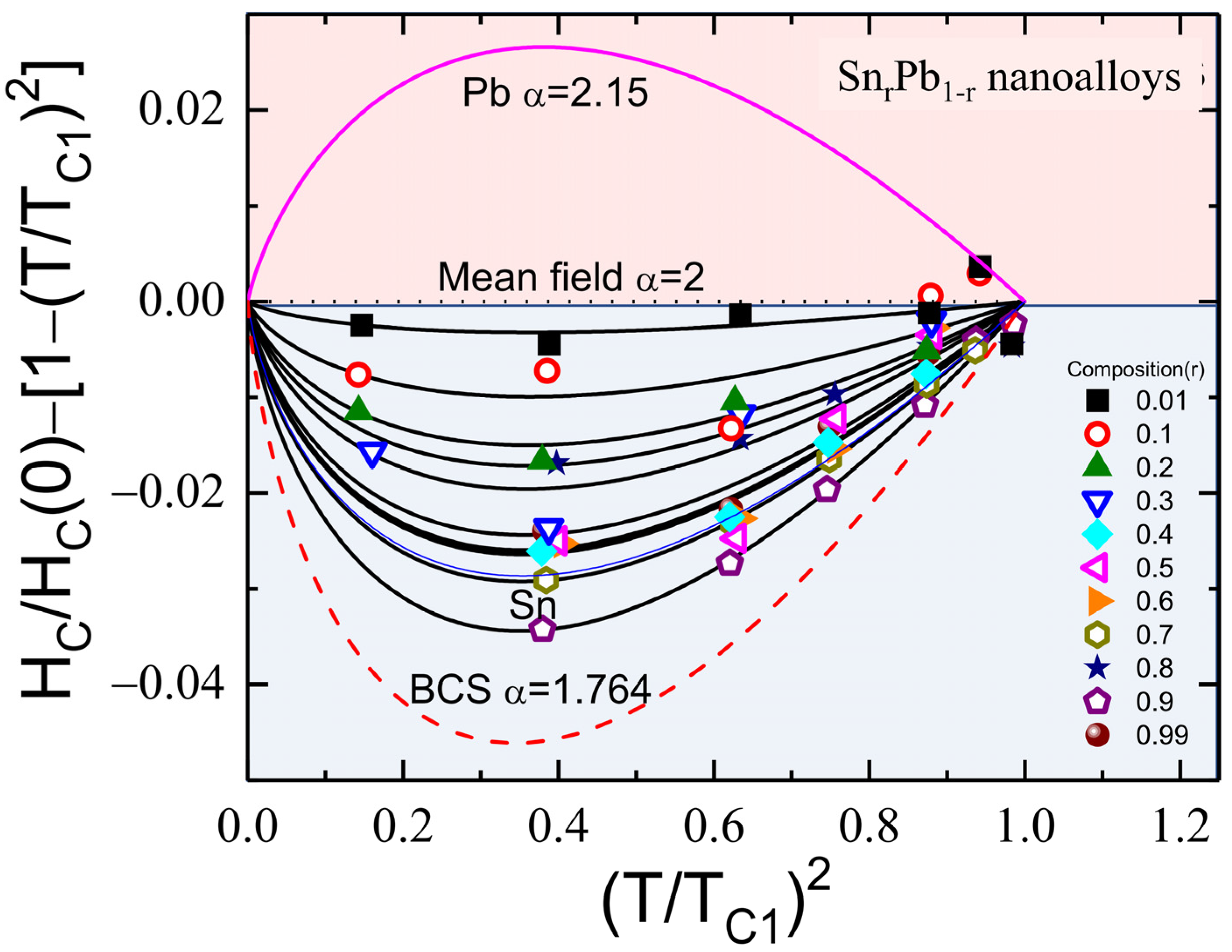
3.4. Critical Fields and Theoretical Analysis
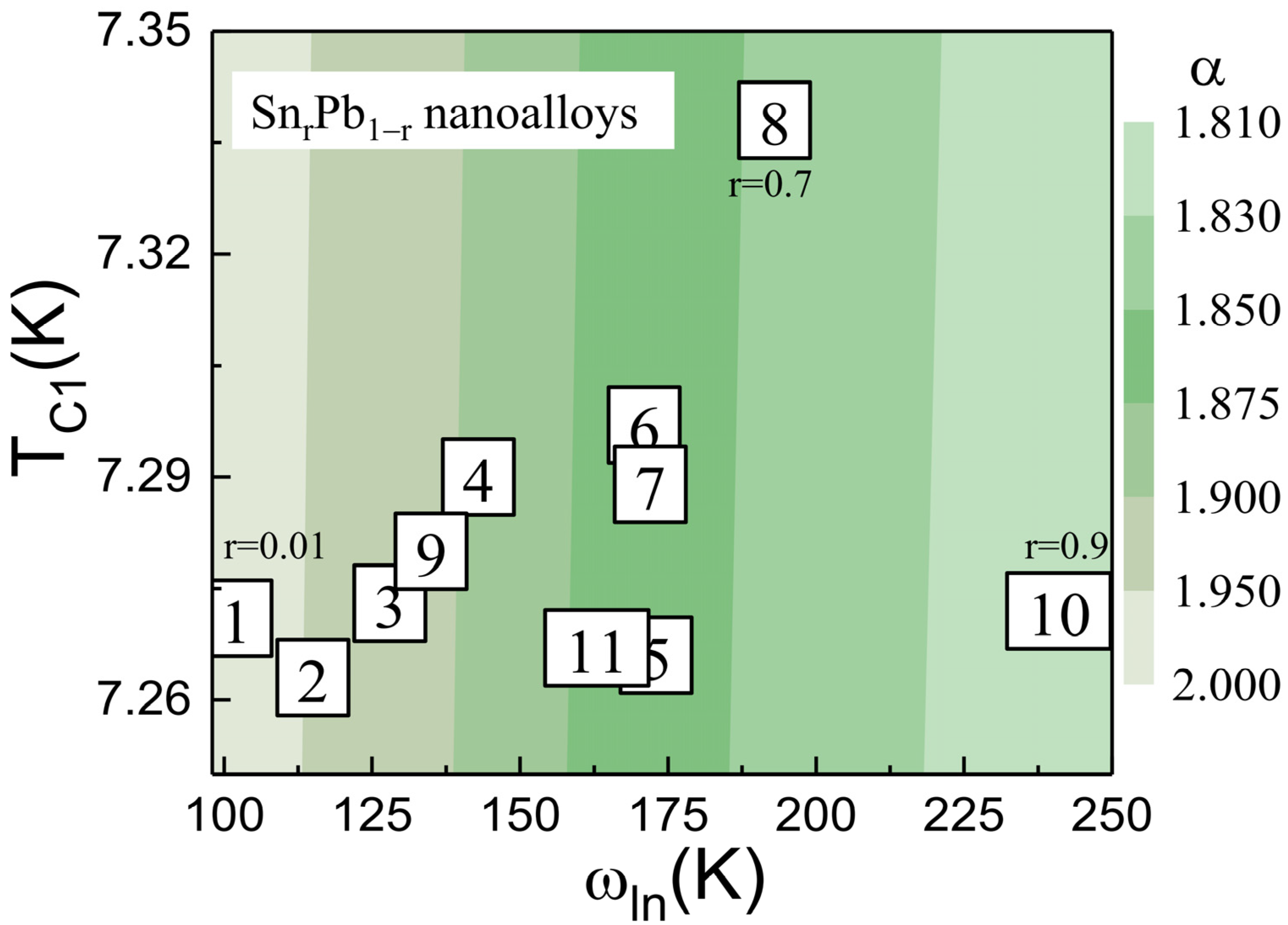
3.5. Superconducting Coupling Strength
4. Discussions
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hauser, J.J.; Theuerer, H.C.; Werthamer, N.R. Proximity effect between a superconducting and a ferromagnetic film. Phys. Lett. 1965, 18, 222–223. [Google Scholar] [CrossRef]
- Qi, X.-L.; Zhang, S.-C. The quantum spin Hall effect and topological insulators. Phys. Today 2010, 63, 33–38. [Google Scholar] [CrossRef]
- He, Q.L.; Pan, L.; Stern, A.L.; Burks, E.C.; Che, X.; Yin, G.; Wang, J.; Lian, B.; Zhou, Q.; Choi, E.S.; et al. Chiral Majorana fermion modes in a quantum anomalous Hall insulator–superconductor structure. Science 2017, 357, 294–299. [Google Scholar] [CrossRef] [PubMed]
- Fang, F.; Ding, Y.; Qu, F.; Shen, J.; Chen, J.; Wei, Z.; Ji, Z.; Liu, G.; Fan, J.; Yang, C.; et al. Proximity effect at superconducting Sn-Bi2Se3 interface. Phys. Rev. B 2012, 85, 104508. [Google Scholar]
- Qu, F.; Yang, F.; Ding, Y.; Chen, J.; Ji, Z.; Liu, G.; Fan, J.; Jing, X.; Yang, C.; Lu, L. Strong Superconducting Proximity Effect in Pb-Bi2Te3 Hybrid Structures. Sci. Rep. 2012, 2, 339. [Google Scholar] [CrossRef]
- de Menten de Horne, F.; Piraux, L.; Michotte, S. Proximity effect in superconducting nanowires. Phys. C Supercond. 2007, 460–462, 1441–1442. [Google Scholar] [CrossRef]
- Garner, J.; Pekarek, T.; Benedek, R. Specific heat of proximity-coupled superconducting multilayers. J. Phys. Chem. Solids 2000, 61, 979–984. [Google Scholar] [CrossRef]
- Rudenko, E.M.; Korotash, I.V.; Krakovny, A.A.; Dubyna, D.S.; Solomakha, D.A.; Shchyptsov, D.S. Nonequilibrium Superconductivity in Double Tunnel Junction with Spatially Inhomogeneous Superconducting Electrode. J. Low. Temp. Phys. 2013, 171, 779–787. [Google Scholar] [CrossRef]
- Eom, D.; Qin, S.; Chou, M.Y.; Shih, C.K. Persistent Superconductivity in Ultrathin Pb Films: A Scanning Tunneling Spectroscopy Study. Phys. Rev. Lett. 2006, 96, 027005. [Google Scholar] [CrossRef]
- Zhang, T.; Cheng, P.; Li, W.-J.; Sun, Y.-J.; Wang, G.; Zhu, X.-G.; He, K.; Wang, L.; Ma, X.; Chen, X.; et al. Superconductivity in one-atomic-layer metal films grown on Si(111). Nat. Phys. 2010, 6, 104–108. [Google Scholar] [CrossRef]
- Raman, V.; Reiley, T.C. Cyclic deformation and fracture in Pb-Sn solid solution alloy. Metall. Trans. A 1988, 19, 1533. [Google Scholar] [CrossRef]
- Hume-Rothery, W. The Structure of Metals and Alloys. J. Chem. Educ. 1936, 13, 350. [Google Scholar]
- Hume-Rothery, W. Atomic Theory for Students of Metallurgy; The Institute of Metals: London, UK, 1969. [Google Scholar]
- Habashi, F. Alloys: Preparation, Properties, Applications; Wiley: Hoboken, NJ, USA, 2008. [Google Scholar]
- Rietveld, H. A profile refinement method for nuclear and magnetic structures. J. Appl. Crystallogr. 1969, 2, 65–71. [Google Scholar] [CrossRef]
- Larson, A.C.; Von Dreele, R.B. General Structure Analysis System (GSAS). Los Alamos Natl. Lab. Rep. LAUR 2000, 86, 221. [Google Scholar]
- Abraimov, V.V.; Kalugin, M.M.; Soldatov, V.P. Influence of magnetic flux trapping on softening effect during superconducting transition in alloys Pb-Ni and Pb-Sn. Cryst. Res. Technol. 1984, 19, 1057–1063. [Google Scholar] [CrossRef]
- London, F.; London, H. The Electromagnetic Equations of the Supraconductor. Proc. R. Soc. Lond. Ser. A-Math. Phys. Sci. 1935, 149, 71–88. [Google Scholar]
- Prozorov, R.; Giannetta, R.W. Magnetic Penetration Depth in Unconventional Superconductors. Supercond. Sci. Technol. 2006, 19, R41–R67. [Google Scholar] [CrossRef]
- Gandhi, A.C.; Chan, T.S.; Wu, S.Y. Phase diagram of PbBi alloys: Structure-property relations and the superconducting coupling. Supercond. Sci. Technol. 2017, 30, 105010. [Google Scholar] [CrossRef]
- Gandhi, A.C.; Wu, S.Y. Phase diagram and superconductivity: New insight into the fundamentals of InSn bimetallic alloys. Inorg. Chem. 2019, 58, 794–802. [Google Scholar] [CrossRef]
- Gandhi, A.C.; Wu, S.Y. Routes to probe Bismuth induced strong-coupling superconductivity in bimetallic BiIn alloys. Sci. Rep. 2017, 7, 9442. [Google Scholar] [CrossRef]
- Ginzburg, V.L. The theory of superconductivity. Zh. Eksp. Teor. Fiz. 1950, 20, 1064. [Google Scholar]
- Engelmann, J.; Grinenko, V.; Chekhonin, P.; Skrotzki, W.; Efremov, D.V.; Oswald, S.; Iida, K.; Hühne, R.; Hänisch, J.; Hoffmann, M.; et al. Strain induced superconductivity in the parent compound BaFe2As2. Nat. Commun. 2003, 4, 2877. [Google Scholar] [CrossRef]
- Levine, J.L. Density of States of a Short-Mean-Free-Path Superconductor in a Magnetic Field by Electron Tunneling. Phys. Rev. 1967, 155, 373–378. [Google Scholar] [CrossRef]
- Müller, W.H.G. Effect of elastic strain and stress on the superconducting transition temperature of quench-condensed films. Z. Phys. B Condens. Matter 1980, 38, 49–58. [Google Scholar] [CrossRef]
- Park, S.; Zhang, C.L.; Lee, N.; Choi, Y.J.; Guha, S.; Cheong, S.-W. Enhanced superconducting Tc in the immiscible system (La1.85Sr0.15CuO4)x(Lu2Cu2O5)1-x. Phys. Rev. B 2011, 83, 220509. [Google Scholar] [CrossRef]
- Clavero, C.; Beringer, D.B.; Roach, W.M.; Skuza, J.R.; Wong, K.C.; Batchelor, A.D.; Reece, C.E.; Lukaszew, R.A. Strain Effects on the Crystal Growth and Superconducting Properties of Epitaxial Niobium Ultrathin Films. Cryst. Growth Des. 2012, 12, 2588–2593. [Google Scholar] [CrossRef]
- Bose, S.; Bhattacharya, V.; Chattopadhyay, K.; Ayyub, P. Proximity effect controlled superconducting behavior of novel biphasic Pb–Sn nanoparticles embedded in an Al matrix. Acta Mater. 2008, 56, 4522–4528. [Google Scholar] [CrossRef]
- Padamsee, H.; Neighbor, J.E.; Shiffman, C.A. Quasiparticle phenomenology for thermodynamics of strong-coupling superconductors. J. Low Temp. Phys. 1973, 12, 387–411. [Google Scholar] [CrossRef]
- Maxwell, E.; Lutes, O.S. Threshold Field Properties of Some Superconductors. Phys. Rev. 1954, 95, 333–338. [Google Scholar] [CrossRef]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Microscopic Theory of Superconductivity. Phys. Rev. 1957, 106, 162–164. [Google Scholar] [CrossRef]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Theory of Superconductivity. Phys. Rev. 1957, 108, 1175–1204. [Google Scholar] [CrossRef]
- McMillan, W.L. Transition temperature of strong-coupled superconductors. Phys. Rev. 1968, 167, 331–344. [Google Scholar] [CrossRef]
- Mitrović, B.; Zarate, H.G.; Carbotte, J.P. The ratio 2Δ/kBTc within Eliashberg theory. Phys. Rev. B 1984, 29, 184–190. [Google Scholar] [CrossRef]
- Pippard, A.B. An Experimental and Theoretical Study of the Relation between Magnetic Field and Current in a Superconductor. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1953, 216, 547–568. [Google Scholar]
- Gao, F.; Bacon, D.J.; Flewitt PE, J.; Lewis, T.A. The effects of electron-phonon coupling on defect production by displacement cascades in α-iron. Model. Simul. Mater. Sci. Eng. 1998, 6, 543. [Google Scholar] [CrossRef]
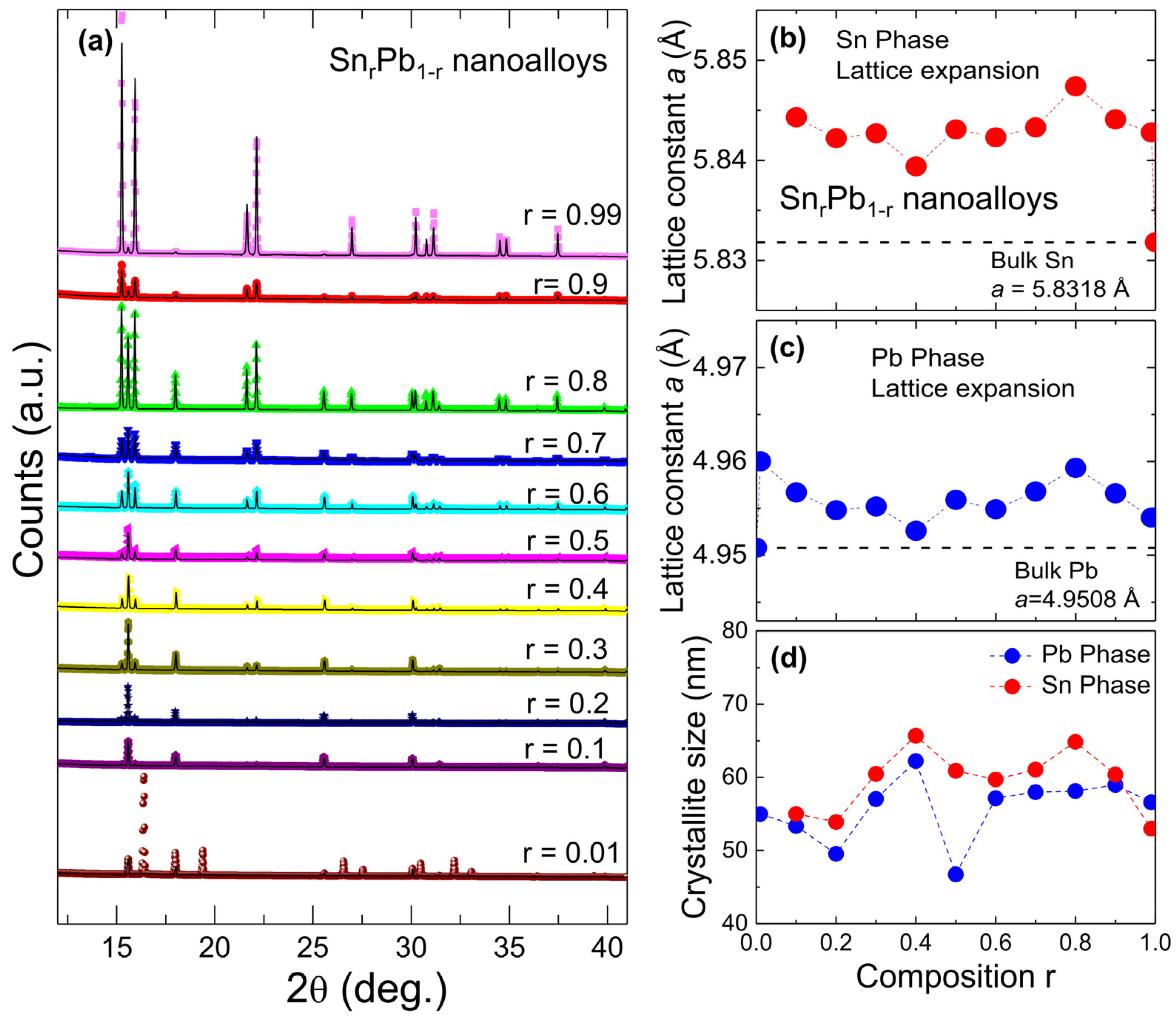

| SnrPb1-r | Lattice Constants (Å) | Rwp | Rp | χ2 | Crystalline Size (nm) | Wt. Fraction (%) | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| Pb Phase | β-Sn Phase | Pb Phase | Sn Phase | |||||||
| a = b = c | a = b | c | Pb | β-Sn | ||||||
| 0.01 | 4.9600 (1) | 0.3554 | 0.1881 | 21.45 | 55 | 100 | 0 | |||
| 0.1 | 4.9567 (1) | 5.8443 (4) | 3.1884 (3) | 0.0265 | 0.0111 | 0.1184 | 53 | 55 | 75.123 | 24.877 |
| 0.2 | 4.9548 (1) | 5.8422 (3) | 3.1859 (2) | 0.0424 | 0.0215 | 0.2699 | 50 | 54 | 69.128 | 30.872 |
| 0.3 | 4.9552(1) | 5.8427 (1) | 3.1875 (1) | 0.031 | 0.017 | 0.1587 | 57 | 60 | 68.782 | 31.218 |
| 0.4 | 4.9526 (1) | 5.8394 (1) | 3.1855 (1) | 0.0344 | 0.018 | 0.1888 | 62 | 66 | 65.555 | 34.445 |
| 0.5 | 4.9559 (1) | 5.8431 (2) | 3.1877 (1) | 0.0442 | 0.0229 | 0.2843 | 47 | 61 | 50.246 | 49.754 |
| 0.6 | 4.9549 (1) | 5.8423 (1) | 3.1872 (1) | 0.0555 | 0.0306 | 0.4864 | 57 | 60 | 36.476 | 63.524 |
| 0.7 | 4.9568 (1) | 5.8433 (1) | 3.1894 (1) | 0.0459 | 0.0246 | 0.3812 | 58 | 61 | 54.848 | 45.152 |
| 0.8 | 4.9593 (1) | 5.8474 (1) | 3.1894 (1) | 0.0716 | 0.04 | 1.175 | 58 | 65 | 41.589 | 58.411 |
| 0.9 | 4.9566 (2) | 5.8441 (1) | 3.1877 (1) | 0.0479 | 0.0236 | 0.3648 | 59 | 60 | 34.294 | 65.706 |
| 0.99 | 4.9540 (9) | 5.8428 (2) | 3.1878 (1) | 0.1424 | 0.0903 | 5.461 | 57 | 53 | 0.775 | 99.225 |
| SnrPb1-r | <d> nm | HC1(0) Oe | HC2(0) (Oe) | HTC(0) (Oe) | ξ(0) (Å) | λ (Å) | ξGL(0) (Å) | ||
|---|---|---|---|---|---|---|---|---|---|
| 0.01 | 121 (10) | 562 (7) | 824 (8) | 681 | 896 | 541 | 632 | 0.856 | 1536 |
| 0.1 | 105 (9) | 563 (8) | 863 (13) | 697 | 897 | 540 | 618 | 0.875 | 1340 |
| 0.2 | 80 (5) | 596 (7) | 886 (16) | 727 | 896 | 525 | 609 | 0.862 | 1260 |
| 0.3 | 79 (4) | 444 (10) | 880 (12) | 625 | 894 | 609 | 612 | 0.995 | 1298 |
| 0.4 | 77 (5) | 340 (4) | 839 (10) | 534 | 897 | 696 | 626 | 1.111 | 1452 |
| 0.5 | 70 (3) | 281 (3) | 867 (17) | 494 | 893 | 765 | 616 | 1.242 | 1358 |
| 0.6 | 81 (4) | 229 (7) | 877 (13) | 448 | 894 | 848 | 613 | 1.384 | 1309 |
| 0.7 | 75 (5) | 269 (5) | 852 (13) | 479 | 888 | 782 | 622 | 1.258 | 1472 |
| 0.8 | 75 (4) | 260 | 854 (10) | 471 | 895 | 795 | 621 | 1.281 | 1397 |
| 0.9 | 69 (3) | 250 | 852 (11) | 462 | 896 | 811 | 622 | 1.305 | 1398 |
| 0.99 | 46 (3) | 240 | 841 (13) | 449 | 897 | 828 | 626 | 1.323 | 1444 |
| Sample# | SnrPb1-r | TC1(0) (K) | HC (Oe) | γ | α | ωln (K) | λep |
|---|---|---|---|---|---|---|---|
| 1 | 0.01 | 7.271 (9) | 823 (5) | 0.505 (8) | 1.983 (10) | 102 | 1.046 |
| 2 | 0.1 | 7.263 (16) | 823 (8) | 0.513 (13) | 1.947 (15) | 115 | 0.97 |
| 3 | 0.2 | 7.273 (16) | 828 (8) | 0.521 (14) | 1.920 (16) | 128 | 0.913 |
| 4 | 0.3 | 7.290 (25) | 850 (15) | 0.528 (23) | 1.896 (23) | 143 | 0.862 |
| 5 | 0.4 | 7.266 (13) | 840 (31) | 0.537 (31) | 1.861 (12) | 173 | 0.785 |
| 6 | 0.5 | 7.297 (22) | 868 (59) | 0.537 (57) | 1.864 (19) | 171 | 0.79 |
| 7 | 0.6 | 7.289 (22) | 878 (64) | 0.537 (60) | 1.863 (20) | 172 | 0.788 |
| 8 | 0.7 | 7.338 (2) | 852 (5) | 0.541 (5) | 1.847 (1) | 193 | 0.751 |
| 9 | 0.8 | 7.280 (10) | 854 (27) | 0.524 (26) | 1.909 (10) | 135 | 0.887 |
| 10 | 0.9 | 7.272 (3) | 853 (8) | 0.549 (8) | 1.821 (3) | 241 | 0.685 |
| 11 | 0.99 | 7.267 (14) | 841 (33) | 0.534 (33) | 1.872 (12) | 163 | 0.807 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gandhi, A.C.; Manjunatha, K.; Chan, T.-S.; Wu, S.Y. Structural and Superconducting Proximity Effect of SnPb Bimetallic Nanoalloys. Nanomaterials 2022, 12, 4323. https://doi.org/10.3390/nano12234323
Gandhi AC, Manjunatha K, Chan T-S, Wu SY. Structural and Superconducting Proximity Effect of SnPb Bimetallic Nanoalloys. Nanomaterials. 2022; 12(23):4323. https://doi.org/10.3390/nano12234323
Chicago/Turabian StyleGandhi, Ashish Chhaganlal, Krishtappa Manjunatha, Ting-Shan Chan, and Sheng Yun Wu. 2022. "Structural and Superconducting Proximity Effect of SnPb Bimetallic Nanoalloys" Nanomaterials 12, no. 23: 4323. https://doi.org/10.3390/nano12234323
APA StyleGandhi, A. C., Manjunatha, K., Chan, T.-S., & Wu, S. Y. (2022). Structural and Superconducting Proximity Effect of SnPb Bimetallic Nanoalloys. Nanomaterials, 12(23), 4323. https://doi.org/10.3390/nano12234323







