Reverse Magnetization Behavior Investigation of Mn-Al-C-(α-Fe) Nanocomposite Alloys with Different α-Fe Content Using First-Order Reversal Curves Analysis
Abstract
1. Introduction
2. Experimental
2.1. Materials and Instrumentation
2.2. Mn52Al45.7C2.3 Nano Powder Fabrication
2.3. Processing of Nanostructured Bulk Magnets
3. Results and Discussions
3.1. Structural Analysis
3.2. Magnetic Properties
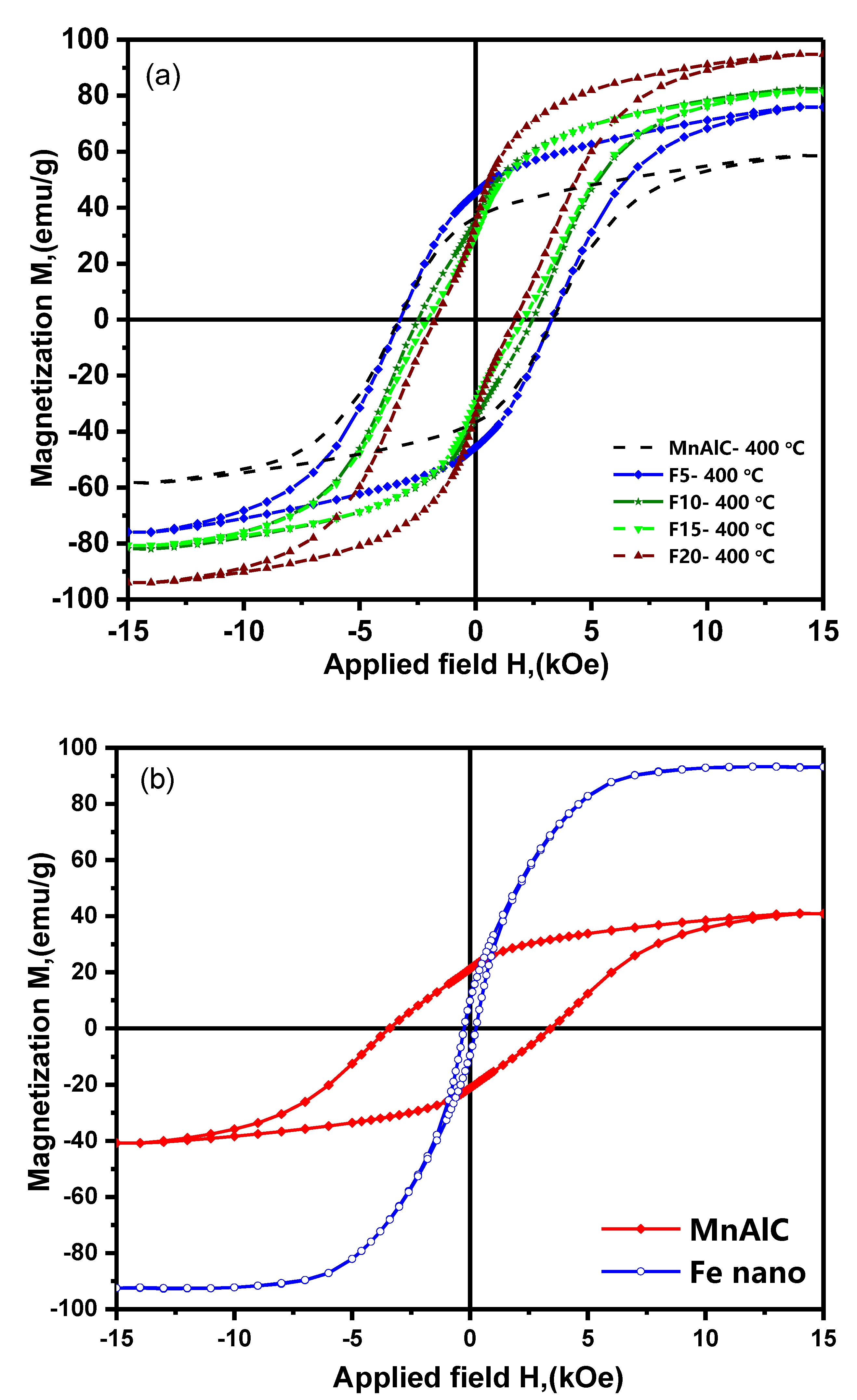
3.2.1. Hysteresis Loops Investigation
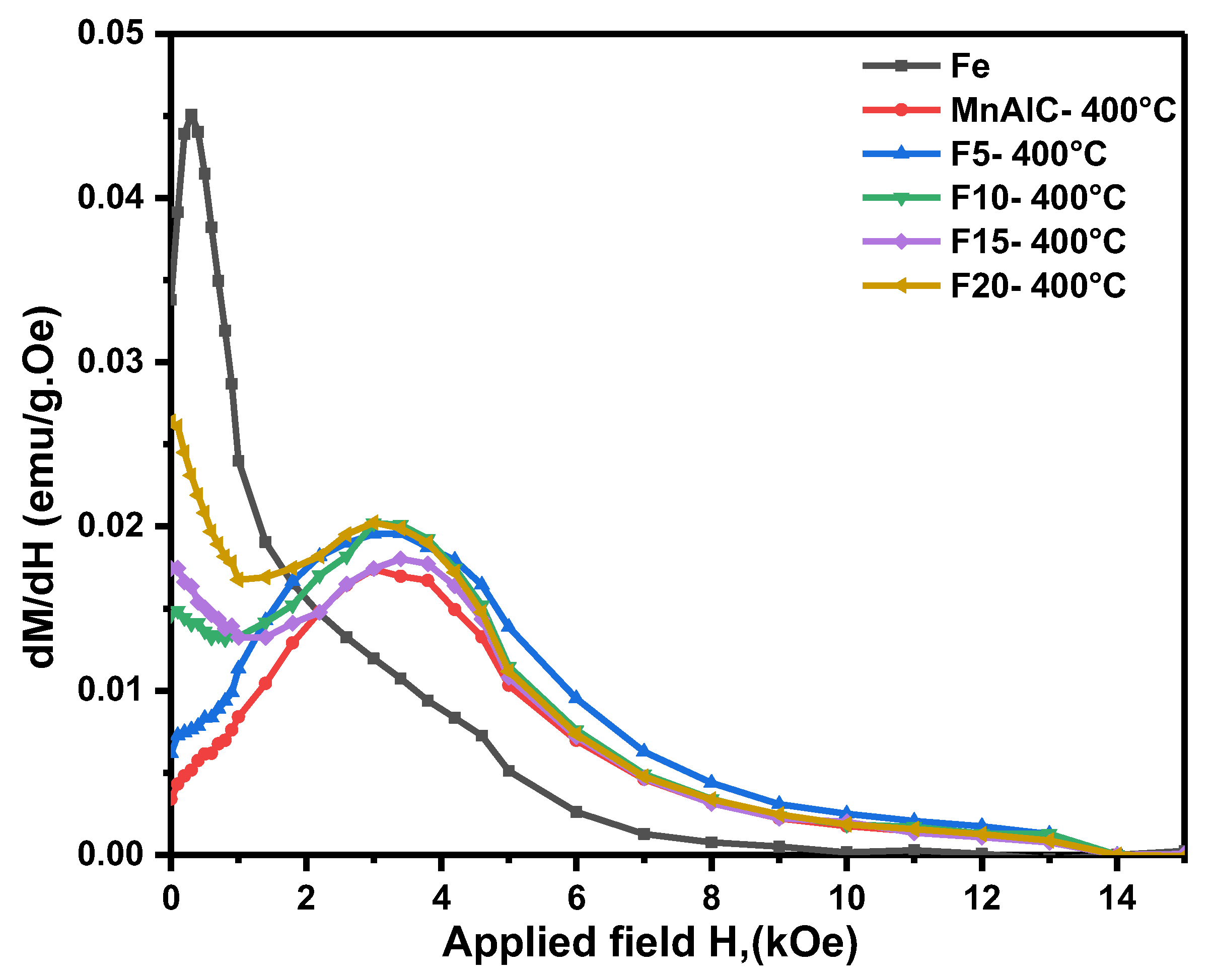
3.2.2. Switching Field Distribution Curves Investigation
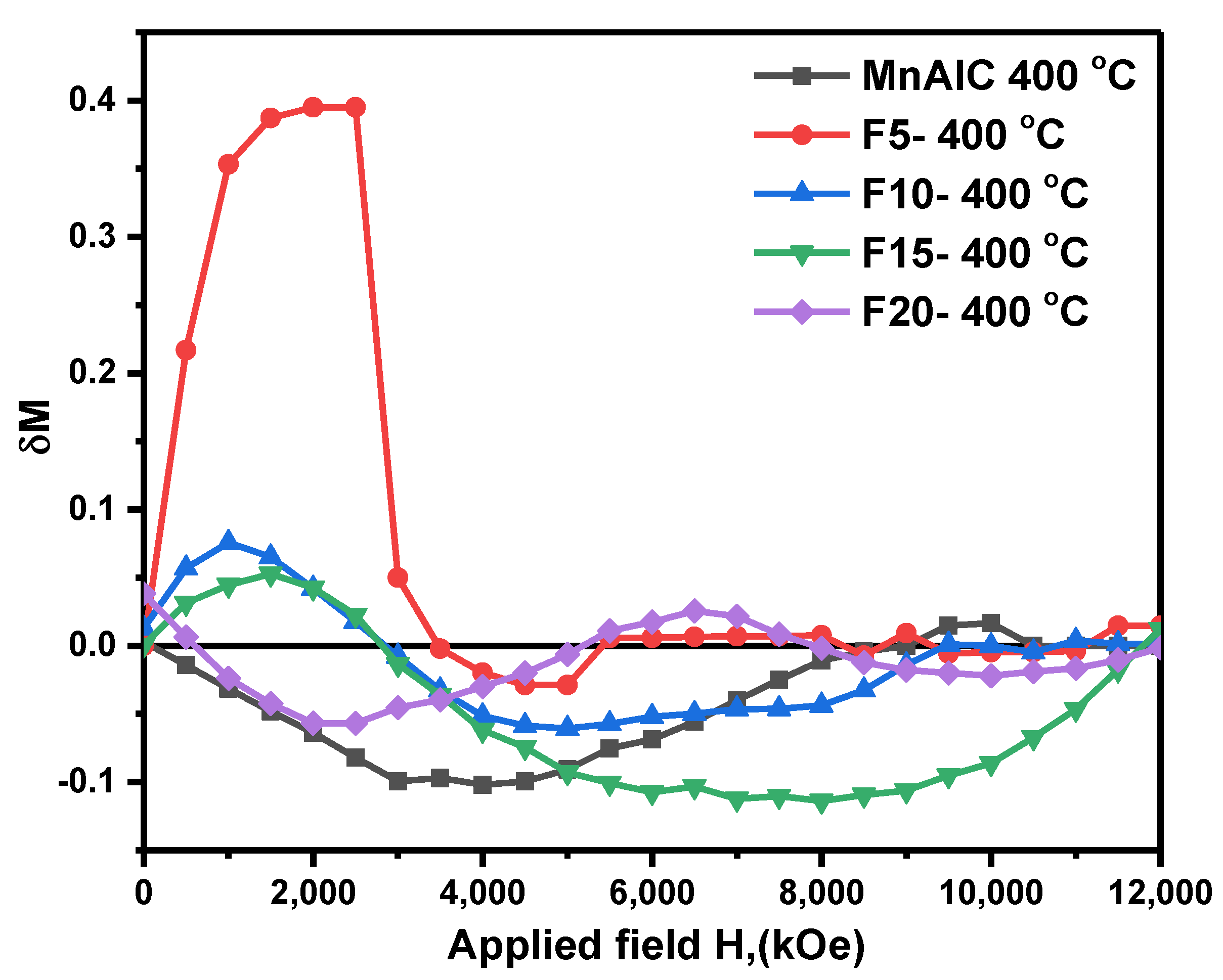
3.2.3. Henkel Plots Investigation
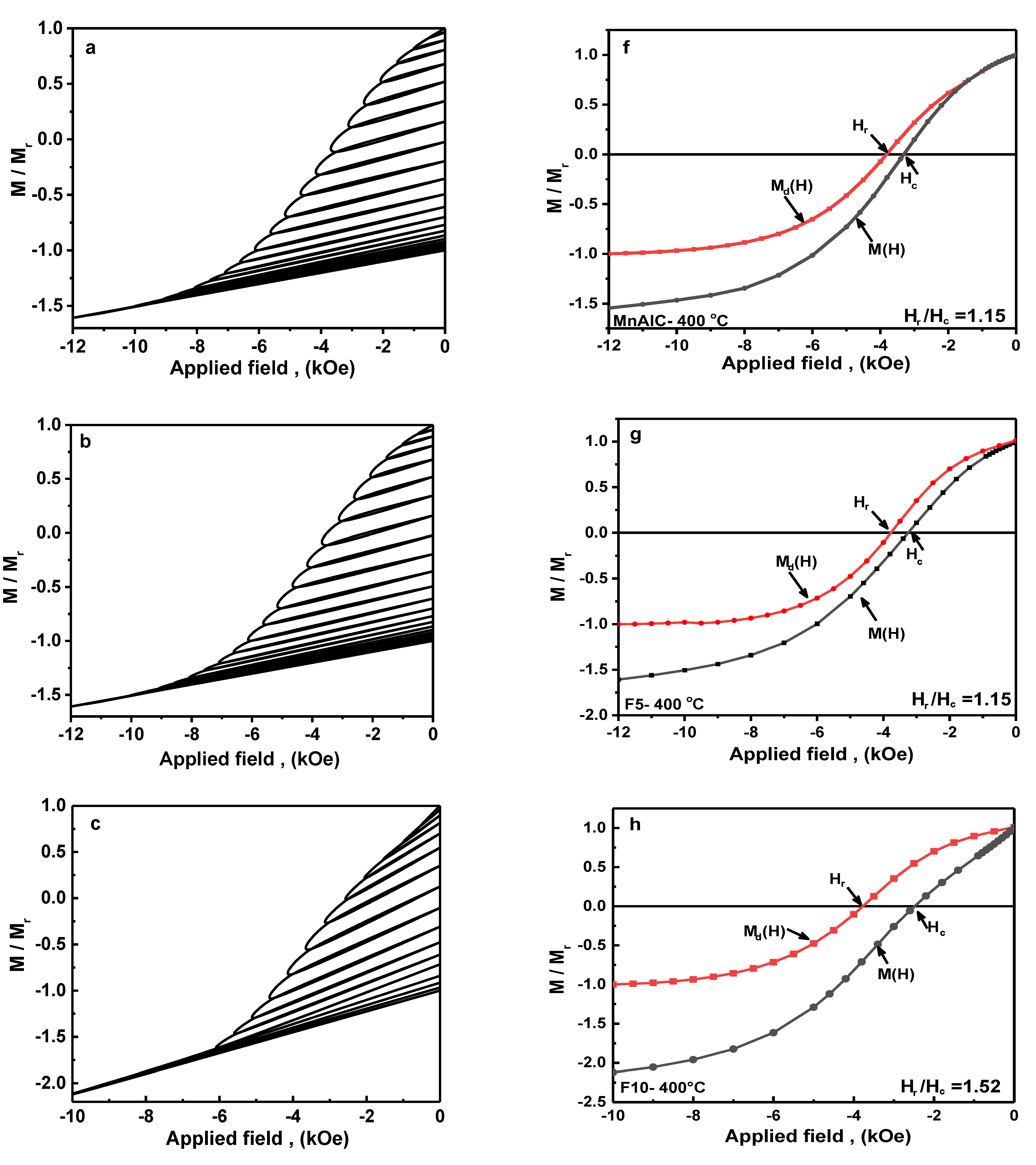
3.2.4. Recoil Curves Investigation
3.2.5. First Order Reversal Curves (FORC) Analysis
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wei, Y.; Li, Y.Q.; Zhang, D.T.; Yue, M. Improvement of magnetic properties of smco 5/α-Fe nanocomposite magnets by magnetic field annealing. J. Magn. 2018, 23, 381–385. [Google Scholar] [CrossRef]
- Ryo, H.S.; Hu, L.X.; Yang, Y.L. Micromagnetic study for magnetic properties of exchange coupled nanocomposite magnetic systems with Nd2Fe14B grains embedded in α-Fe matrix. J. Magn. Magn. Mater. 2017, 426, 46–52. [Google Scholar] [CrossRef]
- Pop, V.; Gutoiu, S.; Dorolti, E.; Leostean, C.; Isnard, O.; Chicinas, I.; Pana, O. The influence of milling and annealing on the structural and magnetic behavior of Nd2Fe14B/α-Fe magnetic nanocomposite. J. Alloys Compd. 2013, 581, 821–827. [Google Scholar] [CrossRef]
- Rong, C.-B.; Zhang, Y.; Kramer, M.J.; Liu, J.P. Correlation between microstructure and first-order magnetization reversal in the SmCo5/α-Fe nanocomposite magnets. Phys. Lett. A 2011, 375, 1329–1332. [Google Scholar] [CrossRef]
- Rong, C.; Zhang, Y.; Poudyal, N.; Wang, D.; Kramer, M.J.; Liu, J.P. Bulk SmCo5/α-Fe nanocomposite permanent magnets fabricated by mould-free Joule-heating compaction. J. Appl. Phys. 2011, 109, 07A735. [Google Scholar] [CrossRef]
- Zhang, P.Y.; Hiergeist, R.; Lüdke, J.; Albrecht, M.; Ge, H.L. Magnetization reversal behavior in high coercivity Zr doped α-Fe/Nd2Fe14B nanocomposite alloys. J. Appl. Phys. 2010, 108, 043905. [Google Scholar] [CrossRef]
- Kneller, E.F.; Hawig, R. The exchange-spring magnet: A new material principle for permanent magnets. IEEE Trans. Magn. 1991, 27, 3588–3600. [Google Scholar] [CrossRef]
- Coey, J.M.D.; Skomski, R. New magnets from interstitial intermetallics. Phys. Scr. 1993, 1993, 315–321. [Google Scholar] [CrossRef]
- Coehoorn, R.; de Mooij, D.; Duchateau, J.; Buschow, K. Novel Permanent Magnetic Materials Made by Rapid Quenching. J. Phys. Colloq. 1988, 49, C8-669–C668-670. [Google Scholar] [CrossRef]
- Buschow, K.H.J.; De Mooij, D.B.; Coehoorn, R. Metastable ferromagnetic materials for permanent magnets. J. Less Common Met. 1988, 145, 601–611. [Google Scholar] [CrossRef]
- Bance, S.; Bittner, F.; Woodcock, T.G.; Schultz, L.; Schrefl, T. Role of twin and anti-phase defects in MnAl permanent magnets. Acta Mater. 2017, 131, 48–56. [Google Scholar] [CrossRef]
- Coey, J.M.D. Permanent magnets: Plugging the gap. Scr. Mater. 2012, 67, 524–529. [Google Scholar] [CrossRef]
- Luo, L.; Anuniwat, N.; Dao, N.; Cui, Y.; Wolf, S.A.; Lu, J. Magneto-transport and domain wall scattering in epitaxy L10 MnAl thin film. J. Appl. Phys. 2016, 119, 103902. [Google Scholar] [CrossRef]
- Wang, H.X.; Si, P.Z.; Jiang, W.; Liu, J.J.; Lee, J.G.; Choi, C.J.; Ge, H.L. Structure and magnetic properties of MnAl/α-Fe nano-composite powders prepared by high-energy ball milling. Adv. Mater. Res. 2011, 287–290, 1492–1495. [Google Scholar]
- Lu, W.; Niu, J.; Wang, T.; Xia, K.; Xiang, Z.; Song, Y.; Zhang, H.; Yoshimura, S.; Saito, H. Low-energy mechanically milled τ-phase MnAl alloys with high coercivity and magnetization. J. Alloys Compd. 2016, 675, 163–167. [Google Scholar] [CrossRef]
- Wei, J.Z.; Song, Z.G.; Yang, Y.B.; Liu, S.Q.; Du, H.L.; Han, J.Z.; Zhou, D.; Wang, C.S.; Yang, Y.C.; Franz, A.; et al. τ−MnAl with high coercivity and saturation magnetization. Am. Inst. Phys. 2014, 4, 127113. [Google Scholar] [CrossRef]
- Chaturvedi, A.; Yaqub, R.; Baker, I. Microstructure and Magnetic Properties of Bulk Nanocrystalline MnAl. Metals 2014, 4, 20–27. [Google Scholar] [CrossRef]
- Law, J.Y.; Rial, J.; Villanueva, M.; López, N.; Camarero, J.; Marshall, L.G.; Blázquez, J.S.; Borrego, J.M.; Franco, V.; Conde, A.; et al. Study of phases evolution in high-coercive MnAl powders obtained through short milling time of gas-atomized particles. J. Alloys Compd. 2017, 712, 373–378. [Google Scholar] [CrossRef]
- Rial, J.; Villanueva, M.; Céspedes, E.; López, N.; Camarero, J.; Marshall, L.G.; Lewis, L.H.; Bollero, A. Application of a novel flash-milling procedure for coercivity development in nanocrystalline MnAl permanent magnet powders. J. Phys. D Appl. Phys. 2017, 50, 105004. [Google Scholar] [CrossRef]
- Muñoz-Rodríguez, C.; Feng, L.; Palmero, E.M.; Mix, T.; Rial, J.; Olsson, F.; Skårman, B.; Vidarsson, H.; Larsson, P.O.; Woodcock, T.G.; et al. Fabrication of bulk τ MnAl–C magnets by hot-pressing from ε-phase gas-atomized and milled powder. J. Alloys Compd. 2020, 847, 156361. [Google Scholar] [CrossRef]
- Rial, J.; Švec, P.; Švec, P.; Bollero, A.; Deledda, S. Coercivity development in MnAl ribbons by microstructural modifications achieved through cold-rolling process. J. Magn. Magn. Mater. 2021, 529, 167826. [Google Scholar] [CrossRef]
- Saravanan, P.; Saju, S.; Vinod, V.T.P.; Černík, M. Structural and magnetic properties of rare-earth-free MnAl(MCNT)/Fe nanocomposite magnets processed by resin-bonding technique. J. Mater. Sci. Mater. Electron. 2020, 31, 9878–9887. [Google Scholar] [CrossRef]
- Rial, J.; Palmero, E.M.; Bollero, A. Efficient Nanostructuring of Isotropic Gas-Atomized MnAl Powder by Rapid Milling (30 s). Engineering 2019, 6, 173–177. [Google Scholar] [CrossRef]
- Bittner, F.; Freudenberger, J.; Schultz, L.; Woodcock, T.G. The impact of dislocations on coercivity in L10-MnAl. J. Alloys Compd. 2017, 704, 528–536. [Google Scholar] [CrossRef]
- Hernandez, J.S.T.; Maccari, F.; Marshall, L.G.; Tabares, J.A.; Alcázar, G.A.P. Exchange Coupling in MnAlC/α-Fe Nanocomposite Magnets. J. Supercond. Nov. Magn. 2018, 31, 3941–3947. [Google Scholar] [CrossRef]
- Koch, C.C.; Ovid’Ko, I.A.; Seal, S.; Veprek, S. Structural Nanocrystalline Materials: Fundamentals and Applications; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Krishnan, K.M. Fundamentals and Applications of Magnetic Materials. MRS Bull. 2017, 42, 540. [Google Scholar]
- Feng, L.; Nielsch, K.; Woodcock, T.G. Enhanced thermal stability of the τ-phase in MnAl-C alloys with Ni additions. J. Alloys Compd. 2021, 871, 159554. [Google Scholar] [CrossRef]
- Xiang, Z.; Deng, B.; Zhang, X.; Wang, X.; Cui, E.; Yu, L.; Song, Y.; Lu, W. Nanocrystalline MnAlV rare-earth-free Permanent Magnetic Alloys with Improved Magnetization and Thermal Stability. Intermetallics 2020, 116, 106638. [Google Scholar] [CrossRef]
- Tong, X.; Sharma, P.; Makino, A. Investigations on low energy product of MnAl magnets through recoil curves. J. Phys. D Appl. Phys. 2020, 53, 175001. [Google Scholar] [CrossRef]
- Si, P.-Z.; Choi, C.-J.; Park, J.; Ge, H.-L.; Du, J. Phase transformation and enhanced coercivity in B-N-doped MnAl nanocrystalline bulk alloys prepared by high pressure torsion. AIP Adv. 2020, 10, 015320. [Google Scholar] [CrossRef]
- Zhao, S.; Wu, Y.; Wang, J.; Jia, Y.; Zhang, T.; Zhang, T.; Jiang, C. Realization of large coercivity in MnAl permanent-magnet alloys by introducing nanoprecipitates. J. Magn. Magn. Mater. 2019, 483, 164–168. [Google Scholar] [CrossRef]
- Sato, S.; Irie, S. Metamagnetic behavior in L10-MnAl synthesized by the post annealing of electrodeposited MnAl powder. AIP Adv. 2019, 9, 035015. [Google Scholar] [CrossRef]
- Si, P.Z.; Park, J.; Qian, H.D.; Choi, C.J.; Li, Y.S.; Ge, H.L. Enhanced magnetic performance of bulk nanocrystalline MnAlC prepared by high pressure compaction of gas atomized powders. Bull. Mater. Sci. 2019, 42, 1–6. [Google Scholar] [CrossRef]
- Ohtani, T.; Kato, N.; Kojima, S.; Kojima, K.; Sakamoto, Y.; Konno, I.; Tsukahara, M.; Kubo, T. Magnetic properties of Mn-Al-C permanent magnet alloys. IEEE Trans. Magn. 1977, 13, 1328–1330. [Google Scholar] [CrossRef]
- Martínez Sánchez, H.; Zamora Alfonso, L.E.; Trujillo Hernandez, J.S.; Pérez Alcázar, G.A. Evidence of exchange coupling in τ-MnAlC/FeCo system. J. Magn. Magn. Mater. 2019, 473, 221–227. [Google Scholar] [CrossRef]
- Xiang, Z.; Wang, X.; Song, Y.; Yu, L.; Cui, E.; Deng, B.; Batalu, D.; Lu, W. Effect of cooling rates on the microstructure and magnetic properties of MnAl permanent magnetic alloys. J. Magn. Magn. Mater. 2019, 475, 479–483. [Google Scholar] [CrossRef]
- Shafeie, S.; Fang, H.; Hedlund, D.; Nyberg, A.; Svedlindh, P.; Gunnarsson, K.; Sahlberg, M. One step towards MnAl-based permanent magnets-Differences in magnetic, and microstructural properties from an intermediate annealing step during synthesis. J. Solid State Chem. 2019, 274, 229–236. [Google Scholar] [CrossRef]
- Zhao, S.; Wu, Y.; Zhang, C.; Wang, J.; Fu, Z.; Zhang, R.; Jiang, C. Stabilization of τ-phase in carbon-doped MnAl magnetic alloys. J. Alloys Compd. 2018, 755, 257–264. [Google Scholar] [CrossRef]
- You, J.; Guo, Y. Plasma enhanced atomic layer deposition of Co thin film on τ-MnAl for effective magnetic exchange coupling and enhanced energy products. J. Alloys Compd. 2018, 758, 116–121. [Google Scholar] [CrossRef]
- Tang Nguyen, V.; Calvayrac, F.; Bajorek, A.; Randrianantoandro, N. Mechanical alloying and theoretical studies of MnAl(C) magnets. J. Magn. Magn. Mater. 2018, 462, 96–104. [Google Scholar] [CrossRef]
- Si, P.Z.; Qian, H.D.; Choi, C.J.; Park, J.; Ge, H.L. A novel method for measuring the phase transformation temperature and enhanced coercivity in cold-rolled MnAlCx (x = 0–5) alloys. J. Magn. Magn. Mater. 2018, 451, 540–545. [Google Scholar] [CrossRef]
- Rial, J.; Švec, P.; Palmero, E.M.; Camarero, J.; Švec, P., Sr.; Bollero, A. Severe tuning of permanent magnet properties in gas-atomized MnAl powder by controlled nanostructuring and phase transformation. Acta Mater. 2018, 157, 42–52. [Google Scholar] [CrossRef]
- Qian, H.D.; Si, P.Z.; Choi, C.J.; Park, J.; Cho, K.M. Phase transformation and magnetic properties of MnAl powders prepared by elemental-doping and salt-assisted ball milling. AIP Adv. 2018, 8, 056216. [Google Scholar] [CrossRef]
- Parvin, M.S.; Oogane, M.; Kubota, M.; Tsunoda, M.; Ando, Y. Epitaxial L10-MnAl Thin Films with High Perpendicular Magnetic Anisotropy and Small Surface Roughness. IEEE Trans. Magn. 2018, 54, 1–4. [Google Scholar] [CrossRef]
- Palmero, E.M.; Rial, J.; de Vicente, J.; Camarero, J.; Skårman, B.; Vidarsson, H.; Larsson, P.O.; Bollero, A. Development of permanent magnet MnAlC/polymer composites and flexible filament for bonding and 3D-printing technologies. Sci. Technol. Adv. Mater. 2018, 19, 465–473. [Google Scholar] [CrossRef]
- Jia, Y.; Wu, Y.; Zhao, S.; Wang, J.; Jiang, C. Relation between solidification microstructure and coercivity in MnAl permanent-magnet alloys. Intermetallics 2018, 96, 41–48. [Google Scholar] [CrossRef]
- Fang, H.; Cedervall, J.; Hedlund, D.; Shafeie, S.; Deledda, S.; Olsson, F.; Von Fieandt, L.; Bednarcik, J.; Svedlindh, P.; Gunnarsson, K.; et al. Structural, microstructural and magnetic evolution in cryo milled carbon doped MnAl. Sci. Rep. 2018, 8, 2525. [Google Scholar] [CrossRef]
- Crisan, A.D.; Vasiliu, F.; Nicula, R.; Bartha, C.; Mercioniu, I.; Crisan, O. Thermodynamic, structural and magnetic studies of phase transformations in MnAl nanocomposite alloys. Mater. Charact. 2018, 140, 1–8. [Google Scholar] [CrossRef]
- Øygarden, V.; Rial, J.; Bollero, A.; Deledda, S. Phase-pure τ-MnAlC produced by mechanical alloying and a one-step annealing route. J. Alloys Compd. 2019, 779, 776–783. [Google Scholar] [CrossRef]
- Si, P.; Qian, H.; Wang, X.; Yang, Y.; Park, J.; Ge, H.; Choi, C.-J. High-Pressure Synthesis of High Coercivity Bulk MnAl-C Magnets from Melt-Spun Ribbons. J. Electron. Mater. 2019, 48, 794–798. [Google Scholar] [CrossRef]
- Shao, Z.; Zhao, H.; Zeng, J.; Zhang, Y.; Yang, W.; Lai, Y.; Guo, S.; Du, H.; Wang, C.; Yang, Y.; et al. One step preparation of pure τ-MnAl phase with high magnetization using strip casting method. AIP Adv. 2017, 7, 056213. [Google Scholar] [CrossRef]
- Dang, R.; Ma, X.; Liu, J.; Zhang, Y.; Qian, S. The fabrication and characterization of MnAl/FeCo composite thin films with enhanced maximum energy products. Mater. Lett. 2017, 197, 8–11. [Google Scholar] [CrossRef]
- Si, P.-Z.; Qian, H.-D.; Choi, C.-J.; Park, J.; Han, S.; Ge, H.-L.; Shinde, K.P. In situ Observation of Phase Transformation in MnAl(C) Magnetic Materials. Materials 2017, 10, 1016. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Su, K.; Cheng, Y.; Ramanujan, R. Structure and Properties Evolutions for Hard Magnetic MnAl and MnGa Based Alloys Prepared by Melt Spinning or Mechanical Milling. Mater. Sci. Eng. Adv. Res. 2015, 1, 12–19. [Google Scholar] [CrossRef]
- Shen, J.; Li, J.; Ren, S. Metal-redox for MnAl-Based ternary magnetic nanocrystals. RSC Adv. 2016, 6, 41781–41784. [Google Scholar] [CrossRef]
- Sato, T.; Ohsuna, T.; Kaneko, Y. Enhanced saturation magnetization in perpendicular L10-MnAl films upon low substitution of Mn by 3d transition metals. J. Appl. Phys. 2016, 120, 243903. [Google Scholar] [CrossRef]
- Marshall, L.G.; McDonald, I.J.; Lewis, L.H. Quantification of the strain-induced promotion of τ-MnAl via cryogenic milling. J. Magn. Magn. Mater. 2016, 404, 215–220. [Google Scholar] [CrossRef]
- Fang, H.; Kontos, S.; Ångström, J.; Cedervall, J.; Svedlindh, P.; Gunnarsson, K.; Sahlberg, M. Directly obtained τ-phase MnAl, a high performance magnetic material for permanent magnets. J. Solid State Chem. 2016, 237, 300–306. [Google Scholar] [CrossRef]
- Singh, N.; Mudgil, V.; Anand, K.; Srivastava, A.K.; Kotnala, R.K.; Dhar, A. Influence of processing on structure property correlations in τ-MnAl rare-earth free permanent magnet material. J. Alloys Compd. 2015, 633, 401–407. [Google Scholar] [CrossRef]
- Bittner, F.; Schultz, L.; Woodcock, T.G. Twin-like defects in L10 ordered τ-MnAl-C studied by EBSD. Acta Mater. 2015, 101, 48–54. [Google Scholar] [CrossRef]
- Chaturvedi, A.; Yaqub, R.; Baker, I. A comparison of τ-MnAl particulates produced via different routes. J. Phys. Condens. Matter 2014, 26, 064201. [Google Scholar] [CrossRef] [PubMed]
- Nie, S.H.; Zhu, L.J.; Lu, J.; Pan, D.; Wang, H.L.; Yu, X.Z.; Xiao, J.X.; Zhao, J.H. Perpendicularly magnetized τ-MnAl(001) thin films epitaxied on GaAs. Appl. Phys. Lett. 2013, 102, 152405. [Google Scholar] [CrossRef]
- Park, J.H.; Hong, Y.K.; Bae, S.; Lee, J.J.; Jalli, J.; Abo, G.S.; Neveu, N.; Kim, S.G.; Choi, C.J.; Lee, J.G. Saturation magnetization and crystalline anisotropy calculations for MnAl permanent magnet. J. Appl. Phys. 2010, 107, 09A731. [Google Scholar] [CrossRef]
- Fischer, G.A.; Rudee, M.L. Effect of magnetic annealing on the τ-phase of MnAl thin films. J. Magn. Magn. Mater. 2000, 213, 335–339. [Google Scholar] [CrossRef]
- Crew, D.C.; McCormick, P.G.; Street, R. MnAl and MnAlC permanent magnets produced by mechanical alloying. Scr. Metall. Mater. 1995, 32, 315–318. [Google Scholar] [CrossRef]
- Yang, Y.C.; Ho, W.W.; Lin, C.; Yang, J.L.; Zhou, H.M.; Zhu, J.X.; Zeng, X.X.; Zhang, B.S.; Jin, L. Neutron diffraction study of hard magnetic alloy MnAlC. J. Appl. Phys. 1984, 55, 2053–2054. [Google Scholar] [CrossRef]
- Houseman, E.L.; Jakubovics, J.P. Domain structure and magnetization processes in MnAl and MnAlC alloys. J. Magn. Magn. Mater. 1983, 31–34 Pt 2, 1005–1006. [Google Scholar] [CrossRef]
- Willens, R.H. Melt extracted MnAl and MnAlC. IEEE Trans. Magn. 1980, 16, 1059–1061. [Google Scholar] [CrossRef]
- Saravanan, P.; Vinod, V.T.P.; Černík, M.; Selvapriya, A.; Chakravarty, D.; Kamat, S.V. Processing of Mn–Al nanostructured magnets by spark plasma sintering and subsequent rapid thermal annealing. J. Magn. Magn. Mater. 2015, 374, 427–432. [Google Scholar] [CrossRef]
- Jian, H.; Skokov, K.P.; Gutfleisch, O. Microstructure and magnetic properties of Mn–Al–C alloy powders prepared by ball milling. J. Alloys Compd. 2015, 622, 524–528. [Google Scholar] [CrossRef]
- Pasko, A.; LoBue, M.; Fazakas, E.; Varga, L.K.; Mazaleyrat, F. Spark plasma sintering of Mn–Al–C hard magnets. J. Phys. Condens. Matter 2014, 26, 064203. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.-G.; Wang, X.-L.; Zhang, Z.-D.; Choi, C.-J. Effect of mechanical milling and heat treatment on the structure and magnetic properties of gas atomized Mn–Al alloy powders. Thin Solid Film. 2011, 519, 8312–8316. [Google Scholar] [CrossRef]
- Lee, J.-G.; Li, P.; Choi, C.-J.; Dong, X.-L. Synthesis of Mn–Al alloy nanoparticles by plasma arc discharge. Thin Solid Film. 2010, 519, 81–85. [Google Scholar] [CrossRef]
- Zeng, Q.; Baker, I.; Cui, J.B.; Yan, Z.C. Structural and magnetic properties of nanostructured Mn–Al–C magnetic materials. J. Magn. Magn. Mater. 2007, 308, 214–226. [Google Scholar] [CrossRef]
- Fazakas, E.; Varga, L.K.; Mazaleyrat, F. Preparation of nanocrystalline Mn–Al–C magnets by melt spinning and subsequent heat treatments. J. Alloys Compd. 2007, 434–435, 611–613. [Google Scholar] [CrossRef]
- Zeng, Q.; Baker, I.; Yan, Z.-C. Nanostructured Mn–Al permanent magnets produced by mechanical milling. J. Appl. Phys. 2006, 99, 08E902. [Google Scholar] [CrossRef]
- Saito, T. Magnetic properties of Mn–Al–C alloy powders produced by mechanical grinding. J. Appl. Phys. 2005, 97, 10F304. [Google Scholar] [CrossRef]
- Saito, T. Magnetic properties of Mn–Al system alloys produced by mechanical alloying. J. Appl. Phys. 2003, 93, 8686–8688. [Google Scholar] [CrossRef]
- Madugundo, R.; Koylu-Alkan, O.; Hadjipanayis, G.C. Bulk Mn-Al-C permanent magnets prepared by various techniques. AIP Adv. 2016, 6, 056009. [Google Scholar] [CrossRef]
- Tyrman, M.; Ahmim, S.; Pasko, A.; Etgens, V.; Mazaleyrat, F.; Quetel-Weben, S.; Perrière, L.; Guillot, I. Anisotropy of the ferromagnetic L10 phase in the Mn-Al-C alloys induced by high-pressure spark plasma sintering. AIP Adv. 2018, 8, 056217. [Google Scholar] [CrossRef]
- Dehghan, H.; Ebrahimi, S.A. Effect of hot deformation conditions on magnetic properties of rare earth free magnetic Mn-Al-C alloy. J. Magn. Magn. Mater. 2019, 477, 55–61. [Google Scholar] [CrossRef]
- Volegov, A.S.; Müller, K.H.; Bittner, F.; Mix, T.; Neznakhin, D.S.; Volegova, E.A.; Nenkov, K.; Schultz, L.; Woodcock, T.G. Magnetic viscosity of L10 structured Mn-Ga and Mn-Al alloys. J. Magn. Magn. Mater. 2017, 441, 750–756. [Google Scholar] [CrossRef]
- Saravanan, P.; Hsu, J.-H.; Vinod, V.T.P.; Černík, M.; Kamat, S.V. MWCNT reinforced τ-Mn-Al nanocomposite magnets through spark plasma sintering. J. Alloys Compd. 2017, 695, 364–371. [Google Scholar] [CrossRef]
- Kobayashi, R.; Mitsui, Y.; Umetsu, R.; Takahashi, K.; Mizuguchi, M.; Koyama, K. Magnetic-Field-Induced Acceleration of Phase Formation in τ-Mn-Al. Mater. Trans. 2017, 58, 1511–1518. [Google Scholar] [CrossRef]
- Madugundo, R.; Hadjipanayis, G.C. Anisotropic Mn-Al-(C) hot-deformed bulk magnets. J. Appl. Phys. 2016, 119, 013904. [Google Scholar] [CrossRef]
- Singh, N.; Shyam, R.; Upadhyay, N.K.; Dhar, A. Development of rare-earth free Mn-Al permanent magnet employing powder metallurgy route. IOP Conf. Ser. Mater. Sci. Eng. 2012, 73, 012042. [Google Scholar] [CrossRef]
- Saravanan, P.; Vinod, V.T.P.; Černík, M.; Chakravarty, D.; Ghosal, P.; Kamat, S.V. Exchange coupled rare-earth free Mn-Al/Fe nanocomposite magnets by spark plasma sintering. Mater. Lett. 2014, 137, 369–372. [Google Scholar] [CrossRef]
- Lucis, M.J.; Prost, T.E.; Jiang, X.; Wang, M.; Shield, J.E. Phase Transitions in Mechanically Milled Mn-Al-C Permanent Magnets. Metals 2014, 4, 130–140. [Google Scholar] [CrossRef]
- Pasko, A.; Mazaleyrat, F.; Lobue, M.; Fazakas, E.; Varga, L.K. Hard magnetic properties of melt-spun Mn-Al-C alloys. EPJ Web Conf. 2013, 40, 06008. [Google Scholar] [CrossRef]
- Obi, O.; Burns, L.; Chen, Y.; Bennett, S.; Sawicki, M.; Kaplan, D.; Arango, A.M.; Lewis, L.H.; Harris, V.G. Effect of ambient aging on heat-treated mechanically alloyed Mn-Al-C powders. IEEE Trans. Magn. 2013, 49, 3372–3377. [Google Scholar] [CrossRef]
- Kohmoto, O.; Kageyama, N.; Kageyama, Y.; Haji, H.; Uchida, M.; Matsushima, Y. Magnetic properties of mechanically alloyed Mn-Al-C powders. J. Phys. Conf. Ser. 2011, 266, 012016. [Google Scholar] [CrossRef]
- Kinemuchi, Y.; Fujita, A.; Ozaki, K. High-pressure synthesis of L10 MnAl with near-stoichiometric composition. Dalton Trans. 2016, 45, 10936–10941. [Google Scholar] [CrossRef]
- Moon, K.-W.; Lee, G.-T.; Jeon, K.-W.; Kim, J. Synthesis and Magnetic Properties of τ-MnAlC Powders. J. Nanosci. Nanotechnol. 2015, 15, 8735–8738. [Google Scholar] [CrossRef]
- Obi, O.; Burns, L.; Chen, Y.; Fitchorov, T.; Kim, S.; Hsu, K.; Heiman, D.; Lewis, L.H.; Harris, V.G. Magnetic and structural properties of heat-treated high-moment mechanically alloyed MnAlC powders. J. Alloys Compd. 2014, 582, 598–602. [Google Scholar] [CrossRef]
- Liu, Z.; Chen, C.; Zheng, Z.; Tan, B.; Ramanujan, R. Phase transitions and hard magnetic properties for rapidly solidified MnAl alloys doped with C, B, and rare earth elements. J. Mater. Sci. 2012, 47, 2333–2338. [Google Scholar] [CrossRef]
- Huang, J.H.; Kuo, P.C. Sintered MnAl and MnAlC magnets. Mater. Sci. Eng. B 1992, 14, 75–79. [Google Scholar] [CrossRef]
- Nagata, Y.; Sano, H.; Ohta, K. Hard Magnetic MnAl and MnBi Ribbons Made from Molten State by Rapid Cooling. Jpn. J. Appl. Phys. 1984, 23, 580–584. [Google Scholar] [CrossRef]
- Skomski, R.; Manchanda, P.; Kumar, P.K.; Balamurugan, B.; Kashyap, A.; Sellmyer, D.J. Predicting the future of permanent-magnet materials. IEEE Trans. Magn. 2013, 49, 3215–3220. [Google Scholar] [CrossRef]
- Hirian, R.; Dudric, R.; Isnard, O.; Barbu-Tudoran, L.; Pop, V. Influence of heat treatment, near the temperature region of Fe α-γ transformation, on the interphase exchange coupling of Nd2Fe14B + Fe nanocomposites. J. Magn. Magn. Mater. 2021, 520, 166960. [Google Scholar] [CrossRef]
- Neupane, D.; Ghimire, M.; Adhikari, H.; Lisfi, A.; Mishra, S.R. Synthesis and magnetic study of magnetically hard-soft SrFe12-yAlyO19-xWt.% Ni0.5Zn0.5Fe2O4 nanocomposites. AIP Adv. 2017, 7, 055602. [Google Scholar] [CrossRef]
- Dahal, J.; Neupane, D.; Mishra, S. Exchange-Coupling Behavior in SrFe12O19/La0.7Sr0.3MnO3 Nanocomposites. Ceramics 2019, 2, 10. [Google Scholar] [CrossRef]
- Gao, R.W.; Zhang, D.H.; Li, W.; Li, X.M.; Zhang, J.C. Hard magnetic property and δM(H) plot for sintered NdFeB magnet. J. Magn. Magn. Mater. 2000, 208, 239–243. [Google Scholar] [CrossRef]
- Attyabi, S.N.; Radmanesh, S.M.A.; Ebrahimi, S.A.S.; Dehghan, H. The Synthesis and Characterization of Hard-Soft Mn52Al45.7C2.3-α-Fe Nanocomposite Magnets. J. Supercond. Nov. Magn. 2022, 35, 1229–1240. [Google Scholar] [CrossRef]
- Ma, C. Magnetic properties of exchange coupled SmCo5/FeCo composite particles synthesized by magnetic self-assembly. Chem. Phys. Lett. 2018, 696, 31–35. [Google Scholar] [CrossRef]
- Coey, J.M.D. Perspectives in permanent magnetism. J. Magn. Magn. Mater. 1995, 140–144 Pt 2, 1041–1044. [Google Scholar] [CrossRef]
- Kang, K.; Lewis, L.H. Recoil hysteresis of Sm-Co/Fe exchange-spring bilayers. J. Appl. Phys. 2005, 98, 113906. [Google Scholar] [CrossRef]
- Zheng, B.; Zhang, H.-W.; Zhao, S.-F.; Chen, J.-L.; Wu, G.-H. The physical origin of open recoil loops in nanocrystalline permanent magnets. Appl. Phys. Lett. 2008, 93, 182503. [Google Scholar] [CrossRef]
- Rong, C.-b.; Liu, J.P. Grain boundary contribution to recoil loop openness of exchange-coupled nanocrystalline magnets. Appl. Phys. Lett. 2009, 94, 172510. [Google Scholar] [CrossRef]
- Choi, Y.; Jiang, J.S.; Pearson, J.E.; Bader, S.D.; Liu, J.P. Origin of recoil hysteresis loops in Sm-Co/Fe exchange-spring magnets. Appl. Phys. Lett. 2007, 91, 022502. [Google Scholar] [CrossRef]
- Wohlfarth, E.P. Relations between Different Modes of Acquisition of the Remanent Magnetization of Ferromagnetic Particles. J. Appl. Phys. 1958, 29, 595–596. [Google Scholar] [CrossRef]
- Zeng, W.; Yang, H.; Wu, Q.; Pan, M.; Yu, N.; Gu, Y.; Ge, H. Effect of the Ga Content on the Magnetic Properties and Microstructure of the Nanocrystalline Ce-Fe-B Alloys. J. Supercond. Nov. Magn. 2021, 34, 1225–1229. [Google Scholar] [CrossRef]
- Birčáková, Z.; Kollár, P.; Jakubčin, M.; Füzer, J.; Bureš, R.; Fáberová, M. Reversible and irreversible magnetization processes along DC hysteresis loops of Fe-based composite materials. J. Magn. Magn. Mater. 2019, 483, 183–190. [Google Scholar] [CrossRef]
- Miyaura, A.; Kawaguchi, T.; Hagiwara, M.; Fujihara, S. Controlled 90° domain wall motion in BaTiO3 piezoelectric ceramics modified with acceptor ions localized near grain boundaries. SN Appl. Sci. 2019, 1, 286. [Google Scholar] [CrossRef]
- Liu, L.; Liu, Z.; Zhang, X.; Feng, Y.; Wang, C.; Sun, Y.; Lee, D.; Yan, A.; Wu, Q. Magnetization reversal process in (Sm, Dy, Gd) (Co, Fe, Cu, Zr)z magnets with different cellular structures. AIP Adv. 2017, 7, 056221. [Google Scholar] [CrossRef]
- Ilse, S.E.; Groß, F.; Schütz, G.; Gräfe, J.; Goering, E. Understanding the interaction of soft and hard magnetic components in NdFeB with first-order reversal curves. Phys. Rev. B 2021, 103, 024425. [Google Scholar] [CrossRef]
- Dobrotă, C.-I.; Stancu, A. What does a first-order reversal curve diagram really mean? A study case: Array of ferromagnetic nanowires. J. Appl. Phys. 2013, 113, 043928. [Google Scholar] [CrossRef]
- Groß, F.; Ilse, S.E.; Schütz, G.; Gräfe, J.; Goering, E. Interpreting first-order reversal curves beyond the Preisach model: An experimental permalloy microarray investigation. Phys. Rev. B 2019, 99, 064401. [Google Scholar] [CrossRef]
- Pike, C.R.; Roberts, A.P.; Verosub, K.L. Characterizing interactions in fine magnetic particle systems using first order reversal curves. J. Appl. Phys. 1999, 85, 6660–6667. [Google Scholar] [CrossRef]
- Pike, C.R.; Roberts, A.P.; Dekkers, M.J.; Verosub, K.L. An investigation of multi-domain hysteresis mechanisms using FORC diagrams. Phys. Earth Planet. Inter. 2001, 126, 11–25. [Google Scholar] [CrossRef]
- Pike, C.R. First-order reversal-curve diagrams and reversible magnetization. Phys. Rev. B 2003, 68, 104424. [Google Scholar] [CrossRef]
- Egli, R.; Winklhofer, M. Recent Developments on Processing and Interpretation Aspects of First-Order Reversal Curves (FORC). Cepия Ecтественные науки 2014, 156, 14–53. [Google Scholar]
- Chen, A.; Egli, R.; Moskowitz, B. First-order reversal curve (FORC) diagrams of natural and cultured biogenic magnetic particles. J. Geophys. Res. 2007, 112, B08S90. [Google Scholar] [CrossRef]
- Roy, D.; Anil Kumar, P.S. Exchange spring behaviour in SrFe12O19-CoFe2O4 nanocomposites. AIP Adv. 2015, 5, 077137. [Google Scholar] [CrossRef]
- Panagiotopoulos, I. A simple approach to the First Order Reversal Curves (FORC) of two-phase magnetic systems. J. Magn. Magn. Mater. 2011, 323, 2148–2153. [Google Scholar] [CrossRef]
- Muxworthy, A.R.; Roberts, A.P. First-Order Reversal Curve (FORC) Diagrams. In Encyclopedia of Geomagnetism and Paleomagnetism; Gubbins, D., Herrero-Bervera, E., Eds.; Springer: Dordrecht, The Netherlands, 2007; pp. 266–272. [Google Scholar]
- Roberts, A.P.; Pike, C.R.; Verosub, K.L. First-order reversal curve diagrams: A new tool for characterizing the magnetic properties of natural samples. J. Geophys. Res. Solid Earth 2000, 105, 28461–28475. [Google Scholar] [CrossRef]
- Volodchenkov, A.D.; Kodera, Y.; Garay, J.E. Synthesis of strontium ferrite/iron oxide exchange coupled nano-powders with improved energy product for rare earth free permanent magnet applications. J. Mater. Chem. C 2016, 4, 5593–5601. [Google Scholar] [CrossRef]
- Egli, R. Theoretical aspects of dipolar interactions and their appearance in first-order reversal curves of thermally activated single-domain particles. J. Geophys. Res. 2006, 111, B12S17. [Google Scholar] [CrossRef]
- Oroujizad, S.; Almasi-Kashi, M.; Alikhanzadeh-Arani, S. A FORC investigation into the effect of Cu additive on magnetic characteristics of Co-Ni alloy nanoparticles. J. Magn. Magn. Mater. 2019, 473, 169–175. [Google Scholar] [CrossRef]
- Kafrouni, L.; Savadogo, O. Recent progress on magnetic nanoparticles for magnetic hyperthermia. Prog. Biomater. 2016, 5, 147–160. [Google Scholar] [CrossRef]
- Butler, R.F.; Banerjee, S.K. Theoretical single-domain grain size range in magnetite and titanomagnetite. J. Geophys. Res. 1975, 80, 4049–4058. [Google Scholar] [CrossRef]

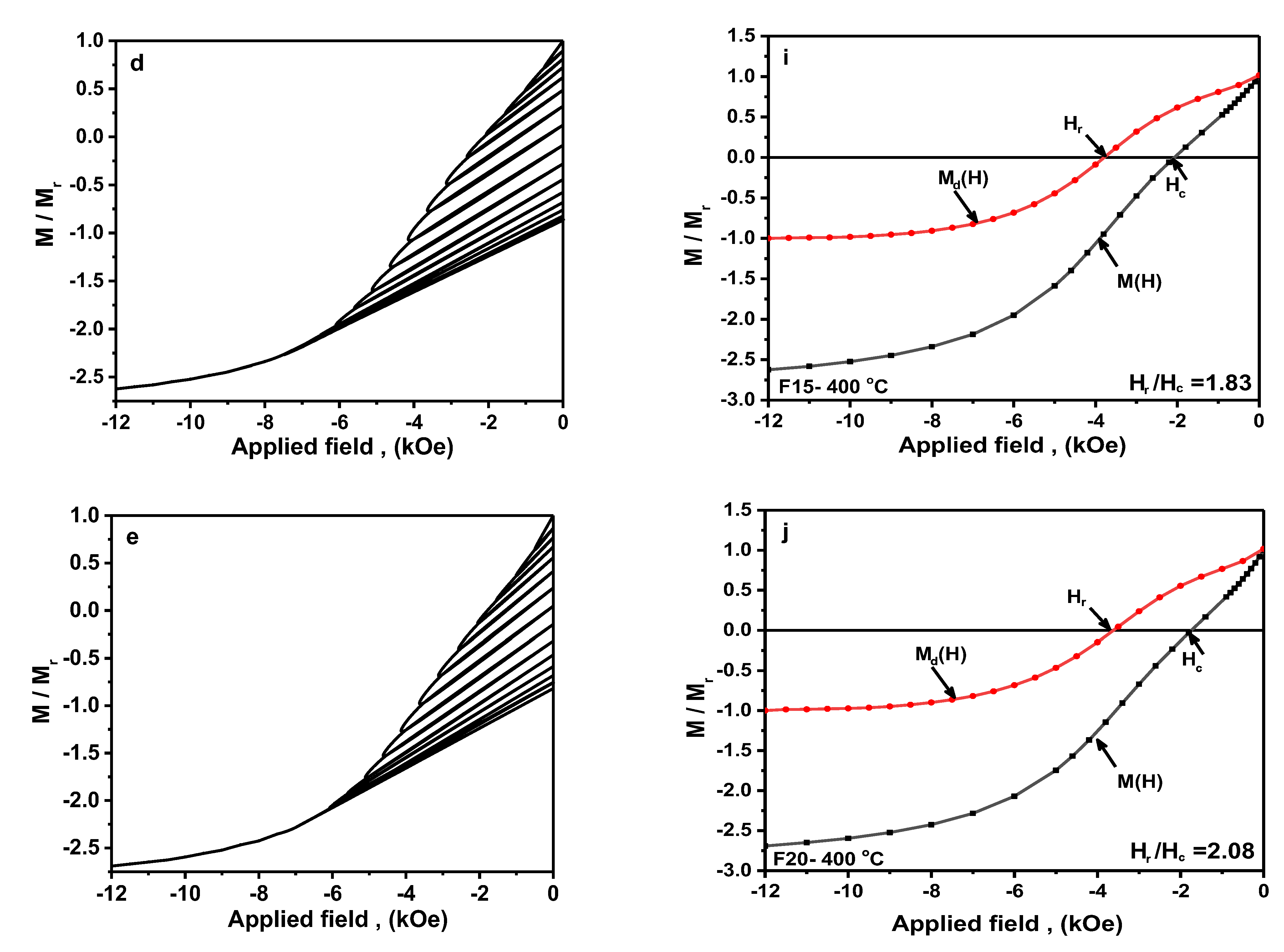
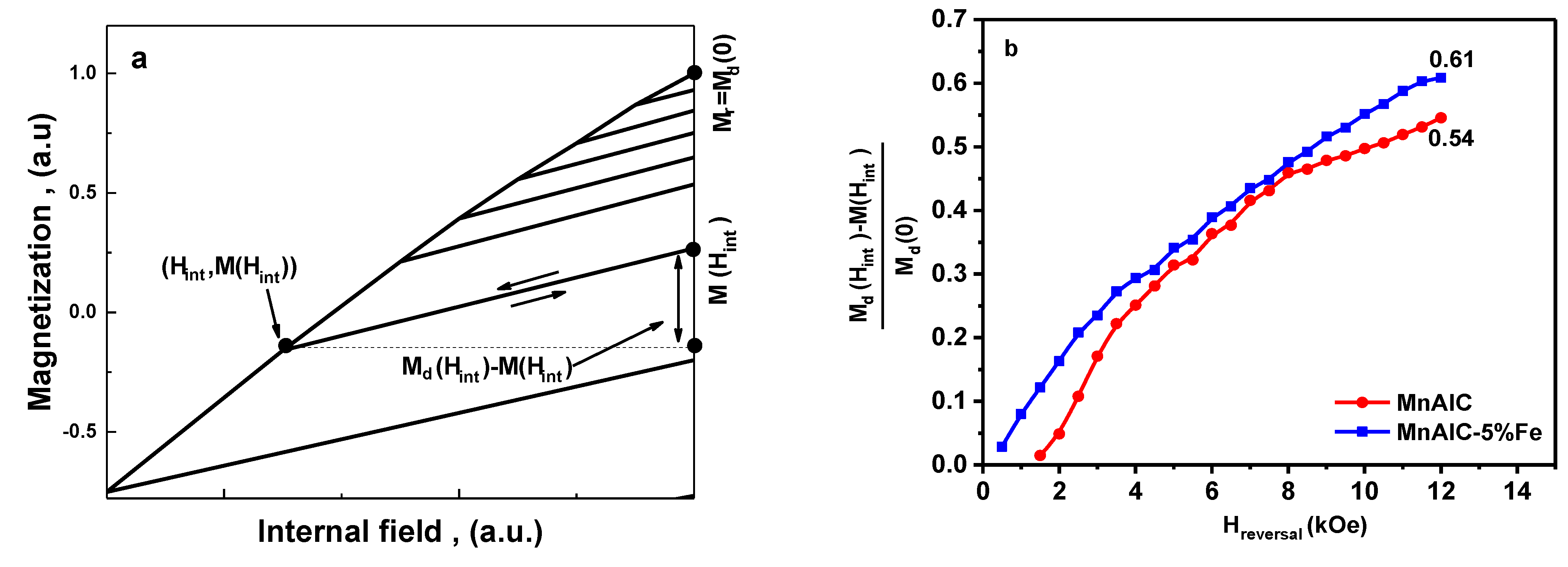
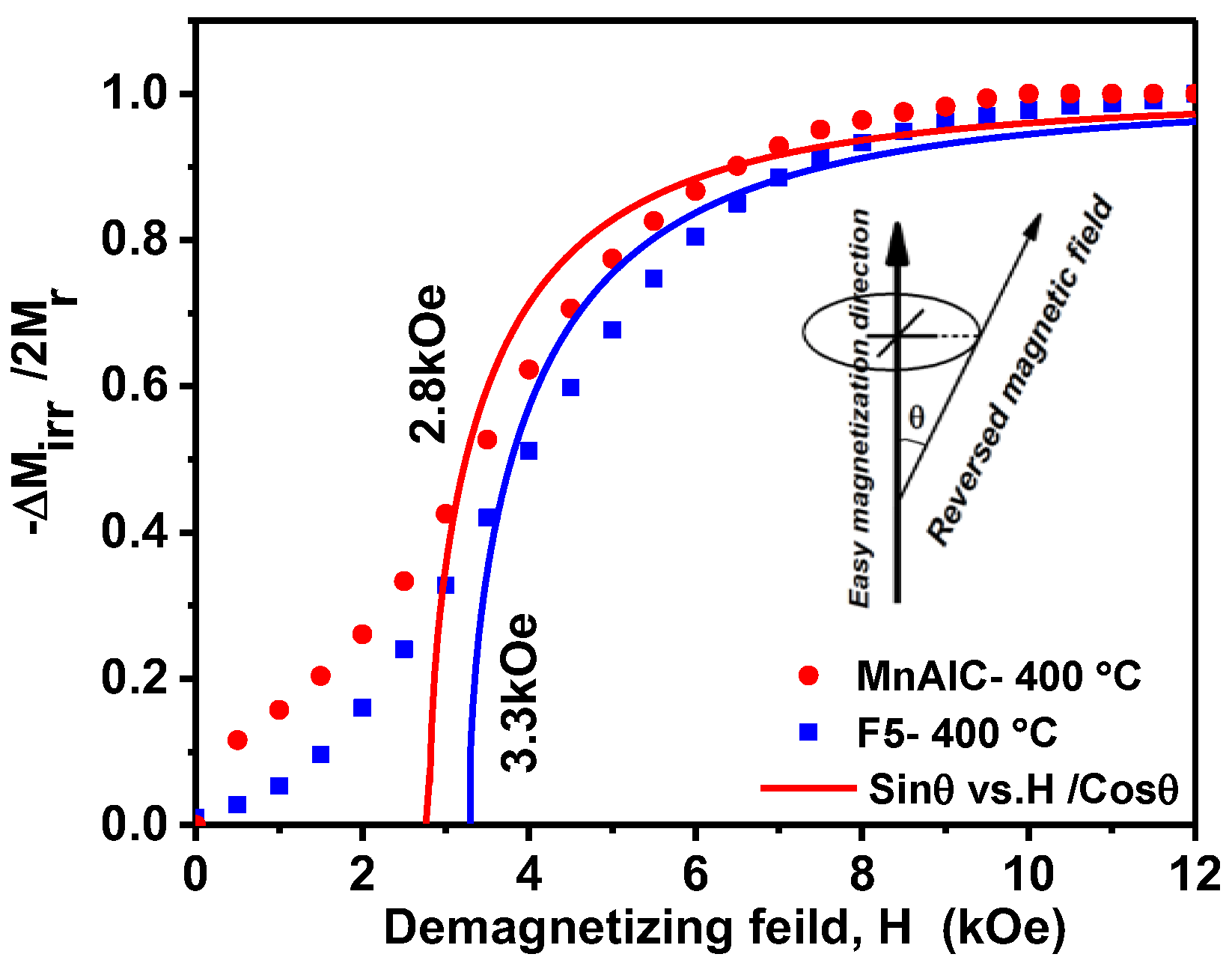

| Sample | Composition |
|---|---|
| F0 | Mn-Al-C |
| F5 | Mn-Al-C-0.05α-Fe |
| F10 | Mn-Al-C-0.1α-Fe |
| F15 | Mn-Al-C-0.15α-Fe |
| F20 | Mn-Al-C-0.2α-Fe |
| Composition | Ms (emu/g) | Mr (emu/g) | Hc (kOe) | (BH)max (MGOe) | Method | Ref. |
|---|---|---|---|---|---|---|
| Mn52Al45.7C2.3 + 5 wt.% Fe | 76 | 46 | 3.3 | 1.6 | VIM + HEBM + mix HEBM + HT | This work |
| Mn55Al45 | 75–65 | 35 | 3.5 | PFC + CR | [21] | |
| Mn53Al45C2 | 58 | ~1.9 * | AM and IM + HD | [28] | ||
| Mn53.35Al43.65V3 | 75.8 | 35 | 2.13 | AM + SRMS + HT | [29] | |
| Mn54Al46 | 125 | ~35 | 2.04 | 1.42 | IM + RMS + HT + BM + SPS | [30] |
| B-N-doped MnAl | 79 | 40 | ~3.9 * | IM + HPT | [31] | |
| (Mn0.54Al0.46) 99.8Tb0.2 | ~31 | ~14 | 5.43 | AM + MSHT + HEBM | [32] | |
| 79.7 | 19 | 12.3 | ED + HT | [33] | ||
| Mn54Al46 | 0–55 | 25–5 | 3–4.2 | GA + SABM | [23] | |
| Mn:Al:C = 54:46:2.44 | ~91 | ~39 | ~2.8–3.4 * | IM + HT + P | [34] | |
| 70.01 wt. %Mn, 29.48 wt. %Al and 0.51 wt. %C | 93 | 2.7 | 7 | IM + E+HT | [35] | |
| Mn53.3Al45C1.7/FeCo (95/5 wt. %) | 76.43 | 32.71 | ~2.77 * | 0.7 | MS and AM + BM + P and S | [36] |
| Mn55Al45 | 118.2 | ~45 | 1.5 | IM + SRMS | [37] | |
| Mn55Al45C2 | 108 | 20 | 0.7 | IM + A | [38] | |
| (Mn0.54Al0.46) 100-xCx, x = 3 | 86.7 | ~35 | 3.26 | AM + HEBM | [39] | |
| MnAl/Co-2 | 52 | 43 | 2.752 | 3.38 | [40] | |
| Mn54.2Al43.8C2 | 111.78 | 50 | 1.8 | 7.8 | HEBM + A+aged | [41] |
| Mn54Al46Cx, x = 2 | ~125 | ~42 | ~2.3 * | IM + CR | [42] | |
| Mn54Al46 | ~55 | ~28 | ~2.5 | GA + SABM + A | [43] | |
| Mn54Al46 (MnAlSi0.5) | 70.3 | 33 | ~4.5 * | IM + SAHEBM | [44] | |
| Mn48Al52 | 96 | ~77 | ~3 | MAS + A | [45] | |
| (Mn57Al43) 100C1.19 | 80 | 36.70 | 1.47 | GA + mixpolymer + E | [46] | |
| (Mn0·54Al0.46) 98C2 | 80 | ~46 | 1.47 | VAM + MS | [47] | |
| Mn54.3Al44C1.7 | 88 | ~37 | 1.6 | 0.66 | AM + cryomilled HEBM+ | [25] |
| Mn1.1Al0.9C0.02 | 99 | 29 | 1.6 | Cryomilled + Flash heat | [48] | |
| Mn55Al45 | ~71 | ~14 | 2 | AM + MS | [49] | |
| Mn0.55Al0.45C0.02 | 85 | ~43 | ~1.5 * | 0.78 | MA + A | [50] |
| Mn54Al46C2.44 | ~60 | ~28 | ~2.9 * | IM + MS + A + P | [51] | |
| MnAlC (70.03 wt.% Mn, 29.28 wt.% Al and 0.69 wt.% C) | 95 | ~40 | ~2.2 * | SSR + A + HT + HP | [14] | |
| Mn56Al44 | 63.9 | ~24 | 2.8 | IM + SC + G | [52] | |
| Mn54Al46 | ~10–43 | ~5–25 | 3–4 | GA + SABM + A | [19] | |
| Mn54Al46 | 22.6 | 12.9 | 4.9 | GA + MM + A | [18] | |
| MnAl thin film/FeCO (8 nm) | ~90 | ~81 | ~3.4 | 4.7 | DC MAS + Post A | [53] |
| Mn53Al45C2 | ~87 | 72 | ~2.85 * | 3.0 | IM + CW + R | [24] |
| Mn54Al46 | 105 | ~20 | ~1.2 * | high-frequency VIM + A | [54] | |
| Mn51Al46C3 | ~44 | 32 | 22.83 | AM + SABM + A | [55] | |
| MnAlCo | 15.1 | ~5 | 0.85 | [56] | ||
| (Mn, Fe) Al | 77 | ~54 | 3.8 | 2.0 | UHV-MAS + A | [57] |
| Mn54Al46 | 50.8 | 24 | ~2.8 * | AM + MS + cryomilled HEBM | [58] | |
| Mn57Al43 | ~62 | ~35 | 5.3 | AM + MS + A+BM | [15] | |
| (Mn0.55Al0.45) 100C2 | 83 | 34 | 2.8 | IM + G + HEBM | [59] | |
| Mn54Al46 | ~29 | 16 | 1.8 | AM + HEBM + A | [60] | |
| Mn53Al45C2 | 98 | 72 | ~1.6 * | IM + HE | [61] | |
| Mn54Al46 | 108 | 73 | ~3.7 | 3.1 | AM + SRMS + A | [16] |
| Mn54Al46 | 39.8 | ~23 | 4.2 | GA + ε-phase + MM + ECAE | [17] | |
| Mn54Al46 | 60 | ~32 | 3.62 | GA + MM + HT | [62] | |
| 70 | ~62 | ~8.1 | 4.44 | MBES + A | [63] | |
| Mn54Al46 + 10 wt%Fe | ~16 | ~6 | ~3.3 * | IM + mix + HSV BM + A | [14] | |
| 98.3 | 4.7 | M + Q(ε-phase) + A | [64] | |||
| Mn68.8Al29.98Ni0.78C0.44 | 48 | ~35 | 1.5 | DC MAS | [65] | |
| 70.7Mn 28.2Al 1.1 C | 85 | 39 | 3.4 | 1.29 | MA(HEBM) + CC + HT | [66] |
| Mn1.074 Al0.871 C0.055 | 84 | 2.8 | 5.5 | WE | [67] | |
| 71.5 Mn, 27.9Al, 0.6 C | 73 | 0.32 | 1.32 | Alloy + Q + HT | [68] | |
| Mn55Al45 | 83 | 49 | 2.14 | 1.6 | M + rapid Q(ε-phase) + HT | [69] |
| Mn (56at. %) Al (44at. %) | 28 | ~12 | 2.44 | MA + SPS + Rapid thermal A | [70] | |
| Mn53.3Al45C1.7 | ~80 | 54.8 | ~2.8 | ~2 | AM + HEBM + A | [71] |
| Mn54Al44C2 | 84 | 48 | ~1.8 | IM + MS + M + SPS | [72] | |
| Mn–30 wt.% Al | ~60 | 41.2 | 3.1 | 1.59 | IM + GA(ε-phase) + BM + HT | [73] |
| (Mn20Al80) 0.95C0.05 | 8.8 | 5.6 | MA + PAD + HT | [74] | ||
| Mn54Al46 | 89 | 45 | 4.8 | AM + HEBM + A | [75] | |
| Mn54Al44C2 | 92 | 52 | 1.7 | 1.5 | VIM + SRMS | [76] |
| Mn54Al46 | 87 | ~38 | 4.8 | AM + HEBM + A | [77] | |
| Mn-29%Al-0.5%C-0.5%Ni | 60 | 1.95 | 1.43 | AM + HEBM + HT | [78] | |
| ~40 | 24 | 2.3 | MA(HEBM) + HT | [79] | ||
| Mn53.5Al44.5C2 | 82 | 50 | 2.2 | 1.8 | AM + HEBM + HT + HD | [80] |
| Mn54Al44C2 | 86 | 41 | ~1.7 * | M + MS + cryogenic MM + SPS | [81] | |
| ~75 | 32 | 2 | IM + HD | [82] | ||
| Mn54Al46 | ~75 | ~41 | ~3.7 * | AM + VibrationM + post A | [83] | |
| Mn55Al44 + 2.0 wt.% MWCNT | 82.3 | 45.6 | 3.64 | 2.26 | AM + mix + HEBM + SPS | [84] |
| Al-55at.%Mn | 72.2 | ~30 | ~1.5 * | IM + Q (ε-phase) + IFHT | [85] | |
| Mn53.5Al44.5C2 | 83 | 51 | 2.2 | 1.8 | AM + HD | [86] |
| Mn54Al46 | ~5 | ~3 | ~2.4 | MA(HEBM) + SPS | [87] | |
| Mn (56 at. %) and Al (44 at. %) | 40.3 | ~19 | 2.3 | MA + mix + HEBM + SPS | [88] | |
| Mn54Al43C3 | ~59 | ~40 | 3.6 | AM + MS(ε phase) + HEBM + HT | [89] | |
| Mn54Al44C2 | ~90 | ~46 | ~1.9 * | IM + MS + A | [90] | |
| Mn0.53 Al 0.46 C0.01 | 81 | 28 | 2.2 | MA + M + HT + AA | [91] | |
| 50%Mn-50%Al | 52 | ~21 | 2.6 | MA(HEBM) + two-step HT | [92] | |
| ~110 | ~36 | 1.95 | mix (HEBM) + M+ vibration M + HPS | [93] | ||
| Mn54Al44C2 | 60.34 | 37.36 | 3.8 | MS + HEBM + A | [94] | |
| Mn0.53Al0.46C0.01 | 72 | 37 | 1.9 | 0.6 | MA(HEBM) + A | [95] |
| Mn53.3Al45C1.7 | 127 | 46 | 1.54 | 1.53 | AM + SRMS | [96] |
| 70.5 wt.%Mn-29.5 wt.%A1, 0.8 wt.% C | 43 | 1.5 | 1.2 | IM + Q (ε-phase) + BM + A + CC + S | [97] | |
| 57 | 1.83 | 2.1 | IM + SRMS + HT | [98] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Attyabi, S.N.; Seyyed Ebrahimi, S.A.; Lalegani, Z.; Hamawandi, B. Reverse Magnetization Behavior Investigation of Mn-Al-C-(α-Fe) Nanocomposite Alloys with Different α-Fe Content Using First-Order Reversal Curves Analysis. Nanomaterials 2022, 12, 3303. https://doi.org/10.3390/nano12193303
Attyabi SN, Seyyed Ebrahimi SA, Lalegani Z, Hamawandi B. Reverse Magnetization Behavior Investigation of Mn-Al-C-(α-Fe) Nanocomposite Alloys with Different α-Fe Content Using First-Order Reversal Curves Analysis. Nanomaterials. 2022; 12(19):3303. https://doi.org/10.3390/nano12193303
Chicago/Turabian StyleAttyabi, Seyed Nourallah, Seyyed Ali Seyyed Ebrahimi, Zahra Lalegani, and Bejan Hamawandi. 2022. "Reverse Magnetization Behavior Investigation of Mn-Al-C-(α-Fe) Nanocomposite Alloys with Different α-Fe Content Using First-Order Reversal Curves Analysis" Nanomaterials 12, no. 19: 3303. https://doi.org/10.3390/nano12193303
APA StyleAttyabi, S. N., Seyyed Ebrahimi, S. A., Lalegani, Z., & Hamawandi, B. (2022). Reverse Magnetization Behavior Investigation of Mn-Al-C-(α-Fe) Nanocomposite Alloys with Different α-Fe Content Using First-Order Reversal Curves Analysis. Nanomaterials, 12(19), 3303. https://doi.org/10.3390/nano12193303







