Modulation of Casimir Force between Graphene-Covered Hyperbolic Materials
Abstract
:1. Introduction
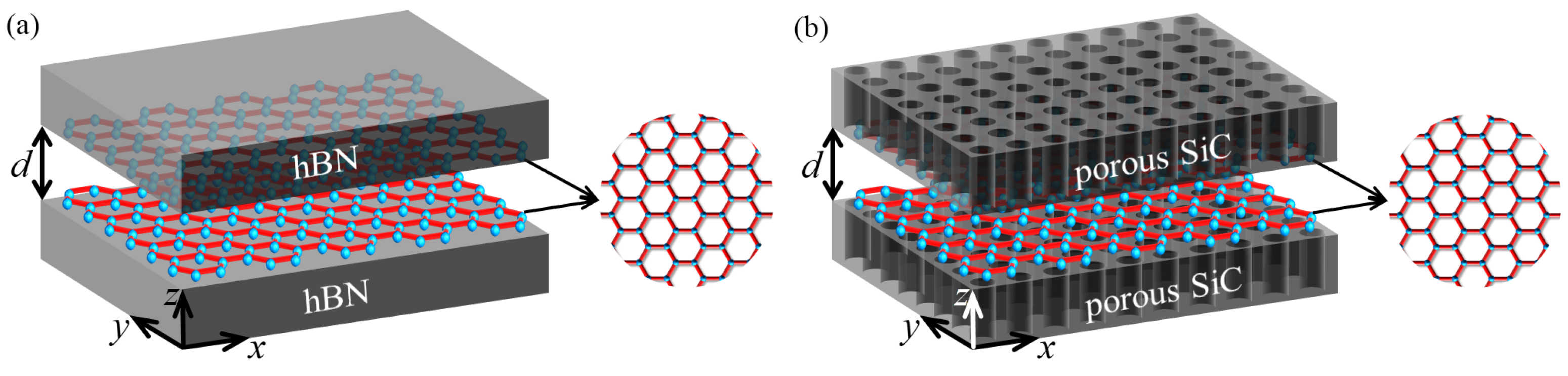
2. Materials and Methods
3. Results and Discussion
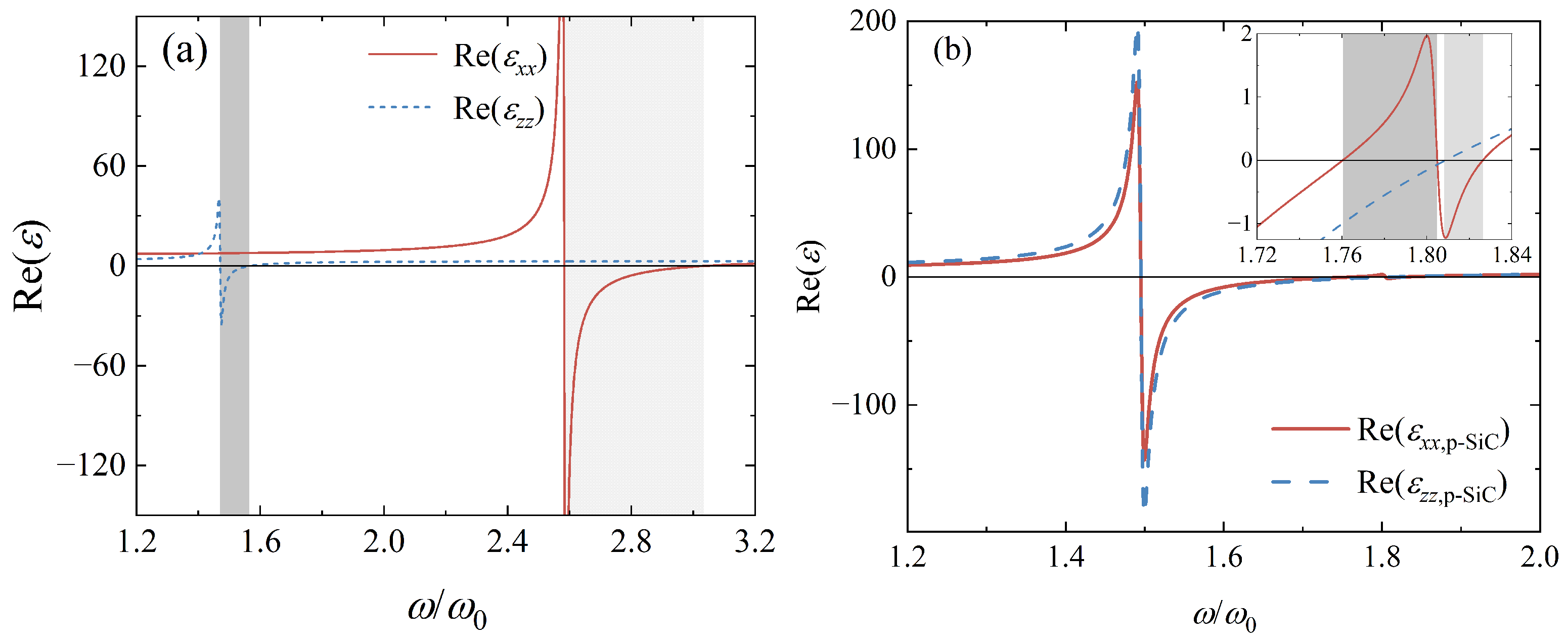
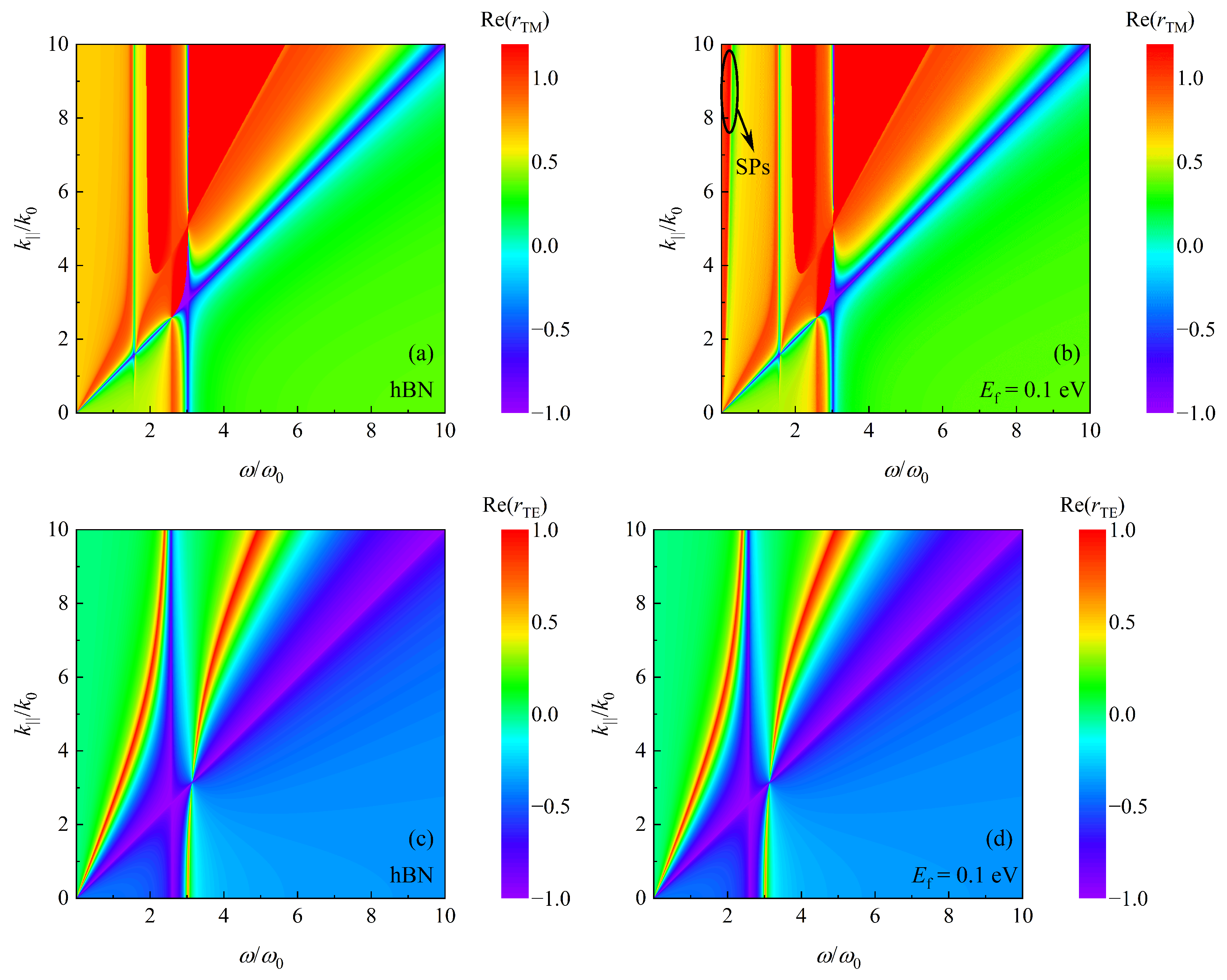
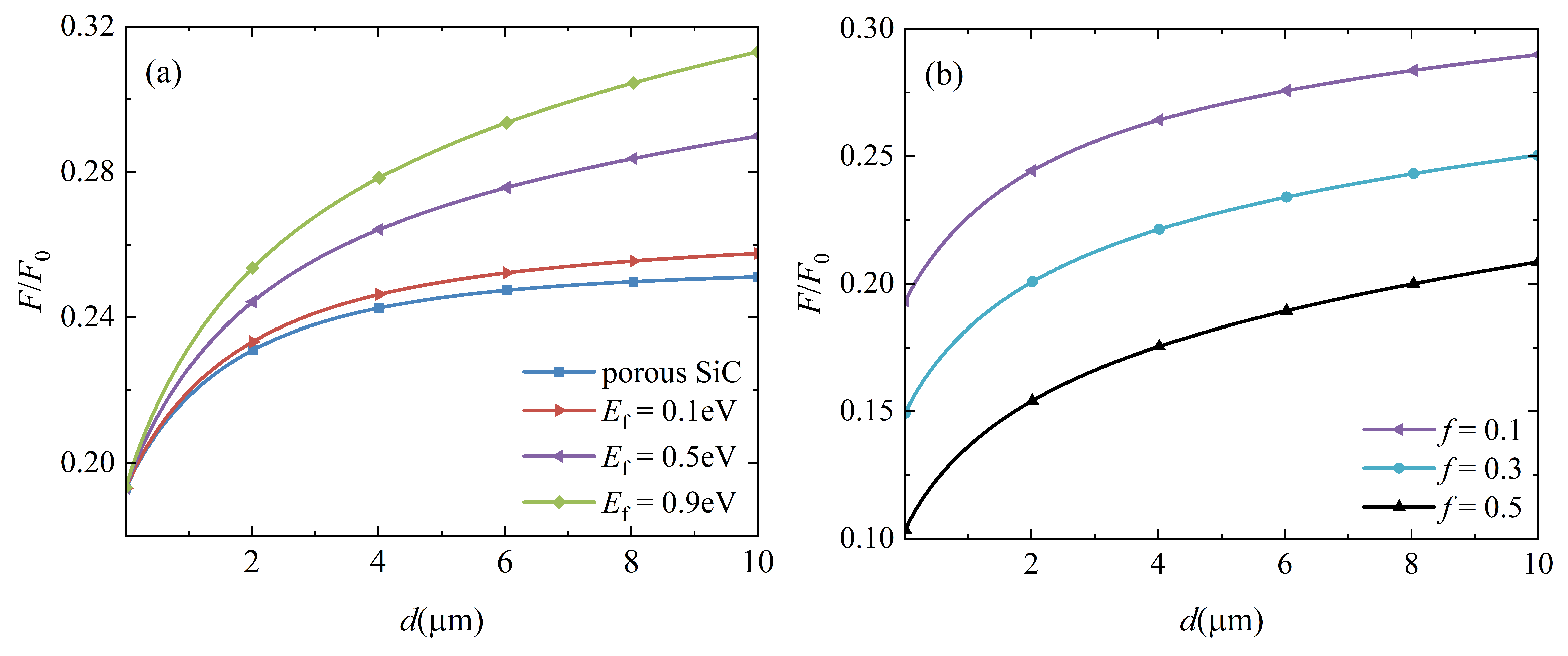
3.1. Casimir Force of Graphene-Covered hBN
3.2. Casimir Force of Graphene-Covered Porous SiC
3.3. Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

References
- Casimir, H.B. On the attraction between two perfectly conducting plates. Proc. K. Ned. Akad. Wet. 1948, 51, 793–795. [Google Scholar]
- Lifshitz, E.M. The theory of molecular attractive forces between solids. Soviet Phys. JETP 1956, 2, 73–83. [Google Scholar]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Experiment and theory in the Casimir effect. Contemp. Phys. 2006, 47, 131–144. [Google Scholar] [CrossRef] [Green Version]
- Chen, F.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Control of the Casimir force by the modification of dielectric properties with light. Phys. Rev. B 2007, 76, 035338. [Google Scholar] [CrossRef] [Green Version]
- Klimchitskaya, G.L.; Mohideen, U.; Mostepanenko, V.M. The Casimir force between real materials: Experiment and theory. Rev. Mod. Phys. 2009, 81, 1827–1885. [Google Scholar] [CrossRef] [Green Version]
- Woods, L.M.; Dalvit, D.A.R.; Tkatchenko, A.; Rodriguez-Lopez, P.; Rodriguez, A.W.; Podgornik, R. Materials perspective on Casimir and van der Waals interactions. Rev. Mod. Phys. 2016, 88, 045003. [Google Scholar] [CrossRef]
- Chang, C.C.; Banishev, A.A.; Castillo-Garza, R.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Gradient of the Casimir force between Au surfaces of a sphere and a plate measured using an atomic force microscope in a frequency-shift technique. Phys. Rev. B 2012, 85, 165443. [Google Scholar] [CrossRef] [Green Version]
- Banishev, A.A.; Chang, C.C.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Measurement of the gradient of the Casimir force between a nonmagnetic gold sphere and a magnetic nickel plate. Phys. Rev. B 2012, 85, 195422. [Google Scholar] [CrossRef] [Green Version]
- Banishev, A.A.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Demonstration of the Casimir Force between Ferromagnetic Surfaces of a Ni-Coated Sphere and a Ni-Coated Plate. Phys. Rev. Lett. 2013, 110, 137401. [Google Scholar] [CrossRef] [Green Version]
- Bimonte, G.; López, D.; Decca, R.S. Isoelectronic determination of the thermal Casimir force. Phys. Rev. B 2016, 93, 184434. [Google Scholar] [CrossRef] [Green Version]
- Xu, J.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Reducing detrimental electrostatic effects in Casimir-force measurements and Casimir-force-based microdevices. Phys. Rev. A 2018, 97, 032501. [Google Scholar] [CrossRef] [Green Version]
- Liu, M.; Xu, J.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Precision measurements of the gradient of the Casimir force between ultraclean metallic surfaces at larger separations. Phys. Rev. A 2019, 100, 052511. [Google Scholar] [CrossRef] [Green Version]
- Liu, M.; Xu, J.; Klimchitskaya, G.L.; Mostepanenko, V.M.; Mohideen, U. Examining the Casimir puzzle with an upgraded AFM-based technique and advanced surface cleaning. Phys. Rev. B 2019, 100, 081406. [Google Scholar] [CrossRef] [Green Version]
- Serry, F.M.; Walliser, D.; Maclay, G.J. The role of the casimir effect in the static deflection and stiction of membrane strips in microelectromechanical systems (MEMS). J. Appl. Phys. 1998, 84, 2501–2506. [Google Scholar] [CrossRef] [Green Version]
- Buks, E.; Roukes, M.L. Stiction, adhesion energy, and the Casimir effect in micromechanical systems. Phys. Rev. B 2001, 63, 033402. [Google Scholar] [CrossRef] [Green Version]
- Rodriguez, A.W.; Woolf, D.; Hui, P.C.; Iwase, E.; McCauley, A.P.; Capasso, F.; Loncar, M.; Johnson, S.G. Designing evanescent optical interactions to control the expression of Casimir forces in optomechanical structures. Appl. Phys. Lett. 2011, 98, 194105. [Google Scholar] [CrossRef]
- Kenneth, O.; Klich, I. Opposites Attract: A Theorem about the Casimir Force. Phys. Rev. Lett. 2006, 97, 160401. [Google Scholar] [CrossRef] [Green Version]
- Buks, E.; Roukes, M.L. Casimir force changes sign. Nature 2002, 419, 119–120. [Google Scholar] [CrossRef]
- Kenneth, O.; Klich, I.; Mann, A.; Revzen, M. Repulsive Casimir Forces. Phys. Rev. Lett. 2002, 89, 033001. [Google Scholar] [CrossRef] [Green Version]
- Schurig, D.; Mock, J.J.; Justice, B.J.; Cummer, S.A.; Pendry, J.B.; Starr, A.F.; Smith, D.R. Metamaterial Electromagnetic Cloak at Microwave Frequencies. Science 2006, 314, 977–980. [Google Scholar] [CrossRef] [Green Version]
- Fan, J.W.; Xu, J.; Cheng, M.T.; Yang, Y. Vacuum induced transparency in metamaterials. Opt. Express 2018, 26, 19498–19512. [Google Scholar] [CrossRef]
- Yang, Y.; Zeng, R.; Chen, H.; Zhu, S.; Zubairy, M.S. Controlling the Casimir force via the electromagnetic properties of materials. Phys. Rev. A 2010, 81, 022114. [Google Scholar] [CrossRef]
- Song, G.; Xu, J.; Zhu, C.; He, P.; Yang, Y.; Zhu, S.Y. Casimir force between hyperbolic metamaterials. Phys. Rev. A 2017, 95, 023814. [Google Scholar] [CrossRef]
- Zeng, R.; Yang, Y.; Zhu, S. Casimir force between anisotropic single-negative metamaterials. Phys. Rev. A 2013, 87, 063823. [Google Scholar] [CrossRef]
- Zeng, R.; Yang, Y. Tunable polarity of the Casimir force based on saturated ferrites. Phys. Rev. A 2011, 83, 012517. [Google Scholar] [CrossRef]
- Phan, A.D.; Viet, N.A.; Poklonski, N.A.; Woods, L.M.; Le, C.H. Interaction of a graphene sheet with a ferromagnetic metal plate. Phys. Rev. B 2012, 86, 155419. [Google Scholar] [CrossRef] [Green Version]
- Grushin, A.G.; Cortijo, A. Tunable Casimir Repulsion with Three-Dimensional Topological Insulators. Phys. Rev. Lett. 2011, 106, 020403. [Google Scholar] [CrossRef]
- Nie, W.; Zeng, R.; Lan, Y.; Zhu, S. Casimir force between topological insulator slabs. Phys. Rev. B 2013, 88, 085421. [Google Scholar] [CrossRef]
- Zeng, R.; Chen, L.; Nie, W.; Bi, M.; Yang, Y.; Zhu, S. Enhancing Casimir repulsion via topological insulator multilayers. Phys. Lett. A 2016, 380, 2861–2869. [Google Scholar] [CrossRef]
- Geim, A.K.; Novoselov, K.S. The rise of graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef]
- Castro Neto, A.H.; Guinea, F.; Peres, N.M.R.; Novoselov, K.S.; Geim, A.K. The electronic properties of graphene. Rev. Mod. Phys. 2009, 81, 109–162. [Google Scholar] [CrossRef] [Green Version]
- Yu, T.; Luo, R.; Wang, T.; Zhang, D.; Liu, W.; Yu, T.; Liao, Q. Enhancement of Casimir Friction between Graphene-Covered Topological Insulator. Nanomaterials 2022, 12, 1148. [Google Scholar] [CrossRef]
- Luo, R.; Yang, J.R.; Wang, T.B.; Zhang, D.J.; Liu, W.X.; Yu, T.B.; Liao, Q.H. Enhancement of Casimir friction between graphene-covered hyperbolic materials. Phys. Lett. A 2021, 387, 127006. [Google Scholar] [CrossRef]
- Fialkovsky, I.V.; Marachevsky, V.N.; Vassilevich, D.V. Finite-temperature Casimir effect for graphene. Phys. Rev. B 2011, 84, 035446. [Google Scholar] [CrossRef] [Green Version]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Van der Waals and Casimir interactions between two graphene sheets. Phys. Rev. B 2013, 87, 075439. [Google Scholar] [CrossRef] [Green Version]
- Klimchitskaya, G.L.; Mostepanenko, V.M.; Sernelius, B.E. Two approaches for describing the Casimir interaction in graphene: Density-density correlation function versus polarization tensor. Phys. Rev. B 2014, 89, 125407. [Google Scholar] [CrossRef] [Green Version]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Classical limit of the Casimir interaction for thin films with applications to graphene. Phys. Rev. B 2014, 89, 035407. [Google Scholar] [CrossRef] [Green Version]
- Drosdoff, D.; Woods, L.M. Casimir interactions between graphene sheets and metamaterials. Phys. Rev. A 2011, 84, 062501. [Google Scholar] [CrossRef] [Green Version]
- Klimchitskaya, G.L.; Mostepanenko, V.M. Casimir and Casimir-Polder Forces in Graphene Systems: Quantum Field Theoretical Description and Thermodynamics. Universe 2020, 6, 150. [Google Scholar] [CrossRef]
- Wang, T.B.; Zhou, Y.; Mu, H.Q.; Shehzad, K.; Zhang, D.J.; Liu, W.X.; Yu, T.B.; Liao, Q.H. Enhancement of lateral Casimir force on a rotating particle near hyperbolic metamaterial. Nanotechnology 2022, 33, 245001. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Fal’ko, V.I.; Colombo, L.; Gellert, P.R.; Schwab, M.G.; Kim, K. A roadmap for graphene. Nature 2012, 490, 192–200. [Google Scholar] [CrossRef]
- Zeng, X.; Al-Amri, M.; Zubairy, M.S. Tunable Goos-Hänchen shift from graphene ribbon array. Opt. Express 2017, 25, 23579–23588. [Google Scholar] [CrossRef]
- Din, R.U.; Zeng, X.; Ahmad, I.; Ge, G.Q. Enhanced and highly tunable Goos-Hänchan shifts at a nanocomposite-graphene interface. Appl. Phys. Lett. 2019, 114, 161902. [Google Scholar] [CrossRef]
- Zeng, X.; Li, Z.; Ge, G.Q.; Zubairy, M.S. Quantum interference near graphene layers: Observing the surface plasmons with transverse electric polarization. Phys. Rev. A 2019, 99, 043811. [Google Scholar] [CrossRef]
- Poddubny, A.; Iorsh, I.; Belov, P.; Kivshar, Y. Hyperbolic metamaterials. Nat. Photonics 2013, 7, 948–957. [Google Scholar] [CrossRef]
- Shekhar, P.; Atkinson, J.; Jacob, Z. Hyperbolic metamaterials: Fundamentals and applications. Nano Converg. 2014, 1, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Lin, Y.; Connell, J.W. Advances in 2D boron nitride nanostructures: Nanosheets, nanoribbons, nanomeshes, and hybrids with graphene. Nanoscale 2012, 4, 6908–6939. [Google Scholar] [CrossRef]
- Su, C.; Fu, C. Surface and volume phonon polaritons in a uniaxial hyperbolic material: Optic axis parallel versus perpendicular to the surface. Opt. Express 2021, 29, 39824–39837. [Google Scholar] [CrossRef]
- Jacob, Z.; Smolyaninov, I.I.; Narimanov, E.E. Broadband Purcell effect: Radiative decay engineering with metamaterials. Appl. Phys. Lett. 2012, 100, 181105. [Google Scholar] [CrossRef] [Green Version]
- Markel, V.A. Introduction to the Maxwell Garnett approximation: Tutorial. J. Opt. Soc. Am. A 2016, 33, 1244–1256. [Google Scholar] [CrossRef] [Green Version]
- Song, J.; Cheng, Q. Near-field radiative heat transfer between graphene and anisotropic magneto-dielectric hyperbolic metamaterials. Phys. Rev. B 2016, 94, 125419. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, G.; Liu, Z.; Jia, L.; Li, C.; Chang, Y. Modulation of Casimir Force between Graphene-Covered Hyperbolic Materials. Nanomaterials 2022, 12, 2168. https://doi.org/10.3390/nano12132168
Song G, Liu Z, Jia L, Li C, Chang Y. Modulation of Casimir Force between Graphene-Covered Hyperbolic Materials. Nanomaterials. 2022; 12(13):2168. https://doi.org/10.3390/nano12132168
Chicago/Turabian StyleSong, Ge, Zhixiang Liu, Lingchun Jia, Cong Li, and Yingli Chang. 2022. "Modulation of Casimir Force between Graphene-Covered Hyperbolic Materials" Nanomaterials 12, no. 13: 2168. https://doi.org/10.3390/nano12132168
APA StyleSong, G., Liu, Z., Jia, L., Li, C., & Chang, Y. (2022). Modulation of Casimir Force between Graphene-Covered Hyperbolic Materials. Nanomaterials, 12(13), 2168. https://doi.org/10.3390/nano12132168





