Computational and Experimental Analysis of Gold Nanorods in Terms of Their Morphology: Spectral Absorption and Local Field Enhancement
Abstract
:1. Introduction
2. Materials and Methods
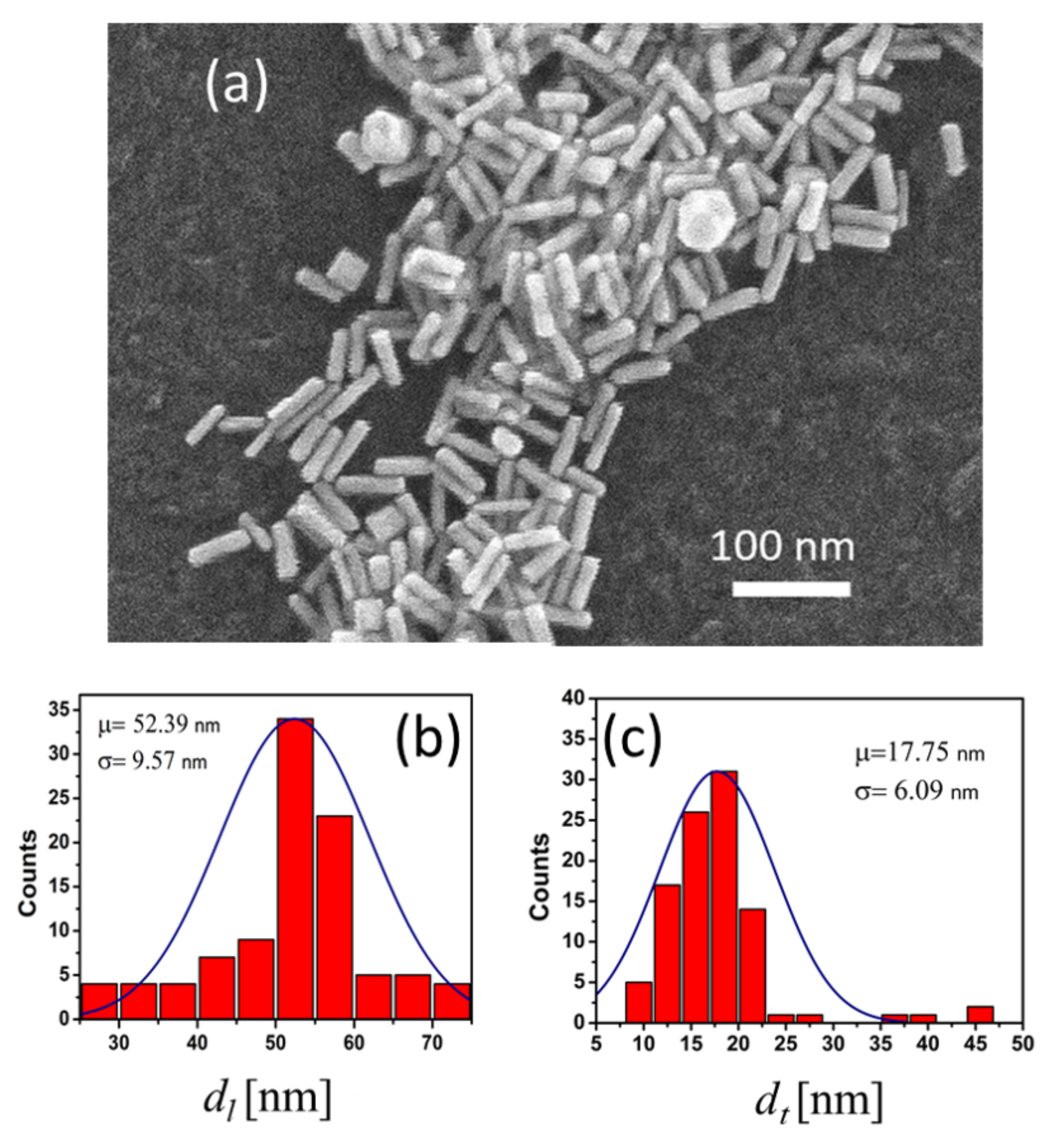
2.1. Synthesis and Morphological Analysis of Gold Nanorods
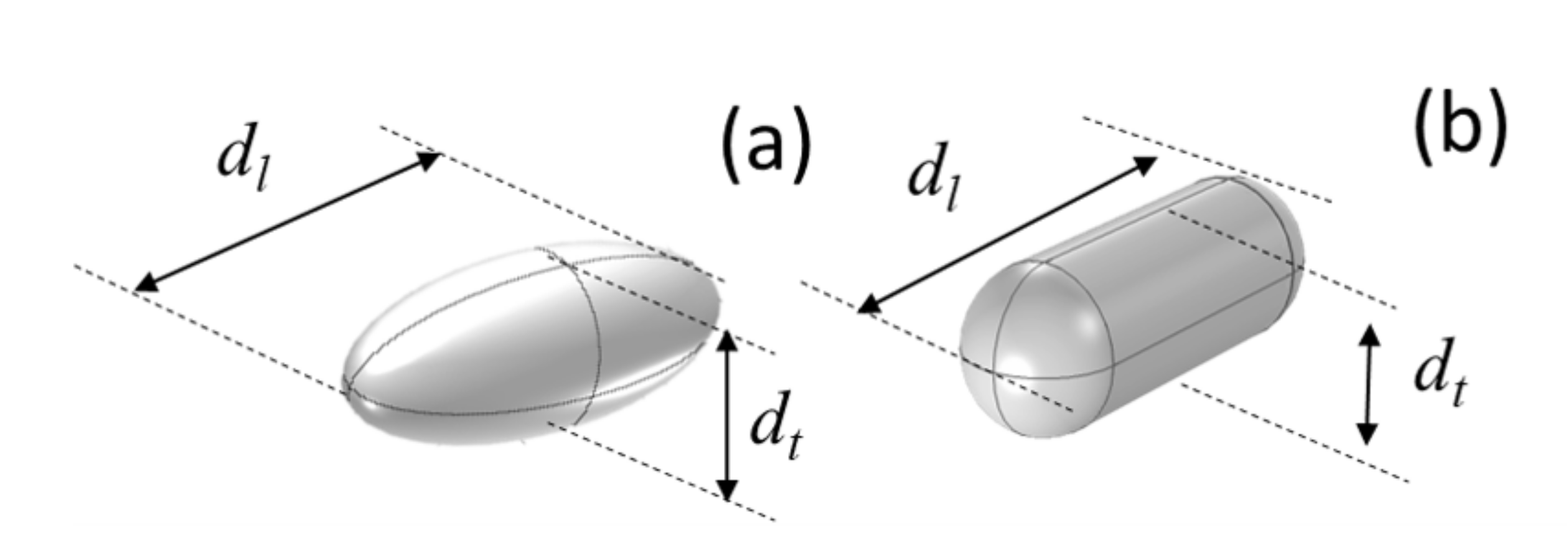
2.2. Computational Analysis of the Electromagnetic Response
2.3. Measurement of the Spectral Absorption
3. Results and Discussion
3.1. Absorption Cross-Section, , and Spectral Absorption
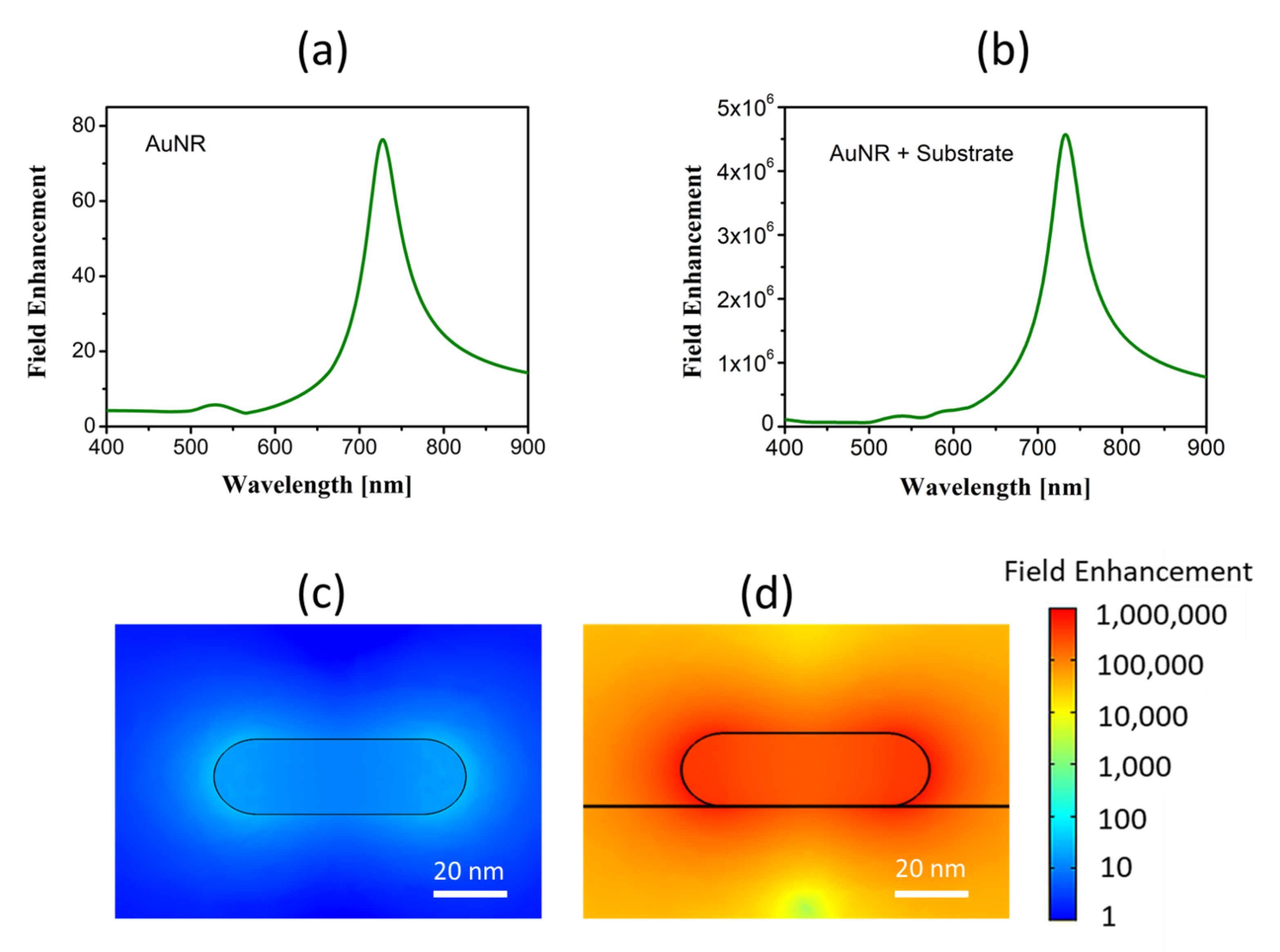
3.2. Near Field Enhancement
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Prasad, P.N. Nanophotonics; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2004. [Google Scholar] [CrossRef]
- Novotny, L.; Hecht, B. Principles of Nano-Optics, 2nd ed.; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Haus, J. 1—Introduction to nanophotonics. In Fundamentals and Applications of Nanophotonics; Haus, J.W., Ed.; Woodhead Publishing: Cambridge, UK, 2016. [Google Scholar] [CrossRef]
- Yan, J.; Liu, X.; Ma, C.; Huang, Y.; Yang, G. All-dielectric materials and related nanophotonic applications. Mater. Sci. Eng. R Rep. 2020, 141, 100563. [Google Scholar] [CrossRef]
- Anker, J.N.; Hall, W.P.; Lyandres, O.; Shah, N.C.; Zhao, J.; Van Duyne, R.P. Biosensing with plasmonic nanosensors. Nat. Mater. 2008, 7, 442–453. [Google Scholar] [CrossRef]
- Sun, M.; Dong, H.; Dougherty, A.W.; Lu, Q.; Peng, D.; Wong, W.T.; Huang, B.; Sun, L.D.; Yan, C.H. Nanophotonic energy storage in upconversion nanoparticles. Nano Energy 2019, 56, 473–481. [Google Scholar] [CrossRef]
- Xavier, J.; Vincent, S.; Meder, F.; Vollmer, F. Advances in optoplasmonic sensors—Combining optical nano/microcavities and photonic crystals with plasmonic nanostructures and nanoparticles. Nanophotonics 2018, 7, 1–38. [Google Scholar] [CrossRef]
- Astafyeva, L.; Pustovalov, V.; Fritzsche, W. Tuning light concentration inside plasmonic core-shell nanoparticles during laser irradiation. Photonics Nanostruct. Fundam. Appl. 2017, 26, 35–40. [Google Scholar] [CrossRef]
- Zhao, H.; Chin, L.K.; Shi, Y.; Liu, P.Y.; Zhang, Y.; Cai, H.; Yap, E.P.H.; Ser, W.; Liu, A.Q. Continuous optical sorting of nanoscale biomolecules in integrated microfluidic-nanophotonic chips. Sensors Actuators B Chem. 2021, 331, 129428. [Google Scholar] [CrossRef]
- Pisanello, F. Implantable micro and nanophotonic devices: Toward a new generation of neural interfaces. Microelectron. Eng. 2019, 215, 110979. [Google Scholar] [CrossRef]
- Luan, E.; Shoman, H.; Ratner, D.M.; Cheung, K.C.; Chrostowski, L. Silicon Photonic Biosensors Using Label-Free Detection. Sensors 2018, 18, 3519. [Google Scholar] [CrossRef] [Green Version]
- Dombrovsky, L.; Baillis, D. Thermal Radiation in Disperse Systems: An Engineering Approach; Begell House: Danbury, CT, USA, 2010. [Google Scholar]
- Jindal, A.B. The effect of particle shape on cellular interaction and drug delivery applications of micro- and nanoparticles. Int. J. Pharm. 2017, 532, 450–465. [Google Scholar] [CrossRef]
- Jo, D.H.; Kim, J.H.; Lee, T.G.; Kim, J.H. Size, surface charge, and shape determine therapeutic effects of nanoparticles on brain and retinal diseases. Nanomed. Nanotechnol. Biol. Med. 2015, 11, 1603–1611. [Google Scholar] [CrossRef]
- Kaya, H.; Alkasem, M.; Arslan, K. Effect of nanoparticle shape of Al2O3/Pure Water nanofluid on evacuated U-Tube solar collector efficiency. Renew. Energy 2020, 162, 267–284. [Google Scholar] [CrossRef]
- Kinnear, C.; Moore, T.L.; Rodriguez-Lorenzo, L.; Rothen-Rutishauser, B.; Petri-Fink, A. Form Follows Function: Nanoparticle Shape and Its Implications for Nanomedicine. Chem. Rev. 2017, 117, 11476–11521. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; Li, L.; Liu, T.; Hao, N.; Liu, H.; Chen, D.; Tang, F. The Shape Effect of Mesoporous Silica Nanoparticles on Biodistribution, Clearance, and Biocompatibility in Vivo. ACS Nano 2011, 5, 5390–5399. [Google Scholar] [CrossRef]
- Heydari, E. Nanoplasmonic biodetection based on bright-field imaging of resonantly coupled gold-silver nanoparticles. Photonics Nanostruct. Fundam. Appl. 2019, 36, 100708. [Google Scholar] [CrossRef]
- Liu, J.; He, H.; Xiao, D.; Yin, S.; Ji, W.; Jiang, S.; Luo, D.; Wang, B.; Liu, Y. Recent Advances of Plasmonic Nanoparticles and their Applications. Materials 2018, 11, 1833. [Google Scholar] [CrossRef] [Green Version]
- Ma, Y.; Cai, F.; Li, Y.; Chen, J.; Han, F.; Lin, W. A review of the application of nanoparticles in the diagnosis and treatment of chronic kidney disease. Bioact. Mater. 2020, 5, 732–743. [Google Scholar] [CrossRef]
- Fu, X.; Cai, J.; Zhang, X.; Li, W.D.; Ge, H.; Hu, Y. Top-down fabrication of shape-controlled, monodisperse nanoparticles for biomedical applications. Adv. Drug Deliv. Rev. 2018, 132, 169–187. [Google Scholar] [CrossRef]
- Jeong, H.H.; Choi, E.; Ellis, E.; Lee, T.C. Recent advances in gold nanoparticles for biomedical applications: From hybrid structures to multi-functionality. J. Mater. Chem. B 2019, 7, 3480–3496. [Google Scholar] [CrossRef] [Green Version]
- Elahi, N.; Kamali, M.; Baghersad, M.H. Recent biomedical applications of gold nanoparticles: A review. Talanta 2018, 184, 537–556. [Google Scholar] [CrossRef]
- Khlebtsov, N.G.; Dykman, L.A. Optical properties and biomedical applications of plasmonic nanoparticles. J. Quant. Spectrosc. Radiat. Transf. 2010, 111, 1–35. [Google Scholar] [CrossRef]
- Austin, L.A.; Mackey, M.A.; Dreaden, E.C.; El-Sayed, M.A. The optical, photothermal, and facile surface chemical properties of gold and silver nanoparticles in biodiagnostics, therapy, and drug delivery. Arch. Toxicol. 2014, 88, 1391–1417. [Google Scholar] [CrossRef] [Green Version]
- Gu, X.; Timchenko, V.; Heng Yeoh, G.; Dombrovsky, L.; Taylor, R. The Effect of Gold Nanorods Clustering on Near-Infrared Radiation Absorption. Appl. Sci. 2018, 8, 1132. [Google Scholar] [CrossRef] [Green Version]
- Dombrovsky, L.A.; Timchenko, V.; Jackson, M.; Yeoh, G.H. A combined transient thermal model for laser hyperthermia of tumors with embedded gold nanoshells. Int. J. Heat Mass Transf. 2011, 54, 5459–5469. [Google Scholar] [CrossRef]
- Hewakuruppu, Y.L.; Dombrovsky, L.A.; Chen, C.; Timchenko, V.; Jiang, X.; Baek, S.; Taylor, R.A. Plasmonic “pump-probe” method to study semi-transparent nanofluids. Appl. Opt. 2013, 52, 6041–6050. [Google Scholar] [CrossRef] [PubMed]
- Wang, A.X.; Kong, X. Review of Recent Progress of Plasmonic Materials and Nano-Structures for Surface-Enhanced Raman Scattering. Materials 2015, 8, 3024–3052. [Google Scholar] [CrossRef]
- Tang, L.; Li, S.; Han, F.; Liu, L.; Xu, L.; Ma, W.; Kuang, H.; Li, A.; Wang, L.; Xu, C. SERS-active Au@Ag nanorod dimers for ultrasensitive dopamine detection. Biosens. Bioelectron. 2015, 71, 7–12. [Google Scholar] [CrossRef]
- Fazio, B.; D’Andrea, C.; Foti, A.; Messina, E.; Irrera, A.; Donato, M.G.; Villari, V.; Micali, N.; Maragò, O.M.; Gucciardi, P.G. SERS detection of Biomolecules at Physiological pH via aggregation of Gold Nanorods mediated by Optical Forces and Plasmonic Heating. Sci. Rep. 2016, 6, 26952. [Google Scholar] [CrossRef] [Green Version]
- Rong, Z.; Wang, C.; Wang, J.; Wang, D.; Xiao, R.; Wang, S. Magnetic immunoassay for cancer biomarker detection based on surface-enhanced resonance Raman scattering from coupled plasmonic nanostructures. Biosens. Bioelectron. 2016, 84, 15–21. [Google Scholar] [CrossRef]
- Kolosovas-Machuca, E.S.; Cuadrado, A.; Ojeda-Galván, H.J.; Ortiz-Dosal, L.C.; Hernández-Arteaga, A.C.; Rodríguez-Aranda, M.d.C.; Navarro-Contreras, H.R.; Alda, J.; González, F.J. Detection of Histamine Dihydrochloride at Low Concentrations Using Raman Spectroscopy Enhanced by Gold Nanostars Colloids. Nanomaterials 2019, 9, 211. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mie, G. Beiträge zur Optik trüber Medien, speziell kolloidaler Metallösungen. Ann. Phys. 1908, 330, 377–445. [Google Scholar] [CrossRef]
- Chýlek, P.; Zhan, J. Absorption and scattering of light by small particles: The interference structure. Appl. Opt. 1990, 29, 3984. [Google Scholar] [CrossRef] [PubMed]
- Horvath, H. Gustav Mie and the scattering and absorption of light by particles: Historic developments and basics. J. Quant. Spectrosc. Radiat. Transf. 2009, 110, 787–799. [Google Scholar] [CrossRef]
- Rother, T.; Schmidt, K. The Discretized Mie-Formalism for Electromagnetic Scattering—Summary. J. Electromagn. Waves Appl. 1997, 11, 1619–1625. [Google Scholar] [CrossRef]
- Ogura, H.; Takahashi, N. Scattering of waves from a random spherical surface—Mie scattering. J. Math. Phys. 1990, 31, 61–75. [Google Scholar] [CrossRef]
- Gans, R. Über die Form ultramikroskopischer Goldteilchen. Ann. Phys. 1912, 342, 881–900. [Google Scholar] [CrossRef] [Green Version]
- Mishchenko, M.I.; Travis, L.D.; Lacis, A.A. Scattering, Absorption, and Emission of Light by Small Particles; Cambridge University Press: Cambrigde, UK, 2002. [Google Scholar]
- Somerville, W.; Auguié, B.; Le Ru, E. smarties: User-friendly codes for fast and accurate calculations of light scattering by spheroids. J. Quant. Spectrosc. Radiat. Transf. 2016, 174, 39–55. [Google Scholar] [CrossRef] [Green Version]
- Kumar, J.; Wei, X.; Barrow, S.; Funston, A.M.; Thomas, K.G.; Mulvaney, P. Surface plasmon coupling in end-to-end linked gold nanorod dimers and trimers. Phys. Chem. Chem. Phys. 2013, 15, 4258–4264. [Google Scholar] [CrossRef]
- Chau, Y.F.; Chen, M.W.; Tsai, D.P. Three-dimensional analysis of surface plasmon resonance modes on a gold nanorod. Appl. Opt. 2009, 48, 617–622. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dong, J.; Zhao, X.; Gao, W.; Han, Q.; Qi, J.; Wang, Y.; Guo, S.; Sun, M. Nanoscale Vertical Arrays of Gold Nanorods by Self-Assembly: Physical Mechanism and Application. Nanoscale Res. Lett. 2019, 14, 118. [Google Scholar] [CrossRef]
- Singh, S.; Kaler, R.; Sharma, S. FEM simulation analysis of fiber optic surface plasmon resonance sensor based on array of circular gold nanorod. Optik 2019, 183, 508–512. [Google Scholar] [CrossRef]
- Davis, T.J.; Vernon, K.C.; Gómez, D.E. Effect of retardation on localized surface plasmon resonances in a metallic nanorod. Opt. Express 2009, 17, 23655–23663. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Zhao, F.; Li, Z.; Fang, Y.; Wang, P. Tailoring of Localized Surface Plasmon Resonances of Core-Shell Au Ag Nanorods by Changing the Thickness of Ag Shell. Plasmonics 2016, 11, 1511–1517. [Google Scholar] [CrossRef]
- Yurkin, M.; Hoekstra, A. The discrete dipole approximation: An overview and recent developments. J. Quant. Spectrosc. Radiat. Transf. 2007, 106, 558–589. [Google Scholar] [CrossRef] [Green Version]
- Dodson, S.L.; Cao, C.; Zaribafzadeh, H.; Li, S.; Xiong, Q. Engineering plasmonic nanorod arrays for colon cancer marker detection. Biosens. Bioelectron. 2015, 63, 472–477. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mu, H.; Lv, J.; Liu, C.; Sun, T.; Chu, P.K.; Zhang, J. Localized surface plasmon resonance properties of Ag nanorod arrays on graphene-coated Au substrate. Opt. Commun. 2017, 402, 216–220. [Google Scholar] [CrossRef]
- Bhatia, P.; Verma, S.; Sinha, M. Tunable plasmonic properties of elongated bimetallic alloys nanoparticles towards deep UV-NIR absorbance and sensing. J. Quant. Spectrosc. Radiat. Transf. 2020, 241, 106751. [Google Scholar] [CrossRef]
- Obare, S.; Alsawafta, M.; Wahbeh, M.; Truong, V.V. Simulated Optical Properties of Gold Nanocubes and Nanobars by Discrete Dipole Approximation. J. Nanomater. 2012, 2012, 283230. [Google Scholar] [CrossRef]
- Bansal, A.; Verma, S. Optical response of noble metal alloy nanostructures. Phys. Lett. A 2015, 379, 163–169. [Google Scholar] [CrossRef]
- Waiwijit, U.; Chananonnawathorn, C.; Eimchai, P.; Bora, T.; Hornyak, G.; Nuntawong, N. Fabrication of Au-Ag nanorod SERS substrates by co-sputtering technique and dealloying with selective chemical etching. Appl. Surf. Sci. 2020, 530, 147171. [Google Scholar] [CrossRef]
- Vassalini, I.; Rotunno, E.; Lazzarini, L.; Alessandri, I. “Stainless” Gold Nanorods: Preserving Shape, Optical Properties, and SERS Activity in Oxidative Environment. ACS Appl. Mater. Interfaces 2015, 7, 18794–18802. [Google Scholar] [CrossRef]
- Zhu, S.Q.; Zhang, T.; Guo, X.L.; Zhang, X.Y. Self-assembly of large-scale gold nanoparticle arrays and their application in SERS. Nanoscale Res. Lett. 2014, 9, 114. [Google Scholar] [CrossRef] [Green Version]
- Khlebtsov, B.; Khanadeev, V.; Khlebtsov, N. Surface-enhanced Raman scattering inside Au@Ag core/shell nanorods. Nano Res. 2016, 9, 2303–2318. [Google Scholar] [CrossRef]
- Martín, A.; Pescaglini, A.; Schopf, C.; Scardaci, V.; Coull, R.; Byrne, L.; Iacopino, D. Surface-Enhanced Raman Scattering of 4-Aminobenzenethiol on Au Nanorod Ordered Arrays. J. Phys. Chem. C 2014, 118, 13260–13267. [Google Scholar] [CrossRef]
- Hyeon Kim, N.; Kim, S.; Choi, M.; Park, H.H.; Kim, N.H.; Park, S.Y.; Byun, K.M.; Lee, S.Y. Combination of periodic hybrid nanopillar arrays and gold nanorods for improving detection performance of surface-enhanced Raman spectroscopy. Sensors Actuators B Chem. 2018, 258, 18–24. [Google Scholar] [CrossRef]
- Gorbunova, M.; Apyari, V.; Dmitrienko, S.; Zolotov, Y. Gold nanorods and their nanocomposites: Synthesis and recent applications in analytical chemistry. TrAC Trends Anal. Chem. 2020, 130, 115974. [Google Scholar] [CrossRef]
- Serrà, A.; Vallés, E. Advanced electrochemical synthesis of multicomponent metallic nanorods and nanowires: Fundamentals and applications. Appl. Mater. Today 2018, 12, 207–234. [Google Scholar] [CrossRef]
- Cheng, X.; Dong, P.; Huang, Z.; Zhang, Y.; Chen, Y.; Nie, X.; Zhang, X. Green synthesis of plasmonic Ag nanoparticles anchored TiO2 nanorod arrays using cold plasma for visible-light-driven photocatalytic reduction of CO2. J. CO2 Util. 2017, 20, 200–207. [Google Scholar] [CrossRef]
- Qiu, L.; Pol, V.G.; Calderon-Moreno, J.; Gedanken, A. Synthesis of tin nanorods via a sonochemical method combined with a polyol process. Ultrason. Sonochem. 2005, 12, 243–247. [Google Scholar] [CrossRef]
- Katsumata, H.; Inoue, K.; Suzuki, T.; Kaneco, S. Facile Synthesis of WO3 Nanorod Thin Films on W Substrate with Enhanced Photocatalytic Performance. Catal. Lett. 2014, 144, 837–842. [Google Scholar] [CrossRef]
- Li, P.; Wu, Y.; Li, D.; Su, X.; Luo, C.; Wang, Y.; Hu, J.; Li, G.; Jiang, H.; Zhang, W. Seed-Mediated Synthesis of Tunable-Aspect-Ratio Gold Nanorods for Near-Infrared Photoacoustic Imaging. Nanoscale Res. Lett. 2018, 13, 313. [Google Scholar] [CrossRef] [Green Version]
- Nikoobakht, B.; El-Sayed, M.A. Preparation and Growth Mechanism of Gold Nanorods (NRs) Using Seed-Mediated Growth Method. Chem. Mater. 2003, 15, 1957–1962. [Google Scholar] [CrossRef]
- Bullen, C.; Zijlstra, P.; Bakker, E.; Gu, M.; Raston, C. Chemical Kinetics of Gold Nanorod Growth in Aqueous CTAB Solutions. Cryst. Growth Des. 2011, 11, 3375–3380. [Google Scholar] [CrossRef]
- Scarabelli, L.; Sánchez-Iglesias, A.; Pérez-Juste, J.; Liz-Marzán, L.M. A “Tips and Tricks” Practical Guide to the Synthesis of Gold Nanorods. J. Phys. Chem. Lett. 2015, 6, 4270–4279. [Google Scholar] [CrossRef] [Green Version]
- Gou, L.; Murphy, C.J. Fine-Tuning the Shape of Gold Nanorods. Chem. Mater. 2005, 17, 3668–3672. [Google Scholar] [CrossRef]
- Wang, C.; Wang, T.; Ma, Z.; Su, Z. pH-tuned synthesis of gold nanostructures from gold nanorods with different aspect ratios. Nanotechnology 2005, 16, 2555–2560. [Google Scholar] [CrossRef]
- Zhu, J.; Yong, K.T.; Roy, I.; Hu, R.; Ding, H.; Zhao, L.; Swihart, M.T.; He, G.S.; Cui, Y.; Prasad, P.N. Additive controlled synthesis of gold nanorods (GNRs) for two-photon luminescence imaging of cancer cells. Nanotechnology 2010, 21, 285106. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Wang, F.; Guo, Y.; Chen, R.; Shen, Y.; Guo, A.; Liu, J.; Zhang, X.; Zhou, D.; Guo, S. Controlled synthesis of monodisperse gold nanorods with different aspect ratios in the presence of aromatic additives. J. Nanoparticle Res. 2014, 16, 2806. [Google Scholar] [CrossRef] [Green Version]
- Thambi, V.; Kar, A.; Ghosh, P.; Paital, D.; Gautam, A.R.S.; Khatua, S. Synthesis of Complex Nanoparticle Geometries via pH-Controlled Overgrowth of Gold Nanorods. ACS Omega 2019, 4, 13733–13739. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gallagher, R.; Zhang, X.; Altomare, A.; Lawrence, D.; Shawver, N.; Tran, N.; Beazley, M.; Chen, G. pH-mediated synthesis of monodisperse gold nanorods with quantitative yield and molecular level insight. Nano Res. 2021, 14, 1167–1174. [Google Scholar] [CrossRef]
- Cuadrado, A.; Alda, J.; Gonzalez, F.J. Distributed bolometric effect in optical antennas and resonant structures. J. Nanophotonics 2012, 6, 063512. [Google Scholar] [CrossRef]
- Mennemanteuil, M.M.; des Francs, G.C.; Buret, M.; Dasgupta, A.; Cuadrado, A.; Alda, J.; Bouhelier, A. Laser-induced thermoelectric effects in electrically biased nanoscale constrictions. Nanophotonics 2018, 7, 1917–1927. [Google Scholar] [CrossRef]
- Fonseca, P.Z.G.; Alda, I.; Marino, F.; Cuadrado, A.; D’Ambrosio, V.; Gieseler, J.; Quidant, R. Slow thermo-optomechanical pulsations in suspended one-dimensional photonic crystal nanocavities. Phys. Rev. A 2020, 102, 053518. [Google Scholar] [CrossRef]
- Bohren, C.; Huffman, D. Absorption and Scattering by an Arbitrary Particle. In Absorption and Scattering of Light by Small Particles; Bohren, C., Huffman, D., Eds.; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 1998. [Google Scholar] [CrossRef]
- Umashankar, K.; Taflove, A. A Novel Method to Analyze Electromagnetic Scattering of Complex Objects. IEEE Trans. Electromagn. Compat. 1982, EMC-24, 397–405. [Google Scholar] [CrossRef]
- Holland, R.; Williams, J.W. Total-Field versus Scattered-Field Finite-Difference Codes: A Comparative Assessment. IEEE Trans. Nucl. Sci. 1983, 30, 4583–4588. [Google Scholar] [CrossRef]
- Bardi, I.; Badics, Z.; Cendes, Z.J. Total and Scattered Field Formulations in the Transfinite Element Method. IEEE Trans. Magn. 2008, 44, 778–781. [Google Scholar] [CrossRef]
- Liu, Z.; Ping, L.; Sun, B.; Sun, G.; He, X. Scattering of 3-D objects with a new total-and scattered-field decomposition technique for FEM. In Proceedings of the 2010 Asia-Pacific International Symposium on Electromagnetic Compatibility, Beijing, China, 12–16 April 2010; pp. 1462–1465. [Google Scholar] [CrossRef]
- Nikoobakht, B.; Wang, J.; El-Sayed, M.A. Surface-enhanced Raman scattering of molecules adsorbed on gold nanorods: Off-surface plasmon resonance condition. Chem. Phys. Lett. 2002, 366, 17–23. [Google Scholar] [CrossRef]
- Le Ru, E.C.; Blackie, E.; Meyer, M.; Etchegoin, P.G. Surface enhanced Raman scattering enhancement factors: A comprehensive study. J. Phys. Chem. C 2007, 111, 13794–13803. [Google Scholar] [CrossRef]
- Loredo-García, E.; Ortiz-Dosal, A.; Núñez-Leyva, J.M.; Cuellar Camacho, J.L.; Alegría-Torres, J.A.; García-Torres, L.; Navarro-Contreras, H.R.; Kolosovas-Machuca, E.S. TNF-α detection using gold nanoparticles as a surface-enhanced Raman spectroscopy substrate. Nanomedicine 2021, 16, 51–61. [Google Scholar] [CrossRef] [PubMed]
- Perez-Mayen, L.; Oliva, J.; Salas, P.; De la Rosa, E. Nanomolar detection of glucose using SERS substrates fabricated with albumin coated gold nanoparticles. Nanoscale 2016, 8, 11862–11869. [Google Scholar] [CrossRef]
- Al-Saadi, A.A.; Haroon, M.; Popoola, S.A.; Saleh, T.A. Sensitive SERS detection and characterization of procaine in aqueous media by reduced gold nanoparticles. Sens. Actuators B Chem. 2020, 304, 127057. [Google Scholar] [CrossRef]

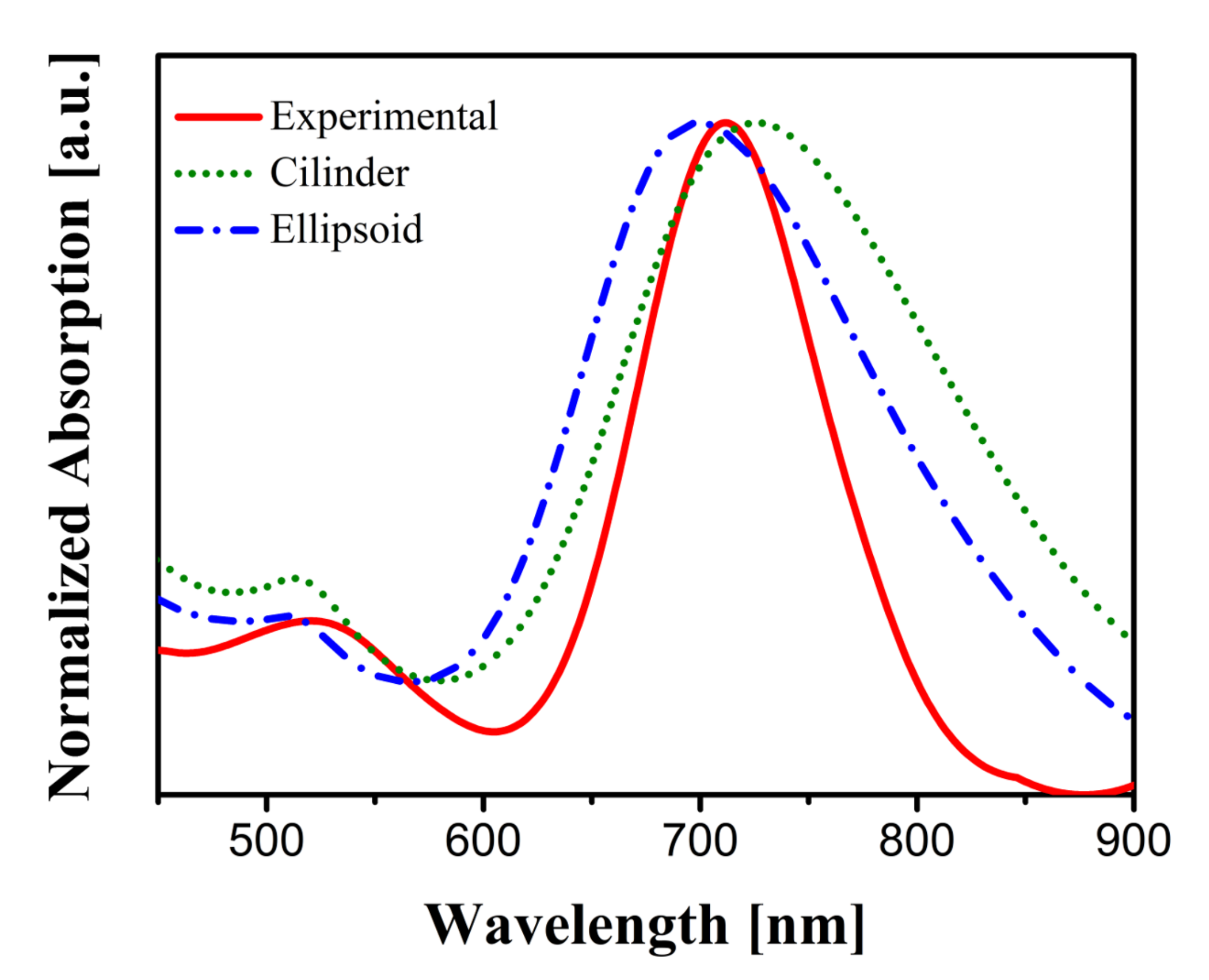
| Prolate Ellipsoid | Cylinder + Hemispheres | Experimental | |
|---|---|---|---|
| [nm] | 698 | 720 | 712 |
| [nm] | 509 | 512 | 521 |
| Prolate Ellipsoid | Cylinder + Hemispheres | |
|---|---|---|
| Longitudinal, | 0.9165 | 0.9799 |
| Transversal, | 0.8587 | 0.8647 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Núñez-Leyva, J.M.; Kolosovas-Machuca, E.S.; Sánchez, J.; Guevara, E.; Cuadrado, A.; Alda, J.; González, F.J. Computational and Experimental Analysis of Gold Nanorods in Terms of Their Morphology: Spectral Absorption and Local Field Enhancement. Nanomaterials 2021, 11, 1696. https://doi.org/10.3390/nano11071696
Núñez-Leyva JM, Kolosovas-Machuca ES, Sánchez J, Guevara E, Cuadrado A, Alda J, González FJ. Computational and Experimental Analysis of Gold Nanorods in Terms of Their Morphology: Spectral Absorption and Local Field Enhancement. Nanomaterials. 2021; 11(7):1696. https://doi.org/10.3390/nano11071696
Chicago/Turabian StyleNúñez-Leyva, Juan Manuel, Eleazar Samuel Kolosovas-Machuca, John Sánchez, Edgar Guevara, Alexander Cuadrado, Javier Alda, and Francisco Javier González. 2021. "Computational and Experimental Analysis of Gold Nanorods in Terms of Their Morphology: Spectral Absorption and Local Field Enhancement" Nanomaterials 11, no. 7: 1696. https://doi.org/10.3390/nano11071696
APA StyleNúñez-Leyva, J. M., Kolosovas-Machuca, E. S., Sánchez, J., Guevara, E., Cuadrado, A., Alda, J., & González, F. J. (2021). Computational and Experimental Analysis of Gold Nanorods in Terms of Their Morphology: Spectral Absorption and Local Field Enhancement. Nanomaterials, 11(7), 1696. https://doi.org/10.3390/nano11071696