Structural Properties of Molecular Sierpiński Triangle Fractals
Abstract
1. Introduction
2. Theoretical Background
2.1. Small-Angle Scattering Method
- when the length of the particle is scaled as ,
- when the particle is translated ,
- , when the particle consists of two non-overlapping subsets I and with surface areas and .
2.2. Form Factor and Scattering Intensity of a Triangle
3. Results and Discussion
3.1. ST Model
3.2. Extensions of ST Model
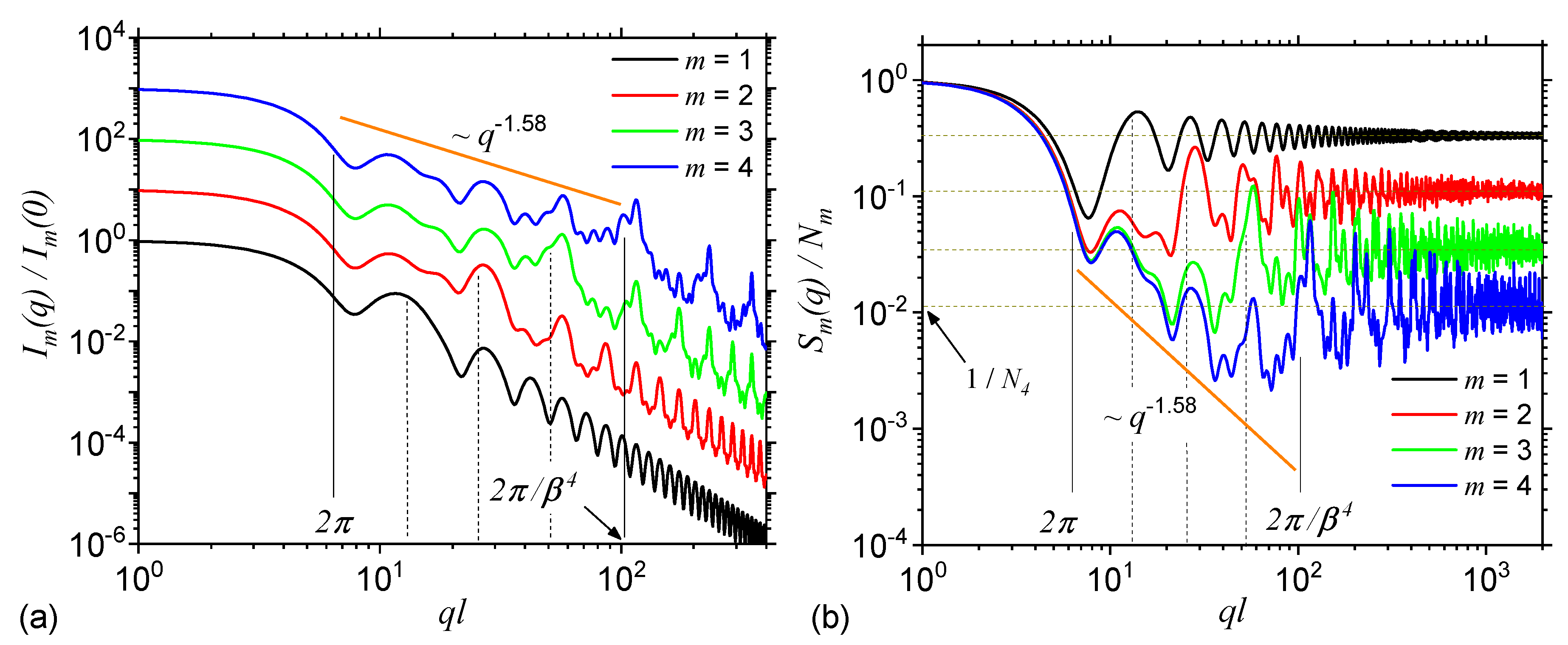
3.3. Structure and Form Factors
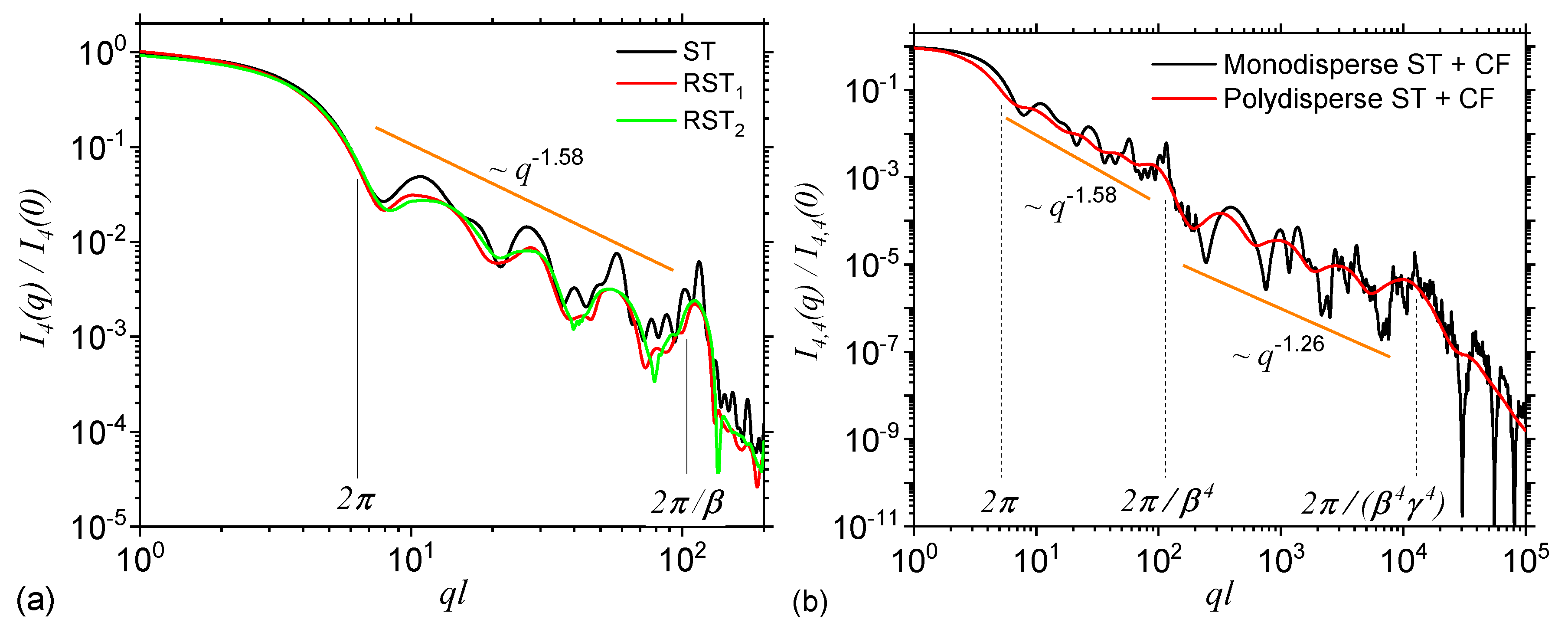
3.4. Influence of Various Sizes of the Basic Units Relative to the Distances Between Them, and of Polydispersity
3.5. Influence of Small Random Displacements and of the Shape of Basic Units
4. Conclusions
Funding
Conflicts of Interest
References
- Hasan, D.; Lee, C. A modified abstraction of Sierpiński fractals towards enhanced sensitivity of a cross-coupled bow-tie nanostructure. Nano Futures 2018, 2, 025005. [Google Scholar] [CrossRef]
- Wei, C.; He, Y.; Shi, X.; Song, Z. Terpyridine-metal complexes: Applications in catalysis and supramolecular chemistry. Coord. Chem. Rev. 2019, 385, 1–19. [Google Scholar] [CrossRef] [PubMed]
- Fan, J.; Yeo, W.; Su, Y.; Hattori, Y.; Lee, W.; Jung, S.H.; Zhang, Y.; Liu, Z.; Cheng, H.; Falgout, L.; et al. Fractal design concepts for stretchable electronics. Nat. Commun. 2014, 5, 3266. [Google Scholar] [CrossRef] [PubMed]
- Allouche, J.P.; Shallit, J. Automatic Sequences: Theory, Applications, Generalizations; Cambridge University Press: Cambridge, UK, 2003; p. 588. [Google Scholar]
- De Nicola, F.; Puthiya Purayil, N.S.; Spirito, D.; Miscuglio, M.; Tantussi, F.; Tomadin, A.; De Angelis, F.; Polini, M.; Krahne, R.; Pellegrini, V. Multiband Plasmonic Sierpinski Carpet Fractal Antennas. ACS Photonics 2018, 5, 2418–2425. [Google Scholar] [CrossRef]
- Malankowska, M.; Schlautmann, S.; Berenschot, E.J.W.; Tiggelaar, R.M.; Pina, M.P.; Mallada, R.; Tas, N.R.; Gardeniers, H. Three-Dimensional Fractal Geometry for Gas Permeation in Microchannels. Micromachines 2018, 9, 45. [Google Scholar] [CrossRef]
- Zhang, X.; Li, N.; Gu, G.C.; Wang, H.; Nieckarz, D.; Szabelski, P.; He, Y.; Wang, Y.; Xie, C.; Shen, Z.Y.; et al. Controlling Molecular Growth between Fractals and Crystals on Surfaces. ACS Nano 2015, 9, 11909–11915. [Google Scholar] [CrossRef]
- Wang, Y.; Xue, N.; Li, R.; Wu, T.; Li, N.; Hou, S.; Wang, Y. Construction and Properties of Sierpiński Triangular Fractals on Surfaces. Chem. Phys. Chem. 2019, 20, 2262–2270. [Google Scholar] [CrossRef]
- Zhang, X.; Li, N.; Liu, L.; Gu, G.; Li, C.; Tang, H.; Peng, L.; Hou, S.; Wang, Y. Robust Sierpiński triangle fractals on symmetry-mismatched Ag(100). Chem. Commun. 2016, 52, 10578–10581. [Google Scholar] [CrossRef]
- He, L.; Wang, S.C.; Lin, L.T.; Cai, J.Y.; Li, L.; Tu, T.H.; Chan, Y.T. Multicomponent Metallo-Supramolecular Nanocapsules Assembled from Calix[4]resorcinarene-Based Terpyridine Ligands. J. Am. Chem. Soc. 2020. [Google Scholar] [CrossRef]
- Cui, D.; Fang, Y.; MacLean, O.; Perepichka, D.F.; Rosei, F.; Clair, S. Covalent organic frameworks from a monomer with reduced symmetry: Polymorphism and Sierpiński triangles. Chem. Commun. 2019, 55, 13586–13589. [Google Scholar] [CrossRef]
- Sarkar, R.; Xie, T.Z.; Endres, K.J.; Wang, Z.; Moorefield, C.N.; Saunders, M.J.; Ghorai, S.; Patri, A.K.; Wesdemiotis, C.; Dobrynin, A.V.; et al. Sierpiński Pyramids by Molecular Entanglement. J. Am. Chem. Soc. 2020, 142, 5526–5530. [Google Scholar] [CrossRef]
- Zhang, X.; Li, R.; Li, N.; Gu, G.; Zhang, Y.; Hou, S.; Wang, Y. Sierpiński triangles formed by molecules with linear backbones on Au(111). Chin. Chem. Lett. 2018, 29, 967–969. [Google Scholar] [CrossRef]
- Kempkes, S.N.; Slot, M.R.; Freeney, S.E.; Zevenhuizen, S.J.M.; Vanmaekelbergh, D.; Swart, I.; Smith, C.M. Design and characterization of electrons in a fractal geometry. Nat. Phys. 2019, 15, 127–131. [Google Scholar] [CrossRef]
- Shang, J.; Wang, Y.; Chen, M.; Dai, J.; Zhou, X.; Kuttner, J.; Hilt, G.; Shao, X.; Gottfried, J.M.; Wu, K. Assembling molecular Sierpiński triangle fractals. Nat. Chem. 2015, 7, 389–393. [Google Scholar] [CrossRef]
- Li, C.; Zhang, X.; Li, N.; Wang, Y.; Yang, J.; Gu, G.; Zhang, Y.; Hou, S.; Peng, L.; Wu, K.; et al. Construction of Sierpiński Triangles up to the Fifth Order. J. Am. Chem. Soc. 2017, 139, 13749–13753. [Google Scholar] [CrossRef]
- Rastgoo-Lahrood, A.; Martsinovich, N.; Lischka, M.; Eichhorn, J.; Szabelski, P.; Nieckarz, D.; Strunskus, T.; Das, K.; Schmittel, M.; Heckl, W.M.; et al. From Au–Thiolate Chains to Thioether Sierpiński Triangles: The Versatile Surface Chemistry of 1,3,5-Tris(4-mercaptophenyl)benzene on Au(111). ACS Nano 2016, 10, 10901–10911. [Google Scholar] [CrossRef]
- Vicsek, T. Fractal Growth Phenomena, 2nd ed.; World Scientific Publishing: Singapore, 1992; p. 510. [Google Scholar]
- Edgar, G.A. Classics On Fractals (Studies in Nonlinearity); Routledge: New York, NY, USA, 1993; p. 384. [Google Scholar]
- Anitas, E.M.; Slyamov, A.; Szakacs, Z. Microstructural characterization of surface fractals using small-angle scattering. Rom. J. Phys. 2018, 63, 104. [Google Scholar]
- Anitas, E.M.; Slyamov, A.; Todoran, R.; Szakacs, Z. Small-Angle Scattering from Nanoscale Fat Fractals. Nanoscale Res. Lett. 2017, 12, 389. [Google Scholar] [CrossRef]
- Feigin, L.A.; Svergun, D.I. Structure Analysis by Small-Angle X-ray and Neutron Scattering; Springer US: Boston, MA, USA, 1987; p. 335. [Google Scholar] [CrossRef]
- Cherny, A.Y.; Anitas, E.M.; Osipov, V.A.; Kuklin, A.I. Deterministic fractals: Extracting additional information from small-angle scattering data. Phys. Rev. E 2011, 84, 036203. [Google Scholar] [CrossRef]
- Porod, G. Die Röntgenkleinwinkelstreuung von dichtgepackten kolloiden Systemen. Kolloid-Zeitschrift 1951, 124, 83–114. [Google Scholar] [CrossRef]
- Cherny, A.Y.; Anitas, E.M.; Osipov, V.A.; Kuklin, A.I. Small-angle scattering from the Cantor surface fractal on the plane and the Koch snowflake. Phys. Chem. Chem. Phys. 2017, 19, 2261–2268. [Google Scholar] [CrossRef] [PubMed]
- Anitas, E.M.; Slyamov, A. Structural characterization of chaos game fractals using small-angle scattering analysis. PLoS ONE 2017, 12, e181385. [Google Scholar] [CrossRef] [PubMed]
- Anitas, E.M. Small-Angle Scattering from Mass and Surface Fractals. In Bifurcations, Solitons and Fractals; Ricardo, L.L., Ed.; Intechopen: London, UK, 2017; Chapter 10. [Google Scholar]
- Peitgen, H.O.; Jürgens, H.; Saupe, D. Chaos and Fractals: New Frontiers of Science, 2nd ed.; Springer: New York, NY, USA, 2004; p. 864. [Google Scholar]
- Hammouda, B. A new Guinier–Porod model. J. Appl. Cryst. 2010, 43, 716–719. [Google Scholar] [CrossRef]
- Pedersen, J.S. Resolution effects and analysis of small-angle neutron scattering data. J. Phys. IV Fr. 1993, 3, 491–498. [Google Scholar] [CrossRef][Green Version]
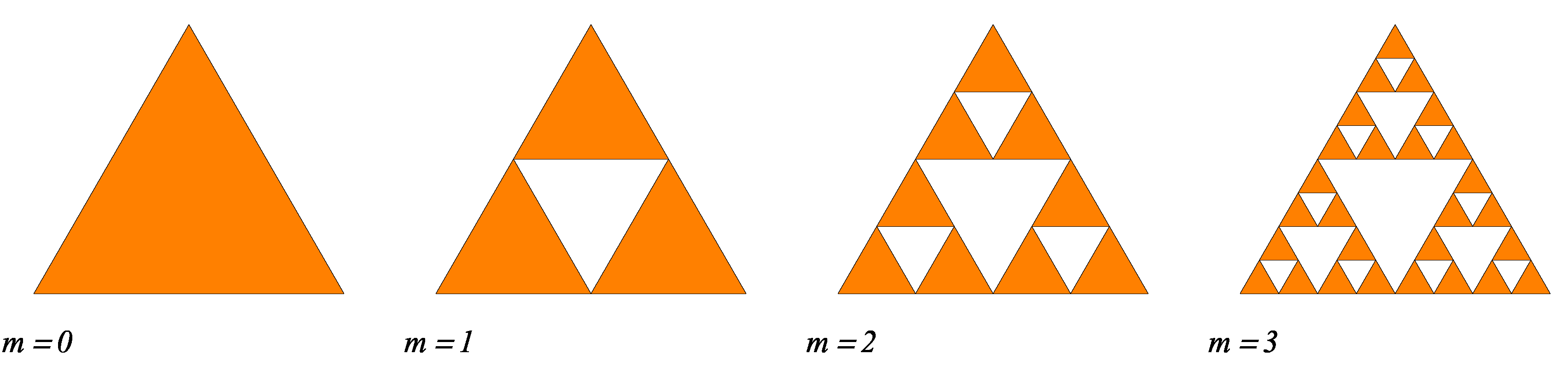
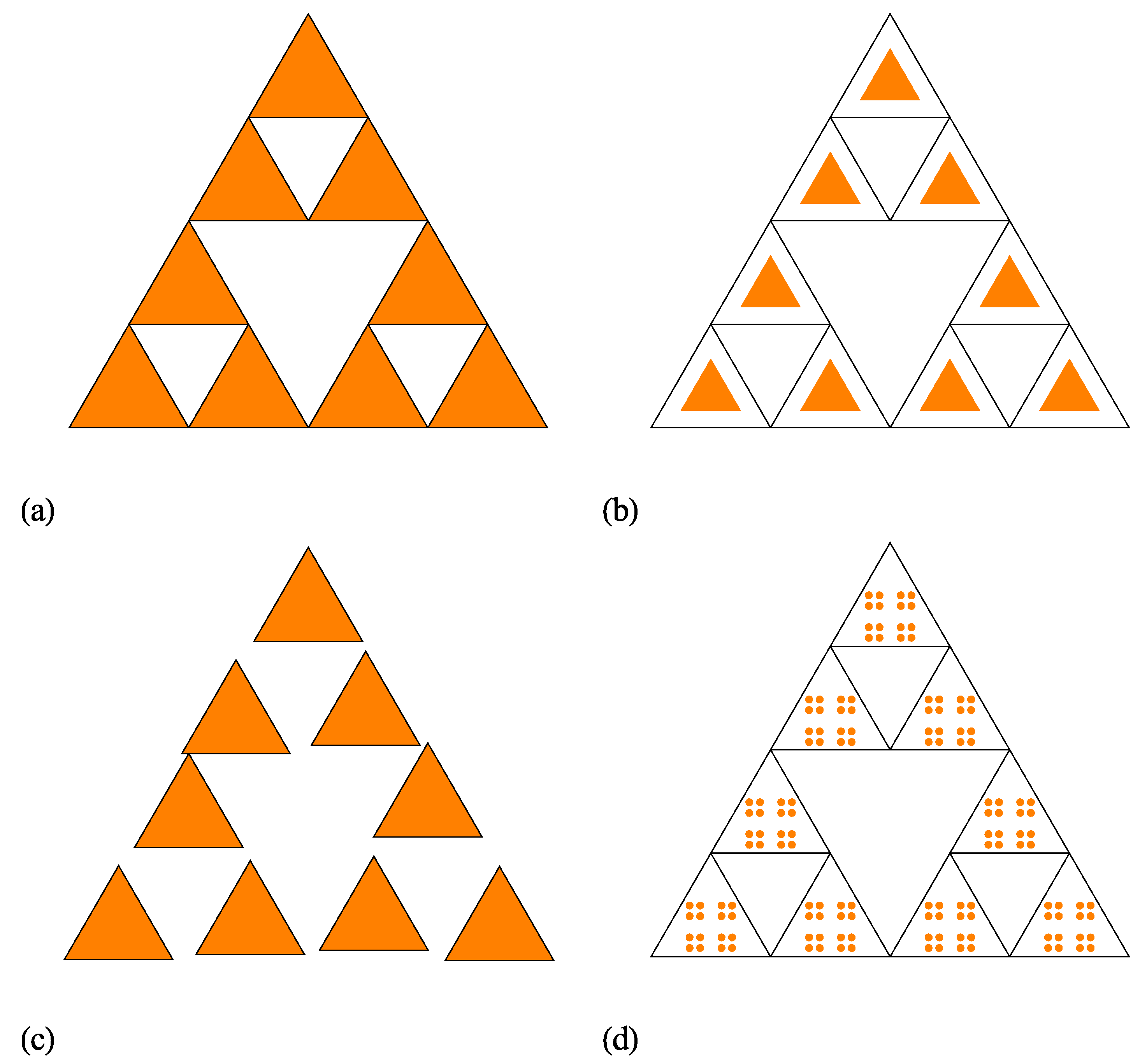

© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anitas, E.M. Structural Properties of Molecular Sierpiński Triangle Fractals. Nanomaterials 2020, 10, 925. https://doi.org/10.3390/nano10050925
Anitas EM. Structural Properties of Molecular Sierpiński Triangle Fractals. Nanomaterials. 2020; 10(5):925. https://doi.org/10.3390/nano10050925
Chicago/Turabian StyleAnitas, Eugen Mircea. 2020. "Structural Properties of Molecular Sierpiński Triangle Fractals" Nanomaterials 10, no. 5: 925. https://doi.org/10.3390/nano10050925
APA StyleAnitas, E. M. (2020). Structural Properties of Molecular Sierpiński Triangle Fractals. Nanomaterials, 10(5), 925. https://doi.org/10.3390/nano10050925




