Simulated Performance of a Xenohybrid Bone Graft (SmartBone®) in the Treatment of Acetabular Prosthetic Reconstruction
Abstract
1. Introduction
2. Results
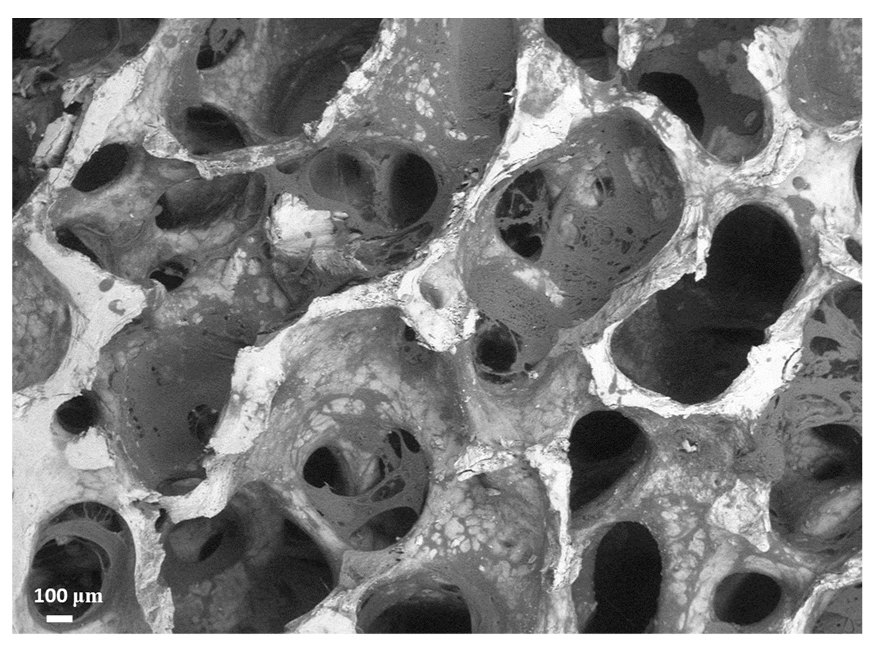
2.1. Mechanical Characteristics of SmartBone®
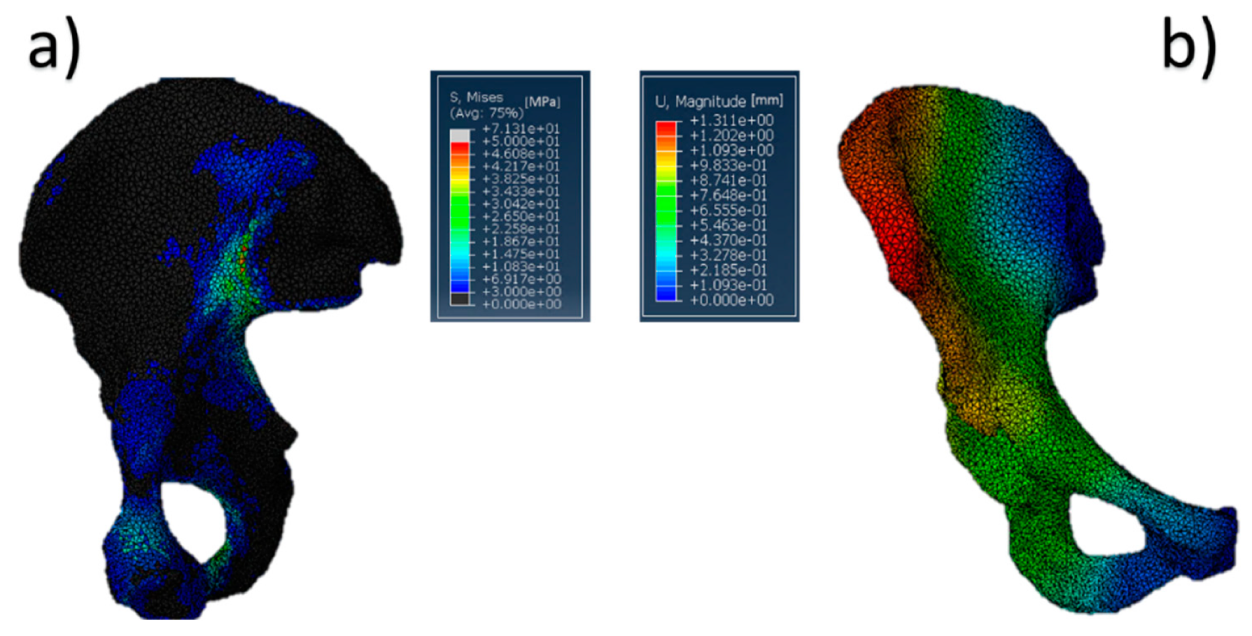
2.2. Pathological Model
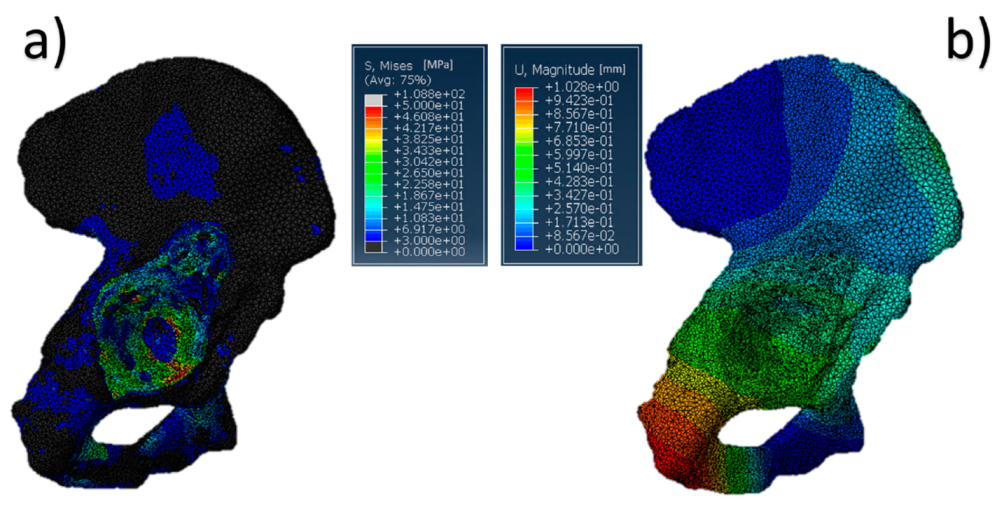
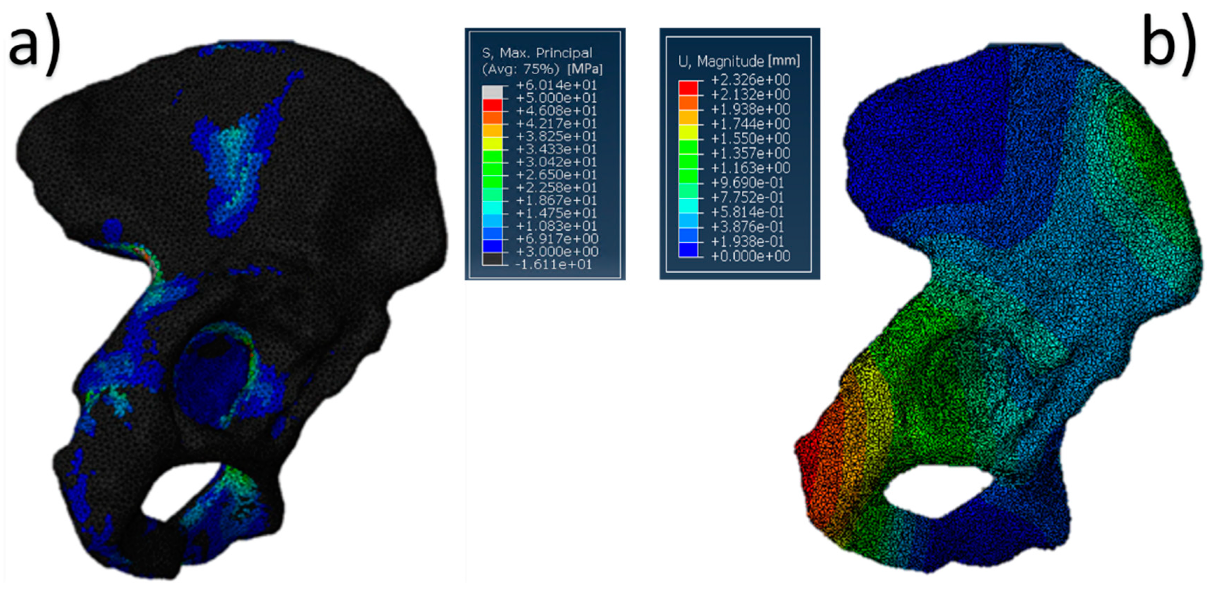
2.3. Pathological Model Treated with SmartBone® Graft
2.4. Comparison among Models
3. Discussion
4. Materials and Methods
4.1. Mechanical Characterization
4.1.1. Sample Preparation
4.1.2. Testing Procedures
4.2. ESEM Analysis
4.3. Models
4.3.1. Development of 3D Physiological Pelvis Model
Assigned Material Properties
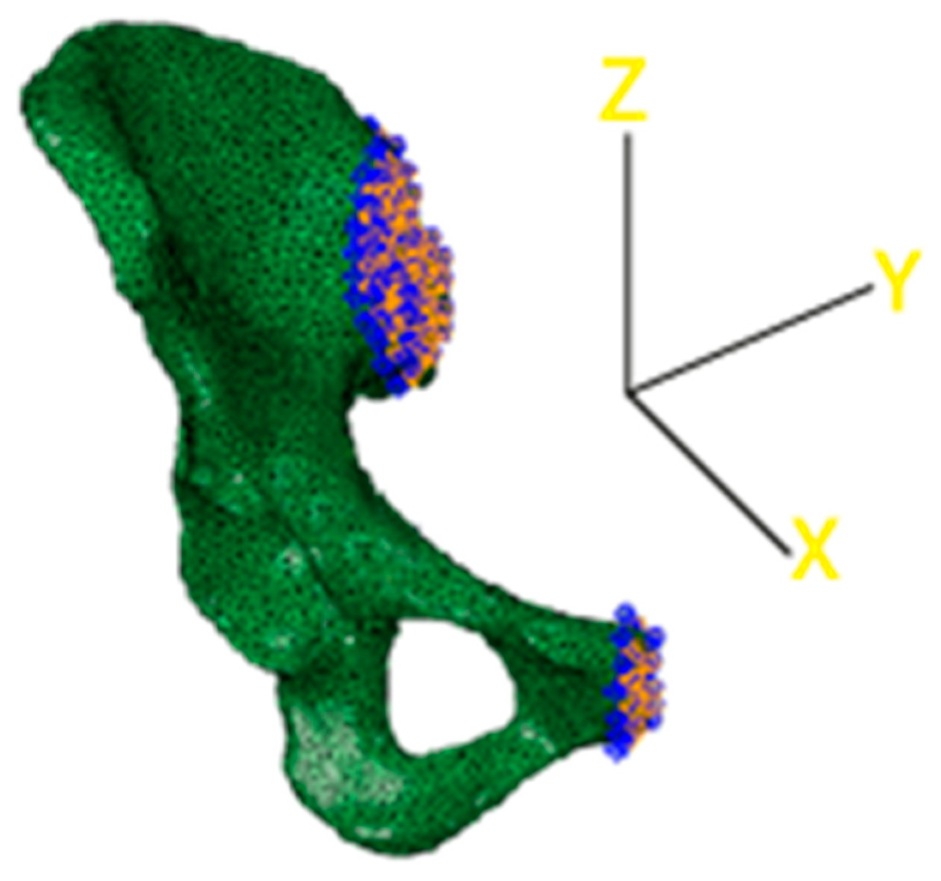
Load Applications, Constraint Definitions, and Boundary Conditions
Behavior of the Physiological Model
4.3.2. Development of the 3D Pelvis Pathological Models
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kurtz, S.; Ong, K.; Lau, E.; Mowat, F.; Halpern, M. Projections of primary and revision hip and knee arthroplasty in the United States from 2005 to 2030. J. Bone Jt. Surg. Am. 2007, 89, 780–785. [Google Scholar] [CrossRef] [PubMed]
- Aynardi, M.; Post, Z.; Ong, A.; Orozco, F.; Sukin, D.C. Outpatient Surgery as a Means of Cost Reduction in Total Hip Arthroplasty: A Case-Control Study. HSS J. 2014, 10, 252–255. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.T.; Ahn, J.-M.; Hur, J.-O.; Lee, J.-S.; Cheon, S.-J. Reconstruction of Acetabular Posterior Wall Fractures. Clin. Orthop. Surg. 2011, 3, 114. [Google Scholar] [CrossRef] [PubMed]
- Logan, Z.S.; Redmond, J.M.; Spelsberg, S.C.; Jackson, T.J.; Domb, B.G. Chondral Lesion of the Hip. Clin. Sports Med. 2016, 35, 361–372. [Google Scholar] [CrossRef] [PubMed]
- Beck, M.; Kalhor, M.; Leunig, M.; Ganz, R. Hip morphology influences the pattern of damage to the acetabular cartilage. J. Bone Joint Surg. Br. 2005, 87-B, 1012–1018. [Google Scholar] [CrossRef]
- Beaule, P.E.; Grammatopoulos, G.; Speirs, A.; Ng, K.C.G.; Carsen, S.; Frei, H.; Melkus, G.; Rakhra, K.; Lamontagne, M. Unravelling the hip pistol grip/cam deformity: Origins to joint degeneration. J. Orthop. Res. 2018, 36, 3125–3135. [Google Scholar] [CrossRef]
- Tsertsvadze, A.; Grove, A.; Freeman, K.; Court, R.; Johnson, S.; Connock, M.; Clarke, A.; Sutcliffe, P. Total hip replacement for the treatment of end stage arthritis of the hip: A systematic review and meta-analysis. PLoS ONE 2014, 9, e99804. [Google Scholar] [CrossRef]
- Chakravarty, R.; Toossi, N.; Katsman, A.; Cerynik, D.L.; Harding, S.P. Percutaneous column fixation and total hip arthroplasty for the treatment of acute acetabular fracture in the elderly. J. Arthroplasty 2014, 29, 817–821. [Google Scholar] [CrossRef]
- Borens, O.; Wettstein, M.; Garofalo, R.; Blanc, C.H.; Kombot, C.; Leyvraz, P.F.; Mouhsine, E. Die behandlung von acetabulumfrakturen bei geriatrischen patienten mittels modifizierter kabelcerclage und primärer hüfttotalprothese. Erste ergebnisse. Unfallchirurg 2004, 107, 1050–1056. [Google Scholar] [CrossRef] [PubMed]
- Boraiah, S.; Ragsdale, M.; Achor, T.; Zelicof, S.; Asprinio, D.E. Open reduction internal fixation and primary total hip arthroplasty of selected acetabular fractures. J. Orthop. Trauma 2009, 23, 243–248. [Google Scholar] [CrossRef] [PubMed]
- Herscovici, D.; Lindvall, E.; Bolhofner, B.; Scaduto, J.M. The combined hip procedure: Open reduction internal fixation combined with total hip arthroplasty for the management of acetabular fractures in the elderly. J. Orthop. Trauma 2010, 24, 291–296. [Google Scholar] [CrossRef] [PubMed]
- Malhotra, R.; Singh, D.P.; Jain, V.; Kumar, V.; Singh, R. Acute total hip arthroplasty in acetabular fractures in the elderly using the Octopus System: Mid term to long term follow-up. J. Arthroplasty 2013, 28, 1005–1009. [Google Scholar] [CrossRef] [PubMed]
- Kobayashi, S.; Saito, N.; Nawata, M.; Horiuchi, H.; Iorio, R.; Takaoka, K. Total hip arthroplasty with bulk femoral head autograft for acetabular reconstruction in developmental dysplasia of the hip. J. Bone Jt. Surg. Am. 2003, 85, 615–621. [Google Scholar] [CrossRef] [PubMed]
- Capone, A.; Peri, M.; Mastio, M. Surgical treatment of acetabular fractures in the elderly: A systematic review of the results. EFORT Open Rev. 2017, 2, 97–103. [Google Scholar] [CrossRef]
- Flivik, G. Fixation of the cemented acetabular component in hip arthroplasty. Acta Orthop. 2005, 76, 1–30. [Google Scholar] [CrossRef]
- Kelley, S.S. High hip center in revision arthroplasty. J. Arthroplast. 1994, 5, 503–510. [Google Scholar] [CrossRef]
- Khatod, M.; Cafri, G.; Namba, R.S.; Inacio, M.C.S.; Paxton, E.W. Risk Factors for Total Hip Arthroplasty Aseptic Revision. J. Arthroplasty 2014, 29, 1412–1417. [Google Scholar] [CrossRef]
- Khatod, D.; Cafri, G.; Inacio, M.C.; Schepps, A.L.; Paxton, E.W.; Bini, S.A. Revision total hip arthoplasty: Factors associated with re-revision surgery. J. Bone Jt. Surgery Am. Vol. 2015, 97, 359–366. [Google Scholar] [CrossRef]
- Pope, D.; Blankenship, S.; Jones, G.; Robinson, B.S.; Maloney, W.J.; Paprosky, W.G. Maximizing function and outcomes in acetabular reconstruction: Segmental bony defects and pelvic discontinuity. Instr. Course Lect. 2014, 63, 187–197. [Google Scholar]
- Oommen, A.T.; Krishnamoorthy, V.P.; Poonnoose, P.M.; Korula, R.J. Fate of bone grafting for acetabular defects in total hip replacement. Indian J. Orthop. 2015, 49, 181. [Google Scholar] [CrossRef]
- Uchiyama, K.; Inoue, G.; Takahira, N.; Takaso, M. Revision total hip arthroplasty—Salvage procedures using bone allografts in Japan. J. Orthop. Sci. 2017, 22, 593–600. [Google Scholar] [CrossRef] [PubMed]
- Ahumada, M.; Jacques, E.; Calderon, C.; Martinez-gomez, F. porosity in biomaterials: A key factor in the development of applied materials in biomedicine. In Handbook of Ecomaterials; Springer International: Cham, Switzerland, 2018; pp. 1–20. ISBN 9783319482811. [Google Scholar]
- Merola, M.; Affatato, S. Materials for hip prostheses: A review of wear and loading considerations. Materials 2019, 12, 495. [Google Scholar] [CrossRef] [PubMed]
- Mour, M.; Das, D.; Winkler, T.; Hoenig, E.; Mielke, G.; Morlock, M.M.; Schilling, A.F. Advances in porous biomaterials for dental and orthopaedic applications. Materials 2010, 3, 2947–2974. [Google Scholar] [CrossRef]
- Khan, F.; Tanaka, M.; Ahmad, S.R. Fabrication of polymeric biomaterials: A strategy for tissue engineering and medical devices. J. Mater. Chem. B 2015, 3, 8224–8249. [Google Scholar] [CrossRef]
- Winkler, T.; Sass, F.A.; Duda, G.N.; Schmidt-Bleek, K. A review of biomaterials in bone defect healing, remaining shortcomings and future opportunities for bone tissue engineering. Bone Joint Res. 2018, 7, 232–243. [Google Scholar] [CrossRef] [PubMed]
- Cingolani, A.; Cuccato, D.; Storti, G.; Morbidelli, M. Control of Pore Structure in Polymeric Monoliths Prepared from Colloidal Dispersions. Macromol. Mater. Eng. 2018, 303, 1700417. [Google Scholar] [CrossRef]
- Babaie, E.; Bhaduri, S.B. Fabrication aspects of porous biomaterials in orthopedic applications: A Review. ACS Biomater. Sci. Eng. 2018, 4, 1–39. [Google Scholar] [CrossRef]
- Wers, E.; Lefeuvre, B.; Pellen-mussi, P.; Novella, A.; Oudadesse, H. New method of synthesis and in vitro studies of a porous biomaterial. Mater. Sci. Eng. C 2016, 61, 133–142. [Google Scholar] [CrossRef]
- Bohner, M. Resorbable biomaterials as bone graft substitutes. Mater. Today 2010, 13, 24–30. [Google Scholar] [CrossRef]
- Zhang, K.; Wang, S.; Zhou, C.; Cheng, L.; Gao, X.; Xie, X.; Sun, J.; Wang, H.; Weir, M.D.; Reynolds, M.A.; et al. Advanced smart biomaterials and constructs for hard tissue engineering and regeneration. Bone Res. 2018, 6. [Google Scholar] [CrossRef]
- Fernandez de Grado, G.; Keller, L.; Idoux-Gillet, Y.; Wagner, Q.; Musset, A.-M.; Benkirane-Jessel, N.; Bornert, F.; Offner, D. Bone substitutes: A review of their characteristics, clinical use, and perspectives for large bone defects management. J. Tissue Eng. 2018, 9, 204173141877681. [Google Scholar] [CrossRef] [PubMed]
- Cingolani, A.; Grottoli, C.F.; Esposito, R.; Villa, T.; Rossi, F.; Perale, G. Improving bovine bone mechanical characteristics for the development of xenohybrid bone grafts. Curr. Pharm. Biotechnol. 2018, 19, 1005–1013. [Google Scholar] [CrossRef] [PubMed]
- Blokhuis, T.J.; Arts, J.J.C. Bioactive and osteoinductive bone graft substitutes: Definitions, facts and myths. Injury 2011, 42, S26–S29. [Google Scholar] [CrossRef]
- Haugen, H.J.; Lyngstadaas, S.P.; Rossi, F.; Perale, G. Bone grafts: Which is the ideal biomaterial? J. Clin. Periodontol. 2019, 46, 92–102. [Google Scholar] [CrossRef] [PubMed]
- Sheikh, Z.; Sima, C.; Glogauer, M. Bone replacement materials and techniques used for achieving vertical alveolar bone augmentation. Materials 2015, 8, 2953–2993. [Google Scholar] [CrossRef]
- Amini, A.R.; Laurencin, C.T.; Nukavarapu, S.P. Bone tissue engineering: Recent advances and challenges. Crit. Rev. Biomed. Eng. 2012, 40, 363–408. [Google Scholar] [CrossRef] [PubMed]
- D’Alessandro, D.; Perale, G.; Milazzo, M.; Moscato, S.; Stefanini, C.; Pertici, G.; Danti, S. Bovine bone matrix/poly(L-lactic-co-ε-caprolactone)/gelatin hybrid scaffold (SmartBone®) for maxillary sinus augmentation: A histologic study on bone regeneration. Int. J. Pharm. 2017, 523, 534–544. [Google Scholar] [CrossRef]
- Stacchi, C.; Lombardi, T.; Ottonelli, R.; Berton, F.; Perinetti, G.; Traini, T. New bone formation after transcrestal sinus floor elevation was influenced by sinus cavity dimensions: A prospective histologic and histomorphometric study. Clin. Oral Implants Res. 2018, 29, 465–479. [Google Scholar] [CrossRef]
- Spinato, S.; Galindo-Moreno, P.; Bernardello, F.; Zaffe, D. Minimum abutment height to eliminate bone loss: Influence of implant neck design and platform switching. Int. J. Oral Maxillofac. Implants 2018, 33, 405–411. [Google Scholar] [CrossRef]
- Grottoli, C.; Ferracini, R.; Compagno, M.; Tombolesi, A.; Rampado, O.; Pilone, L.; Bistolfi, A.; Borre, A.; Cingolani, A.; Perale, G. A radiological approach to evaluate bone graft integration in reconstructive surgeries. Appl. Sci. 2019, 9, 1469. [Google Scholar] [CrossRef]
- Abuelnaga, M.; Elbokle, N.; Khashaba, M. Evaluation of custom made xenogenic bone grafts in mandibular alveolar ridge augmentation versus particulate bone graft with titanium mesh. Egypt. J. Oral Maxillofac. Surg. 2018, 9, 62–73. [Google Scholar] [CrossRef]
- Roato, I.; Belisario, D.C.; Compagno, M.; Lena, A.; Bistolfi, A.; Maccari, L.; Mussano, F.; Genova, T.; Godio, L.; Perale, G.; et al. Concentrated adipose tissue infusion for the treatment of knee osteoarthritis: Clinical and histological observations. Int. Orthop. 2018, 43, 15–23. [Google Scholar] [CrossRef] [PubMed]
- Piana, R.; Boffano, M.; Pellegrino, P.; Ratto, N.; Rossi, L.; Perale, G.; Division, O.O.; Hospital, C.T.O.; Citta, A.O.U. Treating femoral chondrosarcoma with high mechanical performances bio-hybrid bone graft: A case report. In Materials Science and Engineering-Darmstadt 26th–28th September 2018; DGM: Sankt Augustin, Germany, 2018. [Google Scholar]
- Ferracini, R.; Bistolfi, A.; Garibaldi, R.; Furfaro, V.; Battista, A.; Perale, G. Composite xenohybrid bovine bone-derived scaffold as bone substitute for the treatment of tibial plateau fractures. Appl. Sci. 2019, 9, 2675. [Google Scholar] [CrossRef]
- Facciuto, E.; Grottoli, C.F.; Mattarocci, M.; Illiano, F.; Compagno, M.; Ferrari, R.; Perale, G. Three-dimensional craniofacial bone reconstraction with smartbone on demand. J. Craniofac. Surg. 2019, 30, 739–741. [Google Scholar] [CrossRef]
- Chen, P.Y.; McKittrick, J. Compressive mechanical properties of demineralized and deproteinized cancellous bone. J. Mech. Behav. Biomed. Mater. 2011, 4, 961–973. [Google Scholar] [CrossRef]
- Chen, P.Y.; McKittrick, J.; Meyers, M.A. Biological materials: Functional adaptations and bioinspired designs. Prog. Mater. Sci. 2012, 57, 1492–1704. [Google Scholar] [CrossRef]
- Cingolani, A.; Casalini, T.; Caimi, S.; Klaue, A.; Sponchioni, M.; Rossi, F.; Perale, G. A methodologic approach for the selection of bio-resorbable polymers in the development of medical devices: The case of poly(l-lactide-co-ε-caprolactone). Polymers 2018, 10, 851. [Google Scholar] [CrossRef]
- Hooten, J.P.; Engh, C.A.; Engh, C.A. Failure of structural acetabular allografts in cementless revision hip arthroplasty. J. Bone Jt. Surg. Ser. B 1994, 76, 419–422. [Google Scholar] [CrossRef]
- Roato, I.; Belisario, D.C.; Compagno, M.; Verderio, L.; Sighinolfi, A.; Mussano, F.; Genova, T.; Veneziano, F.; Pertici, G.; Perale, G.; et al. Adipose-derived stromal vascular fraction/xenohybrid bone scaffold: An alternative source for bone regeneration. Stem Cells Int. 2018, 2018, 4126379. [Google Scholar] [CrossRef]
- Dalstra, M.; Huiskes, R.; van Erning, L. Development and validation of a three-dimensional finite element model of the pelvic bone. J. Biomech. Eng. 1995, 117, 272–278. [Google Scholar] [CrossRef]
- Dalstra, M.; Huiskes, R. Load Transfer Across the Pelvic. J. Biomech. 1995, 28, 715–724. [Google Scholar] [CrossRef]
- Available online: https://medicine.uiowa.edu/mri/facility-resources/images/visible-human-project-ct-datasets (accessed on 1 December 2017).
- Schreiber, J.J.; Anderson, P.A.; Hsu, W.K. Use of computed tomography for assessing bone mineral density. Neurosurg. Focus 2014, 37, E4. [Google Scholar] [CrossRef] [PubMed]
- Ciarelli, M.J.; Goldstein, S.A.; Kuhn, J.L.; Cody, D.D.; Brown, M.B. Evaluation of orthogonal mechanical properties and density of human trabecular bone from the major metaphyseal regions with materials testing and computed tomography. J. Orthop. Res. 1991, 9, 674–682. [Google Scholar] [CrossRef] [PubMed]
- Shu, Y.; Wen-Chuan, L.; Chang-Hung, C.; Cheng-Kung, H.; Chan, C.K.; Chang, T.K. The elastic modulus and Poisson’s ratio of cortical bone, cancellous bone, cartilage, meniscus and four ligaments. PLoS ONE Dataset 2015. [Google Scholar] [CrossRef]
- Gollwitzer, H.; Suren, C.; Strüwind, C.; Gottschling, H.; Schroder, M.; Gerdesmeyer, L.; Prodinger, P.M.; Burgkart, R. The natural alpha angle of the femoral headneck junction. Bone Jt. J. 2018, 100B, 570–578. [Google Scholar] [CrossRef]
- Dostal, W.F.; Andrews, J.G. A three-dimensional biomechanical model of hip musculature. J. Biomech. 1981, 14. [Google Scholar] [CrossRef]



| Test | Max Stress (MPa) | Max Strain (-) | Elastic Modulus (GPa) |
|---|---|---|---|
| Compression | 25.8 + 7.9 | 0.024 + 0.005 | 1.2456 + 0.2259 |
| Bending | 23.8 + 4.2 | 0.0765 + 0.009 | 0.3406 + 0.063 |
| Torsion | 25.5 + 4.4 | 0.058 + 0.009 | 0.4906 + 0.1037 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grottoli, C.F.; Cingolani, A.; Zambon, F.; Ferracini, R.; Villa, T.; Perale, G. Simulated Performance of a Xenohybrid Bone Graft (SmartBone®) in the Treatment of Acetabular Prosthetic Reconstruction. J. Funct. Biomater. 2019, 10, 53. https://doi.org/10.3390/jfb10040053
Grottoli CF, Cingolani A, Zambon F, Ferracini R, Villa T, Perale G. Simulated Performance of a Xenohybrid Bone Graft (SmartBone®) in the Treatment of Acetabular Prosthetic Reconstruction. Journal of Functional Biomaterials. 2019; 10(4):53. https://doi.org/10.3390/jfb10040053
Chicago/Turabian StyleGrottoli, Carlo Francesco, Alberto Cingolani, Fabio Zambon, Riccardo Ferracini, Tomaso Villa, and Giuseppe Perale. 2019. "Simulated Performance of a Xenohybrid Bone Graft (SmartBone®) in the Treatment of Acetabular Prosthetic Reconstruction" Journal of Functional Biomaterials 10, no. 4: 53. https://doi.org/10.3390/jfb10040053
APA StyleGrottoli, C. F., Cingolani, A., Zambon, F., Ferracini, R., Villa, T., & Perale, G. (2019). Simulated Performance of a Xenohybrid Bone Graft (SmartBone®) in the Treatment of Acetabular Prosthetic Reconstruction. Journal of Functional Biomaterials, 10(4), 53. https://doi.org/10.3390/jfb10040053






