Evaluation of a Moisture Diffusion Model for Analyzing the Convective Drying Kinetics of Lavandula x allardii Leaves
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials, Drying Experiments and Equipment
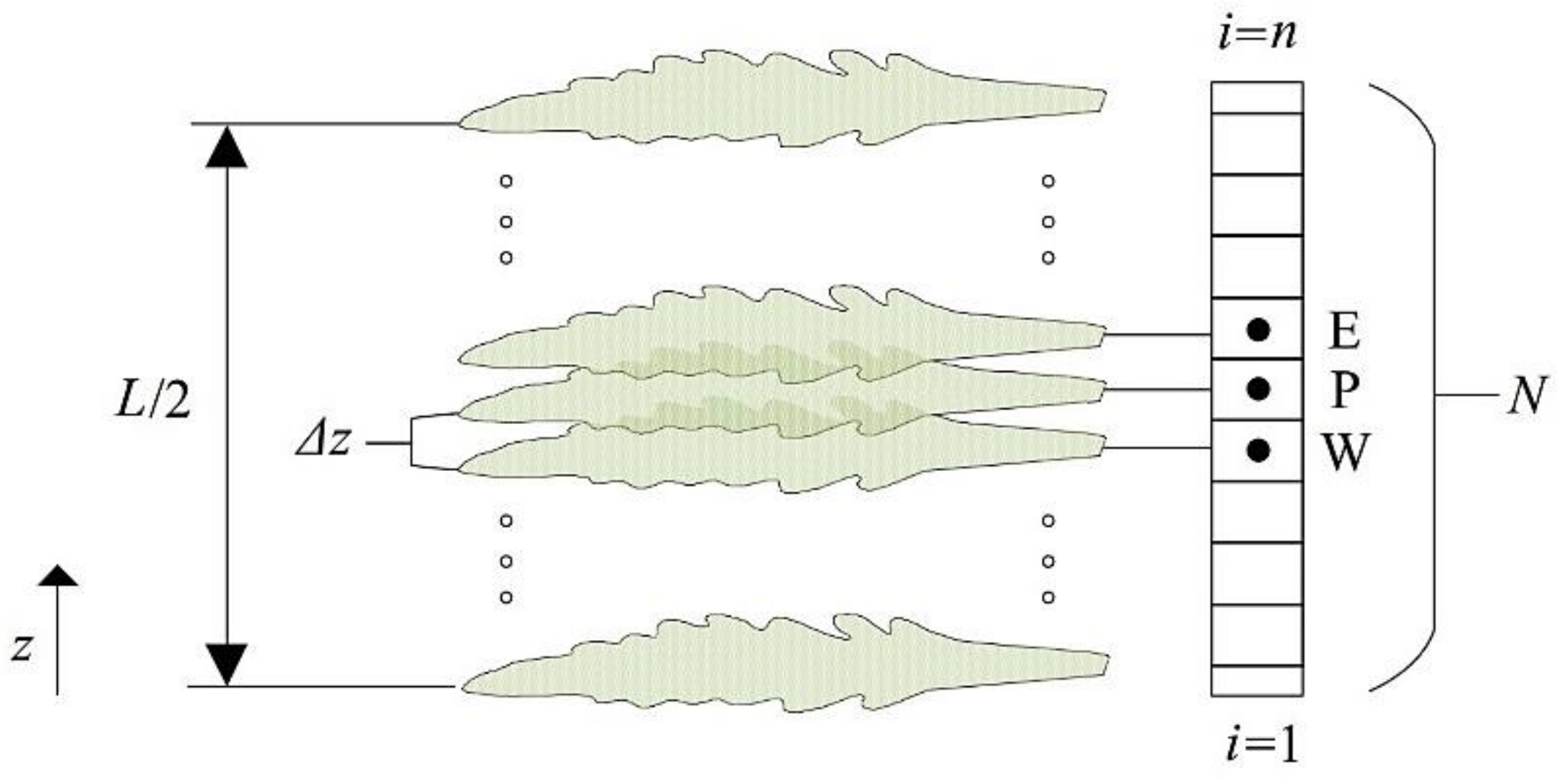
2.2. Formulation of One-Dimensional Diffusion Model
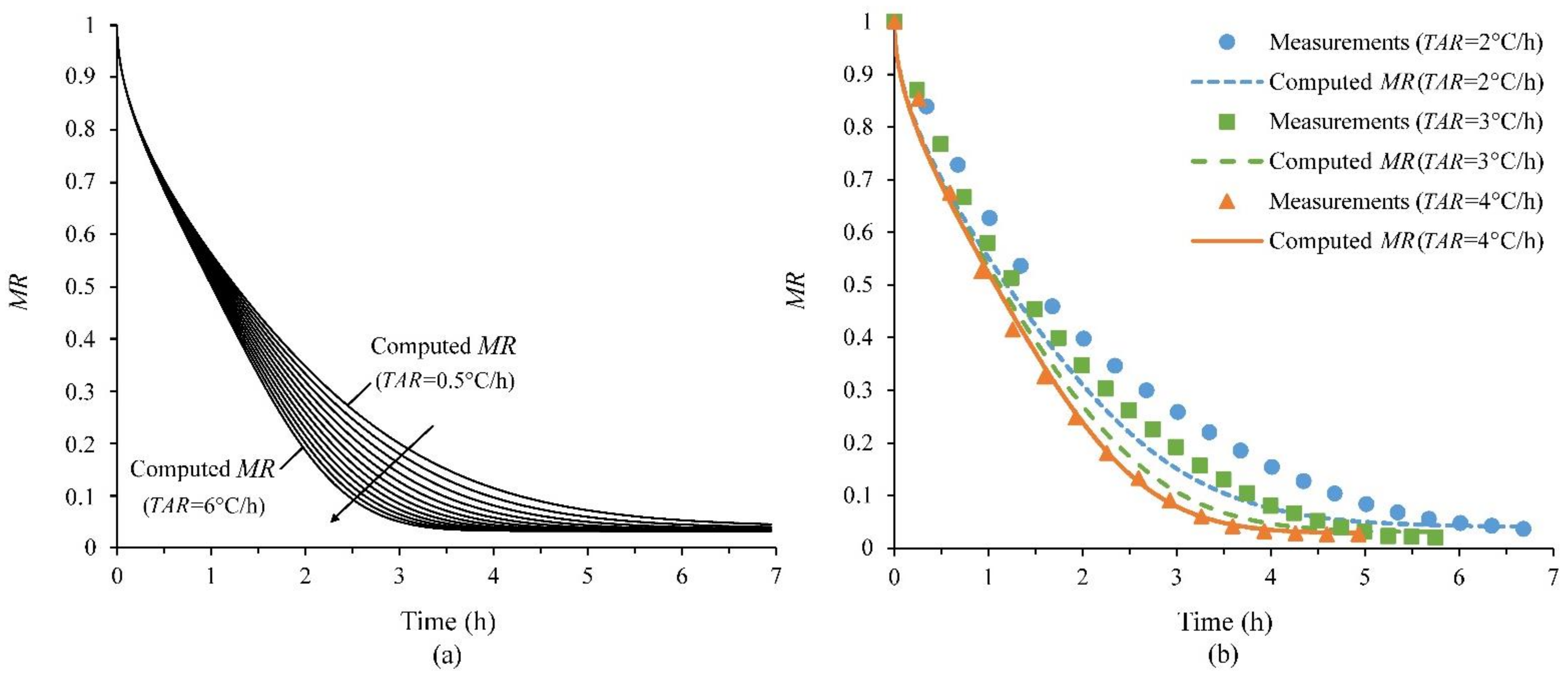
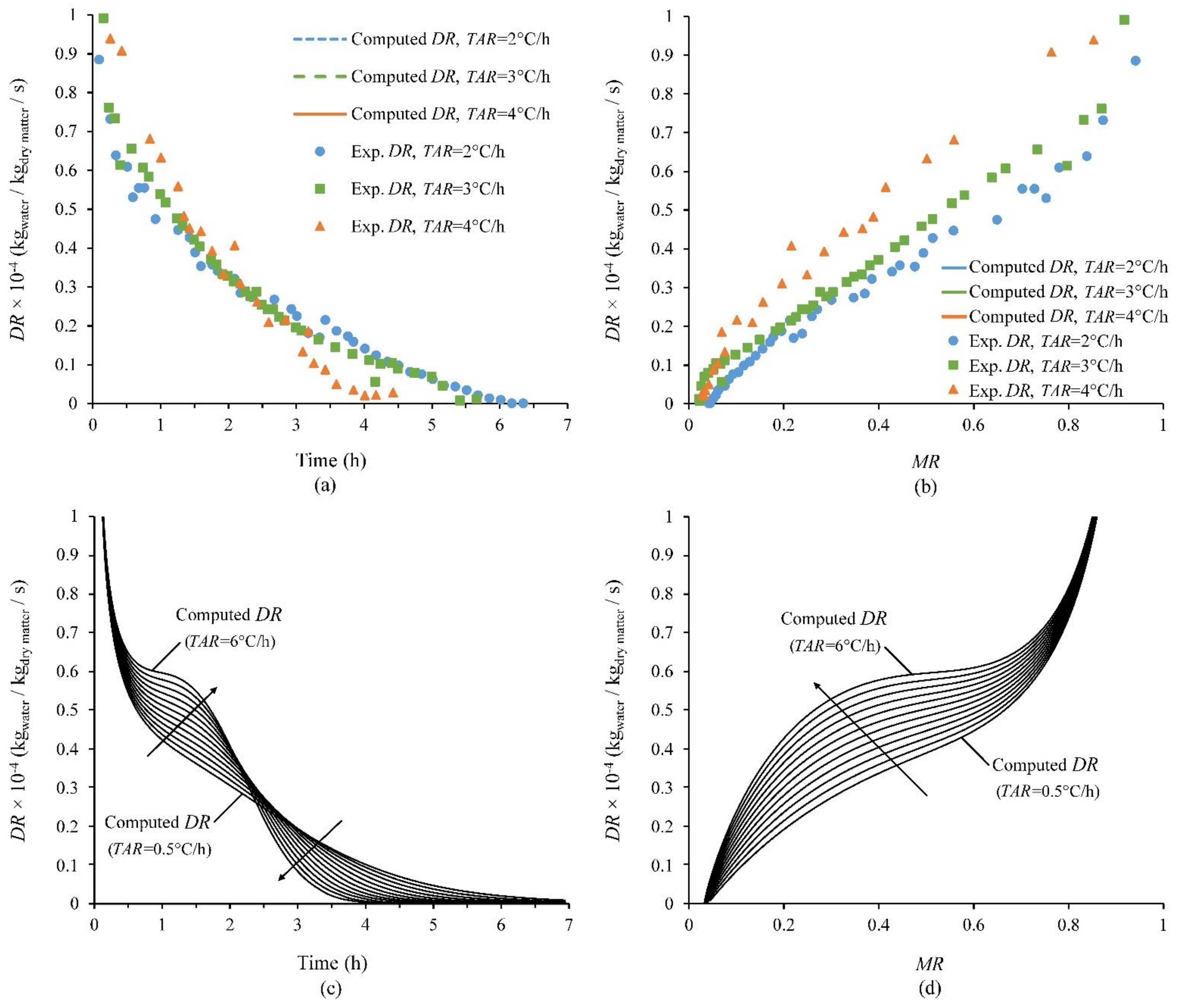
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Defraeye, T. Towards more efficient intermittent drying of fruit: Insights from combined hygrothermal-quality modelling. Innov. Food Sci. Emerg. Technol. 2016, 38, 262–271. [Google Scholar] [CrossRef] [Green Version]
- Metin Ozguven, M.; Tarhan, S.; Polatci, H.; Telci, I. A New Way to Improve the Drying Kinetics and Final Quality of Peppermint. J. Essent. Oil Bear. Plants 2016, 19, 1368–1379. [Google Scholar] [CrossRef]
- Cuervo-Andrade, S.P.; Hensel, O. Stepwise drying of medicinal plants as alternative to reduce time and energy processing. IOP Conf. Ser. Mater. Sci. Eng. 2016, 138, 012014. [Google Scholar] [CrossRef]
- Tarhan, S.; Ertugrul, M. The Change of Drying Time and Quality Parameters of Lemon Balm (Melissa officinalis L.) with Different Temperature Profiles of Drying Air. J. New Results Eng. Nat. Sci. 2019, 9, 1–10. [Google Scholar]
- Erbay, Z.; Icier, F. A Review of Thin Layer Drying of Foods: Theory, Modeling, and Experimental Results. Crit. Rev. Food Sci. Nutr. 2010, 50, 441–464. [Google Scholar] [CrossRef]
- Onwude, D.I.; Hashim, N.; Janius, R.B.; Nawi, N.M.; Abdan, K. Modeling the Thin-Layer Drying of Fruits and Vegetables: A Review. Compr. Rev. Food Sci. Food Saf. 2016, 15, 599–618. [Google Scholar] [CrossRef] [PubMed]
- Botheju, W.S.; Amarathunge, K.S.P.; Abeysinghe, I.S.B. Modeling Trough Withering System to Predict the Moisture Content of Tea Leaves at Real Time using One Dimensional Heat and Mass Transfer Finite Difference Model. Trop. Agric. Res. 2011, 22, 282–295. [Google Scholar] [CrossRef] [Green Version]
- Pilatti, D.; Johann, G.; Palú, F.; da Silva, E.A. Evaluation of a concentrated parameters mathematical model applied to drying of yerba mate leaves with variable mass transfer coefficient. Appl. Therm. Eng. 2016, 105, 483–489. [Google Scholar] [CrossRef]
- Kiranoudis, C.; Maroulis, Z.; Marinos-Kouris, D. Mass Transfer Modeling for Virginia Tobacco Curing. Dry. Technol. 1990, 8, 351–366. [Google Scholar] [CrossRef]
- Babu, A.; Kumaresan, G.; Raj, V.A.A.; Velraj, R. Review of leaf drying: Mechanism and influencing parameters, drying methods, nutrient preservation, and mathematical models. Renew. Sustain. Energy Rev. 2018, 90, 536–556. [Google Scholar] [CrossRef]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method; Pearson Education: New York, NY, USA, 2007. [Google Scholar]
- Ramallo, L.; Mascheroni, R. Effect of Shrinkage on Prediction Accuracy of the Water Diffusion Model for Pineapple Drying. J. Food Process. Eng. 2013, 36, 66–76. [Google Scholar] [CrossRef]
- Hassini, L.; Azzouz, S.; Peczalski, R.; Belghith, A. Estimation of potato moisture diffusivity from convective drying kinetics with correction for shrinkage. J. Food Eng. 2007, 79, 47–56. [Google Scholar] [CrossRef]
- Katekawa, M.E.; Silva, M.A. A Review of Drying Models Including Shrinkage Effects. Dry. Technol. 2006, 24, 5–20. [Google Scholar] [CrossRef]
- Tzempelikos, D.A.; Mitrakos, D.; Vouros, A.P.; Bardakas, A.V.; Filios, A.E.; Margaris, D.P. Numerical modeling of heat and mass transfer during convective drying of cylindrical quince slices. J. Food Eng. 2015, 156, 10–21. [Google Scholar] [CrossRef]
- AOAC. Official Methods of Analysis, 15th ed.; Association of Official Analytical Chemists: Arlington, VA, USA, 1990. [Google Scholar]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow; Hemisphere Publishing Corp: New York, NY, USA, 1980. [Google Scholar]
- Owen, M.S. ASHRAE Handbook: Fundamentals; American Society of Heating, Refrigerating and Air-Conditioning Engineers: Atlanta, GA, USA, 2009. [Google Scholar]
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; DeWitt, D.P. Principles of Heat and Mass Transfer, 7th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Chilton, T.H.; Colburn, A.P. Mass Transfer (Absorption) Coefficients Prediction from Data on Heat Transfer and Fluid Friction. Ind. Eng. Chem. 1934, 26, 1183–1187. [Google Scholar] [CrossRef]
- Defraeye, T.; Verboven, P.; Ho, Q.T.; Nicolai, B. Convective heat and mass exchange predictions at leaf surfaces: Applications, methods and perspectives. Comput. Electron. Agric. 2013, 96, 180–201. [Google Scholar] [CrossRef] [Green Version]
- Chen, C.S. Equilibrium Moisture Curves for Biological Materials. Trans. ASAE 1971, 14, 924–926. [Google Scholar] [CrossRef]
- IBM Corp. IBM SPSS Statistics for Windows; IBM: Armonk, NY, USA, 2020. [Google Scholar]
- Tzempelikos, D.A.; Vouros, A.P.; Bardakas, A.V.; Filios, A.E.; Margaris, D.P. Case studies on the effect of the air drying conditions on the convective drying of quinces. Case Stud. Therm. Eng. 2014, 3, 79–85. [Google Scholar] [CrossRef] [Green Version]
- Crank, J. The Mathematics of Diffusion; Oxford University Press: Oxford, UK, 1979. [Google Scholar]
- Kaya, A.; Aydın, O.; Dincer, I. Experimental and numerical investigation of heat and mass transfer during drying of Hayward kiwi fruits (Actinidia Deliciosa Planch). J. Food Eng. 2008, 88, 323–330. [Google Scholar] [CrossRef]
- Beigi, M. Numerical simulation of potato slices drying using a two-dimensional finite element model. Chem. Ind. Chem. Eng. Q. 2017, 23, 431–440. [Google Scholar] [CrossRef]
- Castro, A.; Mayorga, E.; Moreno, F. Mathematical modelling of convective drying of feijoa (Acca sellowiana Berg) slices. J. Food Eng. 2019, 252, 44–52. [Google Scholar] [CrossRef]
- Nadi, F. Estimation of the mass transfer parameters of Horseradish (Armoracia rusticana L.) under convective drying. In Proceedings of the 2nd Iranian Conference on Heat and Mass Transfer (ICHMT), Semnan, Iran, 19–20 November 2014; pp. 2–5. [Google Scholar]
- Calín-Sánchez, Á.; Figiel, A.; Lech, K.; Szumny, A.; Carbonell-Barrachina, Á.A. Effects of Drying Methods on the Composition of Thyme (Thymus vulgaris L.) Essential Oil. Dry. Technol. 2013, 31, 224–235. [Google Scholar] [CrossRef]
- Chua, L.Y.W.; Chua, B.L.; Figiel, A.; Chong, C.H.; Wojdyło, A.; Szumny, A.; Łyczko, J. Drying of Phyla nodiflora Leaves: Antioxidant Activity, Volatile and Phytosterol Content, Energy Consumption, and Quality Studies. Processes 2019, 7, 210. [Google Scholar] [CrossRef] [Green Version]
- Turan, O.Y.; Firatligil, F.E. Modelling and characteristics of thin layer convective air-drying of thyme (Thymus vulgaris) leaves. Czech J. Food Sci. 2019, 37, 128–134. [Google Scholar] [CrossRef]

| Temperature Advancing Rate (°C/h) | Statistical Parameter | ||
|---|---|---|---|
| R2 | RMSE | SSE | |
| 2 | 0.922 | 0.073 | 0.431 |
| 3 | 0.958 | 0.056 | 0.226 |
| 4 | 0.995 | 0.021 | 0.027 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chasiotis, V.; Tzempelikos, D.; Filios, A. Evaluation of a Moisture Diffusion Model for Analyzing the Convective Drying Kinetics of Lavandula x allardii Leaves. Computation 2021, 9, 141. https://doi.org/10.3390/computation9120141
Chasiotis V, Tzempelikos D, Filios A. Evaluation of a Moisture Diffusion Model for Analyzing the Convective Drying Kinetics of Lavandula x allardii Leaves. Computation. 2021; 9(12):141. https://doi.org/10.3390/computation9120141
Chicago/Turabian StyleChasiotis, Vasileios, Dimitrios Tzempelikos, and Andronikos Filios. 2021. "Evaluation of a Moisture Diffusion Model for Analyzing the Convective Drying Kinetics of Lavandula x allardii Leaves" Computation 9, no. 12: 141. https://doi.org/10.3390/computation9120141
APA StyleChasiotis, V., Tzempelikos, D., & Filios, A. (2021). Evaluation of a Moisture Diffusion Model for Analyzing the Convective Drying Kinetics of Lavandula x allardii Leaves. Computation, 9(12), 141. https://doi.org/10.3390/computation9120141







