Numerical Modelling of Double-Steel Plate Composite Shear Walls
Abstract
:1. Introduction
1.1. Numerical Literature Overview
1.2. Objectives and Scope of Work
2. Numerical Model Formulation
2.1. Analysis Method
2.2. Element Types and Material Properties
2.2.1. Concrete Infill
2.2.2. Steel Plates
2.2.3. Steel Endplates
2.2.4. Shear Connectors
2.2.5. Contact Elements
Concrete Infill—Steel Plates
Concrete Infill—Endplates
2.2.6. Foundation
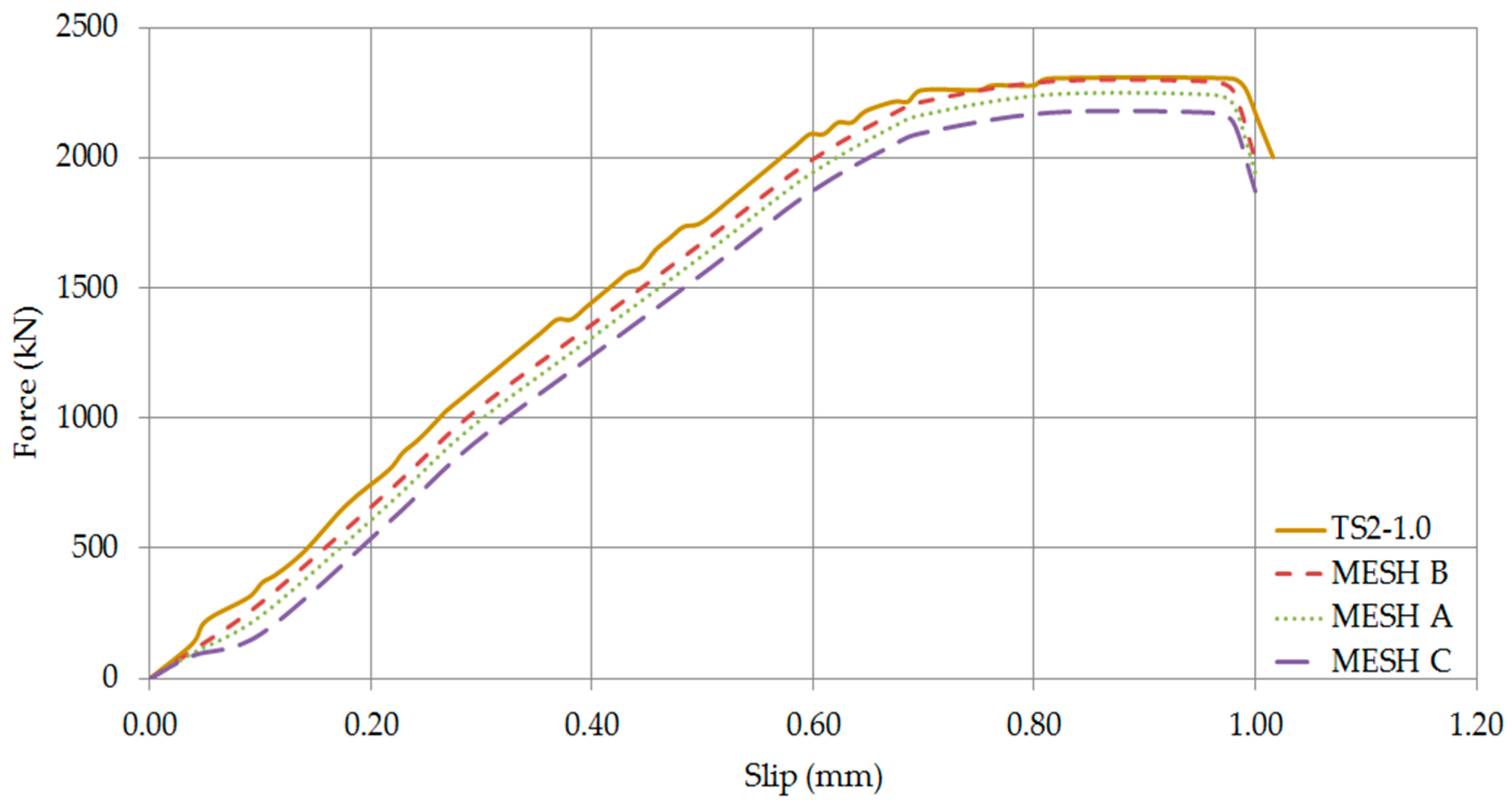
2.3. Meshing
2.4. Loading
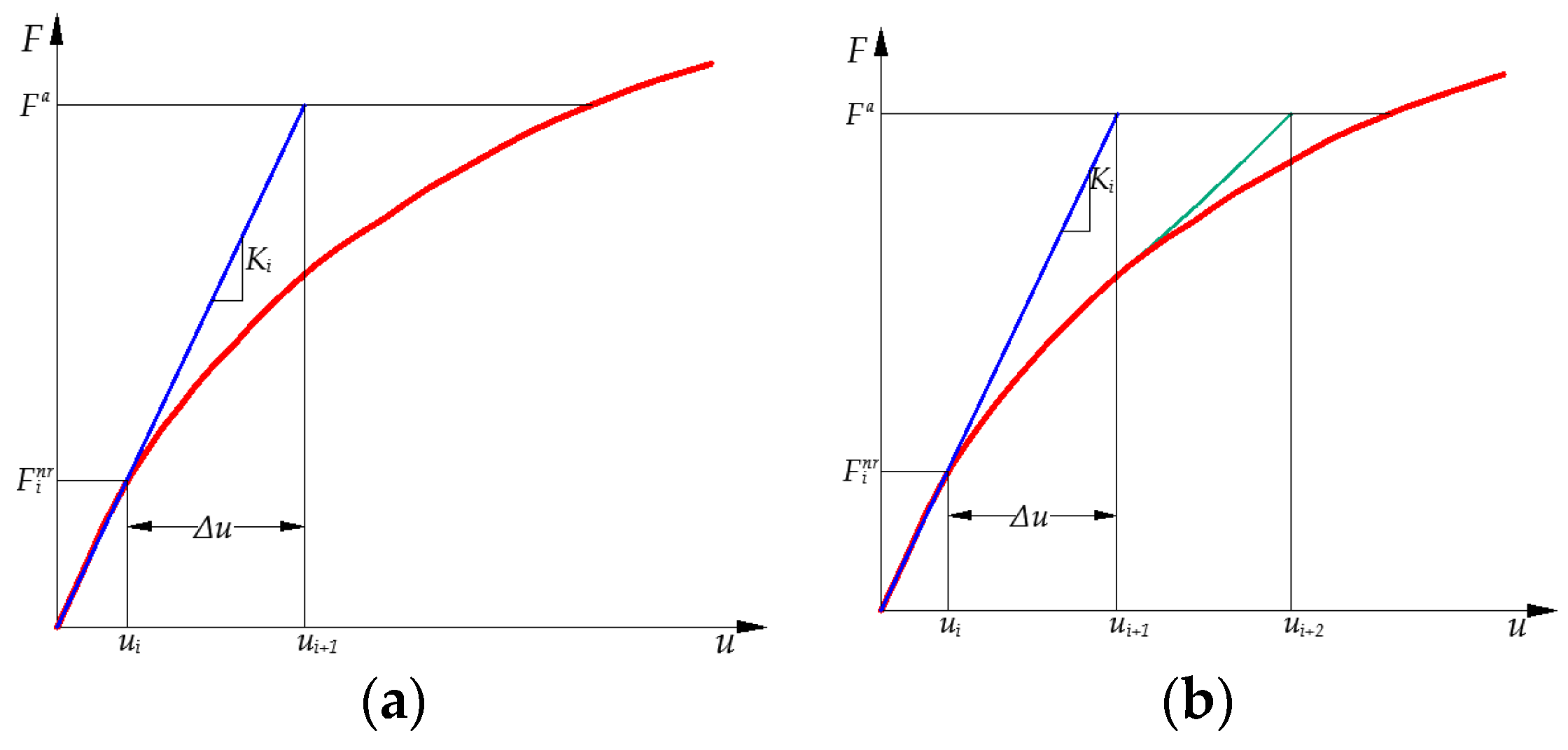
2.5. Nonlinear Solution and Convergence Criteria
- (a)
- The first load step is considered as the load target that the model has to converge on .
- (b)
- The first incremental load vector is applied . It is a vector of restoring loads that corresponds to the internal loads of elements.
- (c)
- DOF values are assumed from the restoring displacements , which derive from the result of the previously convergence attempt or they are equal to zero at the first substep.
- (d)
- The software computes the Jacobean of the coefficient matrix based on the assumed DOFs .
- (e)
- Having obtained the incremental loads , the incremental displacement can now be calculated using Equation (8).
- (f)
- The incremental displacements will be added to the assumed values of displacements and form the second iteration shown in Equation (9). This creates a new vector of restoring loads based on the assumed values of displacements.
3. Validation Results
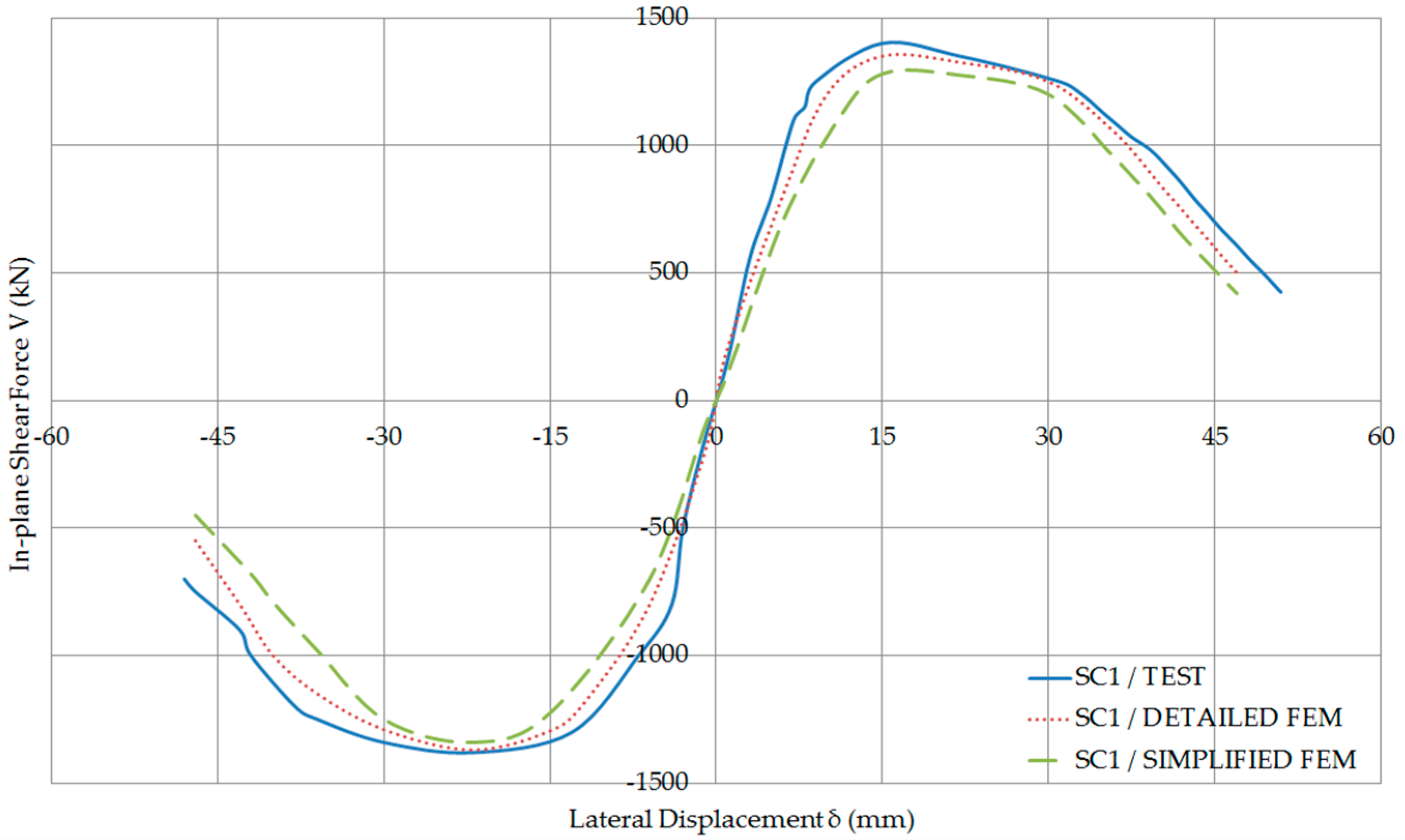
3.1. In-Plane Shear Loading
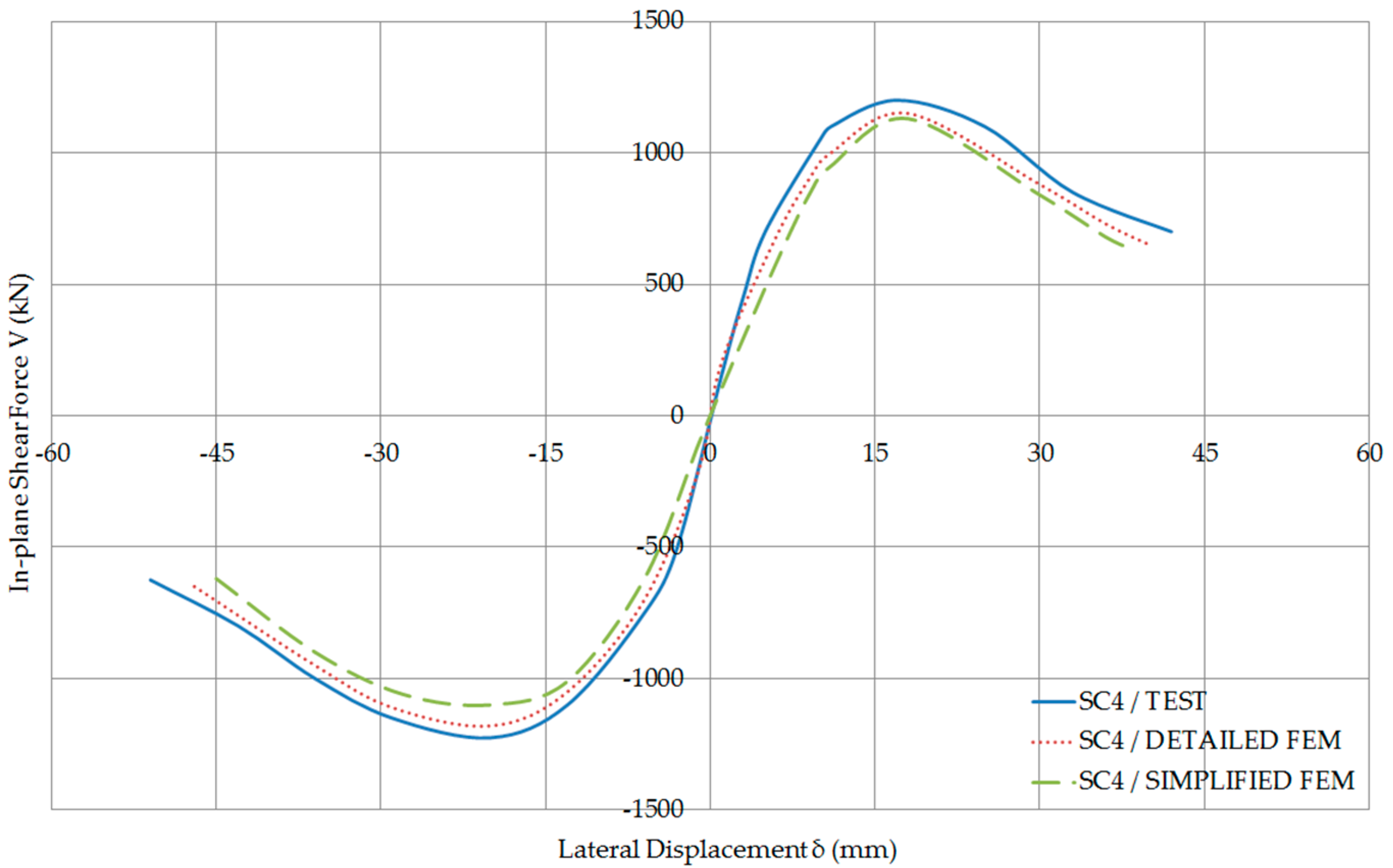
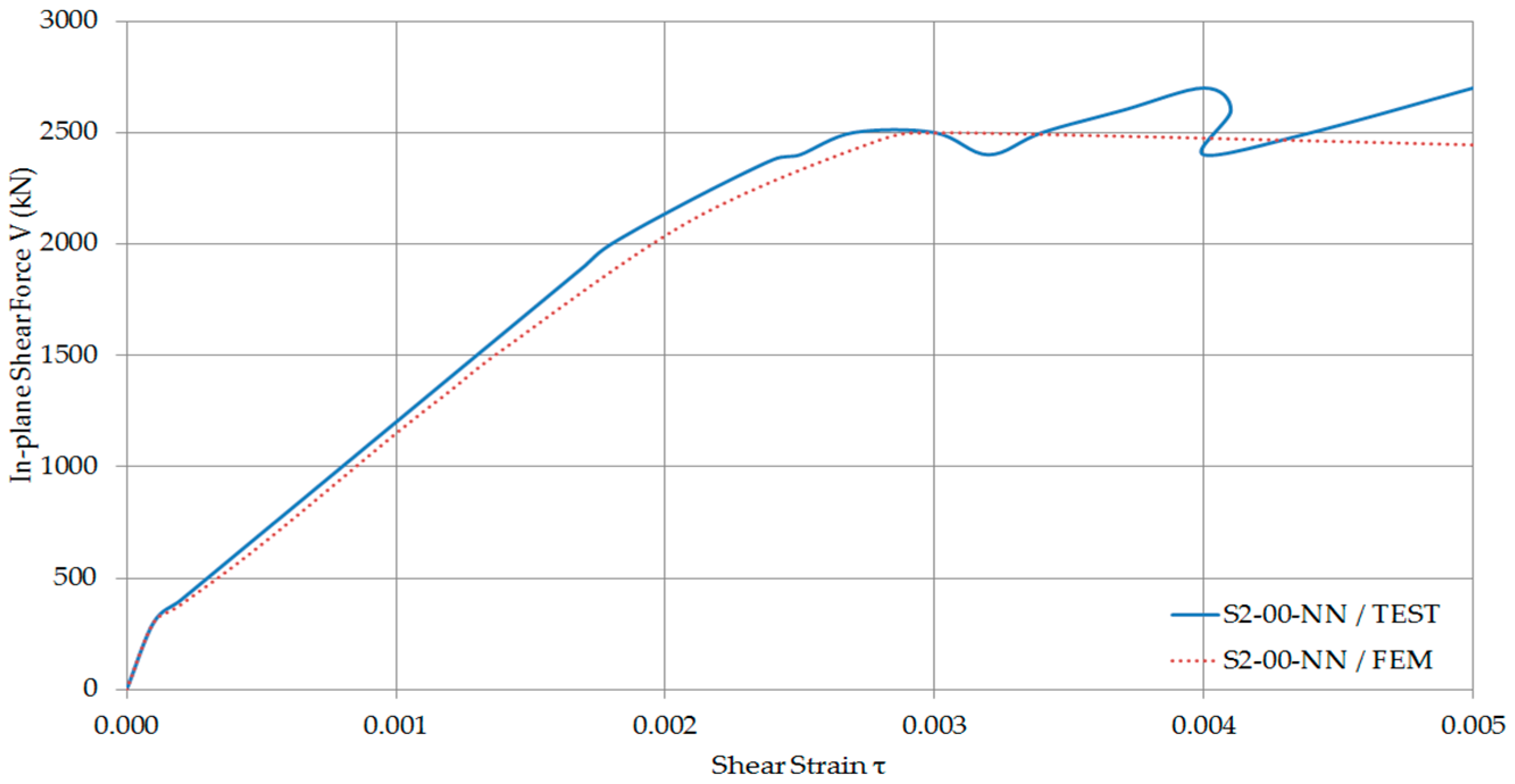
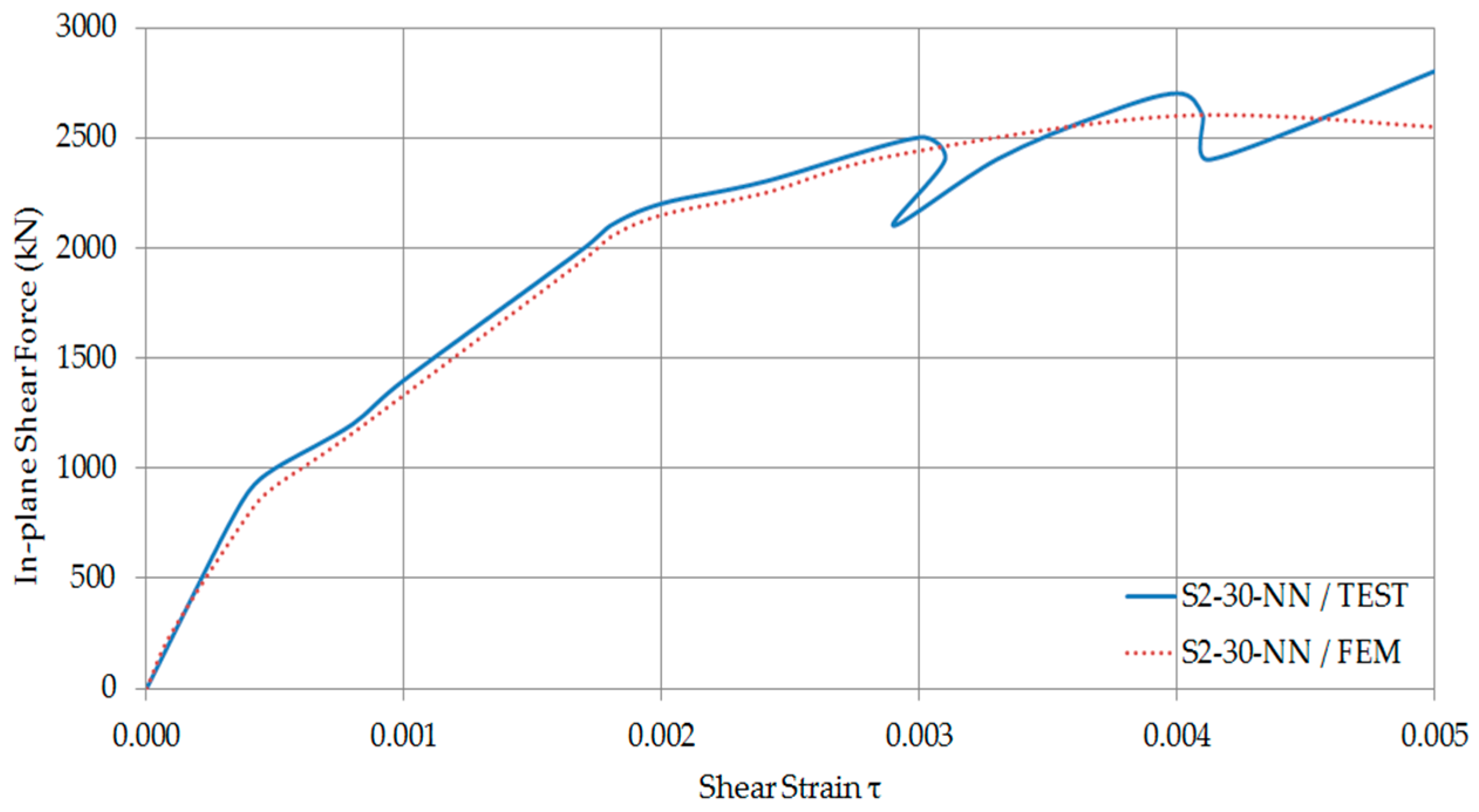
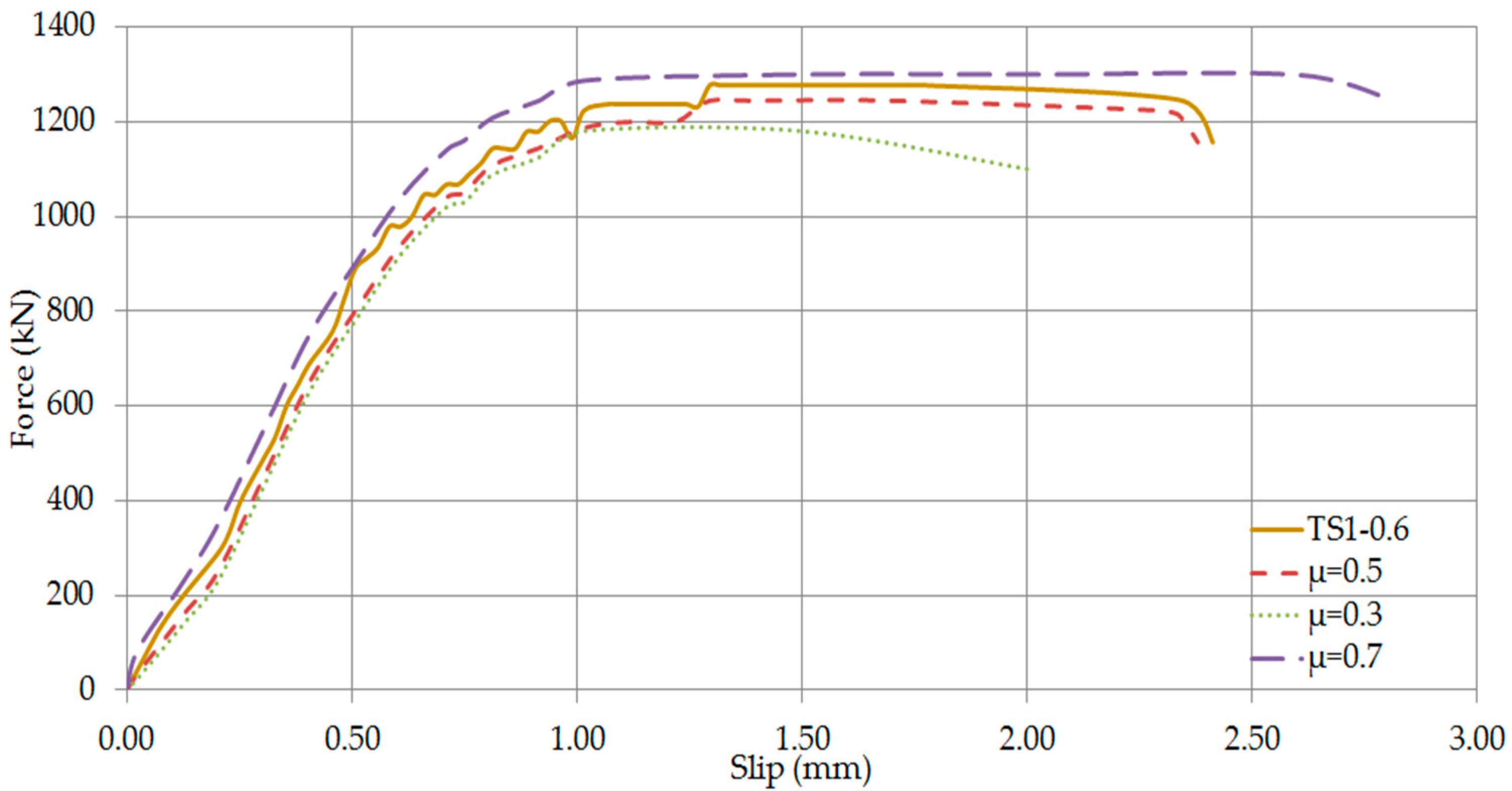
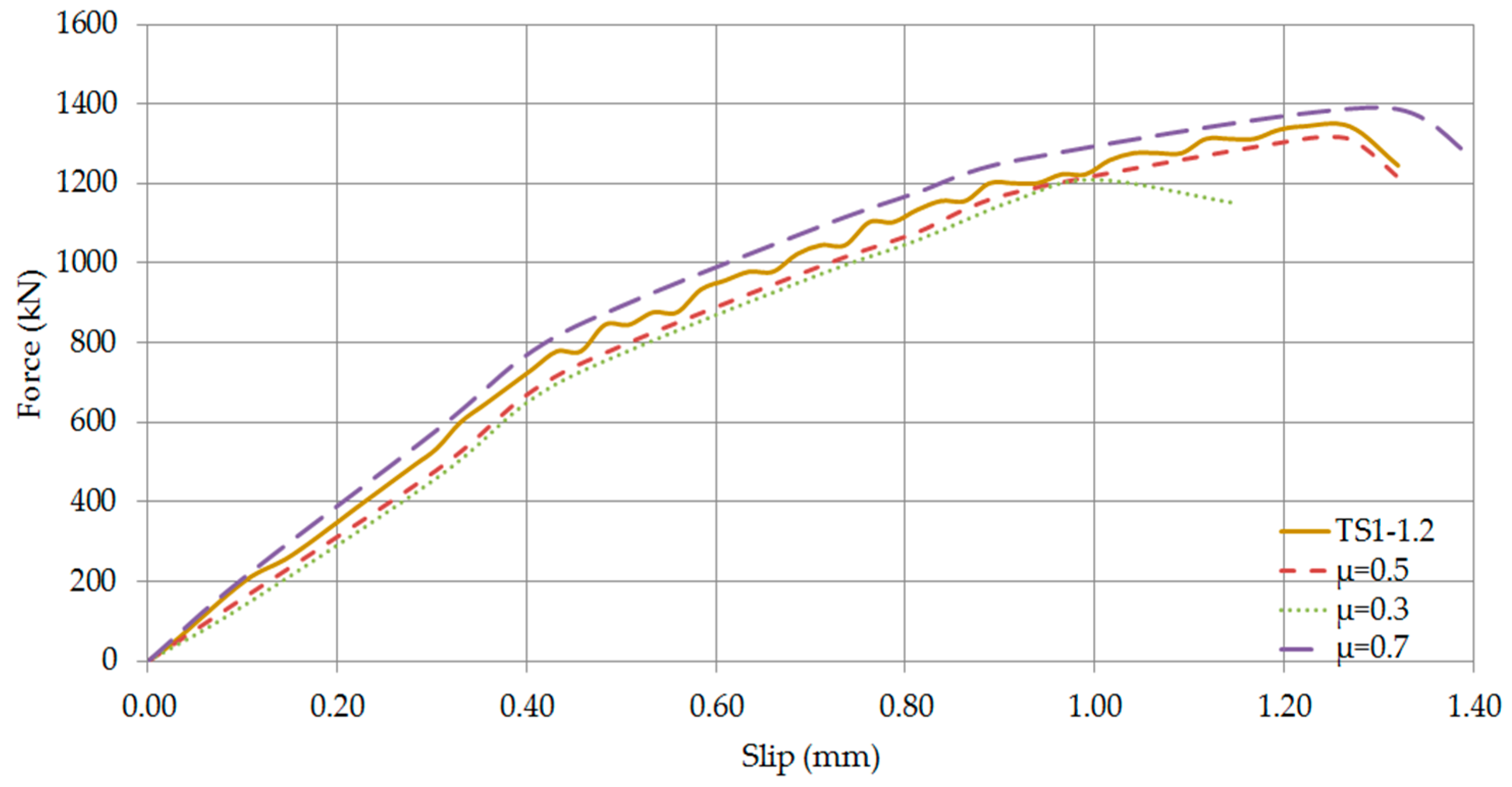
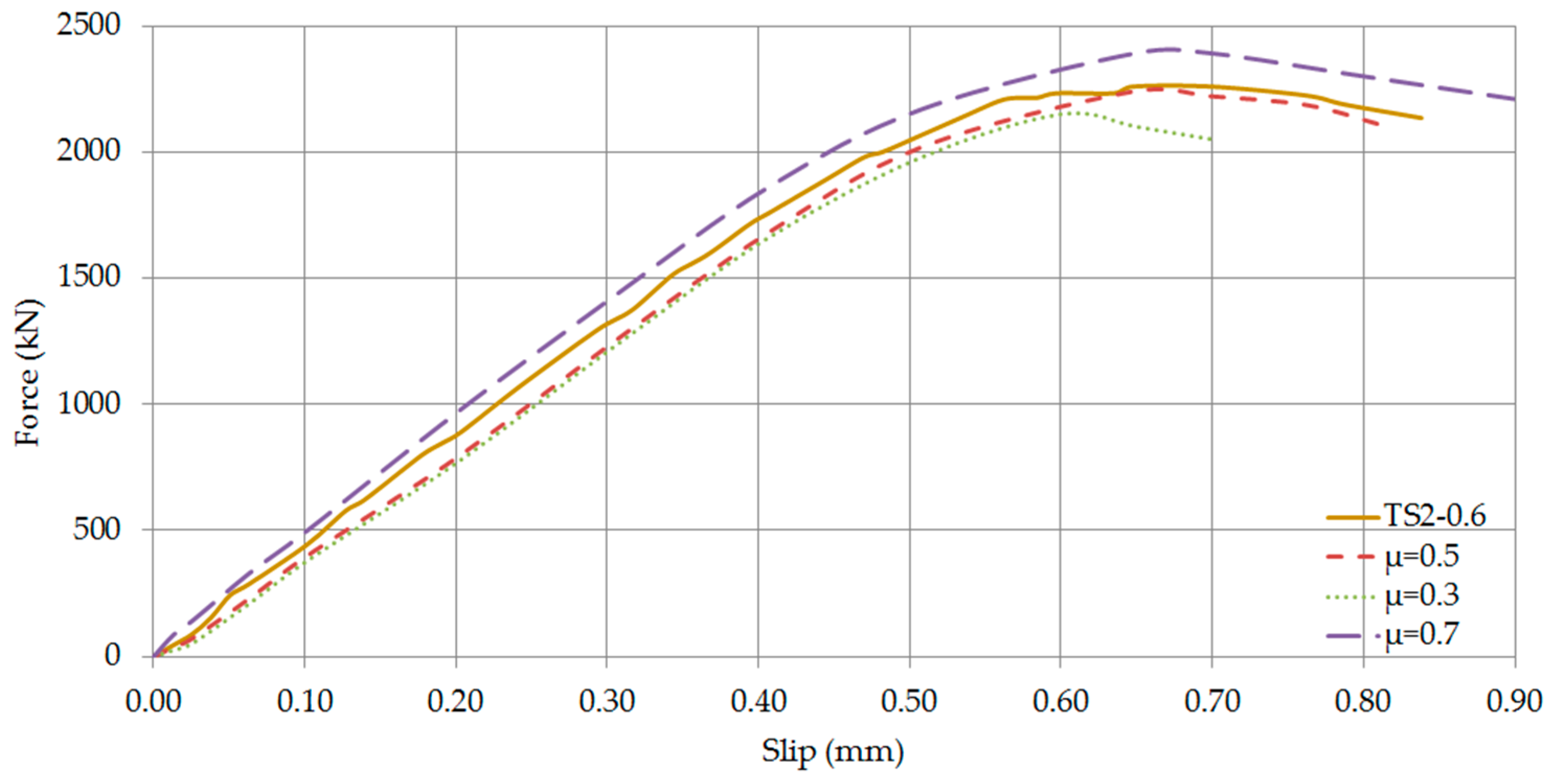
3.1.1. Validation with First Set of Specimens
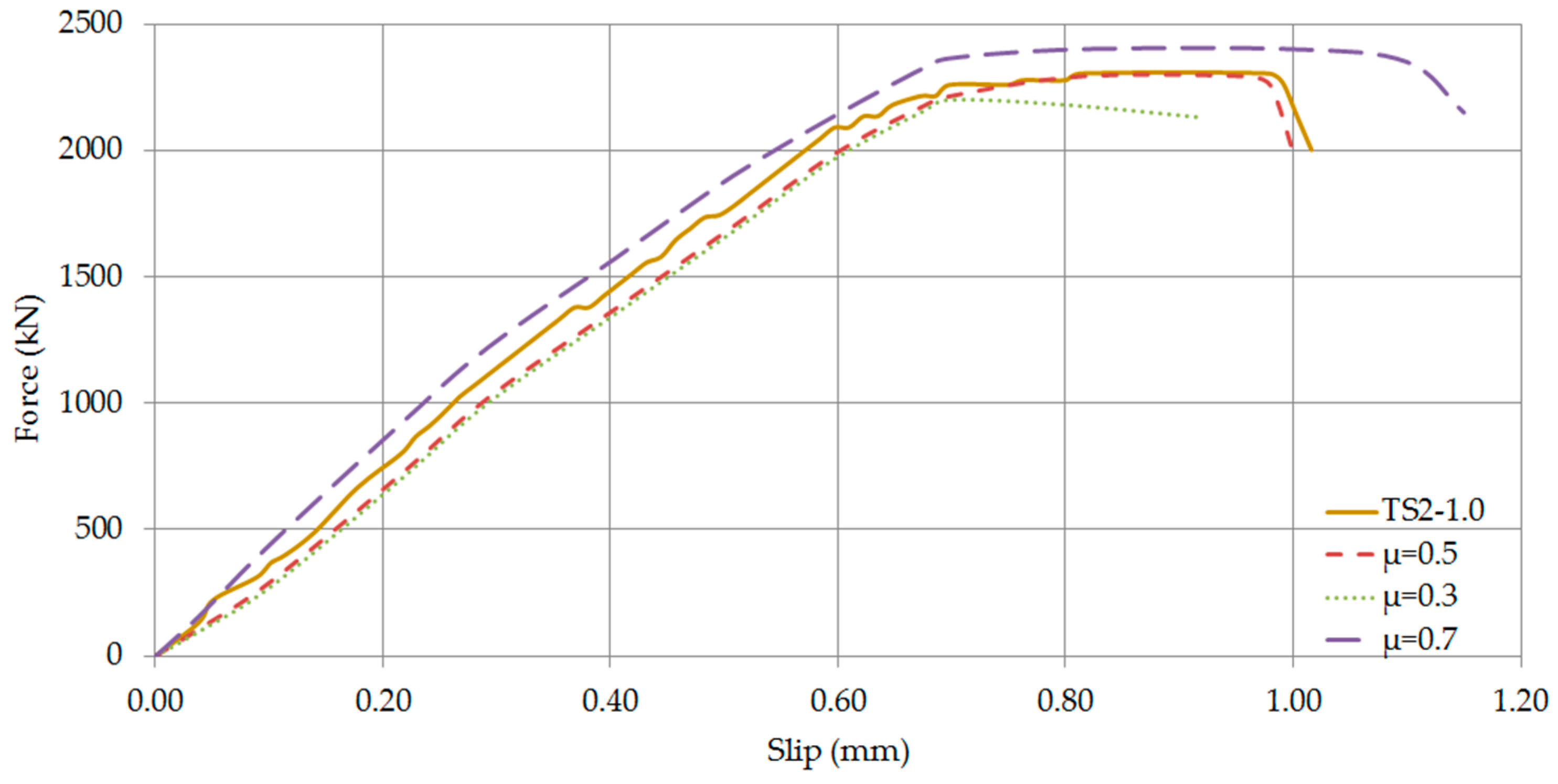
3.1.2. Validation with Second Set of Specimens
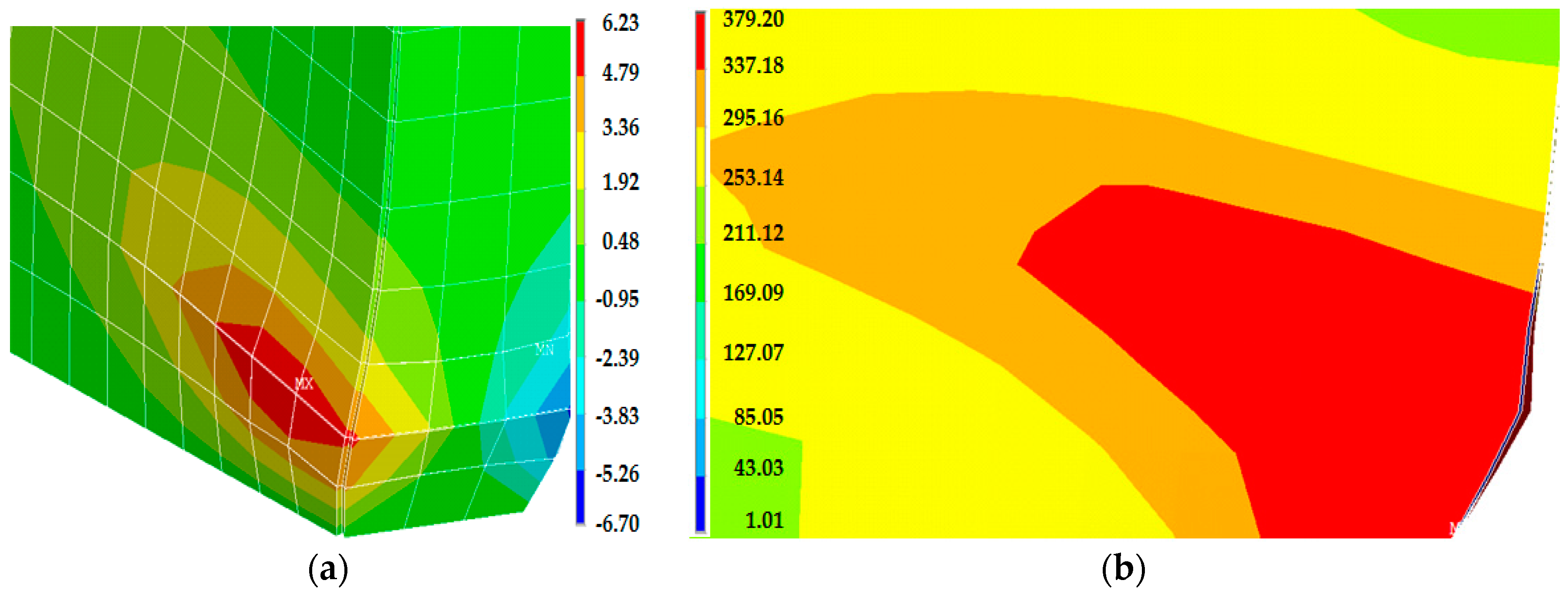
3.2. Compression Loading
4. Parametric Analysis
4.1. Design Procedure
4.2. Analysis Results
5. Conclusions
- A multilinear stress-strain curve for concrete, using user-specified material properties. In the absence of experimental data, the curve can be estimated from relations proposed by EC2 [31]. In the case of partially confined concrete, the best way for indicating the concrete crushing failure mode is by evaluating the concrete strain contour results. Considering fully confined infill concrete, the William-Warnke criterion [29] can be used as failure criterion due to multiaxial stress state.
- A multilinear stress-strain curve for steel based on the von Mises yield criterion with isotropic hardening rule. The strain-hardening branch can be efficiently calculated using the constitutive law proposed by Gattesco [34]. The shortfall in the load-bearing capacity of steel due to cyclic loading should also be accounted for.
- Two load-slip curves for modelling the behaviour of the two nonlinear springs used to model the behaviour of a single shear connector.
- Shell and solid elements to model steel faceplates, infill concrete and steel endplates, respectively.
- Contact elements to simulate the behaviour of the steel-concrete interface.
Author Contributions
Conflicts of Interest
References
- Bruhl, J.C.; Varma, A.H.; Johnson, W.H. Design of composite SC walls to prevent perforation from missile impact. Int. J. Impact Eng. 2014, 75, 75–87. [Google Scholar] [CrossRef]
- Sener, K.C.; Varma, A.H. Steel-plate composite walls: Experimental database and design for out-of-plane shear. J. Constr. Steel Res. 2014, 100, 197–210. [Google Scholar] [CrossRef]
- Varma, A.H.; Zhang, K.; Chi, H.; Booth, P.N.; Baker, T. In-plane shear behaviour of SC composite walls: theory vs. experiment. In Proceedings of the 21th International Conference on Structural Mechanics in Reactor Technology (SMiRT21), International Association for Structural Mechanics in Reactor Technology (IASMiRT), New Delhi, India, 6–11 November 2011.
- Varma, A.H.; Malushte, S.R.; Sener, K.C.; Lai, Z. Steel-plate composite (SC) walls for safety related nuclear facilities: Design for in-plane forces and out-of-plane moments. Nucl. Eng. Des. 2014, 269, 240–249. [Google Scholar] [CrossRef]
- American Institute of Steel Construction. Specification for safety-related steel structures for nuclear facilities/Supplement No. 1; AISC N690-12s1. Available online: https://www.aisc.org/globalassets/aisc/publications/standards/specification-for-safety-related-steel-structures-for-nuclear-facilities-in-cluding-supplement-no.-1-ansiaisc-n690-12-anisaisc-n690s1015.pdf (accessed on 21 February 2017).
- American Institute of Steel Construction. Seismic provisions for structural steel buildings, Public ballot No. 2; AISC 341-16. Available online: http://www.alacero.org/sites/default/files/u16/bc_11-15_3.2_aisc_341-16_ draft_1_marzo_2015.pdf (accessed on 21 February 2017).
- Vecchio, F.J.; McQuade, I. Towards improved modeling of steel-concrete composite wall elements. Nucl. Eng. Des. 2011, 241, 2629–2642. [Google Scholar] [CrossRef]
- Wong, P.S.; Vecchio, F.J.; Trommels, H. VecTor2 and Formworks User’s Manual, 2nd ed.; University of Toronto: Toronto, ON, Canada, 2013. [Google Scholar]
- Usami, S.; Akiyama, H.; Narikawa, M.; Hara, K.; Takeuchi, M.; Sasaki, N. Study on a concrete filled structure for nuclear power plants (Part 2): Compressive loading tests on wall members. In Proceedings of the 13th International Conference on Structural Mechanics in Reactor Technology (SMiRT13), International Association for Structural Mechanics in Reactor Technology (IASMiRT), Porto Alegre, Brazil, 13–18 August 1995.
- Sasaki, N.; Akiyama, H.; Narikawa, M.; Hara, K.; Takeuchi, M.; Usami, S. Study on a concrete filled steel structure for nuclear power plants (Part 2): Shear and bending loading tests on wall member. In Proceedings of the 13th International Conference on Structural Mechanics in Reactor Technology (SMiRT13), International Association for Structural Mechanics in Reactor Technology (IASMiRT), Porto Alegre, Brazil, 13–18 August 1995.
- Ozaki, M.; Akita, S.; Niwa, N.; Matsuo, I.; Usami, S. Study on steel-plate reinforced concrete bearing wall for nuclear power plants part 1: shear and bending loading tests of SC walls. In Proceedings of the 16th International Conference on Structural Mechanics in Reactor Technology (SMiRT16), International Association for Structural Mechanics in Reactor Technology (IASMiRT), Washington, DC, USA, 12 August 2001.
- Zhou, J.; Mo, Y.L.; Sun, X.; Li, J. Seismic performance of composite steel plate reinforced concrete shear wall. In Proceedings of the 12th International Conference on Engineering, Science, Construction, and Operations in Challenging Environments-Earth and Space, Honolulu, Hawaii, USA, 14–17 March 2010.
- Mansour, M.; Hsu, T. Behaviour of Reinforced Concrete Elements under Cyclic Shear I: Experiments. J. Struct. Eng. 2005, 131, 44–53. [Google Scholar] [CrossRef]
- Mansour, M.; Hsu, T. Behaviour of Reinforced Concrete Elements under Cyclic Shear II: Theoretical Model. J. Struct. Eng. 2005, 131, 54–65. [Google Scholar] [CrossRef]
- Zhong, J.X. Model-Based Simulation of Reinforced Concrete Plane Stress Structures; University of Houston: Houston, TX, USA, 2005. [Google Scholar]
- Ma, X.; Nie, J.; Tao, M. Nonlinear finite-element analysis of double-skin steel-concrete composite shear wall structures. IACSIT J. Eng. Technol. 2013, 5, 648–652. [Google Scholar]
- Rafiei, S.; Hossain, K.M.A.; Lachemi, M.; Behdinan, K. Profiled sandwich composite wall with high performance concrete subjected to monotonic shear. J. Constr. Steel Res. 2015, 107, 124–136. [Google Scholar] [CrossRef]
- SIMULIA. ABAQUS Analysis User’s Manual; Version 6.12; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2012. [Google Scholar]
- Ali, A.; Kim, D.; Cho, S.G. Modeling of nonlinear cyclic load behaviour of I-shaped composite steel-concrete shear walls of nuclear power plants. Nucl. Eng. Technol. 2013, 45, 89–98. [Google Scholar] [CrossRef]
- Kurt, E.G.; Varma, A.H.; Booth, P.; Whittaker, A.S. SC wall piers and basemat connections: Numerical investigation of behaviour and design. In Proceedings of the 22nd International Conference on Structural Mechanics in Reactor Technology (SMiRT22), International Association for Structural Mechanics in Reactor Technology (IASMiRT), San Francisco, CA, USA, 18–23 August 2013.
- Livermore Software Technology Corporation (LSTC). LS-DYNA. Keyword User’s Manual, Version 971 R6.0.0; Available online: http://lstc.com/pdf/ls-dyna_971_manual_k.pdf (accessed on 21 February 2017).
- Epackachi, S.; Nguyen, N.H.; Kurt, E.G.; Whittaker, A.S.; Varma, A.H. In-Plane Seismic Behaviour of Rectangular Steel-Plate Composite Wall Piers. J. Struct. Eng. 2014, 1, 1–9. [Google Scholar]
- Vazouras, K.; Avdelas, A. Behaviour of composite steel walls-Numerical Analysis & Preliminary Findings. In Proceedings of the 7th European Conference on Steel and Composite Structures (EUROSTEEL), Naples, Italy, 10–12 September 2014.
- ANSYS Inc. ANSYS Mechanical User’s Guide; Version 15.0; ANSYS Inc, Southpointe: Canonsburg, PA, USA, 2013. [Google Scholar]
- Akiyama, H.; Sekimoto, H.; Fukihara, M.; Nakanishi, K.; Hara, K. A Compression and Shear Loading Tests of Concrete Filled Steel Bearing Wall. In Proceedings of the Transactions of the 11th International Conference on Structural Mechanics in Reactor Technology (SMiRT-11), Tokyo, Japan, 18–23 August 1991.
- Choi, B.J.; Kang, C.K.; Park, H.Y. Strength and behaviour of steel plate–concrete wall structures using ordinary and eco-oriented cement concrete under axial compression. Thin-Walled Struct. 2014, 84, 313–324. [Google Scholar] [CrossRef]
- Zhang, K. Axial Compression Behaviour and Partial Composite Action of SC Walls in Safety-Related Nuclear Facilities. Ph.D. Dissertation, Purdue University, West Lafayette, IN, USA, 2014. [Google Scholar]
- ANSYS Inc. ANSYS Mechanical APDL Element Reference, Version 15.0; ANSYS Inc, Southpointe: Canonsburg, PA, USA, 2013.
- William, K.J.; Warnke, E.D. Constitutive model for the triaxial behaviour of concrete. In Proceedings of the International Association for Bridge and Structural Engineering, ISMES-Bergamo, Italy, 17–19 May 1974.
- Barbosa, A.F.; Ribeiro, G.O. Analysis of Reinforced Concrete Structures Using Ansys Nonlinear Concrete Model. Comput. Mech. New Trends Appl. 1998, 1998, 1–7. [Google Scholar]
- European Committee for Standardization. Eurocode 2—Design of Concrete Structures, Part 1-1: General Rules and Rules for Buildings; European Committee for Standardization (CEN): Brussels, Belgium, 2004. [Google Scholar]
- Ellobody, E.; Young, B.; Lam, D. Behaviour of normal and high strength concrete-filled compact steel tube circular stub columns. J. Constr. Steel Res. 2006, 62, 706–715. [Google Scholar] [CrossRef]
- Lee, P.S.; Noh, H.C. Inelastic buckling behaviour of steel members under reversed cyclic loading. Eng. Struct. 2010, 32, 2579–2595. [Google Scholar] [CrossRef]
- Gattesco, N. Analytical modeling of nonlinear behaviour of composite beams with deformable connection. J. Constr. Steel Res. 1999, 52, 195–218. [Google Scholar] [CrossRef]
- Tsavdaridis, K.D. Seismic Analysis of Steel-Concrete Composite Buildings: Numerical Modeling. Encycl. Earthq. Eng. 2014. [Google Scholar] [CrossRef]
- Wan, S.; Loh, C.H.; Peng, S.Y. Experimental and theoretical study on softening and pinching effects of bridge columns. Soil Dyn. Earthq. Eng. 2001, 21, 75–81. [Google Scholar] [CrossRef]
- White, C.S.; Bronkhorst, C.A.; Anand, L. An improved isotropic-kinematic hardening model for moderate deformation metal plasticity. Mech. Mater. 1990, 10, 127–147. [Google Scholar] [CrossRef]
- Kojic, M.; Bathe, K.J. Inelastic Analysis of Solids and Structures; Springer: New York, NY, USA, 2005. [Google Scholar]
- Ucak, A.; Tsopelas, P. Constitutive model for cyclic response of structural steels with yield plateau. J. Struct. Eng. 2011, 137, 195–206. [Google Scholar] [CrossRef]
- European Committee for Standardization. Eurocode 4—Design of Composite Steel and Concrete Structures, Part 1-1: General Rules and Rules for Buildings; European Committee for Standardization (CEN): Brussels, Belgium, 2004. [Google Scholar]
- Mistakidis, E.S.; Thomopoulos, K.; Avdelas, A.; Panagiotopoulos, P.D. Shear connectors in composite beams: A new accurate algorithm. Thin-Walled Struct. 1994, 18, 191–207. [Google Scholar] [CrossRef]
- Panagiotopoulos, P.D.; Avdelas, A.V. A Hemivariatiοnal Inequality Approach to the Unilateral Contact Problem and Substationarity Principles. Ing. Arch. 1984, 54, 401–412. [Google Scholar] [CrossRef]
- Queiroz, F.D.; Vellasco, P.C.G.S.; Nethercot, D.A. Finite element modelling of composite beams with full and partial shear connection. J. Constr. Steel Res. 2007, 63, 505–521. [Google Scholar] [CrossRef]
- Ollgaard, J.G.; Slutter, R.G.; Fisher, J.W. Shear Strength of Stud Connectors in Lightweight and Normal-Weight Concrete. AISC Eng. J. 1971, 10, 55–64. [Google Scholar]
- American Concrete Institute Committee 318 (ACI). Building Code Requirements for Structural Concrete (ACI 318-08) and Commentary (ACI 318R-08); American Concrete: Farmington Hills, MI, USA, 2008. [Google Scholar]
- Precast/Prestressed Concrete Institute (PCI). PCI Design Handbook: Precast and Prestressed Concrete, 7th ed.; Precast/Prestressed Concrete Institute: Chicago, IL, USA, 2010. [Google Scholar]
- Rabbat, B.G.; Russell, H.G. Friction Coefficient of Steel on Concrete or Grout. J. Struct. Eng. 1985, 111, 505–515. [Google Scholar] [CrossRef]
- Tsavdaridis, K.D.; Mello, C.D. Vierendeel bending study of perforated steel beams with various novel web opening shapes through nonlinear finite-element analyses. J. Struct. Eng. 2012, 138, 1214–1230. [Google Scholar] [CrossRef]
- Bathe, K.J. Finite Element Procedures; Prentice-Hall: Englewood Cliffs, NJ, USA, 1996. [Google Scholar]
- European Committee for Standardization. Eurocode 3—Design of Steel Structures, Part 1-1: General Rules and Rules for Buildings; European Committee for Standardization (CEN): Brussels, Belgium, 2005. [Google Scholar]
- ACI 374 Committee. Acceptance Criteria for Moment Frames Based on Structural Testing and Commentary; American Concrete Institute: Farmington Hills, MI, USA, 2005. [Google Scholar]
- Park, R. Ductility evaluation from laboratory and analytical testing. In Proceedings of the 9th World Conference on Earthquake Engineering, Tokyo-Kyoto, Japan, 2–9 August 1998.

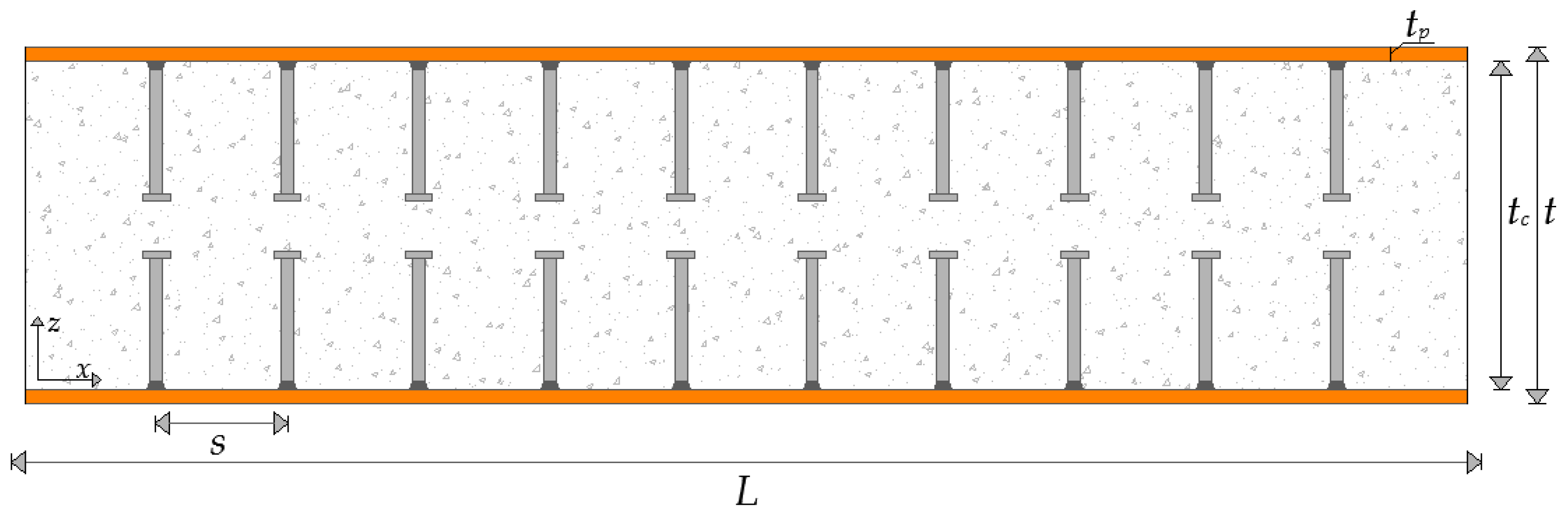



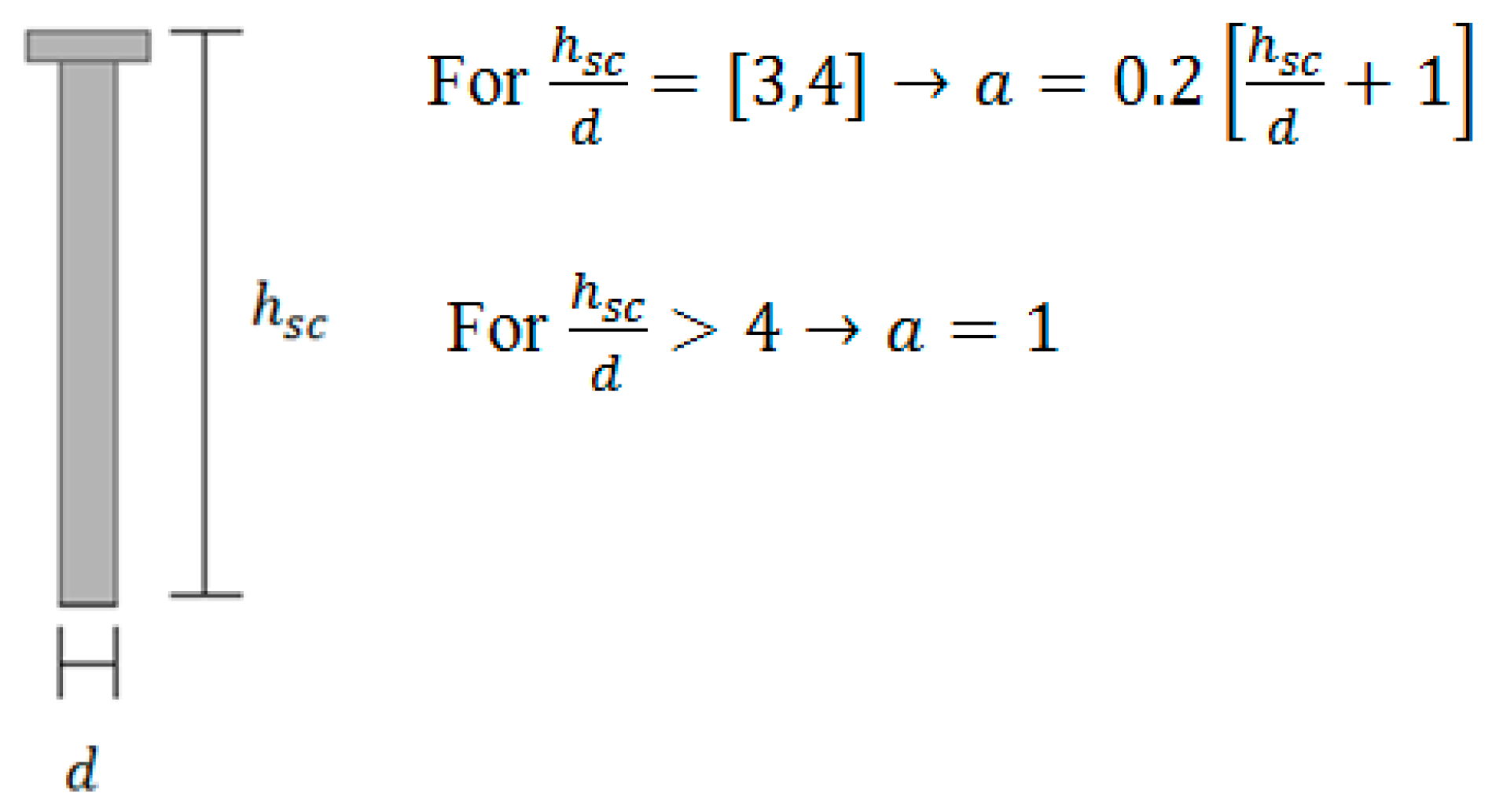





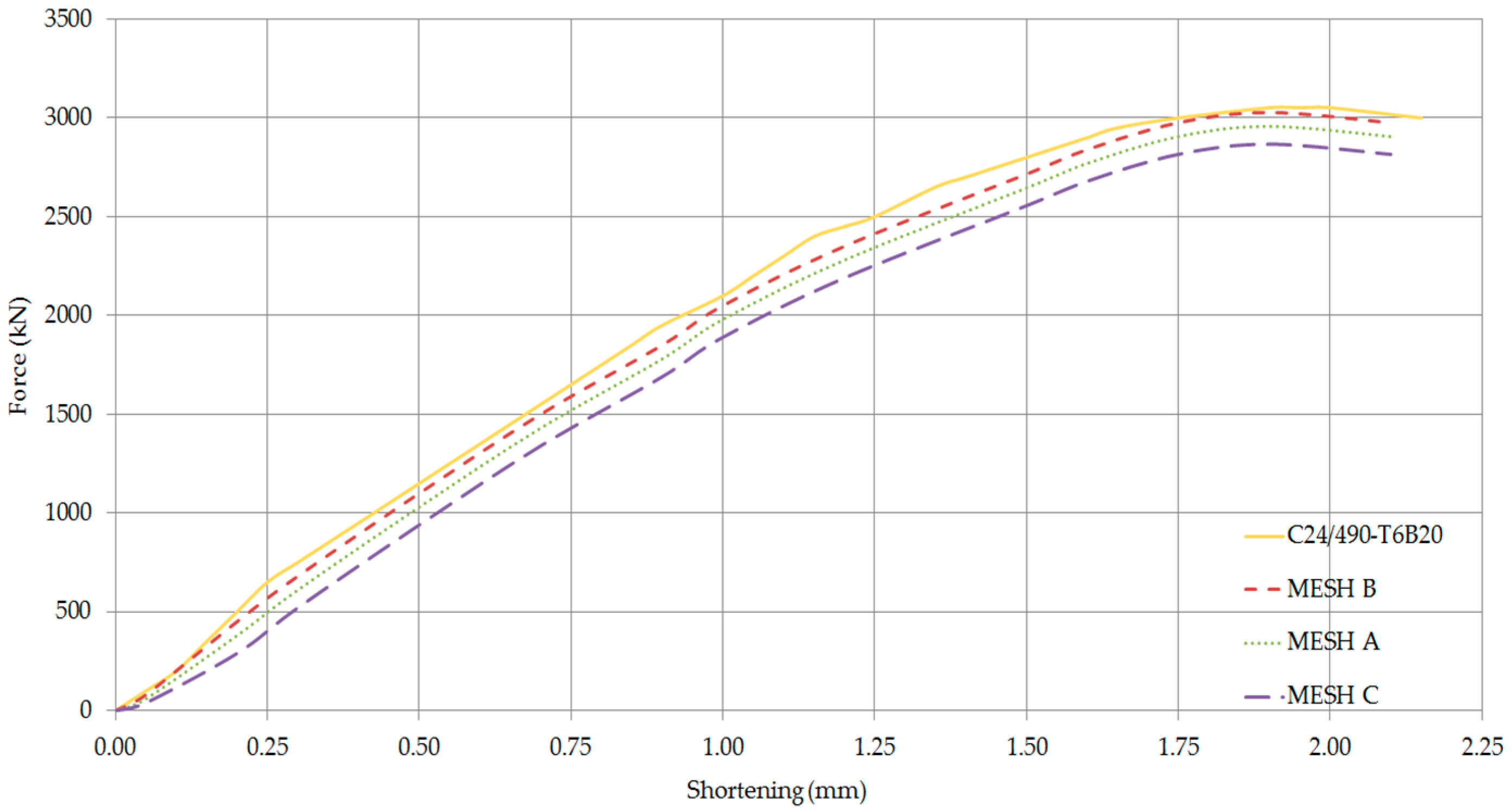
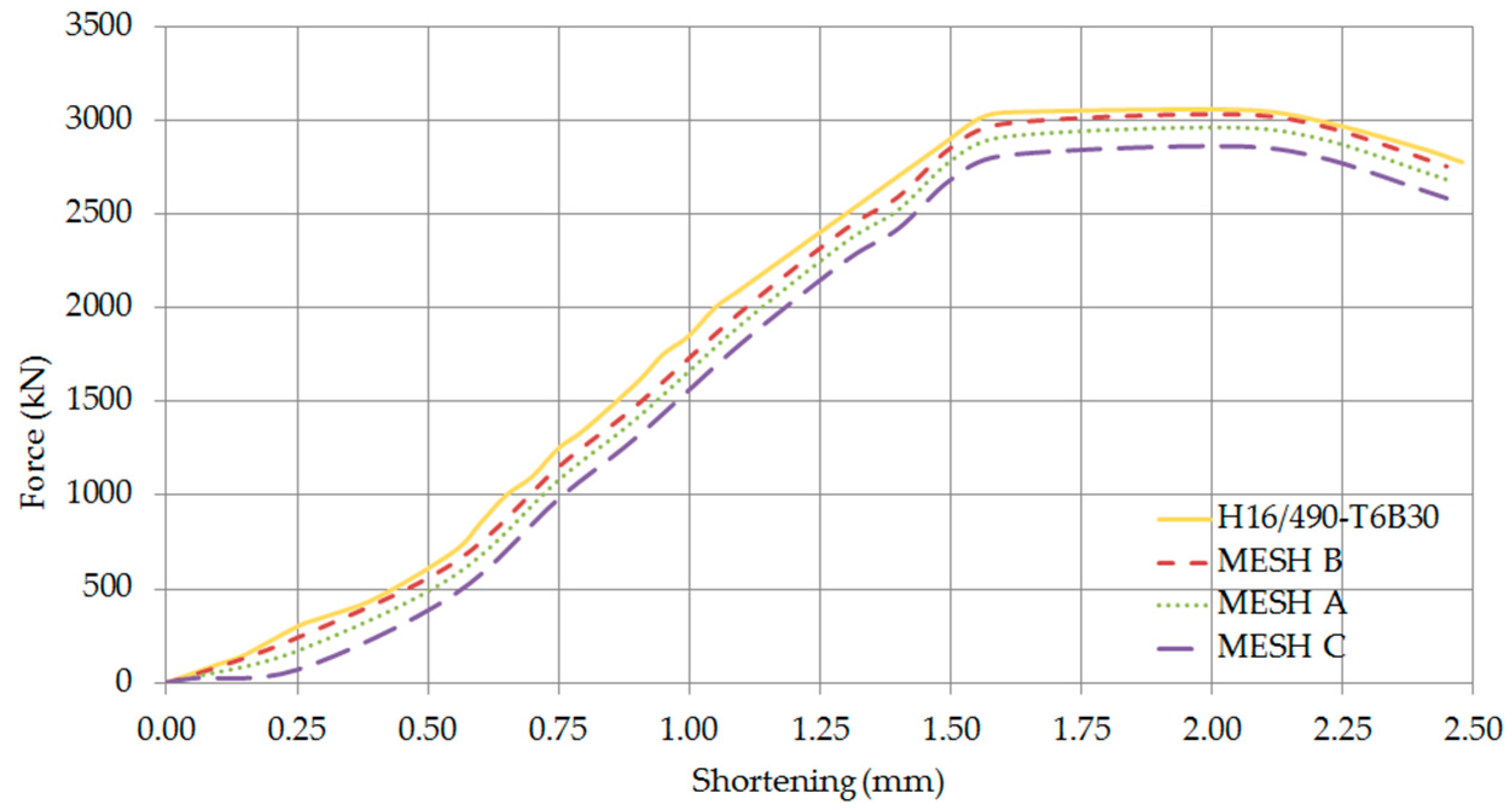
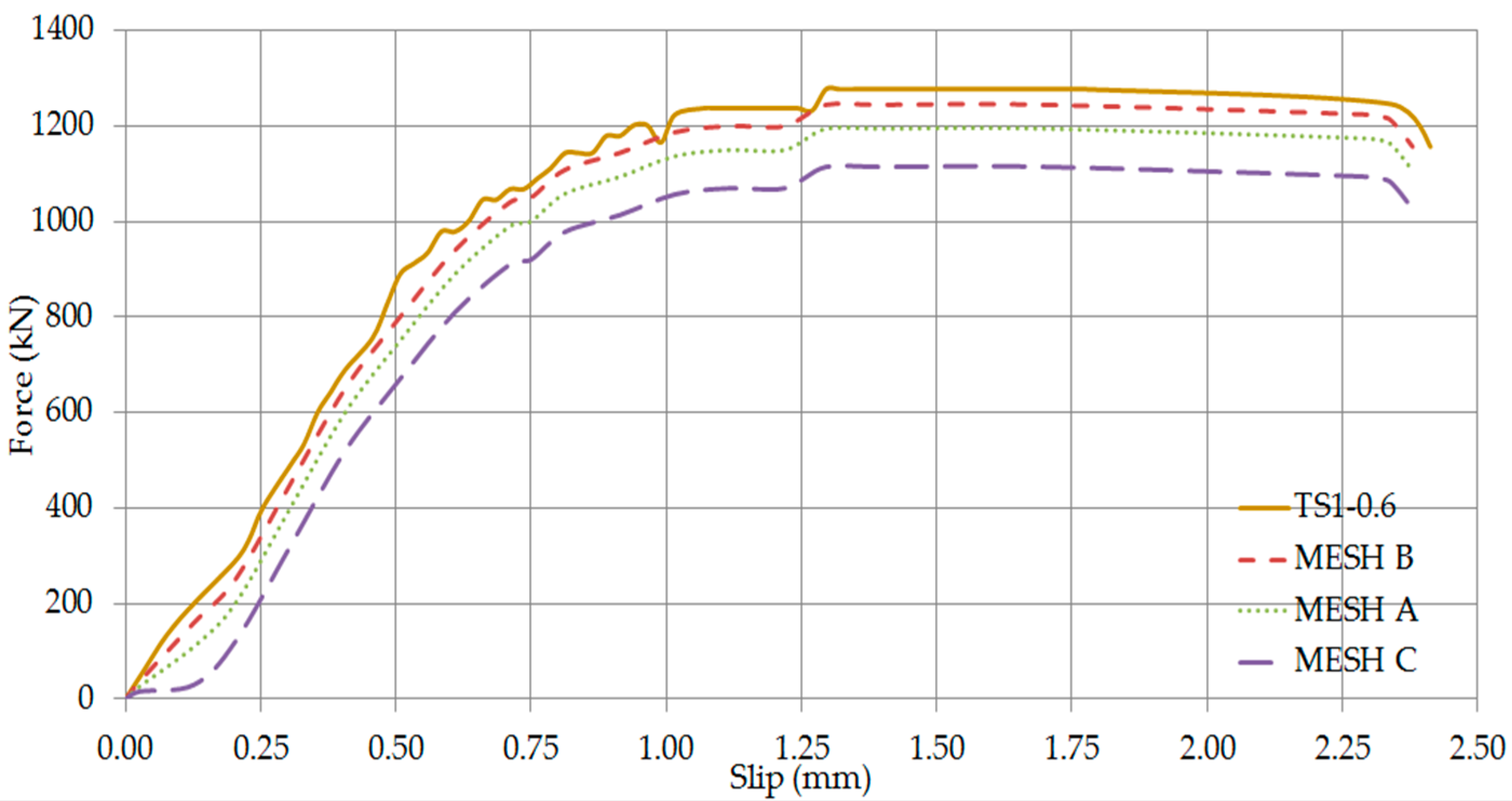
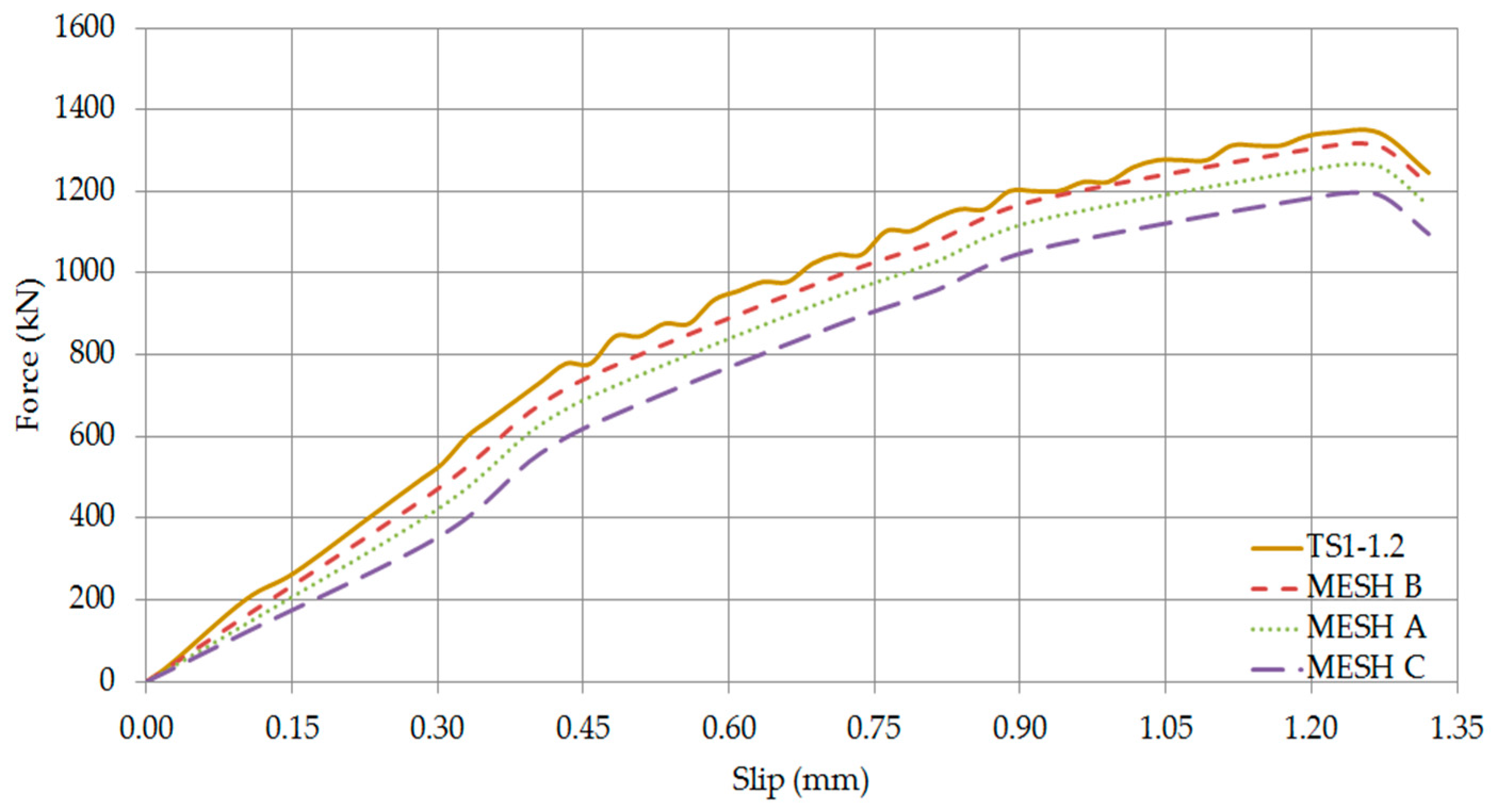


| Loading | Specimen | H | L | t | tp | sx | sy | b | Slenderness | fy | fc |
|---|---|---|---|---|---|---|---|---|---|---|---|
| mm | mm | mm | mm | mm | mm | mm | MPa | MPa | |||
| Axial Compression | NS50 | 960 | 960 | 246.4 | 3.2 | 160.0 | 160.0 | - | 50.0 | 299.1 | 23.5 |
| NS75 | 960 | 960 | 246.4 | 3.2 | 240.0 | 240.0 | - | 75.0 | 299.1 | 23.5 | |
| NS100 | 1280 | 960 | 246.4 | 3.2 | 320.0 | 320.0 | - | 100.0 | 299.1 | 23.5 | |
| NS20 | 640 | 640 | 200.0 | 3.2 | 65.0 | 65.0 | - | 20.0 | 287.0 | 31.2 | |
| NS30 | 640 | 640 | 200.0 | 3.2 | 97.0 | 97.0 | - | 30.0 | 287.0 | 31.2 | |
| NS40 | 640 | 640 | 200.0 | 3.2 | 130.0 | 130.0 | - | 40.0 | 287.0 | 31.2 | |
| NS50 | 640 | 640 | 200.0 | 3.2 | 162.0 | 162.0 | - | 50.0 | 287.0 | 31.2 | |
| C24/490-T6B20 | 380 | 280 | 250.0 | 6.0 | 90.0 | 120.0 | - | 20.0 | 428.5 | 24.0 | |
| C24/490-T6B30 | 500 | 370 | 250.0 | 6.0 | 135.0 | 180.0 | - | 30.0 | 428.5 | 24.0 | |
| C24/490-T6B40 | 620 | 460 | 250.0 | 6.0 | 180.0 | 240.0 | - | 40.0 | 428.5 | 24.0 | |
| H16/490-T6B20 | 380 | 280 | 250.0 | 6.0 | 90.0 | 120.0 | - | 20.0 | 428.5 | 16.0 | |
| H16/490-T6B30 | 500 | 370 | 250.0 | 6.0 | 135.0 | 180.0 | - | 30.0 | 428.5 | 16.0 | |
| H16/490-T6B40 | 620 | 460 | 250.0 | 6.0 | 180.0 | 240.0 | - | 40.0 | 428.5 | 16.0 | |
| TS1-0.6 | 490 | 280 | 139.7 | 4.7 | 70.0 | 70.0 | - | 15.0 | 275.0 | 16.0 | |
| TS1-0.8 | 552 | 276 | 137.2 | 4.7 | 92.0 | 92.0 | - | 19.7 | 275.0 | 16.0 | |
| TS1-1.2 | 560 | 280 | 152.4 | 4.7 | 140.0 | 140.0 | - | 30.0 | 275.0 | 16.0 | |
| TS1-1.4 | 486 | 324 | 146.1 | 4.7 | 162.0 | 162.0 | - | 34.7 | 275.0 | 16.0 | |
| TS1-1.6 | 552 | 368 | 146.1 | 4.7 | 184.0 | 184.0 | - | 39.4 | 275.0 | 16.0 | |
| TS2-0.6 | 495 | 330 | 174.9 | 4.9 | 82.6 | 82.6 | - | 16.9 | 259.2 | 30.3 | |
| TS2-0.8 | 495 | 330 | 174.9 | 4.9 | 111.1 | 111.1 | - | 22.7 | 259.2 | 30.3 | |
| TS2-1.0 | 495 | 330 | 174.9 | 4.9 | 136.5 | 136.5 | - | 27.9 | 259.2 | 32.2 | |
| TS2-1.2 | 495 | 330 | 174.9 | 4.9 | 165.1 | 165.1 | - | 33.7 | 259.2 | 32.2 | |
| Shear& Axial | S2-15-NN | 1200 | 1200 | 200.0 | 2.3 | 70.0 | 70.0 | - | 30.4 | 340.0 | 41.6 |
| S2-30-NN | 1200 | 1200 | 200.0 | 2.3 | 70.0 | 70.0 | - | 30.4 | 340.0 | 42.0 | |
| S3-15-NN | 1200 | 1200 | 200.0 | 3.2 | 100.0 | 100.0 | - | 31.3 | 351.0 | 41.6 | |
| S3-30-NN | 1200 | 1200 | 200.0 | 3.2 | 100.0 | 100.0 | - | 31.3 | 351.0 | 40.1 | |
| In-plane Shear | S2-00-NN | 1200 | 1200 | 200.0 | 2.3 | 70.0 | 70.0 | - | 30.4 | 340.0 | 42.2 |
| S3-00-NN | 1200 | 1200 | 200.0 | 3.2 | 100.0 | 100.0 | - | 31.3 | 351.0 | 41.9 | |
| S4-00-NN | 1200 | 1200 | 200.0 | 4.5 | 135.0 | 135.0 | - | 30.0 | 346.0 | 42.8 | |
| SC1 | 1524 | 1524 | 305.0 | 4.8 | 102.0 | 102.0 | 305.0 | 21.3 | 262.0 | 30.3 | |
| SC2 | 1524 | 1524 | 305.0 | 4.8 | - | - | 152.0 | 31.7 | 262.0 | 30.3 | |
| SC3 | 1524 | 1524 | 229.0 | 4.8 | 114.0 | 114.0 | 229.0 | 23.8 | 262.0 | 36.5 | |
| SC4 | 1524 | 1524 | 229.0 | 4.8 | - | - | 114.0 | 23.8 | 262.0 | 36.5 |
| Specimen | Results | Py | Pu | δy | δu | Failure Mode |
|---|---|---|---|---|---|---|
| (kN) | (kN) | (mm) | (mm) | |||
| NS50 | Test | 5884.0 | 7256.9 | 1.88 | 4.25 | (a) buckling of surface plates (b) yielding of side plates (c) ultimate strength (d) rapid loss of load carrying capacity |
| ANSYS | 5852.2 | 7132.1 | 1.85 | 4.08 | ||
| Error% | 0.54 | 1.72 | 1.60 | 4.00 | ||
| NS75 | Test | 5001.4 | 7011.8 | 1.5 | 3.5 | |
| ANSYS | 4976.5 | 6915.3 | 1.48 | 3.43 | ||
| Error% | 0.50 | 1.38 | 1.33 | 2.00 | ||
| NS100 | Test | 5393.7 | 7364.8 | 2.38 | 4.63 | |
| ANSYS | 5371.2 | 7245.1 | 2.35 | 4.59 | ||
| Error% | 0.42 | 1.63 | 1.26 | 0.86 | ||
| NS20 | Test | 3210.0 | 5730.2 | 0.7 | 2.58 | extensive local buckling & concrete crushing |
| ANSYS | 3189.3 | 5620.1 | 0.66 | 2.41 | ||
| Error% | 0.64 | 1.92 | 5.71 | 6.59 | ||
| NS30 | Test | 4710.0 | 5470.0 | 2.17 | 3.28 | |
| ANSYS | 4683.3 | 5392.1 | 2.14 | 3.16 | ||
| Error% | 0.57 | 1.42 | 1.38 | 3.66 | ||
| NS40 | Test | 4940.0 | 4999.8 | 3.3 | 3.65 | |
| ANSYS | 4916.3 | 4879.9 | 3.26 | 3.58 | ||
| Error% | 0.48 | 2.40 | 1.21 | 1.92 | ||
| NS50 | Test | 4120.0 | 5050.0 | 3.2 | 4.18 | |
| ANSYS | 4101.1 | 4945.9 | 3.19 | 4.11 | ||
| Error% | 0.46 | 2.06 | 0.31 | 1.67 |
| Specimen | Variables | ||||||
|---|---|---|---|---|---|---|---|
| H (mm) | t (mm) | tp (mm) | fy (MPa) | fc (MPa) | Aspect Ratio (H/L) | Reinf. Ratio (%) | |
| AE1 | 1500 | 200 | 4 | 235 | 30 | 0.75 | 4.6 |
| AE2 | 1500 | 200 | 4 | 235 | 40 | 0.75 | 4.6 |
| AE3 | 1500 | 200 | 4 | 355 | 30 | 0.75 | 4.6 |
| AE4 | 1500 | 200 | 4 | 355 | 40 | 0.75 | 4.6 |
| AE5 | 1500 | 200 | 8 | 235 | 30 | 0.75 | 9.6 |
| AE6 | 1500 | 200 | 8 | 235 | 40 | 0.75 | 9.6 |
| AE7 | 1500 | 200 | 8 | 355 | 30 | 0.75 | 9.6 |
| AE8 | 1500 | 200 | 8 | 355 | 40 | 0.75 | 9.6 |
| AE9 | 4000 | 200 | 4 | 235 | 30 | 2.0 | 4.6 |
| AE10 | 4000 | 200 | 4 | 235 | 40 | 2.0 | 4.6 |
| AE11 | 4000 | 200 | 4 | 355 | 30 | 2.0 | 4.6 |
| AE12 | 4000 | 200 | 4 | 355 | 40 | 2.0 | 4.6 |
| AE13 | 4000 | 200 | 8 | 235 | 30 | 2.0 | 9.6 |
| AE14 | 4000 | 200 | 8 | 235 | 40 | 2.0 | 9.6 |
| AE15 | 4000 | 200 | 8 | 355 | 30 | 2.0 | 9.6 |
| AE16 | 4000 | 200 | 8 | 355 | 40 | 2.0 | 9.6 |
| Specimen | Yielding Point | Ultimate Point | Failure Point | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Vy (kN) | δy (mm) | dy (%) | Vu (kN) | δu (mm) | du (%) | Vuf (kN) | δf (mm) | df (%) | |
| AE1 | 1192.1 | 1.54 | 0.103 | 2286.4 | 4.68 | 0.31 | 1829.1 | 21.60 | 1.44 |
| AE2 | 1659.2 | 1.02 | 0.068 | 2851.3 | 5.04 | 0.34 | 2281.0 | 21.90 | 1.46 |
| AE3 | 2197.4 | 2.24 | 0.149 | 3305.0 | 6.30 | 0.42 | 2644.0 | 20.55 | 1.37 |
| AE4 | 2717.9 | 2.05 | 0.136 | 4261.4 | 6.66 | 0.44 | 3409.1 | 21.15 | 1.41 |
| AE5 | 2023.9 | 1.03 | 0.069 | 4225.8 | 4.91 | 0.33 | 3380.6 | 20.40 | 1.36 |
| AE6 | 2571.1 | 1.38 | 0.092 | 5386.8 | 6.35 | 0.42 | 4309.4 | 20.70 | 1.38 |
| AE7 | 3345.0 | 2.70 | 0.180 | 7219.4 | 7.86 | 0.52 | 5775.5 | 19.35 | 1.29 |
| AE8 | 3727.6 | 2.40 | 0.160 | 8233.6 | 7.77 | 0.52 | 6586.9 | 19.95 | 1.33 |
| AE9 | 306.9 | 3.07 | 0.077 | 507.1 | 35.17 | 0.88 | 405.7 | 73.20 | 1.83 |
| AE10 | 385.8 | 2.99 | 0.075 | 564.9 | 39.99 | 1.00 | 451.9 | 76.80 | 1.92 |
| AE11 | 431.5 | 9.13 | 0.228 | 965.3 | 51.19 | 1.28 | 772.2 | 70.40 | 1.76 |
| AE12 | 507.1 | 7.12 | 0.178 | 1045.3 | 52.06 | 1.30 | 836.3 | 75.20 | 1.88 |
| AE13 | 533.8 | 6.00 | 0.150 | 1174.3 | 40.86 | 1.02 | 939.5 | 70.00 | 1.75 |
| AE14 | 649.4 | 5.74 | 0.143 | 1290.0 | 49.00 | 1.22 | 1032.0 | 73.60 | 1.84 |
| AE15 | 840.7 | 11.62 | 0.290 | 2157.4 | 71.65 | 1.79 | 1725.9 | 67.20 | 1.68 |
| AE16 | 916.3 | 10.54 | 0.264 | 2410.9 | 71.38 | 1.78 | 1928.7 | 72.00 | 1.80 |
| Specimen | Yielding Point | Ultimate Point | Failure Point | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Vy (kN) | δy (mm) | Vu (kN) | δu (mm) | Vuf (kN) | δf (mm) | ||||||
| + | - | + | - | + | - | + | - | + | - | ||
| AE1 | 1222.3 | 1185.6 | 1.54 | 2289.6 | 2175.1 | 4.62 | 4.62 | 1831.7 | 1740.1 | 21.56 | 21.56 |
| AE2 | 1692.9 | 1642.5 | 1.02 | 2854.4 | 2739.4 | 5.10 | 5.10 | 2283.5 | 2191.6 | 21.42 | 21.42 |
| AE3 | 2255.2 | 2188.2 | 2.24 | 3309.7 | 3144.2 | 6.50 | 6.50 | 2647.8 | 2515.4 | 20.10 | 20.10 |
| AE4 | 2775.7 | 2693.2 | 2.05 | 4266.3 | 4095.7 | 6.88 | 6.88 | 3413.1 | 3276.6 | 20.66 | 20.66 |
| AE5 | 2054.9 | 1993.8 | 1.03 | 4231.7 | 4062.4 | 5.03 | 5.01 | 3385.4 | 3250.0 | 20.07 | 20.07 |
| AE6 | 2597.5 | 2520.3 | 1.38 | 5394.3 | 5178.5 | 6.53 | 6.52 | 4315.4 | 4142.8 | 20.34 | 20.34 |
| AE7 | 3399.6 | 3298.5 | 2.70 | 7229.5 | 6940.4 | 8.07 | 8.06 | 5783.6 | 5552.3 | 19.04 | 19.04 |
| AE8 | 3769.7 | 3657.6 | 2.40 | 8245.1 | 7915.3 | 7.98 | 7.98 | 6596.1 | 6332.3 | 19.60 | 19.60 |
| AE9 | 290.0 | 303.8 | 3.07 | 489.9 | 470.3 | 33.77 | 37.15 | 392.9 | 376.2 | 70.61 | 77.67 |
| AE10 | 365.7 | 379.9 | 2.99 | 547.4 | 525.5 | 38.87 | 42.76 | 440.6 | 420.4 | 74.75 | 78.5 |
| AE11 | 407.2 | 426.2 | 9.13 | 934.9 | 897.5 | 50.06 | 55.07 | 748.9 | 718.0 | 68.43 | 70.5 |
| AE12 | 481.1 | 498.9 | 7.12 | 1012.8 | 972.2 | 50.86 | 55.94 | 818.3 | 777.8 | 73.32 | 77.7 |
| AE13 | 511.6 | 529.4 | 6.00 | 1149.2 | 1103.2 | 39.97 | 43.97 | 922.0 | 882.6 | 67.20 | 69.2 |
| AE14 | 622.8 | 643.0 | 5.74 | 1253.3 | 1203.2 | 47.91 | 52.71 | 1005.9 | 962.6 | 70.58 | 76.2 |
| AE15 | 805.7 | 832.2 | 11.62 | 2100.6 | 2016.6 | 70.07 | 77.07 | 1699.6 | 1613.3 | 64.38 | 66.3 |
| AE16 | 876.9 | 906.2 | 10.54 | 2343.2 | 2249.5 | 69.74 | 76.71 | 1890.9 | 1799.6 | 69.12 | 74.6 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elmatzoglou, M.; Avdelas, A. Numerical Modelling of Double-Steel Plate Composite Shear Walls. Computation 2017, 5, 12. https://doi.org/10.3390/computation5010012
Elmatzoglou M, Avdelas A. Numerical Modelling of Double-Steel Plate Composite Shear Walls. Computation. 2017; 5(1):12. https://doi.org/10.3390/computation5010012
Chicago/Turabian StyleElmatzoglou, Michaela, and Aris Avdelas. 2017. "Numerical Modelling of Double-Steel Plate Composite Shear Walls" Computation 5, no. 1: 12. https://doi.org/10.3390/computation5010012
APA StyleElmatzoglou, M., & Avdelas, A. (2017). Numerical Modelling of Double-Steel Plate Composite Shear Walls. Computation, 5(1), 12. https://doi.org/10.3390/computation5010012






