A Computational Study of Solid Si Target Dynamics under ns Pulsed Laser Irradiation from Elastic to Melting Regime
Abstract
:1. Introduction
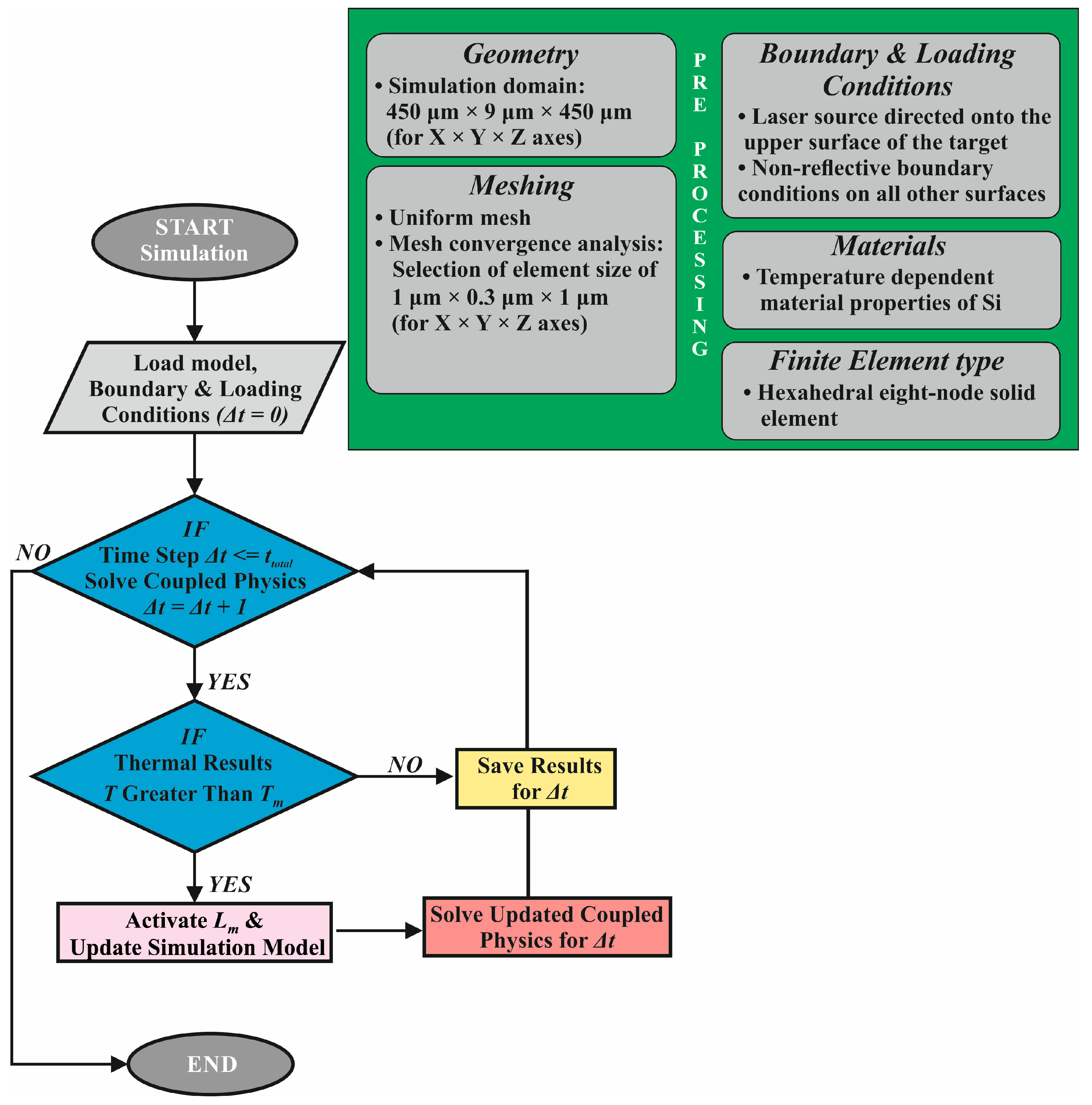
2. Numerical Modeling and Simulation
3. Results and Discussion
3.1. Thermomechanical Response
3.2. Ultrasound Evolution
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- OPTEC. Laser Material Removal: Drilling Cutting and Marking; University of Central Florida: Orlando, FL, USA, 2008. [Google Scholar]
- Steen, W.M.; Mazumder, J. Laser Material Processing, 4th ed.; Springer: London, UK, 2010. [Google Scholar]
- Yang, J.; Sun, S.; Brandt, M.; Yan, W. Experimental investigation and 3D finite element prediction of the heat affected zone during laser assisted machining of Ti6Al4V alloy. J. Mater. Process. Technol. 2010, 210, 2215–2222. [Google Scholar] [CrossRef]
- Available online: https://www.worldoflasers.com/laserapplindustrial.htm (accessed on 26 September 2023).
- Pouli, P.; Papakonstantinou, E.; Frantzikinaki, K.; Panou, A.; Frantzi, G.; Vasiliadis, C.; Fotakis, C. The two-wavelength laser cleaning methodology; theoretical background and examples from its application on CH objects and monuments with emphasis to the Athens Acropolis sculptures. Herit. Sci. 2016, 4, 9. [Google Scholar] [CrossRef]
- Brown, M.S.; Arnold, C.B. Fundamentals of Laser-Material Interaction and Application to Multiscale Surface Modification. In Laser Precision Microfabrication; Springer: Berlin/Heidelberg, Germany, 2010; Available online: https://spikelab.mycpanel.princeton.edu/papers/book02.pdf (accessed on 26 September 2023).
- Guo, L.; Geng, S.; Gao, X.; Wang, W. Numerical simulation of heat transfer and fluid flow during nanosecond pulsed laser processing of Fe78Si9B13 amorphous alloys. Int. J. Heat Mass Transf. 2021, 170, 121003. [Google Scholar] [CrossRef]
- Gao, J.; Cao, Y.; Lu, L.; Hu, Z.; Wang, K.; Guo, F.; Yan, Y. Study on the interaction between nanosecond laser and 6061 aluminum alloy considering temperature dependence. J. Alloys Compd. 2022, 892, 162044. [Google Scholar] [CrossRef]
- Kyota, H.; Higashi, M.; Curosu, T.; Lida, M. Numerical simulation and validation of subsurface modification and crack formation induced by nanosecond-pulsed laser processing in monocrystalline silicon. J. Appl. Phys. 2021, 130, 239901. [Google Scholar] [CrossRef]
- Acosta-Zepeda, C.; Saavedra, P.; Bonse, J.; Haro-Poniatowski, E. Modeling of silicon surface topographies induced by single nanosecond laser pulse induced melt-flows. J. Appl. Phys. 2019, 125, 175101. [Google Scholar] [CrossRef]
- Zeng, W.; Yao, Y. Numerical simulation of laser-generated ultrasonic waves for detection surface defect on a cylinder pipe. Optik 2020, 212, 164650. [Google Scholar] [CrossRef]
- Orphanos, Y.; Dimitriou, V.; Kaselouris, E.; Bakarezos, E.; Vainos, N.; Tatarakis, M.; Papadogiannis, N.A. An integrated method for material properties characterization based on pulsed laser generated surface acoustic waves. Microelectron. Eng. 2013, 112, 249–254. [Google Scholar] [CrossRef]
- Marine, W.; Bulgakova, N.M.; Patrone, L.; Ozerov, I. Insight into electronic mechanisms of nanosecond-laser ablation of silicon. J. Appl. Phys. 2008, 103, 094902. [Google Scholar] [CrossRef]
- Darif, M.; Semmar, N. Numerical Simulation of Si Nanosecond Laser Annealing by COMSOL Multiphysics. In Proceedings of the COMSOL Conference, Hannover, Germany, 5 November 2008. [Google Scholar]
- Erdoğan, E.; Kundakçı, M.; Mantarcı, A. InGaN thin film deposition on Si(100) and glass substrates by termionic vacuum arc. J. Phys. Conf. Ser. 2016, 707, 012019. [Google Scholar] [CrossRef]
- Domke, M.; Egle, B.; Stroj, S.; Bodea, M.; Schwarz, E.; Fasching, G. Ultrafast-laser dicing of thin silicon wafers: Strategies to improve front- and backside breaking strength. Appl. Phys. A 2017, 123, 746. [Google Scholar] [CrossRef]
- Wang, D.; Wang, Z.; Zhang, Z.; Yue, Y.; Li, D.; Maple, C. Direct modification of silicon surface by nanosecond laser interference lithography. Appl. Surf. Sci. 2013, 282, 67–72. [Google Scholar] [CrossRef]
- Ernst, O.C.; Uebel, D.; Kayser, S.; Lange, F.; Teubner, T.; Boeck, T. Revealing all states of dewetting of a thin gold layer on a silicon surface by nanosecond laser conditioning. Appl. Surf. Sci. Adv. 2021, 3, 100040. [Google Scholar] [CrossRef]
- Podymova, N.B.; Kalashnikov, I.E.; Bolotova, L.K.; Kobeleva, L.I. Laser-ultrasonic nondestructive evaluation of porosity in particulate reinforced metal-matrix composites. Ultrasonics 2019, 99, 105959. [Google Scholar] [CrossRef] [PubMed]
- Zhong, D.; Li, Z. Assessing the dynamic characteristics of a femtosecond laser micro plasma expansion process with an optical fiber sensing probe. In Frontier Research and Innovation in Optoelectronics Technology and Industry; CRC Press: Boca Raton, FL, USA, 2018; pp. 235–239. [Google Scholar]
- Kautek, W.; Rudolph, P.; Daminelli, G.; Krüger, J. Physico-chemical aspects of femtosecond-pulse-laser-induced surface nanostructures. Appl. Phys. A 2005, 81, 65–70. [Google Scholar] [CrossRef]
- Doukas, A.G.; Flotte, T.J. Physical characteristics and biological effects of laser-induced stress waves. Ultrasound Med. Biol. 1996, 22, 151–164. [Google Scholar] [CrossRef] [PubMed]
- Lin, Z.; Hong, M. Femtosecond Laser Precision Engineering: From Micron, Submicron, to Nanoscale. Ultrafast Sci. 2021, 2021, 9783514. [Google Scholar] [CrossRef]
- Lim, A.E.; Goh, S. Effect of Microchannel Diameter on Electroosmotic Flow Hysteresis. Energies 2023, 16, 2154. [Google Scholar] [CrossRef]
- Dimitriou, V.; Kaselouris, E.; Orphanos, Y.; Bakarezos, M.; Vainos, N.; Tatarakis, M.; Papadogiannis, N.A. Three-dimensional transient behavior of thin films surface under pulsed laser excitation. Appl. Phys. Lett. 2013, 103, 114104. [Google Scholar] [CrossRef]
- Kaselouris, E.; Nikolos, I.K.; Orphanos, Y.; Bakarezos, E.; Papadogiannis, N.A.; Tatarakis, M.; Dimitriou, V. Elastoplastic study of nanosecond-pulsed laser interaction with metallic films using 3D multiphysics fem modeling. Int. J. Damage Mech. 2016, 25, 42–55. [Google Scholar] [CrossRef]
- Kaselouris, E.; Skarvelakis, E.; Nikolos, I.K.; Stavroulakis, G.E.; Orphanos, Y.; Bakarezos, E.; Papadogiannis, N.A.; Tatarakis, M.; Dimitriou, V. Simulation of the transient behavior of matter with characteristic geometrical variations & defects irradiated by nanosecond laser pulses using FEA. Key Eng. Mater. 2016, 665, 157–160. [Google Scholar]
- Hallquist, J. LS-DYNA Theory Manual; Livermore Software Technology Corporation: Livermore, CA, USA, 2006. [Google Scholar]
- Aris Documentation. Available online: http://doc.aris.grnet.gr/system/hardware/ (accessed on 26 September 2023).
- Orphanos, Y.; Kosma, K.; Kaselouris, E.; Vainos, N.; Dimitriou, V.; Bakarezos, M.; Tatarakis, M.; Papadogiannis, N.A. Integrated nanosecond laser full-field imaging for femtosecond laser-generated surface acoustic waves in metal film-glass substrate multilayer materials. Appl. Phys. A 2019, 125, 269. [Google Scholar] [CrossRef]
- Available online: https://www.azom.com/properties.aspx?ArticleID=599 (accessed on 26 September 2023).
- Available online: https://encyclopediaofmath.org/wiki/Lam%C3%A9_constants (accessed on 26 September 2023).
- Liu, B.; Li, S.; Li, R.; Chen, C.; Liang, L. Finite element simulation and experimental research on microcutting mechanism of single crystal silicon. Int. J. Adv. Manuf. Technol. 2020, 110, 909–918. [Google Scholar] [CrossRef]
- Available online: https://www.virginiasemi.com/?cont_uid=50 (accessed on 26 September 2023).
- Masolin, A.; Bouchard, P.O.; Martini, R.; Bernacki, M. Thermo-mechanical and fracture properties in single-crystal silicon. J. Mater. Sci. 2013, 48, 979–988. [Google Scholar] [CrossRef]
- Available online: http://www.ioffe.ru/SVA/NSM/Semicond/Si/thermal.html (accessed on 26 September 2023).
- Available online: https://www.nuclear-power.com/silicon-specific-heat-latent-heat-vaporization-fusion/ (accessed on 26 September 2023).
- Available online: https://studylib.net/doc/6931620/10.7-given-here-are-the-solidus-and-liquidus-temperatures (accessed on 26 September 2023).
- Zhan, Y.; Liu, C.; Zhang, F.; Qiu, Z. Experimental study and finite element analysis based on equivalent load method for laser ultrasonic measurement of elastic constants. Ultrasonics 2016, 69, 243–247. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Lin, B.; Liang, X.; Du, A.; Ma, X. Fracture of single crystal silicon caused by nonlinear evolution of surface acoustic waves. Eng. Fract. Mech. 2022, 269, 108505. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Papadaki, H.; Kaselouris, E.; Bakarezos, M.; Tatarakis, M.; Papadogiannis, N.A.; Dimitriou, V. A Computational Study of Solid Si Target Dynamics under ns Pulsed Laser Irradiation from Elastic to Melting Regime. Computation 2023, 11, 240. https://doi.org/10.3390/computation11120240
Papadaki H, Kaselouris E, Bakarezos M, Tatarakis M, Papadogiannis NA, Dimitriou V. A Computational Study of Solid Si Target Dynamics under ns Pulsed Laser Irradiation from Elastic to Melting Regime. Computation. 2023; 11(12):240. https://doi.org/10.3390/computation11120240
Chicago/Turabian StylePapadaki, Helen, Evaggelos Kaselouris, Makis Bakarezos, Michael Tatarakis, Nektarios A. Papadogiannis, and Vasilis Dimitriou. 2023. "A Computational Study of Solid Si Target Dynamics under ns Pulsed Laser Irradiation from Elastic to Melting Regime" Computation 11, no. 12: 240. https://doi.org/10.3390/computation11120240
APA StylePapadaki, H., Kaselouris, E., Bakarezos, M., Tatarakis, M., Papadogiannis, N. A., & Dimitriou, V. (2023). A Computational Study of Solid Si Target Dynamics under ns Pulsed Laser Irradiation from Elastic to Melting Regime. Computation, 11(12), 240. https://doi.org/10.3390/computation11120240






