Modeling the Static and Dynamic Behaviors of a Large Heavy-Duty Lathe Machine under Rated Loads
Abstract
1. Introduction
2. Finite Element Modeling Approach
2.1. Description of Lathe Machinery
2.2. Finite Element Model
2.3. Analysis Cases
2.3.1. Static Analysis
2.3.2. Modal Analysis
2.3.3. Harmonic Analysis
2.3.4. Effects of Workpiece Load
3. Results and Discussions
3.1. Static Stiffness
3.2. Vibration Mode Characteristics
3.3. Dynamic Characteristics—FRFs
3.3.1. Spindle Chuck and Tailstock
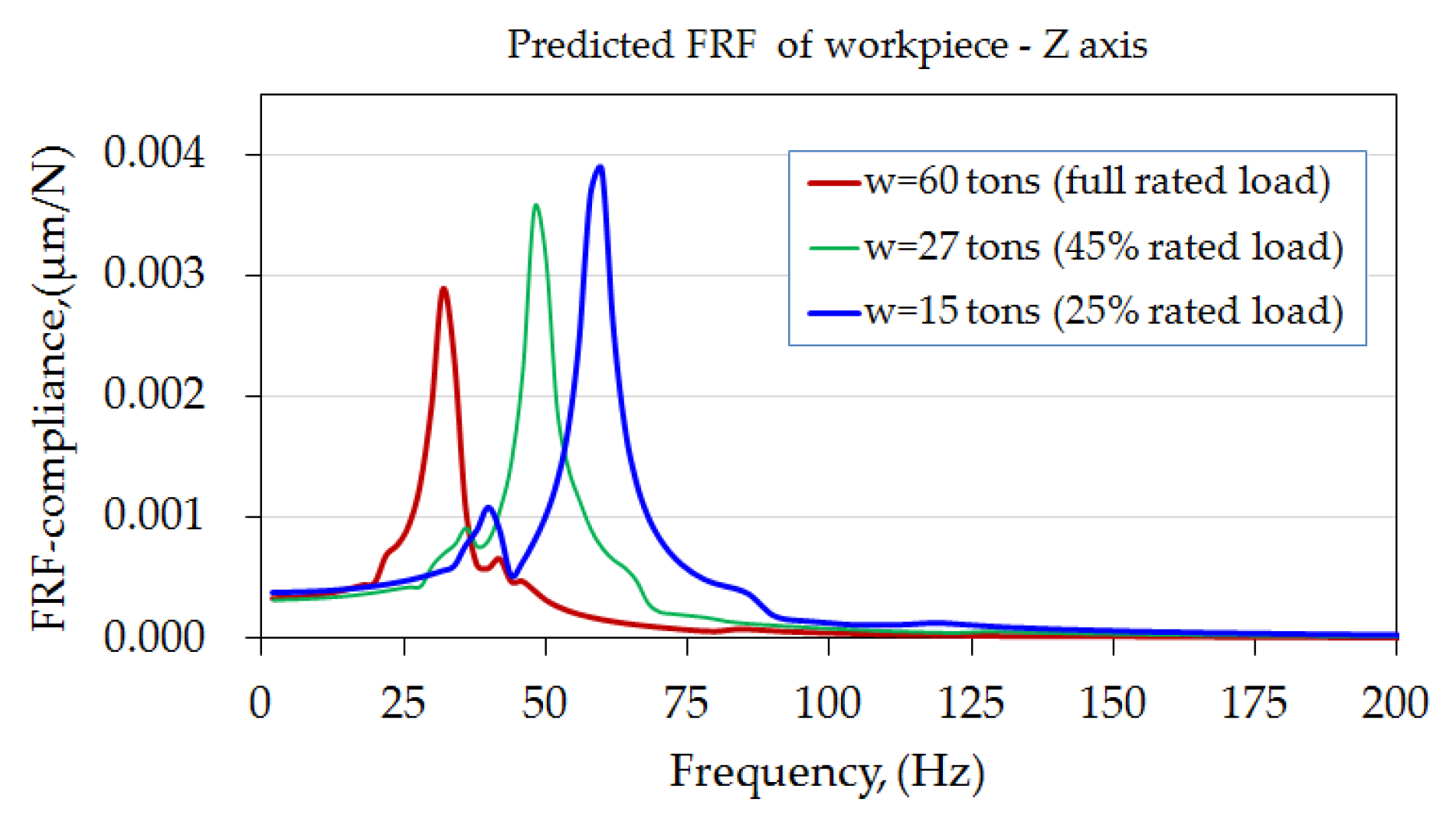
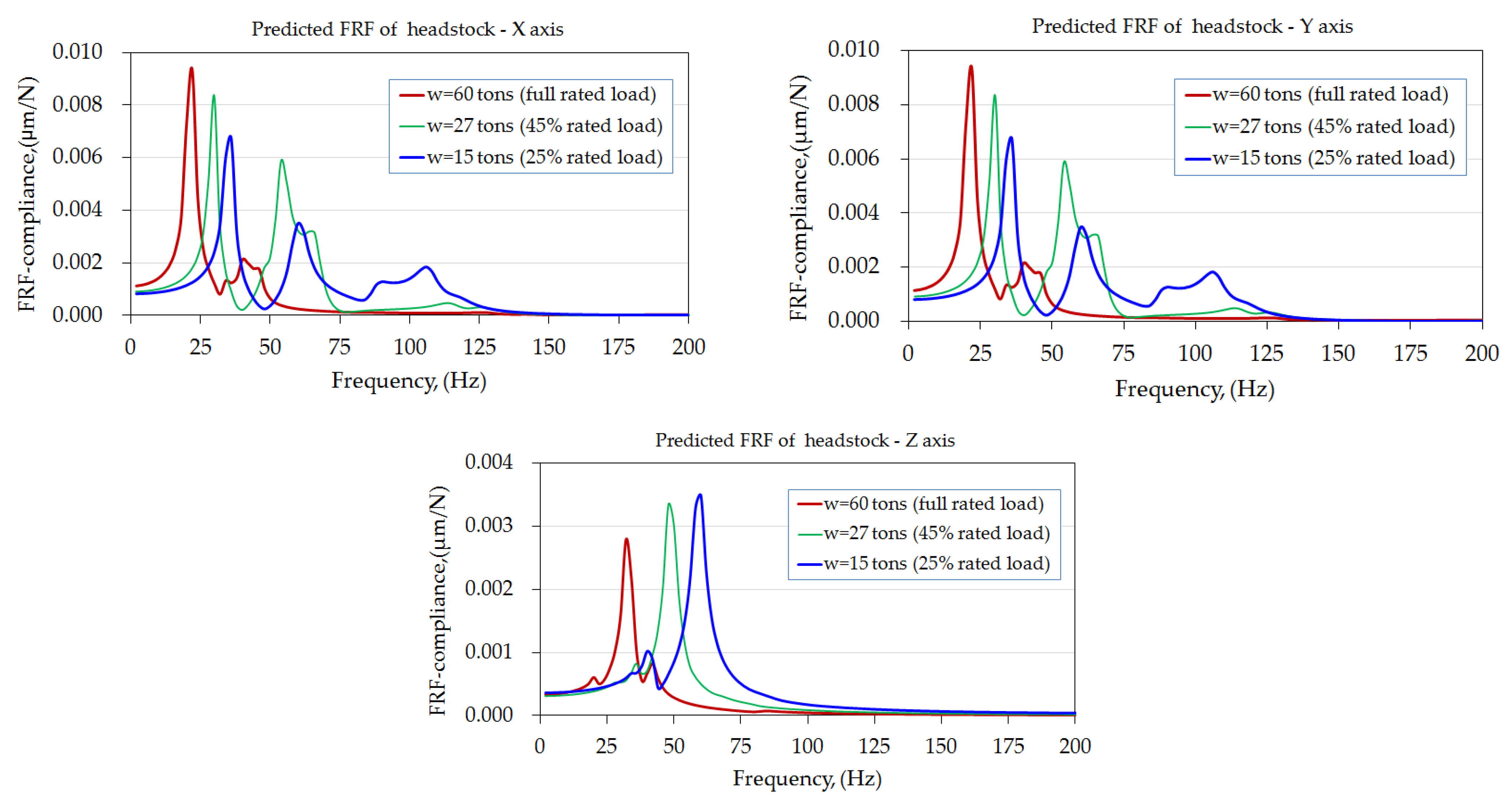
3.3.2. Effects of Workpiece Weight
- (a)
- FRFs of the workpiece
- (b)
- FRFs of the headstock and tailstock
4. Conclusions
- The machine bed had a higher rigidity against workpiece loads, whereas the headstock and tailstock comprised the critical modules dominating the load capacity.
- The dynamic characteristics of the entire machine loaded with a workpiece subjected to a simulated cutting force were affected by the change in compliance and resonant frequency according to the workpiece weight.
- The lowest natural frequency of the lathe machine was approximately 22 Hz—significantly higher than the maximum rotational speed of the spindle, which was rated at 450 rpm. This is a favorable condition for the prevention of vibration resonance during machining.
- Finally, the structural stiffness of the headstock and tailstock substantially influenced the dynamic compliance of the workpiece under the machining force. The two modules exhibited superior rigidity in the longitudinal direction, and the rigidity in the vertical direction was higher than that in the lateral direction. In addition, the static and dynamic stiffness of the large heavy-duty lathe machine exhibited characteristics comparable to those presented in previous literature.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Uriarte, L.; Zatarain, M.; Axinte, D.; Yagüe-Fabra, J.; Ihlenfeldt, S.; Eguia, J.; Olarra, A. Machine tools for large parts. CIRP Ann.-Manuf. Technol. 2013, 62, 731–750. [Google Scholar] [CrossRef]
- An, H.S.; Cho, Y.J.; Choi, Y.H.; Lee, D.W. Development of a multi-tasking machine tool for machining large scale marine engine crankshafts and its design technologies. J. Korean Soc. Precis. Eng. 2012, 29, 139–146. [Google Scholar] [CrossRef][Green Version]
- Jang, S.H.; Choi, Y.H.; Kim, S.T.; An, H.S.; Choi, H.B.; Hong, J.S. Development of core technologies of multi-tasking machine tools for machining highly precision large parts. J. Korean Soc. Precis. Eng. 2012, 29, 129–138. [Google Scholar] [CrossRef]
- Maschinenbau, W. Customized Machine Tools for Heavy-Duty Machining. Available online: https://www.sme.org/technologies/articles/2020/june. (accessed on 5 June 2022).
- Tekeli, A.; Budak, E. Maximization of chatter-free material removal rate in end milling using analytical methods. Mach. Sci. Technol. 2005, 9, 147–167. [Google Scholar] [CrossRef]
- Budak, E.; Tekeli, A. Maximizing chatter free material removal rate in milling through optimal selection of axial and radial depth of cut pairs. CIRP Ann.-Manuf. Technol. 2005, 54, 353–356. [Google Scholar] [CrossRef]
- Altintas, Y.; Budak, E. Analytical prediction of stability lobes in milling. CIRP Ann.-Manuf. Technol. 1995, 44, 357–362. [Google Scholar] [CrossRef]
- Urbikain, G.; Campa, F.J.; Zulaika, J.J.; De Lacalle, L.N.L.; Alonso, M.A.; Collado, V. Preventing chatter vibrations in heavy-duty turning operations in large horizontal lathes. J. Sound Vib. 2015, 340, 317–330. [Google Scholar] [CrossRef]
- Hung, J.P.; Lai, Y.L.; Lou, T.L. Analysis of the machining stability of a milling machine considering the effect of machine frame structure and spindle bearings: Experimental and finite element approaches. Int. J. Adv. Manuf. Technol. 2013, 8, 2393–2405. [Google Scholar] [CrossRef]
- Hong, C.C.; Chang, C.L.; Lin, C.Y. Static structural analysis of great five-axis turning–milling complex CNC machine. Eng. Sci. Technol. Int. J. 2016, 19, 1971–1984. [Google Scholar] [CrossRef]
- Hong, C.C. Dynamic structural analysis of great five-axis turning-milling complex CNC machine. Glob. J. Eng. Res. 2017, 17, 1–8. [Google Scholar]
- Wang, F.Q.; Rui, Z.Y.; Wu, Q. Structural dynamic analysis and optimization of precision NC machine tool bed based on the unit structure. Appl. Mech. Mater. 2011, 44, 200–205. [Google Scholar] [CrossRef]
- Hung, J.P.; Wu, K.D.; Lin, W.Z.; Shih, W.C. Analyzing the dynamic characteristics of milling tool using finite element method and receptance coupling method. Eng. Appl. Sci. Res. 2019, 9, 3918–3923. [Google Scholar] [CrossRef]
- Chen, T.C.; Chen, Y.J.; Hung, M.H.; Hung, J.P. Design analysis of machine tool structure with artificial granite material. Adv. Mech. Eng. 2016, 8, 1687814016656533. [Google Scholar] [CrossRef]
- Ahmad, S.F.; Jagadeesha, T. Dynamic characteristics analysis of kirloskar turn master35 machine tool bed with different polymer concrete materials. In Modeling, Simulation and Optimization; Springer: Singapore, 2022; pp. 329–338. [Google Scholar]
- Chinnuraj, S.; Thyla, P.R.; Elango, S.; Venugopal, P.R.; Mohanram, P.V.; Nataraj, M.; Ayyasamy, S. Static and dynamic behavior of steel-reinforced epoxy granite CNC lathe bed using finite element analysis. Proc. Inst. Mech. Eng. Pt. L J. Mater. Des. Appl. 2020, 234, 595–609. [Google Scholar] [CrossRef]
- Venugopal, P.R.; Kalayarasan, M.; Thyla, P.R.; Mohanram, P.V.; Nataraj, M.; Mohanraj, S.; Sonawane, H. Structural investigation of steel-reinforced epoxy granite machine tool column by finite element analysis. Proc. Inst. Mech. Eng. Pt. L J. Mater. Des. Appl. 2019, 233, 2267–2279. [Google Scholar] [CrossRef]
- Dunaj, P.; Powałka, B.; Berczyński, S.; Chodźko, M.; Okulik, T. Increasing lathe machining stability by using a composite steel–polymer concrete frame. CIRP J. Manuf. Sci. 2020, 31, 1–13. [Google Scholar] [CrossRef]
- Dunaj, P.; Dolata, M.; Tomaszewski, J.; Majda, P. Static stiffness design of vertical lathe with steel-polymer concrete frame. Int. J. Adv. Manuf. Technol. 2022, 121, 1149–1160. [Google Scholar] [CrossRef]
- Lai, Y.L.; Chen, Y.R.; Hung, J.P.; Luo, T.L.; Hsiao, H.H. Effect of the machine frame structures on the frequency responses of spindle tool. Int. J. Mech. Mechatron. Eng. 2012, 6, 1862–1867. [Google Scholar]
- Dounar, S.; Iakimovitch, A.; Jakubowski, A. Finite Element Method analysis of the deformation of the shaft and supports of a large, precise lathe–Cutting force excitation. Sci. J. Marit. Univ. Szczec. 2020, 62, 91–98. [Google Scholar]
- Zhang, Y.; Liu, C. Study on the dynamic performance of spindle assemble and headstock. In Proceedings of the 2010 IEEE International Conference on Mechatronics and Automation, Xi’an, China, 4–7 August 2010; pp. 1121–1126. [Google Scholar]
- Dounar, S.; Jakubowski, A. Dynamic Finite Element Analysis of Rotor-Shaft Fastening into a Heavy Precise Lathe. Sci. J. Marit. Univ. Szczec. 2021, 66, 37–45. [Google Scholar]
- Olvera, D.; de Lacalle, L.N.; Compeán, F.I.; Fz-Valdivielso, A.; Lamikiz, A.; Campa, F.J. Analysis of the tool tip radial stiffness of turn-milling centers. Int. J. Adv. Manuf. Technol. 2012, 60, 883–891. [Google Scholar] [CrossRef]
- Hung, J.P.; Lin, W.Z.; Chen, Y.J.; Luo, T.L. Investigation of the machining stability of a milling machine with hybrid guideway systems. Appl. Sci. 2016, 6, 76. [Google Scholar] [CrossRef]
- Brewe, D.E.; Hamrock, B.J. Simplified solution for elliptical-contact deformation between two elastic solid. J. Lubr. Technol. 1997, 99, 485–487. [Google Scholar] [CrossRef]
- Lundberg, G.; Sjövall, H. Stress and Deformation in Elastic Contacts; Institute of Theory of Elasticity and Strength of Materials, Chalmers Inst. Tech.: Gothenburg, Sweden, 1958. [Google Scholar]
- Palmgren, A. Ball and Roller Bearing Engineering; SKF Industries Inc.: Philadelphia, PA, USA; Burbank, CA, USA, 1959. [Google Scholar]
- de Lacalle, N.L.; Mentxaka, A.L. Machine Tools for High Performance Machining; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Ryabov, O.; Kano, S.; Sawada, H.; Herwan, J. Ratio of cutting force components in turning. Mater. Sci. Forum. 2018, 940, 65–71. [Google Scholar] [CrossRef]
- Guo, C.G.; Bai, L.G.; Zheng, B.L.; Pan, Y.S. Spindle static and dynamic characteristics analysis of precision CNC turning center. Adv. Mat. Res. 2013, 619, 47–50. [Google Scholar] [CrossRef]
- Dounar, S.; Lakimovitch, A.; Ausiyevich, A.; Jakubowski, A. FEA-analysis of shaft and supports deformations for huge precise lathe. Statics and resonances. New Trends Prod. Eng. 2018, 1, 341–348. [Google Scholar] [CrossRef][Green Version]
- Choi, Y.H.; Ha, G.B.; An, H.S. Stiffness evaluation of a heavy-duty multi-tasking lathe for large size crankshaft using random excitation test. J. Korean Soc. Precis. Eng. 2014, 31, 627–634. [Google Scholar] [CrossRef][Green Version]
















| Item | Specification | |
|---|---|---|
| Nominal center distance (headstock to tailstock) | 12,050 mm | |
| Bed length | 17,420 mm | |
| Maximum swing diameter over bed | 2400 mm | |
| Maximum workpiece weight | 60 tons | |
| Spindle headstock | Motor power | 75/90 kw |
| Maximum speed | 450 rpm | |
| Four-jawed chuck diameter | 1520 mm | |
| Clamping force | 980 kg | |
| Tailstock | Shaft diameter | 290 mm |
| Center apex | MT#7 | |
| Axial trust force | 15,000 kg | |
| Characteristic | Primary Module | |||
|---|---|---|---|---|
| Headstock Chuck | Base at Roller 1 | Base at Roller 2 | Tailstock Center | |
| Total reaction (N) | 27,676 | 247,830 | 261,000 | 46,509 |
| Deflection (μm) | 25.1 | 27.2 | 23.8 | 22.65 |
| Rigidity (N/μm) | 1102.64 | 9111.40 | 10,966.39 | 2053.38 |
| Vibration Mode | Modal Frequency (Hz) | ||
|---|---|---|---|
| Workpiece Weight (tons) | |||
| 60 | 27 | 15 | |
| 21.5 | 29.8 | 35.4 |
| 32.8 | 48.8 | 59.2 |
| 40.7 | 37.2 | 41.6 |
| Dynamic Rigidity (N/μm) | |||
|---|---|---|---|
| Workpiece Weight (Ton) | Lateral Direction (X-Axis) | Vertical Direction (Y-Axis) | Longitudinal Direction (Z-Axis) |
| 60 | 107.16 | 595.69 | 358.42 |
| 27 | 119.80 | 721.33 | 299.37 |
| 15 | 148.98 | 669.27 | 287.54 |
| Dynamic Rigidity (N/μm) | |||
|---|---|---|---|
| Workpiece Weight (Ton) | Lateral Direction (X-Axis) | Vertical Direction (Y-Axis) | Longitudinal Direction (Z-Axis) |
| 60 | 46.53 | 236.98 | 376.72 |
| 27 | 55.23 | 131.31 | 302.86 |
| 15 | 80.27 | 131.31 | 289.25 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, C.-Y.; Luh, Y.-P.; Lin, W.-Z.; Lin, B.-C.; Hung, J.-P. Modeling the Static and Dynamic Behaviors of a Large Heavy-Duty Lathe Machine under Rated Loads. Computation 2022, 10, 207. https://doi.org/10.3390/computation10120207
Lin C-Y, Luh Y-P, Lin W-Z, Lin B-C, Hung J-P. Modeling the Static and Dynamic Behaviors of a Large Heavy-Duty Lathe Machine under Rated Loads. Computation. 2022; 10(12):207. https://doi.org/10.3390/computation10120207
Chicago/Turabian StyleLin, Chien-Yu, Yuan-Ping Luh, Wei-Zhu Lin, Bo-Chen Lin, and Jui-Pin Hung. 2022. "Modeling the Static and Dynamic Behaviors of a Large Heavy-Duty Lathe Machine under Rated Loads" Computation 10, no. 12: 207. https://doi.org/10.3390/computation10120207
APA StyleLin, C.-Y., Luh, Y.-P., Lin, W.-Z., Lin, B.-C., & Hung, J.-P. (2022). Modeling the Static and Dynamic Behaviors of a Large Heavy-Duty Lathe Machine under Rated Loads. Computation, 10(12), 207. https://doi.org/10.3390/computation10120207





