An Underground Radio Wave Propagation Prediction Model for Digital Agriculture
Abstract
:1. Introduction
2. Background and Related Work
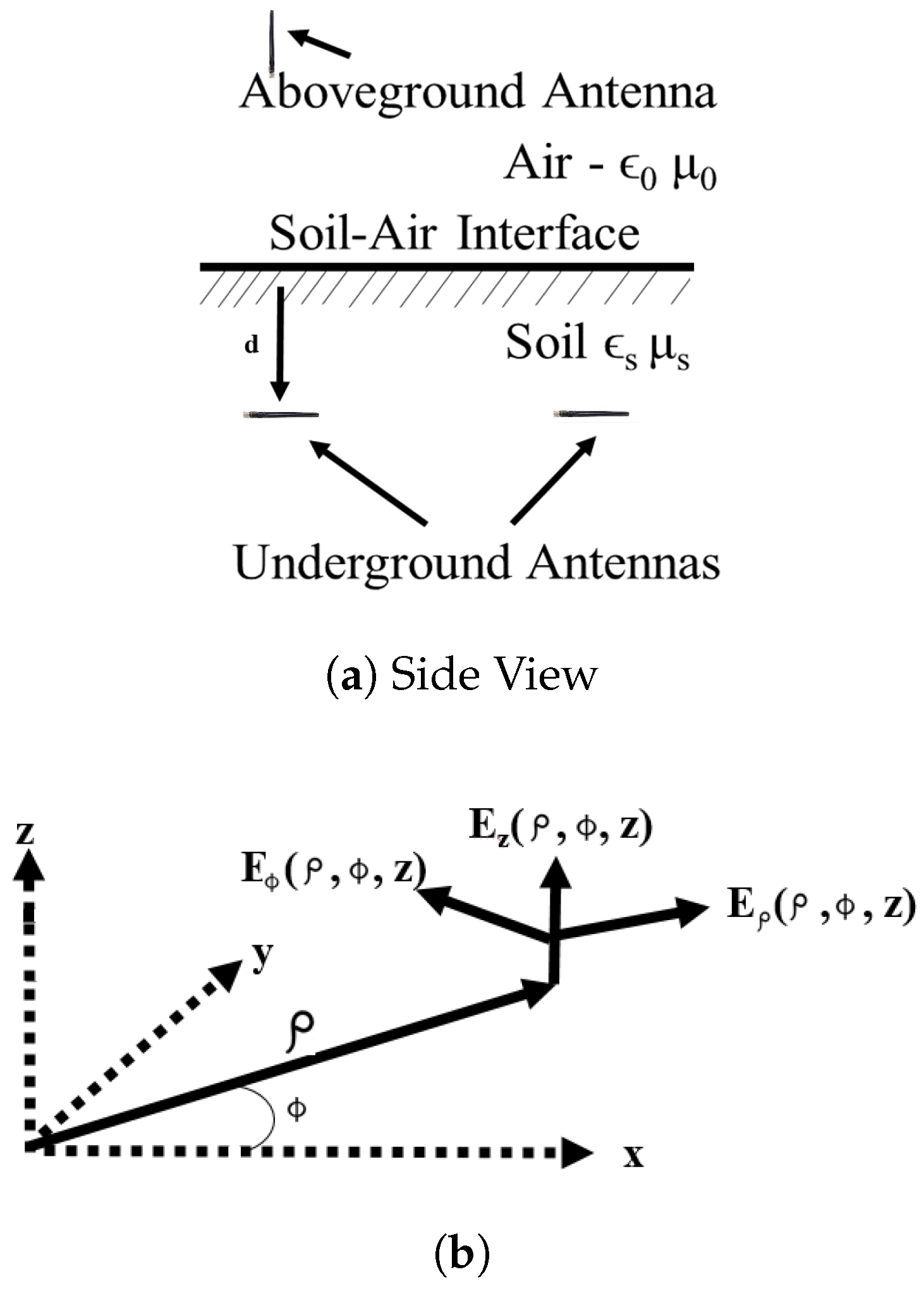
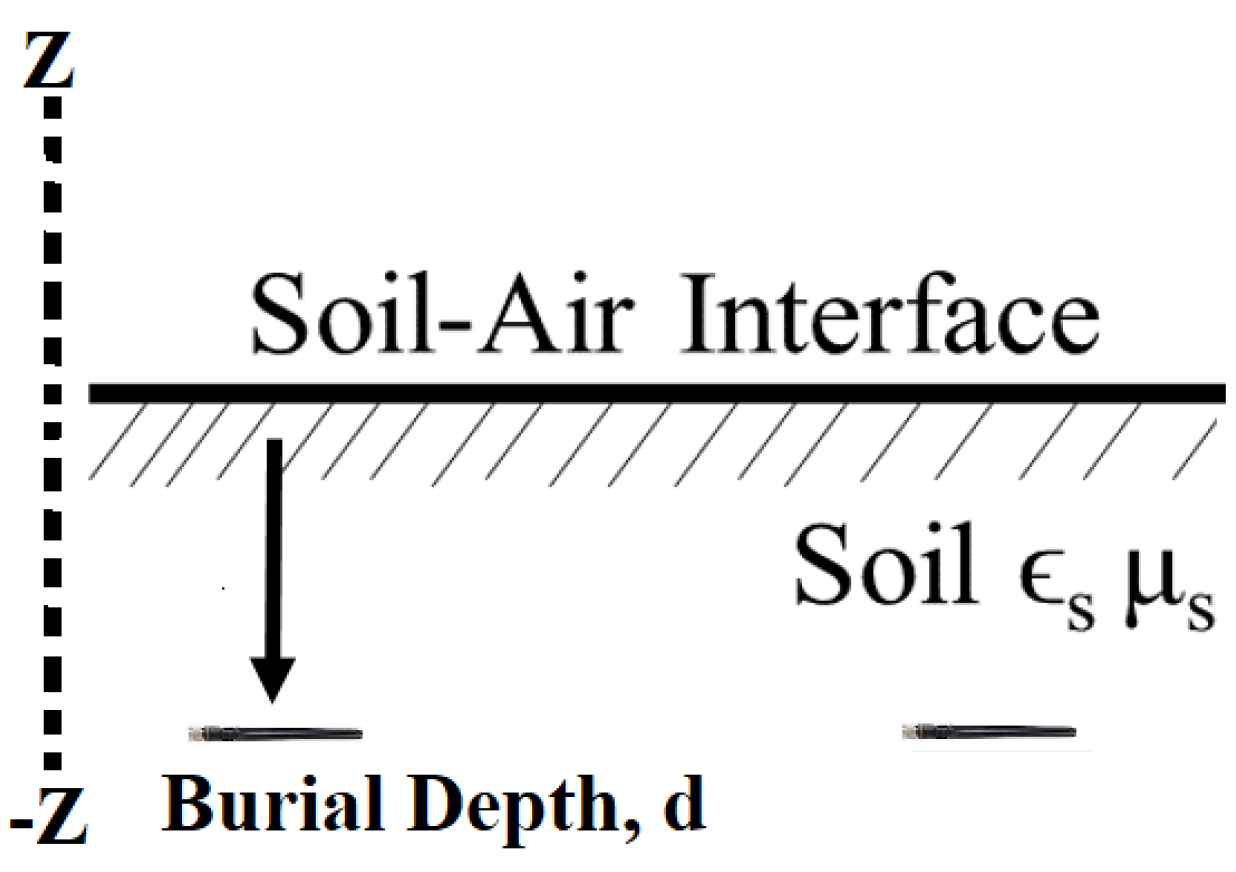
3. The Electromagnetic Field of a Unit Vertical Electric Dipole in the Presence of a Plane Boundary
4. The Electromagnetic Field of a Horizontal Electric Dipole in the Presence of a Plane Boundary
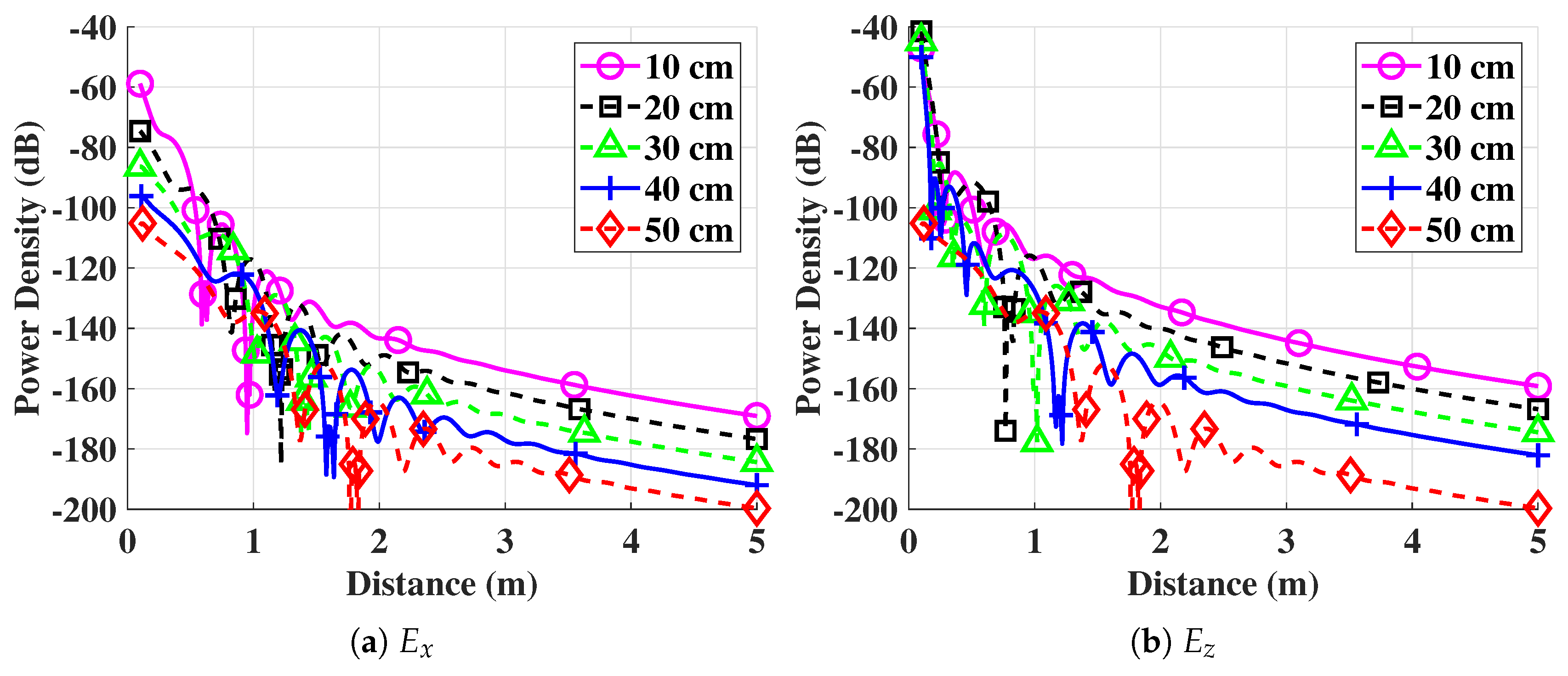
5. Model Evaluations and Results
6. Applications
7. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| Notation | Description |
| wavenumber in the soil | |
| wavenumber in the air | |
| wavelength in the soil | |
| phase constant | |
| attenuation constant | |
| permittivity of soil | |
| permeability of free space | |
| l | half length of antenna |
| angular frequency | |
| antenna impedance | |
| transmission line impedance | |
| reflected electric field | |
| reflected current | |
| current amplitude | |
| reflected impedance | |
| characteristics impedance | |
| radiation resistance | |
| current distribution along the antenna | |
| soil-air interface adjusted impedance | |
| return loss | |
| resonant frequency | |
| over-the-air frequency | |
| bandwidth | |
| h | burial depth |
| refractive index of air | |
| refractive index of soil | |
| volumetric water content | |
| bulk density | |
| particle density | |
| , , and | empirical constant |
| , | real and imaginary part of the permittivity of soil |
| S and C | sand and clay particle percentage |
| and | relative permittivity of free water, real and imaginary |
| effective conductivity of soil | |
| , | limit of permittivity of water |
| static permittivity of water | |
| relaxation time of water |
References
- Vuran, M.C.; Salam, A.; Wong, R.; Irmak, S. Internet of Underground Things in Precision Agriculture: Architecture and Technology Aspects. Ad Hoc Netw. 2018, 81, 160–173. [Google Scholar] [CrossRef]
- Vuran, M.C.; Salam, A.; Wong, R.; Irmak, S. Internet of Underground Things: Sensing and Communications on the Field for Precision Agriculture. In Proceeding of the 2018 IEEE 4th World Forum on Internet of Things (WF-IoT) (WF-IoT 2018), Singapore, 5–8 February 2018. [Google Scholar]
- Salam, A.; Vuran, M.C.; Irmak, S. Di-Sense: In situ real-time permittivity estimation and soil moisture sensing using wireless underground communications. Comput. Netw. 2019, 151, 31–41. [Google Scholar] [CrossRef]
- Akkaş, M.A. Channel Modeling of Wireless Sensor Networks in Oil. Wirel. Pers. Commun. 2017, 95, 4337–4355. [Google Scholar] [CrossRef]
- Akyildiz, I.F.; Stuntebeck, E.P. Wireless Underground Sensor Networks: Research Challenges. Ad Hoc Netw. J. 2006, 4, 669–686. [Google Scholar] [CrossRef]
- Akyildiz, I.F.; Sun, Z.; Vuran, M.C. Signal Propagation Techniques for Wireless Underground Communication Networks. Phys. Commun. J. 2009, 2, 167–183. [Google Scholar] [CrossRef]
- Jabbar, S.; Asif Habib, M.; Minhas, A.A.; Ahmad, M.; Ashraf, R.; Khalid, S.; Han, K. Analysis of Factors Affecting Energy Aware Routing in Wireless Sensor Network. Wirel. Commun. Mob. Comput. 2018, 2018, 9087269. [Google Scholar] [CrossRef]
- Konda, A.; Rau, A.; Stoller, M.A.; Taylor, J.M.; Salam, A.; Pribil, G.A.; Argyropoulos, C.; Morin, S.A. Soft Microreactors for the Deposition of Conductive Metallic Traces on Planar, Embossed, and Curved Surfaces. Adv. Funct. Mater. 2018, 28, 1803020. [Google Scholar] [CrossRef]
- Salam, A.; Vuran, M.C.; Irmak, S. Towards Internet of Underground Things in Smart Lighting: A Statistical Model of Wireless Underground Channel. In Proceedings of the 14th IEEE International Conference on Networking, Sensing and Control (IEEE ICNSC), Calabria, Italy, 16–18 May 2017. [Google Scholar]
- Salam, A.; Vuran, M.C.; Irmak, S. Pulses in the Sand: Impulse Response Analysis of Wireless Underground Channel. In Proceedings of the 35th Annual IEEE International Conference on Computer Communications (IEEE INFOCOM 2016), San Francisco, CA, USA, 10–14 April 2016. [Google Scholar]
- Salam, A.; Vuran, M.C. Wireless Underground Channel Diversity Reception with Multiple Antennas for Internet of Underground Things. In Proceedings of the 2017 IEEE International Conference on Communications (ICC), Paris, France, 21–25 May 2017. [Google Scholar]
- Salam, A.; Vuran, M.C. Smart Underground Antenna Arrays: A Soil Moisture Adaptive Beamforming Approach. In Proceedings of the IEEE Conference on Computer Communications (INFOCOM 2017), Atlanta, GA, USA, 1–4 May 2017. [Google Scholar]
- Salam, A. Pulses in the Sand: Long Range and High Data Rate Communication Techniques for Next Generation Wireless Underground Networks; ETD Collection for University of Nebraska: Lincoln, NE, USA, 2018. [Google Scholar]
- Saeed, N.; Al-Naffouri, T.Y.; Alouini, M.S. Towards the Internet of Underground Things: A Systematic Survey. arXiv 2019, arXiv:1902.03844. [Google Scholar]
- Vuran, M.C.; Akyildiz, I.F. Channel model and analysis for wireless underground sensor networks in soil medium. Phys. Commun. 2010, 3, 245–254. [Google Scholar] [CrossRef]
- Sun, Z.; Akyildiz, I. Magnetic Induction Communications for Wireless Underground Sensor Networks. IEEE Trans. Antennas Propag. 2010, 58, 2426–2435. [Google Scholar] [CrossRef]
- Wait, J.; Fuller, J. On Radio Propagation Through Earth: Antennas and Propagation. IEEE Trans. Antennas Propag. 1971, 19, 796–798. [Google Scholar] [CrossRef]
- King, R.W.P.; Owens, M.; Wu, T.T. Lateral Electromagnetic Waves; Springer: New York, NY, USA, 1992. [Google Scholar]
- King, R.; Wu, T. Lateral waves: Formulas for the magnetic field. J. Appl. Phys. 1983, 54, 507–514. [Google Scholar] [CrossRef]
- Salam, A.; Vuran, M.C. EM-Based Wireless Underground Sensor Networks. In Underground Sensing; Pamukcu, S., Cheng, L., Eds.; Academic Press: Cambridge, MA, USA, 2018; pp. 247–285. [Google Scholar] [CrossRef]
- King, R.W.P. New formulas for the electromagnetic field of a vertical electric dipole in a dielectric or conducting half-space near its horizontal interface. J. Appl. Phys. 1982, 53, 8476–8482. [Google Scholar] [CrossRef]
- Sarkar, T.K.; Lombardi, G.; Monebhurrun, V.; Krairiksh, M. Guest Editorial for the Special Issue on Radio Wave Propagation. IEEE Trans. Antennas Propag. 2018, 66, 6470–6475. [Google Scholar] [CrossRef]
- Ross, I.; McDonough, J.; Miles, J.; Storch, P.; Thelakkat Kochunarayanan, P.; Kalve, E.; Hurst, J.; Dasgupta, S.; Burdick, J. A review of emerging technologies for remediation of PFASs. Remediat. J. 2018, 28, 101–126. [Google Scholar] [CrossRef]
- Benskin, J.P.; Li, B.; Ikonomou, M.G.; Grace, J.R.; Li, L.Y. Per-and polyfluoroalkyl substances in landfill leachate: patterns, time trends, and sources. Environ. Sci. Technol. 2012, 46, 11532–11540. [Google Scholar] [CrossRef]
- Konda, A.; Morin, S.A. Flow-directed synthesis of spatially variant arrays of branched zinc oxide mesostructures. Nanoscale 2017, 9, 8393–8400. [Google Scholar] [CrossRef]
- Buck, R.C.; Franklin, J.; Berger, U.; Conder, J.M.; Cousins, I.T.; De Voogt, P.; Jensen, A.A.; Kannan, K.; Mabury, S.A.; van Leeuwen, S.P. Perfluoroalkyl and polyfluoroalkyl substances in the environment: Terminology, classification, and origins. Integr. Environ. Assess. Manag. 2011, 7, 513–541. [Google Scholar] [CrossRef] [PubMed]
- Backe, W.J.; Day, T.C.; Field, J.A. Zwitterionic, cationic, and anionic fluorinated chemicals in aqueous film forming foam formulations and groundwater from US military bases by nonaqueous large-volume injection HPLC-MS/MS. Environ. Sci. Technol. 2013, 47, 5226–5234. [Google Scholar] [CrossRef]
- Stoller, M.A.; Konda, A.; Kottwitz, M.A.; Morin, S.A. Thermoplastic building blocks for the fabrication of microfluidic masters. RSC Adv. 2015, 5, 97934–97943. [Google Scholar] [CrossRef]
- Allred, B.M.; Lang, J.R.; Barlaz, M.A.; Field, J.A. Orthogonal zirconium diol/C18 liquid chromatography–tandem mass spectrometry analysis of poly and perfluoroalkyl substances in landfill leachate. J. Chromatogr. A 2014, 1359, 202–211. [Google Scholar] [CrossRef]
- Huset, C.A.; Barlaz, M.A.; Barofsky, D.F.; Field, J.A. Quantitative determination of fluorochemicals in municipal landfill leachates. Chemosphere 2011, 82, 1380–1386. [Google Scholar] [CrossRef]
- Perez-Toralla, K.; Konda, A.; Bowen, J.J.; Jennings, E.E.; Argyropoulos, C.; Morin, S.A. Rational Synthesis of Large-Area Periodic Chemical Gradients for the Manipulation of Liquid Droplets and Gas Bubbles. Adv. Funct. Mater. 2018, 28, 1705564. [Google Scholar] [CrossRef]
- Merino, N.; Qu, Y.; Deeb, R.A.; Hawley, E.L.; Hoffmann, M.R.; Mahendra, S. Degradation and removal methods for perfluoroalkyl and polyfluoroalkyl substances in water. Environ. Eng. Sci. 2016, 33, 615–649. [Google Scholar] [CrossRef]
- Rahman, M.F.; Peldszus, S.; Anderson, W.B. Behaviour and fate of perfluoroalkyl and polyfluoroalkyl substances (PFASs) in drinking water treatment: A review. Water Res. 2014, 50, 318–340. [Google Scholar] [CrossRef]
- Hu, X.C.; Andrews, D.Q.; Lindstrom, A.B.; Bruton, T.A.; Schaider, L.A.; Grandjean, P.; Lohmann, R.; Carignan, C.C.; Blum, A.; Balan, S.A.; et al. Detection of poly-and perfluoroalkyl substances (PFASs) in US drinking water linked to industrial sites, military fire training areas, and wastewater treatment plants. Environ. Sci. Technol. Lett. 2016, 3, 344–350. [Google Scholar] [CrossRef]
- Hamid, H.; Li, L.Y.; Grace, J.R. Review of the fate and transformation of per-and polyfluoroalkyl substances (PFASs) in landfills. Environ. Pollut. 2018, 235, 74–84. [Google Scholar] [CrossRef]
- Farooq, M.U.; Ahmad, A.; Hameed, A. Opposition-based initialization and a modified pattern for Inertia Weight (IW) in PSO. In Proceedings of the 2017 IEEE International Conference on INnovations in Intelligent SysTems and Applications (INISTA), Gdynia, Poland, 3–5 July 2017; pp. 96–101. [Google Scholar] [CrossRef]
- Salam, A.; Vuran, M.C. Impacts of Soil Type and Moisture on the Capacity of Multi-Carrier Modulation in Internet of Underground Things. In Proceedings of the 2016 25th International Conference on Computer Communication and Networks (ICCCN), Waikoloa, HI, USA, 1–4 August 2016. [Google Scholar]
- Sommerfeld, A. Über die Ausbreitung der Wellen in der drahtlosen Telegraphie. Ann. Phys. 1909, 333, 665–736. [Google Scholar] [CrossRef]
- Sommerfeld, A. Über die Ausbreitung der Wellen in der drahtlosen Telegraphie. Ann. Phys. 1926, 386, 1135–1153. [Google Scholar] [CrossRef]
- Ling, R.; Scholler, J.; Ufimtsev, P.Y. The propagation and excitation of surface waves in an absorbing layer. Progr. Electromagn. Res. 1998, 19, 49–91. [Google Scholar] [CrossRef]
- Ward, S.H.; Hohmann, G.W.; Nabighian, M. Electromagnetic theory for geophysical applications. In Electromagnetic Methods in Applied Geophysics; Geological Publishing House: Beijing, China, 1988; Volume 1, pp. 131–311. [Google Scholar]
- Bhattacharyya, A.K. Longitudinal Spectral Solutions for the Sommerfeld Half-Space Problem: Presenting New Perspectives for Electromagnetic Field Solutions in an Axially Layered Structure. IEEE Antennas Propag. Mag. 2018, 60, 72–82. [Google Scholar] [CrossRef]
- Bhattacharyya, A.K. Analysis of two-layered structure using longitudinal spectrum: Space wave, surface wave, and Zenneck wave. IEEE Antennas Propag. Mag. 2019, 60, 72–82. [Google Scholar] [CrossRef]
- Dong, X.; Vuran, M.C. A Channel Model for Wireless Underground Sensor Networks Using Lateral Waves. In Proceedings of the 2011 IEEE Global Telecommunications Conference (GLOBECOM 2011), Kathmandu, Nepal, 5–9 December 2011. [Google Scholar]
- Barlow, H.; Cullen, A. Surface waves. Proc. IEE Part III Radio Commun. Eng. 1953, 100, 329–341. [Google Scholar] [CrossRef]
- Peplinski, N.; Ulaby, F.; Dobson, M. Dielectric properties of soil in the 0.3–1.3 GHz range. IEEE Trans. Geosci. Remote Sens. 1995, 33, 803–807. [Google Scholar] [CrossRef]
- Salam, A.; Vuran, M.C.; Dong, X.; Argyropoulos, C.; Irmak, S. A Theoretical Model of Underground Dipole Antennas for Communications in Internet of Underground Things. IEEE Trans. Antennas Propag. 2019, 67. [Google Scholar] [CrossRef]
- Salam, A.; Shah, S. Internet of Things in Smart Agriculture: Enabling Technologies. In Proceedings of the 2019 IEEE 5th World Forum on Internet of Things (WF-IoT 2019), Limerick, Ireland, 15–18 April 2019. [Google Scholar]
- Salam, A. Underground Soil Sensing Using Subsurface Radio Wave Propagation. In Proceedings of the 5th Global Workshop on Proximal Soil Sensing, Columbia, MO, USA, 28–31 May 2019. [Google Scholar]
- Salam, A. A Path Loss Model for Through the Soil Wireless Communications in Digital Agriculture. In Proceedings of the 2019 IEEE International Symposium on Antennas and Propagation, Atlanta, GA, USA, 7–12 July 2019. [Google Scholar]
- Salam, A. Underground Environment Aware MIMO Design Using Transmit and Receive Beamforming in Internet of Underground Things. In Proceedings of the 2019 International Conference on Internet of Things (ICIOT 2019), San Diego, CA, USA, 25–30 June 2019. [Google Scholar]
- Salam, A.; Shah, S. Urban Underground Infrastructure Monitoring IoT: The Path Loss Analysis. In Proceedings of the 2019 IEEE 5th World Forum on Internet of Things (WF-IoT 2019), Limerick, Ireland, 15–18 April 2019. [Google Scholar]
- Salam, A. A Comparison of Path Loss Variations in Soil using Planar and Dipole Antennas. In Proceedings of the 2019 IEEE International Symposium on Antennas and Propagation, Atlanta, GA, USA, 7–12 July 2019. [Google Scholar]


© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salam, A. An Underground Radio Wave Propagation Prediction Model for Digital Agriculture. Information 2019, 10, 147. https://doi.org/10.3390/info10040147
Salam A. An Underground Radio Wave Propagation Prediction Model for Digital Agriculture. Information. 2019; 10(4):147. https://doi.org/10.3390/info10040147
Chicago/Turabian StyleSalam, Abdul. 2019. "An Underground Radio Wave Propagation Prediction Model for Digital Agriculture" Information 10, no. 4: 147. https://doi.org/10.3390/info10040147
APA StyleSalam, A. (2019). An Underground Radio Wave Propagation Prediction Model for Digital Agriculture. Information, 10(4), 147. https://doi.org/10.3390/info10040147




